A ROTI-Aided Equatorial Plasma Bubbles Detection Method
Abstract
:1. Introduction
2. Materials and Methods
2.1. Data
2.2. Methods
3. Results
4. Discussion
5. Conclusions and Perspectives
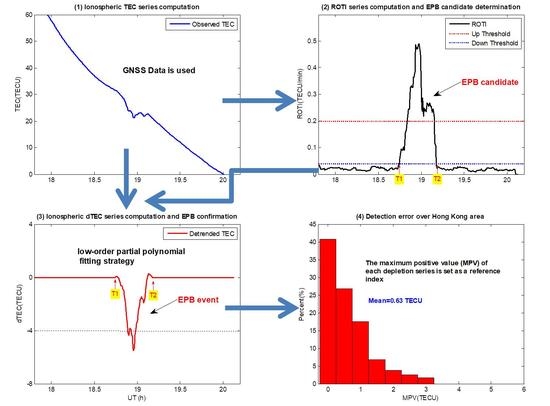
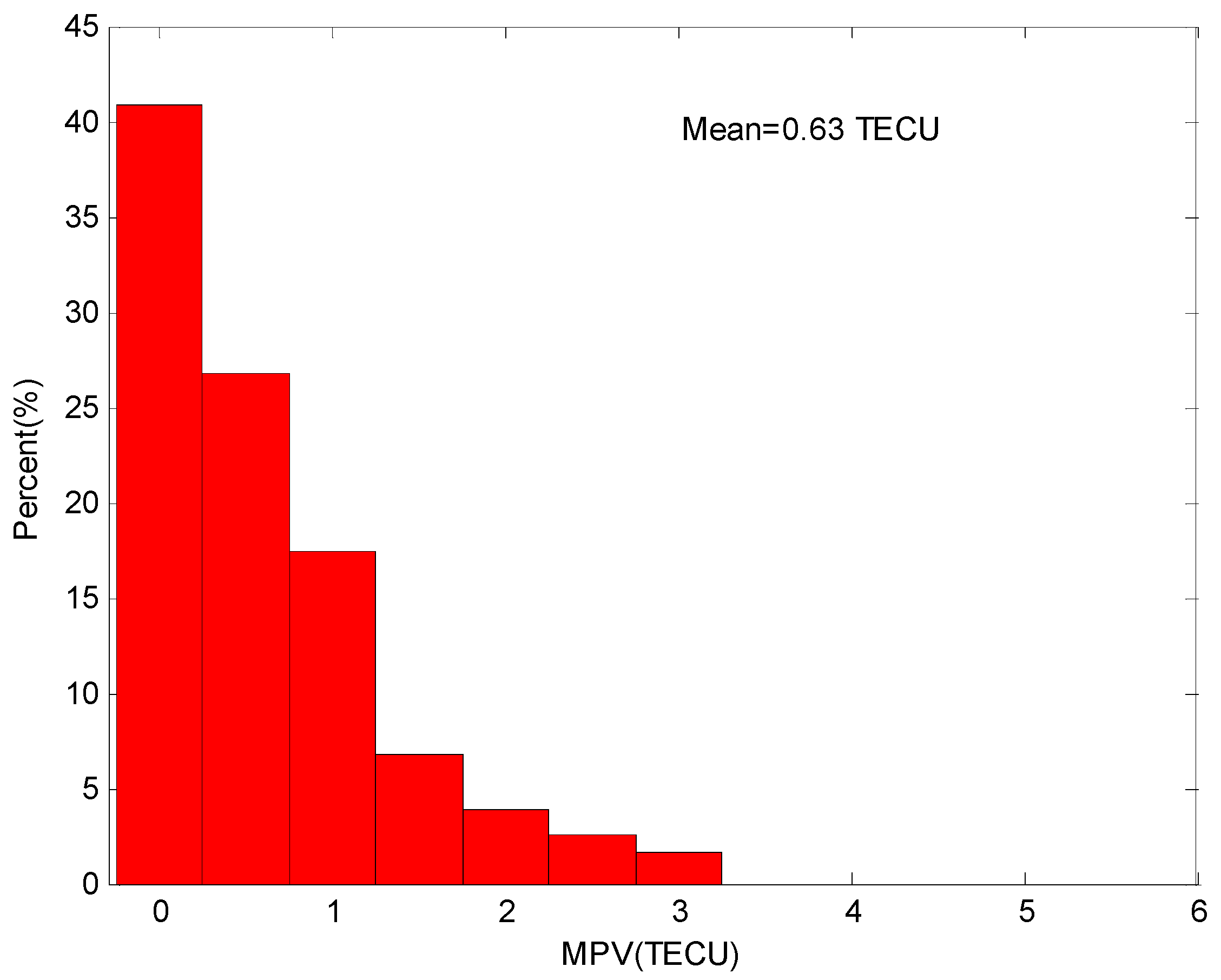
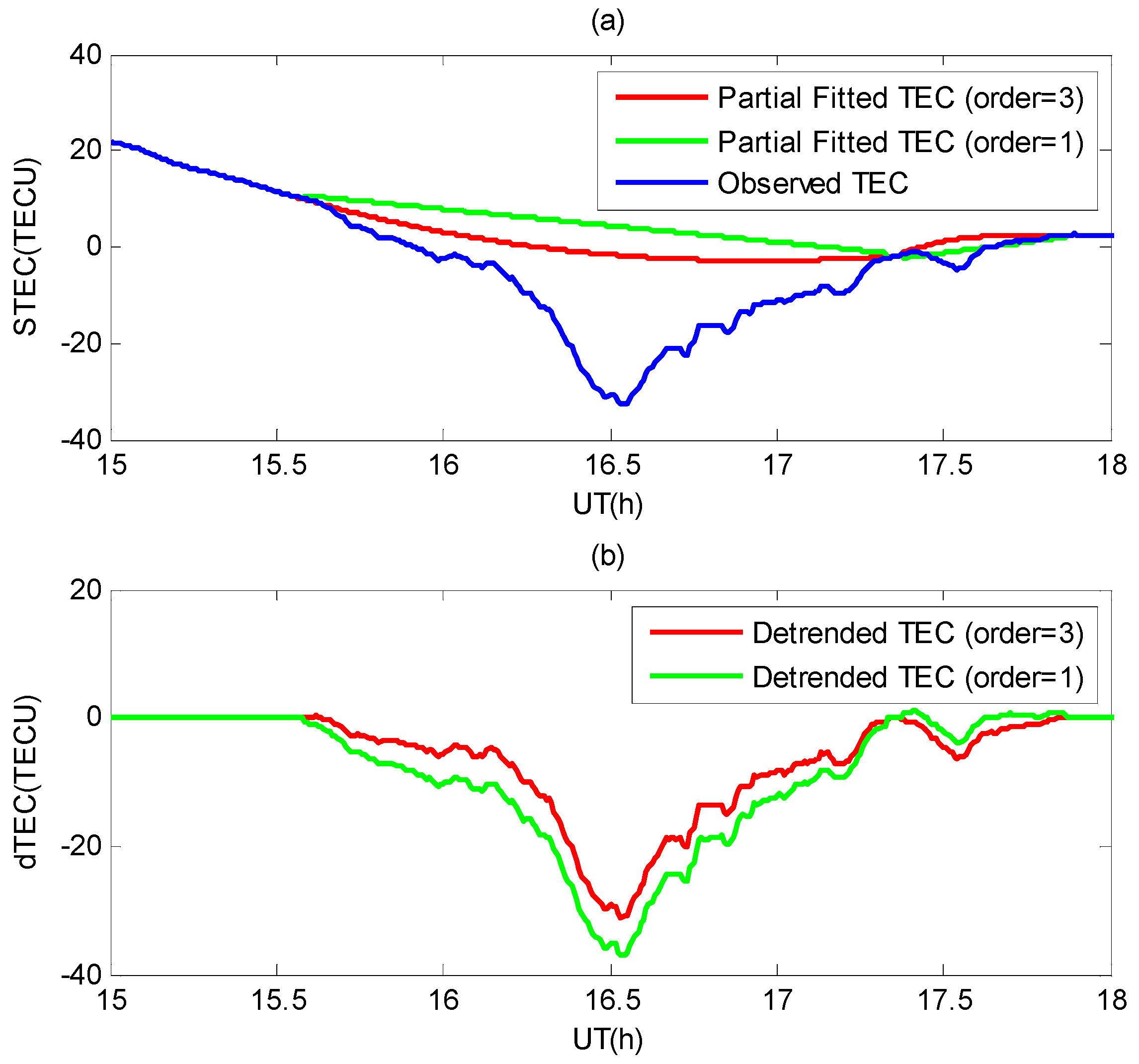
- EPBs generally occur after 20:00 local time and are observed throughout the nighttime and the occurrence during the spring and autumn equinoxes is significantly greater than during the summer and winter solstices, which is also reported in previous studies. In addition, most of the TEC depletion error is smaller than 1.5 TECU and the mean error is 0.63 TECU. These findings suggest that the detection method is feasible and highly accurate.
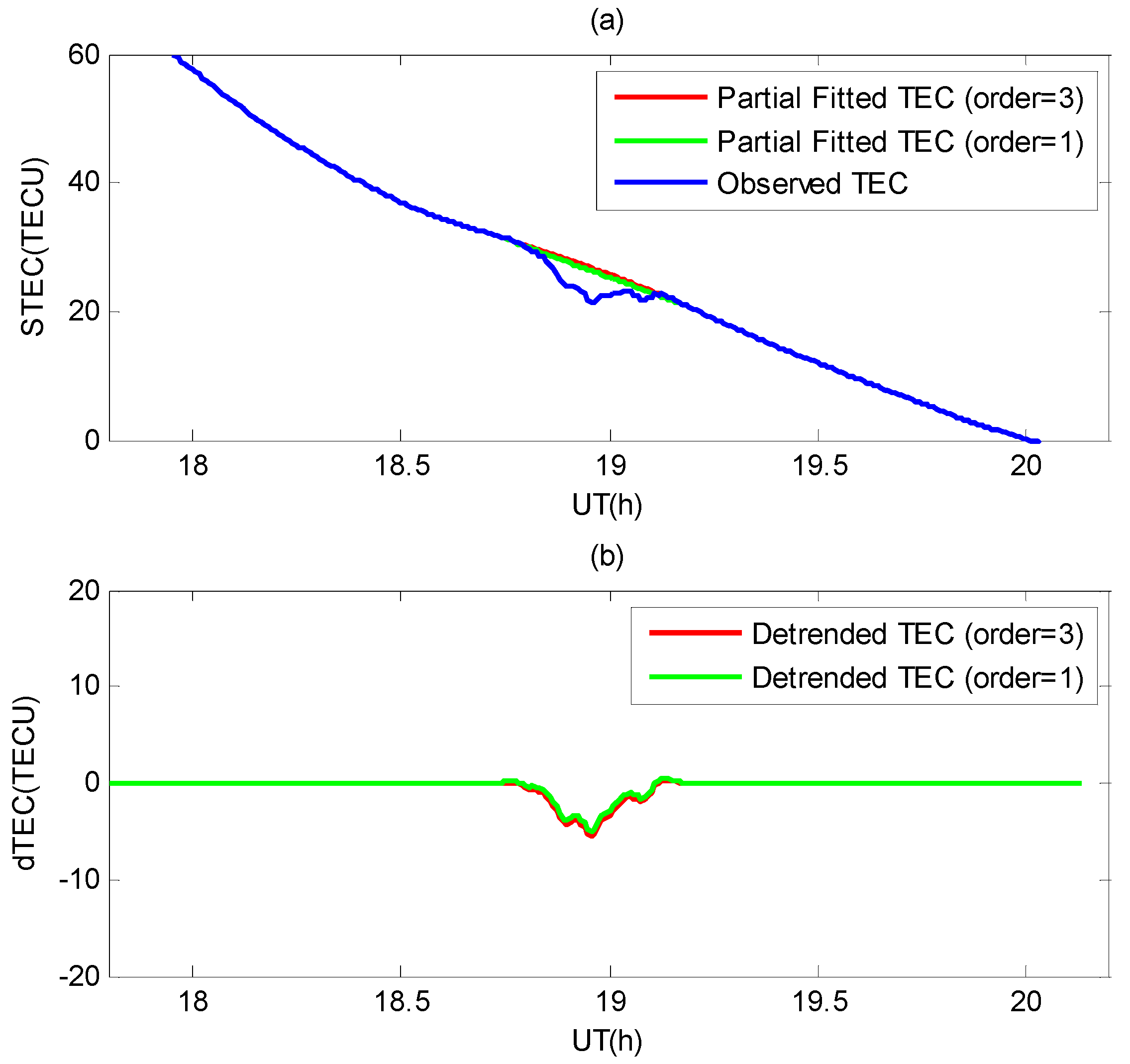
- For short-duration EPB, the fitted TEC series are similar between the third-order polynomial and linear fitting; for long-duration EPB, the fitted TEC series via the third-order polynomial are smoother and more fitting than those via a linear fitting. This suggests that the third-order polynomial fitting is more suitable to fit the background TEC data, and thus adequately extract the TEC depletion by the EPB.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sultan, P.J. Linear theory and modeling of the Rayleigh-Taylor instability leading to the occurrence of equatorial spread F. J. Geophys. Res. Space Phys. 1996, 101, 26875–26891. [Google Scholar] [CrossRef]
- Kelley, M.C. The Earth’s Ionosphere: Plasma Physics and Electrodynamics; Academic Press: Cambridge, MA, USA, 2009. [Google Scholar]
- Chen, W.; Gao, S.; Hu, C.; Chen, Y.; Ding, X. Effects of ionospheric disturbances on GPS observation in low latitude area. GPS Solut. 2008, 12, 33–41. [Google Scholar] [CrossRef]
- Huang, C.Y.; Burke, W.J.; Machuzak, J.S.; Gentile, L.C.; Sultan, P.J. DMSP observations of equatorial plasma bubbles in the topside ionosphere near solar maximum. J. Geophys. Res. Space Phys. 2001, 106, 8131–8142. [Google Scholar] [CrossRef]
- Sobral, J.; Abdu, M.A.; Takahashi, H.; Taylor, M.J.; De Paula, E.R.; Zamlutti, C.J.; De Aquino, M.G.; Borba, G.L. Ionospheric plasma bubble climatology over Brazil based on 22 years (1977–1998) of 630 nm airglow observations. J. Atmos. Sol.-Terr. Phys. 2002, 64, 1517–1524. [Google Scholar] [CrossRef]
- Abdu, M.A.; Macdougall, J.W.; Batista, I.S.; Sobral, J.H.A.; Jayachandran, P.T. Equatorial evening prereversal electric field enhancement and sporadic E layer disruption: A manifestation of E and F region coupling. J. Geophys. Res. Space Phys. 2003, 108. [Google Scholar] [CrossRef] [Green Version]
- Burke, W.J. Longitudinal variability of equatorial plasma bubbles observed by DMSP and ROCSAT-1. J. Geophys. Res. 2004, 109, A12301. [Google Scholar] [CrossRef]
- Kil, H.; Demajistre, R.; Paxton, L.J. F-region plasma distribution seen from TIMED/GUVI and its relation to the equatorial spread F activity. Geophys. Res. Lett. 2004, 31, 179–211. [Google Scholar] [CrossRef]
- Makela, J.J.; Ledvina, B.M.; Kelley, M.C.; Kintner, P.M. Analysis of the seasonal variations of equatorial plasma bubble occurrence observed from Haleakala, Hawaii. Ann. Geophys. 2004, 22, 3109–3121. [Google Scholar] [CrossRef] [Green Version]
- Nishioka, M.; Saito, A.; Tsugawa, T. Occurrence characteristics of plasma bubble derived from global ground-based GPS receiver networks. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Portillo, A.; Herraiz, M.; Radicella, S.M.; Ciraolo, L. Equatorial plasma bubbles studied using African slant total electron content observations. J. Atmos. Sol.-Terr. Phys. 2008, 70, 907–917. [Google Scholar] [CrossRef]
- Ji, S.; Chen, W.; Wang, Z.; Xu, Y.; Weng, D.; Wan, J.; Fan, Y.; Huang, B.; Fan, S.; Sun, G. A study of occurrence characteristics of plasma bubbles over Hong Kong area. Adv. Space Res. 2013, 52, 1949–1958. [Google Scholar] [CrossRef]
- Kumar, S.; Chen, W.; Liu, Z.; Ji, S. Effects of solar and geomagnetic activity on the occurrence of equatorial plasma bubbles over Hong Kong. J. Geophys. Res. Space Phys. 2016, 121, 9164–9178. [Google Scholar] [CrossRef]
- Buhari, S.M.; Abdullah, M.; Yokoyama, T.; Otsuka, Y.; Nishioka, M.; Hasbi, A.M.; Bahari, S.A.; Tsugawa, T. Climatology of successive equatorial plasma bubbles observed by GPS ROTI over Malaysia. J. Geophys. Res. Space Phys. 2017, 122, 2174–2184. [Google Scholar] [CrossRef]
- Tang, L.; Chen, W.; Louis, O.P.; Chen, M. Study on Seasonal Variations of Plasma Bubble Occurrence over Hong Kong Area Using GNSS Observations. Remote Sens. 2020, 12, 2423. [Google Scholar] [CrossRef]
- Vargas, F.; Brum, C.; Terra, P.; Gobbi, D. Mean Zonal Drift Velocities of Plasma Bubbles Estimated from Keograms of Nightglow All-Sky Images from the Brazilian Sector. Atmosphere 2020, 11, 69. [Google Scholar] [CrossRef] [Green Version]
- Magdaleno, S.; Herraiz, M.; Radicella, S.M. Ionospheric Bubble Seeker: A Java Application to Detect and Characterize Ionospheric Plasma Depletion from GPS Data. IEEE Trans. Geosci. Remote Sens. 2012, 50, 1719–1727. [Google Scholar] [CrossRef]
- Hong Kong Satellite Reference Network. Available online: ftp://ftp.geodetic.gov.hk (accessed on 22 September 2021).
- Pi, X.; Mannucci, A.J.; Lindqwister, U.J.; Ho, C.M. Monitoring of global ionospheric irregularities using the worldwide GPS network. Geophys. Res. Lett. 1997, 24, 2283–2286. [Google Scholar] [CrossRef]
- Pi, X.; Iijima, B.A.; Lu, W. Effects of Ionospheric Scintillation on GNSS-Based Positioning. Navig. J. Inst. Navig. 2017, 64, 3–22. [Google Scholar] [CrossRef]
- Cherniak, I.; Krankowski, A.; Zakharenkova, I. ROTI Maps: A new IGS ionospheric product characterizing the ionospheric irregularities occurrence. GPS Solut. 2018, 22, 69. [Google Scholar] [CrossRef]
- Sanz, J.; Juan, J.; Hernández-Pajares, M. GNSS Data Processing, Vol. I: Fundamentals and Algorithms; ESTEC TM-23/1; ESA Communications: Noordwijk, The Netherlands, 2013. [Google Scholar]
- Tang, L.; Li, Z.; Zhou, B. Large-area tsunami signatures in ionosphere observed by GPS TEC after the 2011 Tohoku earthquake. GPS Solut. 2018, 22, 93. [Google Scholar] [CrossRef]
- Jacobsen, K.S. The impact of different sampling rates and calculation time intervals on ROTI values. J. Space Weather Space Clim. 2014, 4, A33. [Google Scholar] [CrossRef] [Green Version]
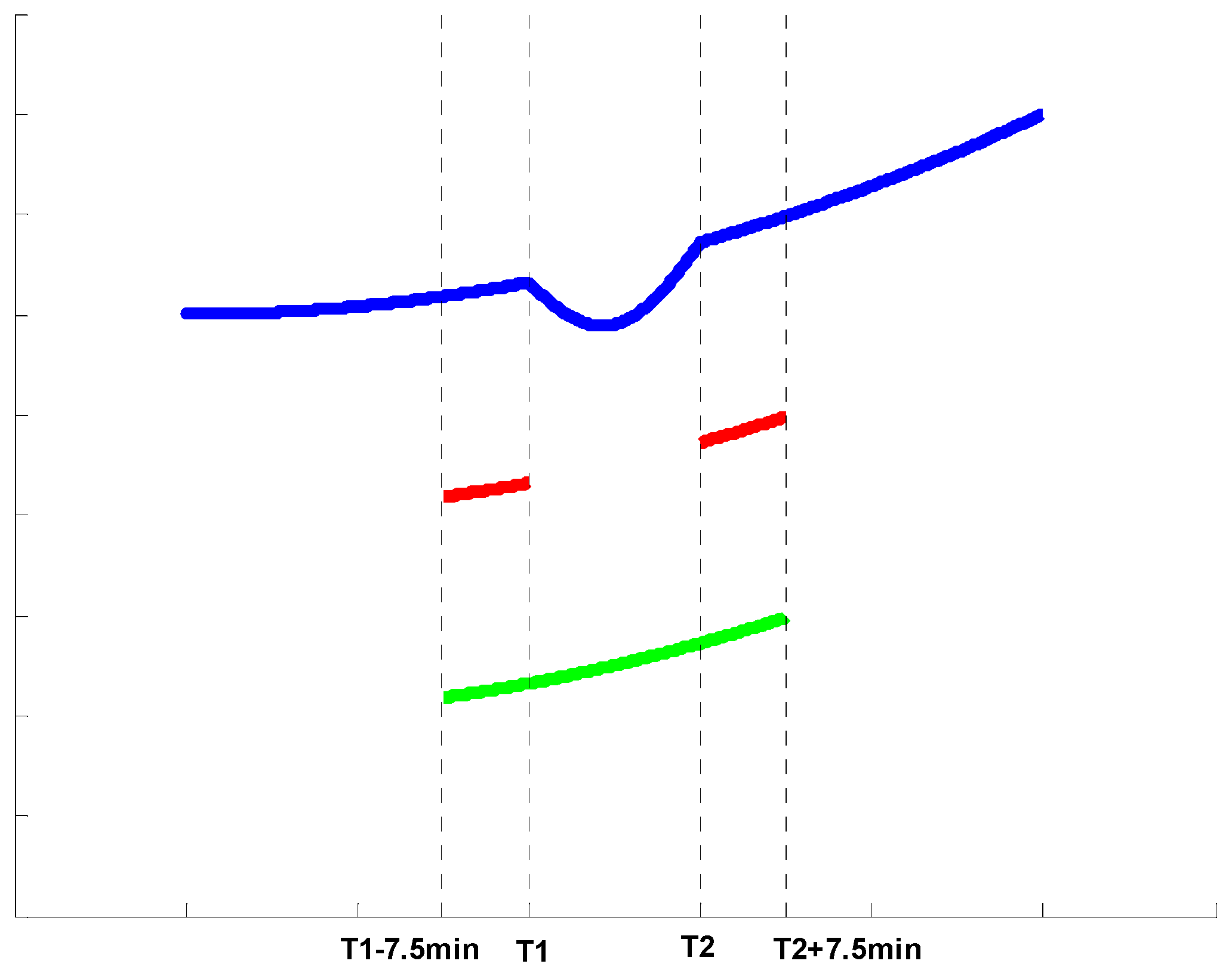




| Parameter | Value |
|---|---|
| Up ROTI | 0.2 TECU/min |
| Down ROTI | 0.04 TECU/min |
| Minimum depth | 4 TECU |
| Minimum duration | 10 min |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, L.; Louis, O.-P.; Chen, W.; Chen, M. A ROTI-Aided Equatorial Plasma Bubbles Detection Method. Remote Sens. 2021, 13, 4356. https://doi.org/10.3390/rs13214356
Tang L, Louis O-P, Chen W, Chen M. A ROTI-Aided Equatorial Plasma Bubbles Detection Method. Remote Sensing. 2021; 13(21):4356. https://doi.org/10.3390/rs13214356
Chicago/Turabian StyleTang, Long, Osei-Poku Louis, Wu Chen, and Mingli Chen. 2021. "A ROTI-Aided Equatorial Plasma Bubbles Detection Method" Remote Sensing 13, no. 21: 4356. https://doi.org/10.3390/rs13214356
APA StyleTang, L., Louis, O.-P., Chen, W., & Chen, M. (2021). A ROTI-Aided Equatorial Plasma Bubbles Detection Method. Remote Sensing, 13(21), 4356. https://doi.org/10.3390/rs13214356