- Review
Quantum-Inspired Neural Radiative Transfer (QINRT): A Multi-Scale Computational Framework for Next-Generation Climate Intelligence
- Muhammad Shoaib Akhtar
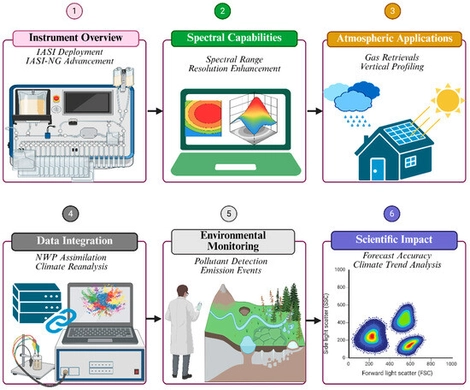
The increasing need for high-resolution, real-time radiative transfer (RT) modeling in climate science, remote sensing, and planetary exploration has exposed limitations of traditional solvers such as the Discrete Ordinate Radiative Transfer (DISORT) and Rapid Radiative Transfer Model for General Circulation Models (RRTMG), particularly in handling spectral complexity, non-local thermodynamic equilibrium (non-LTE) conditions, and computational scalability. Quantum-Inspired Neural Radiative Transfer (QINRT) frameworks, combining tensor-network parameterizations and quantum neural operators (QNOs), offer efficient approximation of high-dimensional radiative fields while preserving key physical correlations. This review highlights the advances of QINRT in enhancing spectral fidelity and computational efficiency, enabling energy-efficient, real-time RT inference suitable for satellite constellations and unmanned aerial vehicle (UAV) platforms. By integrating physics-informed modeling with scalable neural architectures, QINRT represents a transformative approach for next-generation Earth-system digital twins and autonomous climate intelligence.
23 October 2025