Ice Phenology and Thickness Modelling for Lake Ice Climatology
Abstract
1. Introduction
2. Materials and Methods
2.1. Model Equations for Water Temperature
2.2. Ice–Water Model
3. Equilibrium
3.1. Temperature Equilibrium
3.2. Ice Equilibrium
4. Time Evolution
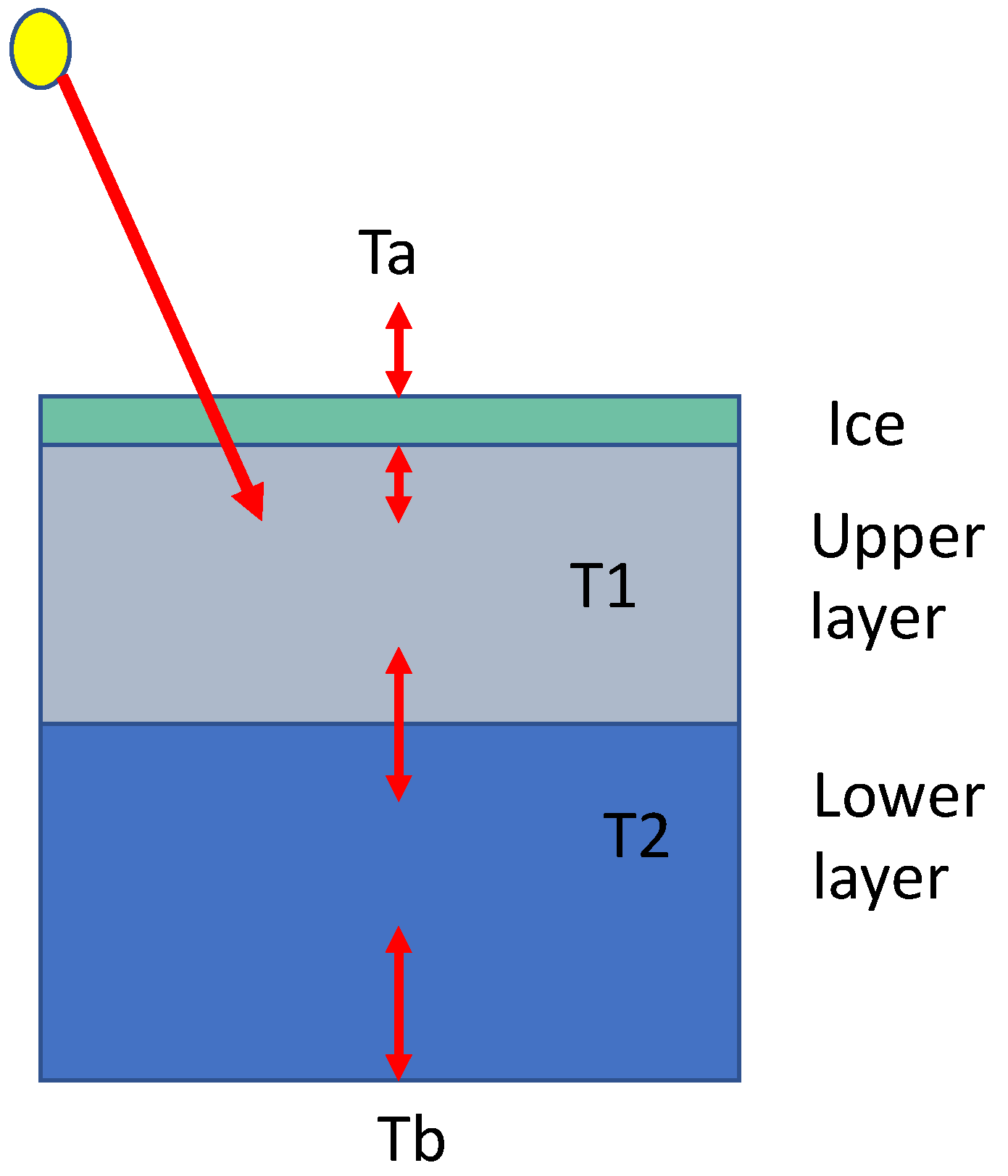
4.1. The Two-Layer System
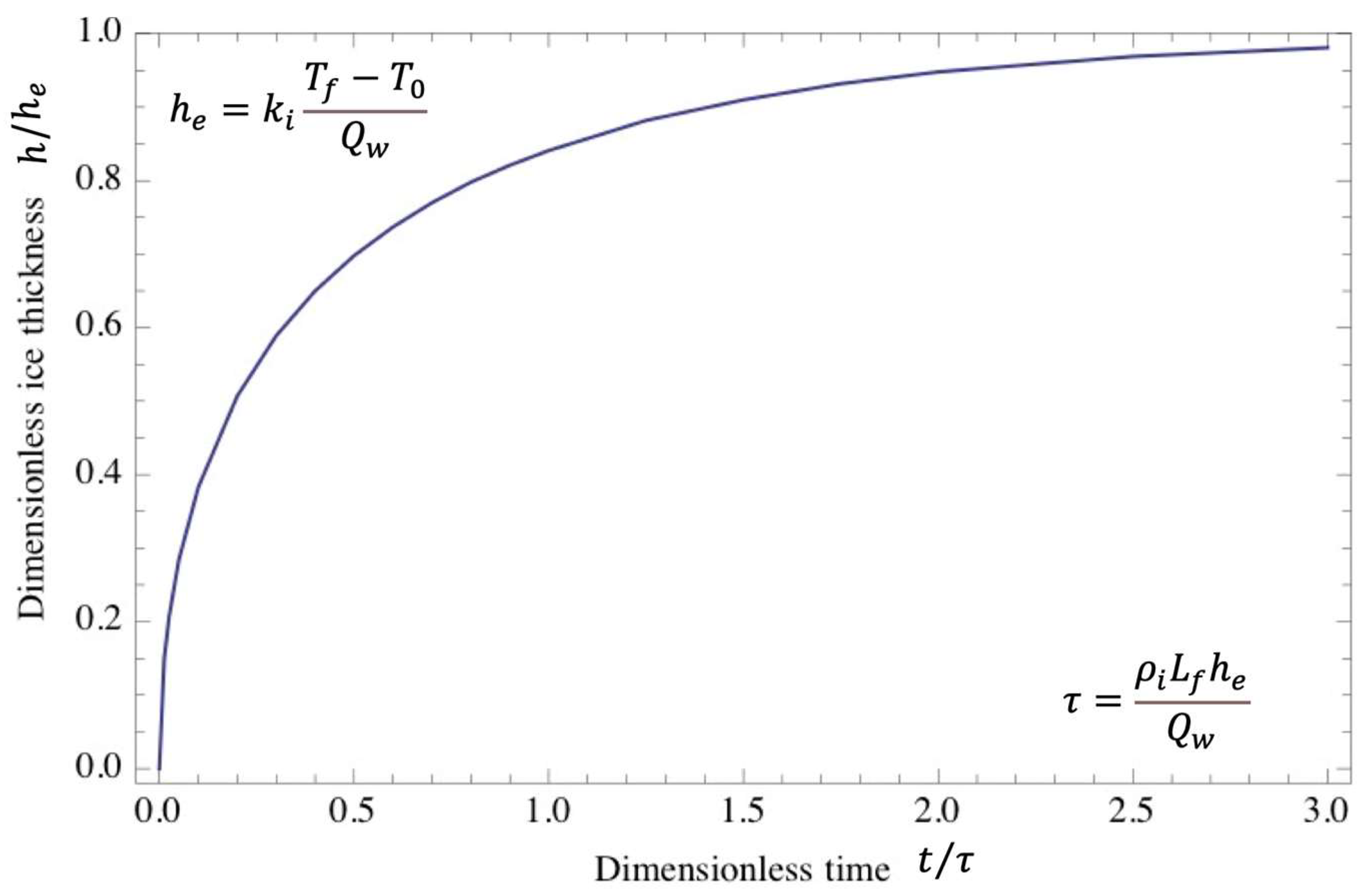
4.2. Ice-Cover Thickness
5. Discussion
6. Concluding Remarks
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Salonen, K.; Leppäranta, M.; Viljanen, M.; Gulati, R. Perspectives in winter limnology: Closing the annual cycle of freezing lakes. Aquat. Ecol. 2009, 43, 609–616. [Google Scholar] [CrossRef]
- George, G. (Ed.) Climate Change Impact on European Lakes; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Knoll, L.B.; Sharma, S.; Denfeld, B.A.; Flaim, G.; Hori, Y.; Magnuson, J.J.; Straile, D.; Weyhenmeyer, G. Consequences of lake and river ice loss on cultural ecosystem services. Limnol. Oceanogr. Lett. 2019, 4, 119–131. [Google Scholar] [CrossRef]
- Leppäranta, M. Freezing of Lakes and the Evolution of Their Ice Cover, 2nd ed.; Springer-Praxis: Heidelberg, Germany, 2023. [Google Scholar]
- Ashton, G. Freshwater ice growth, motion, and decay. In Dynamics of Snow and Ice Masses; Colbeck, S., Ed.; Academic Press: New York, NY, USA, 1980; pp. 261–304. [Google Scholar]
- Sharma, S.; Blagrave, K.; Magnuson, J.J.; O’Reilly, C.M.; Oliver, S.; Batt, R.D.; Magee, R.M.; Straile, D.; Weyhenmeyer, G.A.; Winslow, L.; et al. Widespread loss of lake ice around the Northern Hemisphere in a warming world. Nat. Clim. Chang. 2019, 9, 227–231. [Google Scholar] [CrossRef]
- Noori, R.; Woolway, R.I.; Saari, M.; Pulkkanen, M.; Kløve, B. Six decades of thermal change in a pristine lake situated north of the Arctic Circle. Water Resour. Res. 2022, 58, e2021WR031543. [Google Scholar] [CrossRef]
- Noori, R.; Bateni, S.M.; Saari, M.; Almazroui, M.; Torabi Haghighi, A. Strong warming rates in the surface and bottom layers of a boreal lake: Results from approximately six decades of measurements (1964–2020). Earth Space Sci. 2022, 9, e2021EA001973. [Google Scholar] [CrossRef]
- Magnuson, J.J.; Robertson, D.M.; Benson, B.J.; Wynne, R.H.; Livingstone, D.M.; Arai, T.; Assel, R.A.; Barry, R.G.; Card, V.; Kuusisto, E.; et al. Historical trends in lake and river ice cover in the northern hemisphere. Science 2000, 289, 1743–1746, Erratum in Science 2001, 291, 254. [Google Scholar] [CrossRef] [PubMed]
- Korhonen, J. Long-term changes in lake ice cover in Finland. Nord. Hydrol. 2006, 37, 347–363. [Google Scholar] [CrossRef]
- Livingstone, D.; Adrian, R. Modeling the duration of intermittent ice cover on a lake for climate-change studies. Limnol. Oceanogr. 2009, 54, 1709–1722. [Google Scholar] [CrossRef]
- Bernhardt, J.; Engelhardt, C.; Kirillin, G.; Matschullat, J. Lake ice phenology in Berlin-Brandenburg from 1947–2007: Observations and model hindcasts. Clim. Chang. 2011, 112, 791–817. [Google Scholar] [CrossRef]
- Efremova, T.; Palshin, N. Ice phenomena terms on the water bodies of northwestern Russia. Meteorol. Hydrol. 2011, 36, 559–565. [Google Scholar] [CrossRef]
- Karetnikov, S.; Leppäranta, M.; Montonen, A. Time series over 100 years of the ice season in Lake Ladoga. J. Great Lakes Res. 2017, 43, 979–988. [Google Scholar] [CrossRef]
- Mironov, D.; Ritter, B.; Schulz, J.-P.; Buchhold, M.; Lange, M.; MacHulskaya, E. Parameterisation of sea and lake ice in numerical weather prediction models of the German Weather Service. Tellus A Dyn. Meteorol. Oceanogr. 2012, 64, 17330. [Google Scholar] [CrossRef]
- Yang, Y.; Leppäranta, M.; Li, Z.; Cheng, B. An ice model for Lake Vanajavesi, Finland. Tellus A 2012, 64, 17202. [Google Scholar] [CrossRef]
- Leppäranta, M.; Wen, L. Ice phenology in Eurasian lakes over spatial location and altitude. Water 2022, 14, 1037. [Google Scholar] [CrossRef]
- Wang, J.; Bai, X.; Hu, H.; Clites, A.; Holton, M.; Lofgren, B. Temporal and spatial variability of Great Lakes ice cover, 1973–2010. J. Clim. 2012, 25, 1318–1329. [Google Scholar] [CrossRef]
- Murfitt, J.; Duguay, C.R. 50 years of lake ice research from active microwave remote sensing: Progress and prospects. Remote Sens. Environ. 2021, 264, 112616. [Google Scholar] [CrossRef]
- Wang, X.; Qiu, Y.; Zhang, Y.; Lemmetyinen, J.; Cheng, B.; Liang, E.; Leppäranta, M. A lake ice phenology dataset for the Northern Hemisphere based on passive microwave remote sensing. Big Earth Data 2021, 6, 401–419. [Google Scholar] [CrossRef]
- Stepanenko, V.M.; Repina, I.A.; Ganbat, G.; Davaa, G. Numerical simulation of ice cover in saline lakes. Izv. Atmos. Ocean. Phys. 2019, 55, 129–139. [Google Scholar] [CrossRef]
- Leppäranta, M. Interpretation of statistics of lake ice time series for climate variability. Hydrol. Res. 2014, 45, 673–684. [Google Scholar] [CrossRef]
- Kirillin, G.; Leppäranta, M.; Terzhevik, A.; Bernhardt, J.; Engelhardt, C.; Granin, N.; Golosov, S.; Efremova, T.; Palshin, N.; Sherstyankin, P.; et al. Physics of seasonally ice-covered lakes: Major drivers and temporal/spatial scales. Aquat. Ecol. 2012, 74, 659–682. [Google Scholar]
- Sahlberg, J. A hydrodynamical model for calculating the vertical temperature profile in lakes during cooling. Nord. Hydrol. 1983, 14, 239–254. [Google Scholar] [CrossRef]
- Thompson, R.; Price, D.; Cameron, N.; Jones, V.; Bigler, C.; Catalan, J.; Rosén, P.; Hall, R.I.; Weckström, J.; Korhola, A. Quantitative calibration of remote mountain lake sediments as climate recorders of ice-cover duration. Arct. Antarct. Alp. Res. 2005, 37, 626–635. [Google Scholar] [CrossRef]
- Cao, X.; Lu, P.; Leppäranta, M.; Arvola, L.; Huotari, J.; Shi, X.; Li, G.; Li, Z. Solar radiation transfer for an ice-covered lake in the central Asian arid climate zone. Inland Waters 2020, 11, 89–103. [Google Scholar] [CrossRef]
- Huang, W.F.; Li, Z.; Han, H.; Niu, F.; Lin, Z.; Leppäranta, M. Structural analysis of thermokarst lake ice in Beiluhe Basin, Qinghai–Tibet Plateau. Cold Reg. Sci. Technol. 2012, 72, 33–42. [Google Scholar] [CrossRef]
- Leppäranta, M.; Lindgren, E.; Arvola, L. Heat balance of supraglacial lakes in the western Dronning Maud Land. Ann. Glaciol. 2016, 57, 39–46. [Google Scholar] [CrossRef][Green Version]
- Leppäranta, M.; Lindgren, E.; Wen, L.; Kirillin, G. Ice cover decay and heat balance in Lake Kilpisjärvi in Arctic tundra. J. Limnol. 2019, 78, 163–175. [Google Scholar] [CrossRef]
- Rodhe, B. On the relation between air temperature and ice formation in the Baltic. Geogr. Ann. 1952, 1–2, 176–202. [Google Scholar]
- Hodgson, D.A. Antarctic lakes. In Encyclopedia of Lakes and Reservoirs; Bengtsson, L., Herschy, R.W., Fairbridge, R.W., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 26–31. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leppäranta, M. Ice Phenology and Thickness Modelling for Lake Ice Climatology. Water 2023, 15, 2951. https://doi.org/10.3390/w15162951
Leppäranta M. Ice Phenology and Thickness Modelling for Lake Ice Climatology. Water. 2023; 15(16):2951. https://doi.org/10.3390/w15162951
Chicago/Turabian StyleLeppäranta, Matti. 2023. "Ice Phenology and Thickness Modelling for Lake Ice Climatology" Water 15, no. 16: 2951. https://doi.org/10.3390/w15162951
APA StyleLeppäranta, M. (2023). Ice Phenology and Thickness Modelling for Lake Ice Climatology. Water, 15(16), 2951. https://doi.org/10.3390/w15162951






