The Effect of Sulfuric Acid Concentration on the Physical and Electrochemical Properties of Vanadyl Solutions
Abstract
:1. Introduction
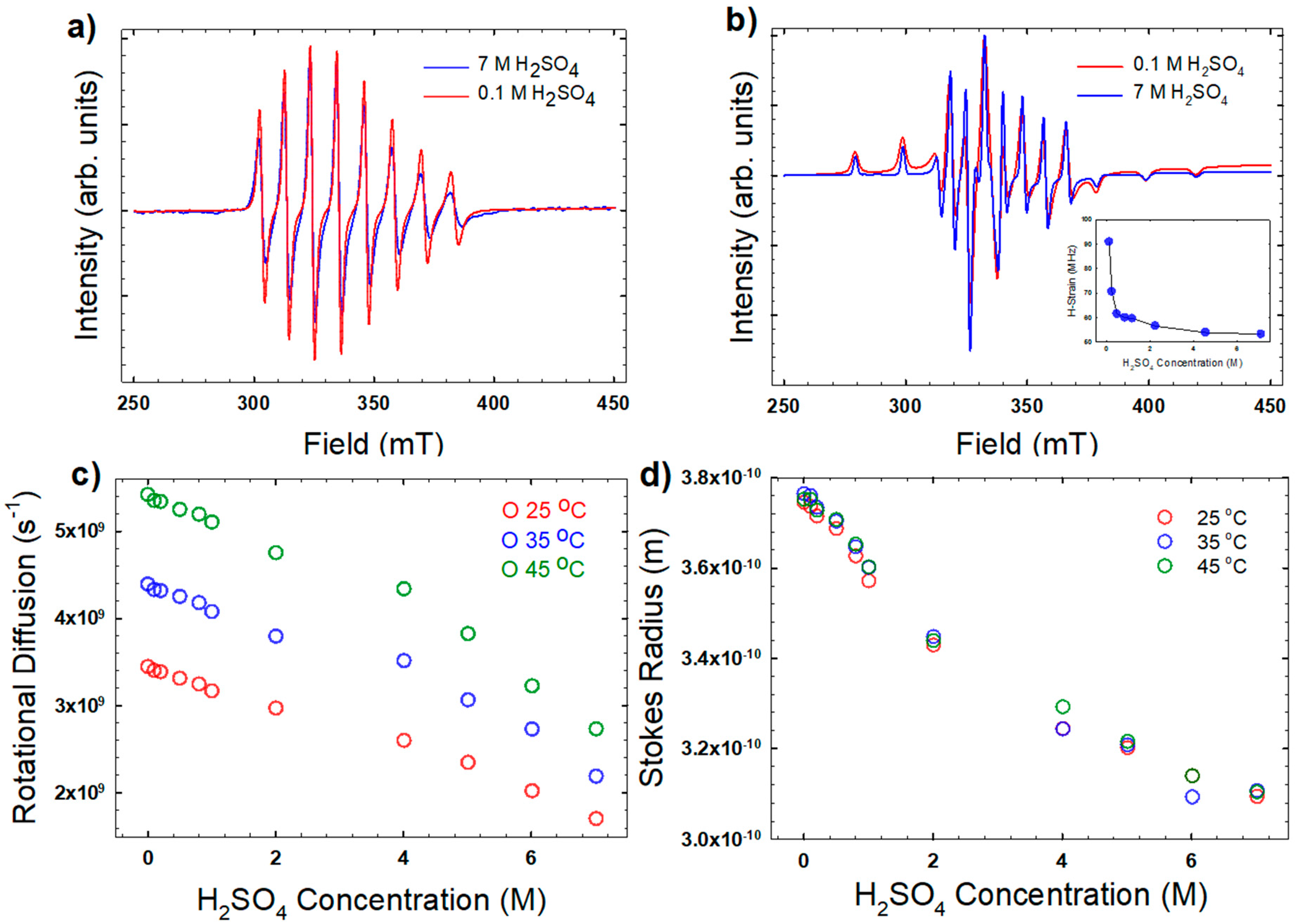
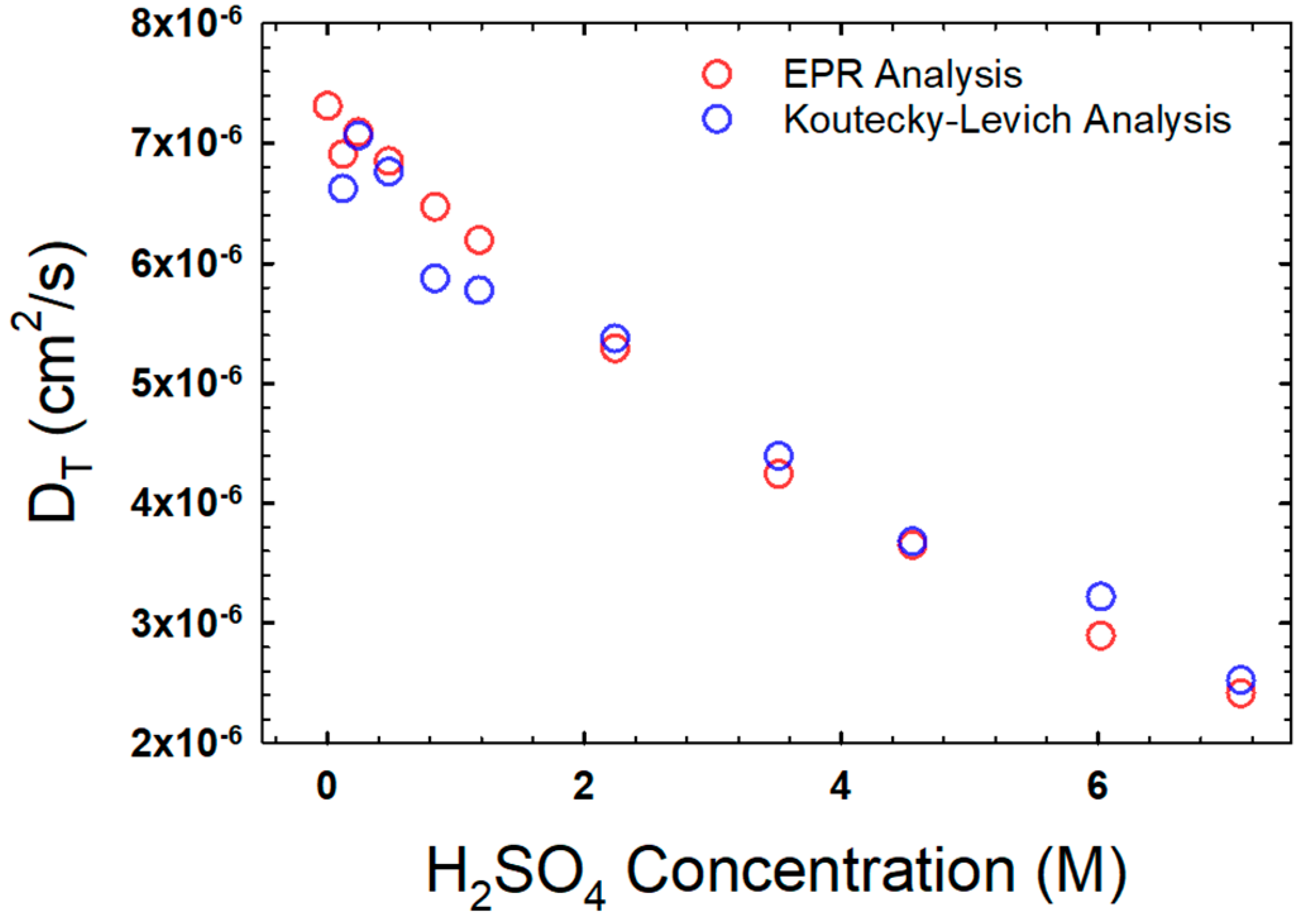
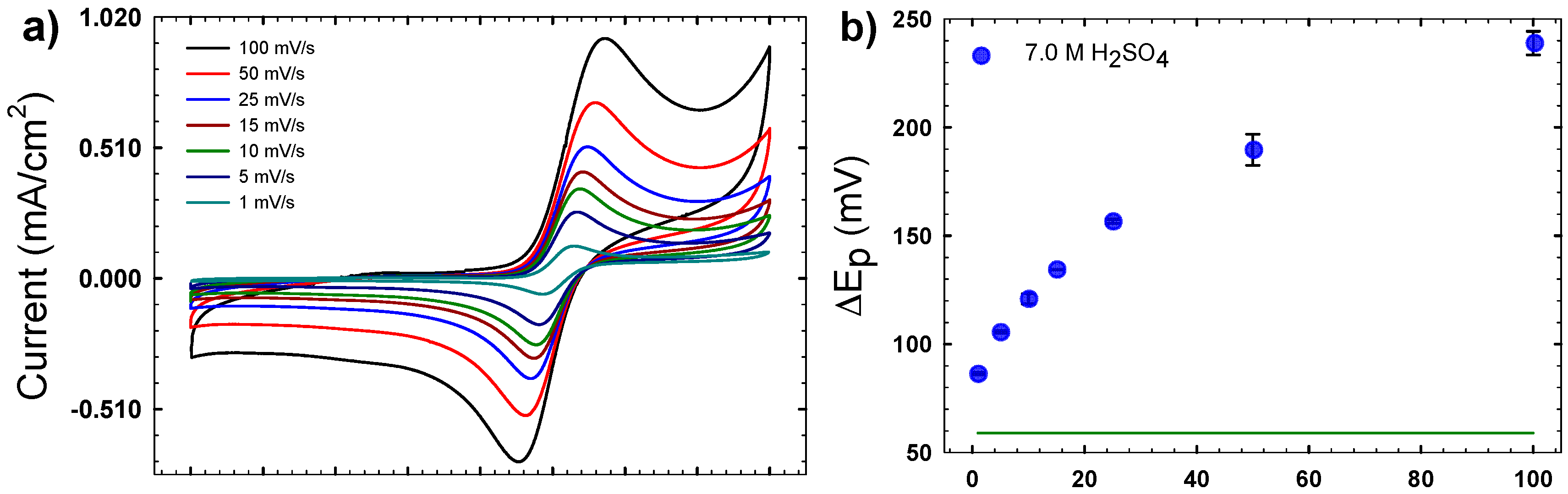
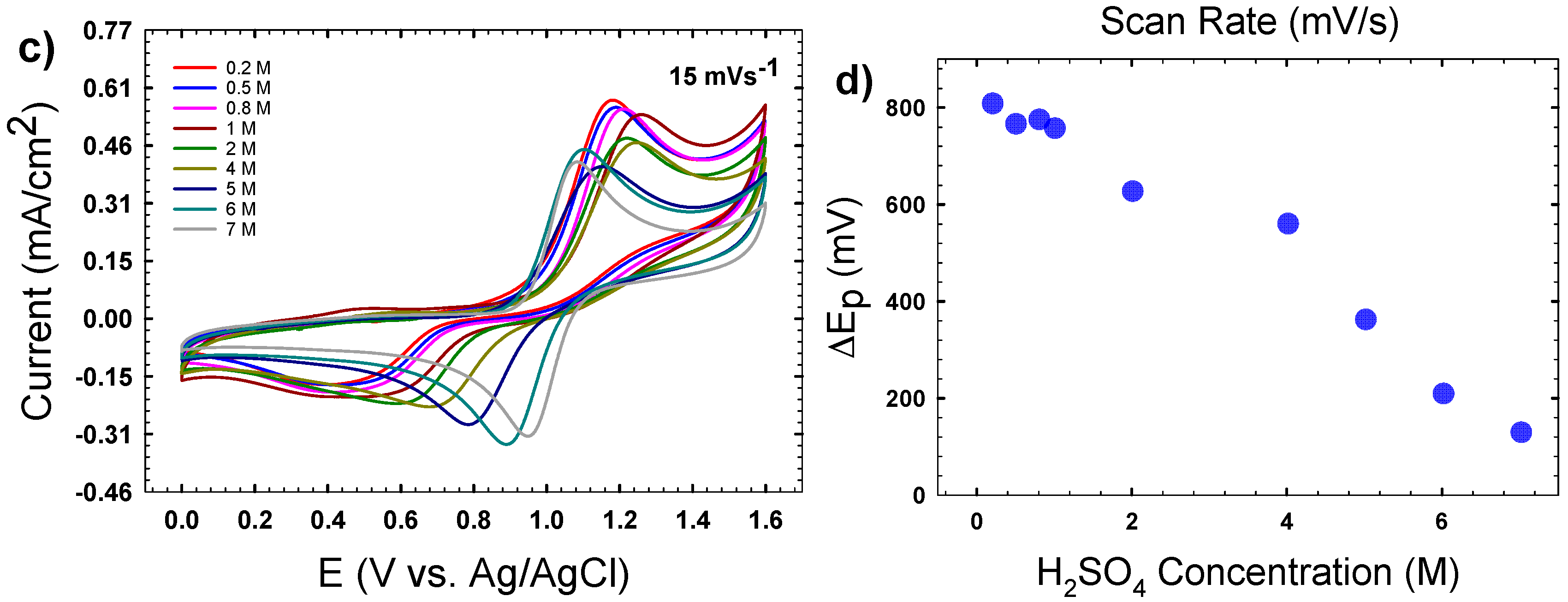
2. Results and Discussion
3. Materials and Methods
3.1. Preparation of Electrolyte Solutions
3.2. Viscosity Measurements
3.3. Electron Paramagnetic Resonance (EPR) Measurements
3.4. Electrochemical Measurements
3.4.1. Rotating Disc Electrode (RDE) Experiments
3.4.2. Cyclic Voltammograms
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Term/Symbol | Definition |
|---|---|
| Hyperfine splitting tensor, MHz | |
| A | Electrode area, cm2 |
| Co | Concentration, mol m−3 |
| DR | Rotational diffusion, s−1 |
| DT | Translational diffusion, m2 s−1 |
| Anodic peak potential, V | |
| Cathodic peak potential, V | |
| Ea | Activation energy, kJ mol−1 |
| F | Faraday’s constant, C mol−1 |
| g-tensor | |
| i | Current, Amperes |
| iK | Kinetic current, Amperes |
| ip,a | Anodic peak current, Amperes |
| ip,c | Cathodic peak current, Amperes |
| (ip,c)o | Cathodic peak current position, Amperes |
| isp | Switching potential current, Amperes |
| kB | Boltzmann Constant, m2 kg s−2 K−1 |
| ko | Rate constant cm s−1 |
| n | Moles |
| ɳ | Viscosity, cP |
| ɳ∞ | Arrhenius preexponential factor cP |
| r | Hydrodynamic radius, m |
| R | Gas constant, J mol−1 K−1 |
| T | Temperature, K |
| α | Electron transfer constant |
| ΔEp | Difference in peak potentials, V |
| ν | Scan rate, V s−1 |
| ω | Rotation rate, rad s−1 |
| No VO2+ | Temperature (K) | ||||
| Sulfuric Acid Conc. (mol L−1) | 298 | 303 | 308 | 313 | 318 |
| 0 | 0.890 | 0.797 | 0.719 | 0.653 | 0.596 |
| 0.1 | 0.909 | 0.809 | 0.730 | 0.663 | 0.603 |
| 0.2 | 0.945 | 0.840 | 0.758 | 0.686 | 0.623 |
| 0.5 | 0.993 | 0.890 | 0.801 | 0.724 | 0.658 |
| 0.8 | 1.046 | 0.929 | 0.839 | 0.756 | 0.685 |
| 1.0 | 1.102 | 0.979 | 0.882 | 0.802 | 0.719 |
| 2.0 | 1.333 | 1.192 | 1.067 | 0.962 | 0.870 |
| 4.0 | 1.679 | 1.499 | 1.344 | 1.208 | 1.090 |
| 5.0 | 1.999 | 1.781 | 1.595 | 1.433 | 1.291 |
| 6.0 | 2.613 | 2.330 | 2.089 | 1.875 | 1.685 |
| 7.0 | 3.242 | 2.869 | 2.562 | 2.296 | 2.092 |
| 0.01 mol L−1 VO2+ | Temperature (K) | ||||
| Sulfuric Acid Conc. (mol L−1) | 298 | 303 | 308 | 313 | 318 |
| 0 | 0.901 | 0.804 | 0.723 | 0.652 | 0.595 |
| 0.1 | 0.913 | 0.812 | 0.736 | 0.658 | 0.607 |
| 0.2 | 0.935 | 0.836 | 0.754 | 0.681 | 0.619 |
| 0.5 | 0.973 | 0.874 | 0.785 | 0.710 | 0.644 |
| 0.8 | 1.037 | 0.927 | 0.836 | 0.753 | 0.684 |
| 1.0 | 1.108 | 0.991 | 0.890 | 0.801 | 0.729 |
| 2.0 | 1.358 | 1.209 | 1.089 | 0.979 | 0.884 |
| 4.0 | 1.768 | 1.579 | 1.414 | 1.268 | 1.144 |
| 5.0 | 2.103 | 1.875 | 1.675 | 1.507 | 1.359 |
| 6.0 | 2.622 | 2.342 | 2.098 | 1.885 | 1.698 |
| 7.0 | 3.215 | 2.887 | 2.583 | 2.339 | 2.095 |
| H2SO4 Concentration (M) No VO2+ | Ea (kJ/mol) | η∞ (cP) |
| 0 | 16.41 | 1.19 × 10−3 |
| 0.1 | 16.19 | 1.32 × 10−3 |
| 0.2 | 16.25 | 1.32 × 10−3 |
| 0.5 | 16.27 | 1.37 × 10−3 |
| 0.8 | 16.37 | 1.40 × 10−3 |
| 1.0 | 16.56 | 1.39 × 10−3 |
| 2.0 | 16.88 | 1.42 × 10−3 |
| 4.0 | 17.18 | 1.72 × 10−3 |
| 5.0 | 17.22 | 2.02 × 10−3 |
| 6.0 | 17.12 | 2.62 × 10−3 |
| 7.0 | 16.81 | 3.63 × 10−3 |
| H2SO4 Concentration (M) With 0.01 M VO2+ | Ea (kJ/mol) | η∞ (cP) |
| 0.1 | 16.08 | 1.37 × 10−3 |
| 0.2 | 16.32 | 1.30 × 10−3 |
| 0.5 | 16.23 | 1.42 × 10−3 |
| 0.8 | 16.58 | 1.29 × 10−3 |
| 1.0 | 16.58 | 1.36 × 10−3 |
| 2.0 | 16.84 | 1.49 × 10−3 |
| 4.0 | 17.01 | 1.75 × 10−3 |
| 5.0 | 17.17 | 1.96 × 10−3 |
| 6.0 | 17.24 | 2.49 × 10−3 |
| 7.0 | 17.33 | 2.97 × 10−3 |
References
- Skyllas-Kazacas, M.; Rychcik, M.; Robins, R.G.; Fane, A.G.; Green, M.A. New All-Vanadium Redox Flow Cell. J. Electrochem. Soc. 1986, 133, 1057–1058. [Google Scholar] [CrossRef]
- Elgammal, R.A.; Tang, Z.; Sun, C.-N.; Lawton, J.; Zawodzinski, T.A. Species Uptake and Mass Transport in Membranes for Vanadium Redox Flow Batteries. Electrochim. Acta 2017, 237, 1–11. [Google Scholar] [CrossRef]
- Gandomi, Y.A.; Aaron, D.S.; Zawodzinski, T.A.; Mench, M.M. In Situ Potential Distribution Measurement and Validated Model for All-Vanadium Redox Flow Battery. J. Electrochem. Soc. 2016, 163, A5188–A5201. [Google Scholar] [CrossRef]
- Lawton, J.S.; Jones, A.; Zawodzinski, T. Concentration dependence of VO2+ crossover of nafion for vanadium redox flow batteries. J. Electrochem. Soc. 2013, 160, A697–A702. [Google Scholar] [CrossRef]
- Lawton, J.S.; Jones, A.M.; Tang, Z.; Lindsey, M.; Zawodzinski, T. Ion effects on vanadium transport in Nafion membranes for vanadium redox flow batteries. J. Electrochem. Soc. 2017, 164, A2987–A2991. [Google Scholar] [CrossRef]
- Gandomi, Y.A.; Aaron, D.S.; Houser, J.R.; Daugherty, M.C.; Clement, J.T.; Pezeshki, A.M.; Ertugrul, T.Y.; Moseley, D.P.; Mench, M.M. Critical Review—Experimental Diagnostics and Material Characterization Techniques Used on Redox Flow Batteries. J. Electrochem. Soc. 2018, 165, A970–A1010. [Google Scholar] [CrossRef] [Green Version]
- Knehr, K.W.; Kumbur, E.C. Open circuit voltage of vanadium redox flow batteries: Discrepancy between models and experiments. Electrochem. Commun. 2011, 13, 342–345. [Google Scholar] [CrossRef]
- Lawton, J.S.; Jones, A.M.; Tang, Z.; Lindsey, M.; Fujimoto, C.; Zawodzinski, T.A. Characterization of Vanadium Ion Uptake in Sulfonated Diels Alder Poly(phenylene) Membranes. J. Electrochem. Soc. 2016, 163, A5229–A5235. [Google Scholar] [CrossRef]
- Lawton, J.S.; Aaron, D.S.; Tang, Z.; Zawodzinski, T.A. Qualitative behavior of vanadium ions in Nafion membranes using electron spin resonance. J. Membr. Sci. 2013, 428, 38–45. [Google Scholar] [CrossRef]
- Kim, S.; Yan, J.; Schwenzer, B.; Zhang, J.; Li, L.; Liu, J.; Yang, Z.; Hickner, M.A. Cycling performance and efficiency of sulfonated poly(sulfone) membranes in vanadium redox flow batteries. Electrochem. Commun. 2010, 12, 1650–1653. [Google Scholar] [CrossRef]
- Schwenzer, B.; Kim, S.; Vijayakumar, M.; Yang, Z.; Liu, J. Correlation of structural differences between Nafion/polyaniline and Nafion/polypyrrole composite membranes and observed transport properties. J. Membr. Sci. 2011, 372, 11–19. [Google Scholar] [CrossRef]
- Liang, X.; Peng, S.; Lei, Y.; Gao, C.; Wang, N.; Liu, S.; Fang, D. Effect of L-glutamic acid on the positive electrolyte for all-vanadium redox flow battery. Electrochim. Acta 2013, 95, 80–86. [Google Scholar] [CrossRef]
- Wu, X.; Liu, S.; Wang, N.; Peng, S.; He, Z. Influence of organic additives on electrochemical properties of the positive electrolyte for all vanadium redox flow battery. Electrochim. Acta 2012, 78, 475–482. [Google Scholar] [CrossRef]
- Roe, S.; Menictas, C.; Skyllas-Kazacos, M. A High Energy Density Vanadium Redox Flow Battery with 3 M Vanadium Electrolyte. J. Electrochem. Soc. 2016, 163, A5023–A5028. [Google Scholar] [CrossRef]
- Skyllas-Kazacos, M.; Cao, L.; Kazacos, M.; Kausar, N.; Mousa, A. Vanadium Electrolyte Studies for the Vanadium Redox Battery—A Review. ChemSusChem 2016, 9. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Schlick, S. Effect of solvents on phase separation in perfluorinated ionomers, from electron spin resonance of VO2+ in swollen membranes, and solutions. Polymer 1995, 36, 1141–1146. [Google Scholar] [CrossRef]
- Szajdzinska-pietek, E.; Pilar, J.; Schlick, S. Structure and Dynamics of Perfluorinated Ionomers in Aqueous Solutions and Swollen Membranes Based on Simulations of ESR Spectra From Spin Propes. J. Phys. Chem. 1995, 99, 313–319. [Google Scholar] [CrossRef]
- Alonso-Amigo, M.G.; Schlick, S. Determination of the Distance between Paramagnetic Centers from Electron Spin Resonance Spectra at L, S, and X Bands. Copper(2+) in Nafion Ionomers. Macromolecules 1989, 22, 2628–2634. [Google Scholar] [CrossRef]
- Lawton, J.S.; Smotkin, E.S.; Budil, D.E. Electron spin resonance investigation of microscopic viscosity, ordering, and polarity in nafion membranes containing methanol-water mixtures. J. Phys. Chem. B 2008, 112, 8549–8557. [Google Scholar] [CrossRef] [PubMed]
- Lawton, J.S.; Budil, D.E. Electron spin resonance investigaion of the effects of methanol on microscopic viscosity, ordering, and polarity in different phases of ionomer membranes with sulfonated polyarylene backbones. J. Membr. Sci. 2010, 357, 47–53. [Google Scholar] [CrossRef]
- Aristov, N.; Habekost, A. Cyclic Voltammetry—A Versatile Electrochemical Method Investigating Electron Transfer Processes. World J. Chem. Educ. 2015, 3, 115–119. [Google Scholar]
- Brownson, D.A.C.; Banks, C.E. The Handbook of Graphene Electrochemistry, 1st ed.; Springer: London, UK, 2014. [Google Scholar]
- Oriji, G.; Katayama, Y.; Miura, T. Investigation on V(IV)/V(V) species in a vanadium redox flow battery. Electrochim. Acta 2004, 49, 3091–3095. [Google Scholar] [CrossRef]
- Aaron, D.; Sun, C.-N.; Bright, M.; Papandrew, A.B.; Mench, M.M.; Zawodzinski, T.A. In Situ Kinetics Studies in All-Vanadium Redox Flow Batteries. ECS Electrochem. Lett. 2013, 2, A29–A31. [Google Scholar] [CrossRef]
- Bourke, A.; Miller, M.A.; Lynch, R.P.; Wainright, J.S.; Savinell, R.F.; Buckley, D.N. Electrode Kinetics in All-Vanadium Flow Batteries: Effects of Electrochemical Treatment. ECS Trans. 2015, 66, 181–211. [Google Scholar] [CrossRef]
- Albanese, N.F.; Chasteen, N.D. Origin of the electron paramagnetic resonance line widths in frozen solution of the oxovanadium(IV) ion. J. Phys. Chem. 1978, 82, 910–914. [Google Scholar] [CrossRef]
- Selbin, J. The Chemistry of Oxovanadium(IV). Chem. Rev. 1965, 65, 153–175. [Google Scholar] [CrossRef]
- Shafique, U.; Anwar, J.; Ali Munawar, M.; Zaman, W.U.; Rehman, R.; Dar, A.; Salman, M.; Saleem, M.; Shahid, N.; Akram, M.; et al. Chemistry of ice: Migration of ions and gases by directional freezing of water. Arab. J. Chem. 2016, 9, S47–S53. [Google Scholar] [CrossRef]
- Barklie, R.C.; Girard, O.; Braddell, O. EPR of vanadyl (2+) in a Nafion membrane. J. Phys. Chem. 1988, 92, 1371–1377. [Google Scholar] [CrossRef]
- Smith, T.S.; LoBrutto, R.; Pecoraro, V.L. Paramagnetic spectroscopy of vanadyl complexes and its applications to biological systems. Coord. Chem. Rev. 2002, 228, 1–18. [Google Scholar] [CrossRef]
- Reuben, J.; Fiat, D. Proton and Deuteron Relaxation in Aqueous Solutions of Vanadyl(IV). Effects of Electron Spin Relaxation and Chemical Exchange. J. Am. Chem. Soc. 1969, 1611, 4652–4656. [Google Scholar] [CrossRef]
- Chasteen, D.; Hanna, M. Resonance Line Widths of vanadyl(IV) hydroxycarboxylates. J. Phys. Chem. 1972, 76, 3951–3959. [Google Scholar] [CrossRef]
- Vijayakumar, M.; Li, L.; Graff, G.; Liu, J.; Zhang, H.; Yang, Z.; Zhi, J. Towards understanding the poor thermal stability of V5+ electrolyte solution in Vanadium Redox Flow Batteries. J. Power Sources 2011, 196, 3669–3672. [Google Scholar] [CrossRef] [Green Version]
- Gattrell, M.; Park, J.; MacDougall, B.; Apte, J.; McCarthy, S.; Wu, C.W. Study of the Mechanism of the Vanadium 4+/5+ Redox Reaction in Acidic Solutions. J. Electrochem. Soc. 2004, 151, A123–A130. [Google Scholar] [CrossRef] [Green Version]
- Nicholson, R.S. Theory and Application of Cyclic Voltammetry for Measurement of Electrode Reaction Kinetics. Anal. Chem. 1965, 37, 1351–1355. [Google Scholar] [CrossRef]
- Elgrishi, N.; Rountree, K.J.; McCarthy, B.D.; Rountree, E.S.; Eisenhart, T.T.; Dempsey, J.L. A Practical Beginner’s Guide to Cyclic Voltammetry. J. Chem. Educ. 2018, 95, 197–206. [Google Scholar] [CrossRef]
- Batchelor-Mcauley, C.; Kätelhön, E.; Barnes, E.O.; Compton, R.G.; Laborda, E.; Molina, A. Recent Advances in Voltammetry. ChemistryOpen 2015, 4, 224–260. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Henstridge, M.C.; Laborda, E.; Dickinson, E.J.F.; Compton, R.G. Redox systems obeying Marcus-Hush-Chidsey electrode kinetics do not obey the Randles-Ševčík equation for linear sweep voltammetry. J. Electroanal. Chem. 2012, 664, 73–79. [Google Scholar] [CrossRef]
- Zhong, S.; Skyllas-Kazacos, M. Electrochemical behaviour of vanadium(V)/vanadium(IV) redox couple at graphite electrodes. J. Power Sources 1992, 39, 1–9. [Google Scholar] [CrossRef]
- Klingler, R.J.; Kochi, J.K. Electron-transfer kinetics from cyclic voltammetry. Quantitative description of electrochemical reversibility. J. Phys. Chem. 1981, 85, 1731–1741. [Google Scholar] [CrossRef]
- Nicholson, R.S.; Shain, I. Theory of Stationary Electrode Polarography: Single Scan and Cyclic Methods Applied to Reversible, Irreversible, and Kinetic Systems. Anal. Chem. 1964, 36, 706–723. [Google Scholar] [CrossRef]
- Lide, D.R. CRC Handbook of Chemistry and Physics, 84th ed.; CRC Press LLC: New York, NY, USA, 2003. [Google Scholar]
- Shoemaker, D.P.G.; Carl, W.; Nibler, J.W. Experiments in Physical Chemistry, 6th ed.; McGraw Hill: Boston, MA, USA, 1996. [Google Scholar]
- Stoll, S.; Schweiger, A. EasySpin, a comprehensive software package for spectral simulation and analysis in EPR. J. Magn. Reson. 2006, 178, 42–55. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stoll, S.; Schweiger, A. EasySpin: Simulating cw ESR spectra. Biol. Magn. Reson. 2007, 27, 299–321. [Google Scholar]



© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lawton, J.S.; Tiano, S.M.; Donnelly, D.J.; Flanagan, S.P.; Arruda, T.M. The Effect of Sulfuric Acid Concentration on the Physical and Electrochemical Properties of Vanadyl Solutions. Batteries 2018, 4, 40. https://doi.org/10.3390/batteries4030040
Lawton JS, Tiano SM, Donnelly DJ, Flanagan SP, Arruda TM. The Effect of Sulfuric Acid Concentration on the Physical and Electrochemical Properties of Vanadyl Solutions. Batteries. 2018; 4(3):40. https://doi.org/10.3390/batteries4030040
Chicago/Turabian StyleLawton, Jamie S., Sophia M. Tiano, Daniel J. Donnelly, Sean P. Flanagan, and Thomas M. Arruda. 2018. "The Effect of Sulfuric Acid Concentration on the Physical and Electrochemical Properties of Vanadyl Solutions" Batteries 4, no. 3: 40. https://doi.org/10.3390/batteries4030040
APA StyleLawton, J. S., Tiano, S. M., Donnelly, D. J., Flanagan, S. P., & Arruda, T. M. (2018). The Effect of Sulfuric Acid Concentration on the Physical and Electrochemical Properties of Vanadyl Solutions. Batteries, 4(3), 40. https://doi.org/10.3390/batteries4030040






