On Two Outer Independent Roman Domination Related Parameters in Torus Graphs
Abstract
:1. Introduction
2. The Outer Independent Roman Domination Number of Regular Graphs
2.1. The Lower Bound on of Regular Graphs
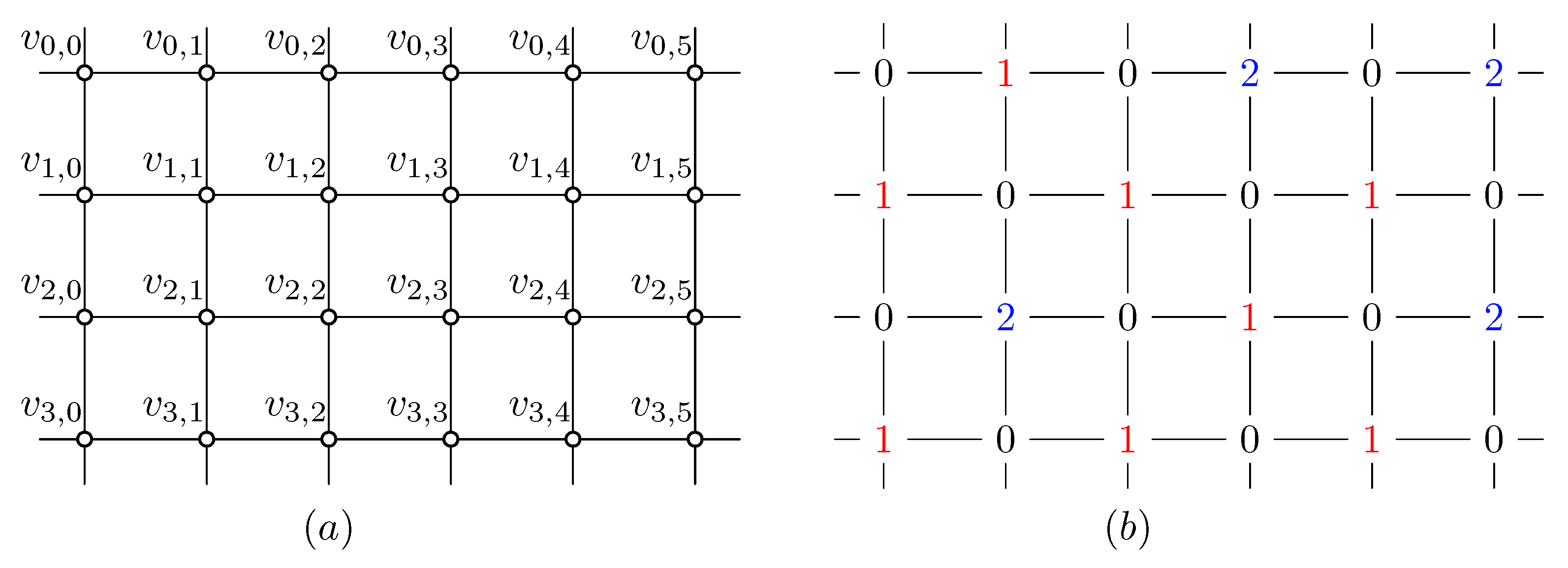
- (a)
- .
- (b)
- .
- (c)
- .
- (d)
- .
2.2. Outer Independent Roman Domination in Torus Graphs
3. The Outer Independent Double Roman Domination Number of Regular Graphs
3.1. The Lower Bound on of Regular Graphs
- (a)
- ,,,.
- (b)
- and .
3.2. Outer Independent Double Roman Domination in Torus Graphs
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cockayne, E.J.; Dreyer, P.A.; Hedetniemi, S.M.; Hedetniemi, S.T. Roman domination in graphs. Discret. Math. 2004, 278, 11–22. [Google Scholar] [CrossRef]
- Henning, M.A.; Hedetniemi, S.T. Defending the Roman empire—A new strategy. Discret. Math. 2003, 266, 239–251. [Google Scholar] [CrossRef]
- Chellali, M.; Haynes, T.W.; Hedetniemi, S.T.; McRae, A.A. Roman {2}-domination. Discret Appl. Math. 2016, 204, 22–28. [Google Scholar] [CrossRef]
- Henning, M.A.; Klostermeyer, W.F.; MacGillivray, G. Perfect Roman domination in trees. Discret Appl. Math. 2018, 236, 235–245. [Google Scholar] [CrossRef]
- Ahangar, H.A.; Henning, M.A.; Löwenstein, C.; Zhao, Y.C.; Samodivkin, V. Signed Roman domination in graphs. J. Comb. Optim. 2014, 27, 241–255. [Google Scholar] [CrossRef]
- Mojdeh, D.A.; Volkmann, L. Roman {3}-domination (double Italian domination). Discret Appl. Math. 2020, 283, 555–564. [Google Scholar] [CrossRef]
- Ahangar, H.A.; Álvarez, M.P.; Chellali, M.; Sheikholeslami, S.M.; Valenzuela-Tripodoro, J.C. Triple Roman domination in graphs. Appl. Math. Comput. 2021, 391, 125444. [Google Scholar]
- Beeler, R.A.; Haynes, T.W.; Hedetniemi, S.T. Double Roman domination. Discret Appl. Math. 2016, 211, 23–29. [Google Scholar] [CrossRef]
- Ahangar, H.A.; Chellali, M.; Samodivkin, V. Outer independent Roman dominating functions in graphs. Int. J. Comput. Math. 2017, 94, 2547–2557. [Google Scholar] [CrossRef]
- Ahangar, H.A.; Chellali, M.; Sheikholeslami, S.M. Outer independent double Roman domination. Appl. Math. Comput. 2020, 364, 124617. [Google Scholar]
- Poureidi, A.; Ghaznavi, M.; Fathali, J. Algorithmic complexity of outer independent Roman domination and outer independent total Roman domination. J. Comb. Optim. 2021, 41, 304–317. [Google Scholar] [CrossRef]
- Martínez, A.C.; García, S.C.; García, A.C.; del Rio, A.M.G. On the outer-independent Roman domination in graphs. Symmetry 2020, 12, 1846. [Google Scholar] [CrossRef]
- Nazari-Moghaddam, S.; Sheikholeslami, S.M. On trees with equal Roman domination and outer-independent Roman domination number. Commun. Comb. Optim. 2019, 4, 185–199. [Google Scholar]
- Mojdeh, D.A.; Samadi, B.; Shao, Z.; Yero, I.G. On the outer independent double Roman domination number. Bull. Iran Math. Soc. 2021, 48, 1789–1803. [Google Scholar] [CrossRef]
- Ahangar, H.A.; Pour, F.N.; Chellali, M.; Sheikholeslami, S.M. Outer independent signed double Roman domination. J. Appl. Math. Comput. 2022, 68, 705–720. [Google Scholar] [CrossRef]
- Fan, W.J.; Ye, A.S.; Miao, F.; Shao, Z.H.; Samodivkin, V.; Sheikholeslami, S.M. Outer-independent Italian domination in graphs. IEEE Access 2019, 7, 22756–22762. [Google Scholar] [CrossRef]
- Li, Z.P.; Shao, Z.H.; Lang, F.N.; Zhang, X.S.; Liu, J.B. Computational complexity of outer-independent total and total Roman domination numbers in trees. IEEE Access 2018, 6, 35544–35550. [Google Scholar] [CrossRef]
- Martínez, A.C.; Kuziak, B.; Yero, I.G. Outer-independent total Roman domination in graphs. Discret Appl. Math. 2019, 269, 107–119. [Google Scholar] [CrossRef]
- Sharma, A.; Reddy, P.V.S. Algorithmic aspects of outer-independent total Roman domination in graphs. Int. J. Found. Comput. Sci. 2021, 32, 331–339. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, H.; Liu, X.; Guo, Y.; Yang, Y. On Two Outer Independent Roman Domination Related Parameters in Torus Graphs. Mathematics 2022, 10, 3361. https://doi.org/10.3390/math10183361
Gao H, Liu X, Guo Y, Yang Y. On Two Outer Independent Roman Domination Related Parameters in Torus Graphs. Mathematics. 2022; 10(18):3361. https://doi.org/10.3390/math10183361
Chicago/Turabian StyleGao, Hong, Xing Liu, Yuanyuan Guo, and Yuansheng Yang. 2022. "On Two Outer Independent Roman Domination Related Parameters in Torus Graphs" Mathematics 10, no. 18: 3361. https://doi.org/10.3390/math10183361
APA StyleGao, H., Liu, X., Guo, Y., & Yang, Y. (2022). On Two Outer Independent Roman Domination Related Parameters in Torus Graphs. Mathematics, 10(18), 3361. https://doi.org/10.3390/math10183361





