Spatial Decay Bounds for the Brinkman Fluid Equations in Double-Diffusive Convection

Abstract

In this paper, we consider the Brinkman equations pipe flow, which includes the salinity and the temperature. Assuming that the fluid satisfies nonlinear boundary conditions at the finite end of the cylinder, using the symmetry of differential inequalities and the energy analysis methods, we establish the exponential decay estimates for homogeneous Brinkman equations. That is to prove that the solutions of the equation decay exponentially with the distance from the finite end of the cylinder. To make the estimate of decay explicit, the bound for the total energy is also derived.

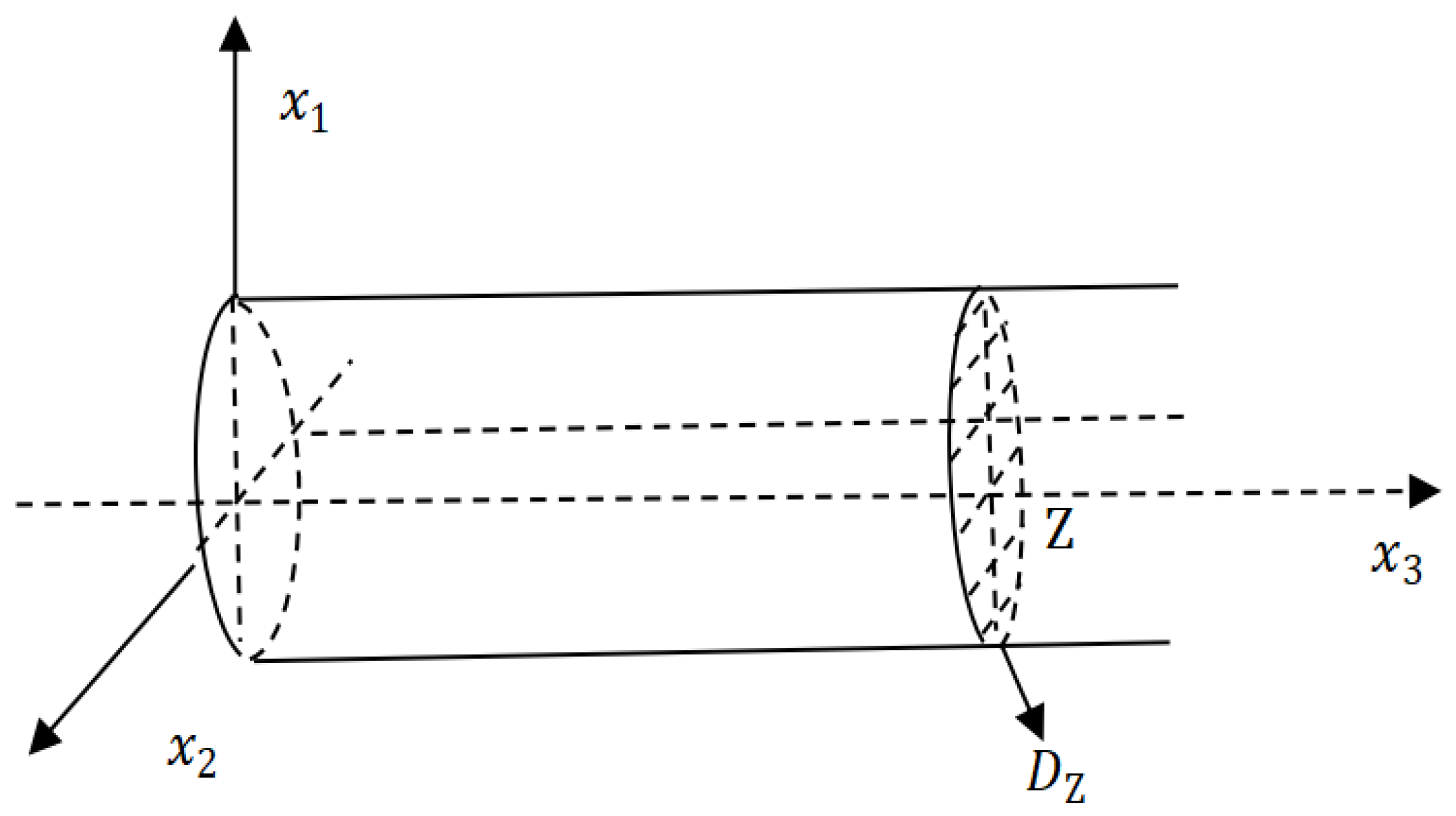
1. Introduction

The Brinkman equations are one of the most important models in fluid mechanics. This model are mainly used to describe flow in a porous medium. For more details, one can refer to Nield and Bejan [1] and Straughan [2]. In the present paper, we define the Brinkman flow depending on the salinity and the temperature in a semi-infinite cylindrical pipe and derive the spatial decay properties. When the homogeneous initial-boundary conditions are applied on the lateral surface of the cylinder, We prove that the solutions of Brinkman equations decays exponentially with spatial variable.

In fact, the Brinkman equations have been studied by many papers in the literature. For example, Straughan [2] considered the mathematical properties of Brinkman equations as well as Darcy and Forchheimer equations, and stated how these equations describe the flow of porous media. Ames and Payne [3] studied the structural stability for the solutions to the viscoelasticity in an ill-posed problem. Franchi and Straughan [4] proved the structural stability for the solutions to the Brinkman equations in porous media in a bounded region. More relevant results one can see [5,6,7,8,9,10]. Paper [11] studied the double diffusive convection in porous medium and obtained the structural stability for the solutions. The continuous dependence for a thermal convection model with temperature-dependent solubility can be found in [12]. For more recent work about continuous dependence, one may refer to [13,14,15,16,17,18,19].

In this paper, let R be a semi-infinite cylinder and $\partial R$ represents the boundary of R. D denotes the cross section of the cylinder with the smooth boundary $\partial D$ (see Figure 1).

In this paper, we also use the following notations

$$R_z=\left\{(x_1,x_2,x_3)\right|(x_1,x_2)\in D,x_3>z\ge 0\},$$

$$D_z=\left\{(x_1,x_2,x_3)\right|(x_1,x_2)\in D,x_3=z\ge 0\},$$

where z is a point along the $x_3$ axis. Clearly, $R_0=R$ and $D_0=D$. Letting $u_i$, T, C and p denote the fluid velocity, temperature, salt concentration and pressure, respectively.

The Brinkman equations we study can be written as [20]

$$\begin{array}{c}\hfill \frac{\partial u_i}{\partial t}=\nu \Delta u_i-k_1{u}_i-p_{,i}+g_{i}T+h_{i}C,inR\times \{t\ge 0\},\end{array}$$

(1)

$$\begin{array}{c}\hfill \frac{\partial T}{\partial t}+u_i\frac{\partial T}{\partial x_i}=k_2\Delta T,inR\times \{t\ge 0\},\end{array}$$

(2)

$$\begin{array}{c}\hfill \frac{\partial C}{\partial t}+u_i\frac{\partial C}{\partial x_i}=k_3\Delta C+\sigma \Delta T,inR\times \{t\ge 0\},\end{array}$$

(3)

$$\begin{array}{c}\hfill u_{i,i}=0,inR\times \{t\ge 0\},\end{array}$$

(4)

where $\nu$, $\sigma >0$ denote the Brinkman coefficient, and the Soret coefficient, respectively. $k_1,k_2,k_3>0$. Without losing generality, we take them equal to 1. $\Delta$ is the Laplacian operator. $g_i\left(x\right)$ and $h_i\left(x\right)$ are gravity field, which are given functions. We suppose that (1)–(4) have the following initial-boundary conditions

$$\begin{array}{c}\hfill u_i=0,T=C=0,on\partial D\times \{t\ge 0\},\end{array}$$

(5)

$$\begin{array}{c}\hfill u_i=0,T=C=0,onR\times \{t=0\}.\end{array}$$

(6)

$$\begin{array}{c}\hfill u_i=f_i(x_1,x_2,t),T=F(x_1,x_2,t),C=G(x_1,x_2,t),on{D}_0\times \{t\ge 0\},\end{array}$$

(7)

$$\begin{array}{c}\hfill u_i,u_{i,j},u_{i,t},T,T_{,i},C,C_{,i},p=o\left(x_3^{-1}\right)uniformlyin{x}_1,x_2,t,as{x}_3\to \infty .\end{array}$$

(8)

In (1)–(8) and in the following, the usual summation convention is employed with repeated Latin subscripts summed from 1 to 3 and repeat Greek subscript summed from 1 to 2. The comma is used to indicate partial differentiation, i.e., $u_{i,j}{u}_{i,j}={\sum }_{i,j=1}^3{\left(\frac{\partial u_i}{\partial x_j}\right)}^2,{\phi }_{\alpha ,\beta }{\phi }_{\alpha ,\beta }={\sum }_{\alpha ,\beta =1}^2{\left(\frac{\partial {\phi }_{\alpha }}{\partial x_{\beta }}\right)}^2$.

The purpose of this paper is to consider the spatial decay properties of the Equations (1)–(8) in a semi-infinite cylindrical pipe by using the symmetry of differential inequalities, that is, to prove that the solutions of the equations decay exponentially with the distance from the finite end of the cylindrical pipe.

In Section 2, some auxiliary inequalities are presented. We establish some useful lemmas in Section 3. The spatial exponential decay estimate for the solution is established in Section 4. Finally, in Section 5 we derive the bounds for the total energies.

2. Auxiliary Results

In this paper, we will use some inequalities in the following sections. Thus, we firstly list them as follows.

Lemma 1.

Let D be a plane domain D with the smooth boundary $\partial D$. If $w=0$ on $\partial D$, then

$$\begin{array}{c}\hfill {\int }_D{w}_{,\alpha }{w}_{,\alpha }dA\ge {\lambda }_1{\int }_{R_z}{w}^{2}dx,\end{array}$$

(9)

where ${\lambda }_1$ is the smallest eigenvalue of the problem

$$\Delta \varphi +\lambda \varphi =0inD,$$

$$\varphi =0on\partial D.$$

Many papers have studied this inequality, e.g., one may see [21,22].

A representation theorem will be also used in next sections. We write this theorem as

Lemma 2.

Let D be a plane Lipschitz bound region and w be a differential function in D which satisfies ${\int }_{D}wdA=0$, then there exists a vector function ${\phi }_{\alpha }(x_1,x_2)$ such that

$${\phi }_{\alpha ,\alpha }=winD,$$

$${\phi }_{\alpha }=0on\partial D,$$

and a positive constant Λ depending only on the geometry of D such that

$$\begin{array}{c}\hfill {\int }_D{\phi }_{\alpha ,\beta }{\phi }_{\alpha ,\beta }dA\le \Lambda {\int }_D{\phi }_{\alpha ,\alpha }^{2}dA.\end{array}$$

(10)

The Lemma 2 was proofed by Babuška and Aziz [23] and Horgan and Wheeler [24] have used the Lemma 1 to viscous flow problems. The explicit upper bound of $\Lambda$ can be found in Horgan and Payne [25]. In this paper, this Lemma 2 is used to eliminate the pressure function difference terms p, since we can prove that $u_3$ satisfy the hypothesis of this Lemma 2 later.

If $w\in C_0^1\left(D\right)$ and $w\in C_0^1\left(R\right)$, the following Sobolev inequalities hold

$$\begin{array}{c}\hfill {\int }_D{w}^{4}dA\le \frac{1}{2}\left[{\int }_D{w}^{2}dA\right]\left[{\int }_D{w}_{,\alpha }{w}_{,\alpha }dA\right],\end{array}$$

(11)

$$\begin{array}{c}\hfill {\int }_{R_z}{w}^{6}dx\le \Omega {\left[{\int }_{R_z}{w}_{,i}{w}_{,i}dx\right]}^3.\end{array}$$

(12)

For (11), we assume that $w\to 0$ as $x_3\to \infty$. Payne [26] has given the derivation of (12). For a special case of the results one can see [27,28]. They have obtained the optimal value of $\Omega$

$$\Omega =\frac{1}{27}{\left(\frac{3}{4}\right)}^4.$$

In the following, we also use the following lemma.

Lemma 3.

If $w\in C^1\left(R_z\right)$, $w_i{|}_{\partial D}=0$ and $w_i\to 0$ as $x_3\to \infty$, then

$$\begin{array}{c}\hfill {\int }_{D_z}{\left(w_i{w}_i\right)}^{2}dA\le 4\sqrt{\Omega }{\left[{\int }_{R_z}{w}_{i,j}{w}_{i,j}dx\right]}^2.\end{array}$$

(13)

We will also use the following lemmas which were derived in [29].

Lemma 4.

Let that the function φ is the solution of the problem

$$\begin{array}{cc}\hfill \Delta \phi & =0in{R}_z,\hfill \\ \hfill \frac{\partial \phi }{\partial n}& =0on\partial D_z,\hfill \\ \hfill \frac{\partial \phi }{\partial n}& =gin{D}_z,\hfill \end{array}$$

(14)

where ${\int }_{D_z}gdA=0$. Then

$$\begin{array}{c}\hfill {\int }_{D_z}{\phi }_{,\alpha }{\phi }_{,\alpha }dA={\int }_{D_z}{g}^{2}dA,\end{array}$$

(15)

$$\begin{array}{c}\hfill {\int }_{R_z}{\phi }_{,i}{\phi }_{,i}dA=\frac{1}{\sqrt{\mu }}{\int }_{D_z}{g}^{2}dA,\end{array}$$

(16)

3. Some Useful Lemmas

In this section, we derive some useful lemmas which will be used in next section. First, we define a weighted energy expression

$$\begin{array}{cc}\hfill E(z,t)& =k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +{\rho }_1{\int }_0^t{\int }_{R_z}(\xi -z)T_{,j}{T}_{,j}dxd\eta +{\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C_{,j}{C}_{,j}dxd\eta \hfill \\ \hfill & =E_1(z,t)+E_2(z,t)+E_3(z,t)+E_4(z,t),\hfill \end{array}$$

(17)

where $k,{\rho }_1,{\rho }_2$ are positive parameters and $\xi >z>0$.

By using the divergence theorem and Equations (1) and (4), we obtain

$$\begin{array}{cc}\hfill E_1(z,t)& =k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }[\nu \Delta u_i-u_i-p_{,i}+g_{i}T+h_{i}C]dxd\eta \hfill \\ \hfill & =k\nu {\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,3}dxd\eta +k{\int }_0^t{\int }_{R_z}{u}_{3,\eta }pdxd\eta \hfill \\ \hfill & +k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{g}_{i}Tdxd\eta +k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{h}_{i}Cdxd\eta \hfill \\ \hfill & -k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}-k{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & \doteq \sum _{i=1}^4{A}_i\hfill \\ \hfill & -\frac{1}{2}k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}-\frac{1}{2}k{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}.\hfill \end{array}$$

(18)

Using the Schwarz inequality, the arithmetic geometric mean inequality and (9), we can obtain

$$\begin{array}{cc}\hfill A_1& \le k\nu {\left[{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta {\int }_0^t{\int }_{R_z}{u}_{i,3}{u}_{i,3}dxd\eta \right]}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{\sqrt{k\nu }}{2}\left[k{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}{u}_{i,3}{u}_{i,3}dxd\eta \right],\hfill \end{array}$$

(19)

$$\begin{array}{cc}\hfill A_3& \le \frac{k}{\sqrt{{\lambda }_1}}{\left[{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta {\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta \right]}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\epsilon }_1}{2}k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\frac{k{\delta }_1^2}{2{\lambda }_1{\epsilon }_1}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta ,\hfill \end{array}$$

(20)

and

$$\begin{array}{cc}\hfill A_4& \le \frac{{\epsilon }_2}{2}k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\frac{k{\delta }_2^2}{2{\lambda }_1{\epsilon }_2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta ,\hfill \end{array}$$

(21)

where ${\epsilon }_1,{\epsilon }_2>0$ will be determined later and

$$\begin{array}{c}\hfill {\delta }_1^2=\underset{D}{max}\left(g_i{g}_i\right),{\delta }_2^2=\underset{D}{max}\left(h_i{h}_i\right),\end{array}$$

(22)

We note that for any $z^{*}>0$, using (4) and (5),

$$\begin{array}{cc}\hfill {\int }_{D_z}{u}_{3,\eta }dA& ={\int }_{D_{z^{*}}}{u}_{3,\eta }dA-{\int }_z^{z^{*}}{\int }_{D_{\xi }}{u}_{3,3\eta }dAd\xi \hfill \\ \hfill & ={\int }_{D_{z^{*}}}{u}_{3,\eta }dA+{\int }_z^{z^{*}}{\int }_{D_{\xi }}{u}_{\alpha ,\alpha \eta }dAd\xi \hfill \\ \hfill & ={\int }_{D_{z^{*}}}{u}_{3,\eta }dA.\hfill \end{array}$$

Since

$$\begin{array}{c}\hfill {\int }_{D_0}{f}_{3,\eta }dA=0,t\ge 0,\end{array}$$

(23)

then,

$$\begin{array}{c}\hfill {\int }_{D_z}{u}_{3,\eta }dA=0.\end{array}$$

Under this assumption, using Lemma 2, there exist vector functions $({\phi }_1,{\phi }_2)$ such that

$$\begin{array}{c}\hfill {\phi }_{\alpha ,\alpha }=u_{3,\eta }inD,{\phi }_{\alpha }=0on\partial D.\end{array}$$

(24)

Hence we have

$$\begin{array}{cc}\hfill A_2& =k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha ,\alpha }pdxd\eta =-k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{p}_{,\alpha }dxd\eta \hfill \\ & =k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }[u_{\alpha ,\eta }-\nu \Delta u_{\alpha }+u_{\alpha }-g_{\alpha }T-h_{\alpha }C]dxd\eta \hfill \\ \hfill & =k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{u}_{\alpha ,\eta }dxd\eta +k\nu {\int }_0^t{\int }_{R_z}{\phi }_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \hfill \\ \hfill & +k\nu {\int }_0^t{\int }_{D_z}{\phi }_{\alpha }{u}_{\alpha ,3}dxd\eta +k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{u}_{\alpha }dxd\eta \hfill \\ \hfill & -k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{g}_{\alpha }Tdxd\eta -k{\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{h}_{\alpha }Cdxd\eta \hfill \\ \hfill & =A_{21}+A_{22}+A_{23}+A_{24}+A_{25}+A_{26}.\hfill \end{array}$$

(25)

Using the Schwarz, Poincaré and the AG mean inequalities, (9) and (10), we can obtain

$$\begin{array}{cc}\hfill A_{21}& \le {\left({\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{\phi }_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{1}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{R_z}{\phi }_{\alpha ,\beta }{\phi }_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\Lambda }^{\frac{1}{2}}}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{R_z}{u}_3^{2}d\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k{\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta ,\hfill \end{array}$$

(26)

$$\begin{array}{cc}\hfill A_{22}& \le k\nu {\left({\int }_0^t{\int }_{R_z}{\phi }_{\alpha ,\beta }{\phi }_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le k\nu {\Lambda }^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k\nu {\Lambda }^{\frac{1}{2}}}{2}{\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2}{\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta ,\hfill \end{array}$$

(27)

$$\begin{array}{cc}\hfill A_{23}& \le k\nu {\left({\int }_0^t{\int }_{D_z}{\phi }_{\alpha }{\phi }_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k\nu {\Lambda }^{\frac{1}{2}}}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{D_z}{u}_{3,\eta }^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta ,\hfill \end{array}$$

(28)

$$\begin{array}{cc}\hfill A_{24}& \le k{\left({\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{\phi }_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha }{u}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\left({\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta ,\hfill \end{array}$$

(29)

$$\begin{array}{cc}\hfill A_{25}& \le k{\left({\int }_0^t{\int }_{R_z}{\phi }_{\alpha }{\phi }_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{g}_{\alpha }{g}_{\alpha }{T}^{2}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k{\delta }_1{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\left({\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{k{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta ,\hfill \end{array}$$

(30)

$$\begin{array}{cc}\hfill A_{26}& \le \frac{k{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta .\hfill \end{array}$$

(31)

Inserting (26)–(31) into (25), then (19)–(21) and (25) into (18), and choosing ${\epsilon }_1={\epsilon }_2=\frac{1}{2}$, we obtain the following lemma.

Lemma 5.

Let $u,T,C,p$ be solutions of Equations (1)–(8) with $g,h\in L_{\infty }(R\times \{t>0\})$ and ${\int }_D{f}_{3}dA=0$. Then

$$\begin{array}{cc}\hfill E_1(z,t)& +k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}+k{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & \le a_{1}k{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +a_2\nu {\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +\frac{k{\delta }_1{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta +\frac{k{\delta }_2{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{3,\eta }^{2}dxd\eta +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \hfill \\ \hfill & +\frac{2k{\delta }_1^2}{{\lambda }_1}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta +\frac{2k{\delta }_2^2}{{\lambda }_1}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta ,\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill a_1& =\sqrt{k\nu }+\frac{{\Lambda }^{\frac{1}{2}}}{\sqrt{{\lambda }_1}}+\nu {\Lambda }^{\frac{1}{2}}+\frac{{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}+\frac{2{\delta }_1{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}+\frac{2{\delta }_2{\Lambda }^{\frac{1}{2}}}{{\lambda }_1},\hfill \\ \hfill a_2& =\frac{\sqrt{k\nu }}{2}+\frac{k{\Lambda }^{\frac{1}{2}}}{2}+\frac{k{\Lambda }^{\frac{1}{2}}}{2\nu \sqrt{{\lambda }_1}}.\hfill \end{array}$$

Similar to Lemma 5, for $E_2(z,t)$ we can obtain the following lemma.

Lemma 6.

Let $u,T,C,p$ be solutions of Equations (1)–(8) with $g,h\in L_{\infty }(R\times \{t>0\})$ and ${\int }_D{f}_{3}dA=0$. Then

$$\begin{array}{cc}\hfill E_2(z,t)& +\frac{1}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{1}{2}{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & \le a_3{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta +\frac{\nu }{2}{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta \hfill \\ \hfill & +\frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +\frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{T}_{,\alpha }{T}_{,\alpha }dxd\eta \hfill \\ \hfill & +\frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{C}_{,\alpha }{C}_{,\alpha }dxd\eta \hfill \\ \hfill & +\frac{{\delta }_1^2}{2{\lambda }_1{\epsilon }_3}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta +\frac{{\delta }_2^2}{2{\lambda }_1{\epsilon }_2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta ,\hfill \end{array}$$

where

$$\begin{array}{cc}\hfill a_3& =\frac{\nu }{2}+\frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}+\frac{\nu {\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{k{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}.\hfill \end{array}$$

Proof. 

By the divergence theorem and Equations (1)–(8), we have

$$\begin{array}{cc}\hfill E_2(z,t)& =-{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta -\frac{1}{2}{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & -\nu {\int }_0^t{\int }_{R_z}{u}_i{u}_{i,3}dxd\eta +{\int }_0^t{\int }_{R_z}(\xi -z)u_i{g}_{i}Tdxd\eta \hfill \\ \hfill & +{\int }_0^t{\int }_{R_z}(\xi -z)u_i{h}_{i}Cdxd\eta +{\int }_0^t{\int }_{R_z}{u}_{3}pdxd\eta \hfill \\ \hfill & \doteq -{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta -\frac{1}{2}{\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & +\sum _{i=1}^4{B}_i.\hfill \end{array}$$

(32)

Using the Schwarz inequality, the Poincaré inequality and the AG mean inequality, we can obtain

$$\begin{array}{cc}\hfill B_1& \le \nu {\left[{\int }_0^t{\int }_{R_z}{u}_{i,3}{u}_{i,3}dxd\eta {\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta \right]}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{\nu }{2}\left[{\int }_0^t{\int }_{R_z}{u}_{i,3}{u}_{i,3}dxd\eta +{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta \right].\hfill \end{array}$$

(33)

Similar to (20) and (21), we have for $B_2$ and $B_3$

$$\begin{array}{cc}\hfill B_2& \le \frac{{\epsilon }_3}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{{\delta }_1^2}{2{\lambda }_1{\epsilon }_3}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta ,\hfill \end{array}$$

(34)

and

$$\begin{array}{cc}\hfill B_3& \le \frac{{\epsilon }_4}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{{\delta }_2^2}{2{\lambda }_1{\epsilon }_2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta ,\hfill \end{array}$$

(35)

where ${\epsilon }_3,{\epsilon }_4$ are positive constants.

To bound $B_4$ in (32), we also require that

$${\int }_D{f}_{3}dA=0.$$

Then using to the Lemma 2 in Section 2, there exist vector functions $({\widehat{\phi }}_1,{\widehat{\phi }}_2)$ such that

$$\begin{array}{c}\hfill {\widehat{\phi }}_{\alpha ,\alpha }=u_3,inD,{\widehat{\phi }}_{\alpha }=0,on\partial D.\end{array}$$

(36)

Therefore, we have

$$\begin{array}{cc}\hfill B_4& ={\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha ,\alpha }pdxd\eta \hfill \\ \hfill & =-{\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{p}_{,\alpha }dxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }[u_{\alpha ,\eta }-\nu \Delta u_{\alpha }+u_{\alpha }-g_{\alpha }T-h_{\alpha }C]dxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{u}_{\alpha ,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta +\nu {\int }_0^t{\int }_{D_z}{\widehat{\phi }}_{\alpha }{u}_{\alpha ,3}dAd\eta \hfill \\ \hfill & +{\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{u}_{\alpha }dxd\eta -{\int }_0^t{\int }_{R_z}{g}_{\alpha }T{\widehat{\phi }}_{\alpha }dxd\eta -{\int }_0^t{\int }_{R_z}{h}_{\alpha }C{\widehat{\phi }}_{\alpha }dxd\eta \hfill \\ \hfill & \doteq \sum _{i=1}^6{B}_{4i}.\hfill \end{array}$$

(37)

As the derivation of (26)–(32), we conclude that

$$\begin{array}{cc}\hfill B_{41}& \le {\left({\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{\widehat{\phi }}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{1}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha ,\beta }{\widehat{\phi }}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\Lambda }^{\frac{1}{2}}}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{R_z}{u}_3^{2}xd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\eta }{u}_{\alpha ,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}\left[{\int }_0^t{\int }_{R_z}{u}_{3,\alpha }{u}_{3,\alpha }xd\eta +{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta \right],\hfill \end{array}$$

(38)

$$\begin{array}{cc}\hfill B_{42}& \le \nu {\left({\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha ,\beta }{\widehat{\phi }}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \nu {\Lambda }^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_3^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha ,\beta }{u}_{\alpha ,\beta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta ,\hfill \end{array}$$

(39)

$$\begin{array}{cc}\hfill B_{43}& \le \nu {\left({\int }_0^t{\int }_{D_z}{\widehat{\phi }}_{\alpha }{\widehat{\phi }}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{\nu {\Lambda }^{\frac{1}{2}}}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_{D_z}{u}_3^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{\nu {\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{u}_{i,j}{u}_{i,j}dxd\eta ,\hfill \end{array}$$

(40)

$$\begin{array}{cc}\hfill B_{44}& \le {\left({\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{\widehat{\phi }}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{u}_{\alpha }{u}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta \hfill \\ \hfill & \le \frac{k{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{i,\alpha }{u}_{i,\alpha }dxd\eta ,\hfill \end{array}$$

(41)

$$\begin{array}{cc}\hfill B_{45}& \le {\left({\int }_0^t{\int }_{R_z}{\widehat{\phi }}_{\alpha }{\widehat{\phi }}_{\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{g}_{\alpha }{g}_{\alpha }{T}^{2}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}{\left({\int }_0^t{\int }_{R_z}{u}_{3,\alpha }{u}_{3,\alpha }dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{T}_{,\alpha }{T}_{,\alpha }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}\left[{\int }_0^t{\int }_{R_z}{u}_{3,\alpha }{u}_{3,\alpha }dxd\eta +{\int }_0^t{\int }_{R_z}{T}_{,\alpha }{T}_{,\alpha }dxd\eta \right],\hfill \end{array}$$

(42)

$$\begin{array}{cc}\hfill B_{46}& \le \frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}\left[{\int }_0^t{\int }_{R_z}{u}_{3,\alpha }{u}_{3,\alpha }dxd\eta +{\int }_0^t{\int }_{R_z}{C}_{,\alpha }{C}_{,\alpha }dxd\eta \right].\hfill \end{array}$$

(43)

Inserting (38)–(43) into (37), we obtain

$$\begin{array}{cc}\hfill B_4& \le [\frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}+\frac{\nu {\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{k{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}]{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +\frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +\frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{T}_{,\alpha }{T}_{,\alpha }dxd\eta \hfill \\ \hfill & +\frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}{\int }_0^t{\int }_{R_z}{C}_{,\alpha }{C}_{,\alpha }dxd\eta .\hfill \end{array}$$

(44)

Inserting (33), (34), (35) and (44) into (32) and choosing ${\epsilon }_3={\epsilon }_4=\frac{1}{2}$, we can obtain Lemma 5.

Next we may bound $E_3(z,t)$. First we let $T_M$ denotes that the maximum of T by using the maximum principle in R, i.e.,

$$\begin{array}{c}\hfill T_M=\underset{D\times \{t>0\}}{max}F(x_1,x_2,t).\end{array}$$

(45)

Integrating by parts, using (3), (5), (6), (7) together with (9) and the AG mean inequality, we have

$$\begin{array}{cc}\hfill E_3(z,t)& =-{\rho }_1{\int }_0^t{\int }_{R_z}T{T}_{,3}dxd\eta -{\rho }_1{\int }_0^t{\int }_{R_z}T(T_{,\eta }+u_i{T}_{,i})dxd\eta \hfill \\ \hfill & =-\frac{{\rho }_1}{2}{\int }_{R_z}(\xi -z)T^{2}dx{|}_{\eta =t}+\frac{{\rho }_1}{2}{\int }_0^t{\int }_{D_z}{T}^{2}dAd\eta +\frac{{\rho }_1}{2}{\int }_0^t{\int }_{R_z}{u}_3{T}^{2}dxd\eta \hfill \\ \hfill & \le -\frac{{\rho }_1}{2}{\int }_{R_z}(\xi -z)T^{2}dx{|}_{\eta =t}+\frac{{\rho }_1}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{T}_{,\alpha }{T}_{,\alpha }dAd\eta \hfill \\ \hfill & +\frac{{\rho }_1{T}_M}{2}{\left({\int }_0^t{\int }_{R_z}{u}_3^{2}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{T}^{2}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le -\frac{{\rho }_1}{2}{\int }_{R_z}(\xi -z)T^{2}dx{|}_{\eta =t}+\frac{{\rho }_1}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{T}_{,\alpha }{T}_{,\alpha }dAd\eta \hfill \\ \hfill & +\frac{{\rho }_1{T}_M}{4{\lambda }_1}\left({\int }_0^t{\int }_{R_z}{u}_{3,\alpha }{u}_{3,\alpha }dxd\eta +{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta \right).\hfill \end{array}$$

(46)

Using Equations (3)–(7) and integrating by parts, we obtain

$$\begin{array}{cc}\hfill E_4(z,t)& =-{\rho }_2{\int }_0^t{\int }_{R_z}C{C}_{,3}dxd\eta -{\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C\left[C_{,\eta }+u_i{C}_{,i}-\sigma \Delta T\right]dxd\eta \hfill \\ \hfill & \le \frac{{\rho }_2}{2}{\int }_0^t{\int }_{D_z}{C}^{2}dAd\eta -\frac{{\rho }_2}{2}{\int }_{R_z}(\xi -z)C^{2}dx{|}_{\eta =t}+\frac{{\rho }_2}{2}{\int }_0^t{\int }_{R_z}{u}_3{C}^{2}dxd\eta \hfill \\ \hfill & -\sigma {\rho }_2{\int }_0^t{\int }_{R_z}C{T}_{,3}dxd\eta -\sigma {\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{T}_{,i}dxd\eta .\hfill \end{array}$$

(47)

By the Schwarz and the AG mean inequalities, it follows that from (47)

$$\begin{array}{cc}\hfill E_4(z,t)& +\frac{{\rho }_2}{2}{\int }_{R_z}(\xi -z)C^{2}dx{|}_{\eta =t}\hfill \\ \hfill & \le \frac{{\rho }_2}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{C}^{2}dAd\eta +\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{T}_{,3}{T}_{,3}dxd\eta +\frac{\sigma {\rho }_2{\epsilon }_5}{2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\frac{\sigma {\rho }_2}{2{\epsilon }_5}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta +\frac{{\rho }_2}{2}{\int }_0^t{\int }_{R_z}{u}_3{C}^{2}dxd\eta ,\hfill \end{array}$$

(48)

for an arbitrary constant ${\epsilon }_5>0$.

In order to bound the last term on the right of (48), using the Equations (9), (11) and (13), the Schwarz inequality and the AG mean inequality to obtain

$$\begin{array}{cc}\hfill \frac{{\rho }_2}{2}& {\int }_0^t{\int }_{R_z}{u}_3{C}^{2}dxd\eta \le \frac{{\rho }_2}{2}{\int }_0^t{\left({\int }_{R_z}{\left(u_{3}C\right)}^{2}dx\right)}^{\frac{1}{2}}{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}d\eta \hfill \\ \hfill & \le \frac{{\rho }_2}{2}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}{\int }_0^t{\int }_z^{\infty }{\left({\int }_{D_{\xi }}{u}_3^{4}dA\right)}^{\frac{1}{4}}{\left({\int }_{D_{\xi }}{C}^{4}dA\right)}^{\frac{1}{4}}d\xi d\eta \hfill \\ \hfill & \le \frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{3}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}{\left({\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}\left({\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta +{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \right),\hfill \end{array}$$

(49)

where the bound for ${max}_t\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}$ will be derived later.

Inserting (49) back into (48), we have

$$\begin{array}{cc}\hfill E_4(z,t)& +\frac{{\rho }_2}{2}{\int }_{R_z}(\xi -z)C^{2}dx{|}_{\eta =t}\hfill \\ \hfill & \le \frac{{\rho }_2}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{C}_{,\alpha }{C}_{,\alpha }dAd\eta +\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta +\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{R_z}{T}_{,3}{T}_{,3}dxd\eta \hfill \\ \hfill & +\frac{\sigma {\rho }_2{\epsilon }_6}{2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta +\frac{\sigma {\rho }_2}{2{\epsilon }_6}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta \hfill \\ \hfill & +\frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}\left({\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta +{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \right).\hfill \end{array}$$

(50)

Combining (46) and (50), we obtain the following Lemma.

Lemma 7.

Let $u,T,C,p$ be solutions of Equations (1)–(8) with $g,h\in L_{\infty }(R\times \{t>0\})$ and ${\int }_D{f}_{3}dA=0$. Then

$$\begin{array}{cc}\hfill E_3(z,t)& +E_4(z,t)\frac{1}{2}{\int }_{R_z}(\xi -z)\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dx{|}_{\eta =t}\hfill \\ \hfill & \le \frac{{\rho }_1}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{T}_{,\alpha }{T}_{,\alpha }dAd\eta +\frac{{\rho }_2}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{C}_{,\alpha }{C}_{,\alpha }dAd\eta \hfill \\ \hfill & +\left[\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}+\frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}\right]{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\left[\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}+\frac{{\rho }_1{T}_M}{4{\lambda }_1}\right]{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta \hfill \\ \hfill & +\frac{\sigma {\rho }_2{\epsilon }_6}{2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta +\frac{\sigma {\rho }_2}{2{\epsilon }_6}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta \hfill \\ \hfill & +\left[\frac{{\rho }_1{T}_M}{4{\lambda }_1}+\frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}\right]{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta ,\hfill \end{array}$$

where ${\epsilon }_6$ is a positive constant. Next, we use Lemmas 5–7 to prove our main result.

□

4. Main Result

First, we introduce a new function

$$\begin{array}{cc}\hfill \psi (z,t)& =k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +{\rho }_1{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta +{\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}+(k+\frac{1}{2}){\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & +\frac{1}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{1}{2}{\int }_{R_z}(\xi -z)\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dx{|}_{\eta =t}.\hfill \end{array}$$

(51)

Using Lemmas 4–6 and in view of (51), we have

$$\begin{array}{cc}\hfill \psi (z,t)& \le a_4{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +a_5{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +a_6{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta +\frac{\nu }{2}{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta +a_7{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{3,\eta }^{2}dAd\eta +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta \hfill \\ \hfill & +\frac{{\rho }_1}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{T}_{,\alpha }{T}_{,\alpha }dAd\eta +\frac{{\rho }_2}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{C}_{,\alpha }{C}_{,\alpha }dAd\eta \hfill \\ \hfill & +\frac{2k{\delta }_1^2}{{\lambda }_1}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta +\frac{2k{\delta }_2^2}{{\lambda }_1}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta \hfill \\ \hfill & +\frac{{\delta }_1^2}{2{\lambda }_1{\epsilon }_3}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,\alpha }{T}_{,\alpha }dxd\eta +\frac{{\delta }_2^2}{2{\lambda }_1{\epsilon }_2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,\alpha }{C}_{,\alpha }dxd\eta \hfill \\ \hfill & +\frac{\sigma {\rho }_2{\epsilon }_6}{2}{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta +\frac{\sigma {\rho }_2}{2{\epsilon }_6}{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta ,\hfill \end{array}$$

(52)

where

$$\begin{array}{cc}\hfill a_4& =a_{1}k+\frac{{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1},a_5=a_2\nu +a_3+\frac{{\rho }_1{T}_M}{4{\lambda }_1}+\frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\},\hfill \\ \hfill a_6& =\frac{k{\delta }_1{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}+\frac{{\delta }_1{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1},a_7=\frac{k{\delta }_2{\Lambda }^{\frac{1}{2}}}{{\lambda }_1}+\frac{{\delta }_2{\Lambda }^{\frac{1}{2}}}{2{\lambda }_1}+\frac{\sigma {\rho }_2}{2\sqrt{{\lambda }_1}}+\frac{{\rho }_2{\Omega }^{\frac{1}{8}}}{2^{\frac{7}{4}}{\lambda }_1^{\frac{1}{4}}}\underset{t}{max}\left\{{\left({\int }_{R_z}{C}^{2}dx\right)}^{\frac{1}{2}}\right\}.\hfill \end{array}$$

Choosing ${\epsilon }_6=\frac{1}{2\sigma },{\rho }_2=\frac{8k{\delta }_2^2}{{\lambda }_1}+\frac{2{\delta }_2^2}{{\lambda }_1{\epsilon }_2},{\rho }_1=\frac{4k{\delta }_1^2}{{\lambda }_1}+\frac{{\delta }_1^2}{{\lambda }_1{\epsilon }_3}+\frac{\sigma {\rho }_2}{{\epsilon }_6}$ and define

$$\begin{array}{cc}\hfill \Psi (z,t)& =k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +\frac{1}{2}{\rho }_1{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta +\frac{1}{2}{\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}+(k+\frac{1}{2}){\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & +\frac{1}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{1}{2}{\int }_{R_z}(\xi -z)\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dx{|}_{\eta =t},\hfill \end{array}$$

(53)

we can have from (52)

$$\begin{array}{cc}\hfill \Psi (z,t)& \le a_4{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +a_5{\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +a_6{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta +\frac{\nu }{2}{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta +a_7{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{3,\eta }^{2}dAd\eta +\frac{k\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}}{\int }_0^t{\int }_{D_z}{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta \hfill \\ \hfill & +\frac{{\rho }_1}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{T}_{,\alpha }{T}_{,\alpha }dAd\eta +\frac{{\rho }_2}{2{\lambda }_1}{\int }_0^t{\int }_{D_z}{C}_{,\alpha }{C}_{,\alpha }dAd\eta .\hfill \end{array}$$

(54)

From (53), we have

$$\begin{array}{cc}\hfill -\frac{\partial \Psi (z,t)}{\partial z}& =k{\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}{u}_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +\frac{1}{2}{\rho }_1{\int }_0^t{\int }_{R_z}{T}_{,i}{T}_{,i}dxd\eta +\frac{1}{2}{\rho }_2{\int }_0^t{\int }_{R_z}{C}_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +k\nu {\int }_{R_z}{u}_{i,j}{u}_{i,j}dx{|}_{\eta =t}+(k+\frac{1}{2}){\int }_{R_z}{u}_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & +\frac{1}{2}{\int }_0^t{\int }_{R_z}{u}_i{u}_{i}dxd\eta +\frac{1}{2}{\int }_{R_z}\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dx{|}_{\eta =t}\hfill \end{array}$$

(55)

and

$$\begin{array}{cc}\hfill \frac{{\partial }^2\Psi (z,t)}{\partial z^2}& =k{\int }_0^t{\int }_{D_z}{u}_{i,\eta }{u}_{i,\eta }dAd\eta +\nu {\int }_0^t{\int }_{D_z}{u}_{i,j}{u}_{i,j}dAd\eta \hfill \\ \hfill & +\frac{1}{2}{\rho }_1{\int }_0^t{\int }_{D_z}{T}_{,i}{T}_{,i}dAd\eta +\frac{1}{2}{\rho }_2{\int }_0^t{\int }_{D_z}{C}_{,i}{C}_{,i}dAd\eta \hfill \\ \hfill & +k\nu {\int }_{D_z}{u}_{i,j}{u}_{i,j}dA{|}_{\eta =t}+(k+\frac{1}{2}){\int }_{D_z}{u}_i{u}_{i}dA{|}_{\eta =t}\hfill \\ \hfill & +\frac{1}{2}{\int }_0^t{\int }_{D_z}{u}_i{u}_{i}dAd\eta +\frac{1}{2}{\int }_{D_z}\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dA{|}_{\eta =t}.\hfill \end{array}$$

(56)

Combining (54), (55) and (56), we have

Thus

$$\begin{array}{c}\hfill \Psi (z,t)\le K_1\left[-\frac{\partial \Psi (z,t)}{\partial z}\right]+K_2\frac{{\partial }^2\Psi (z,t)}{\partial z^2},\end{array}$$

(57)

where

$$\begin{array}{cc}\hfill K_1& =max\{\frac{a_4}{k},\frac{a_5}{\nu },\nu ,\frac{a_6}{{\rho }_1},\frac{a_7}{{\rho }_2}\},\hfill \\ \hfill K_2& =max\{\frac{\nu {\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}},\frac{k{\Lambda }^{\frac{1}{2}}}{2\sqrt{{\lambda }_1}},\frac{1}{2{\lambda }_1}\}.\hfill \end{array}$$

Inequality (57) can be rewritten as

$$\begin{array}{c}\hfill \frac{\partial }{\partial z}\left\{e^{-{\ell }_{1}z}\left(\frac{\partial \Psi }{\partial z}+{\ell }_2\Psi \right)\right\}\ge 0,\end{array}$$

(58)

where

$${\ell }_1=\frac{K_1}{2K_2}+\frac{1}{2}\sqrt{\frac{K_1^2}{K_2^2}+\frac{4}{K_2}},{\ell }_2=-\frac{K_1}{2K_2}+\frac{1}{2}\sqrt{\frac{K_1^2}{K_2^2}+\frac{4}{K_2}}.$$

Integrating (58) from z to ∞ leads to

$$\begin{array}{c}\hfill \frac{\partial \Psi }{\partial z}+{\ell }_2\Psi \le 0,\end{array}$$

and hence

$$\begin{array}{c}\hfill \Psi (z,t)\le \Psi (0,t)e^{-{\ell }_{2}z}.\end{array}$$

(59)

Combining (53) and (59), we can obtain the following theorem.

Theorem 1.

Let $u,T,C,p$ be solutions of Equations (1)–(8) with $g,h\in L_{\infty }(R\times \{t>0\})$ and ${\int }_D{f}_{3}dA=0$. Then

$$\begin{array}{cc}\hfill & k{\int }_0^t{\int }_{R_z}(\xi -z)u_{i,\eta }{u}_{i,\eta }dxd\eta +\nu {\int }_0^t{\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dxd\eta \hfill \\ \hfill & +\frac{1}{2}{\rho }_1{\int }_0^t{\int }_{R_z}(\xi -z)T_{,i}{T}_{,i}dxd\eta +\frac{1}{2}{\rho }_2{\int }_0^t{\int }_{R_z}(\xi -z)C_{,i}{C}_{,i}dxd\eta \hfill \\ \hfill & +k\nu {\int }_{R_z}(\xi -z)u_{i,j}{u}_{i,j}dx{|}_{\eta =t}+(k+\frac{1}{2}){\int }_{R_z}(\xi -z)u_i{u}_{i}dx{|}_{\eta =t}\hfill \\ \hfill & +\frac{1}{2}{\int }_0^t{\int }_{R_z}(\xi -z)u_i{u}_{i}dxd\eta +\frac{1}{2}{\int }_{R_z}(\xi -z)\left[{\rho }_1{T}^2+{\rho }_2{C}^2\right]dx{|}_{\eta =t}\hfill \\ \hfill & \le \Psi (0,t)e^{-{\ell }_{2}z}.\hfill \end{array}$$

(60)

Remark 1.

The result of Theorem 1 belongs to the study of Saint-Venant principle, which shows that the fluid decays exponentially with spatial variables on the cylinder.

Remark 2.

Theorem 1 shows that the solutions of Equations (1)–(8) decays exponentially as $z\to \infty$. To make the decay bound explicit, we have to derive the bounds for $\Psi (0,t)$ and ${max}_t{\int }_R{C}^{2}dx$ in next section.

5. Bounds of $\Psi (\mathbf{0},\mathit{t})$ and ${max}_{\mathit{t}}{\int }_{\mathbf{R}}{\mathit{C}}^{\mathbf{2}}\mathit{dx}$

From the previous section, we can see that $a_3$ involves the quantities ${max}_t{\int }_R{C}^{2}dx$. To make our main result explicit, we have to derive bounds of $\Psi (0,t)$ and ${max}_t{\int }_R{C}^{2}dx$ in term of the physical parameters $\sigma ,\nu ,g_i,h_i$, the boundary data and so on. To do this, we begin with

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta & =-{\int }_0^t{\int }_{D}F{T}_{,3}dAd\eta -{\int }_0^t{\int }_{R}T\Delta Tdxd\eta .\hfill \end{array}$$

(61)

Now we assume that S is a sufficiently smooth function satisfying the same initial and boundary conditions as T. Thus,

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta & =-{\int }_0^t{\int }_{D}S{T}_{,3}dAd\eta -{\int }_0^t{\int }_{R}T\Delta Tdxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_R{S}_{,i}{T}_{,i}dxd\eta -{\int }_0^t{\int }_R(T-S)\Delta Tdxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_R{S}_{,i}{T}_{,i}dxd\eta -{\int }_0^t{\int }_R(T-S)(T_{,\eta }+u_i{T}_{,i})dxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_R{S}_{,i}{T}_{,i}dxd\eta -{\int }_R{T}^{2}dx{|}_{\eta =t}+{\int }_{R}TSdx{|}_{\eta =t}\hfill \\ \hfill & -{\int }_0^t{\int }_R{S}_{,\eta }Tdxd\eta -{\int }_0^t{\int }_R{S}_{,i}T{u}_{i}dxd\eta -\frac{1}{2}{\int }_0^t{\int }_{D}f{F}^{2}dAd\eta .\hfill \end{array}$$

(62)

Using the Schwarz and the arithmetic-geometric mean inequalities, we can obtain

$$\begin{array}{cc}\hfill {\int }_R{T}^{2}dx{|}_{\eta =t}& +{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta \le \frac{1}{2}{\int }_R{S}^{2}dx{|}_{\eta =t}-\frac{1}{2}{\int }_0^t{\int }_{D}f{F}^{2}dAd\eta \hfill \\ \hfill & +\left(\frac{{ϵ}_1}{2}{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta +\frac{1}{2{ϵ}_1}{\int }_0^t{\int }_R{S}_{,i}{S}_{,i}dxd\eta \right)\hfill \\ \hfill & +\left(\frac{{ϵ}_2}{2{\lambda }_1}{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta +\frac{1}{2{ϵ}_2{\lambda }_1}{\int }_0^t{\int }_R{S}_{,\eta }{S}_{,\eta }dxd\eta \right)\hfill \\ \hfill & +\left(\frac{{ϵ}_3{T}_M}{2}{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta +\frac{T_M}{2{ϵ}_3}{\int }_0^t{\int }_R{S}_{,i}{S}_{,i}dxd\eta \right),\hfill \end{array}$$

(63)

where ${ϵ}_1,{ϵ}_2,{ϵ}_3$ are positive constants. Choosing

$$\begin{array}{c}\hfill {ϵ}_1=\frac{1}{2},{ϵ}_2=\frac{{\lambda }_1}{2},\end{array}$$

(64)

we can obtain

$$\begin{array}{cc}\hfill {\int }_R{T}^{2}dx{|}_{\eta =t}+{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta & \le \frac{{ϵ}_3{T}_M}{2}{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta +data.\hfill \end{array}$$

(65)

Obviously, the data terms in (65) involve $\frac{1}{2}{\int }_R{S}^{2}dx{|}_{\eta =t},{\int }_0^t{\int }_R{S}_{,i}{S}_{,i}dxd\eta ,{\int }_0^t{\int }_R{S}_{,\eta }{S}_{,\eta }dxd\eta$ and $-\frac{1}{2}{\int }_0^t{\int }_{D}f{F}^{2}dAd\eta$. Similarly, we can bound ${\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta$ as well as ${max}_t{\int }_R{C}^{2}dx$. Firstly, we introduce a function H:

$$\begin{array}{cc}\hfill \frac{\partial H}{\partial t}+u_i{H}_{,i}& =\Delta H,inR\times \{t>0\},\hfill \\ \hfill H& =0,inR\times \{t=0\},\hfill \\ \hfill H& =0,on\partial D\times \{x_3>0\}\times \{t\ge 0\},\hfill \\ \hfill H& =G(x_1,x_2,t),onD\times \{t>0\},\hfill \end{array}$$

(66)

Then we have

$$\begin{array}{cc}\hfill {(C-H)}_{,t}+u_i{(C-H)}_{,i}& =\Delta (C-H)+\sigma \Delta T,inR\times \{t>0\},\hfill \\ \hfill C-H& =0inR\times \{t=0\},\hfill \\ \hfill C-H& =0on\partial D\times \{x_3>0\}\times \{t\ge 0\},\hfill \\ \hfill C-H& =0onD\times \{t>0\}.\hfill \end{array}$$

(67)

By the triangle inequality, we obtain that

$$\begin{array}{c}\hfill {\left({\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta \right)}^{\frac{1}{2}}\le {\left[{\int }_0^t{\int }_R{(C-H)}_{,i}{(C-H)}_{,i}dxd\eta \right]}^{\frac{1}{2}}+{\left[{\int }_0^t{\int }_R{H}_{,i}{H}_{,i}dxd\eta \right]}^{\frac{1}{2}},\end{array}$$

(68)

and

$$\begin{array}{c}\hfill {\left[\underset{t}{max}{\int }_R{C}^{2}dx\right]}^{\frac{1}{2}}\le {\left[\underset{t}{max}{\int }_R{(C-H)}^{2}dx\right]}^{\frac{1}{2}}+{\left[\underset{t}{max}{\int }_R{H}^{2}dx\right]}^{\frac{1}{2}}.\end{array}$$

(69)

Then,

$$\begin{array}{cc}\hfill \frac{1}{2}{\int }_R{(C-H)}^{2}dx{|}_{\eta =t}+{\int }_0^t{\int }_R{(C-H)}_{,i}{(C-H)}_{,i}dxd\eta & =-\sigma {\int }_0^t{\int }_R{(C-H)}_{,i}{T}_{,i}dxd\eta ,\hfill \end{array}$$

(70)

which follows that

$$\begin{array}{cc}\hfill \frac{1}{2}{\int }_R{(C-H)}^{2}dx{|}_{\eta =t}& +{\int }_0^t{\int }_R{(C-H)}_{,i}{(C-H)}_{,i}dxd\eta \hfill \\ \hfill & \le {\sigma }^2{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta \hfill \\ \hfill & \le \frac{{ϵ}_3{T}_M{\sigma }^2}{2}{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta +data.\hfill \end{array}$$

(71)

Just as in the computation for T, we have the following inequality

$$\begin{array}{cc}\hfill \frac{1}{2}{\int }_R{H}^2{dx|}_{\eta =t}+{\int }_0^t{\int }_R{H}_{,i}{H}_{,i}dxd\eta & \le {ϵ}_4{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta +data.\hfill \end{array}$$

(72)

Thus,

$$\begin{array}{cc}\hfill \frac{1}{2}{\int }_R{C}^2{dx|}_{\eta =t}+{\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta & \le {ϵ}_5{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta +data,\hfill \end{array}$$

(73)

where ${ϵ}_5>0$ depends on ${ϵ}_3,{ϵ}_4$ and $\sigma$. Next we have to derive a bound for ${\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta$ in term of data. To do this, we define a function

$$\begin{array}{c}\hfill {Ꮗ}_i=f_i{e}^{-{\varsigma }_{1}z},\end{array}$$

(74)

for some positive constant ${Ꮗ}_i$. Then,

$$\begin{array}{cc}{\left[{\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \right]}^{\frac{1}{2}}\hfill & \le {\left[{\int }_0^t{\int }_R{(u_i-{Ꮗ}_i)}_{,j}{(u_i-{Ꮗ}_i)}_{,j}dxd\eta \right]}^{\frac{1}{2}}\hfill \\ \hfill & +{\left[{\int }_0^t{\int }_R{Ꮗ}_{i,j}{Ꮗ}_{i,j}dxd\eta \right]}^{\frac{1}{2}}.\hfill \end{array}$$

Obviously, we find that the last term of (75) is a data term. Now

$$\begin{array}{cc}\hfill \nu & {\int }_0^t{\int }_R{(u_i-{Ꮗ}_i)}_{,j}{(u_i-{Ꮗ}_i)}_{,j}dxd\eta \hfill \\ \hfill & =-{\int }_0^t{\int }_R{(u_i-{Ꮗ}_i)}_{,j}\left[(u_i-{Ꮗ}_i)+p_{,i}-g_{i}T-h_{i}C+{Ꮗ}_i-\nu \Delta {Ꮗ}_i\right]dxd\eta \hfill \end{array}$$

(75)

or

$$\begin{array}{cc}\hfill \frac{\nu }{2}& {\int }_0^t{\int }_R{(u_i-{Ꮗ}_i)}_{,j}{(u_i-{Ꮗ}_i)}_{,j}dxd\eta \hfill \\ \hfill & \le -{\int }_0^t{\int }_{R}p{Ꮗ}_{i,i}dxd\eta +\frac{{\delta }_1^2}{2}{\int }_0^t{\int }_R{T}^{2}dxd\eta +\frac{{\delta }_2^2}{2}{\int }_0^t{\int }_R{C}^{2}dxd\eta +data.\hfill \end{array}$$

(76)

Noting that

$$\begin{array}{c}\hfill {Ꮗ}_{i,i}=(f_{\alpha ,\alpha }-{\varsigma }_1{f}_3)e^{-{\varsigma }_{1}z}=0,\end{array}$$

(77)

in R for ${\varsigma }_1=\frac{f_{\alpha ,\alpha }}{f_3}$, we can rewrite (76) as

$$\begin{array}{cc}\hfill \frac{\nu }{2}& {\int }_0^t{\int }_R{(u_i-{Ꮗ}_i)}_{,j}{(u_i-{Ꮗ}_i)}_{,j}dxd\eta \hfill \\ \hfill & \le \frac{{\delta }_1^2}{2{\lambda }_1}{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta +\frac{{\delta }_2^2}{2{\lambda }_1}{\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta +data.\hfill \end{array}$$

(78)

Inserting (78) back into (75), we may have a bound of the form

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \le C_1{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta +C_2{\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta +data,\end{array}$$

(79)

for computable $C_1$ and $C_2$. Combining (65) and (73) and by inequality (17), we have

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \le \frac{C_1{T}_M}{2{\lambda }_1}{ϵ}_3{\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta +\frac{C_2}{{\lambda }_1}{ϵ}_5{\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta +data.\end{array}$$

(80)

It is clear to see that

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \le data,\end{array}$$

(81)

for ${ϵ}_3=\frac{{\lambda }_1}{2C_1{T}_M},{ϵ}_5=\frac{{\lambda }_1}{4C_2}$. From (65) and (73), we can obtain

$$\begin{array}{c}\hfill \underset{t}{max}{\int }_R{T}^{2}dx\le data,\underset{t}{max}{\int }_R{C}^{2}dx\le data,\end{array}$$

(82)

and

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta \le data,{\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta \le data.\end{array}$$

(83)

Next we seek bound for the total energy $\Psi (0,t)$. From (54) we can obtain for $\Psi (0,t)$

$$\begin{array}{cc}\hfill \Psi (0,t)& \le a_4{\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta +\frac{\nu }{2}{\int }_0^t{\int }_R{u}_i{u}_{i}dxd\eta \hfill \\ \hfill & +b_1{\int }_0^t{\int }_D{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta +data.\hfill \end{array}$$

(84)

We are left to derive bounds for ${\int }_0^t{\int }_{R_z}{u}_{i,\eta }{u}_{i,\eta }dxd\eta$ and ${\int }_0^t{\int }_D{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta$. Multiplying (1) with $u_{i,\eta }$ and integrating in the region $R\times [0,t]$, we have

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta & ={\int }_0^t{\int }_R{u}_{i,\eta }[\nu \Delta u_i-u_i-p_{,i}+g_{i}T+h_{i}C]dxd\eta ,\hfill \end{array}$$

(85)

which follows that

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta & \le -2\nu {\int }_0^t{\int }_D{u}_{\alpha ,3}{u}_{\alpha ,\eta }dAd\eta +2{\int }_0^t{\int }_D{u}_{3,\eta }pdAd\eta \hfill \\ \hfill & +\frac{{\delta }_1^2}{2{\lambda }_1}{\int }_0^t{\int }_R{T}_{,i}{T}_{,i}dxd\eta +\frac{{\delta }_1^2}{2{\lambda }_1}{\int }_0^t{\int }_R{C}_{,i}{C}_{,i}dxd\eta +data\hfill \\ \hfill & \le -2\nu {\int }_0^t{\int }_D{u}_{\alpha ,3}{f}_{\alpha ,\eta }dAd\eta +2{\int }_0^t{\int }_D{f}_{3,\eta }pdAd\eta +data,\hfill \end{array}$$

(86)

where we have used the fact $u_{3,3}=-u_{\alpha ,\alpha }=-f_{\alpha ,\alpha }$ on $D_0$ and (83), and ${\epsilon }_6$ is a positive constants. For the first term of (86), using the Schwarz and the AG mean inequalities we have

$$\begin{array}{cc}\hfill -2\nu {\int }_0^t{\int }_{D_0}{f}_{\alpha ,\eta }{u}_{\alpha ,3}dxd\eta & \le 2\nu {\left({\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_{D_0}{f}_{\alpha ,\eta }{f}_{\alpha ,\eta }dAd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & \le {\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dAd\eta +data.\hfill \end{array}$$

(87)

To bound the second term on the right of (86), we define $\overline{p}$ to be the mean value of p over $D_0$, i.e.,

$$\begin{array}{c}\hfill \overline{p}=\frac{1}{|D_0|}{\int }_{D_0}pdA,\end{array}$$

(88)

where $|D_0|$ is the measure of $D_0$. Since

$$\begin{array}{c}\hfill {\int }_{D_0}{f}_{3,\eta }\overline{p}dA=\overline{p}{\int }_{D_0}{f}_{3,\eta }dA=0,\end{array}$$

(89)

we obtain

$$\begin{array}{c}\hfill {\int }_{D_0}{f}_{3,\eta }pdA={\int }_{D_0}{f}_{3,\eta }(p-\overline{p})dA.\end{array}$$

(90)

It follows by using Schwarz inequality that

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_{D_0}{f}_{3,\eta }pdxd\eta & ={\int }_0^t{\int }_{D_0}{f}_{3,\eta }(p-\overline{p})dAd\eta \le data+{ϵ}_6{\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta ,\hfill \end{array}$$

(91)

where ${ϵ}_6$ is a positive constant to be determined later.

To deal with the integral ${\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta$, we let an auxiliary function $\chi$ satisfying:

$$\begin{array}{c}\hfill \Delta \chi =0,\frac{\partial \chi }{\partial n}=0on\partial D_0,\frac{\partial \chi }{\partial n}=p-\overline{p},in{D}_0.\end{array}$$

(92)

From the definition of $\overline{p}$ in (88), it is clear that ${\int }_{D_0}(p-\overline{p})dA=0$. Thus, the necessary condition for the existence of a solution is satisfied and we compute

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta & ={\int }_0^t{\int }_{\partial R}(p-\overline{p})\frac{\partial \chi }{\partial n}dxd\eta ={\int }_0^t{\int }_R{(p-\overline{p})}_{,i}{\chi }_{,i}dxd\eta \hfill \\ \hfill & ={\int }_0^t{\int }_R{\chi }_{,i}[-u_{i,\eta }+\nu u_{i,jj}-u_i+g_{i}T+h_{i}C]dxd\eta .\hfill \end{array}$$

(93)

Since

$$\begin{array}{cc}\hfill \nu {\int }_0^t{\int }_R{\chi }_{,i}{u}_{i,jj}dxd\eta & =-\nu {\int }_0^t{\int }_D{\chi }_{,i}{u}_{i,3}dxd\eta -\nu {\int }_0^t{\int }_R{\chi }_{,ij}{u}_{i,j}dxd\eta \hfill \\ \hfill & =\nu {\int }_0^t{\int }_D{\chi }_{,3}{f}_{\alpha ,\alpha }dxd\eta -\nu {\int }_0^t{\int }_D{\chi }_{,\alpha }{u}_{\alpha ,3}dxd\eta \hfill \\ \hfill & +\nu {\int }_0^t{\int }_D{\chi }_{,j}{u}_{3,j}dxd\eta +\nu {\int }_0^t{\int }_R{\chi }_{,j}{u}_{i,ij}dxd\eta \hfill \\ \hfill & =-\nu {\int }_0^t{\int }_D{\chi }_{,\alpha }{u}_{\alpha ,3}dxd\eta +\nu {\int }_0^t{\int }_D{\chi }_{,\alpha }{f}_{3,\alpha }dxd\eta .\hfill \end{array}$$

(94)

From (93), we can obtain

$$\begin{array}{cc}\hfill {\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta & ={\int }_0^t{\int }_R{\chi }_{,i}[-u_{i,\eta }+\nu u_{i,jj}-u_i+g_{i}T+h_{i}C]dxd\eta \hfill \\ \hfill & \le {\left({\int }_0^t{\int }_R{\chi }_{,i}{\chi }_{,i}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\nu {\left({\int }_0^t{\int }_D{\chi }_{,\alpha }{\chi }_{,\alpha }dAd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_D{u}_{3,\alpha }{u}_{3,\alpha }dAd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\nu {\left({\int }_0^t{\int }_D{\chi }_{,\alpha }{\chi }_{,\alpha }dAd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_D{f}_{3,\alpha }{f}_{3,\alpha }dAd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\frac{1}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_R{\chi }_{,i}{\chi }_{,i}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\frac{{\delta }_1}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_R{\chi }_{,i}{\chi }_{,i}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_R{T}_{,j}{T}_{,j}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\frac{{\delta }_2}{\sqrt{{\lambda }_1}}{\left({\int }_0^t{\int }_R{\chi }_{,i}{\chi }_{,i}dxd\eta \right)}^{\frac{1}{2}}{\left({\int }_0^t{\int }_R{C}_{,j}{C}_{,j}dxd\eta \right)}^{\frac{1}{2}}.\hfill \end{array}$$

(95)

Making use of (15), (16), (81) and (83) with $g=p-\overline{p}$, we have

$$\begin{array}{cc}\hfill [{\int }_0^t{\int }_{D_0}& {(p-\overline{p})}^{2}dAd\eta {]}^{\frac{1}{2}}\hfill \\ \hfill & \le \frac{1}{\sqrt{\mu }}{\left({\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta \right)}^{\frac{1}{2}}+\nu {\left({\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\nu {\left({\int }_0^t{\int }_{D_0}{f}_{3,\alpha }{f}_{3,\alpha }dxd\eta \right)}^{\frac{1}{2}}+\frac{1}{\sqrt{\mu {\lambda }_1}}{\left({\int }_0^t{\int }_R{u}_{i,j}{u}_{i,j}dxd\eta \right)}^{\frac{1}{2}}\hfill \\ \hfill & +\frac{{\delta }_1}{\sqrt{\mu {\lambda }_1}}{\left({\int }_0^t{\int }_R{T}_{,j}{T}_{,j}dxd\eta \right)}^{\frac{1}{2}}+\frac{{\delta }_2}{\sqrt{\mu {\lambda }_1}}{\left({\int }_0^t{\int }_R{C}_{,j}{C}_{,j}dxd\eta \right)}^{\frac{1}{2}},\hfill \end{array}$$

(96)

which follows that

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta \le data+c_3{\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta +c_4{\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta .\end{array}$$

(97)

Obviously, from (97) we must establish a bound for the term ${\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta$. To do this, we begin with the identity

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{u}_{i,3}\left[\nu u_{i,jj}-u_i-p_{,i}-u_{i,\eta }+g_{i}T+h_{i}C\right]dxd\eta =0.\end{array}$$

(98)

Integrating (98) by parts, we can have

$$\begin{array}{cc}\hfill & -\nu {\int }_0^t{\int }_{D_0}{u}_{i,3}{u}_{i,3}dxd\eta +\nu {\int }_0^t{\int }_R{u}_{i,j3}{u}_{i,j}dxd\eta +{\int }_0^t{\int }_R{u}_{i,3}{u}_{i}dxd\eta \hfill \\ & +{\int }_0^t{\int }_R{u}_{i,3}{p}_{,i}dxd\eta +{\int }_0^t{\int }_R{u}_{i,3}{u}_{i,\eta }dxd\eta +{\int }_0^t{\int }_R{u}_{i,3}{g}_{i}Tdxd\eta \hfill \\ & +{\int }_0^t{\int }_R{u}_{i,3}{h}_{i}Cdxd\eta =0,\hfill \end{array}$$

(99)

which follows that

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \le data+{\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}pdAd\eta +{ϵ}_7{\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta .\end{array}$$

(100)

where ${ϵ}_7$ is a positive constant.

As the derivation of (91), for the term ${\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}pdAd\eta$ we can obtain

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}pdAd\eta \le data+{ϵ}_8{\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta ,\end{array}$$

(101)

where ${ϵ}_8$ is a positive constant.

Combining (97), (100) and (101), we have

$$\begin{array}{c}\hfill \left(1-{ϵ}_8{c}_3\right){\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dxd\eta \le data+c_3{ϵ}_7{\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta .\end{array}$$

(102)

Combing (86), (87), (91) and (100), we obtain

$$\begin{array}{c}\hfill (1-{ϵ}_7){\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta \le data+({ϵ}_6+{ϵ}_7){\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dxd\eta .\end{array}$$

(103)

Choosing ${\epsilon }_7$ and ${ϵ}_8$ small enough such that $1-{ϵ}_8{c}_3>0$ and $1-{ϵ}_7>0$, from (102) and (103) we can obtain

$$\begin{array}{c}\hfill {\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta \le data,\end{array}$$

(104)

and

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta \le data.\end{array}$$

(105)

Inserting (101) back into (100), we obtain

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \le data+{ϵ}_8{\int }_0^t{\int }_{D_0}{(p-\overline{p})}^{2}dAd\eta +{ϵ}_7{\int }_0^t{\int }_R{u}_{i,\eta }{u}_{i,\eta }dxd\eta .\end{array}$$

(106)

In light of (104) and (105), we have

$$\begin{array}{c}\hfill {\int }_0^t{\int }_{D_0}{u}_{\alpha ,3}{u}_{\alpha ,3}dxd\eta \le data.\end{array}$$

(107)

Recalling (84) and using (104) and (107), we obtain

$$\begin{array}{c}\hfill \Psi (0,t)\le data,\end{array}$$

(108)

which is to say that we have bounded the total energy.

6. Conclusions

In this paper, we consider the spatial decay bounds for the Brinkman equations in double-diffusive convection in a semi-infinite pipe. Using the results of this paper, we can continue to study the continuous dependence of the solution on the parameters in the system of equations. In addition, Using the results of this paper, we can continue to study the continuous dependence of the solution on the parameters in the system of equations. This research can refer to the method of [30,31]. In addition, if Equation (1) is replaced by a nonlinear problem (e.g., Forchheimer equations), it will be a more interesting topic.