Mining Height Effect and Application of Upper Protected Layer Mining Pressure Relief
Abstract
:1. Introduction
2. Methods
2.1. Stress Distribution Mechanisms in Protected Layers Affected by Mining Height
2.1.1. Stress Distribution Mechanisms in the Extraction Zone Affected by Mining Height
- ①
- Transport pattern of the roof rock caused by mining height
- ②
- Influence of different roof collapse structures on stress distribution in goaf
2.1.2. Analysis of the Effect of Height Adjustment on Pressure Relief by the Protected Layer
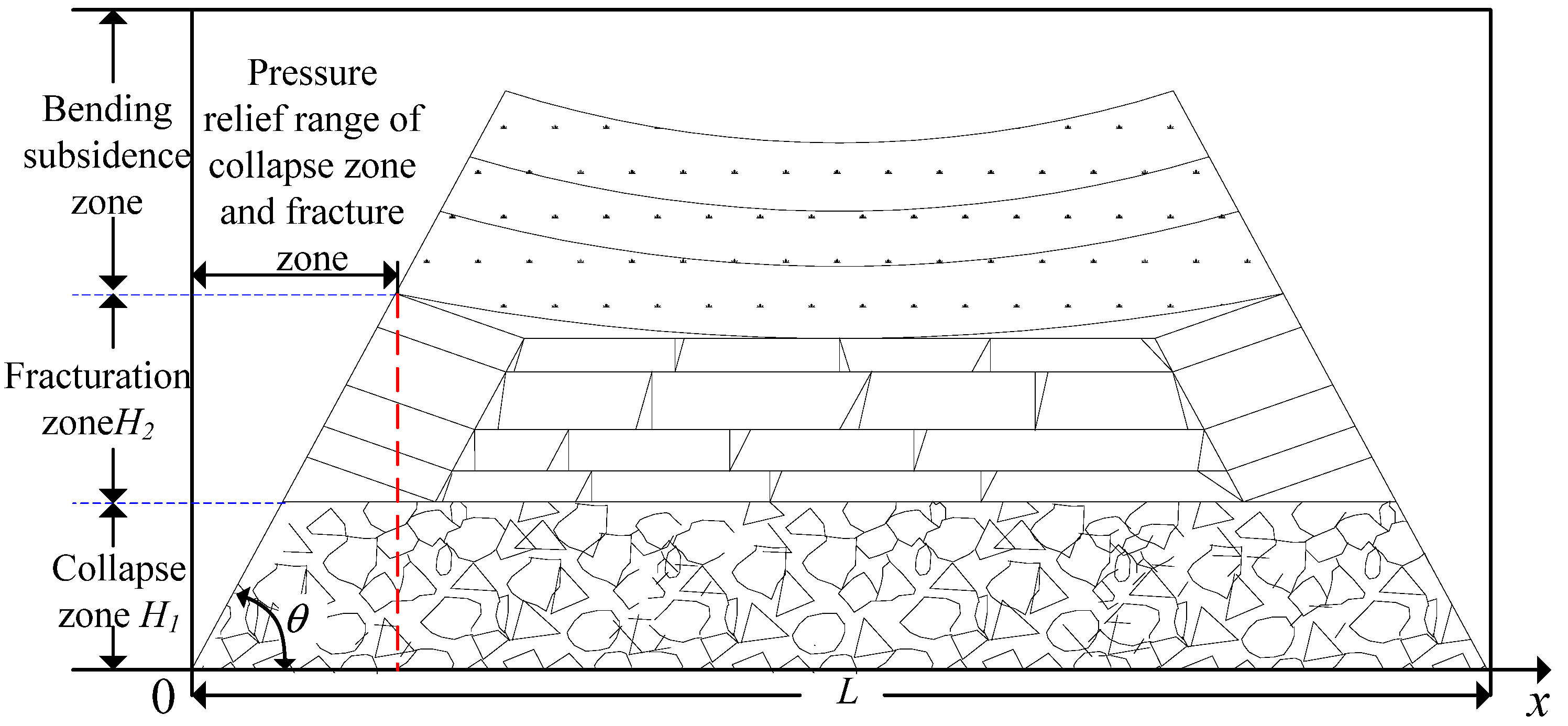
2.2. Calculation Model of Stress Distribution in Goaf with Different Mining Heights
2.2.1. Calculation of the Forces Acting on the Goaf from the Fracture Zone and Collapse Zone
2.2.2. Calculation of the Forces acting on the Goaf from the Curved Sinking Zone
2.3. Stress Distribution Pattern of the Goaf affected by Mining Height
2.3.1. Stress Distribution Pattern of Collapse Zone and Fracture Zone in Goaf under the Influence of Mining Height
2.3.2. Stress Distribution Pattern in the Bending and Sinking Zone of Goaf under the Influence of Mining Height
2.3.3. Theoretical Analysis of Stress Distribution in Goaf Affected by Mining Heights
- ①
- Background of the project
- ②
- Calculation of stress distribution patterns in the collapse zone and fracture zone corresponding to the goaf
- a.
- Height estimation of collapse zone and fracture zone
- b.
- Analysis of the extent of pressure relief in the goaf
- ③
- Calculation of the stress distribution law in the goaf corresponding to the bending and sinking zone
- a.
- Foundation coefficient ki analysis of the collapse zone
- b.
- Analysis of foundation coefficients in the area of unbent rock kj
- Determination of the bending rock beam
- Foundation coefficients in the area of unbroken rock
- c.
- Calculation of stress distribution in the extraction zone of the bending and sinking zone
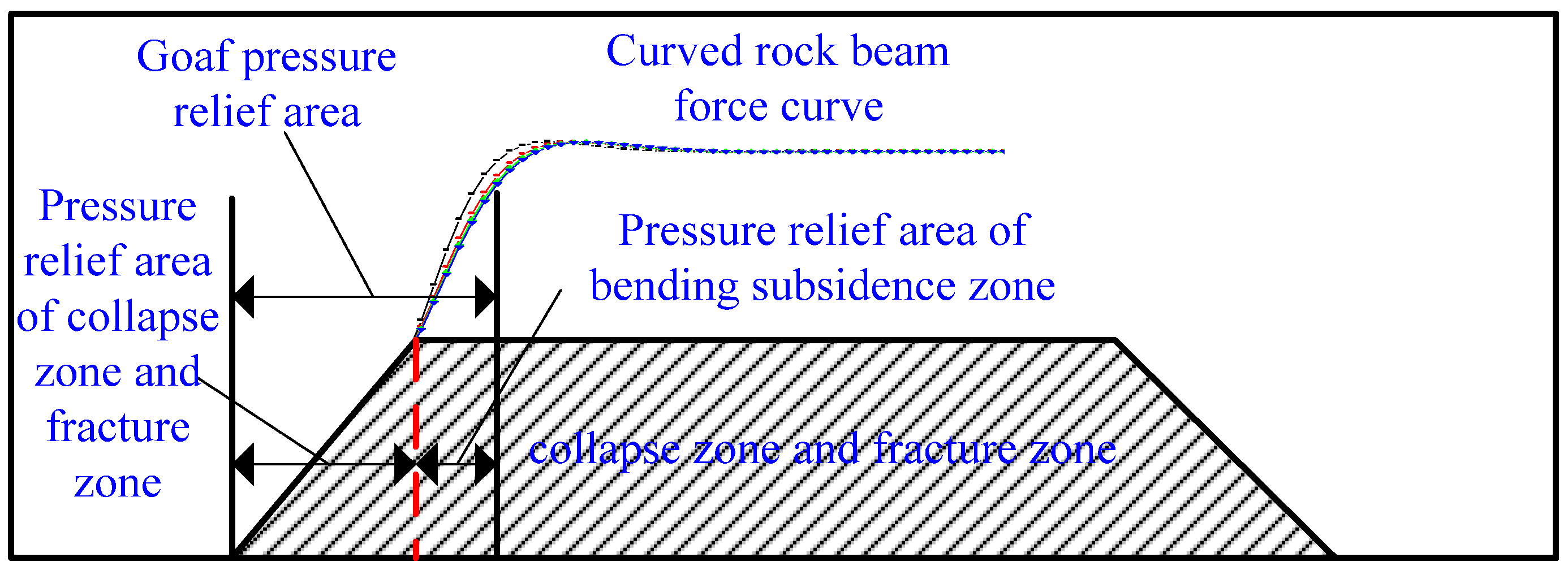
2.3.4. Determination of the Stress Distribution Law in the Goaf
- ①
- Determination of the pressure-relief area in the goaf
- ②
- Determination of the stress distribution law in the goaf
3. Results
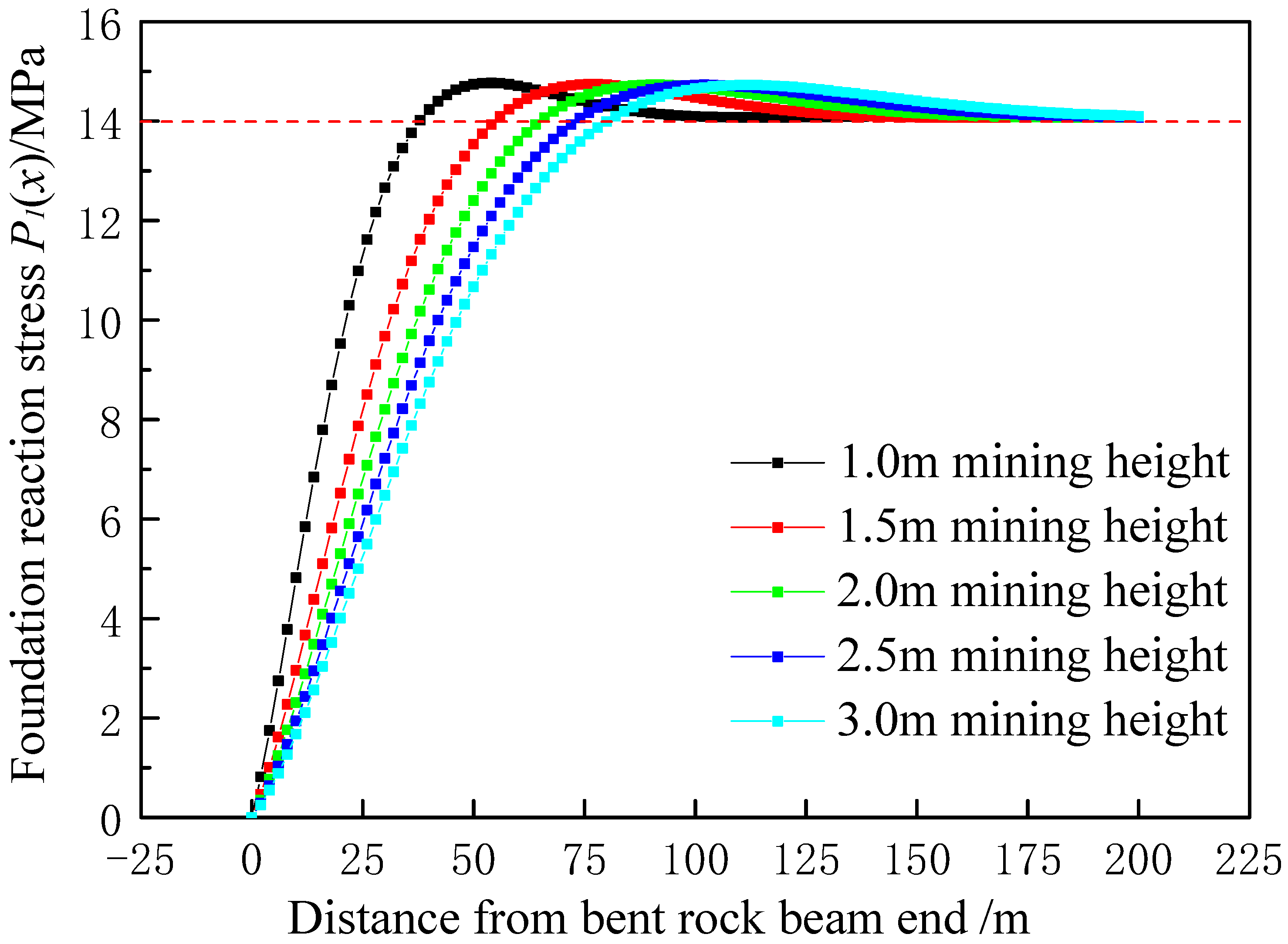
3.1. Calculation of Stresses at Different Levels of the Substrate
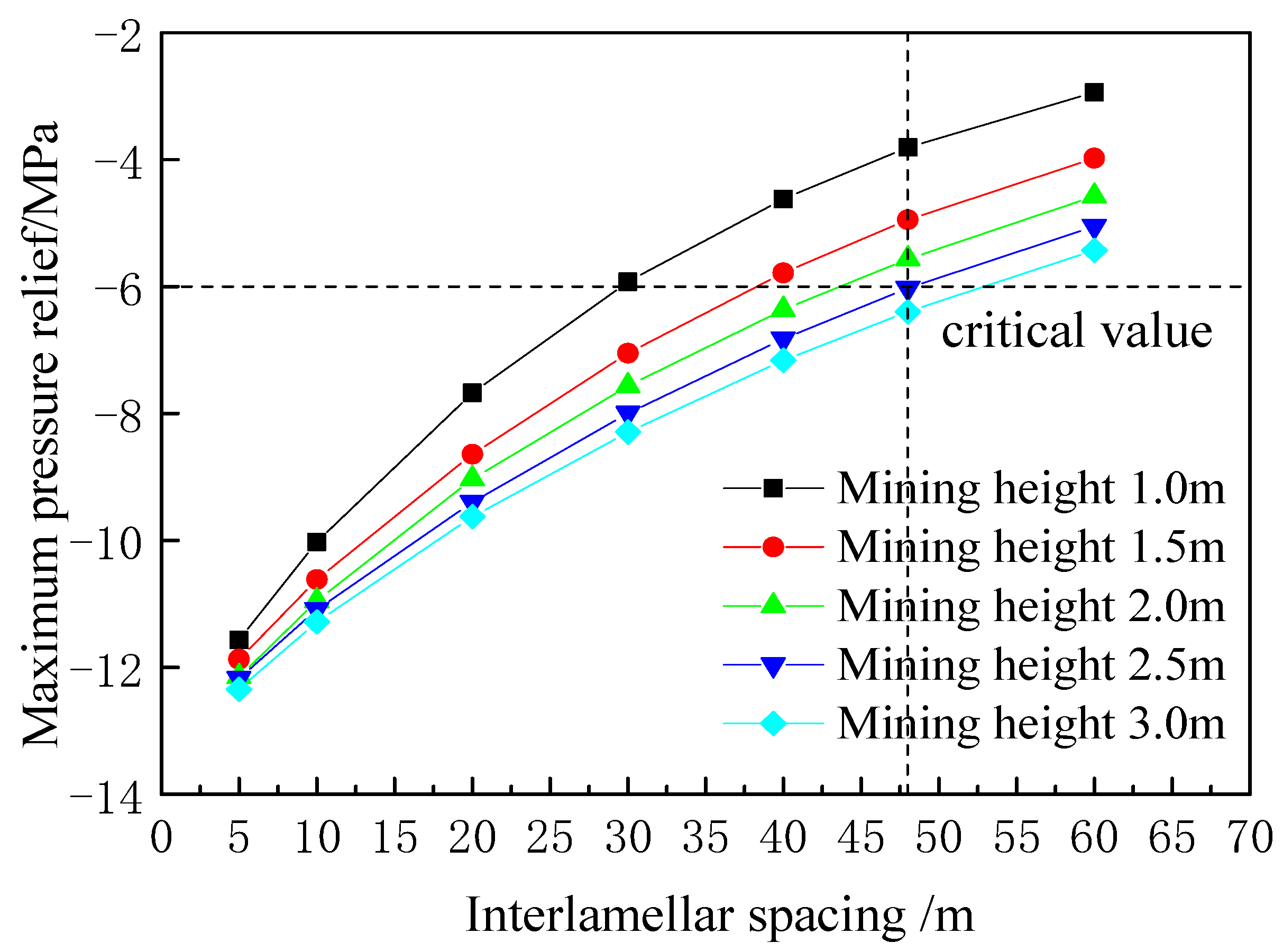
3.2. Determination of Minimum Layer Spacing
3.3. Verification of Calculation Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, M.J.; Fan, H.J.; Tian, J.J.; Lu, K.P. Study on outburst prevention effect of mining in long distance and ultra thin underneath protective seam. J. Coal Sci. Technol. 2017, 45, 67–72+83. [Google Scholar] [CrossRef]
- Jiang, F.X.; Liu, Y.; Liu, J.; Zhang, M.; Du, J.P.; Sun, W.S.; Zhang, W.P. Pressure-releasing mechanism of local protective layer in coal seam with rock burst. Chin. J. Geotech. Eng. 2019, 41, 368–375. [Google Scholar]
- Brandt, J.; Sdunowski, R. Gas drainage in high efficiency workings in German Coal mines. In Proceedings of the 2007 China (Huainan) International Symposium on Coal Gas Control Technology, Huainan, China, 25–27 October 2007; pp. 22–29. [Google Scholar]
- Ren, W.G.; Zhou, H.W.; Xue, D.J.; Wang, L.J.; Rong, T.L.; Liu, J.F. Mechanical behavior and permeability of coal and rock under strong mining disturbance in protected coal seam mining. J. China Coal Soc. 2019, 44, 1473–1481. [Google Scholar] [CrossRef]
- Wang, L.; Lu, Z.; Chen, D.P.; Liu, Q.Q.; Chu, P.; Shu, L.Y.; Barkat, U.; Wen, Z.J. Safe strategy for coal and gas outburst prevention in deep-and-thick coal seams using a soft rock protective layer mining. J. Saf. Sci. 2020, 129, 104800. [Google Scholar] [CrossRef]
- Pang, L.L.; Xu, X.F.; Si, L.; Zhang, H.; Li, Z.K. Analysis of prevention mechanism of upper protective seam mining on rock rockburst induced by thick conglomerate. Rock Soil Mech. 2016, 37, 120–128. [Google Scholar] [CrossRef]
- Tu, Q.Y.; Cheng, Y.P. Stress evolution and coal seam deformation through the mining of a remote upper protective layer. Energy Sources Part A Recovery Util. Environ. Eff. 2019, 41, 338–348. [Google Scholar] [CrossRef]
- Zhang, C.; Tu, S.; Zhang, L. Mining Thickness Determination of Upper and Lower Protective Coal Seam in Pressure Relief Mining. Geotech. Geol. Eng. 2019, 37, 1813–1827. [Google Scholar] [CrossRef]
- Zhang, M.; Shimada, H.; Sasaoka, T.; Matsui, K.; Dou, L.M. Evolution and effect of the stress concentration and rock failure in the deep multi-seam coal mining. Environ. Earth Sci. 2014, 72, 629–643. [Google Scholar] [CrossRef]
- Sun, Q.; Zhang, J.X.; Zhang, Q.; Yin, W.; Germain, D. A protective seam with nearly whole rock mining technology for controlling coal and gas outburst hazards: A case study. Nat. Hazards 2016, 84, 1793–1806. [Google Scholar] [CrossRef]
- Liu, Y.Z.; Zhang, Q.; Zhang, J.G.; Quan, K.; Wei, S.X. Theoretical research on protective seam mining in full rock stratum and engineering application. J. Min. Saf. Eng. 2018, 35, 146–154. [Google Scholar] [CrossRef]
- Cheng, X.; Zhao, G.M.; Li, Y.M.; Meng, X.R.; Liu, Z.H.; Ma, H.F.; Yuan, B.Q. Evolution of overburden mining-induced fractured zone and pressure-relief gas drainage in soft rock protective seam. J. Min. Saf. Eng. 2020, 37, 533–542. [Google Scholar] [CrossRef]
- Li, J.H.; Xu, Y.C.; Xie, X.F.; Yao, Y.L.; Gao, Y.B. Influence of mining height on coal seam floor failure depth. J. China Coal Soc. 2015, 40, 303–310. [Google Scholar] [CrossRef]
- Liang, B.; Shi, Z.S.; Jiang, F.L.; Ma, W. Research on protection result of extra-thin protective coal seam mining. China Saf. Sci. J. 2015, 25, 17–22. [Google Scholar] [CrossRef]
- Li, D.Q. Mining thin sub-layer as self-protective coal seam to reduce the danger of coal and gas outburst. Nat. Hazards 2014, 71, 41–52. [Google Scholar] [CrossRef]
- Wang, H.F.; Cheng, Y.P.; Yuan, L. Gas outburst disasters and the mining technology of key protective seam in coal seam group in the Huainan coalfield. Nat. Hazards 2013, 67, 763–782. [Google Scholar] [CrossRef]
- Cheng, X.; Zhao, G.M.; Li, Y.M.; Meng, X.R.; Tu, Q.Y. Key technologies and engineering practices for soft-rock protective seam mining. Int. J. Min. Sci. Technol. 2020, 67, 763–782. [Google Scholar] [CrossRef]
- State Administration of Coal Mine Safety. 2019 New Edition of Detailed Rules for Prevention and Control of Coal and Gas Outburst; Coal Industry Press: Beijing, China, 2019; pp. 43–45.
- Yu, B.F. Theoretical aspects of the protective coal seam. J. China Coal Soc. 1980, 4, 36–43. [Google Scholar]
- Liu, H.Y.; Cheng, Y.P.; Zhao, C.C.; Zhou, H.X.; Zhang, Z.P. Classification and Judgment Method of the Protective Layers. J. Min. Saf. Eng. 2010, 27, 468–474. [Google Scholar]
- Chen, Y.L.; Wu, H.S.; Zhang, M.W.; Wu, Y.; Zhang, H.Q.; Zhang, G.M. Research on the upper protective coal seam mining effect induced by coal thickness and interburden rock properties. J. Min. Saf. Eng. 2016, 33, 578–584. [Google Scholar] [CrossRef]
- Lu, S.Q.; Cheng, Y.P.; Wang, H.F.; Wang, L.; Li, W.; Mou, J.H. Numerical simulation research on the Hongling Coal Mine’s minimum mining thickness of upper protective layer. J. China Coal Soc. 2012, 37, 43–47. [Google Scholar] [CrossRef]
- Liu, H.B.; Cheng, Y.P.; Song, J.C.; Shang, Z.J. The change and distribution law of outburst coal seam permeability induced by drilling an extra-thin protective coal seam. J. China Coal Soc. 2010, 35, 411–416. [Google Scholar] [CrossRef]
- Hui, G.L.; Song, J.H. Parameter Calculation Analysis of the Coal Rock Dynamical Evolution of the Deep Protective Seam Mining. Saf. Coal Mines 2013, 44, 168–171. [Google Scholar] [CrossRef]
- Majkherchik, T.; Gajko, G.I.; Malkowski, P. Deformation process around a heading investigation when front of longwall face advancing. Ugol 2002, 11, 27–29. [Google Scholar]
- Dychkovskyi, R.; Shavarskyi, I.; Saik, P.; Lozynskyi, V.; Falshtynskyi, V.; Cabana, E. Research into stress-strain state of the rock mass condition in the process of the operation of double-unit longwalls. Min. Miner. Depos. 2020, 14, 85–94. [Google Scholar] [CrossRef]
- Slashchov, I.; Shevchenko, V.; Kurinnyi, V.; Slashchova, O.; Yalanskyi, O. Forecast of potentially dangerous rock pressure manifestations in the mine roadways by using information technology and radiometric control methods. Min. Miner. Depos. 2019, 13, 9–17. [Google Scholar] [CrossRef]
- Iordanov, I.; Buleha, I.; Bachurina, Y.; Boichenko, H.; Dovgal, V.; Kayun, O.; Kohtieva, O.; Podkopayev, Y. Experimental research on the haulage drifts stability in steeply dipping seams. Min. Miner. Depos. 2021, 15, 56–67. [Google Scholar] [CrossRef]
- Snihur, V.; Bondarenko, V.; Kovalevska, I.; Husiev, O.; Shaikhlislamova, I. Optimization solution substantiation for resource-saving maintenance of workings. Min. Miner. Depos. 2022, 16, 9–18. [Google Scholar] [CrossRef]
- Jin, J.; Qin, Z.; Lü, X.; Liu, T.; Zhang, G.; Shi, J.; Zuo, S.; Li, D. Rheology control of self-consolidating cement-tailings grout for the feasible use in coal gangue-filled backfill. Constr. Build. Mater. 2022, 316, 125836. [Google Scholar] [CrossRef]
- Wang, X.B.; Zhu, M.Z.; Cen, Z.H. Simulation on strata motion of S6–8 working face in Changcun coal mine with continuum-discontinuum method. J. Saf. Sci. Technol. 2020, 16, 82–87. [Google Scholar]
- Zhang, H.T.; Wen, Z.H.; Yao, B.H.; Chen, X.Q. Numerical simulation on stress evolution and deformation of overlying coal seam in lower protective layer mining. Alex. Eng. J. 2020, 59, 3623–3633. [Google Scholar] [CrossRef]
- Liu, S.J.; Lin, B.Q.; Gao, J.; Hao, Z.Y.; Li, Q.G.; Meng, J. Similar Simulation of Fracture Deformation in Overlying Coal and Rock in Far-Distance-Lower-Protective-Layer Mining. J. Min. Saf. Eng. 2011, 28, 51–55+60. [Google Scholar]
- Huang, Q.X.; Xia, X.G. Division of “four zones” in mining strata and surface movement. J. Min. Saf. Eng. 2016, 33, 393–397. [Google Scholar] [CrossRef]
- Shi, Z.S.; Liang, B.; Sun, W.J. Study on floor pressure-relief evolution characteristics at goaf stress adjustment stage. J. Min. Saf. Eng. 2019, 36, 51–58. [Google Scholar] [CrossRef]
- Chen, R.Z.; Tu, Q.Y.; Cheng, Y.P.; Liu, Q.F. Analysis of the fracture spatial-temporal development process of underlying coal (rock) through the mining of a close upper protective layer. J. Xi’an Univ. Sci Technol. 2018, 38, 71–78. [Google Scholar] [CrossRef]
- Long, Y.Q. Calculation of Beam on Elastic Foundation; People’s Education Press: Beijing, China, 1981; pp. 21–36. [Google Scholar]
- State Administration of Work Safety; State Administration of Coal Mine Safety; State Energy Administration; State Railway Administration. Code for Coal Pillar Reservation and Coal Pressure Mining of Buildings, Water Bodies, Railways and Main Shafts and Roadways; Coal Industry Press: Beijing, China, 2017; pp. 231–255.
- Pappas, D.M.; Mark, C. Behavior of Simulated Longwall Gob Material; United States Department of the Interior Bureau of Mines: Washington, DC, USA, 1993. [Google Scholar]
- Jin, Z.M.; Wei, J.P.; Jin, W.X. Distributive characterisic of front abutment pressure in top-coal caving face. J. Taiyuan Univ. Technol. 2001, 2, 216–218. [Google Scholar] [CrossRef]
- Wang, W.X. Cover Stress Re-Establishment and Its Permeability Evolution in Mining-Induced Fracture Rock Mass; China University of Mining and Technology: Xuzhou, China, 2014. [Google Scholar]
- Wu, R.L. Study on the Scope of the “Three Zones” of Gas Pressure Relief and Extraction in Coal Seam Group Mining; China University of Mining and Technology: Xuzhou, China, 2011. [Google Scholar]
- Zhang, C. Coupling Mechanism of Stress-Fracture-Flow in High Gas Coal Seam Group and Its Impact on Pressure Relief Extraction; China University of Mining and Technology: Xuzhou, China, 2017. [Google Scholar]




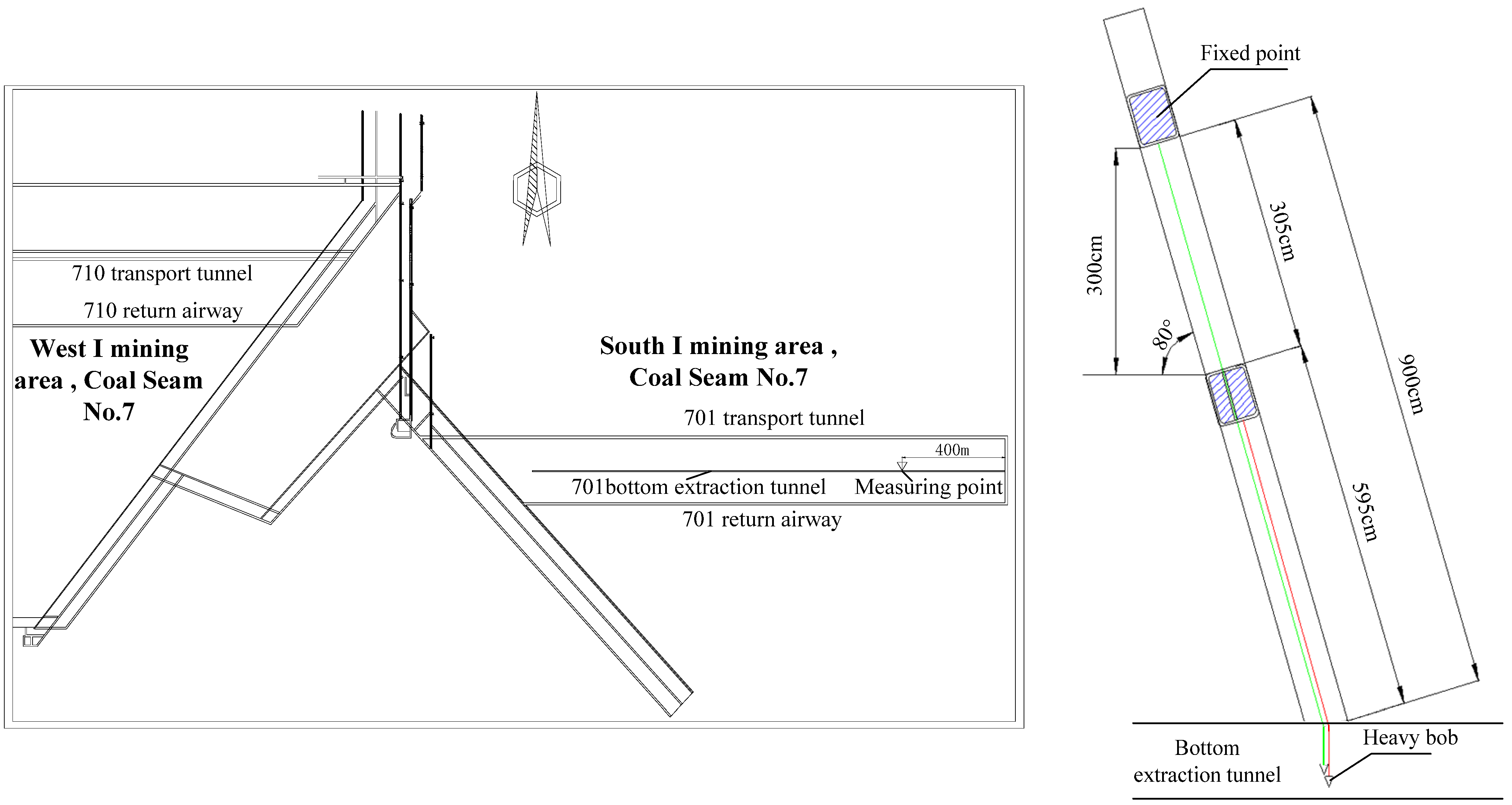
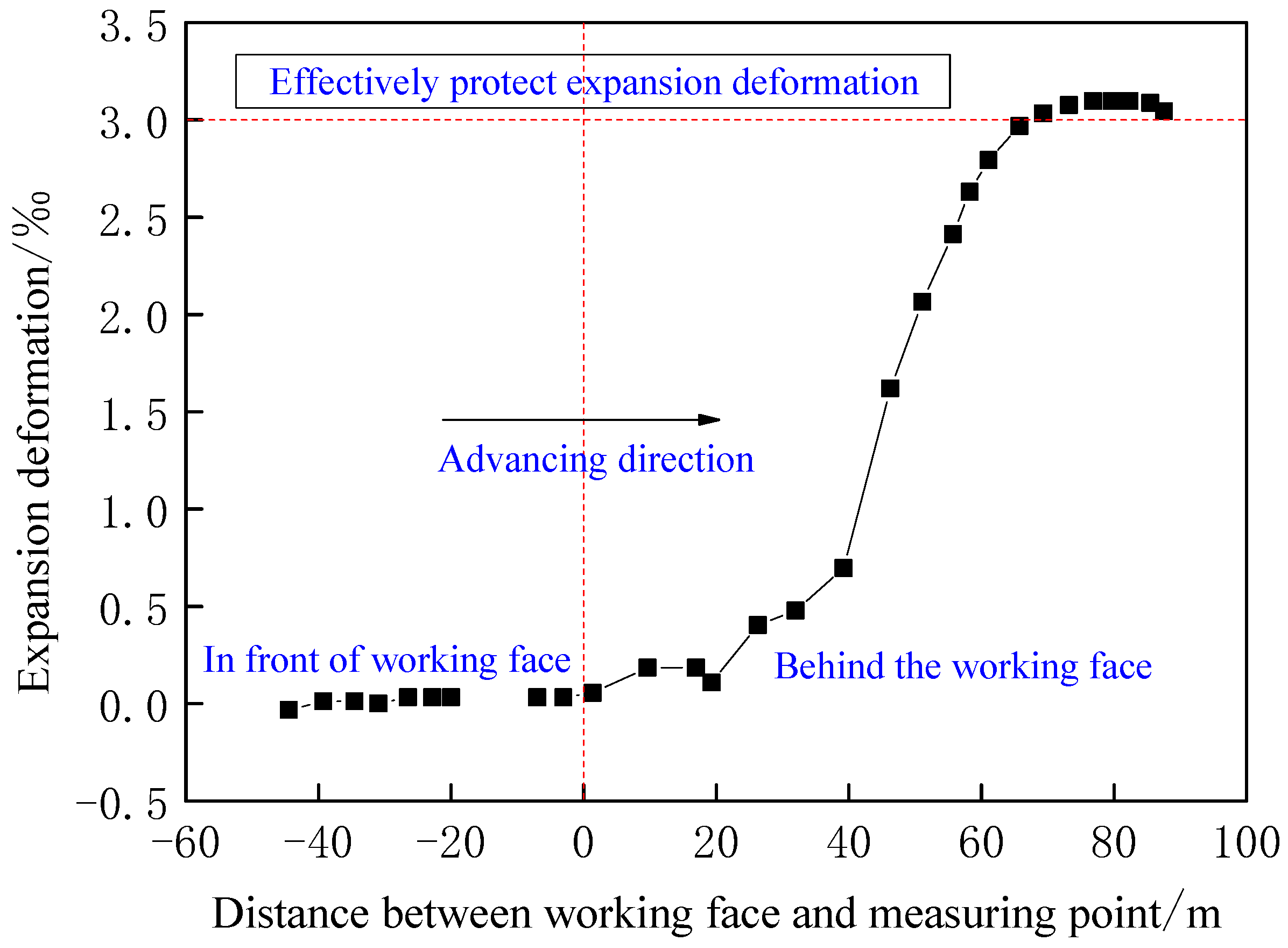
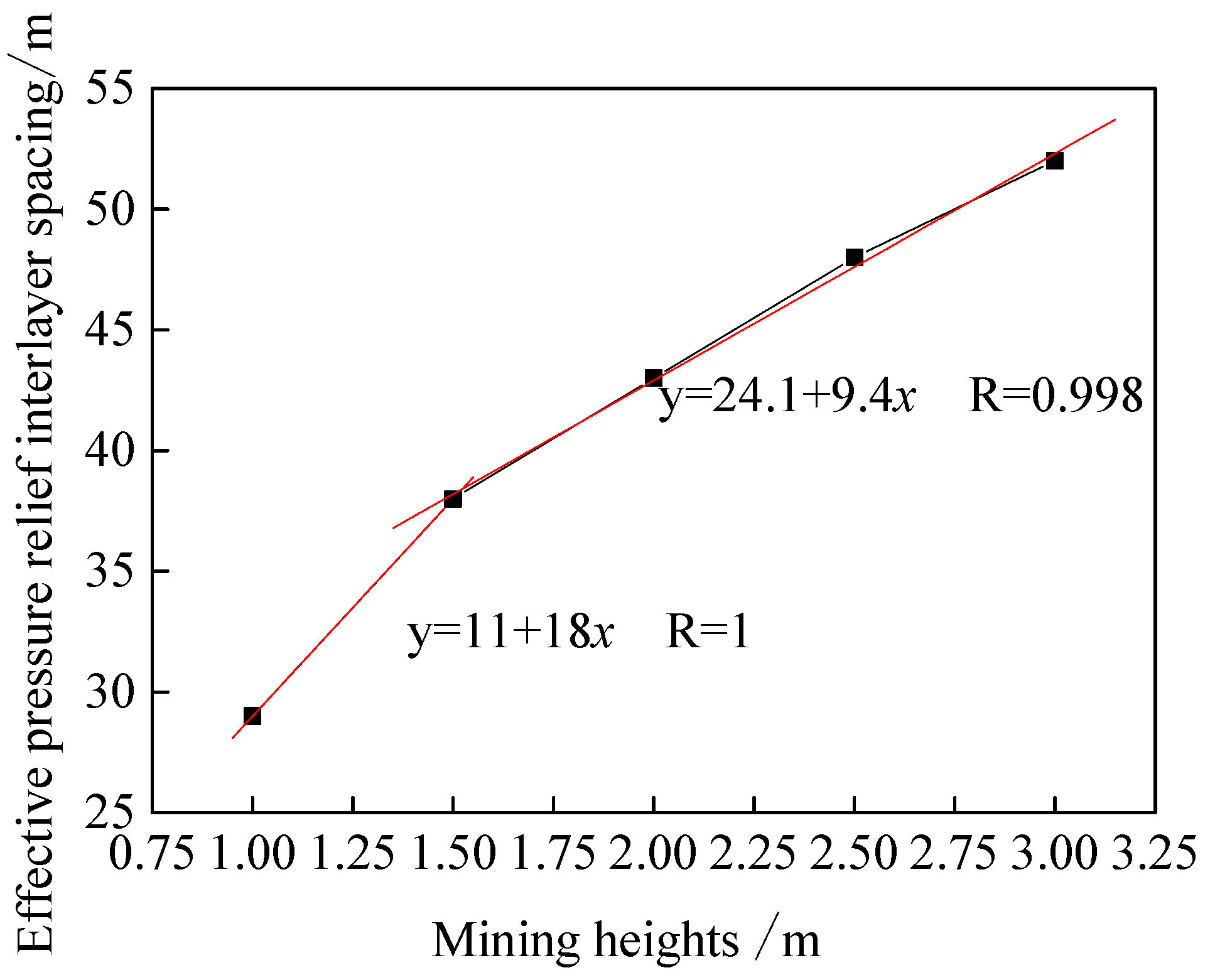
| No. | Lithology | Thickness of Each Layer/m | Compressive Strength/MPa | Tensile Strength/MPa | Modulus of Elasticity GPa | Depending on the Density/(g/cm3) | Poisson’s Ratio | |
|---|---|---|---|---|---|---|---|---|
| 18 | Medium sandstone | 3.8 | 33.38 | 3.7 | 33 | 2.73 | 0.29 | |
| 17 | Fine sandstone | 9.24 | 24.8 | 2.5 | 32 | 2.74 | 0.20 | Primary Key Layer |
| 16 | Medium sandstone | 8.92 | 24.8 | 3.7 | 33 | 2.74 | 0.23 | Sub-Key layer 2 |
| 15 | Mudstone | 3.67 | 12.87 | 1.7 | 20 | 2.72 | 0.3 | |
| 14 | Siltstone | 1.77 | 24.8 | 3.0 | 35.9 | 2.74 | 0.29 | |
| 13 | No.1 coal seam | 0.29 | 12.25 | 1.5 | 13 | 1.71 | 0.23 | |
| 12 | Fine sandstone | 0.74 | 24.8 | 2.5 | 32 | 2.74 | 0.3 | |
| 11 | Siltstone | 2.27 | 24.8 | 3.0 | 35.9 | 2.74 | 0.23 | |
| 10 | Medium sandstone | 5.9 | 24.8 | 3.7 | 33 | 2.74 | 0.29 | |
| 9 | Mudstone | 2.64 | 12.87 | 1.7 | 20 | 2.72 | 0.28 | |
| 8 | Claystone | 1.93 | 12.87 | 1.1 | 20 | 2.72 | 0.23 | |
| 7 | Mudstone | 4 | 12.87 | 1.7 | 20 | 2.72 | 0.25 | |
| 6 | Mudstone | 1.87 | 12.87 | 1.7 | 20 | 2.72 | 0.29 | |
| 5 | Fine sandstone | 2.92 | 23.99 | 2.5 | 32 | 2.57 | 0.23 | |
| 4 | Medium sandstone | 10.64 | 24.83 | 3.7 | 33 | 2.66 | 0.28 | |
| 3 | Mudstone | 0.62 | 14.87 | 1.7 | 20 | 2.72 | 0.3 | |
| 2 | No.3 coal seam | 1 | 12.25 | 1.5 | 13 | 1.71 | 0.36 | |
| 1 | Mudstone | 0.66 | 14.87 | 1.7 | 20 | 2.72 | 0.28 |
| Mining Height/m | Collapse Zone Rang H1/m | Rift Zone Extent H2/m | Rift Zone Height Hd/m |
|---|---|---|---|
| 1 | 6.4 | 18.4 | 24.8 |
| 1.5 | 8.0 | 22.6 | 30.6 |
| 2 | 9.2 | 25.8 | 35.0 |
| 2.5 | 10.3 | 28.2 | 38.5 |
| 3 | 11.3 | 30 | 41.3 |
| Mining Height/m | Crushing and Swelling Factor B |
|---|---|
| 1 | 1.156 |
| 1.5 | 1.188 |
| 2 | 1.217 |
| 2.5 | 1.243 |
| 3 | 1.265 |
| Mining Height/m | ki (Collapse Zone Foundation Factor)/(GPa/m3) | kj (Unbroken Rock Foundation Factor)/(GPa/m3) | q0 Load/MPa |
|---|---|---|---|
| 1 | 0.0587 | 4.29 | 14.1 |
| 1.5 | 0.0158 | 4.29 | 14.1 |
| 2 | 0.00851 | 4.29 | 14.1 |
| 2.5 | 0.00554 | 4.29 | 14.1 |
| 3 | 0.00392 | 4.29 | 14.1 |
| Mining Height/m | Collapse Zone Fissure Zone Pressure Relief Area/m | Bending Down with Pressure Relief Area/m | Pressure Relief Area in the Extraction Zone/m |
|---|---|---|---|
| 1 | 14 | 36 | 50 |
| 1.5 | 18 | 54 | 72 |
| 2 | 20 | 64 | 84 |
| 2.5 | 22 | 72 | 94 |
| 3 | 24 | 78 | 102 |
| Mining Height/m | K | ② Range/m | ③ Range/m | ④ Rang/m |
|---|---|---|---|---|
| 1 | 2.82 | 24.1 | 5.2 | 50 |
| 1.5 | 2.52 | 34.1 | 6.9 | 72 |
| 2 | 2.2 | 42.2 | 7.9 | 84 |
| 2.5 | 2.04 | 50.5 | 8.9 | 94 |
| 3 | 1.88 | 57.6 | 9.5 | 102 |
| Layer Spacing/m | E/GPa | /kN/m3 | H/m | Residual Critical Stress Value after Effective Protection Unloading/MPa | Unloading Pressure Threshold Values | ||
|---|---|---|---|---|---|---|---|
| 48 | 2 | 25 | 800 | 0.28 | 1 | 14.026 | 5.974 |
| 10 | 2 | 25 | 762 | 0.28 | 1 | 13.075 | 5.975 |
| 20 | 2 | 25 | 772 | 0.28 | 1 | 13.325 | 5.975 |
| 30 | 2 | 25 | 782 | 0.28 | 1 | 13.576 | 5.974 |
| 40 | 2 | 25 | 792 | 0.28 | 1 | 13.826 | 5.974 |
| 50 | 2 | 25 | 802 | 0.28 | 1 | 14.076 | 5.974 |
| 60 | 2 | 25 | 812 | 0.28 | 1 | 14.327 | 5.973 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Z.; Ye, D.; Qin, B.; Hao, J.; Sun, W.; Fang, S. Mining Height Effect and Application of Upper Protected Layer Mining Pressure Relief. Sustainability 2022, 14, 10119. https://doi.org/10.3390/su141610119
Shi Z, Ye D, Qin B, Hao J, Sun W, Fang S. Mining Height Effect and Application of Upper Protected Layer Mining Pressure Relief. Sustainability. 2022; 14(16):10119. https://doi.org/10.3390/su141610119
Chicago/Turabian StyleShi, Zhanshan, Donglin Ye, Bing Qin, Jianfeng Hao, Weiji Sun, and Shengjie Fang. 2022. "Mining Height Effect and Application of Upper Protected Layer Mining Pressure Relief" Sustainability 14, no. 16: 10119. https://doi.org/10.3390/su141610119
APA StyleShi, Z., Ye, D., Qin, B., Hao, J., Sun, W., & Fang, S. (2022). Mining Height Effect and Application of Upper Protected Layer Mining Pressure Relief. Sustainability, 14(16), 10119. https://doi.org/10.3390/su141610119






