Administrative Costs and Tariff Rates in the Presence of Customs Evasion: Evidence from Ecuador
Abstract
1. Introduction
- (a)
- The creation of some trade policy-governing entities and suppression of other to provide a greater institutional framework for changing the production structure. Among the most important elements are the creation of the Ministry of Foreign Trade and Investment and the Foreign Trade Committee, the entity responsible for approving public policies on trade;
- (b)
- The public investment plan was ambitious and grew considerably from 7.5% of GDP in 2007 to 17% of GDP in 2014, decelerating to 10.6% of GDP in 2017 due to an economic recession driven by the weakening of the international price of oil and the appreciation of the US dollar, which is the legal tender in Ecuador. The government prioritized public investment in production and the strategic sectors identified as priorities for changing the productive matrix. Other sectors targeted for investment were social and human development, security and justice, economic policy and patrimony;
- (c)
- Between 2007 and 2017, the simple average applied most favored nation1 (MFN) tariff went from 11.7% to 12.2% (all products), from 14.6% to 18.3% (agricultural) and from 11.3% to 11.2% (non-agricultural) (World Tariff Profiles 2008, 2018). Among the products with the highest average tariff between 2007 and 2017 are dairy products (18.9% to 32.8%), sugar and confectionery (14.5% to 12.7%), animals and their products (17.1% to 28.2%), and fish and their products (19% to 25.4%). Likewise, the clothing sector experiences tariff increases from 20% to 22.7%. The sector of textiles (17.5% to 18.1%), leather and footwear (12.9% to 13.6%), electrical machinery (10.1% to 10.6%), manufacturing (13.3% to 15.8%) and transportation material (10.5% to 12.1%) continue with the same protectionist pace. Additionally, tariffs suffer an additional increase in certain years, the most representative being 2009, 2013, 2014 and 2015;
- (d)
- In 2009, the country began applying compounds duties to different products such as clothing, footwear, alcoholic beverages, motor vehicles, etc.; it affected to five percent of harmonized system (HS) subheadings;
- (e)
- In 2009, 2015 and 2016, the government resorted to the implementation of a safeguard measure to compensate the balance of payments. The measure applied as a tariff surcharge to approximately 38% of the corresponding tariff subheadings. The level of the tariff surcharge varied between 5% and 45%, with the 25% and 45% surcharges levied on 58.6% of the universe of products subject to the safeguard measure;
- (f)
- For the period 2011–2018, the applied MFN tariff had already exceeded the bound tariff by 28% of the total set of tariff lines, according to Ecuador’s Trade Policy Review Report;
- (g)
- Within the period 2007 and 2017, some reforms introduced in relation to control requirements prior to import; for example, the requirement for inspection at origin eliminated. On the other hand, a series of measures and requirements applied to allow the import of certain products such as cotton, clothing, fruits and vegetables, among others. These measures include the use of an importer registry, certificate of recognition, automatic and non-automatic import licenses, certificates of recognition for products subject to technical regulations, as well as import quotas for motor vehicles and cell phones;
- (h)
- The customs administration chose to prioritize a risk management system in order to reduce physical inspections in dispatch, as well as to strengthen audits in a post-clearance phase.
2. Related Literature
3. Methodology and Data
4. Results and Discussion
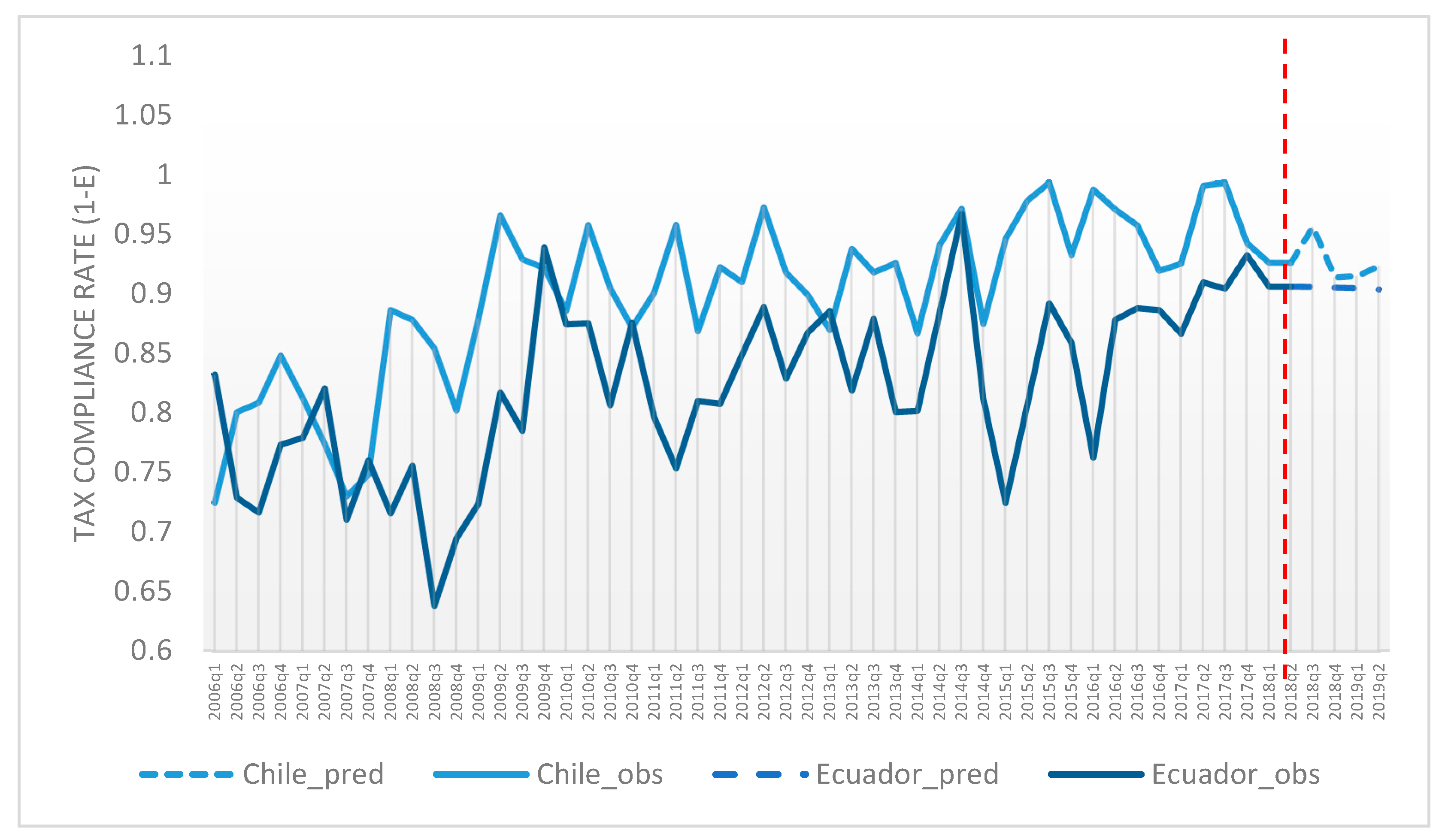
4.1. Ecuador Case Study
0.05480Iao2014q3 − 0.06221Iao2008q3 + 0.06142Iao2009q4 − 0.05475Iao2015q1 − 0.04908Iao2007q3 − 0.04198Iao2011q2 −
0.03826Iao2013q2 − 0.03504Iao2008q1 + (1/1 − 0.71136 L)at
4.2. Chile Case Study
0.04843Iao2006q1 + 0.03928Iao2006q4 − 0.03680Iao2007q3 − 0.02938Iao2010q4 + (1/1 − 0.64758L4) at
0.06532Iao2007q4 + 0.03765Iao2009q4 − 0.06216Iao2011q2 − 0.05936 I ao2013q2 − 0.03896Iao2015q1 + (1 + 0.62297L4) at
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Agha, Ali, and Jonathan Haughton. 1996. Designing Vat Systems: Some Efficiency Considerations. The Review of Economics and Statistics 2: 303–8. [Google Scholar] [CrossRef]
- Allingham, Michael G., and Agnar Sandmo. 1972. Income Tax Evasion: A Theoretical. Analysis Journal of Public Economics 1: 323–80. [Google Scholar] [CrossRef]
- Alm, James, Betty Jackson, and Michael McKee. 1992. Institutional Uncertainty and Taxpayer Compliance. American Economic Review 82: 1018–26. [Google Scholar]
- Arrow, Kenneth J. 1965. Essays in the Theory of Risk-Bearing. Helsinki: Yrjö Jahnsson Lectures. [Google Scholar]
- Baldry, Jonathan C. 1987. Income Tax Evasion and the Tax Schedule: Some Experimental Results. Public Finance 42: 357–83. [Google Scholar]
- Barone, Guglielmo, and Sauro Mocetti. 2009. Tax Morale and Public Spending Inefficiency. In Bank of Italy Temi di Discussione. (Working Paper) (732). Roma: Bank of Italy, Economic Research Unit, Bologna Branch. [Google Scholar] [CrossRef]
- Becker, Gary S. 1968. Crime and Punishment: An Economic Approach. Journal of Political Economy 76: 169–217. [Google Scholar] [CrossRef]
- Bhagwati, Jagdish N. 1964. On the Underinvoicing of Imports. Bulletin of the Oxford University Institute of Economics and Statistics 26: 389–97. [Google Scholar]
- Box, George E. P., Gwilym M. Jenkins, Gregory C. Reinsel, and Greta M. Ljung. 2016. Transfer Function and Multivariate Model Building, 5th ed. Time Series Analysis: Forecasting and Control; Hoboken: John Wiley & Sons, Inc., pp. 395–502. [Google Scholar]
- Central Bank of Chile. 2018a. Statistical Database, Exchange Rate. Available online: https://si3.bcentral.cl/siete/en (accessed on 15 October 2018).
- Central Bank of Chile. 2018b. Statistical Database, Foreign Trade of Goods. Available online: https://www.bcentral.cl/en/areas/statistics/external-sector/foreign-trade-of-goods (accessed on 15 October 2018).
- Central Bank of Ecuador. 2018a. Macroeconomic Statistics, Structural Presentation; Quito: Ecuadorian Central Bank. Available online: https://www.bce.fin.ec/index.php/estadisticas-economicas (accessed on 8 November 2018).
- Central Bank of Ecuador. 2018b. Quarterly Balance of Payments Bulletin No 64 and Monthly Statistical Information No. 1847, 1930 and 2000; Quito: Ecuadorian Central Bank. Available online: https://www.bce.fin.ec/index.php/component/k2/item/297-bolet%C3%ADn-trimestral and https://contenido.bce.fin.ec/home1/estadisticas/bolmensual/IEMensual.jsp (accessed on 18 October 2018).
- Central Bank of Ecuador. 2018c. Foreign Trade Statistics Database. Available online: https://www.bce.fin.ec/index.php/comercio-exterior (accessed on 15 October 2018).
- Central Bank of Ecuador. 2018d. Quarterly National Accounts Bulletin. Available online: https://contenido.bce.fin.ec/home1/estadisticas/cntrimestral/CNTrimestral.jsp (accessed on 15 October 2018).
- Centre for Tax Policy and Administration. 2010. Tax Administration in OECD and Selected Non-OECD Countries: Comparative Information Series. Paris: OECD Publishing, vol. 4, p. 119. [Google Scholar]
- Chen, Chung, Lon-Mu Liu, and Gregory B. Hudak. 1990. Outliers Detection and Adjustment in Time Series Modeling and Forecasting. Working Papers and Reprint Series; DeKalb: Scientific Computing Associates. [Google Scholar]
- Clotfelter, Charles T. 1983. Tax Evasion and Tax Rates: An Analysis of Individual Returns. The Review of Economics and Statistics 65: 273–363. [Google Scholar] [CrossRef]
- Crane, Steven E., and Farrokh Nourzad. 1986. Inflation and Tax Evasion: An Empirical Analysis. The Review of Economics and Statistics 68: 217–23. [Google Scholar] [CrossRef]
- De La Torre, C. 2017. Ecuador Pierde 700 Millones Anuales por Contrabando, Dice Carlos De la Torre, Ministro de Finanzas del Ecuador. Available online: https://www.elcomercio.com/actualidad/ecuador-pierde-contrabando-ministro-finanzas.html (accessed on 4 December 2018).
- DIPRES. 2018. Directorate of Budget, Republic of Chile, Dipres. Available online: http://www.dipres.gob.cl/598/w3-propertyvalue-2129.html (accessed on 15 October 2018).
- Doob, Anthony N., and Cheryl Marie Webster. 2003. Sentence Severity and Crime: Accepting the Null Hypothesis. Crime and Justice 30: 143–95. [Google Scholar] [CrossRef]
- Dubin, Jeffrey A. 2007. Criminal Investigation Enforcement Activities and Taxpayer Noncompliance. Public Finance Review 35: 500–29. [Google Scholar] [CrossRef]
- Dubin, Jeffrey A., and Louis L. Wilde. 1988. An Empirical Analysis of Federal Income Tax Auditing and Compliance. National Tax Journal 41: 61–74. [Google Scholar]
- Dubin, Jeffrey A., Michael J. Graetz, and Louis L. Wilde. 1990. The Effects of Audit Rates on the Federal Income Tax 1977–1986. National Tax Journal 43: 395–409. [Google Scholar]
- Engel, Eduardo Goetz, Alexander Galetovic Potsch, and Claudio E. Raddatz. 2001. A Note on Enforcement Spending and VAT Revenue. The Review of Economics and Statistics 83: 384–87. [Google Scholar] [CrossRef]
- Evans, Chris, Shirley Carlon, and Darren Massey. 2005. Record Keeping Practices and Tax Compliance of SME’s. eJournal of Tax Research 3: 288–334. [Google Scholar]
- Fisman, Raymond, and Shang-Jin Wei. 2877. Tax rates and Tax Evasion: Evidence from “Missing Imports” in China. National Bureau of Economic Research (NBER) Working Paper: w8551. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=287748 (accessed on 22 December 2017).
- FLACSO. 2015. FLACSO—Latin American Faculty of Social Sciences, Ecuador. Revista Perfil Criminológico (15). Available online: https://repositorio.flacsoandes.edu.ec/xmlui/bitstream/handle/10469/7512/BFLACSO-PC15.pdf?sequence=2&isAllowed=y (accessed on 15 October 2019).
- Friedland, Nehemiah, Shlomo Maital, and Aryeh Rutenberg. 1978. A Simulation Study of Income Tax Evasion. Journal of Public Economics 10: 107–16. [Google Scholar] [CrossRef]
- Javorcik, Beate S., and Gaia Narciso. 2008. Diferentiated products and evasion of import tariffs. Journal of International Economics 76: 208–22. [Google Scholar] [CrossRef]
- National Customs Service of Ecuador, Senae. 2018. Open data Portal for transparency. Available online: https://www.aduana.gob.ec/transparencia/ and https://www.aduana.gob.ec/de-interes/recaudaciones/ (accessed on 15 October 2018).
- Park, Chang-Gyun, and Jin Kwon Hyun. 2003. Examining the determinants of tax compliance by experimental data: A case of Korea. Journal of Policy Modeling 25: 673–84. [Google Scholar] [CrossRef]
- Pérez, Cesar. 2011. Series Temporales: Técnicas y Herramientas. Madrid: Ibergarceta Publicaciones, S.L. [Google Scholar]
- Pommerehne, Werner W., and Hannelore Weck-Hannemann. 1996. Tax Rates, Tax Administration and Income Tax Evasion in Switzerland. Public Choice 88: 161–70. [Google Scholar] [CrossRef]
- Poterba, James M. 1987. Tax Evasion and Capital Gains Taxation. American Economic Review 77: 234–39. [Google Scholar]
- Resolution No. SENAE-2017-001-RE-M. 2017. First Supplement to the Official Registry 115 of 8 November 2017. Quito: National Customs Service of Ecuador. Available online: https://www.aduana.gob.ec/wp-content/uploads/2017/11/SENAE-SENAE-2017-001-RE-M-ESTABLECER-LA-TASA-DE-SERVICIO-DE-CONTROL-ADUANERO.pdf (accessed on 4 December 2018).
- Sancak, Cemile, Ricardo Velloso, and Jing Xing. 2010. Tax Revenue Response to the Business Cycle. Fondo Monetario Internacional. Working Paper No. WP/10/71. Available online: https://www.imf.org/en/Publications (accessed on 15 November 2018).
- National Customs Service of Ecuador (SENAE). 2017. Exportadores Cuestionan Tasa de Control Aduanero. Available online: https://www.eltelegrafo.com.ec/noticias/economia/8/exportadores-cuestionan-tasa-de-control-aduanero (accessed on 17 November 2017).
- Slemrod, Joel. 1985. An Empirical Test for Tax Evasion. The Review of Economics and Statistics 67: 232–38. [Google Scholar] [CrossRef]
- Slemrod, Joel, and Shlomo Yitzhaki. 2002. Tax avoidance, evasion, and administration. Handbook of Public Economics. In Handbook of Public Economics, 1st ed. Amsterdam: Elsevie, vol. 3, chp. 22. pp. 1423–70. [Google Scholar]
- Stiglitz, Joseph E. 1969. A Re-examination of the Modigliani-Miller Theorem. The American Economic Review 59: 784–93. [Google Scholar]
- Tariff Profiles in the World. n.d. Tariff Profiles in the World Is a Co-Publication of the WTO, ITC and UNCTAD on Market Access for Goods (2007 to 2018). Available online: https://www.wto.org/english/res_e/reser_e/tariff_profiles_e.htm (accessed on 15 October 2019).
- Tauchen, Helen V., Ann Dryden Witte, and Kurt J. Beron. 1993. Tax Compliance: An Investigation Using Individual Taxpayer Compliance Measurement Program (TCMP) Data. Journal of Quantitative Criminology 9: 177–202. [Google Scholar] [CrossRef]
- Trade Map. 2018. Trade Map of the International Trade Centre, ITC. The Tool is Freely Accessible. Available online: www.trademap.org (accessed on 15 October 2018).
- Witte, Ann D., and Diane F. Woodbury. 1985. The effect of tax laws and tax administration on tax compliance: The case of the U.S. individual income tax. National Tax Journal 38: 1–13. [Google Scholar]
- World Tariff Profiles. 2008. Geneva: World Trade Organization (WTO), International Trade Centre (ITC) and e United Nations Conference on Trade and Development (UNCTAD). Available online: https://www.wto.org/english/res_e/statis_e/its2008_e/its08_toc_e.htm (accessed on 15 October 2019).
- World Tariff Profiles. 2018. Geneva: World Trade Organization (WTO), International Trade Centre (ITC) and e United Nations Conference on Trade and Development (UNCTAD). Available online: https://www.wto.org/english/res_e/reser_e/tariff_profiles_e.htm (accessed on 15 October 2019).
- World Trade Organization. 2020. Annual Report by General Director. Available online: https://www.wto.org/english/news_e/news20_e/trdev_11dec20_e.htm (accessed on 14 December 2020).
- Yitzhaki, Shlomo. 1974. A note of income tax evasion: A theorical analysis. Journal of Public Economics 3: 202–2. [Google Scholar] [CrossRef]
| 1 | The simple average of MFN applied duties is the simple average of most favored nation (MFN) applied duties. The “most favored nation” are the highest (most restrictive) tariffs that members of the WTO charge one another, unless the country is part of a preferential trade agreement. |
| 2 | The data for mirror imports are provisional; some countries may take several months to report their trade data to the International Trade Center, known as ITC. For more information, please refer to the following link https://www.trademap.org/stDataAvailability.aspx?nvpm=3|152|||||||2|1|1|1|2|2|2|1|1 (accessed on 15 October 2018). Similar situation is presented with the registration of imports from Ecuador, which could vary by the time of registration in which the nationalization of the good has occurred that can be after the date of disclosure of the Quarterly Bulletin of Balance of Payment, so there could be reprocessing of information with the presentation of a new bulletin quarterly. In the case of the information obtained from the ECB (imports) and the ITC (mirror imports), the cutoff date was 5 February 2019. |





| Variable | Arithmetic Mean | Standard Deviation | Extreme Values | |||||
|---|---|---|---|---|---|---|---|---|
| Minimum | Maximum | |||||||
| Chile | Ecuador | Chile | Ecuador | Chile | Ecuador | Chile | Ecuador | |
| Voluntary compliance rate (1 − e) | 0.9 | 0.81 | 0.068 | 0.077 | 0.73 | 0.63 | 0.99 | 0.97 |
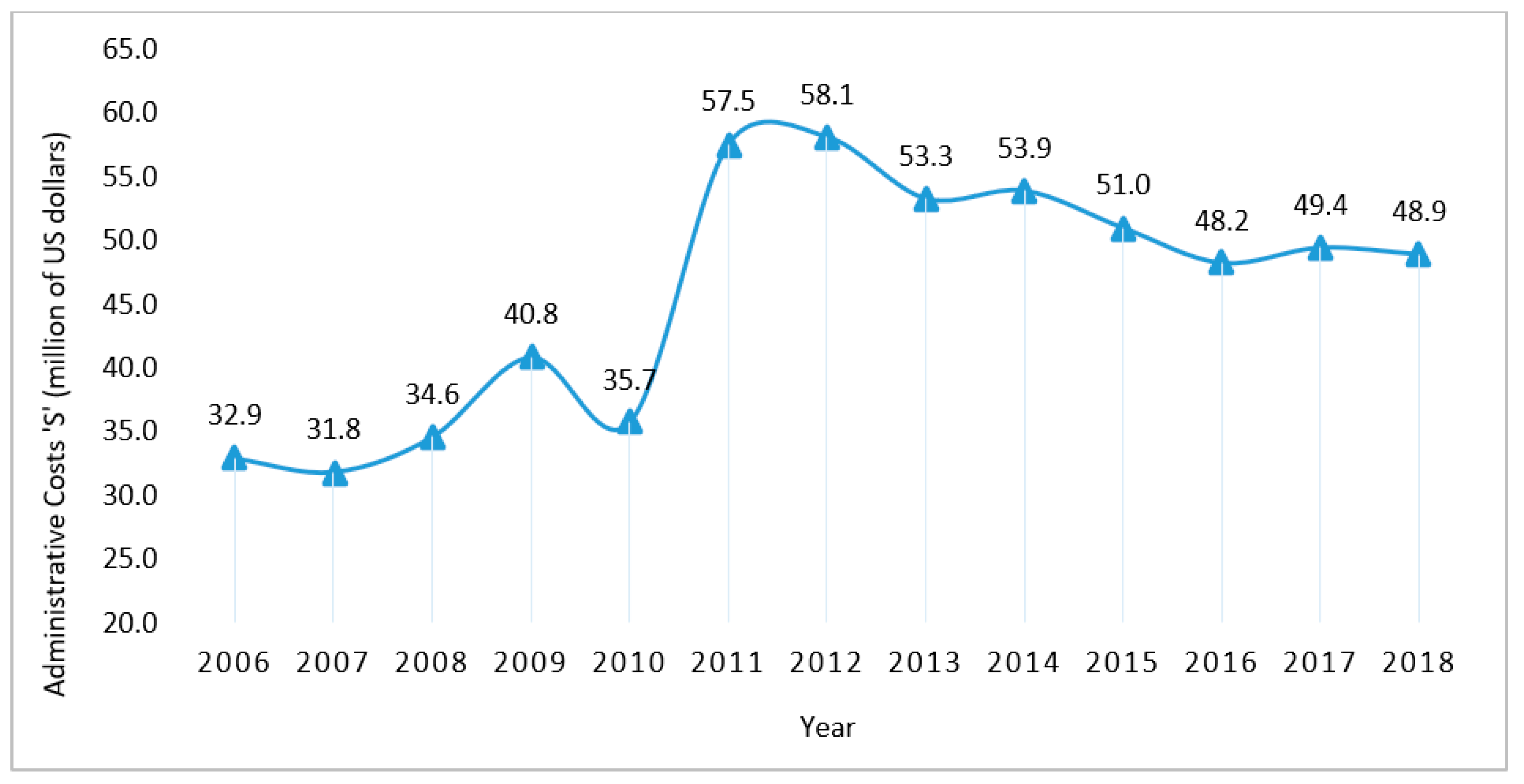
| Administrative cost for each US dollar collected in customs (S/R) | 0.0083 | 0.0144 | 0.0019 | 0.0043 | 0.0051 | 0.0061 | 0.0126 | 0.0263 |
| Simple average of applied tariffs, all products (τ) (%) | 5.98 | 11.61 | 0.0047 | 0.8872 | 5.98 | 10.1 | 5.99 | 13.47 |
| Variation in economic cycle (PIB/PIB−1) | 1.007 | 1.008 | 0.0678 | 0.0104 | 0.859 | 0.984 | 1.158 | 1.032 |
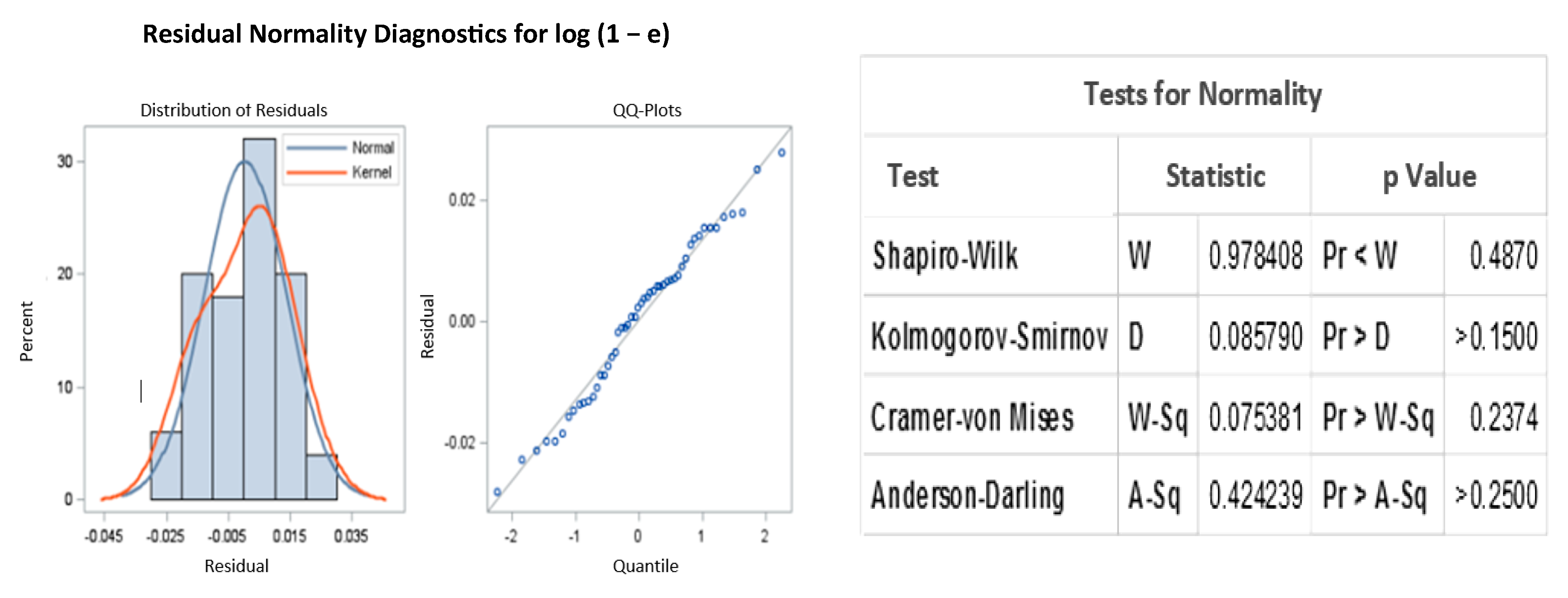
| Autocorrelation Check Residuals | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| To Lag | Chi-Squared | DF | PR > ChiSq | Autocorrelations | |||||
| 6 | 2.23 | 5 | 0.8165 | 0.091 | −0.109 | 0.055 | −0.062 | −0.112 | −0.027 |
| 12 | 10.65 | 11 | 0.4732 | −0.140 | 0.088 | 0.235 | 0.216 | 0.046 | −0.036 |
| 18 | 14.36 | 17 | 0.6413 | −0.055 | 0.108 | −0.135 | −0.077 | −0.010 | −0.102 |
| 24 | 15.75 | 23 | 0.8656 | −0.018 | 0.072 | −0.049 | 0.083 | −0.025 | 0.001 |
| Conditional Least Squares Estimation | |||||||
|---|---|---|---|---|---|---|---|
| Parameter | Estimate | Standard Error | t Value | Approx. Pr > |t| | Lag | Variable | Shift |
| AR1,1 | 0.71136 | 0.11627 | 6.12 | <0.0001 | 1 | log_1_e | 0 |
| NUM1 | −0.03723 | 0.02306 | −1.61 | 0.1149 | 0 | log_S_R | 0 |
| NUM2 | −0.15756 | 0.04258 | −3.70 | 0.0007 | 0 | log τ | 0 |
| NUM3 | 2.26551 | 0.80211 | 2.82 | 0.0076 | 0 | log_pib | 0 |
| NUM4 | 0.04907 | 0.01820 | 2.70 | 0.0105 | 0 | ls2016q2 | 0 |
| NUM5 | 0.05480 | 0.01804 | 3.04 | 0.0044 | 0 | ao2014q3 | 0 |
| NUM6 | −0.06221 | 0.01802 | −3.45 | 0.0014 | 0 | ao2008q3 | 0 |
| NUM7 | 0.06142 | 0.01828 | 3.36 | 0.0018 | 0 | ao2009q4 | 0 |
| NUM8 | −0.05475 | 0.01819 | −3.01 | 0.0047 | 0 | ao2015q1 | 0 |
| NUM9 | −0.04908 | 0.01807 | −2.72 | 0.0100 | 0 | ao2007q3 | 0 |
| NUM10 | −0.04198 | 0.01854 | −2.26 | 0.0295 | 0 | ao2011q2 | 0 |
| NUM11 | −0.03826 | 0.01812 | −2.11 | 0.0415 | 0 | ao2013q2 | 0 |
| NUM12 | −0.03504 | 0.01847 | −1.90 | 0.0656 | 0 | ao2008q1 | 0 |
| Autocorrelation Check Residuals | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| To Lag | Chi-Squared | DF | PR > ChiSq | Autocorrelations | |||||
| 6 | 4.27 | 5 | 0.5115 | 0.203 | 0.066 | −0.176 | −0.016 | −0.014 | 0.042 |
| 12 | 12.81 | 11 | 0.3056 | 0.102 | 0.020 | −0.136 | −0.235 | −0.184 | −0.112 |
| 18 | 16.99 | 17 | 0.4552 | 0.063 | −0.017 | 0.092 | 0.041 | 0.034 | −0.193 |
| 24 | 24.57 | 23 | 0.3729 | 0.011 | 0.212 | 0.196 | 0.043 | 0.000 | 0.028 |
| Conditional Least Square Estimation | |||||||
|---|---|---|---|---|---|---|---|
| Parameter | Estimate | Standard Error | t Value | Approx. Pr >|t| | Lag | Variable | Shift |
| AR1,1 | 0.64758 | 0.12651 | 5.12 | <0.0001 | 4 | log_1_e | 0 |
| NUM1 | 0.09533 | 0.02526 | 3.77 | 0.0005 | 0 | log_S_R | 0 |
| NUM2 | 0.13048 | 0.06676 | 1.95 | 0.0575 | 0 | log τ | 0 |
| NUM3 | 0.13874 | 0.07766 | 1.79 | 0.0814 | 0 | log_PIB | 0 |
| NUM4 | 0.06165 | 0.0074901 | 8.23 | <0.0001 | 0 | ls2008q1 | 0 |
| NUM5 | −0.04843 | 0.01282 | −3.78 | 0.0005 | 0 | ao2006q1 | 0 |
| NUM6 | 0.03928 | 0.01305 | 3.01 | 0.0044 | 0 | ao2006q4 | 0 |
| NUM7 | −0.03680 | 0.01285 | −2.86 | 0.0066 | 0 | ao2007q3 | 0 |
| NUM8 | −0.02938 | 0.01262 | −2.33 | 0.0250 | 0 | ao2010q4 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
González Aguirre, J.; Del Villar, A. Administrative Costs and Tariff Rates in the Presence of Customs Evasion: Evidence from Ecuador. Economies 2021, 9, 21. https://doi.org/10.3390/economies9010021
González Aguirre J, Del Villar A. Administrative Costs and Tariff Rates in the Presence of Customs Evasion: Evidence from Ecuador. Economies. 2021; 9(1):21. https://doi.org/10.3390/economies9010021
Chicago/Turabian StyleGonzález Aguirre, Jazmín, and Alberto Del Villar. 2021. "Administrative Costs and Tariff Rates in the Presence of Customs Evasion: Evidence from Ecuador" Economies 9, no. 1: 21. https://doi.org/10.3390/economies9010021
APA StyleGonzález Aguirre, J., & Del Villar, A. (2021). Administrative Costs and Tariff Rates in the Presence of Customs Evasion: Evidence from Ecuador. Economies, 9(1), 21. https://doi.org/10.3390/economies9010021




