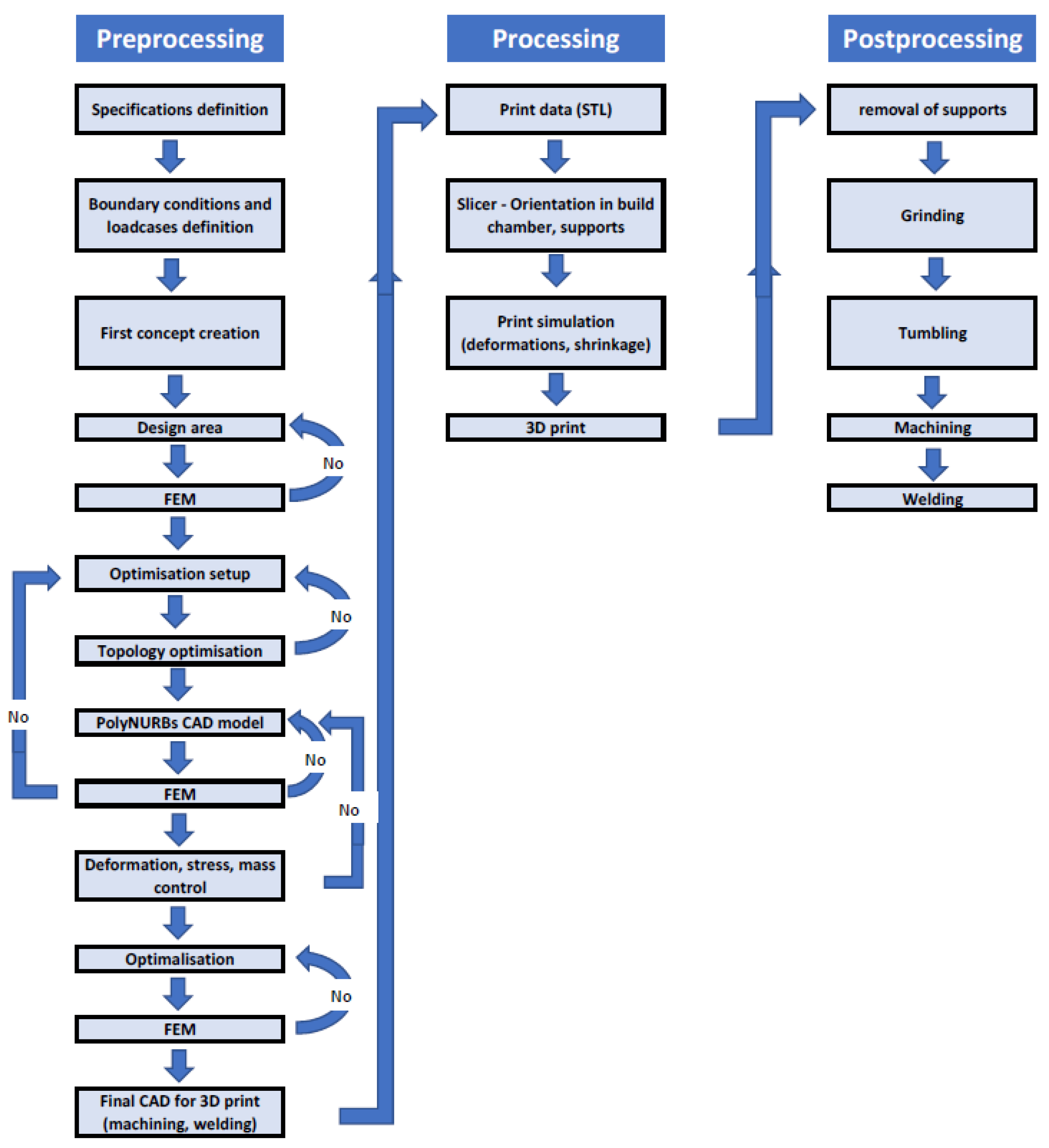
2.2. Design Area
The first boundary condition of the topological optimization is to determine the design area. It is a geometrically determined space in which optimization can take place, with respect to the maximum volume in which the future analysis solution must be located. The limiting factors in the design area creation are, in particular, the maximum possible installation dimensions, in which the part will still be able to be mounted with no collisions with the surroundings. In essence, the resulting design area itself should be a mountable and usable part that will perform its function and will not interfere with the rest of the mechanism [
9].
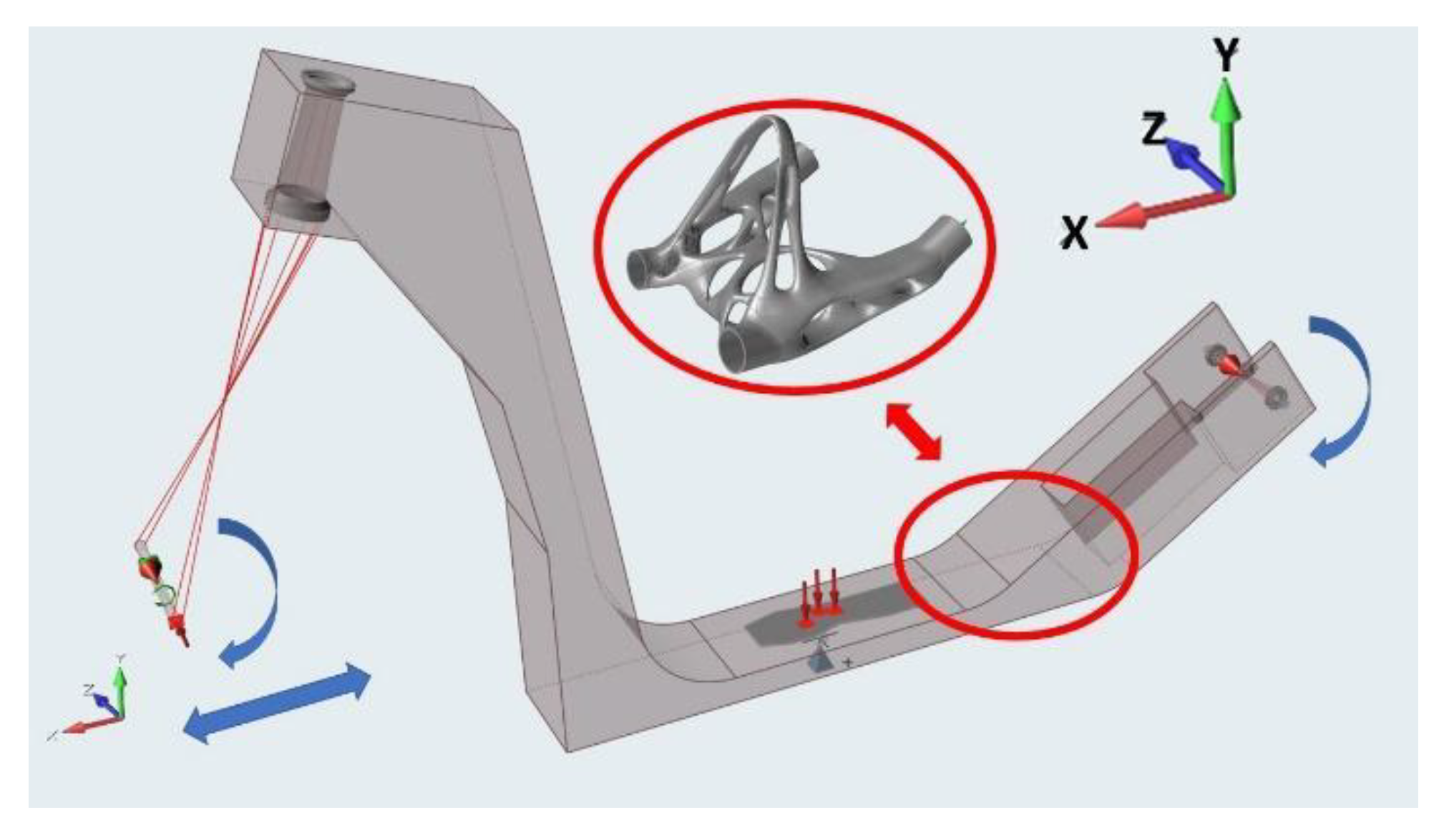
The goal is to make this area as voluminous as possible for analysis, allowing software to be as spacious as possible. First, the design area of the entire scooter frame, shown in
Figure 5, was developed. This respects the necessary geometric parameters such as fork tilt, footbar height, chassis ground clearance, and wheelbase.
This design area was then divided into parts corresponding to individual frame parts. In the case of the rear heel, the limiting geometrical condition was the overall width. As such, a 100 mm maximum was determined. When exceeded, it would be no longer comfortable to kick with the foot while riding. In addition, the rear wheel cut-out mounting holes for later bonding of the connecting profiles and the protective bar to protect the foot from entering the wheel when the foot slips were designed.
After defining these requirements, the design area of the heel was finally adjusted to the shape seen in
Figure 6.
2.3. Load Cases
For topological analysis, it is necessary to define the load cases, for which the calculation and then the FEM analysis will be calculated [
10]. These load cases were created for the entire scooter frame and then referenced to the heel part itself. Four critical states were defined in total.
2.3.1. Vertical Impact
This load case is considered as a vertical impact caused by free fall of the scooter with a rider from a height of 1 m. It simulates a jump with a scooter, and subsequently, a steep crossing of an obstacle like a kerb or hump. A 75 kg rider imposes a static load of 736 N on the frame, a value which has been multiplied by a dynamic load factor of 3 g, resulting in a loadcase value of 2210 N.
2.3.2. Braking
The load case was simulated as braking with a slowdown of 5 m·s−2, when the mass is transferred to the front wheel and mainly the front frame part and the fork neck is stressed.
2.3.3. Driving on a Flat with Allowed Deflection
This case should take into account the maximum possible deflection of the frame under the load of the rider in order to avoid excessive frame distortion while riding and collision of the chassis with the road during kicking. The case was determined experimentally by measuring the properties of a conventional scooter, where the maximum deflection of the lower chassis under the load of a rider weighing 80 kg was set at 11 mm while maintaining a minimum chassis clearance of 60 mm.
2.3.4. Torsion
This case takes into account the torsional stiffness of the scooter so that the frame is not twisted and deformed when cornering. It was also determined experimentally on a conventional frame. The frame was firmly fixed and the front fork was gradually loaded to the maximum 100 N force perpendicular to the transverse symmetry plane of the frame. The frame deflection was then measured at the neck. At 100 N, a maximum allowable neck deflection of 30 mm was determined. The experiment is shown in
Figure 7.
2.3.5. Final Load Case
The resulting representative load case was determined after several analyses to reflect the critical stress for the heel part. It consists of a combination of vertical impact and torsion, with maximum deflection checked after analyses. Basically, braking was eliminated because it mainly strains the front of the frame and relieves the rear.
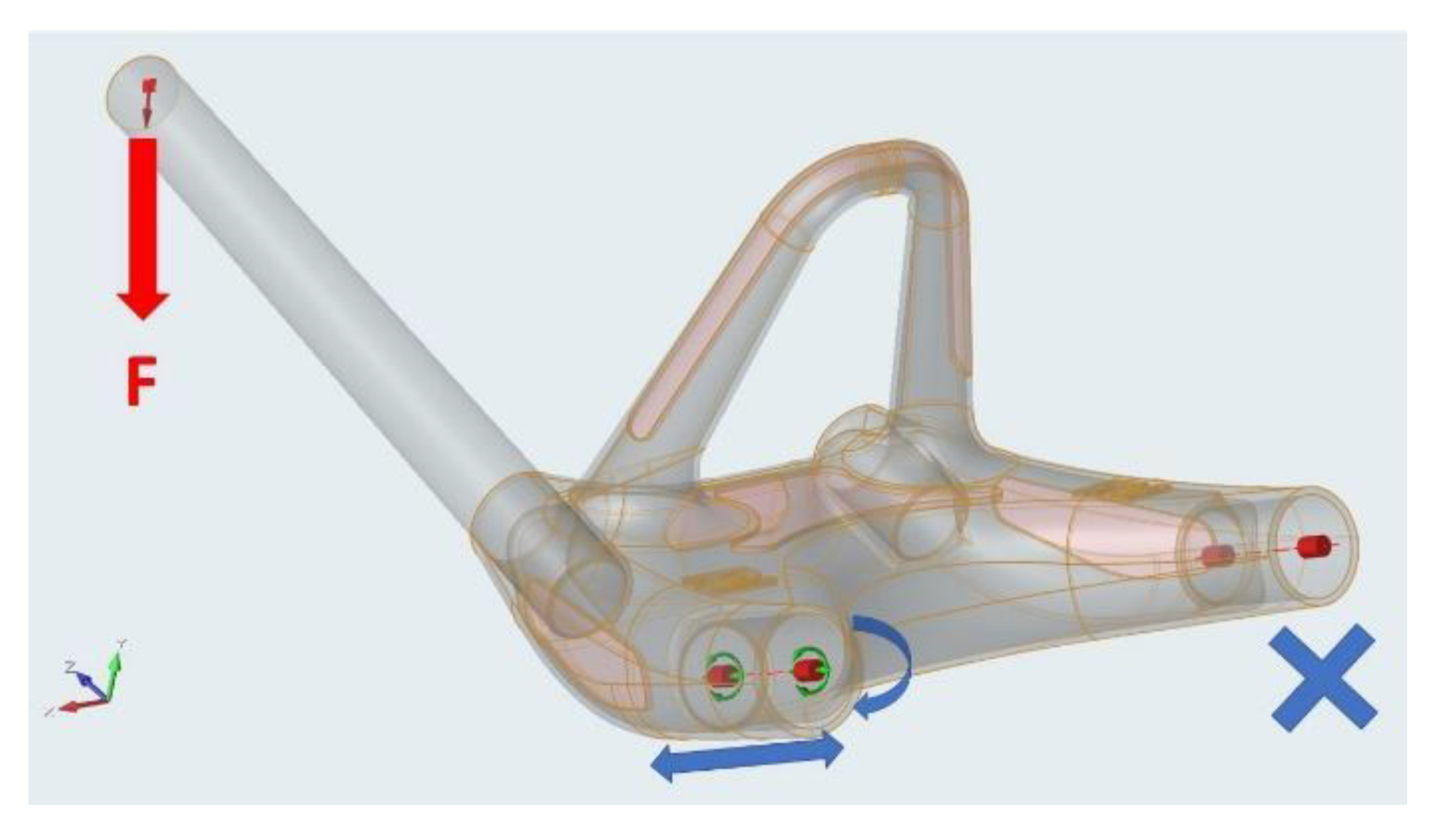
The support pattern in the computing model is shown in
Figure 8. The heel is clamped from one rear sleeve, which is fixed. The second rear sleeve has the ability to move and rotate in its axis (sliding), but not perpendicular to the axis (it represents the support in the rear wheel). One front sleeve is left loose and the other is loaded with a force on the length equal to the distance of the step centre. This force simulates the load of vertical impact, explained in
Section 2.3.1, with the rider standing unevenly on the scooter axis, representing the torsion. The static force in one arm is increased with a dynamic load factor of 3 g to 2210 N.
2.3.6. Material
For the correct analysis setting, it is also necessary to know the material from which the optimized component will be manufactured. The heel is made of 316 L stainless steel. The part will be printed from atomised powder supplied by the machine manufacturer, RENISHAW. An advantage of the powder-printed part is the improved mechanical properties in comparison with the same material in the form of metallurgical preform products, especially Yield Strength and Tensile Strength [
11].
Table 1 lists the important mechanical properties of 316 L-printed material [
12].
2.4. Topological Calculation
The topological calculation itself was performed using the SolidThinking Inspire (Altair, Troy, MI, USA) software [
13]. The software uses OptiStruct solver core for optimization. OptiStruct uses the density method, called also the SIMP method (Solid Isotropic Material with Penalisation) to solve topological optimization tasks [
14].
The process of topological optimization is related to the distribution of material and the way of joining members within the structure. For each element, the so-called “equivalent density” is defined and treated as a design variable. The value of this variable is then calculated for each element. A value of 1 is equivalent to 100% of the material and a value of 0 is equivalent to no material. The solver first attempts to assign low stress and equivalent density value elements before analyzing the impact on the remaining structure. In this manner, the density of the extraneous elements is close to 0, with the ideal design approaching a value of 1. The assumption is that the stiffness of the material is linearly dependent on its density. It is a designer’s judgment which dictates where the limit is, which elements should be left, and which ones omitted. To display the “remaining” structure, an iso-plot of element densities is used, hiding elements with a density below a certain value, so that an optimal design can be seen.
Therefore, to enforce the final design to be expressed by densities of 1 or 0 for each element, we need to use the techniques to penalize intermediate densities. The “power law representation of elasticity properties” penalization technique is exploited in OptiStruct. For any 3D or 2D element it can be expressed as shown in Equation (1):
K and K represent the penalised and the real stiffness matrix of an element. ρ is the density and p the factor of the penalisation which is always greater than 1.
The DISCRETE parameter correlates with (
p − 1) in OptiStruct, DISCRETE can be defined on the DOPTPRM bulk data entry. A value between 2.0 and 4.0 is usual for factor
p. For example, if we compare the non-penalised formulation (which is equivalent to
p = 1) at
ρ = 0.3, with
p = 2 the stiffness of the element is reduced from 0.3 to 0.09 times the stiffness of the fully dense element. For shell-dominant structures, the default DISCRETE is 1.0 and for solid-dominant structures is 2.0. The dominance is defined by the number of elements ratio. DISCRT1D is an additional parameter which can also be defined on the DOPTPRM bulk data entry. DISCRT1D enables 1D elements to use a different penalisation to 2D or 3D elements [
15].
The penalty starts at 2 for the first iteration, when the minimum member size control is used. For the second and third iterations, it increases to 3. This approach provides a more discrete solution. It is clear that when entering the next iteration phase with a different penalisation factor, the analysis results may vary significantly due to the existence of semi-dense element.
The method of topological calculation was set to maximize the rigidity of the structure but to utilize just a defined portion of the inceptive volume/mass and “shape” to make the shape as stiff as possible [
16]. The progressive analysis settings were performed with a final element size of 1 mm and a contact setting as a sliding only. This setting shown in
Table 2 has proven to be the most suitable over several iterations [
17].
Before the calculation, the planar symmetry was set. This symmetry constraint is used to generate symmetric shapes by specifying symmetry planes in the design space. In this case, the longitudinal plane of the scooter was used. This constraint can be used even under asymmetric conditions.
It is also necessary to define “partitions” in the analysis. These are places that are not subject to analysis and should remain in their original shape. Typically, these are different mounting holes and functional surfaces. In the case of the heel, the partitions were defined at the point of attachment of the composite tubes, shown in
Figure 6 in yellow.
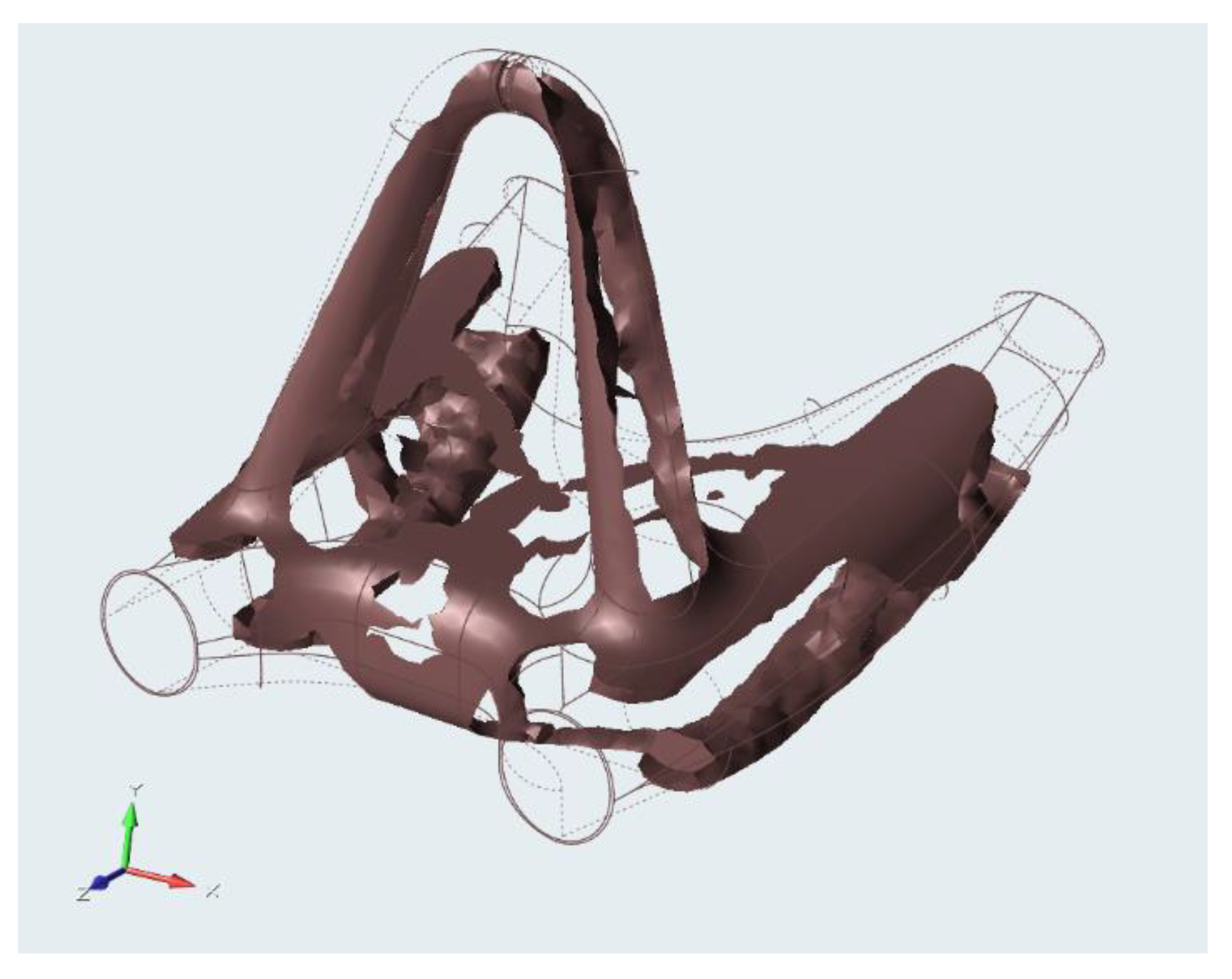
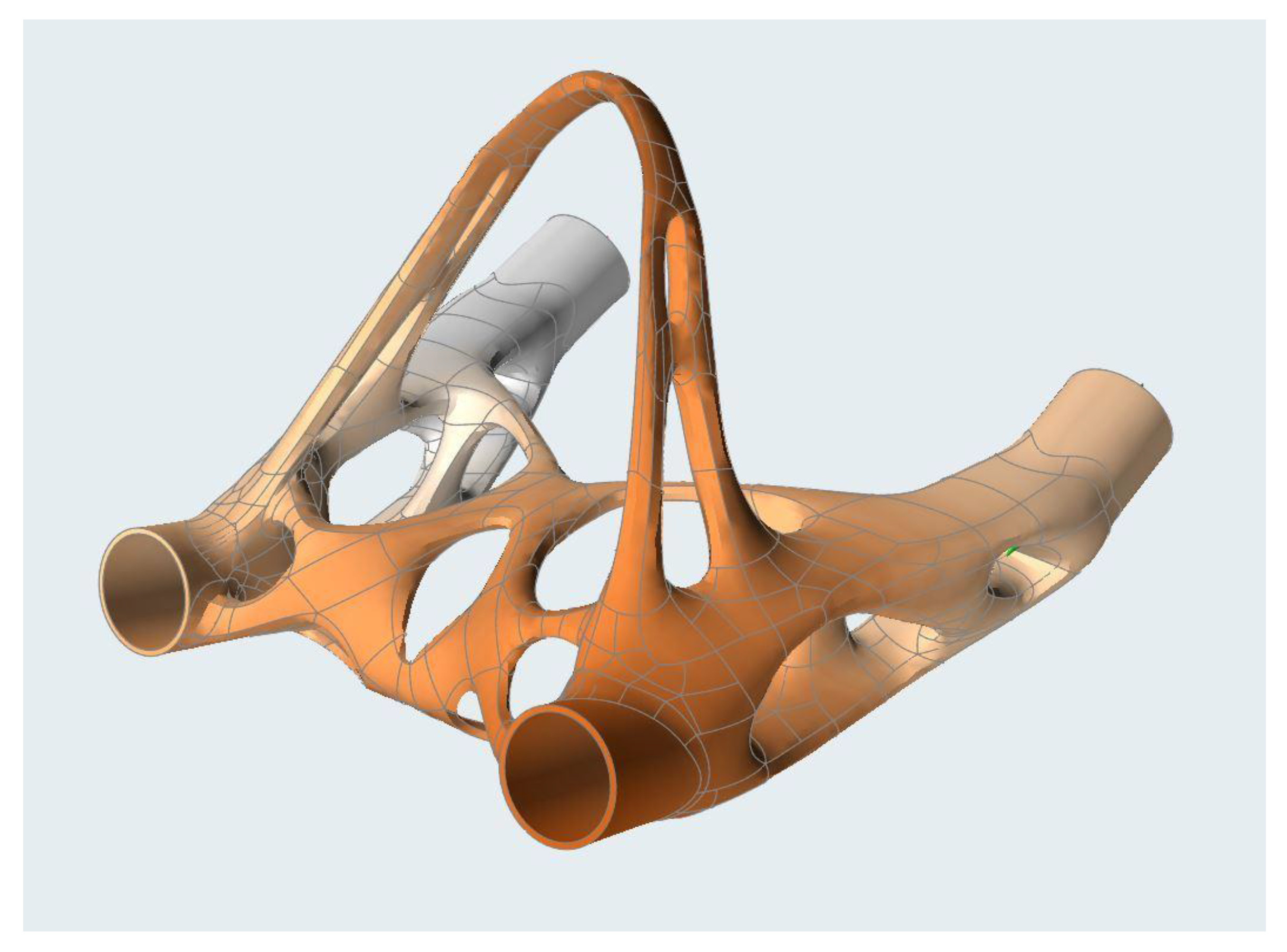
The result of the analysis is the structure of the elements, shown in
Figure 9. The calculation leaves the elements only where the load is transmitted [
18]. After the analysis, the percentage of the material represented as the density of the elements can be additionally tuned. This element network serves as a support for subsequent modelling afterwards [
19]. The output from the optimization process provide the draft design of the part. The concept shape needs to be smoothed and remodeled. The correct interpretation (smoothing) of the draft design actually has a huge impact on the end result, especially on the weight.
2.5. Solid Model Creation
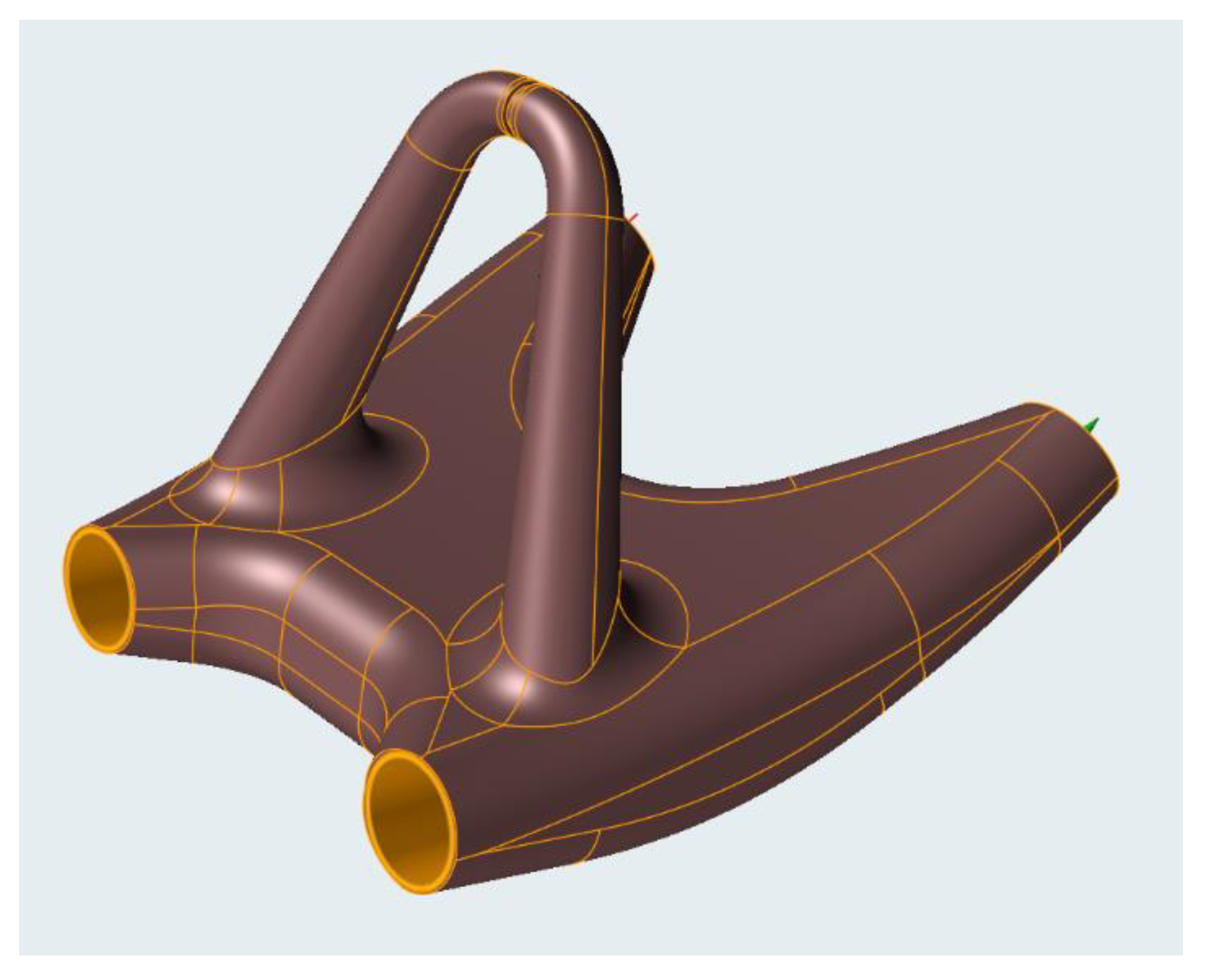
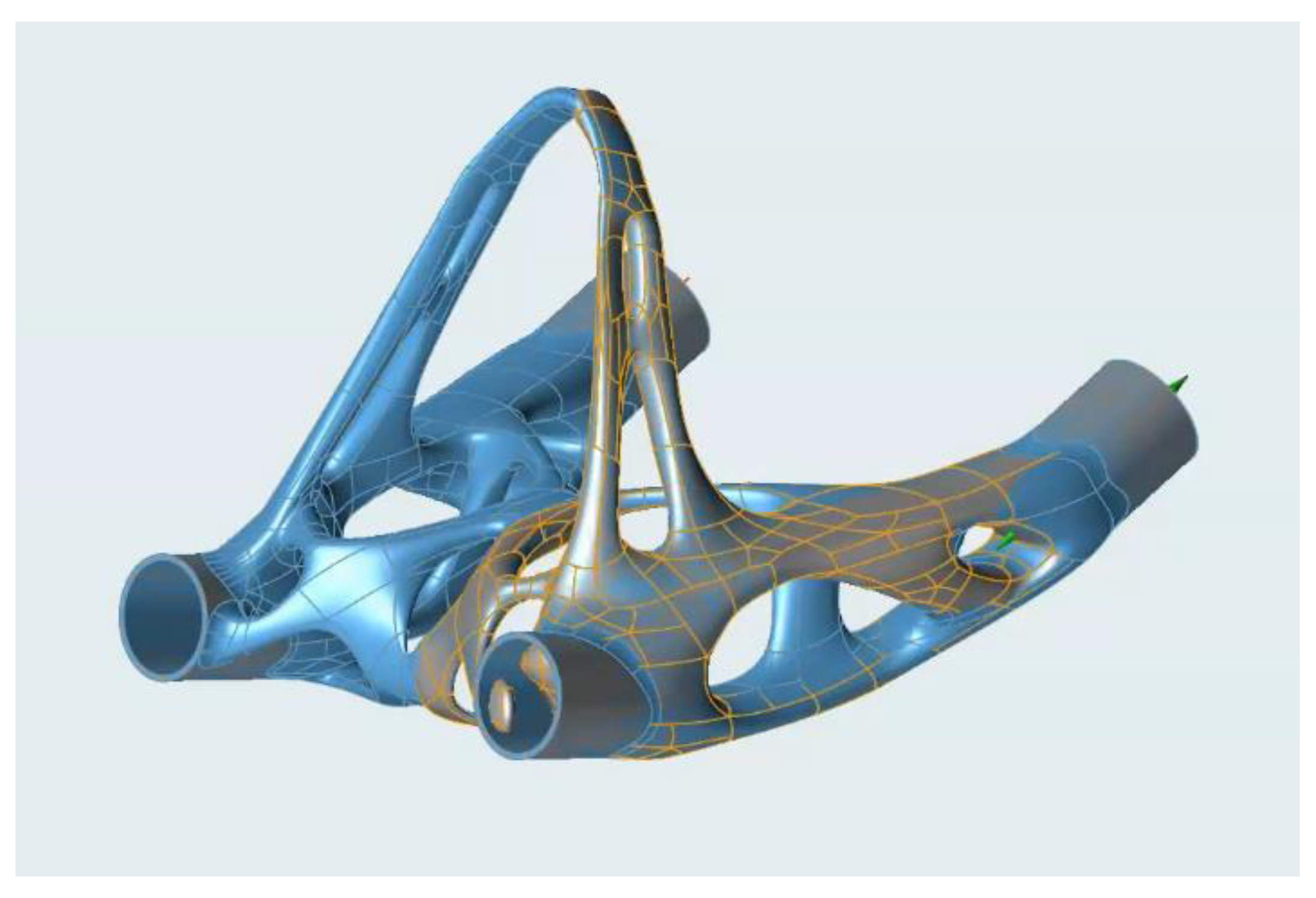
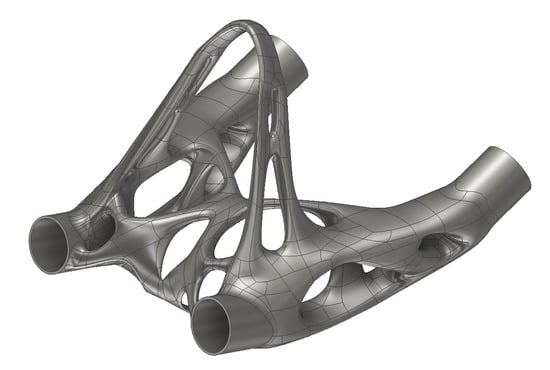
The CAD model itself is created using non-parametric volume modelling based on the analysis results. In the case of the heel, the initial organic model was created in SolidThinking Inspire software, which allows PolyNURBS based modelling. It is a freeform modelling method where a non-parametric model is formed by connecting organic blocks and surfaces to each other. It is a manual way of modelling performed by the designer, where these geometric elements are being extracted from the topological optimization result. The organic shape should trace over the optimization result precisely. The elements are further shaped, interconnected, and formed into a final appearance, as shown in
Figure 10.
One iteration of the rear heel considered was the shell part, where further lightening would occur. Moreover, the solid model created above would be partially hollowed in thick areas. It was a repetitive process of finding the ideal wall thickness, which was always verified by an FEM analysis. Ultimately, the sufficient thickness of the wall was found to be 1.3 mm. Shell modelling was performed using Autodesk Inventor software by surface modelling.
However, this shell heel part shown in
Figure 11 was not used on the frame. It would be technologically difficult to remove unbaked powder from the cavities (requiring drilling holes). The weight reduction was minimal, but the maximum von Mises stress increased.
2.6. FEM Analysis
The FEM analysis served both to validate the individual steps during the design and to final check the stiffness and strength of the frame part. The analysis was also performed in SolidThinking Inspire software.
Inspire creates and uses mesh during optimization as well during analysis. Meshing setup and creation happens in an automated meshing step in the background using a powerful algorithm to calculate mesh size. Inspire uses a combination of HyperMesh and Simlab for meshing—both could be considered the best meshing tools. If needed, a user can take control of mesh sizes for different components, but usually, the default mesh size gives the best results. The final analysis was performed as a structural analysis with a mesh element size of 1 mm. Supports and load forces were used the same as in the optimization calculation.
In particular, two values were investigated during the analysis: the maximum stress and the flow of stress in the structure, then the maximum displacement/rotation of the heel under the load [
20].
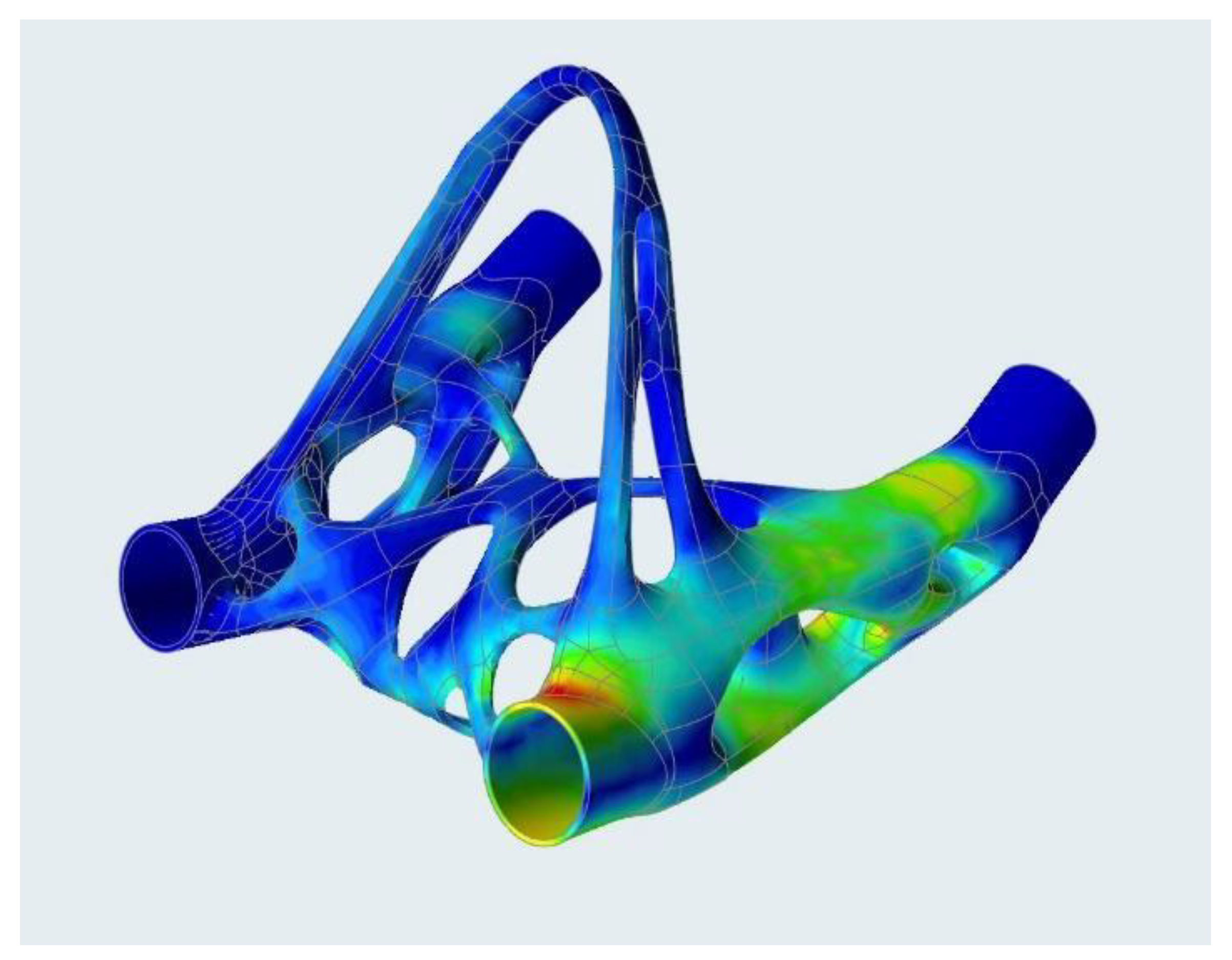
Thanks to the bionic design, which already contains the minimum of stress concentrators from the design principle, the stress is evenly distributed throughout the part and the stress peaks, common in machined parts, are eliminated by optimization itself. The stress flow and stress peak at the connection point of the sleeve is shown in
Figure 12.
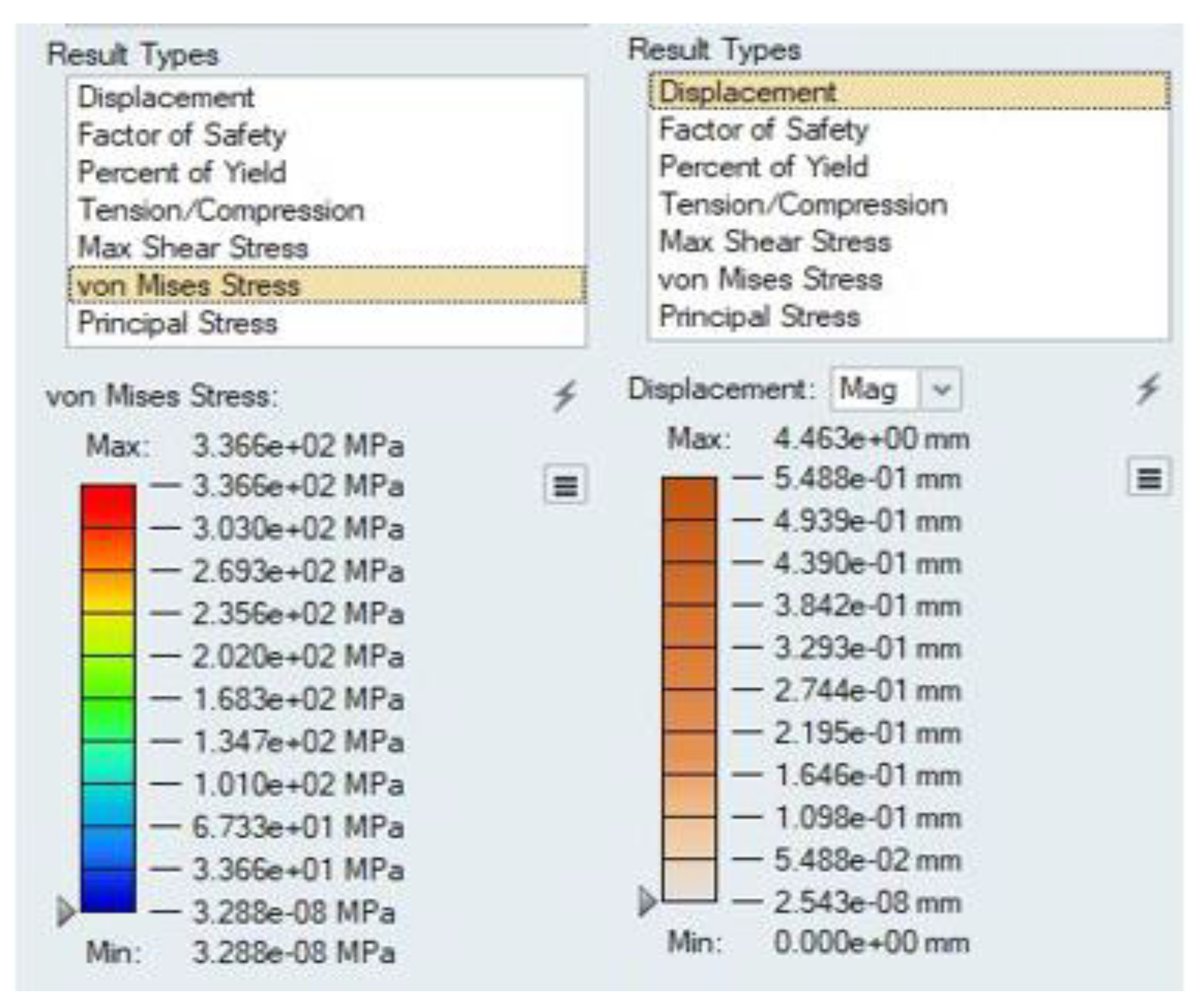
The maximum stress value in the material is 336 MPa, with the maximum displacement at the point of connection of the chassis tube being 5.5 mm (see
Figure 13). After checking the geometry in the CAD software, this displacement value was shown to satisfy the maximum allowable tread deflection condition. The heel twisting under the load and displacement distribution is shown in
Figure 14.
Compared to the initial design area, the volume of material was reduced to 22%, from the original 385 cm3 to 83 cm3. The weight of the part decreased from 3075 g for the design area to 600 g for the optimized part. This calculated weight differs slightly from the real weight due to the subsequent machining and grinding.
There was no FEM analysis performed for the design area since the area is only a volume boundary condition. Moreover, the foot was created as a completely new part, so it is not possible to compare it with the original variant. However, we can say that thanks to the use of additive production, material savings were made since 3D printing is an almost waste-free technology. Moreover, there is no need for complex machining with several clamping operations, which reduces production time and cost.