The Influence of Different Loads on the Footbridge Dynamic Parameters
Abstract
1. Introduction
2. Materials and Methods
- -
- A random gait, when the step frequency of passing pedestrians distributes under the probability curve and the phase angle of the first harmonic is random;
- -
- A synchronic gait when pedestrian gait coincides, that is, a uniform frequency and phase.
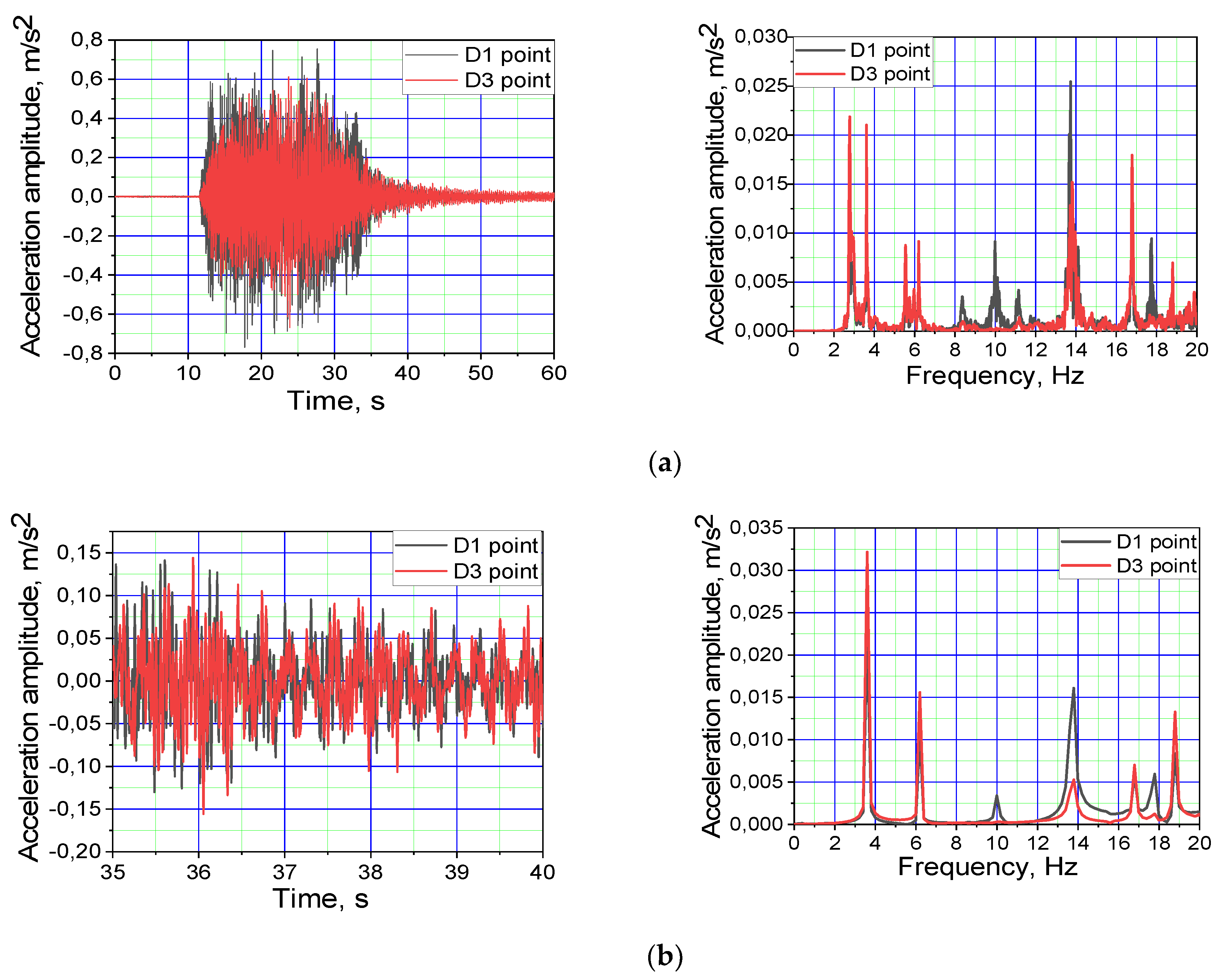
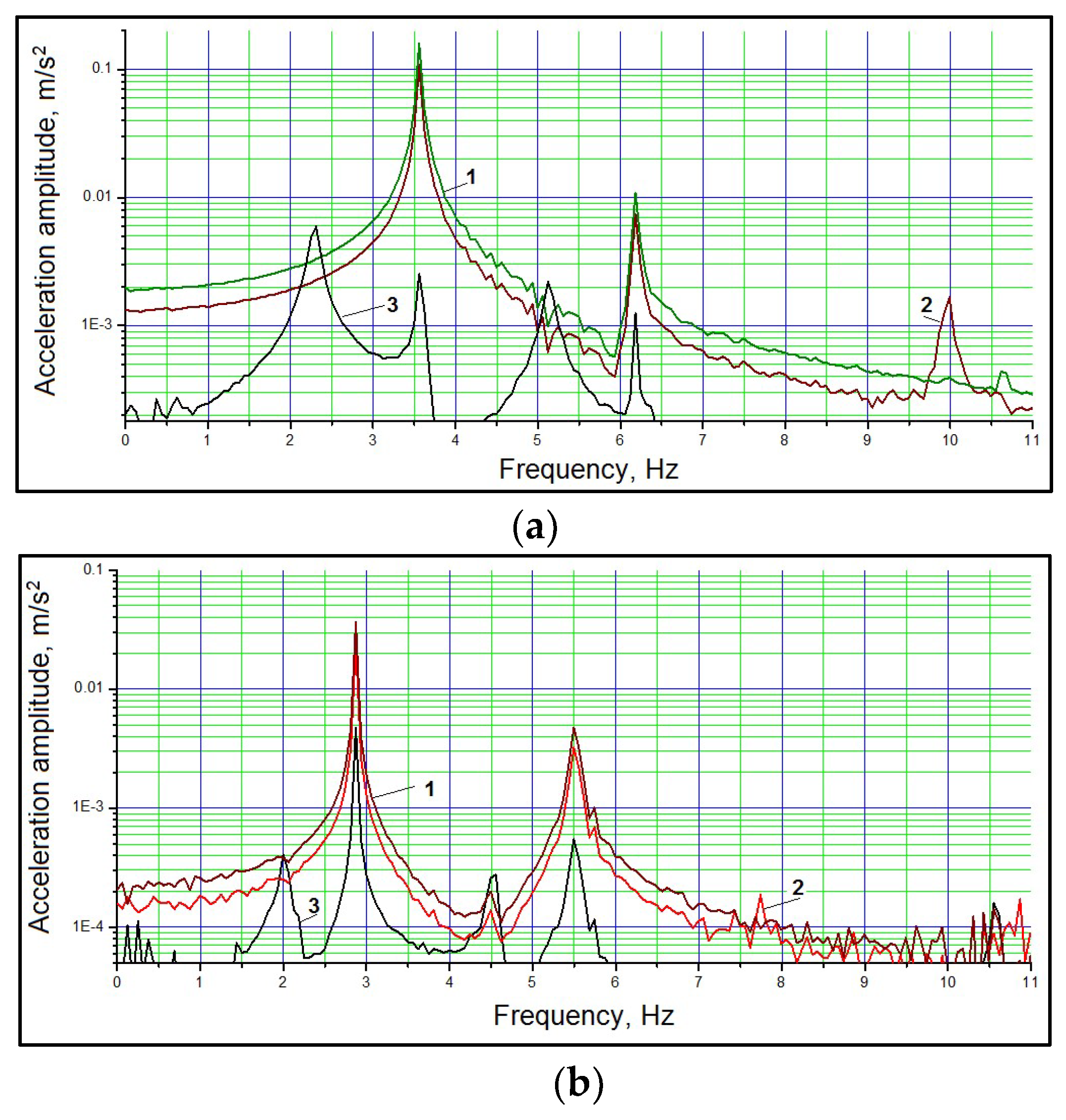
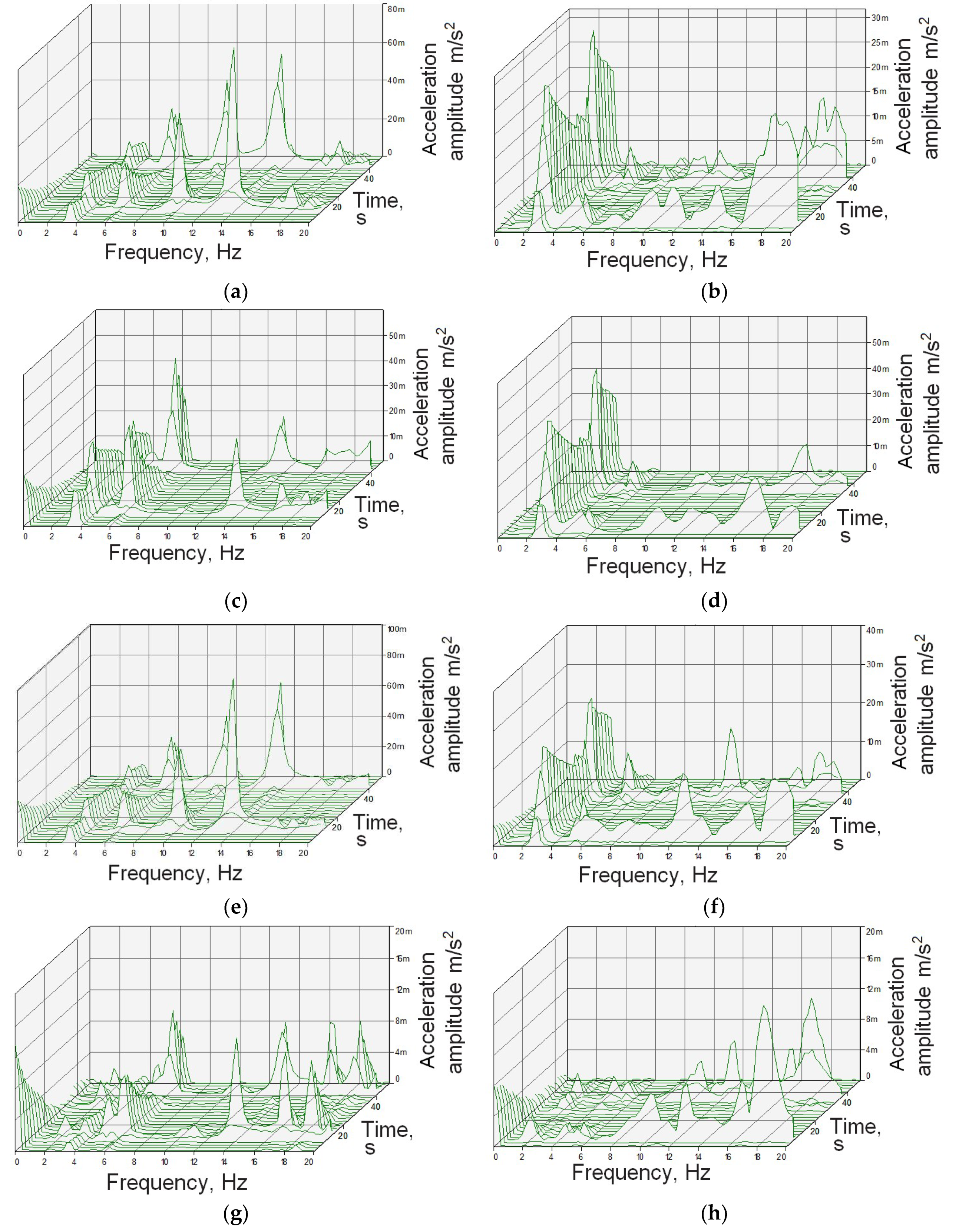
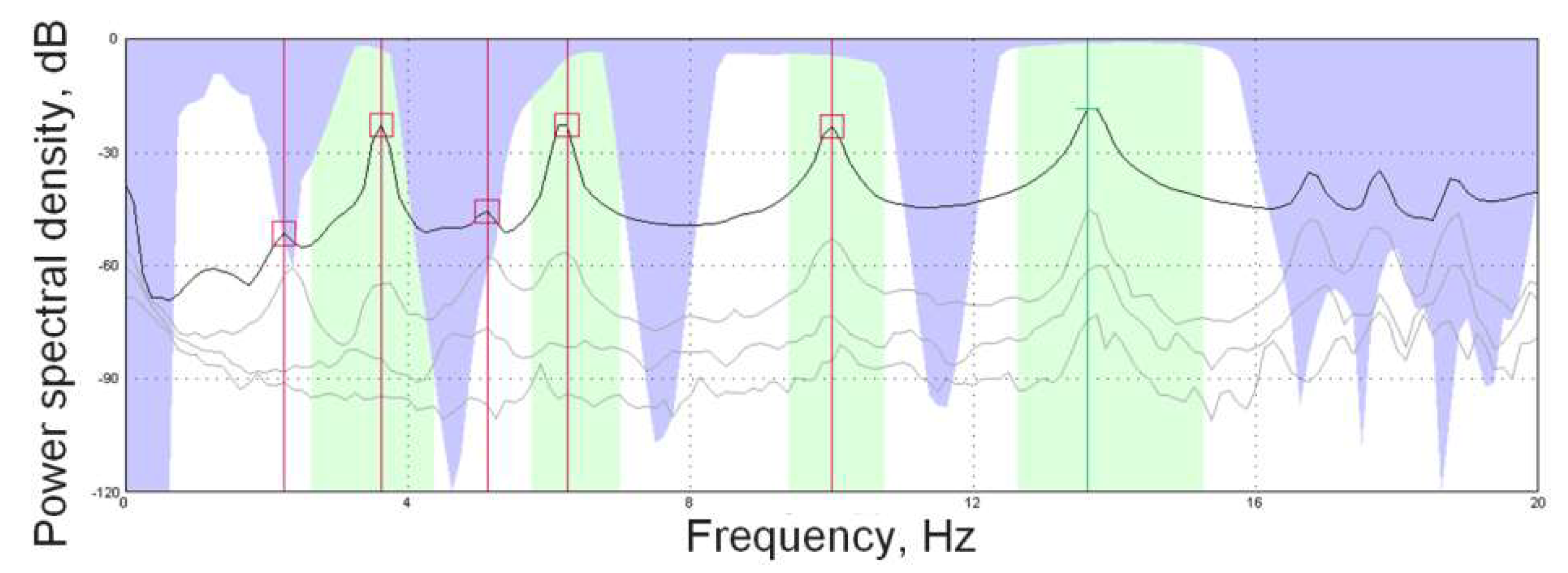
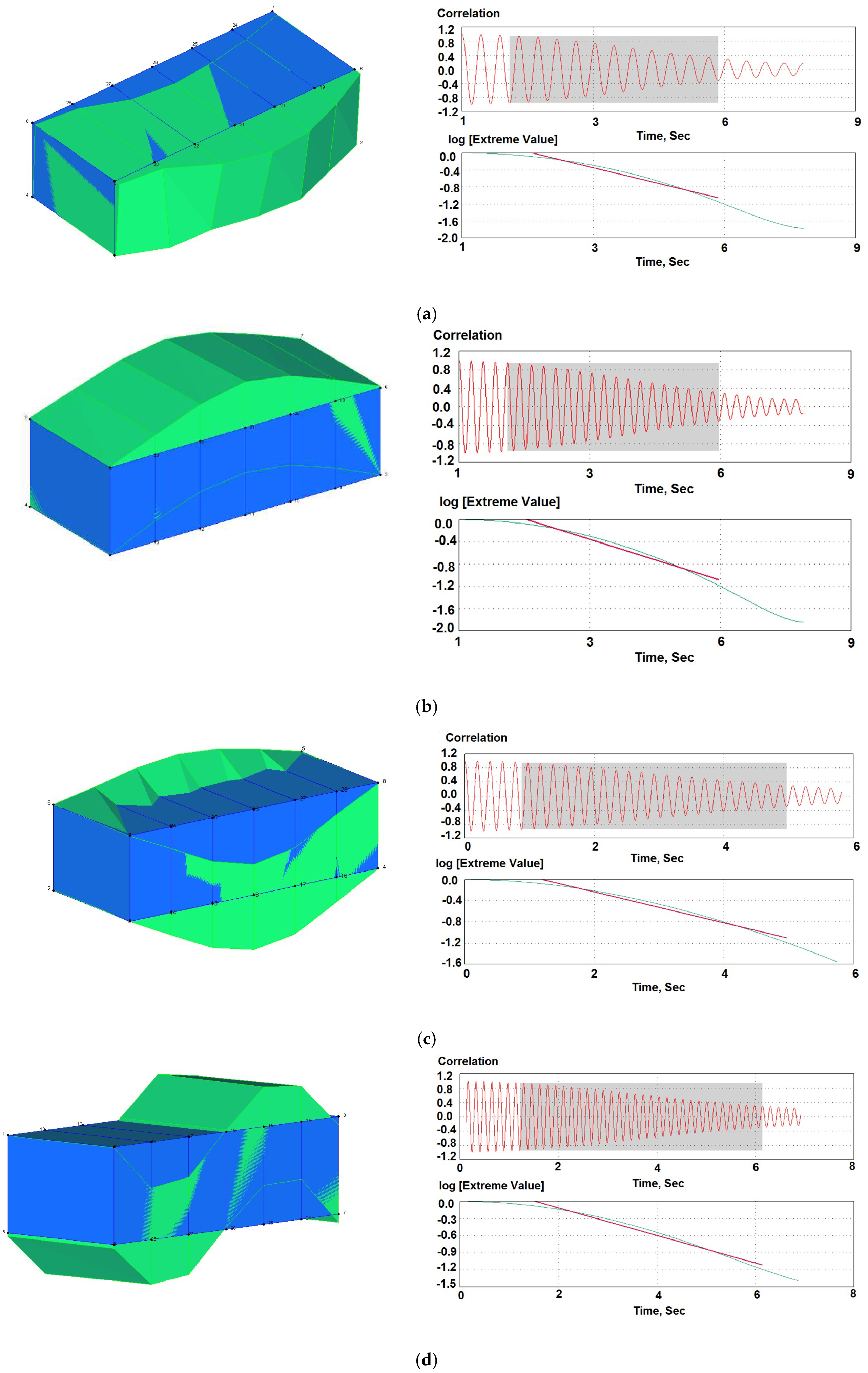
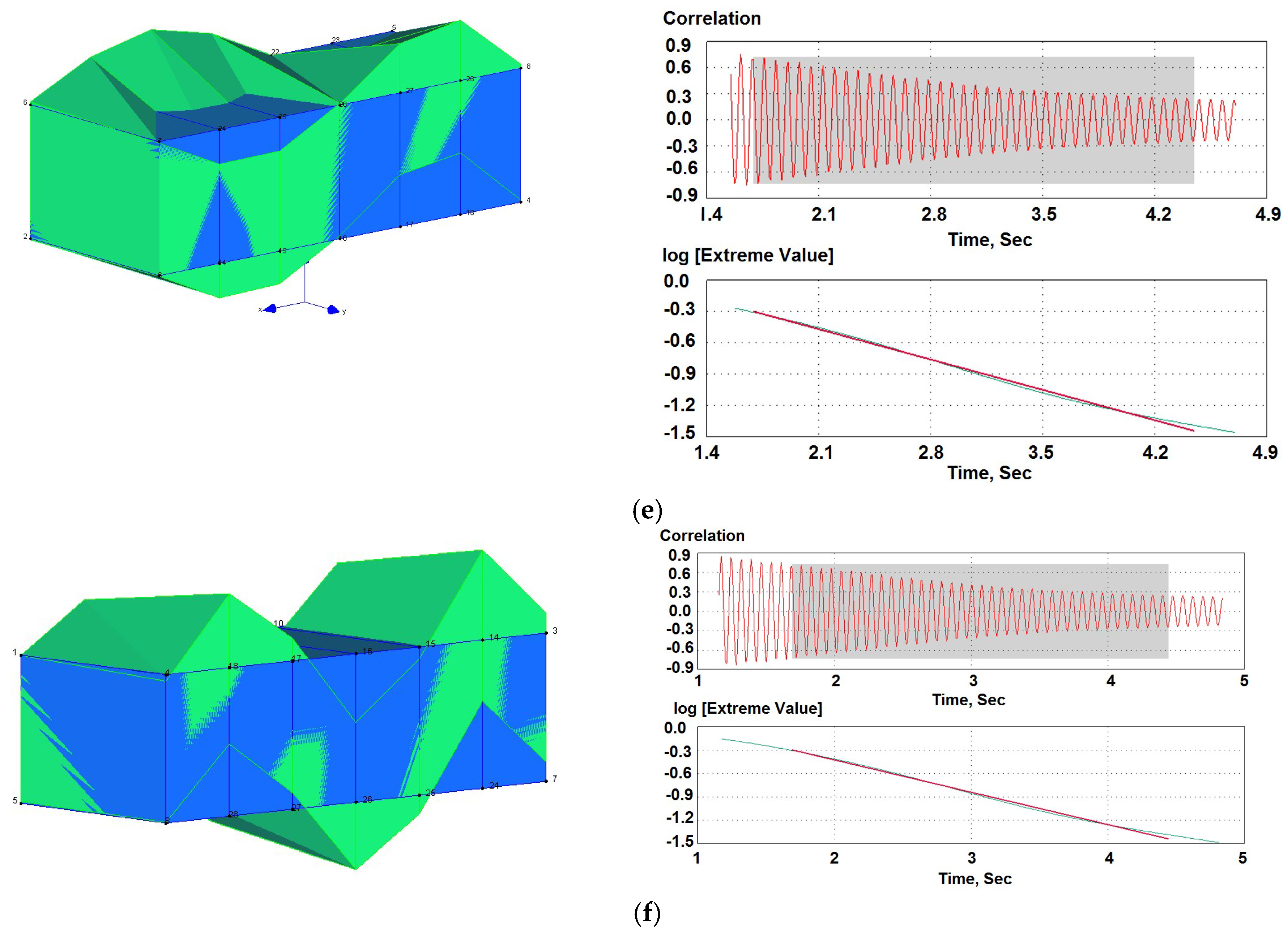
3. Results
4. Discussion
- arithmetic mean:where n is the number of the measurement results, xi—the measurement result.
- standard deviation:coefficient of variation
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ono, K. Structural Health Monitoring of Large Structures Using Acoustic Emission–Case Histories. Appl. Sci. 2019, 9, 4602. [Google Scholar] [CrossRef]
- Kilikevičius, A.; Skeivalas, J.; Kilikevičienė, K.; Matijošius, J. Analysis of Dynamic Parameters of a Railway Bridge. Appl. Sci. 2019, 9, 2545. [Google Scholar] [CrossRef]
- García, D.A.; Rosales, M.B.; Sampaio, R. Dynamic behaviour of a timber footbridge with uncertain material properties under a single deterministic walking load. Struct. Saf. 2019, 77, 10–17. [Google Scholar] [CrossRef]
- Wu, T.; Qiu, W. Dynamic analyses of pile-supported bridges including soil-structure interaction under stochastic ice loads. Soil Dyn. Earthq. Eng. 2020, 128, 105879. [Google Scholar] [CrossRef]
- Yazdani, M.; Jahdngiri, V.; Marefat, M.S. Seismic performance assessment of plain concrete arch bridges under near-field earthquakes using incremental dynamic analysis. Eng. Fail. Anal. 2019, 106, 104170. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Q.; Fan, F.; Shen, S. A method for calculating uncomfortable rates of people due to vertical floor vibrations using corresponding assessment values based on fuzzy reliability theory. J. Build. Eng. 2020, 28, 101061. [Google Scholar] [CrossRef]
- Casagrande, D.; Giongo, I.; Pederzolli, F.; Franciosi, A.; Piazza, M. Analytical, numerical and experimental assessment of vibration performance in timber floors. Eng. Struct. 2018, 168, 748–758. [Google Scholar] [CrossRef]
- EN 1990:2002+A1. Eurocode-Basis of Structural Design. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1990.2002.pdf (accessed on 6 April 2020).
- ISO. ISO 10137:2007-Bases for Design of Structures-Serviceability of Buildings and Walkways Against Vibrations. Available online: https://www.iso.org/standard/37070.html (accessed on 20 November 2019).
- EN 1991-2. Eurocode 1: Actions on structures-Part 2: Traffic loads on bridges. Available online: https://www.phd.eng.br/wp-content/uploads/2015/12/en.1991.2.2003.pdf (accessed on 6 April 2020).
- Alocci, C.; Valvo, P.S. Feasibility study of a hybrid FRP-steel cable-stayed pedestrian swing bridge. Eng. Struct. 2019, 189, 359–372. [Google Scholar] [CrossRef]
- Peng, W.; Ding, R.; Xu, W.; Xu, X.; Dai, F.; Taciroglu, E. A forensic analysis of the Florida International University pedestrian bridge collapse using incident video footages. Eng. Struct. 2019, 200, 109732. [Google Scholar] [CrossRef]
- Patra, M.; Perumal, V.; Rao, K.V.K. Modelling the effects of risk factor and time savings on pedestrians’ choice of crossing facilities at signalised intersections. Case Stud. Transp. Policy 2019. [Google Scholar] [CrossRef]
- Frýba, L. A rough assessment of railway bridges for high speed trains. Eng. Struct. 2001, 23, 548–556. [Google Scholar] [CrossRef]
- Papán, D.; Demeterová, K. The Dynamic FEM Models Results Comparison of the New Bridge Over Danube River in Bratislava. Procedia Eng. 2016, 161, 1300–1307. [Google Scholar] [CrossRef][Green Version]
- Nguyen, K.; Freytag, B.; Ralbovsky, M.; Rio, O. Assessment of serviceability limit state of vibrations in the UHPFRC-Wild bridge through an updated FEM using vehicle-bridge interaction. Comput. Struct. 2015, 156, 29–41. [Google Scholar] [CrossRef]
- Živanović, S.; Pavic, A.; Reynolds, P. Vibration serviceability of footbridges under human-induced excitation: A literature review. J. Sound Vib. 2005, 279, 1–74. [Google Scholar] [CrossRef]
- Mercier, H.; Ammann, W.J.; Deischl, F.; Eisenmann, J.; Floegl, I.; Hirsch, G.H.; Klein, G.K.; Lande, G.J.; Mahrenholtz, O.; Natke, H.G.; et al. Vibration Problems in Structures: Practical Guidelines; Euro-International Committee for Concrete, Ed.; CEB bulletin d’information; Comite Euro-International du Beton: Lausanne, Switzerland, 1991; ISBN 978-2-88394-014-7. [Google Scholar]
- Ahmadi, E.; Caprani, C.; Živanović, S.; Heidarpour, A. Assessment of human-structure interaction on a lively lightweight GFRP footbridge. Eng. Struct. 2019, 199, 109687. [Google Scholar] [CrossRef]
- Brownjohn, J.; Bocian, M.; Hester, D. Forced vibration testing of footbridges using calibrated human shaker and wireless sensors. Procedia Eng. 2017, 199, 417–422. [Google Scholar] [CrossRef][Green Version]
- Vladimír, Š.; Michal, P.; Tomáš, P. A Dynamic Analysis of the Cable-Stayed Footbridge in Čelákovice Town. Procedia Eng. 2017, 199, 2877–2882. [Google Scholar] [CrossRef]
- Zäll, E.; Andersson, A.; Ülker-Kaustell, M.; Karoumi, R. An efficient approach for considering the effect of human-structure interaction on footbridges. Procedia Eng. 2017, 199, 2913–2918. [Google Scholar] [CrossRef]
- Liu, X.; Schauer, T.; Goldack, A.; Schlaich, M. Multi-Modal Active Vibration Control of a Lightweight Stress-Ribbon Footbridge Based on Subspace Identification. IFAC-Pap. 2017, 50, 7058–7063. [Google Scholar] [CrossRef]
- Martínez-Rodrigo, M.D.; Moliner, E.; Romero, A.; De Roeck, G.; Galvín, P. Maximum resonance and cancellation phenomena in orthotropic plates traversed by moving loads: Application to railway bridges. Int. J. Mech. Sci. 2020, 169, 105316. [Google Scholar] [CrossRef]
- Yang, Y.; He, R.; Sneed, L.; Saiidi, M.S.; Belarbi, A. Truss modeling of as-built and CFRP-repaired RC bridge columns subjected to combined cyclic lateral loading and torsion. Eng. Struct. 2019, 200, 109664. [Google Scholar] [CrossRef]
- Frøseth, G.T.; Rönnquist, A. Load model of historic traffic for fatigue life estimation of Norwegian railway bridges. Eng. Struct. 2019, 200, 109626. [Google Scholar] [CrossRef]
- Ranjbarian, M.; Mechtcherine, V. Influence of loading parameters in cyclic tension-compression regime on crack-bridging behaviour of PVA microfibres embedded in cement-based matrix. Constr. Build. Mater. 2019, 228, 116760. [Google Scholar] [CrossRef]
- Ti, Z.; Zhang, M.; Li, Y.; Wei, K. Numerical study on the stochastic response of a long-span sea-crossing bridge subjected to extreme nonlinear wave loads. Eng. Struct. 2019, 196, 109287. [Google Scholar] [CrossRef]
- Cao, J.; Ren, S.; Cao, S.; Ge, Y. Physical simulations on wind loading characteristics of streamlined bridge decks under tornado-like vortices. J. Wind Eng. Ind. Aerodyn. 2019, 189, 56–70. [Google Scholar] [CrossRef]
- Kaddar, B.; Aoustin, Y.; Chevallereau, C. Arm swing effects on walking bipedal gaits composed of impact, single and double support phases. Robot. Auton. Syst. 2015, 66, 104–115. [Google Scholar] [CrossRef]
- Silva, F.M.; Machado, J.A.T. Dynamic Efficiency During Bipedal Walking. IFAC Proc. Vol. 1998, 31, 195–200. [Google Scholar] [CrossRef]
- Lv, Y.; Wang, H.; Zhou, Y.; Yoshino, H.; Yonekura, H.; Takaki, R.; Kurihara, G. The influence of ventilation mode and personnel walking behavior on distribution characteristics of indoor particles. Build. Environ. 2019, 149, 582–591. [Google Scholar] [CrossRef]
- Chang, L.; Tu, S.; Ye, W.; Zhang, X. Dynamic simulation of contaminant inleakage produced by human walking into control room. Int. J. Heat Mass Transf. 2017, 113, 1179–1188. [Google Scholar] [CrossRef]
- Li, H.; Thrash, T.; Hölscher, C.; Schinazi, V.R. The effect of crowdedness on human wayfinding and locomotion in a multi-level virtual shopping mall. J. Environ. Psychol. 2019, 65, 101320. [Google Scholar] [CrossRef]
- Schlageter-Tello, A.; Van Hertem, T.; Bokkers, E.A.M.; Viazzi, S.; Bahr, C.; Lokhorst, K. Performance of human observers and an automatic 3-dimensional computer-vision-based locomotion scoring method to detect lameness and hoof lesions in dairy cows. J. Dairy Sci. 2018, 101, 6322–6335. [Google Scholar] [CrossRef]
- Acevedo, M.; Ponce, H. Multibody dynamics for human-like locomotion. In Design and Operation of Human Locomotion Systems; Elsevier: Amsterdam, The Netherlands, 2020; pp. 51–81. ISBN 978-0-12-815659-9. [Google Scholar]
- Huang, W.-N.W.; VanSwearingen, J. An observational treatment-based gait pattern classification method for targeting interventions for older adult males with mobility problems: Validity based on movement control and biomechanical factors. Gait Posture 2019, 71, 192–197. [Google Scholar] [CrossRef]
- Barrio, R.; Lozano, Á.; Rodríguez, M.; Serrano, S. Numerical detection of patterns in CPGs: Gait patterns in insect movement. Commun. Nonlinear Sci. Numer. Simul. 2020, 82, 105047. [Google Scholar] [CrossRef]
- van Andel, S.; Cole, M.H.; Pepping, G.-J. Influence of age and falls incidence on tau guidance of centre of pressure movement during gait initiation. Gait Posture 2019, 70, 104–108. [Google Scholar] [CrossRef]
- Oliveira, N.; Ehrenberg, N.; Cheng, J.; Blochlinger, S.; Barrance, P. Visual kinematic feedback enhances the execution of a novel knee flexion gait pattern in children and adolescents. Gait Posture 2019, 74, 94–101. [Google Scholar] [CrossRef]
- Li, T.; Li, Q.; Liu, T. An actuated dissipative spring-mass walking model: Predicting human-like ground reaction forces and the effects of model parameters. J. Biomech. 2019, 90, 58–64. [Google Scholar] [CrossRef]
- Kumar, P.; Kumar, A.; Racic, V.; Erlicher, S. Modelling vertical human walking forces using self-sustained oscillator. Mech. Syst. Signal Process. 2018, 99, 345–363. [Google Scholar] [CrossRef]
- Hao, M.; Chen, K.; Fu, C. Effects of hip torque during step-to-step transition on center-of-mass dynamics during human walking examined with numerical simulation. J. Biomech. 2019, 90, 33–39. [Google Scholar] [CrossRef]
- Leander, J. Reliability evaluation of the Eurocode model for fatigue assessment of steel bridges. J. Constr. Steel Res. 2018, 141, 1–8. [Google Scholar] [CrossRef]
- Hobbacher, A.F.; Hicks, S.J.; Karpenko, M.; Thole, F.; Uy, B. Transfer of Australasian bridge design to fatigue verification system of Eurocode 3. J. Constr. Steel Res. 2016, 122, 532–542. [Google Scholar] [CrossRef]
- Kuhlmann, U.; Bove, S.; Breunig, S.; Drebenstedt, K. Fatigue design of selected details in steel bridges. Structures 2019, 21, 75–89. [Google Scholar] [CrossRef]
- Matsuoka, K.; Collina, A.; Somaschini, C.; Sogabe, M. Influence of local deck vibrations on the evaluation of the maximum acceleration of a steel-concrete composite bridge for a high-speed railway. Eng. Struct. 2019, 200, 109736. [Google Scholar] [CrossRef]
- Enríquez-Zárate, J.; Silva-Navarro, G.; Abundis-Fong, H.F. Active vibration suppression through positive acceleration feedback on a building-like structure: An experimental study. Mech. Syst. Signal Process. 2016, 72–73, 451–461. [Google Scholar] [CrossRef]
- Guozhen, Y.; Yongqian, L.; Zhi, Y. A novel fiber Bragg grating acceleration sensor for measurement of vibration. Optik 2016, 127, 8874–8882. [Google Scholar] [CrossRef]
- Bruno, L.; Corbetta, A. Uncertainties in crowd dynamic loading of footbridges: A novel multi-scale model of pedestrian traffic. Eng. Struct. 2017, 147, 545–566. [Google Scholar] [CrossRef]
- Gonilha, J.A.; Correia, J.R.; Branco, F.A. Dynamic response under pedestrian load of a GFRP–SFRSCC hybrid footbridge prototype: Experimental tests and numerical simulation. Compos. Struct. 2013, 95, 453–463. [Google Scholar] [CrossRef]
- Ansys manual Theory reference. Available online: http://research.me.udel.edu/~lwang/teaching/MEx81/ansys56manual.pdf (accessed on 20 November 2019).
- Gaspar, C.M.R.; Santos da Silva, J.G.; Costa-Neves, L.F. Multimode vibration control of building steel–concrete composite floors submitted to human rhythmic activities. Comput. Struct. 2016, 165, 107–122. [Google Scholar] [CrossRef]
- Mohamed Fahmy, Y.G.; Sidky, A.N.M. An experimental investigation of composite floor vibration due to human activities. A case study. HBRC J. 2012, 8, 228–238. [Google Scholar] [CrossRef]
- ISO. ISO 2631-2:2003 Mechanical vibration and shock—Evaluation of human exposure to whole-body vibration—Part 2: Vibration in buildings (1 Hz to 80 Hz). Available online: https://www.iso.org/standard/23012.html (accessed on 20 November 2019).
- Griffin, M.J. Handbook of Human Vibration, 1. print. paperback ed.; Academic Press: London, UK, 1996; ISBN 978-0-12-303040-5. [Google Scholar]
- Li, X.F.; Li, H.B.; Zhang, G.K. Damage assessment and blast vibrations controlling considering rock properties of underwater blasting. Int. J. Rock Mech. Min. Sci. 2019, 121, 104045. [Google Scholar] [CrossRef]
- Venkatesh, G.; Kamal, C.; Vennila, P.; Govindaraju, M.; Mary, Y.S.; Armakovic, S.; Armakovic, S.J.; Kaya, S.; Panicker, C.Y. Molecular dynamic simulations, ALIE surface, Fukui functions geometrical, molecular docking and vibrational spectra studies of tetra chloro p and m-xylene. J. Mol. Struct. 2018, 1171, 253–267. [Google Scholar] [CrossRef]
- Pietropolli Charmet, A.; Bizzocchi, L.; Giuliano, B.M.; Caselli, P.; Craig, N.C.; Krasnoshchekov, S.V. Disentangling the IR spectra of 2,3,3,3-tetrafluoropropene using an ab initio description of vibrational polyads by means of canonical Van Vleck perturbation theory. J. Quant. Spectrosc. Radiat. Transf. 2019, 239, 106656. [Google Scholar] [CrossRef]
- Kamiński, T.; Bień, J. Application of Kinematic Method and FEM in Analysis of Ultimate Load Bearing Capacity of Damaged Masonry Arch Bridges. Procedia Eng. 2013, 57, 524–532. [Google Scholar] [CrossRef]
- Pivovarov, D.; Steinmann, P.; Willner, K. Acceleration of the spectral stochastic FEM using POD and element based discrete empirical approximation for a micromechanical model of heterogeneous materials with random geometry. Comput. Methods Appl. Mech. Eng. 2019, 360, 112689. [Google Scholar] [CrossRef]
- Li, H.; O’Hara, P.; Duarte, C.A. A two-scale generalized FEM for the evaluation of stress intensity factors at spot welds subjected to thermomechanical loads. Eng. Fract. Mech. 2019, 213, 21–52. [Google Scholar] [CrossRef]
- Xu, Y.; Jung, J.; Nojavan, S.; Yang, Q. An orthotropic augmented finite element method (A-FEM) for high-fidelity progressive damage analyses of laminated composites. Compos. Struct. 2019, 229, 111416. [Google Scholar] [CrossRef]
- Maleska, T.; Beben, D. Numerical analysis of a soil-steel bridge during backfilling using various shell models. Eng. Struct. 2019, 196, 109358. [Google Scholar] [CrossRef]



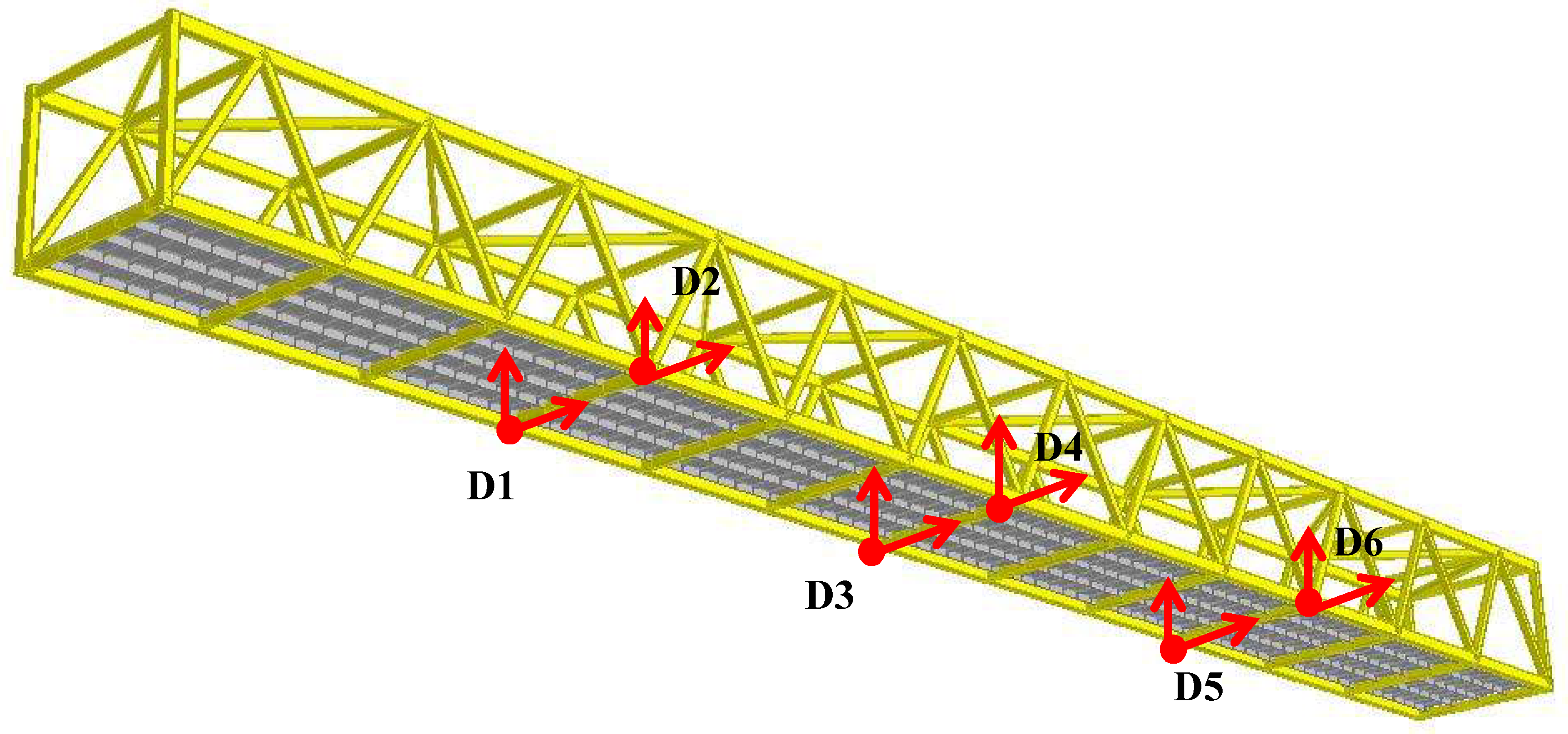





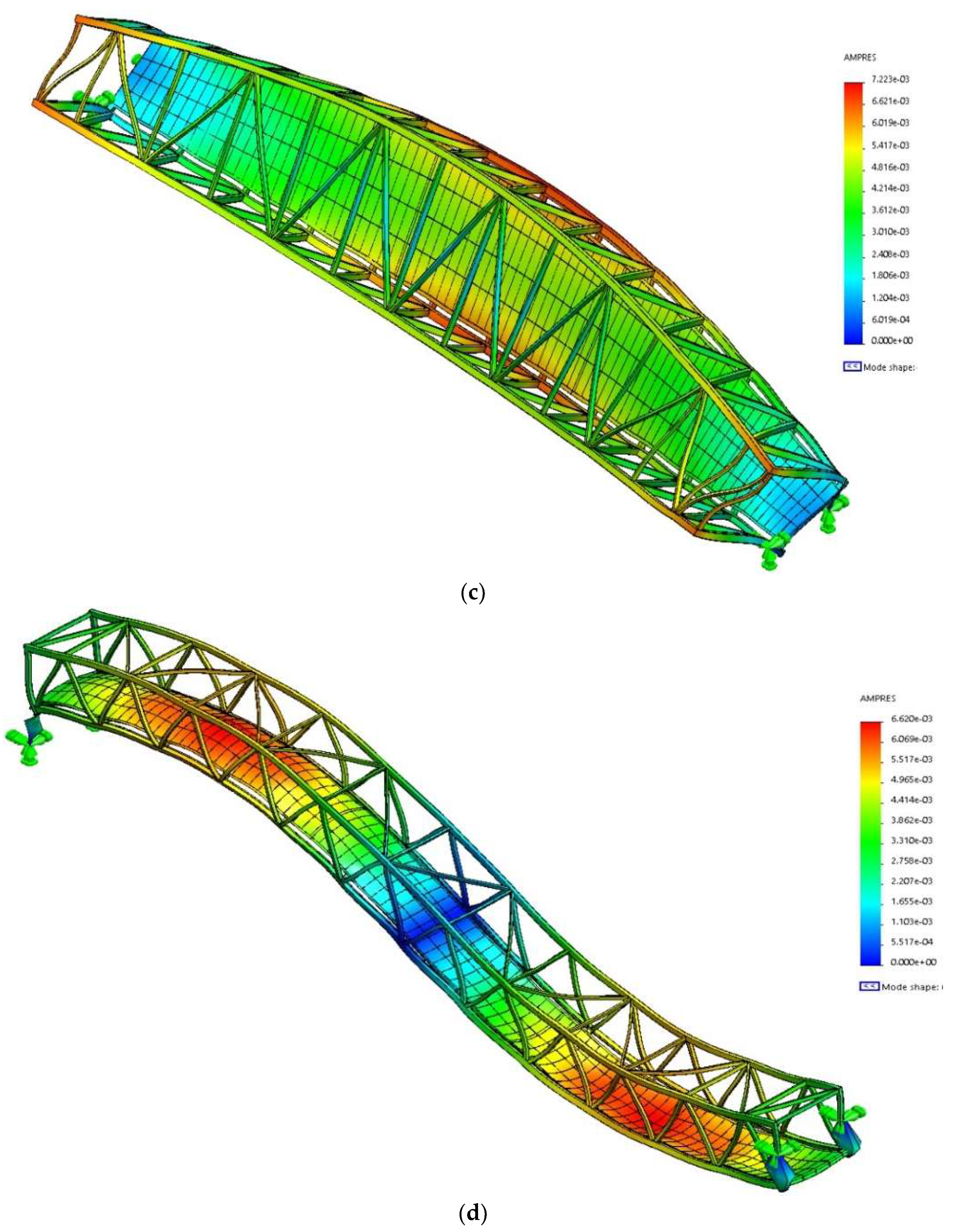
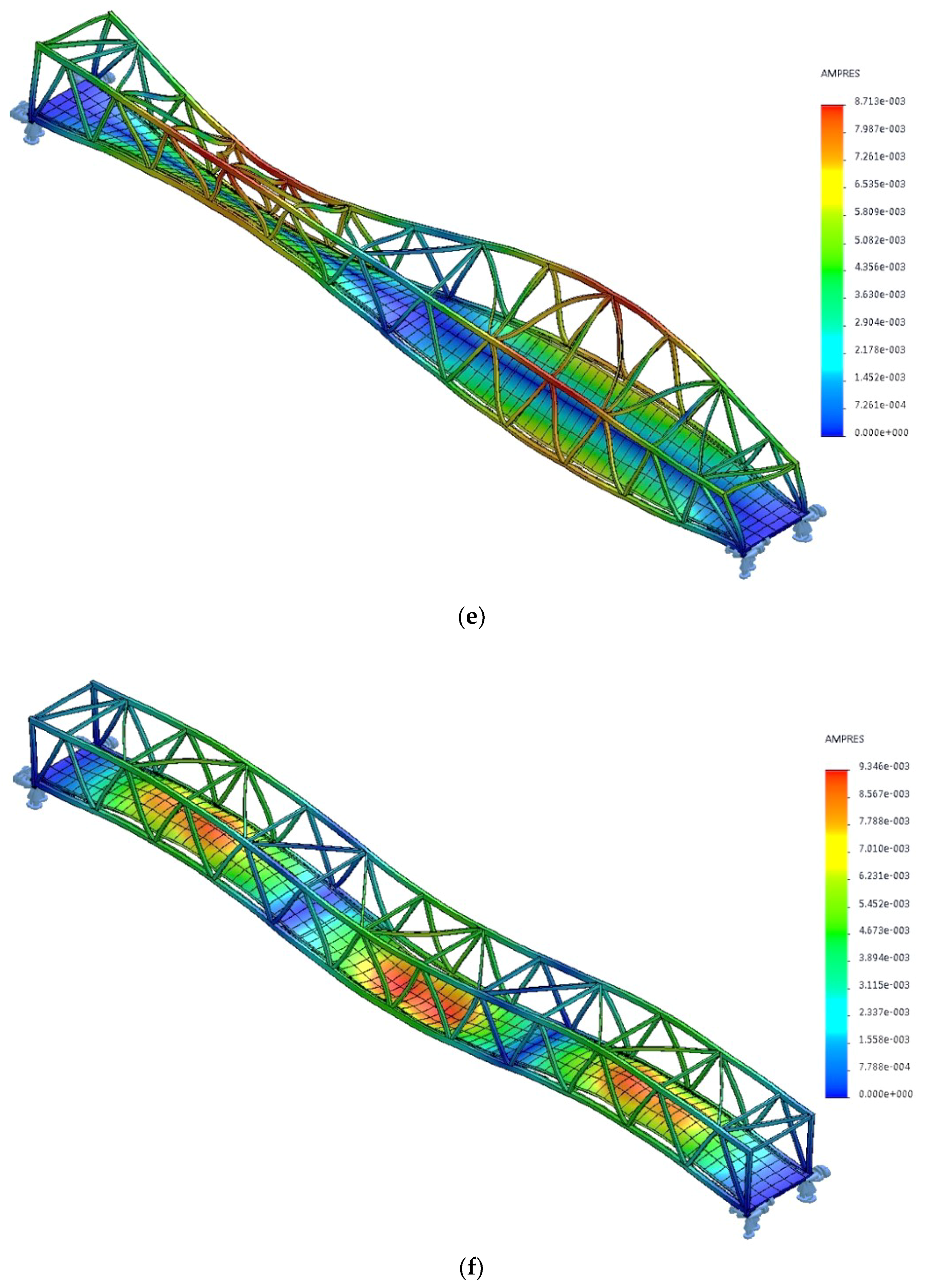
| Top Chord | Bottom Chord | Vertical | Diagonal * (from 1 to 5 and from 20 to 24) | Diagonal * (from 6 to 19) | Deck Beam | Wind Bracing |
|---|---|---|---|---|---|---|
| RHS 300 × 200 × 12 | RHS 300 × 200 × 12 | SHS 200 × 200 × 10 | SHS 180 × 180 × 10 | SHS 180 × 180 × 5 | SHS 200 × 200 × 10 | RHS 200 × 100 × 5 |
| Experiment | Statistical Characteristics | Experimental Modes and Corresponding Natural Frequencies | |||||
|---|---|---|---|---|---|---|---|
| 1 Mode | 2 Mode | 3 Mode | 4 Mode | 5 Mode | 6 Mode | ||
| Impact excitation (1—without load) | , Hz | 2.28 | 3.56 | 5.16 | 6.20 | 9.98 | 13.79 |
| , Hz | 0.074 | 0.14 | 0.37 | 0.16 | 0 | 0.32 | |
| COV, % | 3.25 | 3.93 | 7.17 | 2.55 | 0 | 2.32 | |
| Impact excitation (2—semi-loaded) | , Hz | 2.02 | 2.88 | 4.52 | 5.52 | 7.70 | 12.50 |
| , Hz | 0.16 | 0.03 | 0.21 | 0.08 | 0.55 | 0.18 | |
| COV, % | 7.92 | 1.04 | 4.65 | 1.45 | 7.14 | 1.44 | |
| OMA (1—without load) | , Hz | 2.25 | 3.50 | 5.00 | 6.25 | 10.0 | 13.60 |
| , Hz | 0.074 | 0.14 | 0.37 | 0.16 | 0 | 0.45 | |
| COV, % | 3.29 | 4.00 | 7.40 | 2.56 | 0 | 3.31 | |
| Damping Ratio [%] | 1.713 | 1.077 | 0.905 | 0.621 | 0.440 | 0.521 | |
| OMA (2—semi-loaded) | , Hz | 2.00 | 2.75 | 4.50 | 5.50 | 7.75 | 12.25 |
| , Hz | 0.16 | 0.03 | 0.21 | 0.08 | 0.55 | 0.24 | |
| COV, % | 8.00 | 1.09 | 4.67 | 1.45 | 7.10 | 1.96 | |
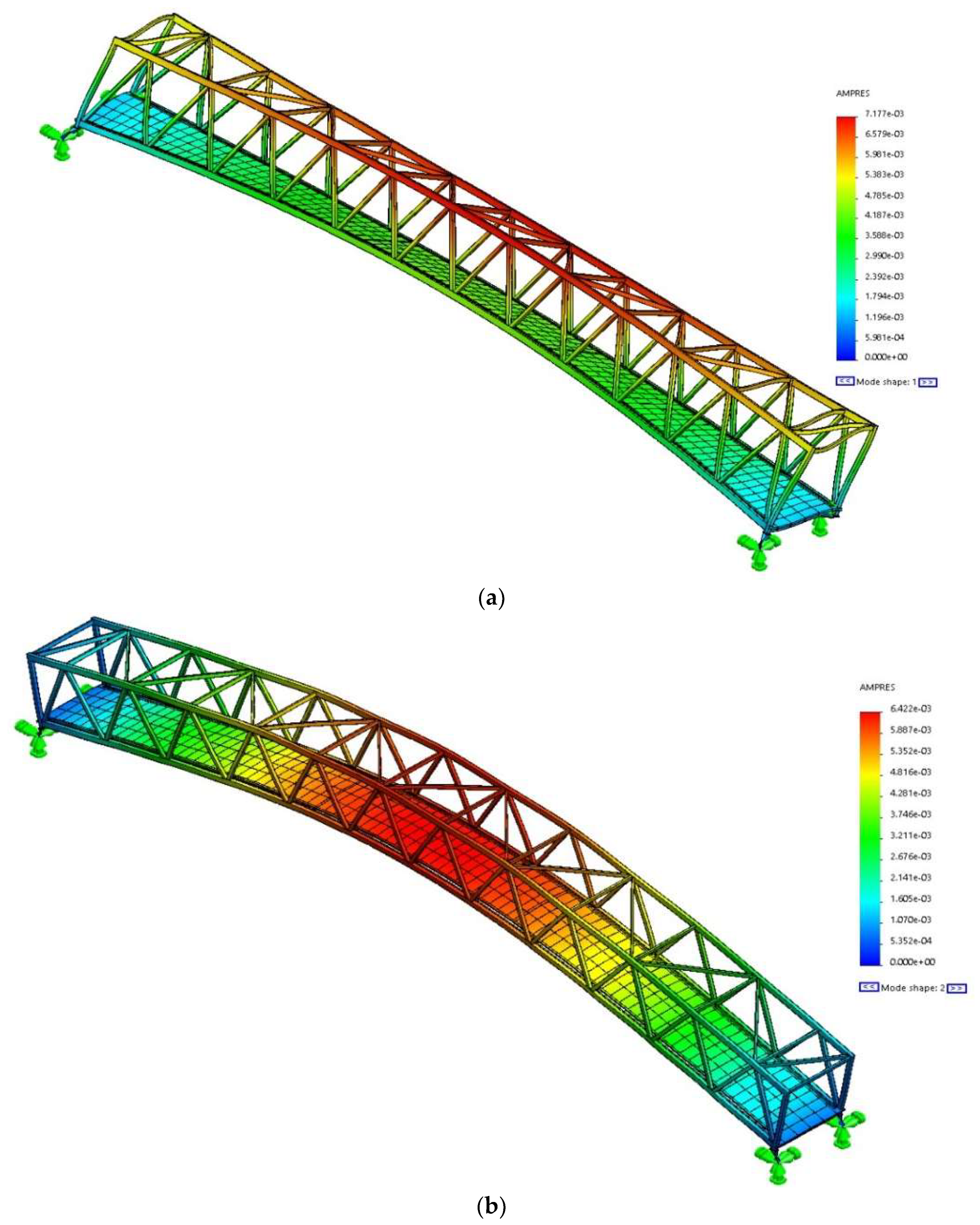
| Mode | Frequencies (1—without Load; 2—Semi-Loaded) | Mode Type | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| fEXP,OMA, Hz | fEXP impact, Hz | fFEM, Hz | Δ = fFE/fEXP,OMA | Δ = fFE/fEXP impact | |||||||
| No | 1 | 2 | 1 | 2 | 1 | 2 | 1 | 2 | 1 | 2 | |
| 1 | 2.25 | 2.00 | 2.28 | 2.02 | 2.32 | 2.08 | 1.03 | 1.04 | 1.02 | 1.03 | 1st bending horizontal |
| 2 | 3.50 | 2.75 | 3.56 | 2.88 | 3.62 | 2.89 | 1.03 | 1.05 | 1.02 | 1.00 | 1st bending vertical |
| 3 | 5.00 | 4.50 | 5.16 | 4.52 | 5.01 | 4.72 | 1.00 | 1.05 | 0.97 | 1.04 | 1st torsional |
| 4 | 6.25 | 5.50 | 6.20 | 5.52 | 7.13 | 6.03 | 1.14 | 1.10 | 1.15 | 1.09 | 2nd bending vertical |
| 5 | 10.00 | 7.75 | 9.98 | 7.70 | 9.7 | 8.76 | 0.97 | 1.13 | 0.97 | 1.14 | 2nd torsional |
| 6 | 13.79 | 12.50 | 13.60 | 12.25 | 13.52 | 12.05 | 0.98 | 0.96 | 0.99 | 0,98 | 3rd bending vertical |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kilikevičius, A.; Bačinskas, D.; Selech, J.; Matijošius, J.; Kilikevičienė, K.; Vainorius, D.; Ulbrich, D.; Romek, D. The Influence of Different Loads on the Footbridge Dynamic Parameters. Symmetry 2020, 12, 657. https://doi.org/10.3390/sym12040657
Kilikevičius A, Bačinskas D, Selech J, Matijošius J, Kilikevičienė K, Vainorius D, Ulbrich D, Romek D. The Influence of Different Loads on the Footbridge Dynamic Parameters. Symmetry. 2020; 12(4):657. https://doi.org/10.3390/sym12040657
Chicago/Turabian StyleKilikevičius, Artūras, Darius Bačinskas, Jaroslaw Selech, Jonas Matijošius, Kristina Kilikevičienė, Darius Vainorius, Dariusz Ulbrich, and Dawid Romek. 2020. "The Influence of Different Loads on the Footbridge Dynamic Parameters" Symmetry 12, no. 4: 657. https://doi.org/10.3390/sym12040657
APA StyleKilikevičius, A., Bačinskas, D., Selech, J., Matijošius, J., Kilikevičienė, K., Vainorius, D., Ulbrich, D., & Romek, D. (2020). The Influence of Different Loads on the Footbridge Dynamic Parameters. Symmetry, 12(4), 657. https://doi.org/10.3390/sym12040657








