Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
4.6
2.5
Journals
Atmosphere
Special Issues
Geometry in Meteorology and Climatology
share announcement

Geometry in Meteorology and Climatology

A special issue of Atmosphere (ISSN 2073-4433).

Deadline for manuscript submissions: closed (28 June 2024) | Viewed by 5270

Special Issue Editor

Dr. Robert Monjo

E-Mail Website
Guest Editor
1. Department of Algebra, Geometry and Topology, Complutense University of Madrid, 28040 Madrid, Spain
2. Climate Research Foundation-Fundación Para la Investigación del Clima (FIClima), 28013 Madrid, Spain
Interests: fractal geometry; climate change; extreme precipitation; earth system models; multi-scale analysis; astrophysics

Special Issue Information

Dear Colleagues,

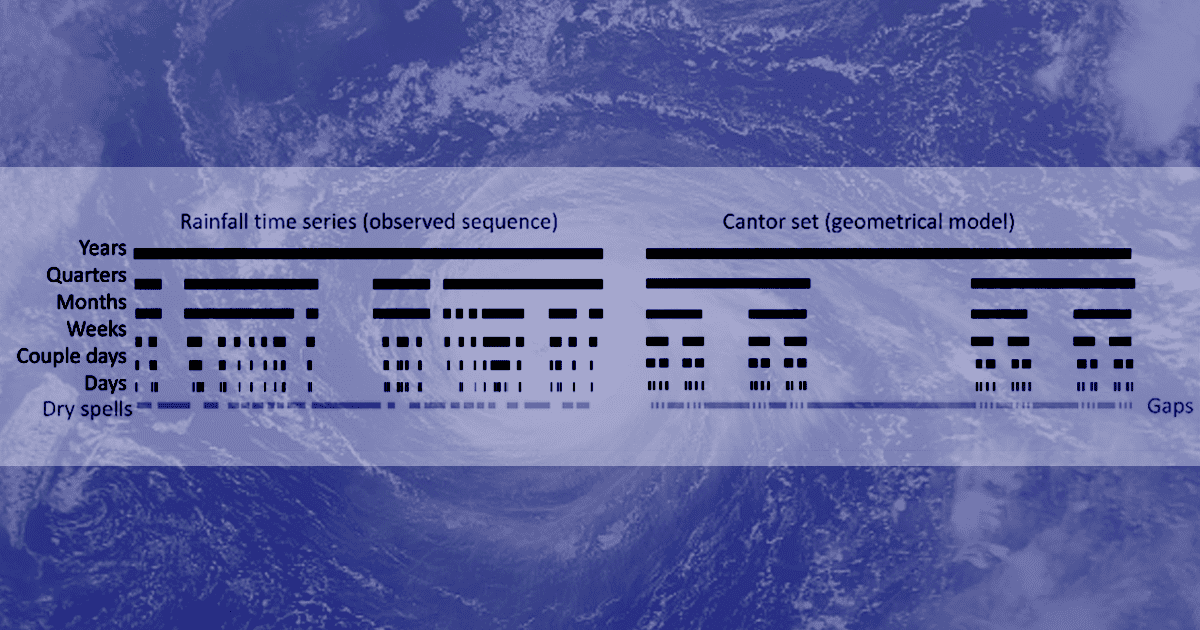
The atmosphere is a highly non-linear dynamic system, where equations can present some solutions known as strange attractors since they have non-integer dimensions, or in other words,  fractional volumes. The fractality in meteorology and climatology is reflected in the self-similarity at different spatial and temporal scales. For instance, atmospheric patterns present quasiperiodic oscillations that are reproduced at all spatial scales (from the eddy and the polar vortex to the Quasi-Biennial Oscillation and the Arctic Oscillation). Other examples are the disaggregation methods of sub-daily rainfall and the distribution of dry spells within a drought, which closely resemble the gaps in the Cantor set. Therefore, this Special Issue aims to review the state of the art and boost cutting-edge methods in “Geometry in Meteorology and Climatology” by collecting original contributions covering transdisciplinary approaches. Due to the strong link between atmospheric sciences and mathematics, this Special Issue will be focused on geometrical methods applied to characterize, analyze, predict or attribute physical phenomena linked to atmospheric dynamics and the effects. This includes characterization by geometrical approaches such as multifractal cascading, time scaling, rainfall concentration and drought lacunarity, as well as an empirical orthogonal basis of atmospheric patterns, principal components, compound events and extreme value theory of atmospheric variables. This Special Issue also accepts advanced methods for climate change attribution analysis, forensic meteorology and hindcast or operational forecasting that use geometrical perspectives such as performance metrics in supervised learning algorithms, similarity measures and analogue stratification, among others. Finally, the geometrical interpretation of time series analysis (breaking points, transitivity, information power, entropy, and Lyapunov and Hurst exponents) should also be adjusted to the scope when atmospheric variables are a key part of the papers.

Dr. Robert Monjo
Guest Editor

Manuscript Submission Information

Manuscripts should be submitted online at www.mdpi.com by registering and logging in to this website. Once you are registered, click here to go to the submission form. Manuscripts can be submitted until the deadline. All submissions that pass pre-check are peer-reviewed. Accepted papers will be published continuously in the journal (as soon as accepted) and will be listed together on the special issue website. Research articles, review articles as well as short communications are invited. For planned papers, a title and short abstract (about 100 words) can be sent to the Editorial Office for announcement on this website.

Submitted manuscripts should not have been published previously, nor be under consideration for publication elsewhere (except conference proceedings papers). All manuscripts are thoroughly refereed through a single-blind peer-review process. A guide for authors and other relevant information for submission of manuscripts is available on the Instructions for Authors page. Atmosphere is an international peer-reviewed open access monthly journal published by MDPI.

Please visit the Instructions for Authors page before submitting a manuscript. The Article Processing Charge (APC) for publication in this open access journal is 2400 CHF (Swiss Francs). Submitted papers should be well formatted and use good English. Authors may use MDPI's English editing service prior to publication or during author revisions.

Keywords

  • fractal
  • metrics
  • similarity
  • empirical orthogonal functions
  • dimension reduction
  • classification
  • time series analysis
  • supervised learning

Benefits of Publishing in a Special Issue

  • Ease of navigation: Grouping papers by topic helps scholars navigate broad scope journals more efficiently.
  • Greater discoverability: Special Issues support the reach and impact of scientific research. Articles in Special Issues are more discoverable and cited more frequently.
  • Expansion of research network: Special Issues facilitate connections among authors, fostering scientific collaborations.
  • External promotion: Articles in Special Issues are often promoted through the journal's social media, increasing their visibility.
  • e-Book format: Special Issues with more than 10 articles can be published as dedicated e-books, ensuring wide and rapid dissemination.

Further information on MDPI's Special Issue polices can be found here.

Published Papers (4 papers)

Download All Papers
Order results
Result details
Show export options expand_more Show export options expand_less
Select all
Export citation of selected articles as:

Research

Jump to: Review

13 pages, 3522 KiB  
Article
Geometry of Non-Diffusive Tracer Transport in Gridded Atmospheric Models
by Robert McGraw and Tamanna Subba
Atmosphere 2024, 15(10), 1151; https://doi.org/10.3390/atmos15101151 - 25 Sep 2024
Viewed by 629
Abstract
A first-order linear and numerically non-diffusive Eulerian transport algorithm, minVAR, was recently developed for preservation of correlations between interrelated tracers during advective transport. The present study extends this work by: (1) providing further investigation of several interesting geometric constructions found in contours of [...] Read more.
A first-order linear and numerically non-diffusive Eulerian transport algorithm, minVAR, was recently developed for preservation of correlations between interrelated tracers during advective transport. The present study extends this work by: (1) providing further investigation of several interesting geometric constructions found in contours of constant minVAR, short for minimum variance, through extension to three coordinate dimensions. These contours capture point-by-point representations of thousands of individual atmospheric aerosol and/or cloud particles as they evolve and are rendered on Eulerian grids at a level of sub-grid resolution limited only by numerical precision; and (2) exploration of geometric similarities between the Arakawa C-grid, used to obtain interpolated values of the wind field at grid scale and minVAR. In particular, we consider interpolation of the u and v horizontal components of wind velocity from grid to sub-grid scales. The last results are motivated by recent applications of the Weather Research and Forecasting (WRF) model applied in the coastal Houston region, where the recent TRacking Aerosol Convection Interactions ExpeRiment (TRACER) field campaign was organized. A unique and fully consistent mapping is obtained between particles moving along meteorological wind trajectories and the non-diffusive, non-dispersive representation of such trajectories on an Eulerian grid. Full article
(This article belongs to the Special Issue Geometry in Meteorology and Climatology)
►▼ Show Figures

Figure 1

22 pages, 3406 KiB  
Article
Short Review of Current Numerical Developments in Meteorological Modelling
by Jürgen Steppeler
Atmosphere 2024, 15(7), 830; https://doi.org/10.3390/atmos15070830 - 10 Jul 2024
Viewed by 669
Abstract
This paper reviews current numerical developments for atmospheric modelling. Numerical atmospheric modelling now looks back to a history of about 70 years after the first successful numerical prediction. Currently, we face new challenges, such as variable and adaptive resolution and ultra-highly resolving global [...] Read more.
This paper reviews current numerical developments for atmospheric modelling. Numerical atmospheric modelling now looks back to a history of about 70 years after the first successful numerical prediction. Currently, we face new challenges, such as variable and adaptive resolution and ultra-highly resolving global models of 1 km grid length. Large eddy simulation (LES), special applications like the numerical prediction of pollution and atmospheric contaminants belong to the current challenges of numerical developments. While pollution prediction is a standard part of numerical modelling in case of accidents, models currently being developed aim at modelling pollution at all scales from the global to the micro scale. The methods discussed in this paper are spectral elements and other versions of Local-Galerkin (L-Galerkin) methods. Classic numerical methods are also included in the presentation. For example, the rather popular second-order Arakawa C-grid method can be shown to result as a special case of an L-Galerkin method using low-order basis functions. Therefore, developments for Galerkin methods also apply to this classic C-grid method, and this is included in this paper. The new generation of highly parallel computers requires new numerical methods, as some of the classic methods are not well suited for a high degree of parallel computing. It will be shown that some numerical inaccuracies need to be resolved and this indicates a potential for improved results by going to a new generation of numerical methods. The methods considered here are mostly derived from basis functions. Such methods are known under the names of Galerkin, spectral, spectral element, finite element or L-Galerkin methods. Some of these new methods are already used in realistic models. The spectral method, though highly used in the 1990s, is currently replaced by the mentioned local L-Galerkin methods. All methods presented in this review have been tested in idealized numerical situations, the so-called toy models. Waypoints on the way to realistic models and their mathematical problems will be pointed out. Practical problems of informatics will be highlighted. Numerical error traps of some current numerical approaches will be pointed out. These are errors not occurring with highly idealized toy models. Such errors appear when the test situation becomes more realistic. For example, many tests are for regular resolution and results can become worse when the grid becomes irregular. On the sphere no regular grids exist, except for the five derived from Platonic solids. Practical problems beyond mathematics on the way to realistic applications will also be considered. A rather interesting and convenient development is the general availability of computer power. For example, the computational power available on a normal personal computer is comparable to that of a supercomputer in 2005. This means that interesting developments, such as the small sphere atmosphere with a resolution of 1 km and a spherical circumference between 180 and 360 km are available to the normal owner of a personal computer (PC). Besides the mathematical problems of new approaches, we will also consider the informatics challenges of using the new generation of models on mainframe computers and PCs. Full article
(This article belongs to the Special Issue Geometry in Meteorology and Climatology)
►▼ Show Figures

Figure 1

20 pages, 1014 KiB  
Article
Temporal Distribution of Extreme Precipitation in Barcelona (Spain) under Multi-Fractal n-Index with Breaking Point
by Benoît Gacon, David Santuy and Darío Redolat
Atmosphere 2024, 15(7), 804; https://doi.org/10.3390/atmos15070804 - 4 Jul 2024
Viewed by 804
Abstract
Rainfall regimes are experiencing variations due to climate change, and these variations are adequately simulated by Earth System Models at a daily scale for most regions. However, there are not enough raw outputs to study extreme and sub-daily precipitation patterns on a local [...] Read more.
Rainfall regimes are experiencing variations due to climate change, and these variations are adequately simulated by Earth System Models at a daily scale for most regions. However, there are not enough raw outputs to study extreme and sub-daily precipitation patterns on a local scale. To address this challenge, Monjo developed the n-index by characterizing the intensity and concentration of precipitation based on mono-fractal theory. In this study, we explore the use of a multi-fractal approach to establish a more accurate method of time scaling useful to study extreme precipitation events at a finer temporal resolution. This study was carried out on the reference station of Barcelona (Spain) and its surroundings in order to be representative of the Mediterranean climate. For return periods between 2 and 50 years, two variables were analyzed: the n-index and the reference intensity I0. Moreover, a new parameter, the so-called “breaking point”, was designed here to describe the reference intensity I0, which is predominant for low time ranges. The results showed that both parameters are dependent on the time steps and the return period, and the scores confirmed the validity of our approach. Finally, the n-index was projected under downscaled CMIP6 climate scenarios by 2100, showing a sustained increase of up to +10%. Full article
(This article belongs to the Special Issue Geometry in Meteorology and Climatology)
►▼ Show Figures

Graphical abstract

Review

Jump to: Research

24 pages, 1942 KiB  
Review
Review: Fractal Geometry in Precipitation
by Robert Monjo and Oliver Meseguer-Ruiz
Atmosphere 2024, 15(1), 135; https://doi.org/10.3390/atmos15010135 - 22 Jan 2024
Cited by 1 | Viewed by 2133
Abstract
Rainfall, or more generally the precipitation process (flux), is a clear example of chaotic variables resulting from a highly nonlinear dynamical system, the atmosphere, which is represented by a set of physical equations such as the Navier–Stokes equations, energy balances, and the hydrological [...] Read more.
Rainfall, or more generally the precipitation process (flux), is a clear example of chaotic variables resulting from a highly nonlinear dynamical system, the atmosphere, which is represented by a set of physical equations such as the Navier–Stokes equations, energy balances, and the hydrological cycle, among others. As a generalization of the Euclidean (ordinary) measurements, chaotic solutions of these equations are characterized by fractal indices, that is, non-integer values that represent the complexity of variables like the rainfall. However, observed precipitation is measured as an aggregate variable over time; thus, a physical analysis of observed fluxes is very limited. Consequently, this review aims to go through the different approaches used to identify and analyze the complexity of observed precipitation, taking advantage of its geometry footprint. To address the review, it ranges from classical perspectives of fractal-based techniques to new perspectives at temporal and spatial scales as well as for the classification of climatic features, including the monofractal dimension, multifractal approaches, Hurst exponent, Shannon entropy, and time-scaling in intensity–duration–frequency curves. Full article
(This article belongs to the Special Issue Geometry in Meteorology and Climatology)
►▼ Show Figures

Figure 1

Show export options expand_more Show export options expand_less
Select all
Export citation of selected articles as:
 
Displaying articles 1-4
Back to TopTop