Numerical Investigation of Thermally Developing and Fully Developed Electro-Osmotic Flow in Channels with Rounded Corners
Abstract
1. Introduction
2. Mathematical Model
2.1. Electric Potential Field
2.2. Velocity Field
2.3. Temperature Field
3. Materials and Methods
3.1. Numerical Modeling
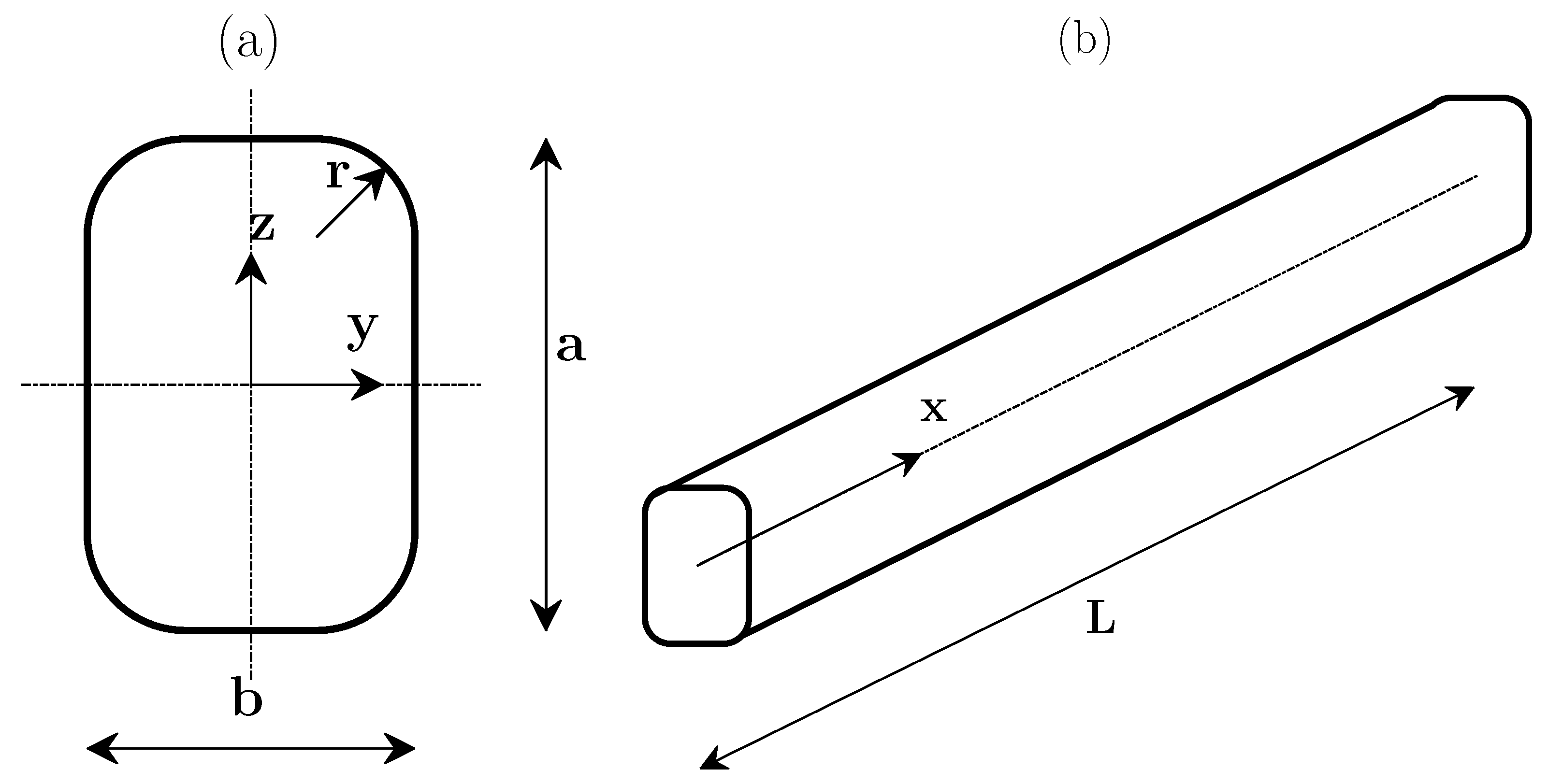
3.2. Problem Setup
- aspect ratio of the channel cross section;
- nondimensional smoothing radius ;
- nondimensional Stern potential ;
- nondimensional parameter , defined by Equation (2), which affects the electric potential field;
- nondimensional electric field , which causes fluid motion;
- nondimensional heat source Q due to Joule heating; and
- reference Peclet number,where is the reference velocity, calculated through Equation (A6).
3.3. Grid Independence Analysis
4. Results and Discussion
4.1. Model Validation
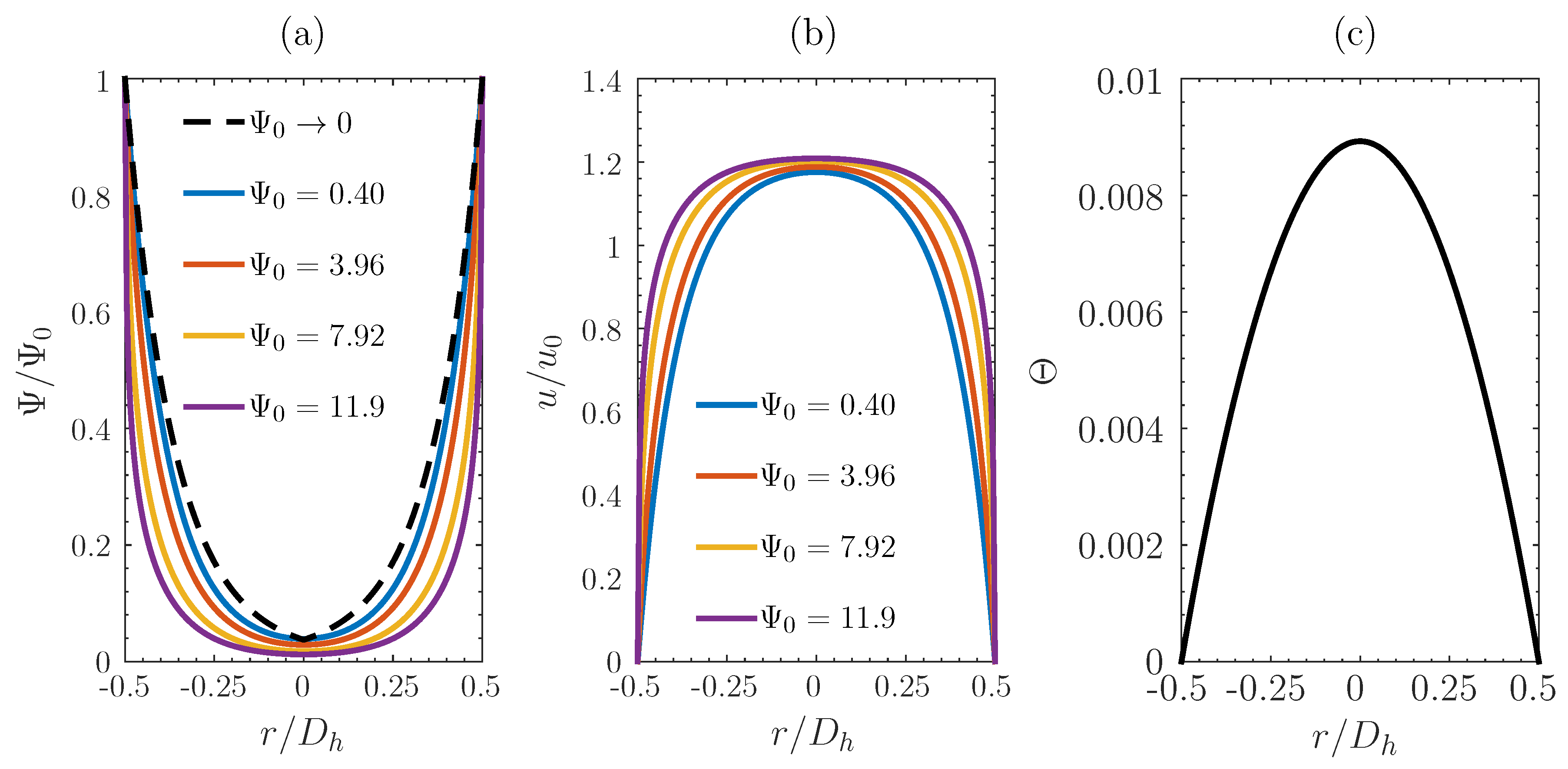
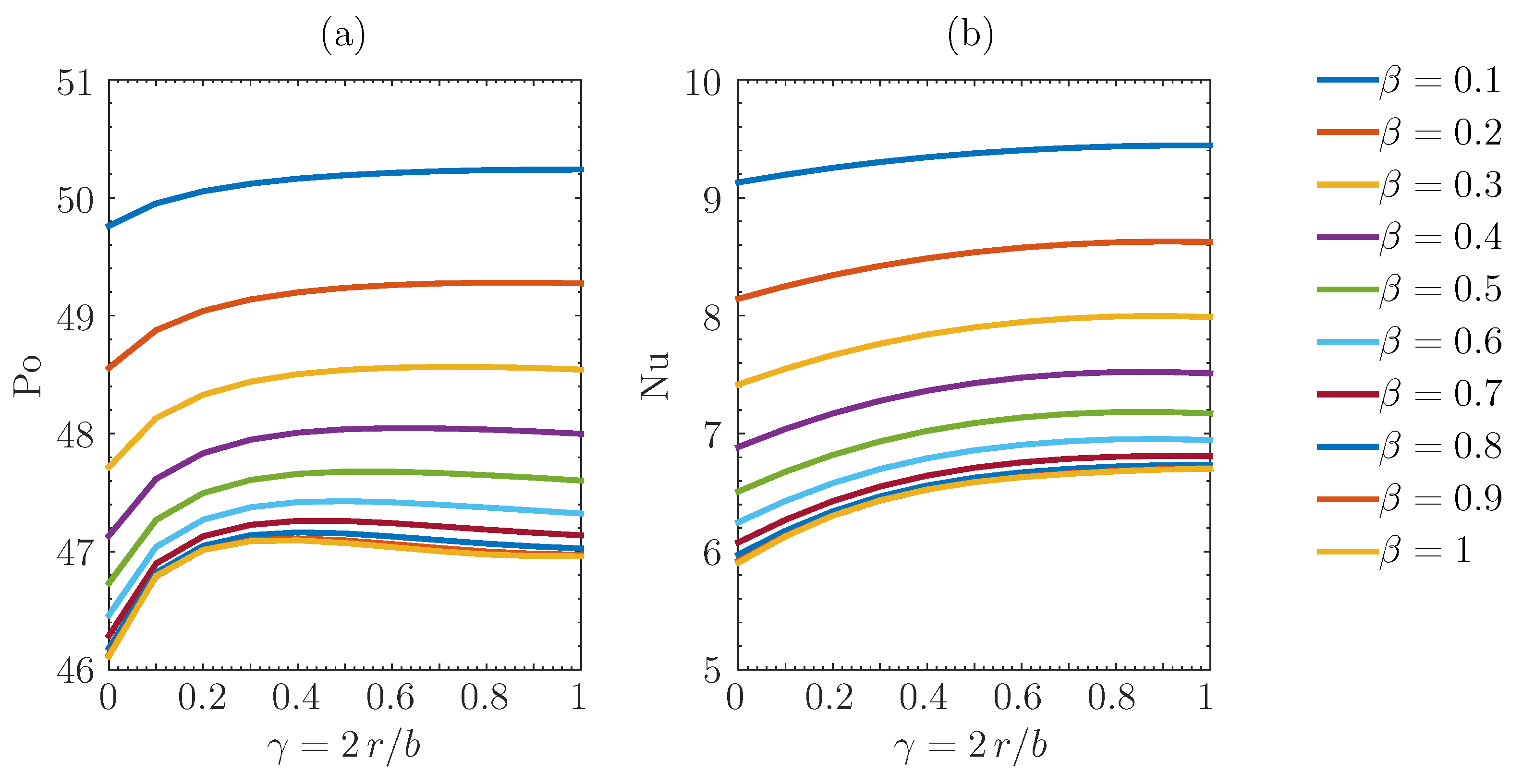
4.2. Fully Developed Flow Solution
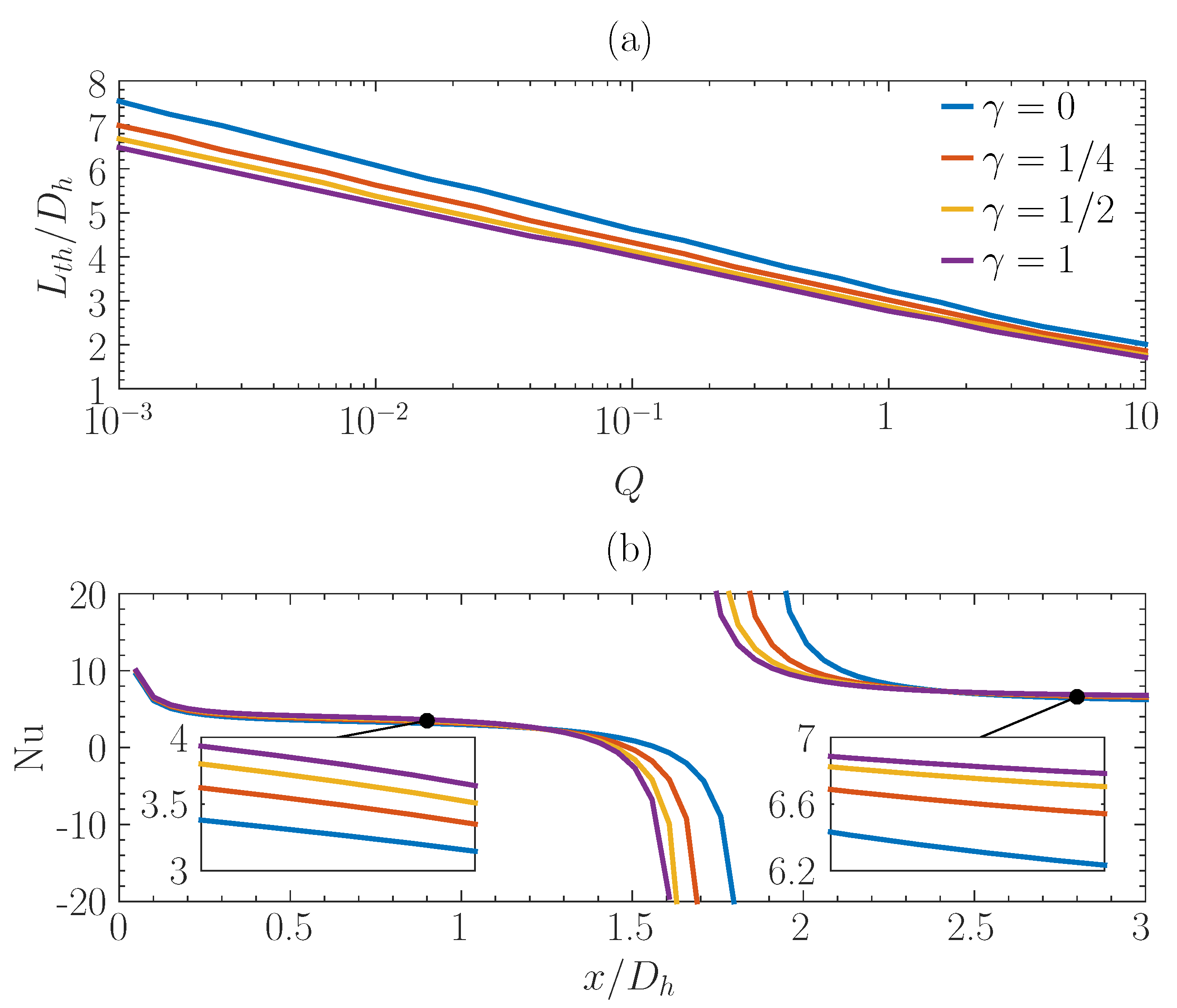
4.3. Graetz Problem Solution
- close to the entry section, the contribution of Joule heating is still negligible and the heat transfer process is mainly driven by conduction and convection, which is the most profitable operating condition for a microchannel heat sink;
- looking at the mean Nusselt number along the channel axis in Figure 8, a vertical asymptote at is observed, corresponding to the change in sign of the fluid bulk temperature;
- for , the heat generation due to Joule heating drives the heat transfer process and the microchannel heat sink is no longer able to dissipate heat from the walls; and
- the thermal entry length is reached at .
- smoothing the corners shortens the thermal entry length, and, as a consequence, the efficacious channel length (i.e., useful to remove heat from the walls) at high Joule heating, that is for high values of Q;
- heat transfer performance decreases when sharp corners are considered, since lower temperature gradients are observed close to them, meaning that lower Nusselt numbers over the entry region are reached. As an example, in Figure 9 for Nu goes from 3.95 for (maximum smoothing) to 3.38 for (sharp corners);
- small changes in correspond to significant variations of at different values of the smoothing radius .
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Specific heat capacity at constant pressure | J·kgK | |
| Reference hydraulic diameter | m | |
| Hydraulic diameter | m | |
| e | Unit electron charge | C |
| E | Electric field component | V·m |
| Electric field | V·m | |
| Nondimensional electric field | - | |
| Current density vector | A·m | |
| Boltzmann Constant | J·K | |
| Debye–Hückel parameter | m | |
| Grid spacing parameter | - | |
| L | Channel length | m |
| Thermal entry length | m | |
| Adiabatic channel length | m | |
| Number of nodes | - | |
| Number of triangular elements | - | |
| Nusselt number | - | |
| Peclet number | - | |
| Prandtl number | - | |
| Joule heating | W·m | |
| Q | Quantity defined by Equation (A11) | - |
| R | Nondimensional radial coordinate | - |
| Reynolds number | - | |
| T | Temperature | K |
| u | Velocity component along the x-axis | m·s |
| Velocity vector | m·s | |
| U | Nondimensional velocity | - |
| x | Axial coordinate | m |
| Nondimensional coordinate vector | - | |
| X | Nondimensional x-coordinate | - |
| Y | Nondimensional y-coordinate | - |
| Ion valence | - | |
| Z | Nondimensional z-coordinate | - |
| Greek letters | ||
| Aspect ratio of the channel | - | |
| Nondimensional smoothing radius | - | |
| Cross-sectional heated perimeter (nondimensional) | - | |
| Nondimensional perimeter | - | |
| Absolute permittivity | F·m | |
| thermal conductivity | W·mK | |
| fluid viscosity | Pa·s | |
| Mobility factor | mVs | |
| Nondimensional cross-sectional area | - | |
| P | Power | W |
| electric potential | V | |
| nondimensional electric potential | - | |
| nondimensional Stern potential | - | |
| Fluid density | kg·m | |
| electric charge density | C·m | |
| electric conductivity | S·m | |
| Nondimensional temperature | K | |
| Stern potential | V | |
| Subscripts | ||
| b | Bulk | |
| i | Inlet | |
| Thermal | ||
| w | Wall | |
| x | Component along the x-direction | |
| ∞ | Fully developed |
Appendix A
Appendix A.1. Electric Potential Field
Appendix A.2. Velocity Field
Appendix A.3. Temperature Field
References
- Tuckerman, D.; Pease, R. High-Performance Heat Sinking for VLSI. IEEE Electron. Device Lett. 1981, 2, 126–129. [Google Scholar] [CrossRef]
- Schubert, K.; Brandner, J.; Fichtner, M.; Linder, G.; Schygulla, U.; Wenka, A. Microstructure devices for applications in thermal and chemical process engineering. Microscale Thermophys. Eng. 2001, 5, 17–39. [Google Scholar]
- Rostami, A.; Mujumdar, A.; Saniei, N. Flow and heat transfer for gas flowing in microchannels: A review. Heat Mass Transf. Stoffuebertragung 2002, 38, 359–367. [Google Scholar] [CrossRef]
- Venkatesan, S.; Jerald, J.; Asokan, P.; Prabakaran, R. A Comprehensive Review on Microfluidics Technology and its Applications. Lect. Notes Mech. Eng. 2020, 235–245. [Google Scholar] [CrossRef]
- Gilmore, N.; Timchenko, V.; Menictas, C. Microchannel cooling of concentrator photovoltaics: A review. Renew. Sustain. Energy Rev. 2018, 90, 1041–1059. [Google Scholar] [CrossRef]
- Hossan, M.; Dutta, D.; Islam, N.; Dutta, P. Review: Electric field driven pumping in microfluidic device. Electrophoresis 2018, 39, 702–731. [Google Scholar] [CrossRef] [PubMed]
- Tuckerman, D.; Pease, R.; Guo, Z.; Hu, J.; Yildirim, O.; Deane, G.; Wood, L. Microchannel heat transfer: Early history, commercial applications, and emerging opportunities. In Proceedings of the ASME 2011 9th International Conference on Nanochannels, Microchannels, and Minichannels, Edmonton, AB, Canada, 19–22 June 2011; Volume 2, pp. 739–756. [Google Scholar] [CrossRef]
- Kuznetsov, V. Fundamental Issues Related to Flow Boiling and Two-Phase Flow Patterns in Microchannels–Experimental Challenges and Opportunities. Heat Transf. Eng. 2019, 40, 711–724. [Google Scholar] [CrossRef]
- Morini, G.L.; Lorenzini, M.; Colin, S.; Geoffroy, S. Experimental investigation of the compressibility effects on the friction factor of gas flows in microtubes. In Proceedings of the 4th International Conference on Nanochannels, Microchannels and Minichannels, ICNMM2006, Limerick, Ireland, 19–21 June 2006; Volume 47608, pp. 411–418. [Google Scholar]
- Cavazzuti, M.; Corticelli, M.; Karayiannis, T. Compressible Fanno flows in micro-channels: An enhanced quasi-2D numerical model for laminar flows. Therm. Sci. Eng. Prog. 2019, 10, 10–26. [Google Scholar] [CrossRef]
- Cavazzuti, M.; Corticelli, M.; Karayiannis, T. Compressible Fanno flows in micro-channels: An enhanced quasi-2D numerical model for turbulent flows. Int. Commun. Heat Mass Transf. 2020, 111. [Google Scholar] [CrossRef]
- Morini, G.; Yang, Y.; Lorenzini, M. Experimental analysis of gas micro-convection through commercial microtubes. Exp. Heat Transf. 2012, 25, 151–171. [Google Scholar] [CrossRef]
- Cavazzuti, M.; Corticelli, M. Numerical modelling of Fanno flows in micro channels: A quasi-static application to air vents for plastic moulding. Therm. Sci. Eng. Prog. 2017, 2, 43–56. [Google Scholar] [CrossRef]
- Yang, Y.; Chalabi, H.; Lorenzini, M.; Morini, G. The effect on the nusselt number of the nonlinear axial temperature distribution of gas flows through microtubes. Heat Transf. Eng. 2014, 35, 159–170. [Google Scholar] [CrossRef]
- Kockmann, N.; Holvey, C.; Roberge, D. Transitional flow and related transport phenomena in complex microchannels. In Proceedings of the 7th International Conference on Nanochannels, Microchannels, and Minichannels 2009, ICNMM2009, Pohang, Korea, 22–24 June 2009; Volume 43499, pp. 1301–1312. [Google Scholar]
- Lorenzini, M.; Daprá, I.; Scarpi, G. Heat Transfer for a Giesekus Fluid in a Rotating Concentric Annulus. Appl. Therm. Eng. 2017, 122, 118–125. [Google Scholar] [CrossRef]
- Ohadi, M.; Choo, K.; Dessiatoun, S.; Cetegen, E.E. Next Generation Microchannel Heat Exchangers; Springer Briefs in Applied Sciences and Technology; Springer: New York, NY, USA; Berlin/Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Han, Y.; Liu, Y.; Li, M.; Huang, J. A review of development of micro-channel heat exchanger applied in air-conditioning system. Energy Procedia 2012, 14, 148–153. [Google Scholar] [CrossRef]
- Kew, P.; Reay, D. Compact/micro-heat exchangers - Their role in heat pumping equipment. Appl. Therm. Eng. 2011, 31, 594–601. [Google Scholar] [CrossRef][Green Version]
- Henning, T.; Brandner, J.; Schubert, K.; Lorenzini, M.; Morini, G. Low-frequency instabilities in the operation of metallic multi-microchannel evaporators. Heat Transf. Eng. 2007, 28, 834–841. [Google Scholar] [CrossRef]
- Keepaiboon, C.; Dalkilic, A.; Mahian, O.; Ahn, H.; Wongwises, S.; Mondal, P.; Shadloo, M. Two-phase flow boiling in a microfluidic channel at high mass flux. Phys. Fluids 2020, 32. [Google Scholar] [CrossRef]
- Wei, T.W.; Oprins, H.; Fang, L.; Cherman, V.; De Wolf, I.; Beyne, E.; Baelmans, M. Nozzle scaling effects for the thermohydraulic performance of microjet impingement cooling with distributed returns. Appl. Therm. Eng. 2020, 180. [Google Scholar] [CrossRef]
- Morini, G.; Spiga, M. The role of the viscous dissipation in heated microchannels. J. Heat Transf. 2007, 129, 308–318. [Google Scholar] [CrossRef]
- Kirby, B. Micro- and Nanoscale Fluid Mechanics; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Bruus, H. Theoretical Microfluidics; OUP: Oxford, UK, 2010. [Google Scholar]
- Al-Rjoub, M.F.; Roy, A.K.; Ganguli, S.; Banerjee, R.K. Assessment of an active-cooling micro-channel heat sink device, using electro-osmotic flow. Int. J. Heat Mass Transf. 2011, 54, 4560–4569. [Google Scholar] [CrossRef]
- Rice, C.; Whitehead, R. Electrokinetic flow in a narrow cylindrical capillary. J. Phys. Chem. 1965, 69, 4017–4024. [Google Scholar] [CrossRef]
- Mala, G.; Li, D.; Werner, C.; Jacobasch, H.J.; Ning, Y. Flow characteristics of water through a microchannel between two parallel plates with electrokinetic effects. Int. J. Heat Fluid Flow 1997, 18, 489–496. [Google Scholar] [CrossRef]
- Wang, C.Y.; Chang, C.C. Electro-osmotic flow in polygonal ducts. Electrophoresis 2011, 32, 1268–1272. [Google Scholar] [CrossRef] [PubMed]
- Vocale, P.; Geri, M.; Cattani, L.; Morini, G.; Spiga, M. Electro-osmotic heat transfer in elliptical microchannels under H1 boundary condition. Int. J. Therm. Sci. 2013, 72, 92–101. [Google Scholar] [CrossRef]
- Vocale, P.; Geri, M.; Morini, G.; Spiga, M. Electro-osmotic flows inside triangular microchannels. J. Phys. Conf. Ser. 2014, 501, 012026. [Google Scholar] [CrossRef]
- Geri, M.; Lorenzini, M.; Morini, G. Effects of the Channel Geometry and of the Fluid Composition on the Performance of DC Electro-osmotic Pumps. Int. J. Therm. Sci. 2012, 55, 114–121. [Google Scholar] [CrossRef]
- Morini, G.L.; Lorenzini, M.; Salvigni, S.; Spiga, M. Thermal performance of silicon micro heat-sinks with electrokinetically-driven flows. In Proceedings of the 3rd International Conference on Microchannels and Minichannels, Toronto, ON, Canada, 13–15 June 2005; Volume B, pp. 231–236. [Google Scholar]
- Al-Rjoub, M.; Roy, A.; Ganguli, S.; Banerjee, R. Enhanced electro-osmotic flow pump for micro-scale heat exchangers. In Proceedings of the ASME 2012 3rd International Conference on Micro/Nanoscale Heat and Mass Transfer, MNHMT 2012, Atlanta, GA, USA, 3–6 March 2012; pp. 829–833. [Google Scholar]
- Pramod, K.; Sen, A. Flow and heat transfer analysis of an electro-osmotic flow micropump for chip cooling. J. Electron. Packag. Trans. ASME 2014, 136, 03101201–03201214. [Google Scholar] [CrossRef]
- Shamshiri, M.; Khazaeli, R.; Ashrafizaadeh, M.; Mortazavi, S. Electroviscous and thermal effects on non-Newtonian liquid flows through microchannels. J. Non-Newton. Fluid Mech. 2012, 173–174, 1–12. [Google Scholar] [CrossRef]
- Shit, G.; Mondal, A.; Sinha, A.; Kundu, P. Electro-osmotic flow of power-law fluid and heat transfer in a micro-channel with effects of Joule heating and thermal radiation. Phys. A Stat. Mech. Its Appl. 2016, 462, 1040–1057. [Google Scholar] [CrossRef]
- Al-Rjoub, M.; Roy, A.; Ganguli, S.; Banerjee, R. Enhanced heat transfer in a micro-scale heat exchanger using nano-particle laden electro-osmotic flow. Int. Commun. Heat Mass Transf. 2015, 68, 228–235. [Google Scholar] [CrossRef]
- Ray, S.; Misra, D. Laminar fully developed flow through square and equilateral triangular ducts with rounded corners subjected to H1 and H2 boundary conditions. Int. J. Therm. Sci. 2010, 49, 1763–1775. [Google Scholar] [CrossRef]
- Chakraborty, S.; Ray, S. Performance optimisation of laminar fully developed flow through square ducts with rounded corners. Int. J. Therm. Sci. 2011, 50, 2522–2535. [Google Scholar] [CrossRef]
- Lorenzini, M.; Morini, G. Single-phase, Laminar Forced Convection in Microchannels with Rounded Corners. Heat Transf. Eng. 2011, 32, 1108–1116. [Google Scholar] [CrossRef]
- Lorenzini, M. The Influence of Viscous Dissipation on Thermal Performance of Microchannels with Rounded Corners. La Houille Blanche 2013, 64–71. [Google Scholar] [CrossRef]
- Lorenzini, M.; Suzzi, N. The influence of geometry on the thermal performance of microchannels in laminar flow with viscous dissipation. Heat Trans. Eng. 2016, 37, 1096–1104. [Google Scholar] [CrossRef]
- Lorenzini, M. Electro-osmotic Flow in Rectangular Microchannels: Geometry Optimisation. J. Phys. Conf. Ser. 2017, 923, 1–8. [Google Scholar] [CrossRef]
- Lorenzini, M. Electro-osmotic non-isothermal flow in rectangular channels with smoothed corners. J. Therm. Sci. Eng. Appl. 2020, 19, 100617. [Google Scholar] [CrossRef]
- Webb, R. Principles of Enhanced Heat Transfer; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Shah, R.K.; London, A.K. Laminar Flow Forced Convection in Ducts—A Source Book for Heat Exchanger Analytical Data; Academic Press: New York, NY, USA, 1978. [Google Scholar]
- Barletta, A.; Magyari, E. The Graetz-Brinkman problem in a plane-parallel channel with adiabatic-to-isothermal entrance. Int. Commun. Heat Mass 2006, 33, 677–685. [Google Scholar] [CrossRef]
- Suzzi, N.; Lorenzini, M. Viscous heating of a laminar flow in the thermal entrance region of a rectangular channel with rounded corners and uniform wall temperature. Int. J. Therm. Sci. 2019, 145, 106032. [Google Scholar] [CrossRef]
- Morini, G.L.; Lorenzini, M.; Salvigni, S.; Spiga, M. Thermal performance of silicon micro heat-sinks with electrokinetically-driven flows. Int. J. Therm. Sci. 2006, 45, 955–961. [Google Scholar] [CrossRef]
- Jian, Y.; Yang, L.; Liu, Q. Time periodic electro-osmotic flow through amicroannulus. Phys. Fluids 2010, 22, 042001. [Google Scholar] [CrossRef]
- Sadr, R.; Yoda, M.; Zheng, Z.; Conlisk, T. An experimental study of electro-osmotic flow in rectangular microchannels. J. Fluid Mech. 2004, 506, 357–367. [Google Scholar] [CrossRef]
- Zimparov, V. Extended performance evaluation criteria for enhanced heat transfer surfaces: Heat transfer through ducts with constant wall temperature. Int. J. Heat Mass Transf. 2000, 43, 3137–3155. [Google Scholar] [CrossRef]
- Zimparov, V. Extended performance evaluation criteria for enhanced heat transfer surfaces: Heat transfer through ducts with constant heat flux. Int. J. Heat Mass Transf. 2001, 44, 169–180. [Google Scholar] [CrossRef]
- Tabeling, P. Introduction to Microfluidics; Oxford University Press: Oxford, UK, 2010. [Google Scholar]
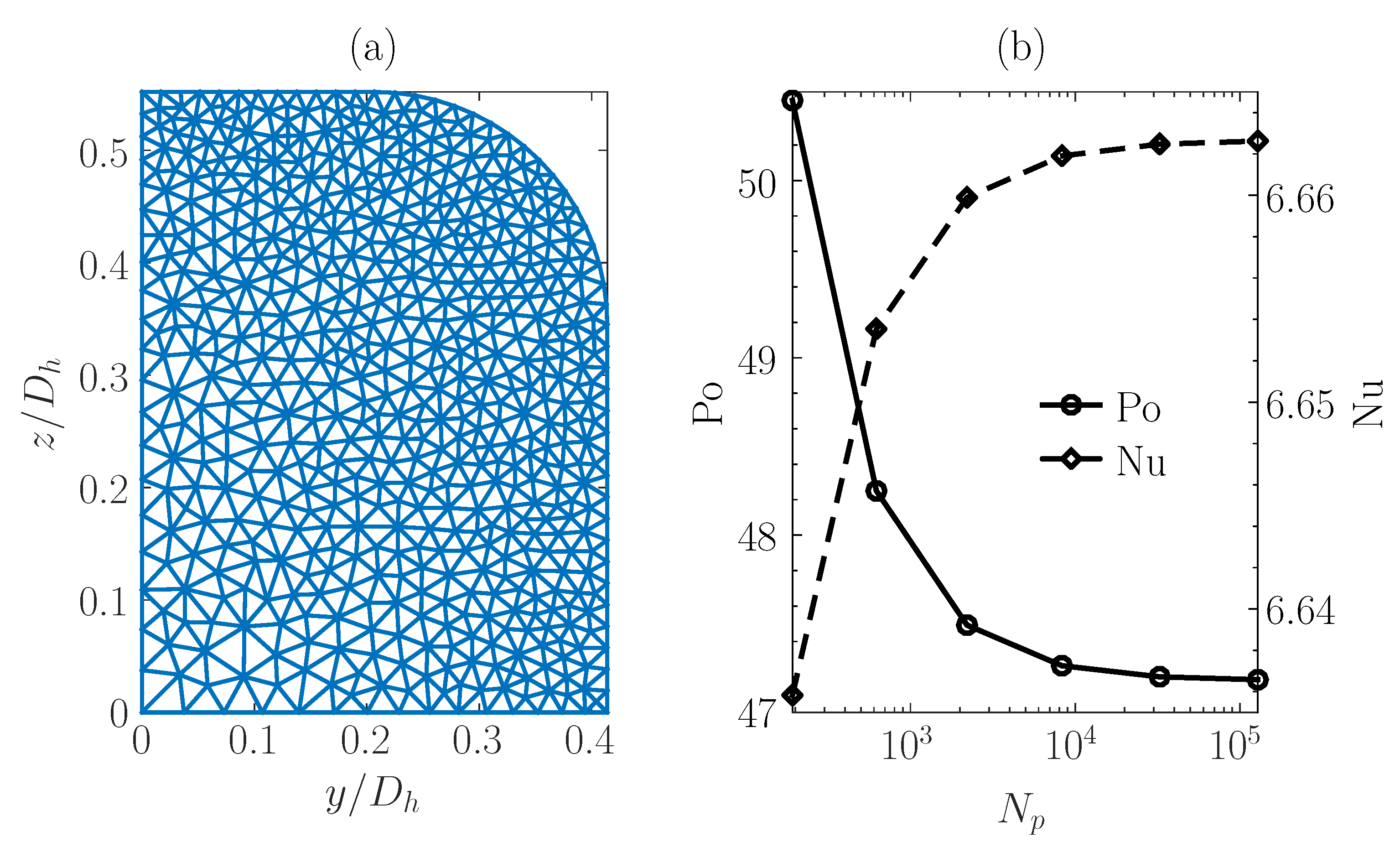



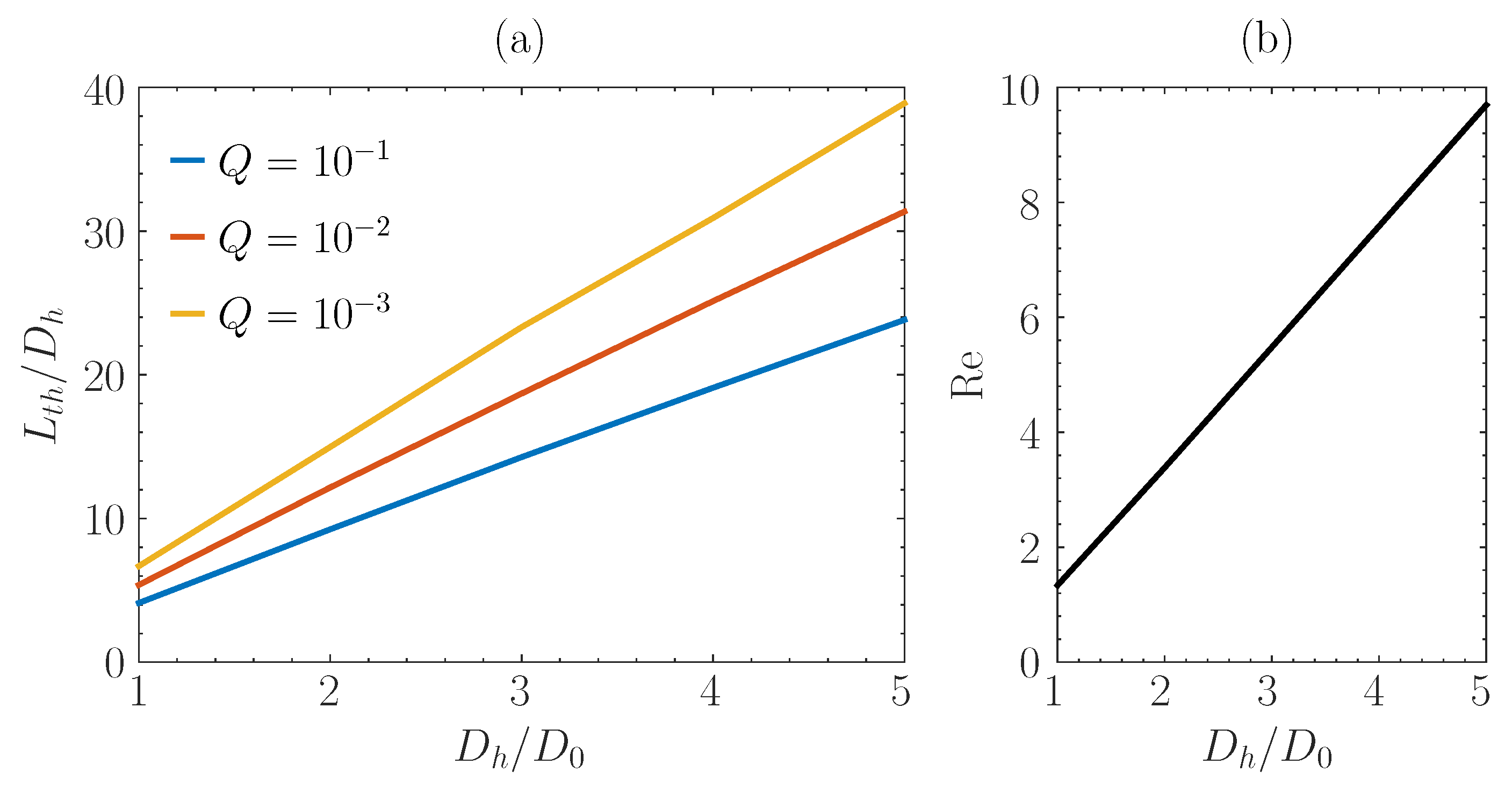
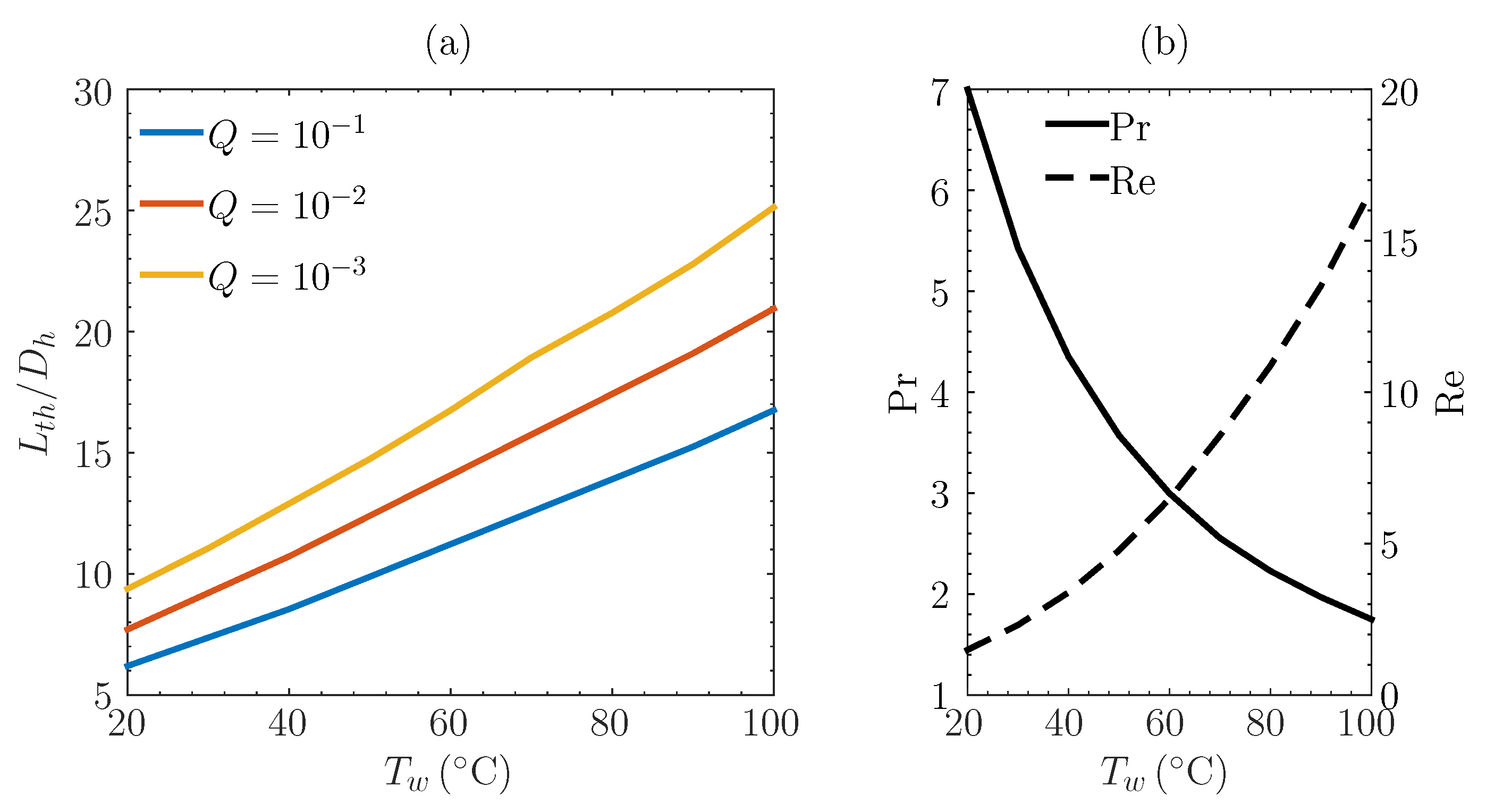
| 192 | 338 | 50.4520 | 6.635831 | |
| 621 | 1157 | 48.2496 | 6.653541 | |
| 2205 | 4248 | 47.4922 | 6.659893 | |
| 8309 | 16303 | 47.2633 | 6.661907 | |
| 32598 | 64571 | 47.2007 | 6.662473 | |
| 128655 | 256067 | 47.1843 | 6.662622 |
| [45] | |||
|---|---|---|---|
| 1 | 0 | ||
| 0 | |||
| 0 | |||
| 1 | 1 |
| Poiseuille Number | Nusselt Number | ||
|---|---|---|---|
| Equation (23) | Equation () | ||
| +50.8162 | +10.1790 | ||
| −14.0158 | −12.4539 | ||
| +14.6258 | +12.3036 | ||
| −5.19479 | −4.11363 | ||
| +3.59595 | +1.03360 | ||
| −6.58458 | −1.25171 | ||
| +3.66162 | +0.500750 | ||
| +2.01872 | +1.87850 | ||
| −1.29980 | −0.751240 | ||
| −0.761764 | −0.672452 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suzzi, N.; Lorenzini, M. Numerical Investigation of Thermally Developing and Fully Developed Electro-Osmotic Flow in Channels with Rounded Corners. Fluids 2021, 6, 22. https://doi.org/10.3390/fluids6010022
Suzzi N, Lorenzini M. Numerical Investigation of Thermally Developing and Fully Developed Electro-Osmotic Flow in Channels with Rounded Corners. Fluids. 2021; 6(1):22. https://doi.org/10.3390/fluids6010022
Chicago/Turabian StyleSuzzi, Nicola, and Marco Lorenzini. 2021. "Numerical Investigation of Thermally Developing and Fully Developed Electro-Osmotic Flow in Channels with Rounded Corners" Fluids 6, no. 1: 22. https://doi.org/10.3390/fluids6010022
APA StyleSuzzi, N., & Lorenzini, M. (2021). Numerical Investigation of Thermally Developing and Fully Developed Electro-Osmotic Flow in Channels with Rounded Corners. Fluids, 6(1), 22. https://doi.org/10.3390/fluids6010022






