Efficiency Improvement of Miniaturized Heat Exchangers
Abstract
1. Introduction
2. Materials and Methods
2.1. Fluids
2.2. Microstructure Designs
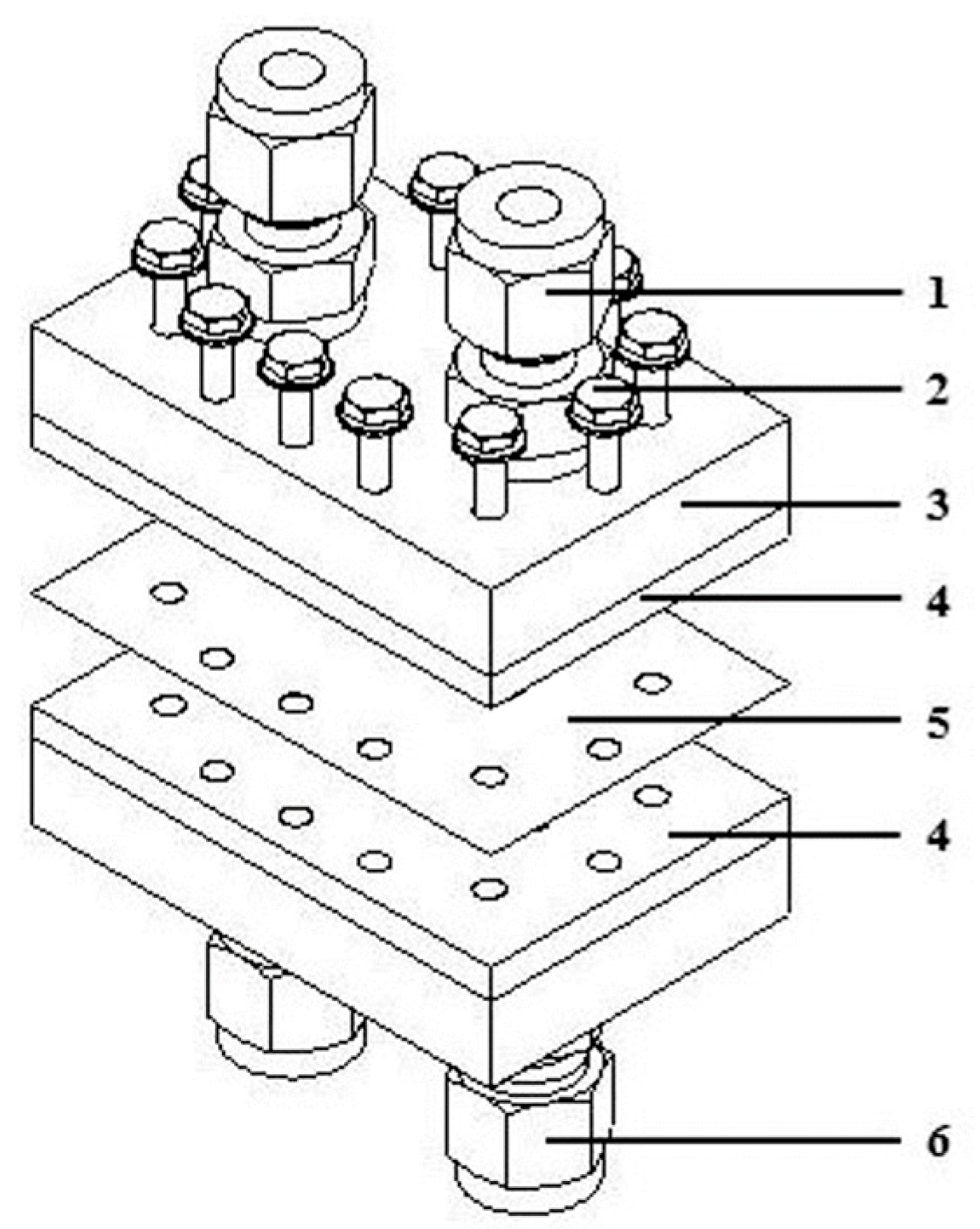
2.3. Assessment Method
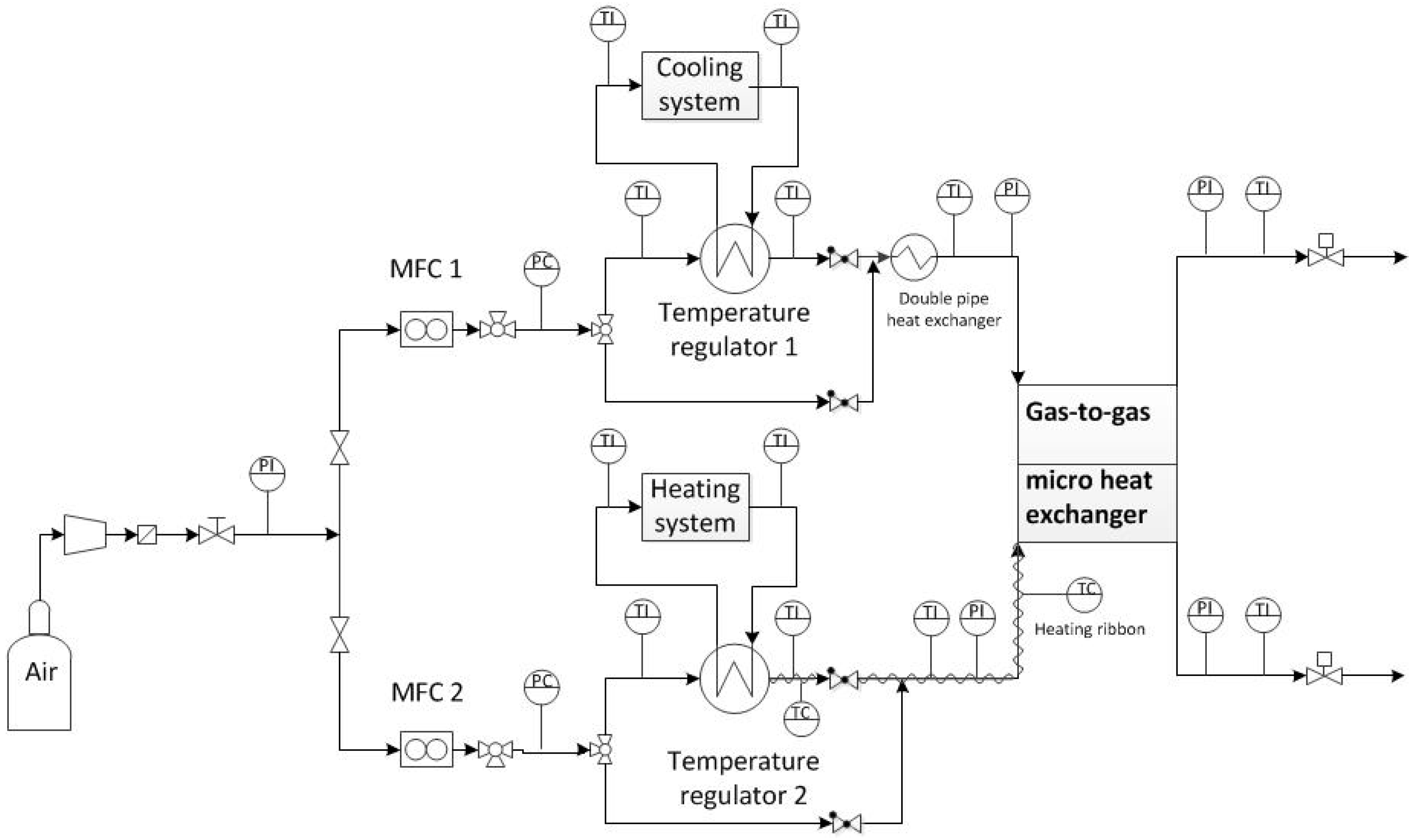
2.4. Experimental Procedure
3. Results
3.1. Straight Microchannels
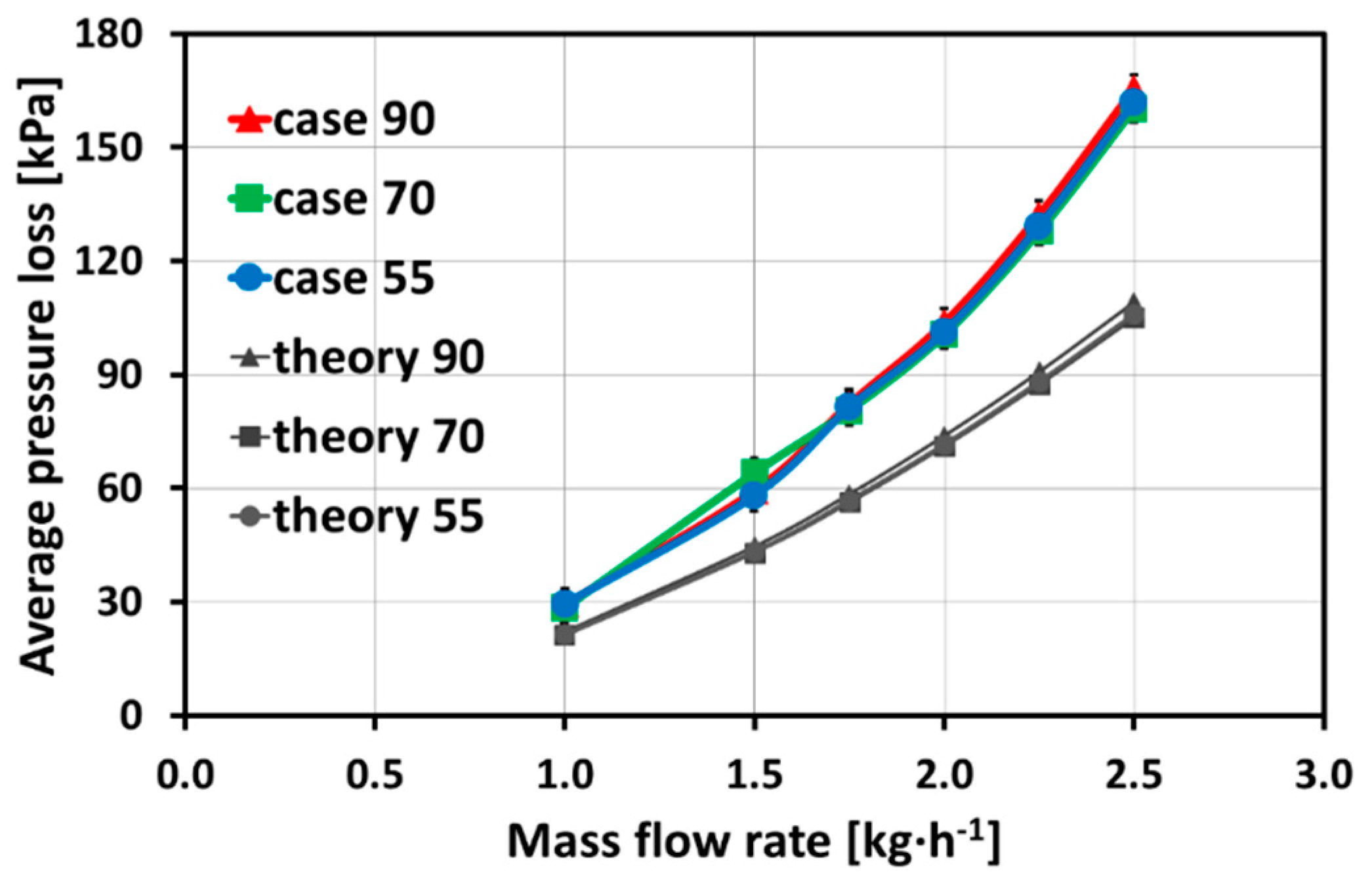
3.1.1. Pressure Loss Behavior
3.1.2. Heat Transfer Behavior
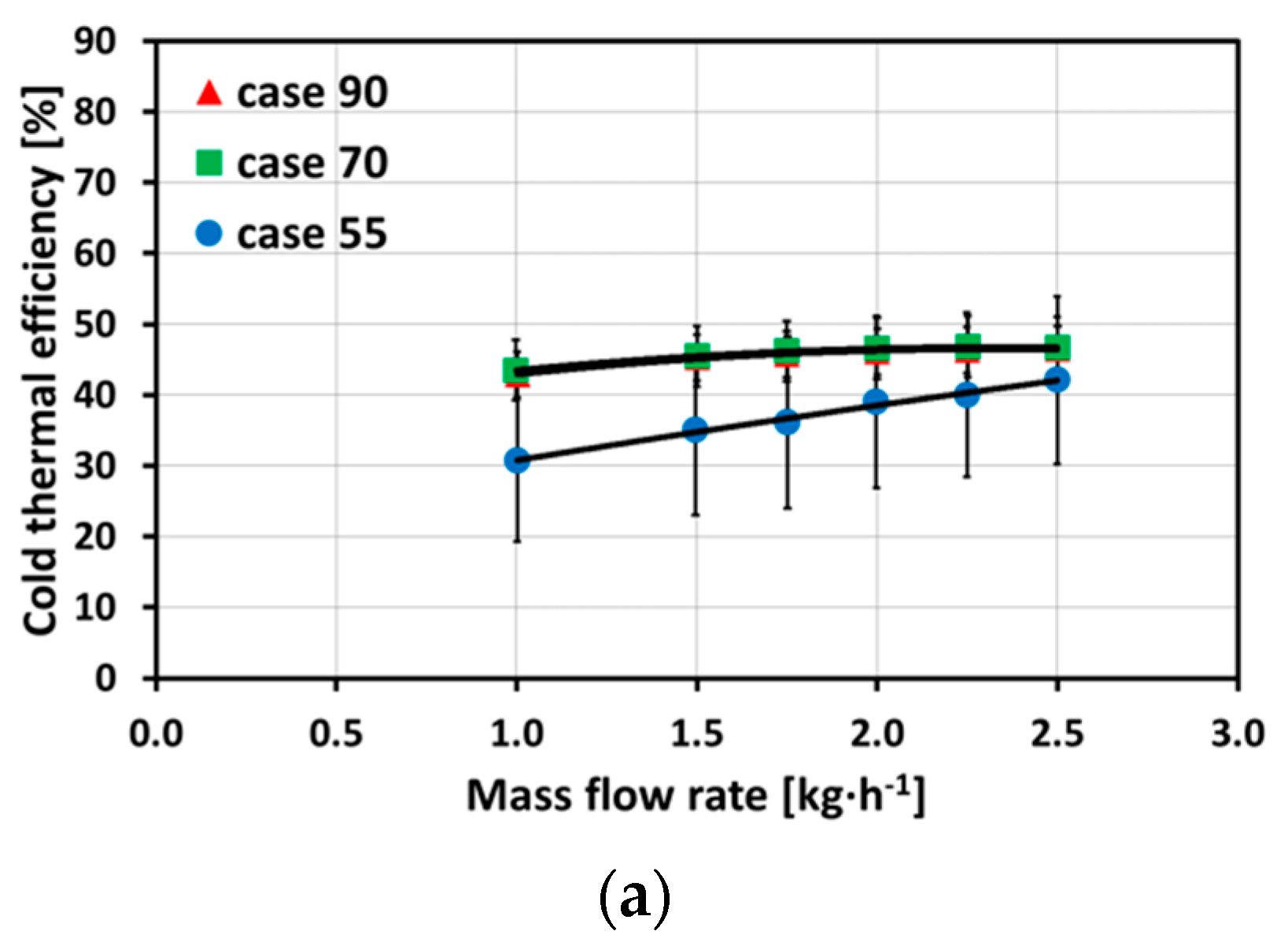
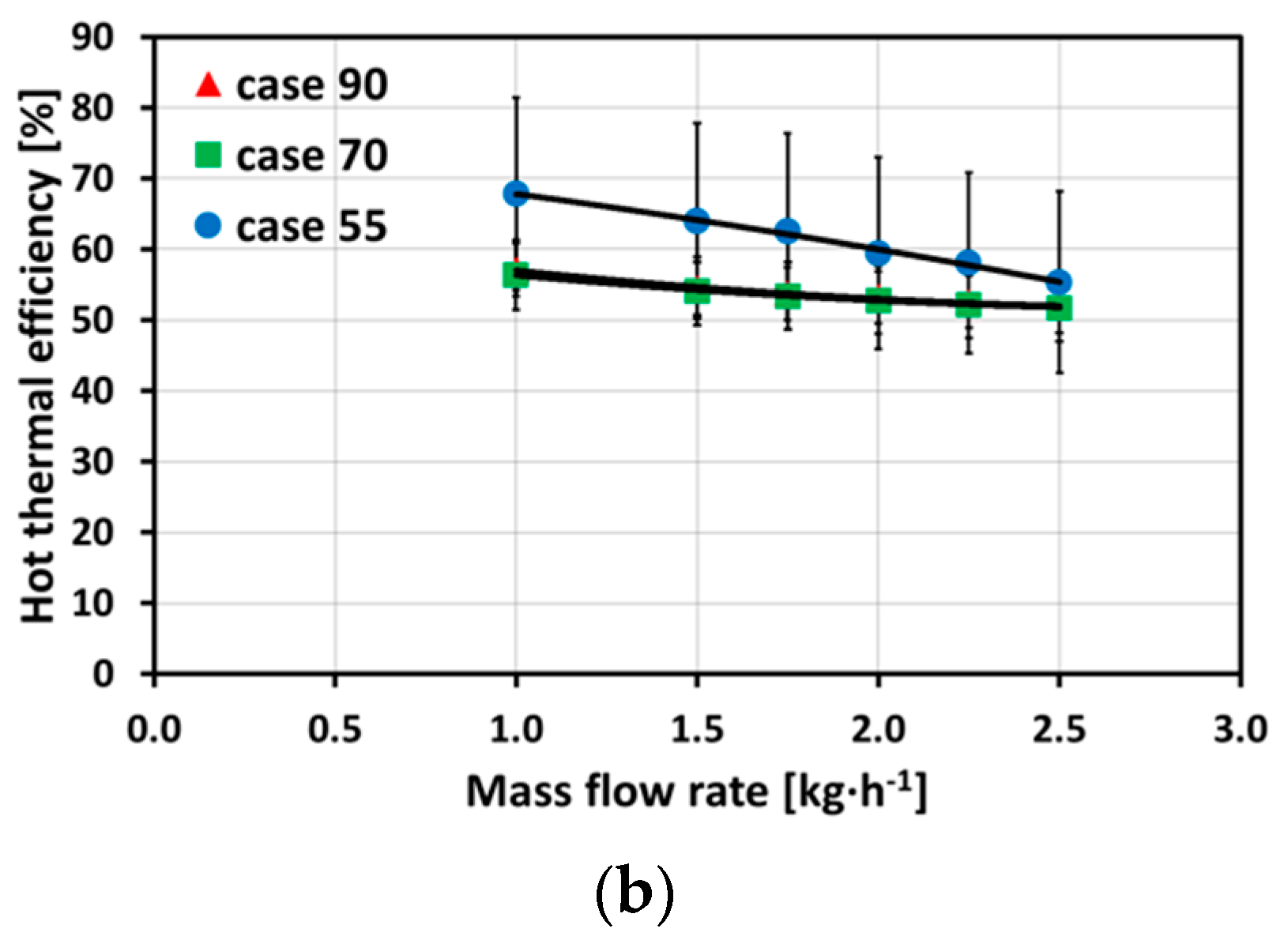
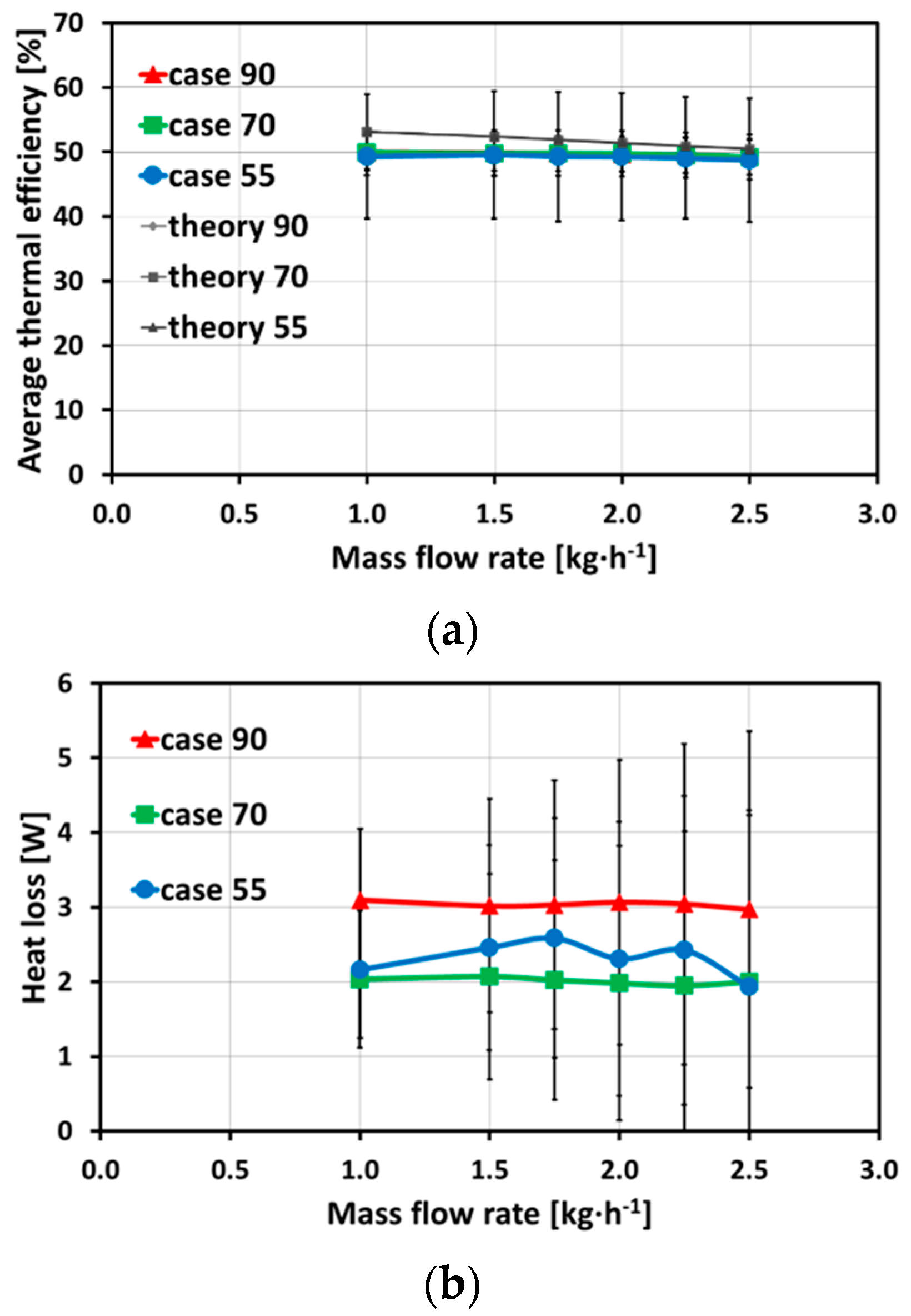
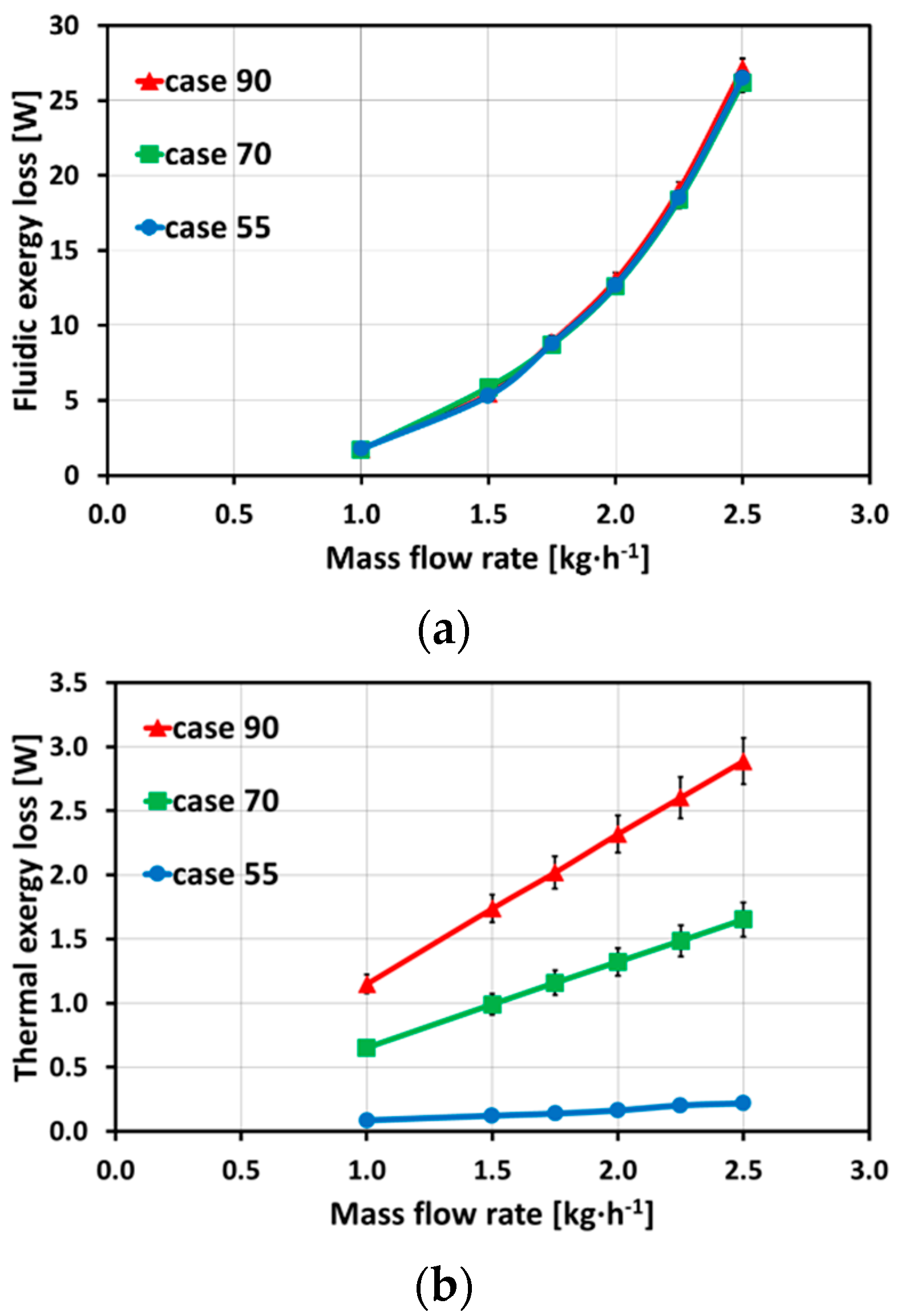
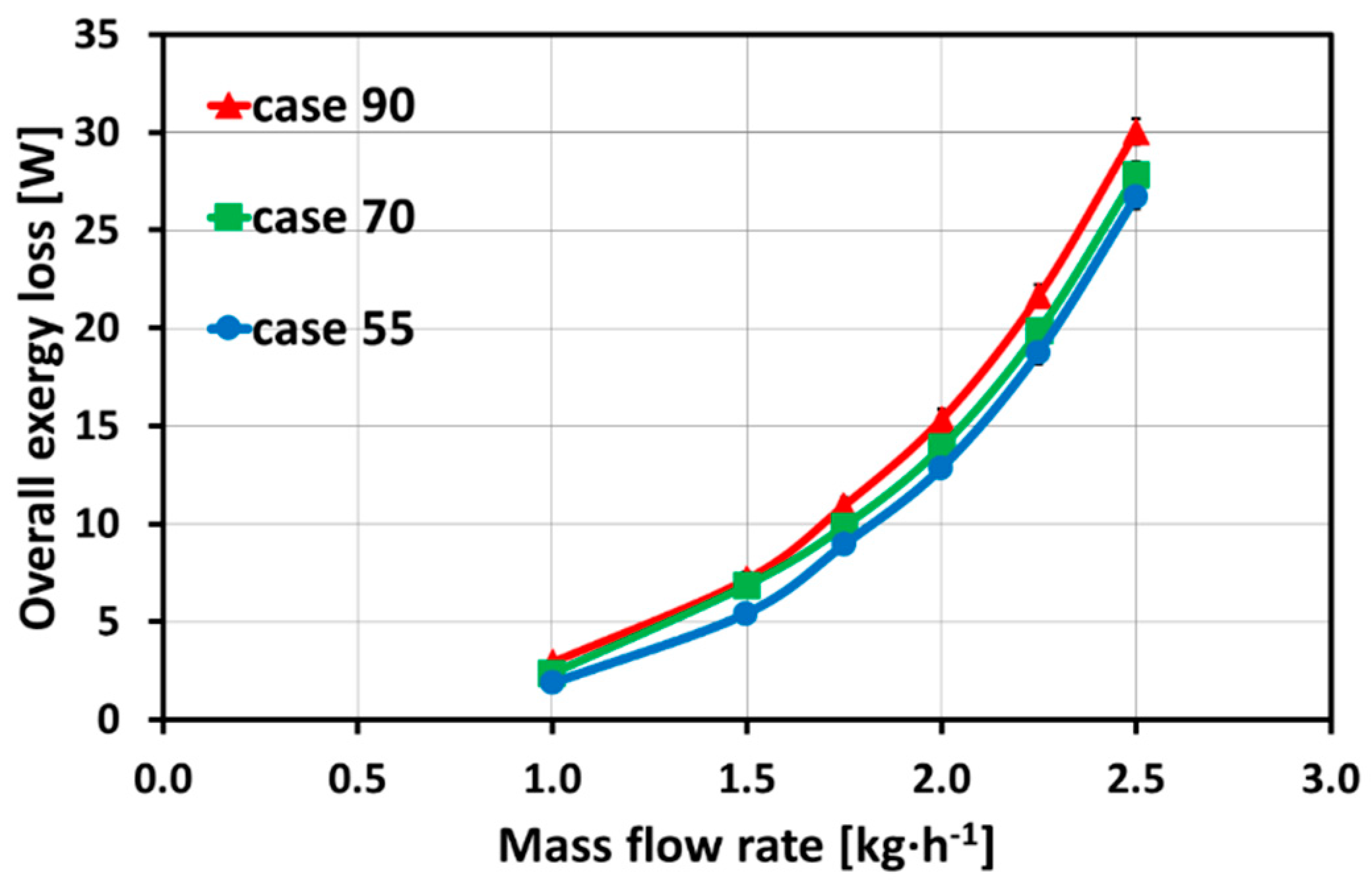
3.1.3. Overall Heat Exchanger Efficiency
3.2. Enhanced Microstructures
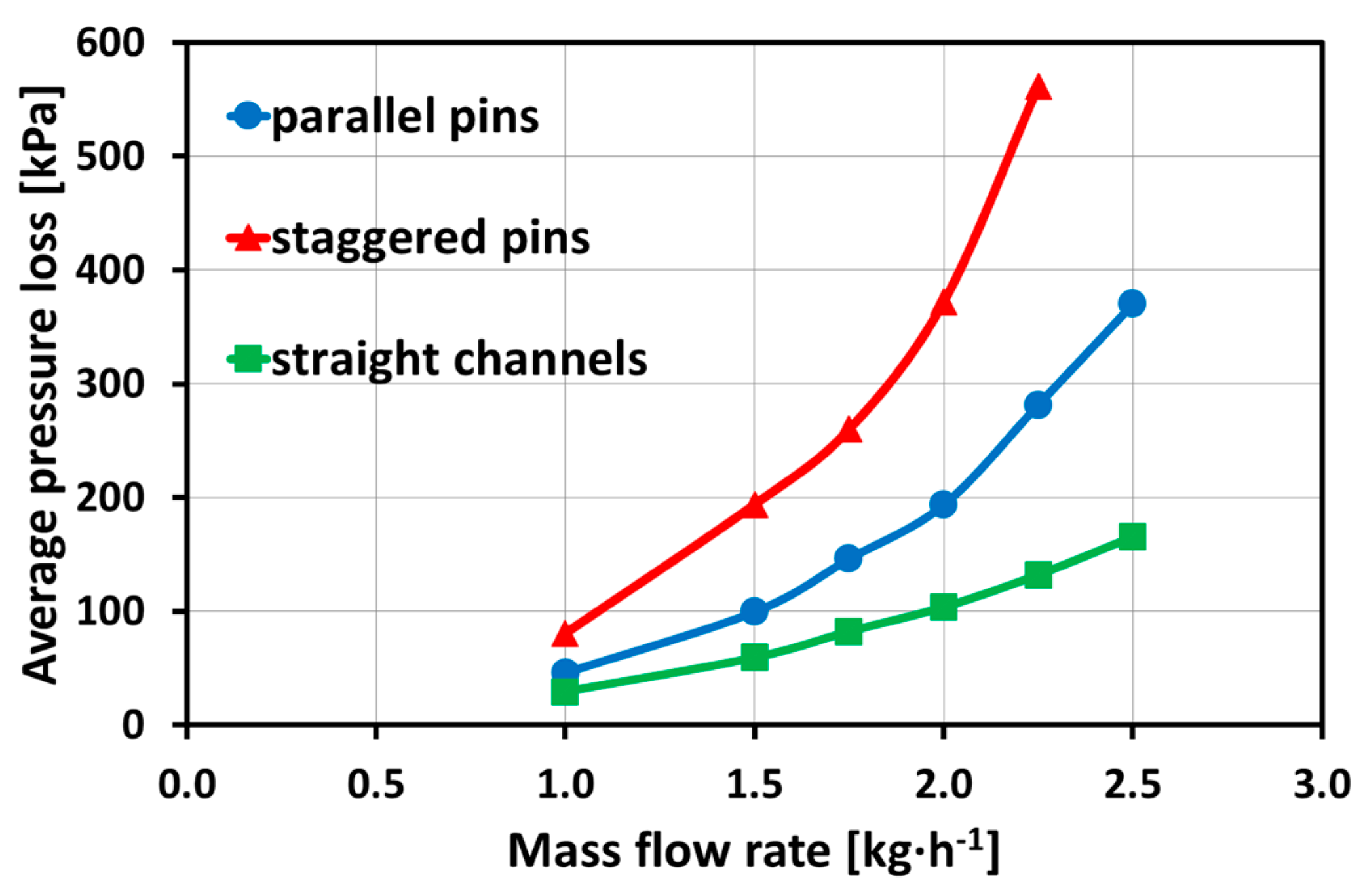
3.2.1. Pressure Loss Behavior
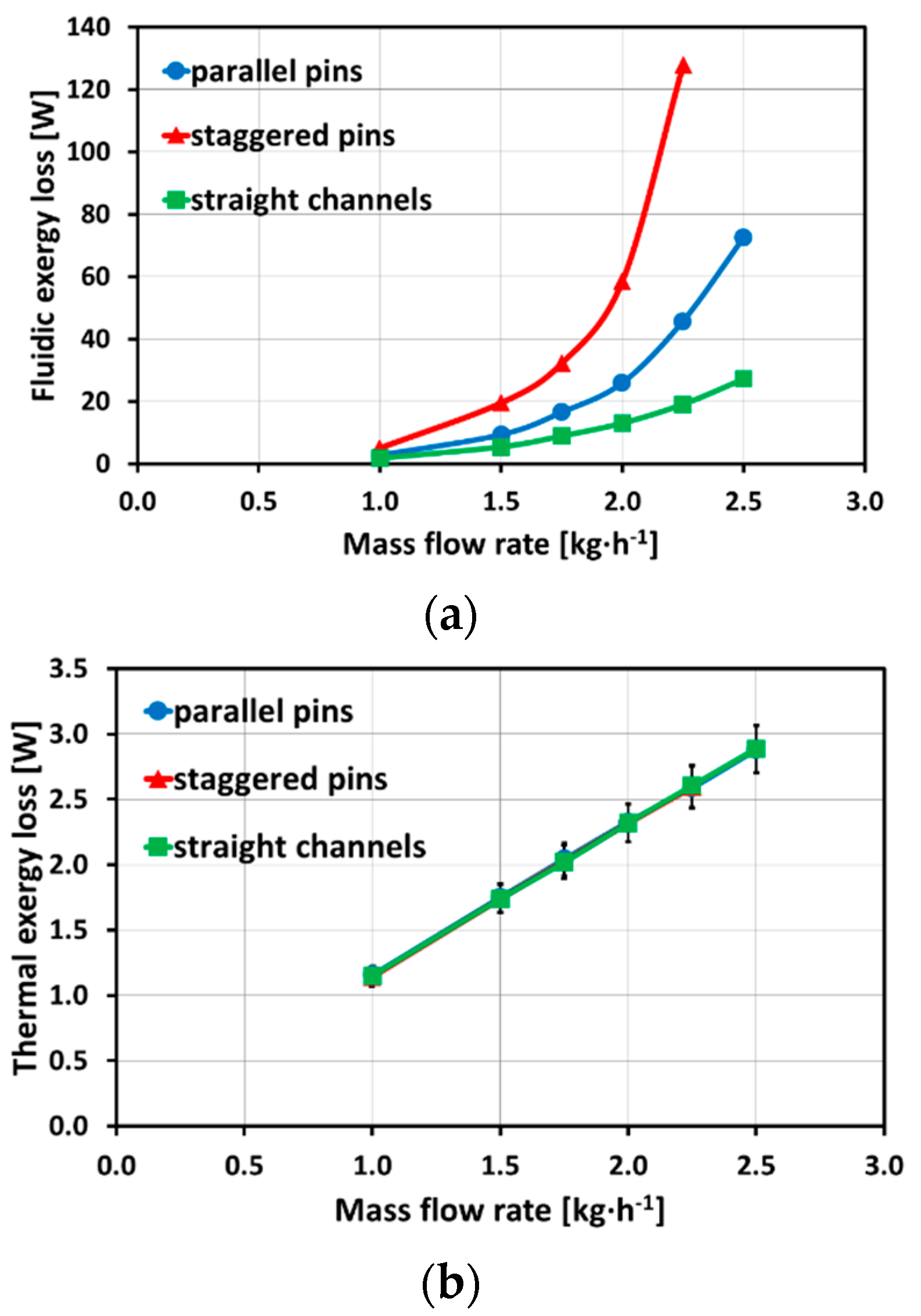
3.2.2. Heat Transfer Behavior
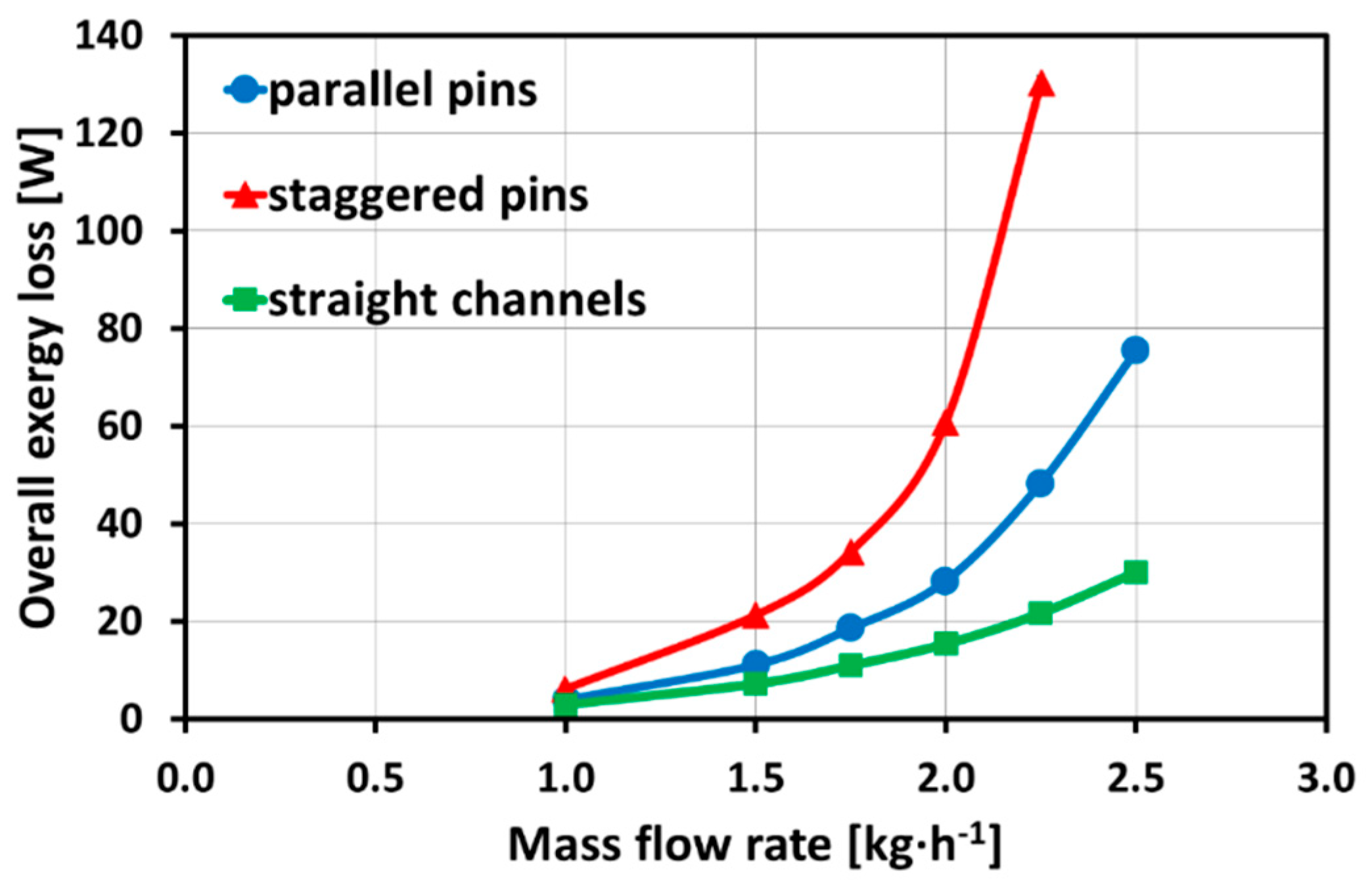
3.2.3. Overall Heat Transfer Efficiency
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tuckerman, B.D.; Pease, R.F. High performance heat sinking for VLSI. IEEE Electron. Device Lett. 1981, EDL-2, 126–129. [Google Scholar] [CrossRef]
- Brandner, J.J.; Bohn, L.; Henning, T.; Schygulla, U.; Schubert, K. Microstructure heat exchanger applications in laboratory and industry. Heat Transf. Eng. 2007, 28, 761–771. [Google Scholar] [CrossRef]
- Kandlikar, S.G.; Colin, S.; Peles, Y.; Garimella, S.; Pease, R.F.; Brandner, J.J.; Tuckerman, D.B. Heat Transfer in Microchannels—2012 Status and Research Needs. J. Heat Transf. 2013, 135, 091001. [Google Scholar] [CrossRef]
- Schubert, J.B.K. Microstructure devices for applications in thermal and chemical process engineering. Microscale Thermophys. Eng. 2001, 5, 17–39. [Google Scholar] [CrossRef]
- Joseph, J.; Delanaye, M.; Nacereddine, R.; Giraldo, A.; Rouabah, M.; Korvink, J.; Brandner, J.J. Numerical and experimental investigation of a wire-net compact heat exchanger performance for high-temperature applications. Appl. Therm. Eng. 2019, 154, 208–216. [Google Scholar] [CrossRef]
- Joseph, J.; Delanaye, M.; Nacereddine, R.; Giraldo, A.; Rouabah, M.; Korvink, J.G.; Brandner, J.J. Numerical Study of Perturbators Influence on Heat Transfer and Investigation of Collector Performance for a Micro-Combined Heat and Power System Application. Heat Transf. Eng. 2020, 1–23. [Google Scholar] [CrossRef]
- Joseph, J.; Nacereddine, R.; Delanaye, M.; Korvink, J.G.; Brandner, J.J. Advanced Numerical Methodology to Analyze High-Temperature Wire-Net Compact Heat Exchangers for a Micro-Combined Heat and Power System Application. Heat Transf. Eng. 2019, 41, 934–946. [Google Scholar] [CrossRef]
- Rehman, D.; Morini, G.L.; Hong, C. A comparison of data reduction methods for average friction factor calculation of adiabatic gas flows in microchannels. Micromachines 2020, 11, 218. [Google Scholar] [CrossRef]
- Brandner, J.J. Microstructure devices for process intensification: Influence of manufacturing tolerances and design. Appl. Therm. Eng. 2013, 59, 745–752. [Google Scholar] [CrossRef]
- Brandner, J.; Anurjew, E.; Bohn, L.; Hansjosten, E.; Henning, T.; Schygulla, U.; Wenka, A.; Schubert, K. Concepts and realization of microstructure heat exchangers for enhanced heat transfer. Exp. Therm. Fluid Sci. 2006, 30, 801–809. [Google Scholar] [CrossRef]
- Gerken, I.; Brandner, J.J.; Dittmeyer, R. Heat transfer enhancement with gas-to-gas micro heat exchangers. Appl. Therm. Eng. 2016, 93, 1410–1416. [Google Scholar] [CrossRef]
- Yang, Y.; Brandner, J.J.; Morini, G.L. Hydraulic and thermal design of a gas microchannel heat exchanger. J. Phys. Conf. Ser. 2012, 362, 362. [Google Scholar] [CrossRef]
- Yang, Y.; Gerken, I.; Brandner, J.J.; Morini, G.L. Design and Experimental Investigation of a Gas-to-Gas Counter-Flow Micro Heat Exchanger. Exp. Heat Transf. 2014, 27, 340–359. [Google Scholar] [CrossRef]
- Yang, Y.; Morini, G.; Brandner, J.J. Experimental analysis of the influence of wall axial conduction on gas-to-gas micro heat exchanger effectiveness. Int. J. Heat Mass Transf. 2014, 69, 17–25. [Google Scholar] [CrossRef]
- Bejan, A. General criterion for rating heat-exchanger performance. Int. J. Heat Mass Transf. 1978, 21, 655–658. [Google Scholar] [CrossRef]
- Bejan, A. The thermodynamic design of heat and mass transfer processes and devices. Int. J. Heat Fluid Flow 1987, 8, 258–276. [Google Scholar] [CrossRef]
- VDI Gesellschaft fuer Verfahrenstechnik und Chemieingenieurwesen, V.G.V. VDI Heat Atlas; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Hessel, V.; Renken, A.; Schouten, J.C.; Yoshida, J.I. Micro Process Engineering: A Comprehensive Handbook; John Wiley & Sons: Hoboken, NJ, USA, 2009; Volume 1. [Google Scholar]
- Brandner, J.J. Microfabrication in metals, ceramics and polymers. Russ. J. Gen. Chem. 2012, 82, 2025–2033. [Google Scholar] [CrossRef]
- Kockmann, N. Micro Process Engineering: Fundamentals, Devices, Fabrication, and Applications; Wiley-VCH: Weinheim, Germany, 2006. [Google Scholar]
- Madou, M.J. Fundamentals of Microfabrication: The Science of Miniaturization, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Colin, S. Gas Microflows in the Slip Flow Regime: A Critical Review on Convective Heat Transfer. J. Heat Transf. 2011, 134, 020908. [Google Scholar] [CrossRef]
- Turner, S.E.; Lam, L.C.; Faghri, M.; Gregory, O.J. Experimental Investigation of Gas Flow in Microchannels. J. Heat Transf. 2004, 126, 753–763. [Google Scholar] [CrossRef]
- Bier, W.; Keller, W.; Linder, G.; Seidel, D.; Schubert, K.; Martin, H. Gas to gas heat transfer in micro heat exchangers. Chem. Eng. Process. Process Intensif. 1993, 32, 33–43. [Google Scholar] [CrossRef]
- Morini, G.L.; Lorenzini, M.; Salvigni, S. Friction characteristics of compressible gas flows in microtubes. Exp. Therm. Fluid Sci. 2006, 30, 733–744. [Google Scholar] [CrossRef]
- Kee, R.J.; Almand, B.B.; Blasi, J.M.; Rosen, B.L.; Hartmann, M.; Sullivan, N.P.; Zhu, H.; Manerbino, A.R.; Menzer, S.; Coors, W.G.; et al. The design, fabrication, and evaluation of a ceramic counter-flow microchannel heat exchanger. Appl. Therm. Eng. 2011, 31, 2004–2012. [Google Scholar] [CrossRef]
- Steinke, M.; Kandlikar, S. Review of single-phase heat transfer enhancement techniques for application in microchannels, minichannels and microdevices. Int. J. Heat Technol. 2004, 22, 3–11. [Google Scholar]
- Webb, R.L.; Kim, N.-H. Principles of Enhanced Heat Transfer; Informa UK Limited: Colchester, UK, 2004. [Google Scholar]
- Sciacovelli, A.; Verda, V.; Sciubba, E. Entropy generation analysis as a design tool—A review. Renew. Sustain. Energy Rev. 2015, 43, 1167–1181. [Google Scholar] [CrossRef]
- Awad, M. A review of entropy generation in microchannels. Adv. Mech. Eng. 2015, 7, 1687814015590297. [Google Scholar] [CrossRef]
- Wu, S.; Li, Y. Exergy-economic criteria for evaluating heat exchanger performance. J. Therm. Sci. 2001, 10, 218–222. [Google Scholar] [CrossRef]
- International Bureau of Weights and Measures; International Federation of Clinical Chemistry and Laboratory Medicine; ISO. IUPAP and OIML. 2008, pp. 1–134. Available online: https://www.bipm.org/en/publications/guides/gum.html (accessed on 18 December 2020).
- Colin, S. Rarefaction and compressibility effects on steady and transient gas flows in microchannels. Microfluid. Nanofluidics 2004, 1, 268–279. [Google Scholar] [CrossRef]
- Gerken, I. Efficiency Improvement of Miniaturized Heat Exchangers. Ph.D. Thesis, Karlsruhe Institute of Technology (KIT), Karlsruhe, Germany, 2020. [Google Scholar]
- Renault, C.; Colin, S.; Orieux, S.; Cognet, P.; Tzedakis, T. Optimal design of multi-channel microreactor for uniform residence time distribution. Microsyst. Technol. 2011, 18, 209–223. [Google Scholar] [CrossRef][Green Version]


| Property (at 293.15 K and p = 100 kPa (rel)) | Unit | Air |
|---|---|---|
| Density | 1.19 | |
| Dynamic viscosity | Pa·s | 0.000018 |
| Kinematic viscosity | 0.000015 | |
| Specific heat capacity | 1006 | |
| Heat conductivity | 0.03 | |
| Prandtl number | – | 0.71 |
| Thermal Conductivity | Dynamic Viscosity | Specific Heat Capacity | Compressibility Factor | Density | ||
|---|---|---|---|---|---|---|
| (%) | (%) | Isobaric (%) | Isochoric (%) | (%) | (%) | |
| Maximum deviation from temperature of T = 273.15 K | 43.68 | 39.55 | 1.09 | 1.76 | 0.76 | 35.95 |
| Maximum deviation from pressure of p = 100 kPa (rel) | 1.40 | 0.81 | 1.79 | 0.39 | 0.50 | 904.70 |
| Microstructure Arrangement | Y |
|---|---|
| Straight channels | nchannel − 1 |
| Parallel pins | |
| Staggered pins |
| Straight Micro Channels | Parallel Pins | Staggered Pins | |
|---|---|---|---|
| Height (m) | 0.0001 | 0.0001 | 0.0001 |
| Dimensions of pins | |||
| Length (m) | 0.0235 | 0.0010 | 0.0010 |
| Width (m) | 0.0005 | 0.0005 | 0.0005 |
| Dimensions of pitch | |||
| Length (m) | 0.0235 | 0.0010 | 0.0010 |
| Width (m) | 0.0010 | 0.0010 | 0.0010 |
| Number of rows per column | 11 channels | 11 columns, 11 rows per column | 11 columns, 6 rows per column 10 columns, 5 rows per column |
| Heat transfer area (m2) | 0.00057 | 0.00067 | 0.00066 |
| Active volume (m3) | 2.6 × 10−8 | 3.1 × 10−8 | 3.1 × 10−8 |
| Operating Condition | Unit | Clamped Micro Heat Exchanger |
|---|---|---|
| Mass flow rate | 1.00, 1.50, 1.75, 2.00, 2.25, 2.50 | |
| Inlet pressure (hot and cold fluid flow) | kPa (rel) | 700 |
| Heat Exchanger | Fluid | Object of Investigation | Case Name | |
|---|---|---|---|---|
| Clamped micro heat exchanger | Air | Straight micro channel, different inlet temperatures: | ||
| Hot fluid flow (K) | Cold fluid flow (K) | |||
| 363.15 | 288.15 | Case 90 | ||
| 343.15 | 288.15 | Case 70 | ||
| 328.15 | 308.15 | Case 55 | ||
| Pin fin geometries and rectangular channel (case 90, aluminum) | Parallel pins | |||
| Staggered pins | ||||
| Straight channels | ||||
| Maximum Mach Number (-) Macritical > 0.3 | Maximum Pressure Ratio (-) (Δp/pin)critical > 0.05 | |
|---|---|---|
| Straight micro channels (Aluminum) | ||
| 0.25 | 0.21 |
| 0.25 | 0.20 |
| 0.25 | 0.20 |
| Parallel pins (Case 90, Aluminum) | 0.38 | 0.48 |
| Staggered pins (Case 90, Aluminum) | 0.70 | 0.74 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gerken, I.; Wetzel, T.; Brandner, J.J. Efficiency Improvement of Miniaturized Heat Exchangers. Fluids 2021, 6, 25. https://doi.org/10.3390/fluids6010025
Gerken I, Wetzel T, Brandner JJ. Efficiency Improvement of Miniaturized Heat Exchangers. Fluids. 2021; 6(1):25. https://doi.org/10.3390/fluids6010025
Chicago/Turabian StyleGerken, Iris, Thomas Wetzel, and Jürgen J. Brandner. 2021. "Efficiency Improvement of Miniaturized Heat Exchangers" Fluids 6, no. 1: 25. https://doi.org/10.3390/fluids6010025
APA StyleGerken, I., Wetzel, T., & Brandner, J. J. (2021). Efficiency Improvement of Miniaturized Heat Exchangers. Fluids, 6(1), 25. https://doi.org/10.3390/fluids6010025







