Hierarchical Modeling for Diagnostic Test Accuracy Using Multivariate Probability Distribution Functions
Abstract
1. Introduction
2. Materials and Methods
2.1. Statistical Methods
2.2. Evaluation of Heterogeneity
2.3. Threshold Effect
2.4. Bivariate and Hierarchical Approach
2.5. A Statistical Method for Meta-Analysis of Diagnostic Tests Using a Copula Approach
2.6. The Hierarchical Copula Model
2.7. Selection of a Model Copula
3. Simulation Study and Goodness-of-Fit of Copula Models
3.1. Generation of Simulated Data
3.2. Adjusting the Hierarchical HSROC and Copula Models
4. Results of the Adjustment to AUDIT-C Data and Simulated Data
Simulation Results
5. Conclusions and Remarks
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
List of Abbreviations
| AUDIT | Alcohol Use Disorder Identification Test |
| AUDIT-C | Alcohol Use Disorder Identification Test abbreviated version |
| BRMA | Bivariate random-effects meta-analysis |
| C270 | Clayton 270 |
| C90 | Clayton 90 |
| CI | confidence interval |
| FGM | Farlie–Gumbel–Morgenstern |
| FN | False negative |
| FP | False positive |
| HSROC | Hierarchical summary ROC |
| MCMC | Markov chain Monte Carlo |
| ROC | Receiver operating characteristic |
| Se | Sensibility |
| SE | Standard error |
| Sp | Specificity |
| SROC | Summary ROC |
| TN | True negative |
| TP | True positive |
Appendix A
Beta Distribution Combined with a Binomial Distribution
Appendix B

Appendix C

Appendix D
Example R Code
References
- Ahn, E.; Kang, H. Introduction to systematic review and meta-analysis. Korean J. Anesthesiol. 2018, 71, 103–112. [Google Scholar] [CrossRef]
- Doebler, P.; Holling, H.; Böhning, D. A mixed model approach to meta-analysis of diagnostic studies with binary test outcome. Psychol. Methods 2012, 17, 418–436. [Google Scholar] [CrossRef]
- Califf, R.M. Biomarker definitions and their applications. Exp. Biol. Med. 2018, 243, 213–221. [Google Scholar] [CrossRef] [PubMed]
- Egger, M.; Smith, G.D.; Schneider, M. (Eds.) Systematic Reviews of Observational Studies. In Systematic Reviews in Health Care: Meta-Analysis in Context, 2nd ed.; BMJ Publishing Group: London, UK, 2001; Volume 8, pp. 211–227. [Google Scholar] [CrossRef]
- Hasselblad, V.; Hedges, L.V. Meta-analysis of screening and diagnostic tests. Psychol. Bull. 1995, 117, 167–178. [Google Scholar] [CrossRef] [PubMed]
- Rutter, C.M.; Gatsonis, C.A. A hierarchical regression approach to meta-analysis of diagnostic test accuracy evaluations. Stat. Med. 2001, 20, 2865–2884. [Google Scholar] [CrossRef]
- Reitsma, J.B.; Glas, A.S.; Rutjes, A.W.; Scholten, R.J.; Bossuyt, P.M.; Zwinderman, A.H. Bivariate analysis of sensitivity and specificity produces informative summary measures in diagnostic reviews. J. Clin. Epidemiol. 2005, 58, 982–990. [Google Scholar] [CrossRef] [PubMed]
- Chu, H.; Nie, L.; Chen, Y.; Huang, Y.; Sun, W. Bivariate random effects models for meta-analysis of comparative studies with binary outcomes: Methods for the absolute risk difference and relative risk. Stat. Methods Med. Res. 2010, 21, 621–633. [Google Scholar] [CrossRef]
- Takwoingi, Y.; Guo, B.; Riley, R.D.; Deeks, J.J. Performance of methods for meta-analysis of diagnostic test accuracy with few studies or sparse data. Stat. Methods Med. Res. 2017, 26, 1896–1911. [Google Scholar] [CrossRef]
- Chu, H.; Guo, H. Letter to the editor. Biostatistics 2008, 10, 201–203. [Google Scholar] [CrossRef]
- Kuss, O.; Hoyer, A.; Solms, A. Meta-analysis for diagnostic accuracy studies: A new statistical model using beta-binomial distributions and bivariate copulas. Stat. Med. 2014, 33, 17–30. [Google Scholar] [CrossRef]
- Lee, J.; Kim, K.W.; Choi, S.H.; Huh, J.; Park, S.H. Systematic Review and Meta-Analysis of Studies Evaluating Diagnostic Test Accuracy: A Practical Review for Clinical Researchers-Part II. Statistical Methods of Meta-Analysis. Korean J. Radiol. 2015, 16, 1188–1196. [Google Scholar] [CrossRef]
- Moses, L.E.; Shapiro, D.; Littenberg, B. Combining independent studies of a diagnostic test into a summary roc curve: Data-analytic approaches and some additional considerations. Stat. Med. 1993, 12, 1293–1316. [Google Scholar] [CrossRef]
- Schwarzer, G.; Carpenter, J.R.; Rücker, G. Meta-Analysis with R; Springer: Berlin, Germany, 2015. [Google Scholar]
- Riley, R.D.; Dodd, S.R.; Craig, J.V.; Thompson, J.R.; Williamson, P.R. Meta-analysis of diagnostic test studies using individual patient data and aggregate data. Stat. Med. 2008, 27, 6111–6136. [Google Scholar] [CrossRef] [PubMed]
- Steinhauser, S.; Schumacher, M.; Rücker, G. Modelling multiple thresholds in meta-analysis of diagnostic test accuracy studies. BMC Med. Res. Methodol. 2016, 16, 1–15. [Google Scholar] [CrossRef]
- Bauz, S.; Pambabay-Calero, J.J.; Nieto-Librero, A.B.; Galindo-Villardón, M.P. Meta-Analysis in DTA with Hierarchical Models Bivariate and HSROC: Simulation Study. In Selected Contributions on Statistics and Data Science in Latin America; Antoniano-Villalobos, I., Mena, R., Mendoza, M., Naranjo, L., Nieto-Barajas, L., Eds.; Springer: Cham, Switzerland, 2019; Volume 301, pp. 33–42. [Google Scholar] [CrossRef]
- Arends, L.R.; Hamza, T.H.; van Houwelingen, J.C.; Heijenbrok-Kal, M.H.; Hunink, M.G.M.; Stijnen, T. Bivariate Random Effects Meta-Analysis of ROC Curves. Med. Decis. Mak. 2008, 28, 621–638. [Google Scholar] [CrossRef]
- Harbord, R.M.; Deeks, J.J.; Egger, M.; Whiting, P.; Sterne, J. A unification of models for meta-analysis of diagnostic accuracy studies. Biostatistics 2007, 8, 239–251. [Google Scholar] [CrossRef]
- Macaskill, P.; Gatsonis, C.; Deeks, J.; Harbord, R.; Takwoingi, Y. Cochrane Handbook for Systematic Reviews of Diagnostic Test Accuracy; Chapter 10 Analysing and Presenting Results; The Cochrane Collaboration: London, UK, 2010. [Google Scholar]
- Senturk, D. Explanatory Item Response Models: A Generalized Linear and Nonlinear Approach. Technometrics 2006, 48, 568–569. [Google Scholar] [CrossRef]
- Nikoloulopoulos, A.K. A mixed effect model for bivariate meta-analysis of diagnostic test accuracy studies using a copula representation of the random effects distribution. Stat. Med. 2015, 34, 3842–3865. [Google Scholar] [CrossRef]
- Kriston, L.; Hölzel, L.; Weiser, A.-K.; Berner, M.M.; Härter, M. Meta-analysis: Are 3 Questions Enough to Detect Unhealthy Alcohol Use? Ann. Intern. Med. 2008, 149, 879–888. [Google Scholar] [CrossRef]
- Babor, T.; Higgins-Biddle, J.C.; Saunders, J.B.; Monteiro, M.G. The Alcohol Use Disorders Identification Test: Guidelines for Use in Primary Care; World Health Organization: Geneva, Switzerland, 2001; pp. 1–40. [Google Scholar]
- Muhammad, N.; Coolen-Maturi, T.; Coolen, F.P. Nonparametric predictive inference with parametric copulas for combining bivariate diagnostic tests. Stat. Optim. Inf. Comput. 2018, 6, 398–408. [Google Scholar] [CrossRef][Green Version]
- Ghalibaf, M.B. Relationship Between Kendall’s tau Correlation and Mutual Information. Revista Colombiana de Estadística 2020, 43, 3–20. [Google Scholar] [CrossRef]
- SKLAR, M. Fonctions de repartition a n dimensions et leurs marges. Publ. Inst. Stat. Univ. Paris 1959, 8, 229–231. [Google Scholar]
- Nelsen, R.B. An Introduction to Copulas; Springer Science & Business Media: Berlin, Germany, 2000; Volume 42, p. 317. [Google Scholar]
- Schweizer, B.; Wolff, E.F. On Nonparametric Measures of Dependence for Random Variables. Ann. Stat. 1981, 9, 879–885. [Google Scholar] [CrossRef]
- Hoyer, A.; Kuss, O. Meta-analysis of diagnostic tests accounting for disease prevalence: A new model using trivariate copulas. Stat. Med. 2015, 34, 1912–1924. [Google Scholar] [CrossRef]
- Hoyer, A.; Kuss, O. Meta-analysis for the comparison of two diagnostic tests to a common gold standard: A generalized linear mixed model approach. Stat. Methods Med Res. 2016, 27, 1410–1421. [Google Scholar] [CrossRef]
- Zapf, A.; Hoyer, A.; Kramer, K.; Kuss, O. Nonparametric meta-analysis for diagnostic accuracy studies. Stat. Med. 2015, 34, 3831–3841. [Google Scholar] [CrossRef]
- Coakley, C.W.; Conover, W.J. Practical Nonparametric Statistics. J. Am. Stat. Assoc. 2000, 95, 332. [Google Scholar] [CrossRef]
- Bachmann, L.M.; Puhan, M.A.; Ter Riet, G.; Bossuyt, P.M. Sample sizes of studies on diagnostic accuracy: Literature survey. BMJ 2006, 332, 1127–1129. [Google Scholar] [CrossRef] [PubMed]
- Morris, T.P.; White, I.R.; Crowther, M.J. Using simulation studies to evaluate statistical methods. Stat. Med. 2019, 38, 2074–2102. [Google Scholar] [CrossRef]
- Nyaga, V.N.; Arbyn, M.; Aerts, M. CopulaDTA: An R Package for Copula-Based Bivariate Beta-Binomial Models for Diagnostic Test Accuracy Studies in a Bayesian Framework. J. Stat. Softw. 2017, 82, 1–27. [Google Scholar] [CrossRef]
- Schiller, I.; Dendukuri, N. HSROC: An R Package for Bayesian Meta-Analysis of Diagnostic Test Accuracy; Version 2.1.8; R Core Team: Vienna, Austria, 2015. [Google Scholar]
- Huerta, M.; Leiva, V.; Lillo, C.; Rodriguez, M.; Leiva-Sanchez, V. A beta partial least squares regression model: Diagnostics and application to mining industry data. Appl. Stoch. Model. Bus. Ind. 2018, 34, 305–321. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Q.; Hou, H.-D.; Rho, S.; Gupta, B.; Mu, Y.-X.; Shen, W.-Z. Big data driven outlier detection for soybean straw near infrared spectroscopy. J. Comput. Sci. 2018, 26, 178–189. [Google Scholar] [CrossRef]
- Dukic, V.; Gatsonis, C. Meta-analysis of diagnostic test accuracy assessment studies with varying number of thresholds. Biometrics 2003, 59, 936–946. [Google Scholar] [CrossRef]
- Genest, C.; Nešlehová, J. A Primer on Copulas for Count Data. ASTIN Bull. 2007, 37, 475–515. [Google Scholar] [CrossRef]
- Mikosch, T. Copulas: Tales and Facts—Rejoinder. Extremes 2006, 9, 55–62. [Google Scholar] [CrossRef]
- Chu, H.; Nie, L.; Cole, S.R.; Poole, C. Meta-analysis of diagnostic accuracy studies accounting for disease prevalence: Alternative parameterizations and model selection. Stat. Med. 2009, 28, 2384–2399. [Google Scholar] [CrossRef]
- Pambabay-Calero, J.J.; Bauz-Olvera, S.A.; Nieto-Librero, A.B.; Galindo-Villardon, M.P.; Hernandez-Gonzalez, S. An alternative to the Cochran-(Q) statistic for analysis of heterogeneity in meta-analysis of diagnostic tests based on HJ BIPLOT. Investig. Oper. 2018, 39, 536–545. [Google Scholar]
- Pambabay-Calero, J.J.; Bauz-Olvera, S.A.; Nieto-Librero, A.B.; Galindo-Villardón, M.P.; Sánchez-García, A.B. A tutorial for meta-analysis of diagnostic tests for low-prevalence diseases: Bayesian models and software. Methodology 2020, 16, 258–277. [Google Scholar] [CrossRef]
- Liu, Y.; DeSantis, S.M.; Chen, Y. Bayesian mixed treatment comparisons meta-analysis for correlated outcomes subject to reporting bias. J. R. Stat. Soc. Ser. C 2017, 67, 127–144. [Google Scholar] [CrossRef] [PubMed]
- Krog, J.; Jacobsen, L.H.; Lund, F.W.; Wüstner, D.; Lomholt, M.A. Bayesian model selection with fractional Brownian motion. J. Stat. Mech. Theory Exp. 2018, 2018, 093501. [Google Scholar] [CrossRef]
- Krog, J.; Lomholt, M.A. Bayesian inference with information content model check for Langevin equations. Phys. Rev. E 2017, 96, 062106. [Google Scholar] [CrossRef]
- Thapa, S.; Lomholt, M.A.; Krog, J.; Cherstvy, A.G.; Metzler, R. Bayesian analysis of single-particle tracking data using the nested-sampling algorithm: Maximum-likelihood model selection applied to stochastic-diffusivity data. Phys. Chem. Chem. Phys. 2018, 20, 29018–29037. [Google Scholar] [CrossRef]
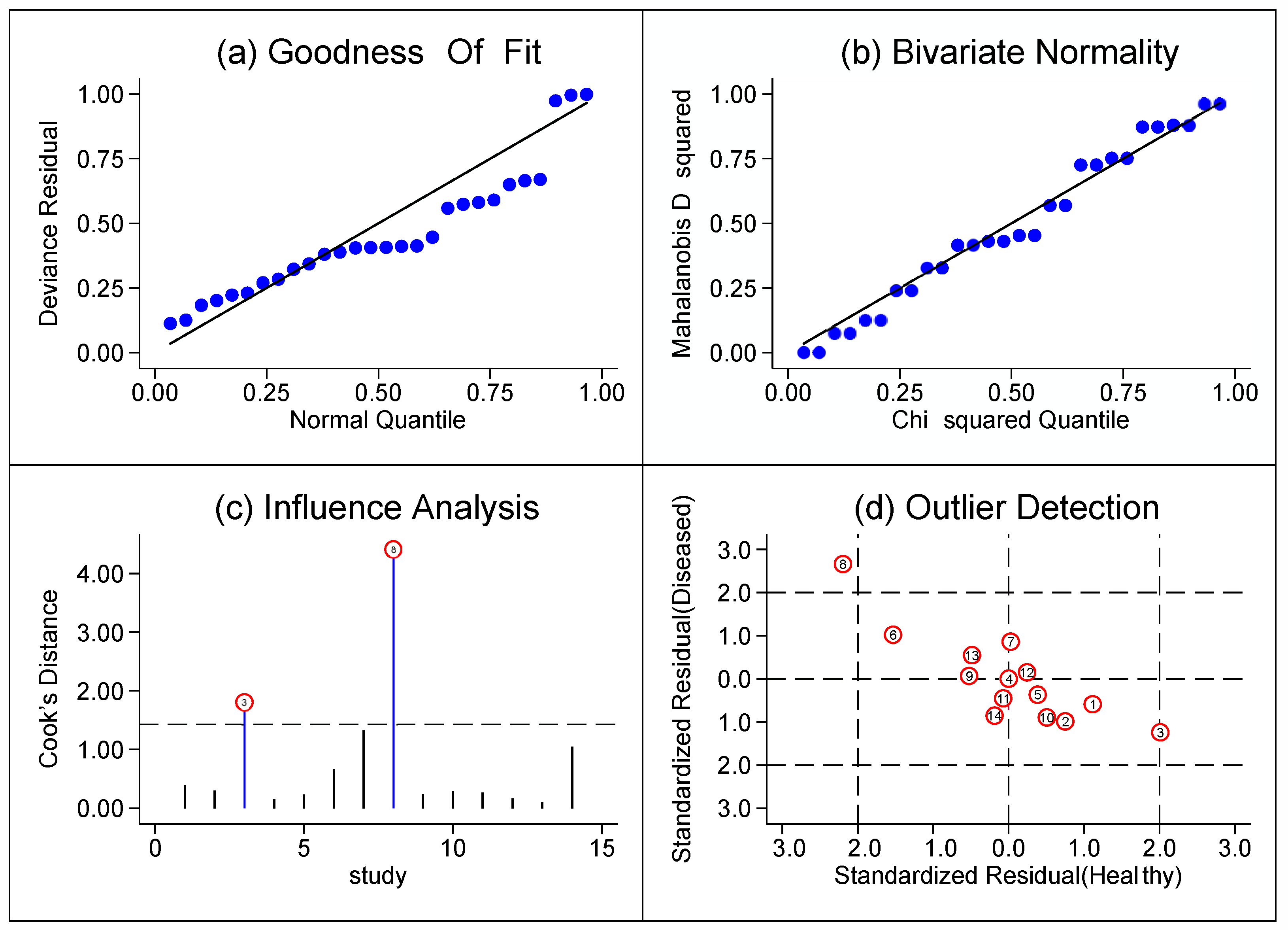
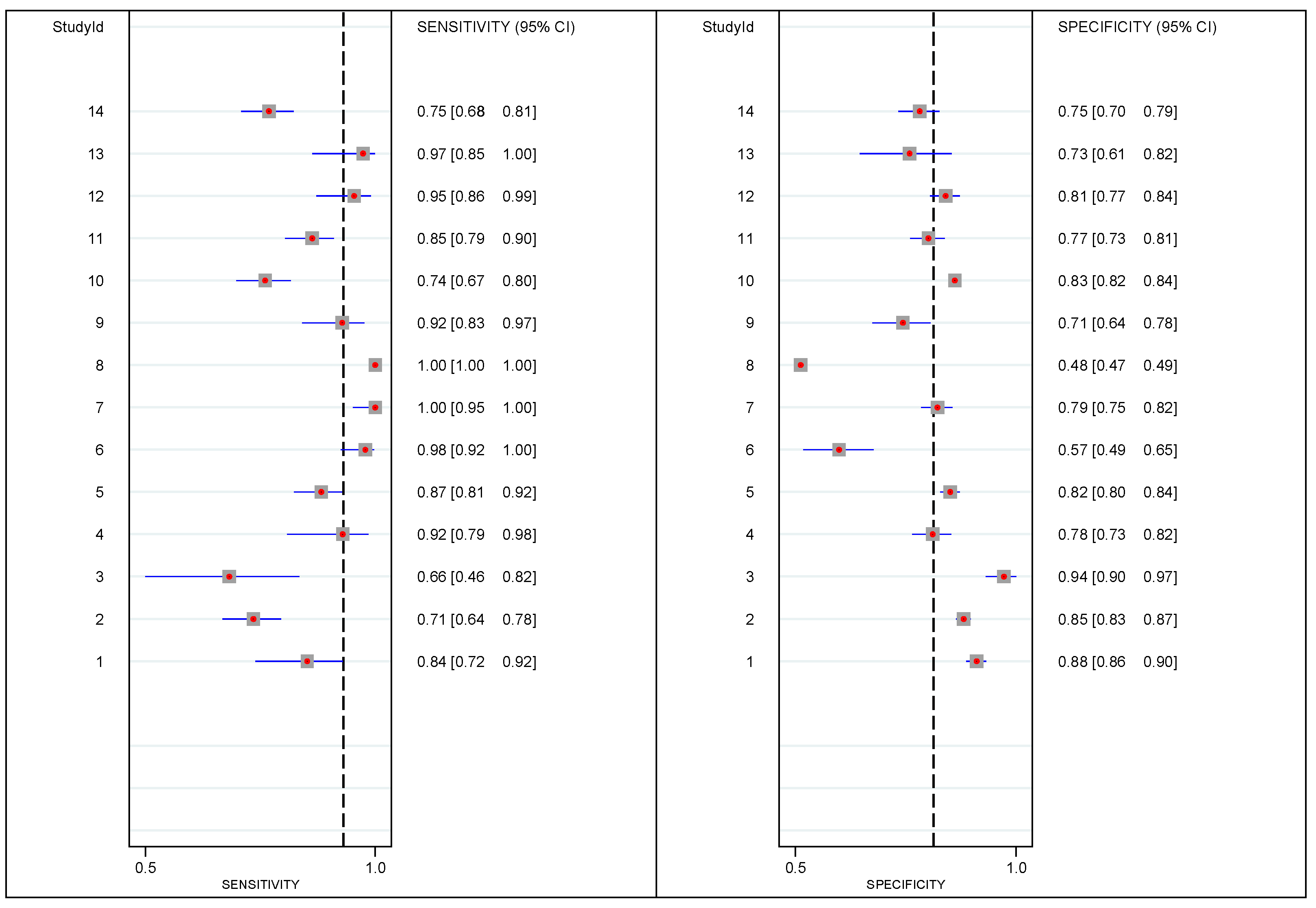

| ID | TP | FP | TN | FN |
|---|---|---|---|---|
| 1 | 47 | 101 | 738 | 9 |
| 2 | 126 | 272 | 1543 | 51 |
| 3 | 19 | 12 | 192 | 10 |
| 4 | 36 | 78 | 276 | 3 |
| 5 | 130 | 211 | 959 | 19 |
| 6 | 84 | 68 | 89 | 2 |
| 7 | 68 | 112 | 423 | 0 |
| 8 | 752 | 3226 | 2977 | 0 |
| 9 | 59 | 55 | 136 | 5 |
| 10 | 142 | 571 | 2788 | 50 |
| 11 | 137 | 107 | 358 | 24 |
| 12 | 57 | 103 | 437 | 3 |
| 13 | 34 | 21 | 56 | 1 |
| 14 | 152 | 88 | 264 | 51 |
| Copulas | Parameter | Mean | Lower | Upper |
|---|---|---|---|---|
| Gauss | Se | 0.862 | 0.766 | 0.920 |
| Sp | 0.755 | 0.6898 | 0.811 | |
| Correlation | −0.570 | −0.799 | −0.289 | |
| C90 | Se | 0.865 | 0.777 | 0.922 |
| Sp | 0.760 | 0.695 | 0.813 | |
| Correlation | −0.466 | −0.801 | −0.0005 | |
| C270 | Se | 0.854 | 0.743 | 0.920 |
| Sp | 0.752 | 0.680 | 0.810 | |
| Correlation | −0.324 | −0.758 | −2.219 × 10−17 | |
| FGM | Se | 0.871 | 0.780 | 0.932 |
| Sp | 0.756 | 0.692 | 0.812 | |
| Correlation | −0.214 | −0.222 | −0.121 | |
| Frank | Se | 0.858 | 0.754 | 0.929 |
| Sp | 0.751 | 0.773 | 0.808 | |
| Correlation | −0.600 | −0.767 | 1.000 |
| Copula Model | Statistic | p-Value |
|---|---|---|
| Gauss | 0.06547 | 0.301 |
| Clayton | 0.05539 | 0.659 |
| FGM | 0.02287 | 0.903 |
| Frank | 0.06647 | 0.296 |
| Number of Studies in the Meta-Analysis | ||||||
|---|---|---|---|---|---|---|
| Copula | Parameter | 5–10 | 11–16 | 17–22 | 23–38 | 29–35 |
| Gauss | Statistic | 0.11823 | 0.06988 | 0.050 97 | 0.04320 | 0.03739 |
| SE | 0.00252 | 0.00113 | 0.00064 | 0.00069 | 0.00061 | |
| p-value | 0.50309 | 0.49000 | 0.47070 | 0.44991 | 0.49072 | |
| Clayton | Statistic | 0.16819 | 0.16851 | 0.13742 | 0.11023 | 0.03460 |
| SE | 0.03237 | 0.05993 | 0.06356 | 0.07039 | 0.00061 | |
| p-value | 0.52656 | 0.52146 | 0.52786 | 0.48326 | 0.49891 | |
| FGM | Statistic | 0.06490 | 0.050 25 | 0.04871 | 0.06154 | 0.06717 |
| SE | 0.00293 | 0.00159 | 0.00152 | 0.00032 | 0.00416 | |
| p-value | 0.71763 | 0.62812 | 0.48737 | 0.34574 | 0.30307 | |
| Frank | Statistic | 0.11127 | 0.06867 | 0.05102 | 0.04355 | 0.03735 |
| SE | 0.00361 | 0.00123 | 0.00075 | 0.00078 | 0.00067 | |
| p-value | 0.43157 | 0.48938 | 0.46523 | 0.43418 | 0.46655 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pambabay-Calero, J.; Bauz-Olvera, S.; Nieto-Librero, A.; Sánchez-García, A.; Galindo-Villardón, P. Hierarchical Modeling for Diagnostic Test Accuracy Using Multivariate Probability Distribution Functions. Mathematics 2021, 9, 1310. https://doi.org/10.3390/math9111310
Pambabay-Calero J, Bauz-Olvera S, Nieto-Librero A, Sánchez-García A, Galindo-Villardón P. Hierarchical Modeling for Diagnostic Test Accuracy Using Multivariate Probability Distribution Functions. Mathematics. 2021; 9(11):1310. https://doi.org/10.3390/math9111310
Chicago/Turabian StylePambabay-Calero, Johny, Sergio Bauz-Olvera, Ana Nieto-Librero, Ana Sánchez-García, and Puri Galindo-Villardón. 2021. "Hierarchical Modeling for Diagnostic Test Accuracy Using Multivariate Probability Distribution Functions" Mathematics 9, no. 11: 1310. https://doi.org/10.3390/math9111310
APA StylePambabay-Calero, J., Bauz-Olvera, S., Nieto-Librero, A., Sánchez-García, A., & Galindo-Villardón, P. (2021). Hierarchical Modeling for Diagnostic Test Accuracy Using Multivariate Probability Distribution Functions. Mathematics, 9(11), 1310. https://doi.org/10.3390/math9111310









