ANN-Based Estimation of the Defect Severity in the Drilling of GFRP/Ti Multilayered Composite Structure
Abstract
1. Introduction
- -
- Low thermal conductivity and high hardness of reinforcing glass fibers, leading to wear and blunting of the cutting edges of the tool.
- -
- The dependence of the rheological state (viscosity) of both thermosetting and thermoplastic matrices on the temperature during drilling makes it difficult to remove chips from the hole and degrade the properties of the resin when heated. Moreover, the adhesion of the resin to the metal layers is significantly reduced as a result of its heating due to the heat generated during cutting.
- -
- Moisture absorption, inherent in most polymer composites, does not allow the use of cooling fluids during drilling to reduce the temperature and degradation of resins.
- -
- A sharp difference in the mechanical properties and machinability of titanium and polymer composite causes crushing of thin interlayer titanium plates near the surface of the hole to be machined, contributing to the emergence of the splintering or fiber breakout, fiber pullouts, and delaminations.
2. Materials and Tools
3. Experiments
4. Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- M’Saoubi, R. High performance cutting of advanced aerospace alloys and composite materials. CIRP Ann. 2015, 64, 557–580. [Google Scholar] [CrossRef]
- Aamir, M.; Tolouei-Rad, M.; Giasin, K.; Nosrati, A. Recent advances in drilling of carbon fiber–reinforced polymers for aerospace applications: A review. Int. J. Adv. Manuf. Technol. 2019, 105, 2289–2308. [Google Scholar] [CrossRef]
- Hassan, M.H.; Abdullah, J.; Franz, G.; Shen, C.Y.; Mahmoodian, R. Effect of twist drill geometry and drilling parameters on hole quality in single-shot drilling of CFRP/Al7075-T6 Composite Stack. J. Compos. Sci. 2021, 5, 189. [Google Scholar] [CrossRef]
- Fard, M.G.; Baseri, H.; Zolfagari, A. Experimental investigation of the effective parameters and performance of different types of structural tools in drilling CFRP/AL alloy stacks. J. Compos. Mater. 2022, 56, 4461–4471. [Google Scholar] [CrossRef]
- Mazumdar, S. Composites Manufacturing: Materials, Product, and Process Engineering; CRC Press: Boca Raton, FL, USA, 2001; 390p. [Google Scholar]
- Paleen, M.J.; Kilwin, J.J. Hole Drilling in Polymer-Matrix Composites. In ASM Handbook: Composites; Miracle, D.B., Donaldson, S.L., Eds.; ASM International: Almere, The Netherlands, 2001; Volume 21, pp. 1508–1518. [Google Scholar]
- Zitoune, R.; Krishnaraj, V.; Collombet, F. Study of drilling of composite material and aluminum stack. Compos. Struct. 2010, 92, 1246–1255. [Google Scholar] [CrossRef]
- Campbell, F.C., Jr. Manufacturing Technology for Aerospace Structural Materials; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Murugesh, M.C.; Sadashivappa, K. Influence of filler material on Glass fiber epoxy composite laminates during drilling. Int. J. Adv. Eng. Technol. 2012, 3, 233–239. [Google Scholar]
- Gaitonde, V.N. A study aimed at minimizing delamination during drilling of CFRP composites. J. Compos. Mater. 2011, 45, 2359–2368. [Google Scholar] [CrossRef]
- Pelivanov, I.; Ambrozinski, L.; O’Donnell, M. Heat damage evaluation in carbon fiber-reinforced composites with a kHz A-scan rate fiber-optic pump-probe laser-ultrasound system. Compos. Part A-Appl. Sci. Manuf. 2016, 84, 417–427. [Google Scholar] [CrossRef]
- Ghasemi, F.A.; Hyvadi, A.; Payganeh, G.; Arab, N.B.M. Effects of Drilling Parameters on Delamination of Glass-Epoxy Composites. Aust. J. Basic Appl. Sci. 2011, 5, 1433–1440. [Google Scholar]
- El Bouami, S.; Habak, M.; Franz, G.; Velasco, R.; Vantomme, P. Effect of tool geometry and cutting parameters on delamination and thrust forces in drilling CFRP/Al-Li. AIP Conf. Proc. 2016, 1769, 080012. [Google Scholar]
- Kaul, J.; Pettermann, H.E. A constitutive model for fiber reinforced polymer plies–Implicit anisotropic evolution of damage and plasticity based on effective strengths. Compos. Part C 2021, 4, 100097. [Google Scholar] [CrossRef]
- Shalwan, A.; Alsaeed, T.; Yousif, B. Machinability of Polymeric Composites and Future Reinforcements—A Review. J. Mater. Sci. Chem. Eng. 2022, 10, 40–72. [Google Scholar] [CrossRef]
- Wang, B.; Gao, H.; Cao, B.; Zhuang, Y.; Zhao, Z. Mechanism of damage generation during drilling of carbon/epoxy composites and titanium alloy stacks. Proc. Inst. Mech. Eng. Part B Eng. Manuf. 2014, 228, 698–706. [Google Scholar] [CrossRef]
- Mahdi, A.; Turki, Y.; Habak, M.; Salem, M.; Bouaziz, Z. Experimental study of thrust force and surface quality when drilling hybrid stacks. Int. J. Adv. Manuf. Technol. 2020, 107, 3981–3994. [Google Scholar] [CrossRef]
- Jia, Z.Y.; Chen, C.; Wang, F.J.; Zhang, C.; Wang, Q. Analytical model for delamination of CFRP during drilling of CFRP/metal stacks. Int. J. Adv. Manuf. Technol. 2020, 106, 5099–5109. [Google Scholar] [CrossRef]
- Altinkok, N.; Koker, R. Modelling of the prediction of tensile and density properties in particle reinforced metal matrix composites by using neural networks. Mater Des. 2006, 27, 625–631. [Google Scholar] [CrossRef]
- Kilickap, E. Investigation into the effect of drilling parameters on delamination in drilling GFRP. J. Reinf. Plast. Comp. 2010, 29, 3498–3503. [Google Scholar] [CrossRef]
- Xu, J.; Lin, T.; Davim, J.P. On the Machining Temperature and Hole Quality of CFRP Laminates When Using Diamond-Coated Special Drills. J. Compos. Sci. 2022, 6, 45. [Google Scholar] [CrossRef]
- Genculu, S. Factors Affecting Machinability of Metals. 2018. Available online: https://www.cabinc.com/uploads/case_studies/MachinabilityFactors-wp.pdf (accessed on 31 October 2022).
- Chen, W.C. Some experimental investigations in the drilling of carbon fiber-reinforced plastic (CFRP) composite laminates. Int. J. Mach. Tools Manuf. 1997, 37, 1097–1108. [Google Scholar] [CrossRef]
- Bhatnagar, N.; Singh, I.; Nayak, D. Damage investigations in drilling of glass fiber reinforced plastic composite laminates. Mater. Manuf. Process. 2004, 19, 995–1007. [Google Scholar] [CrossRef]
- Lopez-Arraiza, A.; Amenabar, I.; Agirregomezkorta, A.; Sarrionandia, M.; Aurrekoetxea, J. Experimental analysis of drilling damage in carbon-fiber reinforced thermoplastic laminates manufactured by resin transfer molding. J. Compos. Mater. 2011, 46, 717–725. [Google Scholar] [CrossRef]
- Faraz, A.; Biermann, D. Subsequent drilling on pilot holes in woven carbon fibre reinforced plastic epoxy laminates: The effect of drill chisel edge on delamination. Proc. Inst. Mech. Eng. Part B Eng. Manuf. 2011, 225, 1493–1504. [Google Scholar] [CrossRef]
- Kerrigan, K.; O’Donnell, G. On the relationship between cutting temperature and workpiece polymer degradation during CFRP edge trimming. Procedia CIRP 2015, 55, 170–175. [Google Scholar] [CrossRef]
- Ray, S.; Cooney, R.P. Thermal degradation of polymer and polymer composites. In Handbook of Environmental Degradation of Materials, 3rd ed.; Kutz, M., Ed.; William Andrew Publishing: Norwich, CT, USA, 2018; pp. 185–206. [Google Scholar]
- Jiao, F.; Li, Y.; Niu, Y.; Zhang, Z.; Bie, W. A review on the drilling of CFRP/Ti stacks: Machining characteristics, damage mechanisms and suppression strategy at stack interface. Compos. Struct. 2023, 305, 116489. [Google Scholar] [CrossRef]
- Sorrentino, L.; Turchetta, S.; Colella, C. In-process force and temperature monitoring while drilling composites. JEC Compos. Mag. 2016, 102, 46–48. [Google Scholar]
- Sorrentino, L.; Turchetta, S.; Colella, C.; Bellini, C. Analysis of thermal damage in FRP drilling. Procedia Eng. 2016, 167, 206–2015. [Google Scholar] [CrossRef]
- Zaghloul, M.M.Y.; Zaghloul, M.Y.M.; Zaghloul, M.M.Y. Experimental and modeling analysis of mechanical-electrical behaviors of polypropylene composites filled with graphite and MWCNT fillers. Polym. Test. 2017, 63, 467–474. [Google Scholar] [CrossRef]
- Zaghloul, M.Y.M.; Zaghloul, M.M.Y.; Zaghloul, M.M.Y. Developments in polyester composite materials–An in-depth review on natural fibres and nano fillers. Compos. Struct. 2021, 278, 114698. [Google Scholar] [CrossRef]
- Zaghloul, M.M.Y.M. Mechanical properties of linear low-density polyethylene fire-retarded with melamine polyphosphate. J. Appl. Polym. Sci. 2018, 135, 46770. [Google Scholar] [CrossRef]
- Fuseini, M.; Zaghloul, M.M.Y. Qualitative and statistical approaches of the electrophoretic deposition kinetics of polyaniline copper coating. Prog. Org. Coat. 2022, 171, 107015. [Google Scholar] [CrossRef]
- Vedernikov, A.; Minchenkov, K.; Gusev, S.; Sulimov, A.; Zhou, P.; Li, C.; Xian, G.; Akhatov, I.; Safonov, A. Effects of the Pre-Consolidated Materials Manufacturing Method on the Mechanical Properties of Pultruded Thermoplastic Composites. Polymer 2022, 14, 2246. [Google Scholar] [CrossRef] [PubMed]
- Vedernikov, A.; Gemi, L.; Madenci, E.; Özkılıç, Y.O.; Yazman, Ş.; Gusev, S.; Sulimov, A.; Bondareva, J.; Evlashin, S.; Konev, S.; et al. Effects of high pulling speeds on mechanical properties and morphology of pultruded GFRP composite flat laminates. Compos. Struct. 2022, 301, 116216. [Google Scholar] [CrossRef]
- Vedernikov, A.; Safonov, A.; Tucci, F.; Carlone, P.; Akhatov, I. Analysis of Spring-in Deformation in L-shaped Profiles Pultruded at Different Pulling Speeds: Mathematical Simulation and Experimental Results. In Proceedings of the 24th International Conference on Material Forming, Liège, Belgique, 14–16 April 2021. [Google Scholar]
- Minchenkov, K.; Vedernikov, A.; Kuzminova, Y.; Gusev, S.; Sulimov, A.; Gulyaev, A.; Kreslavskaya, A.; Prosyanoy, I.; Xian, G.; Akhatov, I.; et al. Effects of the quality of pre-consolidated materials on the mechanical properties and morphology of thermoplastic pultruded flat laminates. Compos. Commun. 2022, 35, 101281. [Google Scholar] [CrossRef]
- Ashworth, S. Varying CFRP workpiece temperature during slotting: Effects on surface metrics, cutting forces and chip geometry. Procedia CIRP 2019, 85, 37–42. [Google Scholar] [CrossRef]
- Vajdi, M.; Moghanlou, F.S.; Sharifianjazi, F.; Asl, M.S.; Shokouhimehr, M. A review on the COMSOL Multiphysics studies of heat transfer in advanced ceramics. J. Compos. Compd. 2020, 2, 35–43. [Google Scholar] [CrossRef]
- Giasin, K.; Ayvar-Soberanis, S. An investigation of burrs, chip formation, hole size, circularity and delamination during drilling operation of GLARE using ANOVA. Compos. Struct. 2017, 159, 745–760. [Google Scholar] [CrossRef]
- Bekele, A.E.; Lemu, H.G.; Jiru, M.G. Study of Machinability in Drilling Operation of Enset–Sisal Hybrid Polyester Composite. J. Compos. Sci. 2022, 6, 205. [Google Scholar] [CrossRef]
- Chakraborty, D. Artificial neural network based delamination prediction in laminated composites. Mater. Des. 2005, 26, 1–7. [Google Scholar] [CrossRef]
- Mishra, R.; Malik, J.; Singh, I. Prediction of drilling-induced damage in unidirectional glass-fibre-reinforced plastic laminates using an artificial neural network. Proc. Inst. Mech. Eng. Part B Eng. Manuf. 2010, 224, 733–738. [Google Scholar] [CrossRef]
- Chinchan, L.; Shevtsov, S.; Soloviev, A.; Shevtsova, V.; Huang, J.P. Mechanical testing of polymeric composites for aircraft applications: Standards, requirements and limitations. In Advanced Materials; Chang, S.H., Parinov, I., Topolov, V., Eds.; Springer: Cham, Switzerland, 2014; Volume 152, pp. 201–222. [Google Scholar]
- Durão, L.M.; Gonçalves, D.J.; Tavares, J.M.; de Albuquerque, V.H.; Marques, A.T. Comparative analysis of drills for composite laminates. J. Compos. Mater. 2011, 46, 1649–1659. [Google Scholar] [CrossRef]
- Okutan, E.; Karabay, S.; Sinmazcelik, T.; Avcu, E. A Study on the Derivation of Parametric Cutting Force Equations in Drilling of GFRP Composites. Stroj. Vestn. J. Mech. Eng. 2013, 59, 97–107. [Google Scholar] [CrossRef]
- Hornik, K.; Stinchcombe, M.; White, H. Multilayer feedforward networks are universal approximators. Neural. Netw. 1989, 2, 359–366. [Google Scholar] [CrossRef]
- Hornik, K. Approximation capabilities of multilayer feedforward networks. Neural. Netw. 1991, 4, 251–257. [Google Scholar] [CrossRef]
- Lu, Z.; Pu, H.; Wang, F.; Hu, Z.; Wang, L. The Expressive Power of Neural Networks: A View from the Width. Adv. Neur. 2017, 30, 6231–6239. [Google Scholar]
- Yarotsky, D. Universal approximations of invariant maps by neural networks. Constr. Approx 2022, 55, 407–474. [Google Scholar] [CrossRef]
- MATLAB. 2022. Available online: https://www.mathworks.com (accessed on 31 October 2022).
- Zou, F.; Dang, J.; Chen, T.; An, Q.; Chen, M. Evaluation of typical hole-making strategies on mechanical behavior of CFRP/Ti single-lap bolted joints. Compos. Struct. 2023, 305, 116511. [Google Scholar] [CrossRef]
- Machinability. 2022. Available online: https://en.wikipedia.org/wiki/Machinability (accessed on 31 October 2022).

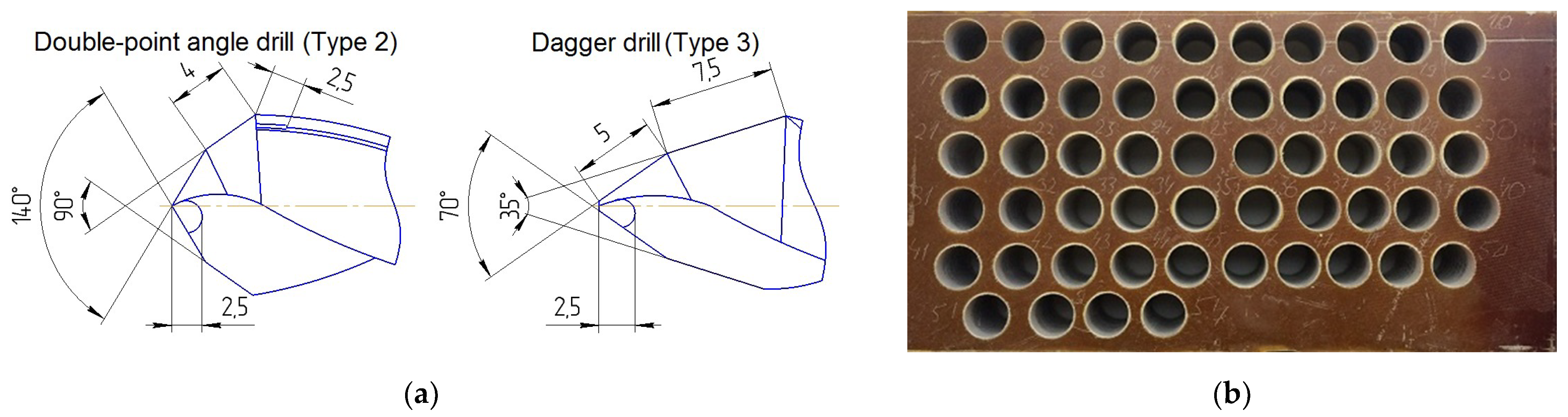

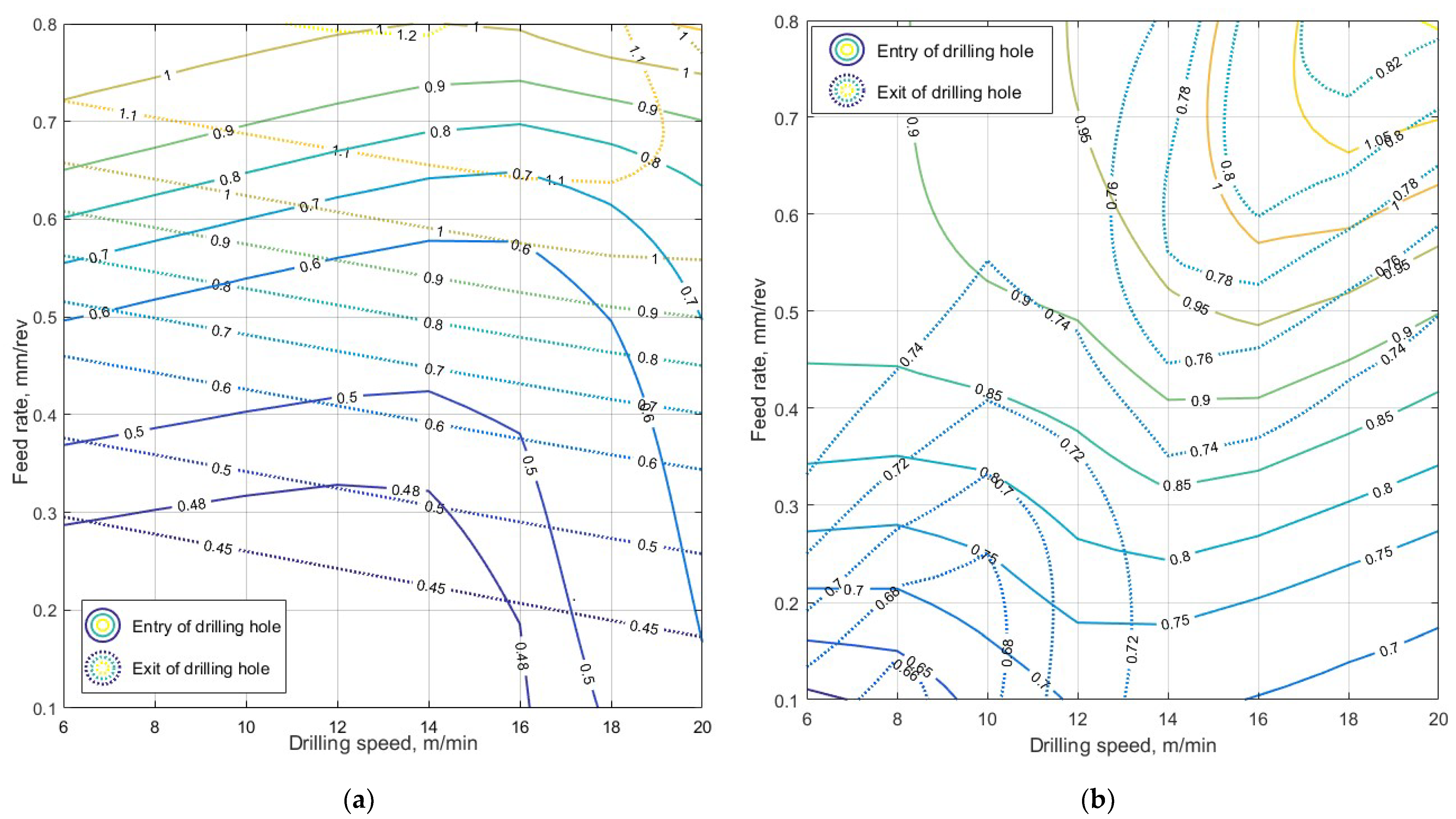
 ), upper (
), upper ( ), and lower (
), and lower ( ) bounds of the confidence intervals for each process test.
) bounds of the confidence intervals for each process test.
 ), upper (
), upper ( ), and lower (
), and lower ( ) bounds of the confidence intervals for each process test.
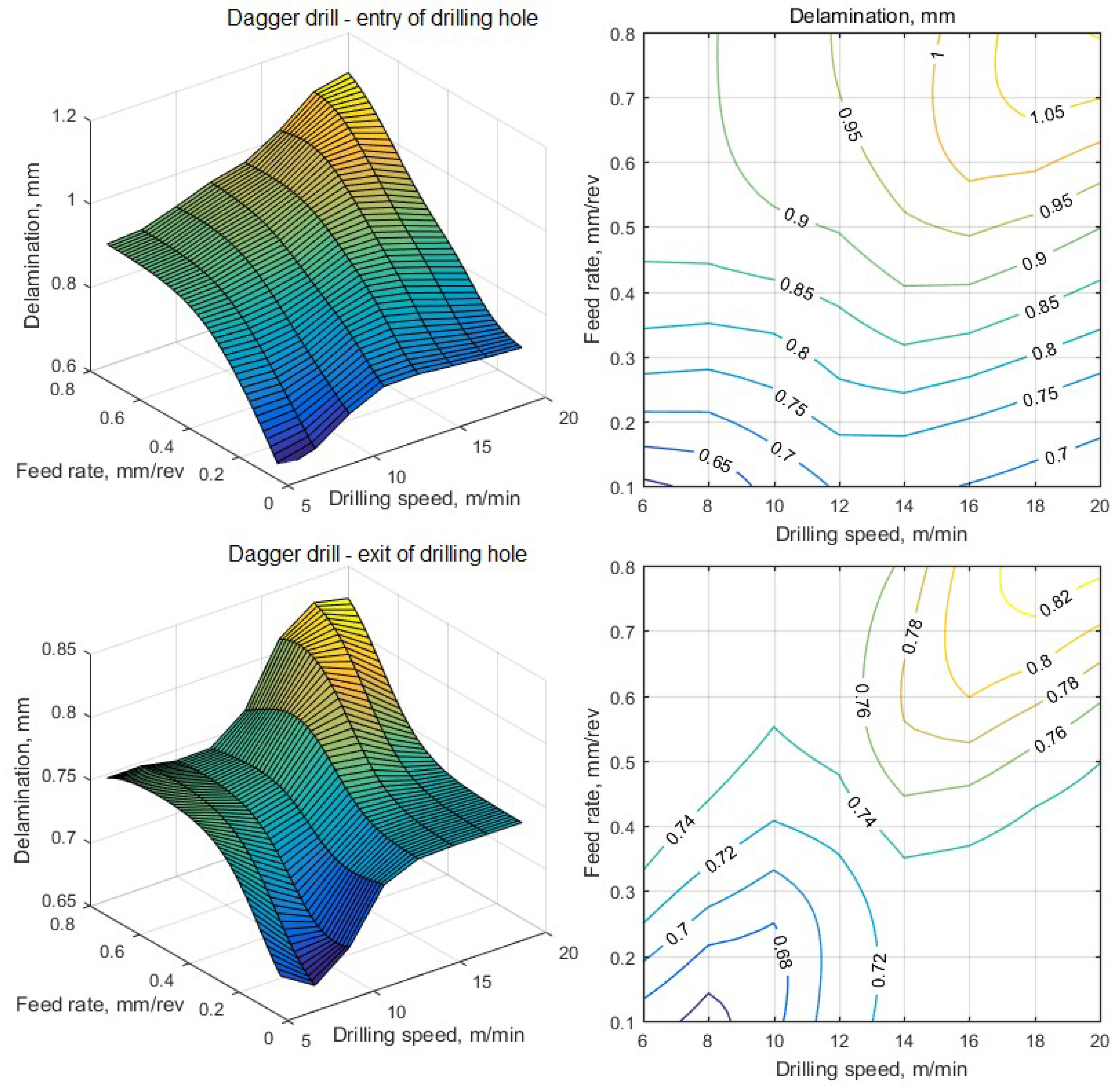
) bounds of the confidence intervals for each process test.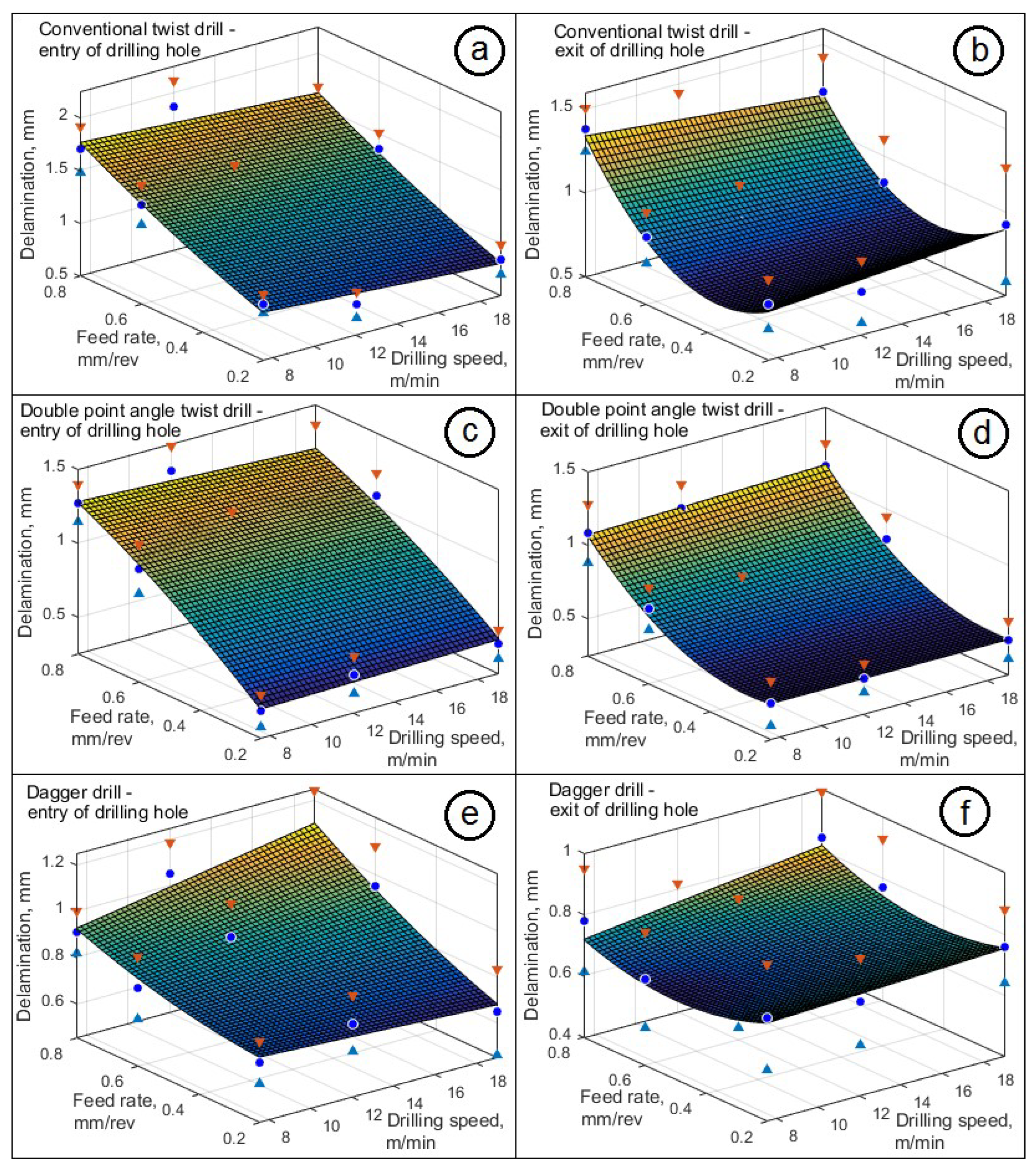
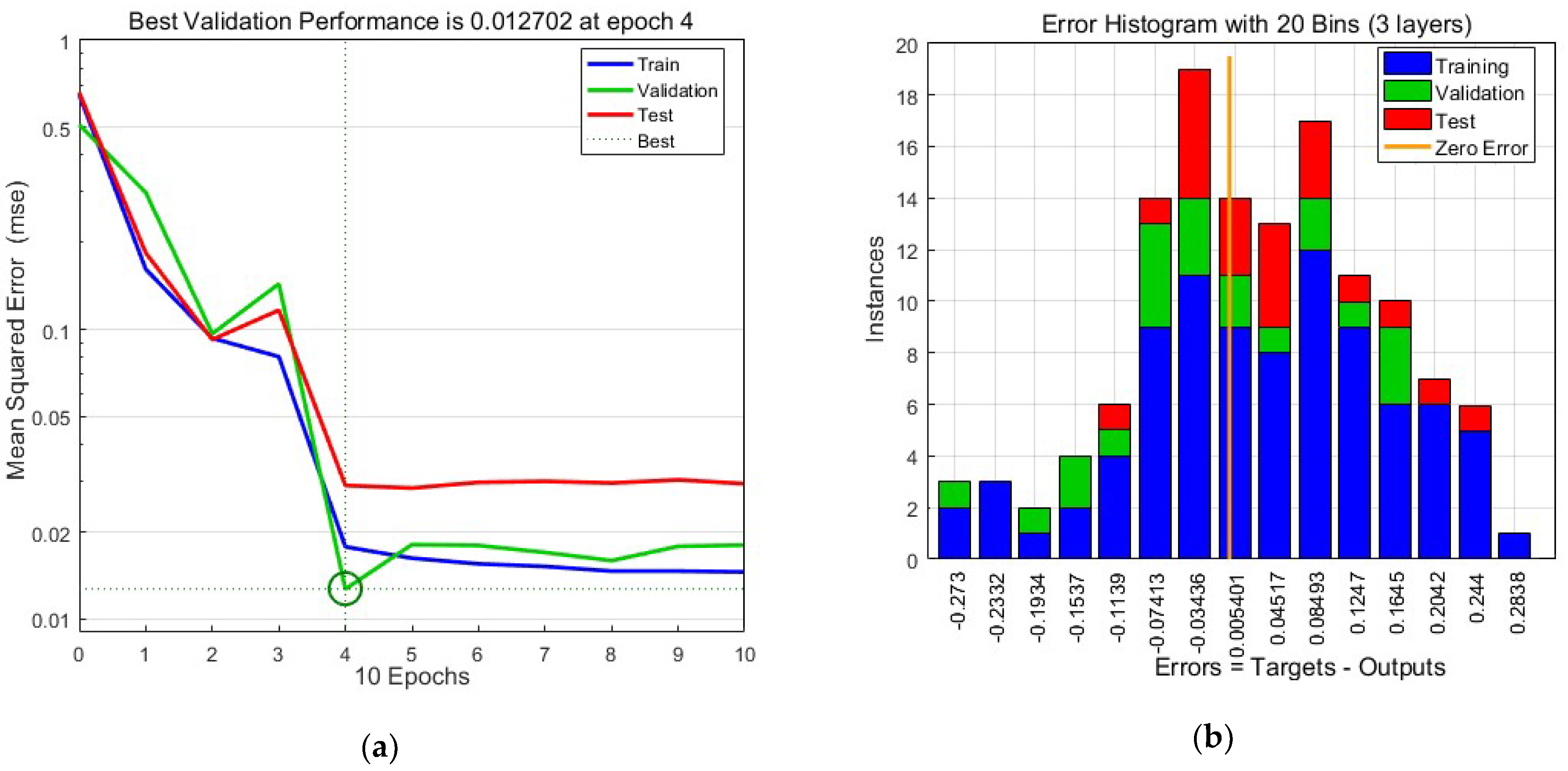


| Property | Value |
|---|---|
| Mass density, kg/m3 | 2400…2550 |
| Fiberglass diameter, μm | 6 |
| Tensile strength, ultimate, MPa, | 2500…3200 |
| Elastic modulus, GPa | 72…78 |
| Poisson ratio | 0.185…0.215 |
| Shear modulus, GPa | 28…30 |
| CTE, linear, μm/m·°C | 5.2…5.5 |
| Specific heat capacity, J/kg·°C | 960…1050 |
| Thermal conductivity, W/m·°C | 1.2…1.25 |
| Property | Value |
|---|---|
| Mass density, kg/m3 | 1200 |
| Elastic modulus, GPa | 2.4…2.7 |
| Poisson ratio | 0.353…0.375 |
| Softening point, °C | 150 |
| CTE, linear, μm/m·°C | 25…30 |
| Linear mold shrinkage, μm/cm | 12…15 |
| Specific heat capacity, J/kg·°C | 960…1050 |
| Thermal conductivity, W/m·°C | 0.32…0.39 |
| Property | Value |
|---|---|
| Mass density, kg/m3 | 1800 |
| Fiber volume fraction | 0.52…0.54 |
| Maximum elastic modulus (±45°), GPa | 24…28 |
| Minimum elastic modulus (0°; 180°), GPa | 8.5…10.0 |
| Poisson ratio (±45°) | 0.72…0.76 |
| Shear modulus (±45°), GPa | 18…22 |
| Property | Value |
|---|---|
| Mass density, kg/m3 | 4600…4750 |
| Titanium ribbon thickness, mm | 0.1 |
| Hardness, Brinell | 170…200 |
| Tensile strength, yield, MPa | 350…380 |
| Shear strength, MPa | 380…400 |
| Elastic modulus, GPa | 102…110 |
| Poisson ratio | 0.32…0.34 |
| Shear modulus, GPa | 39…42 |
| CTE, linear, μm/m °C | 10…12 |
| Specific heat capacity, J/kg °C | 510…540 |
| Thermal conductivity, W/m °C | 16…18 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhilyaev, I.; Chigrinets, E.; Shevtsov, S.; Chotchaeva, S.; Snezhina, N. ANN-Based Estimation of the Defect Severity in the Drilling of GFRP/Ti Multilayered Composite Structure. J. Compos. Sci. 2022, 6, 370. https://doi.org/10.3390/jcs6120370
Zhilyaev I, Chigrinets E, Shevtsov S, Chotchaeva S, Snezhina N. ANN-Based Estimation of the Defect Severity in the Drilling of GFRP/Ti Multilayered Composite Structure. Journal of Composites Science. 2022; 6(12):370. https://doi.org/10.3390/jcs6120370
Chicago/Turabian StyleZhilyaev, Igor, Evgeny Chigrinets, Sergey Shevtsov, Samira Chotchaeva, and Natalia Snezhina. 2022. "ANN-Based Estimation of the Defect Severity in the Drilling of GFRP/Ti Multilayered Composite Structure" Journal of Composites Science 6, no. 12: 370. https://doi.org/10.3390/jcs6120370
APA StyleZhilyaev, I., Chigrinets, E., Shevtsov, S., Chotchaeva, S., & Snezhina, N. (2022). ANN-Based Estimation of the Defect Severity in the Drilling of GFRP/Ti Multilayered Composite Structure. Journal of Composites Science, 6(12), 370. https://doi.org/10.3390/jcs6120370






