Methodology for Evaluating the Performance Data of Practical Honeycomb Fairing
Abstract
:1. Introduction
- selection of the optimal geometric shape of the shelter, based on the layout solution of the entire structure of the object;
- choice of materials and technological solution when creating a shelter shell;
- analysis of the strength component of the manufacture of fairings.
2. Methodology
2.1. Study of Electrical Indicators as Parameters for Assessing the Quality of Honeycomb Fillers
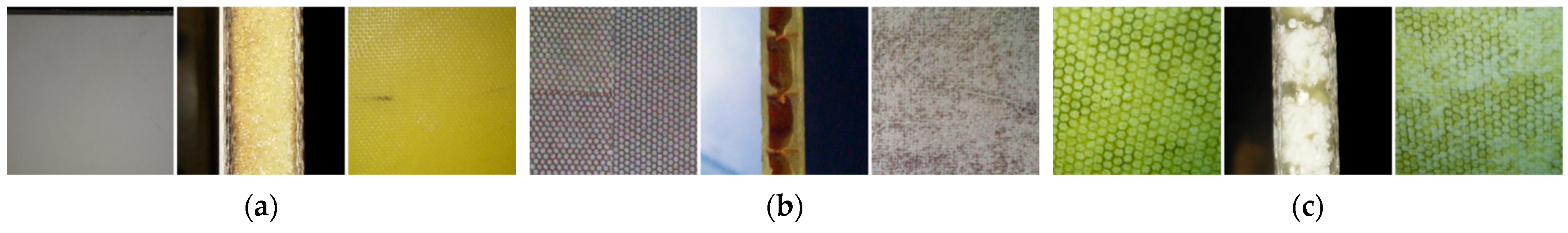
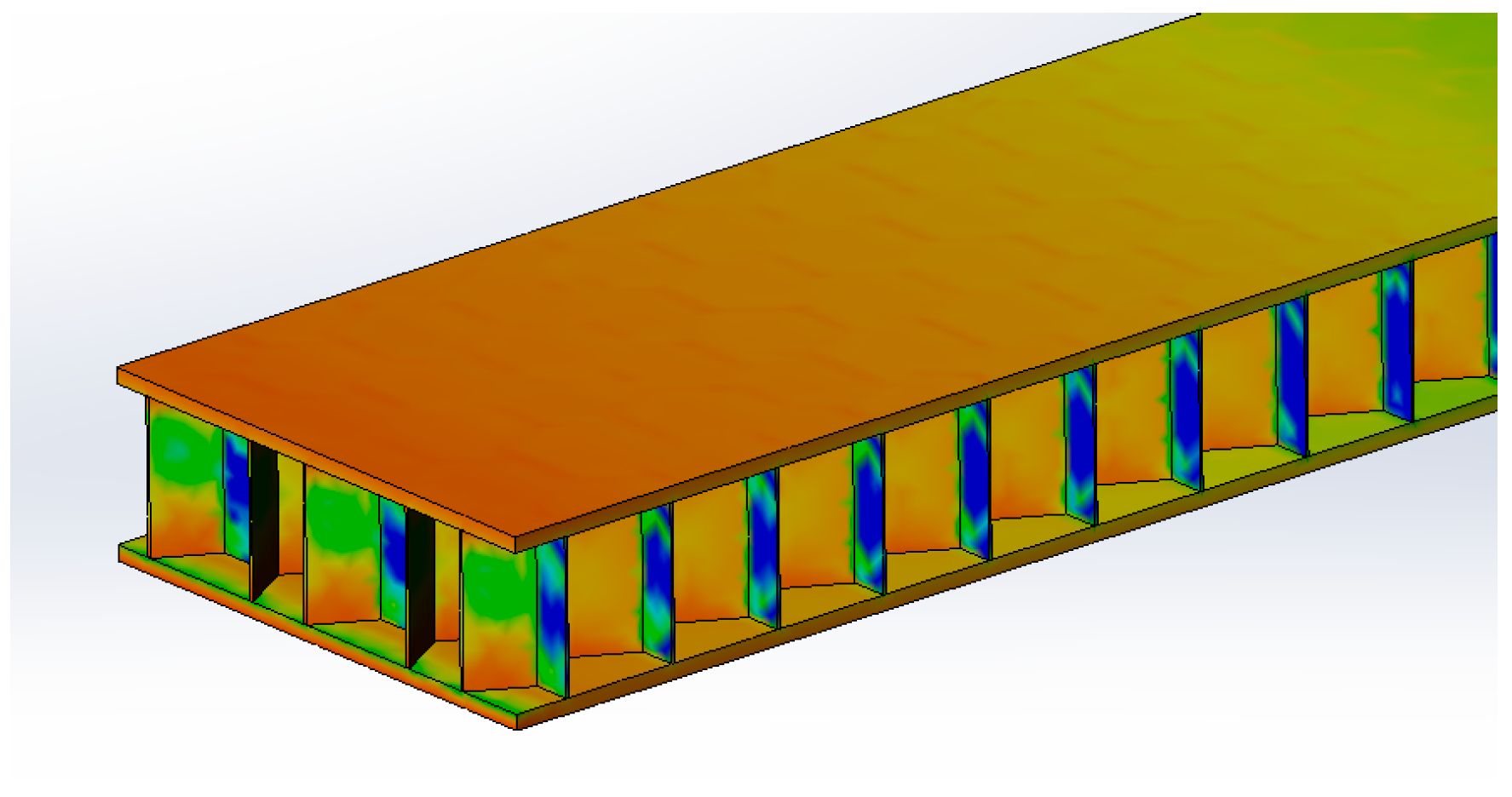
2.2. Methods for Determining the Strength Characteristics of Honeycomb Sandwiches
- for open cell fillers:
- for close cell fillers:
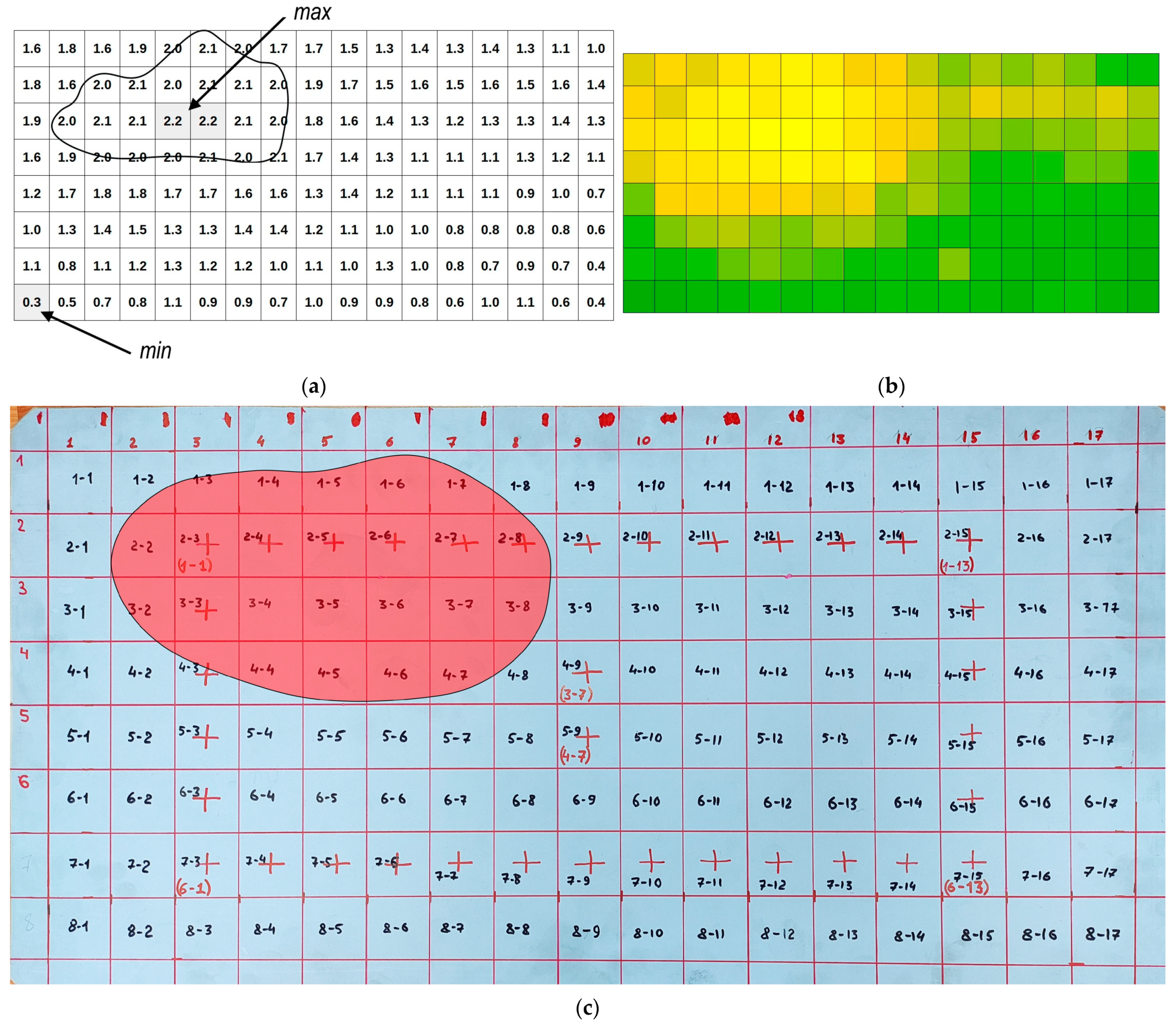
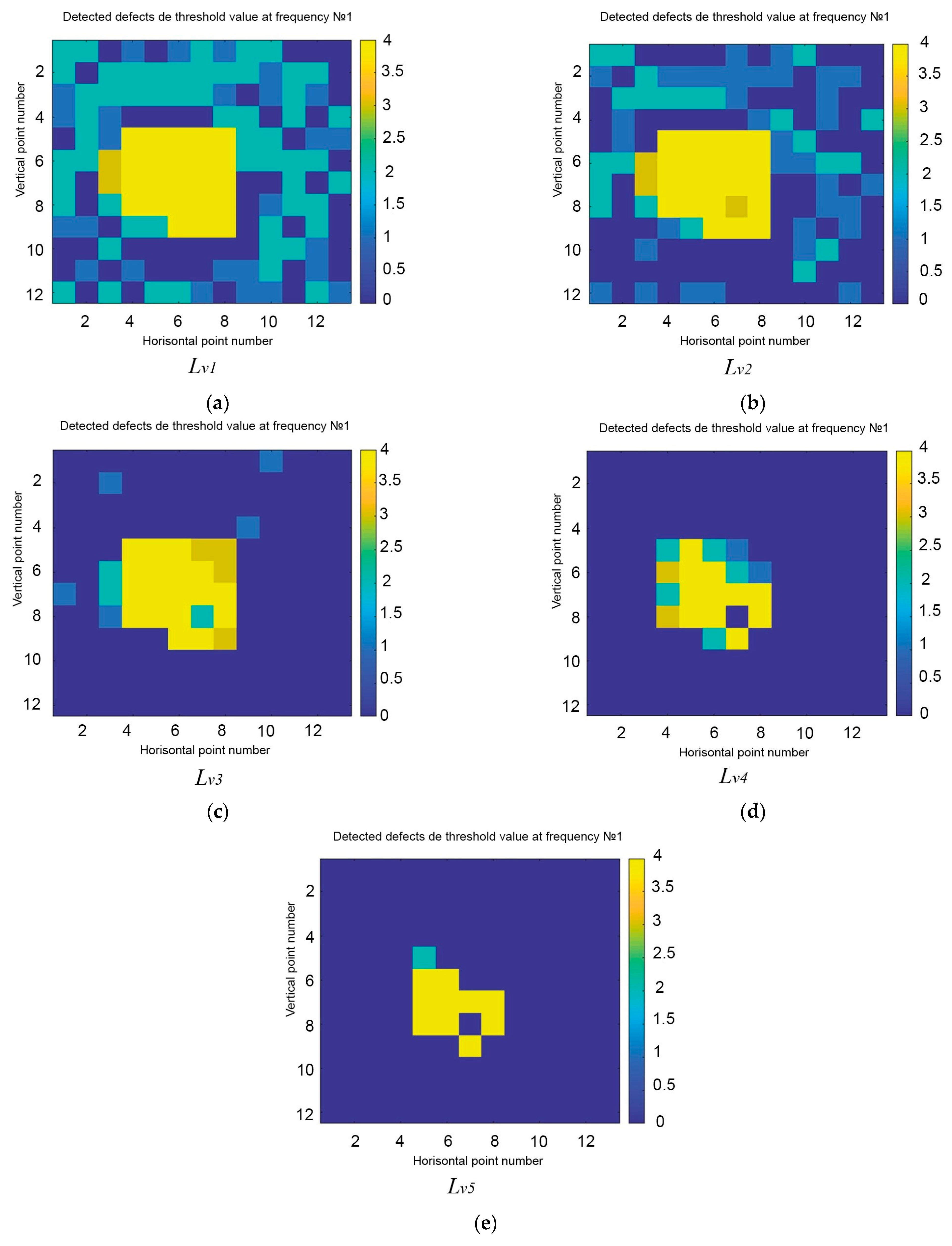
2.3. Evaluation of the Quality of Products by the Parameter of EMW Loss. Determination of Defect Zones
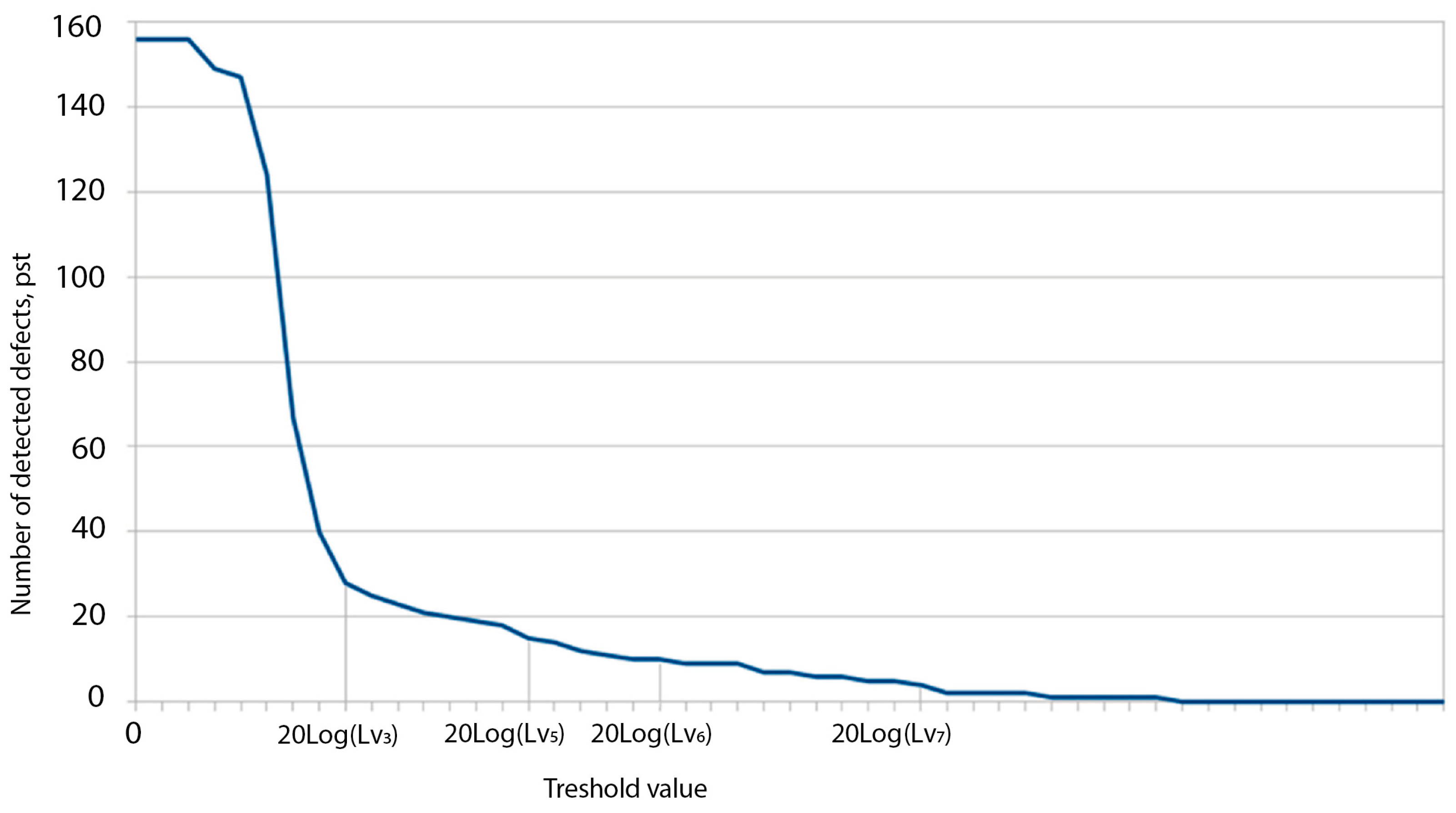
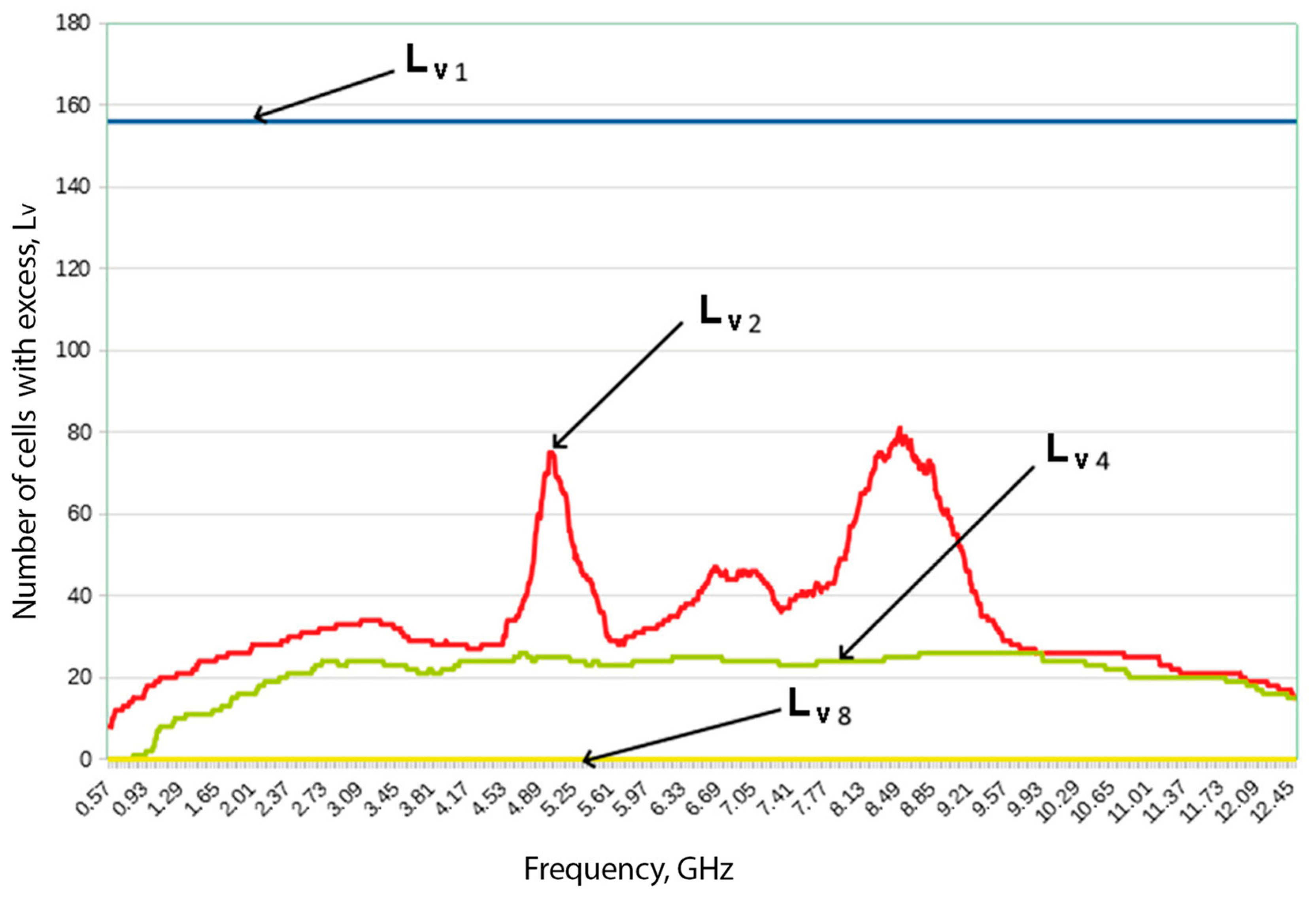
- Assessment of the identity of the structure manufacture by the parameter L.
- Evaluation of the homogeneity of the structure by assessing the stability L in its individual sections.
- Determination of defect zones, their geometric dimensions and position on the structure.
- finding the distribution of EMW losses over the area of defect zone and estimating the coordinates of the cells with the minimum and maximum values;
- calculation of the distance between defect zones;
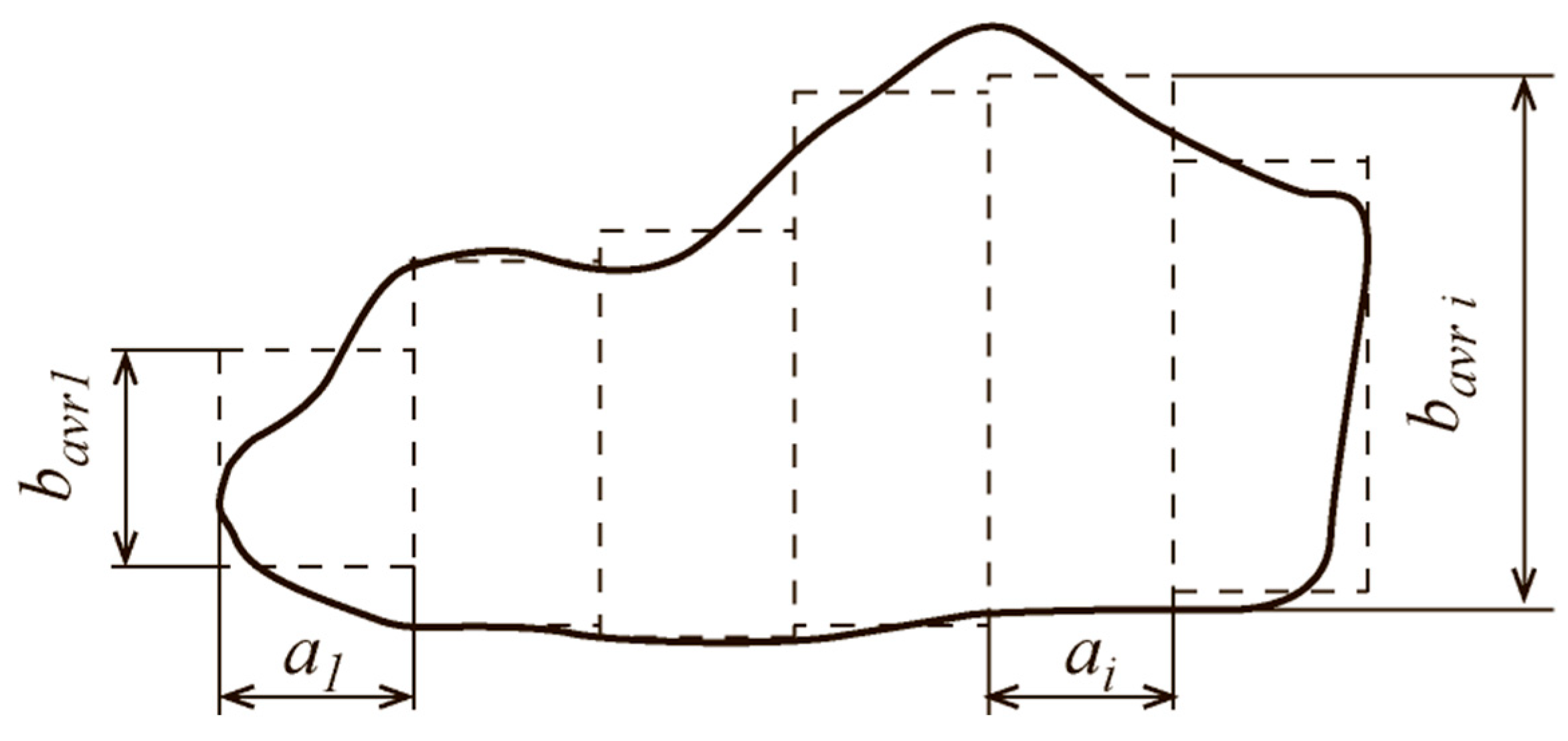
- calculation of the area of defect zone;
- determination of the “center of gravity” (CG) of the defect zone.
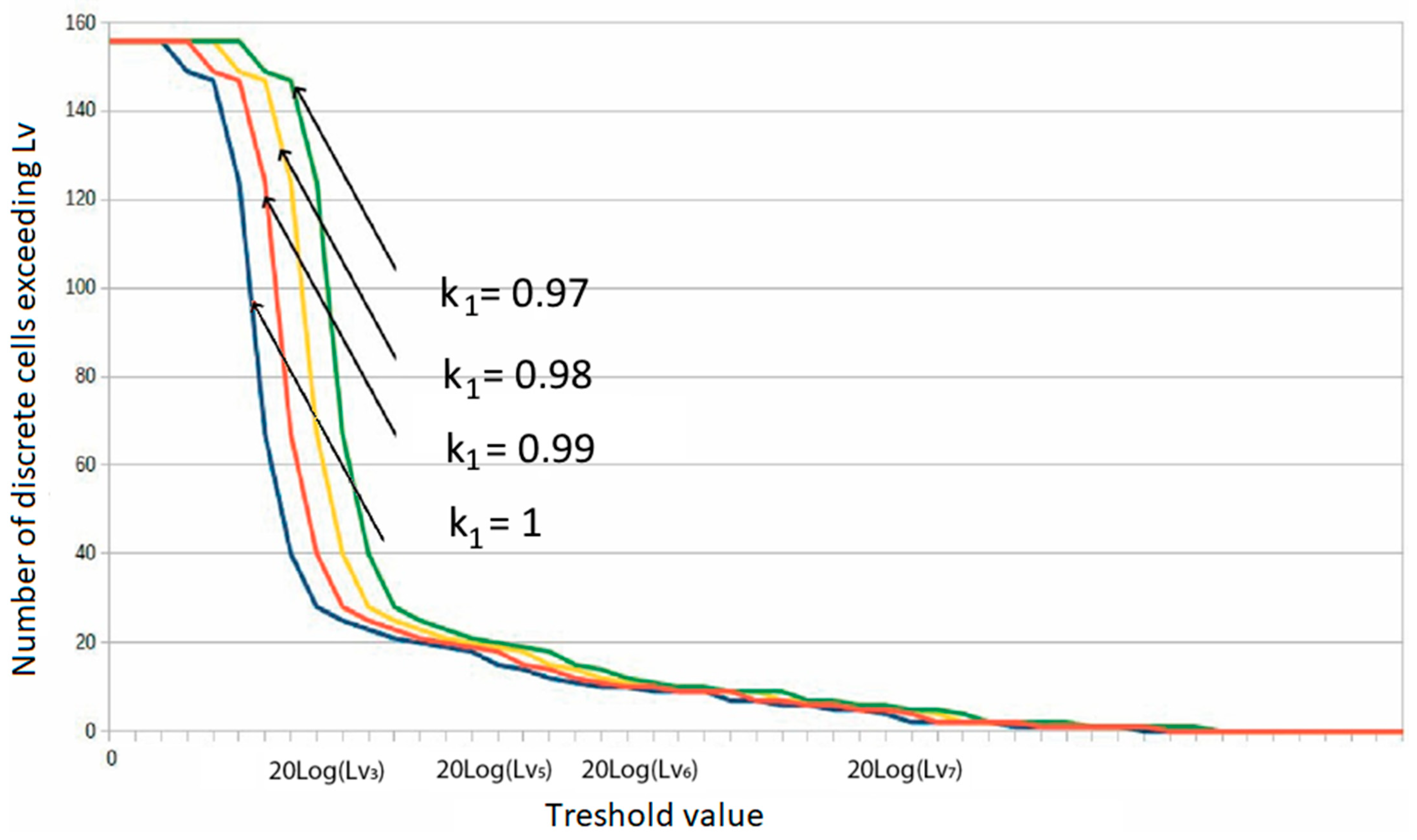
3. Results and Discussion
- evaluate the total area of the defect;
- detect the defect epicenter;
- detect the direction of defect propagation;
- detect critical zones (zones with losses close to Lv).
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AD | antenna devices; |
| RS | radar stations; |
| EMW | electromagnetic wave; |
| RAS | radome antenna system; |
| RTS | radio-transparent shelters; |
| D | directivity of the antenna-fairing; |
| F-test | Fisher’s test; |
| CG | center of gravity. |
References
- Binzer, T.; Waldschmidt, C.; Hellinger, R.; Hansen, T. Radome for a Radar Sensor in a Motor Vehicle and Corresponding Radar Sensor, WO2012013398A1. Available online: https://patentimages.storage.googleapis.com/61/86/3a/0ebe0c954038f2/WO2012013398A1.pdf (accessed on 30 December 2022).
- Chen, H.; Hou, X.; Deng, L. Design of frequency-selective surfaces radome for a planar slotted waveguide antenna. IEEE Antenn. Wir. Prop. Lett. 2009, 8, 1231–1233. [Google Scholar] [CrossRef]
- Gavrilov, D.; Babayan, G.; Losev, V. Thermal imaging control of radio-transparent shelters transmitting headlamps. In Prospects for the Development of Early Warning Radars and Integrated Systems and Complexes for Information Support of Aerospace Defense (RTI Systems VKO-2014); Bauman, N.E., Ed.; Moscow State Technical University: Moscow, Russia, 2014; pp. 165–173. [Google Scholar]
- Bodryshev, V.V.; Larin, A.A.; Rabinsky, L.N. Flaw detection method for radomes in weakly anechoic conditions. TEM J. 2020, 9, 169–176. [Google Scholar]
- Samburov, N.V. Multi-Frequency Method for Measuring Losses in Fairings. Bull. South Ural State Univ. Ser. Comp. Technol. Control Rad. El. 2015, 15, 83–94. [Google Scholar]
- Shalgunov, S.I.; Sokolov, V.I.; Morozova, I.V.; Prokhorova, Y.S. Features of the design and development of radio-transparent fairings and shelters operating in the centimeter and millimeter ranges of radio waves. Antennas 2015, 3, 63–68. [Google Scholar]
- Zhidkova, O.G.; Borodavin, A.V.; Mityushkina, D.V.; Bersekova, N.V. Design of radio transparent structures from composite materials. Struct. Comp. Mat. 2020, 1, 6–12. [Google Scholar]
- Bodryshev, V.V.; Larin, A.A. Analysis of the Influence of the Form of Large-Scale Fairings on the Accuracy of Measuring the Energy Loss Value. News Tula State Univ. Technol. Sci. 2022, 2, 348–353. [Google Scholar]
- Larin, A.A. Method of Evaluation of the Errors of Measuring the Value of Energy Losses in Spherical or Spherocylindrical Fairings. In II International Conference "Composite materials and structures". Abstracts; Moscow Aviation Institute: Moscow, Russia, 2021. [Google Scholar]
- Aksenov, A.V.; Larin, A.A.; Samburov, N.V. An anechoic chamber built into industrial premises. Bull. South Ural State Univ. Ser. Comp. Technol. Man. Radio Electr. 2021, 21, 66–74. [Google Scholar]
- Baskov, K.M.; Politiko, A.A.; Semenenko, V.N.; Chistyaev, V.A.; Akimov, D.I.; Krasnolobov, I.I. Radio wave control of the parameters of samples of multilayer walls of radio-transparent shelters. J. Rad. Electr. 2019, 11, 6. [Google Scholar]
- Endogur, A.I. Design of Aircraft. Airframe Assembly Design; MAI-Print: Moscow, Russia, 2012. [Google Scholar]
- Eliseeva, I.I.; Yuzbashev, M.M. General Theory of Statistics; Finance and Statistics: Moscow, Russia, 2008. [Google Scholar]
- Voskresensky, D.I.; Granovskaya, R.A.; Gostyukhin, V.L.; Filippov, V.S. Antennas and Microwave Devices. Calculation and Design of Antenna Arrays and Their Radiating Elements: Textbook for Universities; Soviet Radio: Moscow, Russia, 1972. [Google Scholar]
- Gibson, L.J.; Ashby, M.F. Cellular Solid Structure and Properties; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Gurtovnik, I.G.; Sokolov, V.I.; Trofimov, N.N.; Shalgunov, S.I. Radiotransparent Products from Fiberglass; Mir: Moscow, Russia, 2002. [Google Scholar]
- Bitkin, V.E.; Zhilkova, O.G.; Denisov, A.V.; Borodavin, A.V.; Matyushit, D.V.; Rodionov, A.V.; Nonin, A.S. Mathematical modeling of the stress-strain state of dimensionally stable composite elements of optical telescope structures using the finite element method. Bull. State Samara Technol. Univ. Ser. Phys. Math. Sci. 2016, 20, 707–729. [Google Scholar]
- Yang, C.C.; Nakae, H. Foaming characteristics control during production of aluminum alloy foam. J. Alloys Compd. 2000, 313, 188–191. [Google Scholar] [CrossRef]

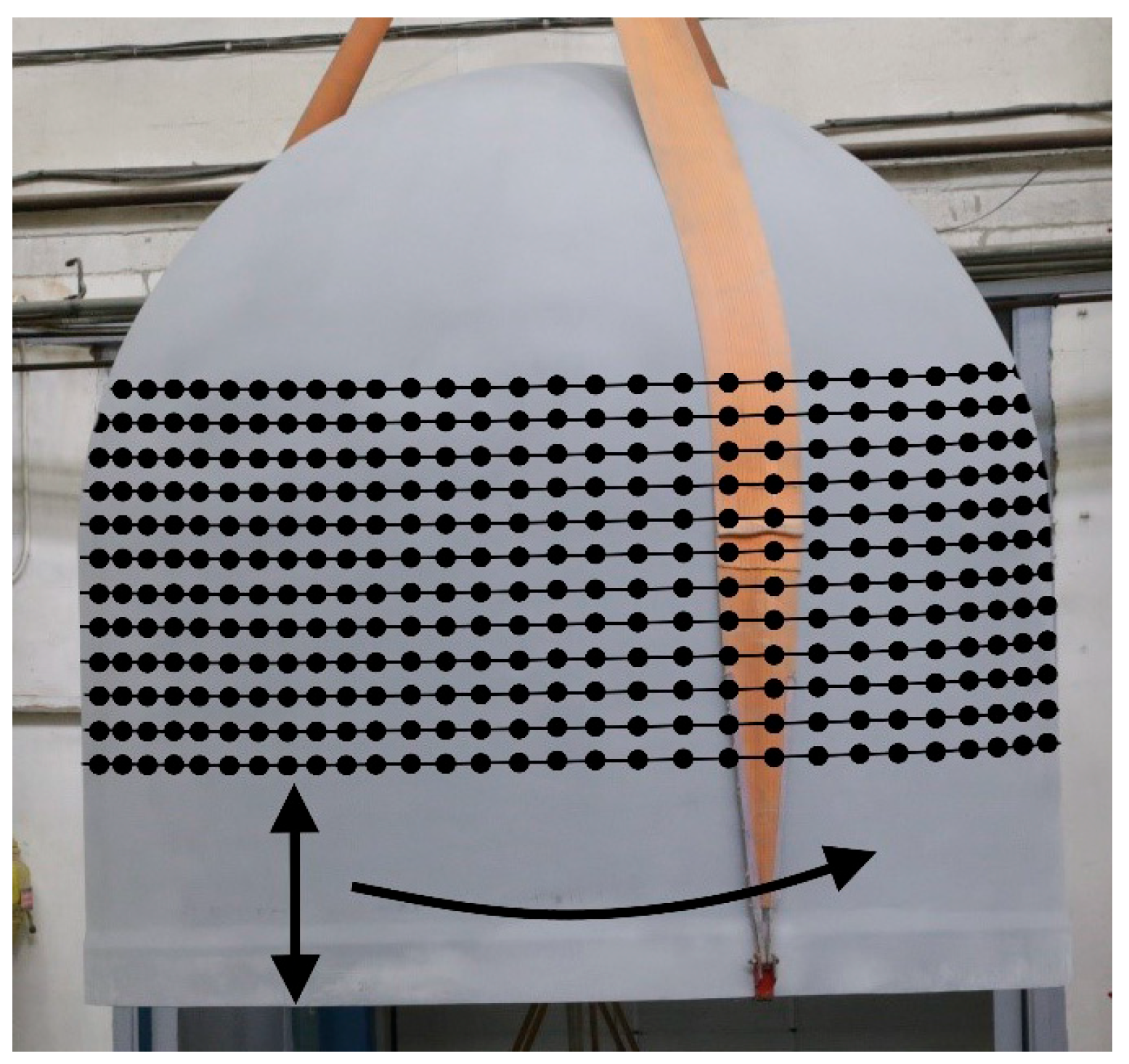
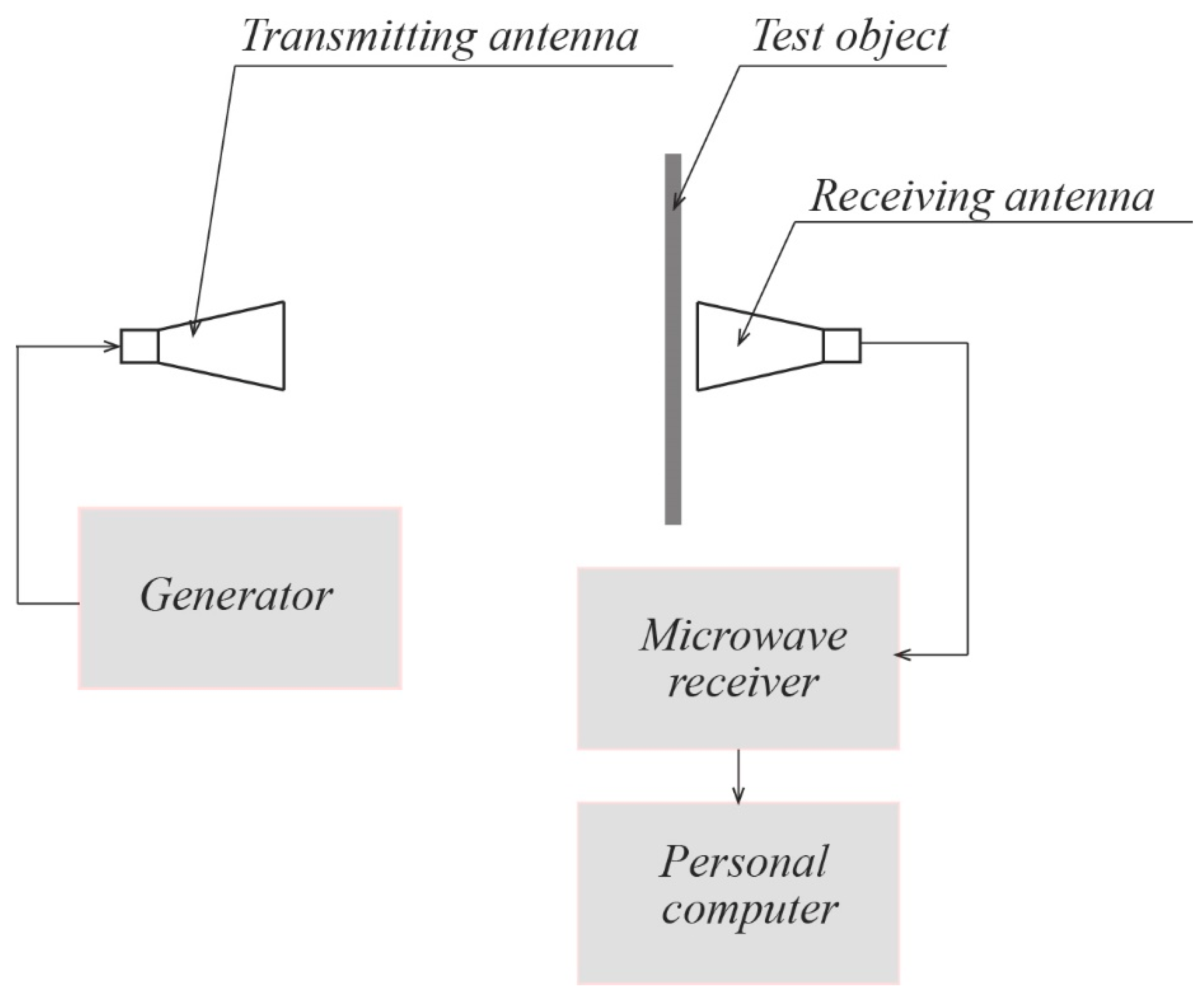

| Stages of Assessment of the Technological Component | Identity Fabrication Design | Technological Identity of Construction Sections | Determination of Defect Zones |
|---|---|---|---|
| Assessment method | 1. One-way analysis of variance using F-test 2. Testing the hypothesis about the average values (t-test) | 1. One-way analysis of variance using F-test 2. Testing the hypothesis about the average values (t-test) | Application of the method of receptor models |
| Tasks to be solved | The problem of checking for the identity of products is considered | The uniformity of the quality of manufacturing of different parts of the product is analyzed. The zones with the largest spread in parameter P are determined | The question of the position of the defect on the product, its area and the center of “severity” is considered, the number of defects and the distance between them are estimated |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bodryshev, V.V.; Larin, A.A.; Rabinskiy, L.N. Methodology for Evaluating the Performance Data of Practical Honeycomb Fairing. Inventions 2023, 8, 42. https://doi.org/10.3390/inventions8010042
Bodryshev VV, Larin AA, Rabinskiy LN. Methodology for Evaluating the Performance Data of Practical Honeycomb Fairing. Inventions. 2023; 8(1):42. https://doi.org/10.3390/inventions8010042
Chicago/Turabian StyleBodryshev, Valeriy V., Artem A. Larin, and Lev N. Rabinskiy. 2023. "Methodology for Evaluating the Performance Data of Practical Honeycomb Fairing" Inventions 8, no. 1: 42. https://doi.org/10.3390/inventions8010042
APA StyleBodryshev, V. V., Larin, A. A., & Rabinskiy, L. N. (2023). Methodology for Evaluating the Performance Data of Practical Honeycomb Fairing. Inventions, 8(1), 42. https://doi.org/10.3390/inventions8010042





