Effect of Temperature and Time on Oxygen Consumption by Olive Fruit: Empirical Study and Simulation in a Non-Ventilated Container
Abstract
1. Introduction
2. Materials and Methods
2.1. Olive Samples
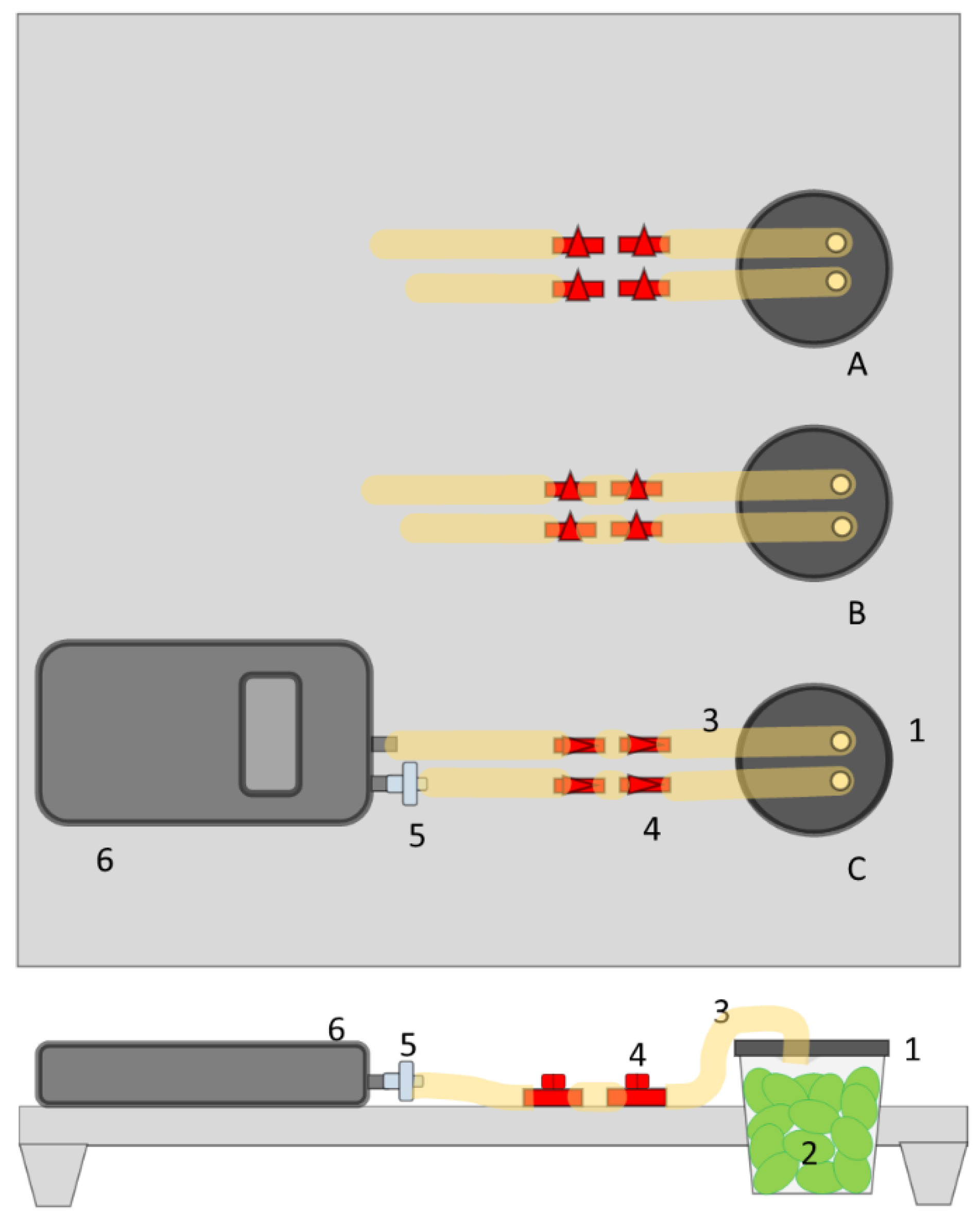
2.2. Experimental Set-Up
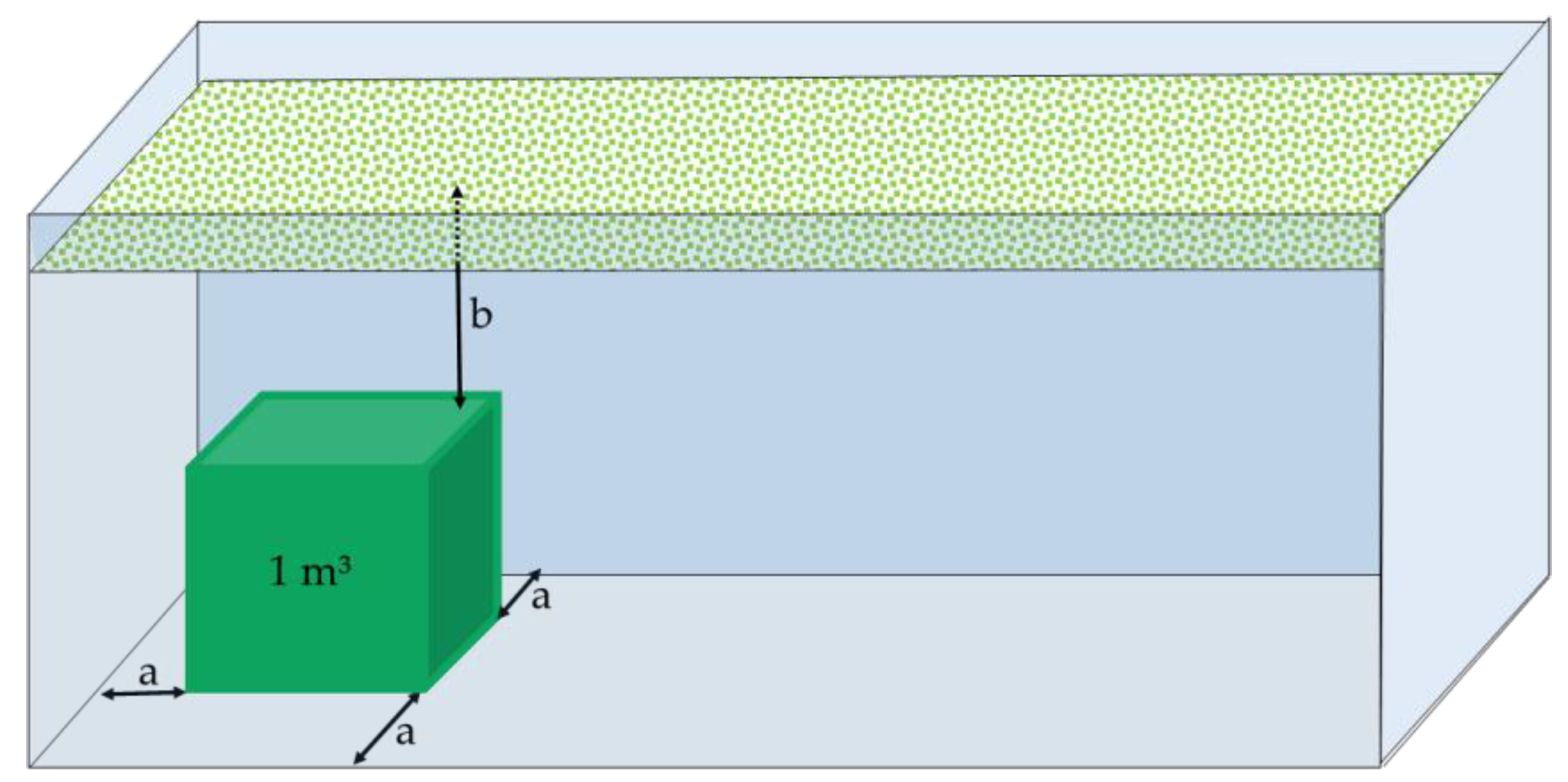
2.3. Theory and Calculus
2.4. Simulation Model
3. Results
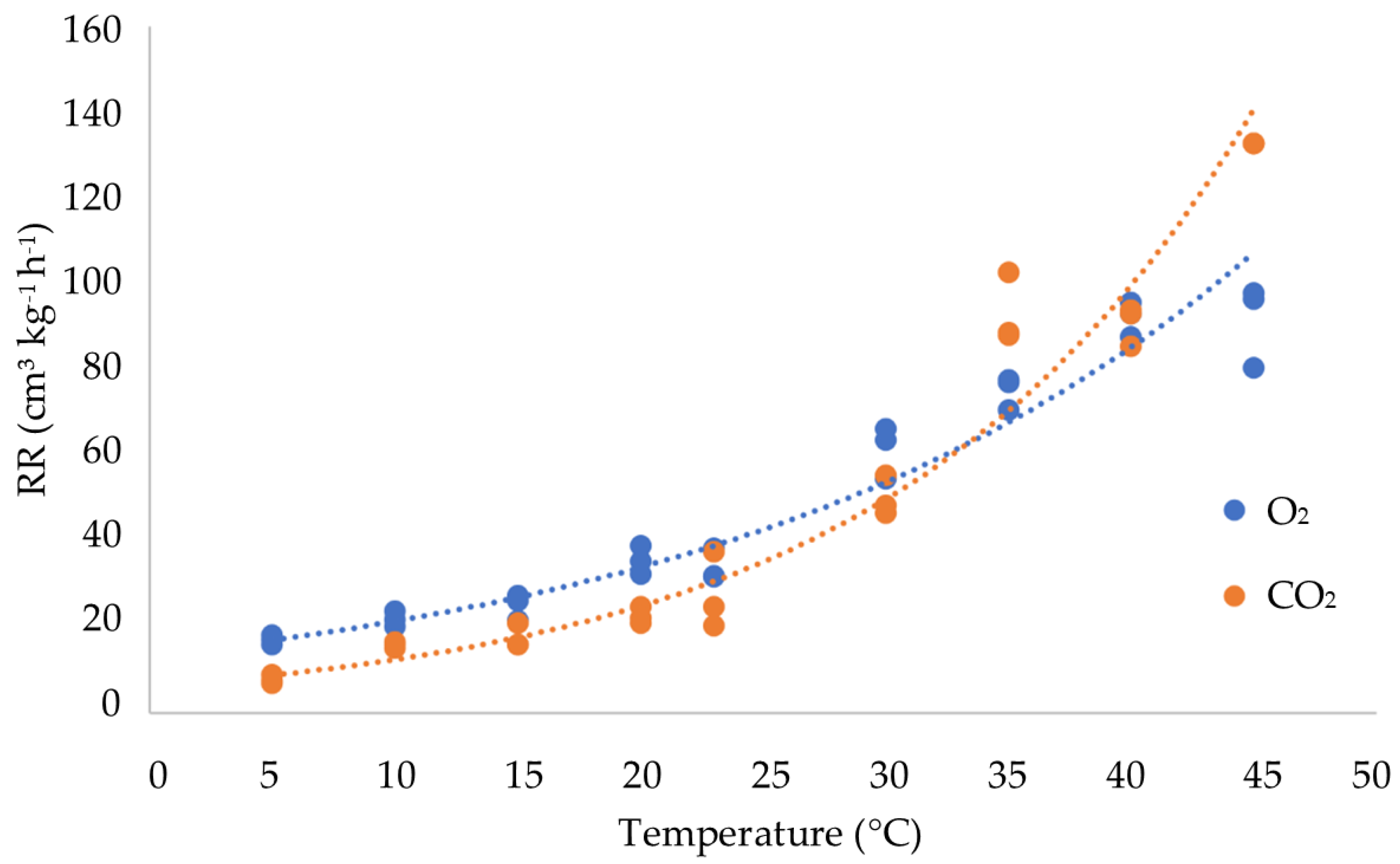
3.1. Concentrations of O2 and CO2 over Time
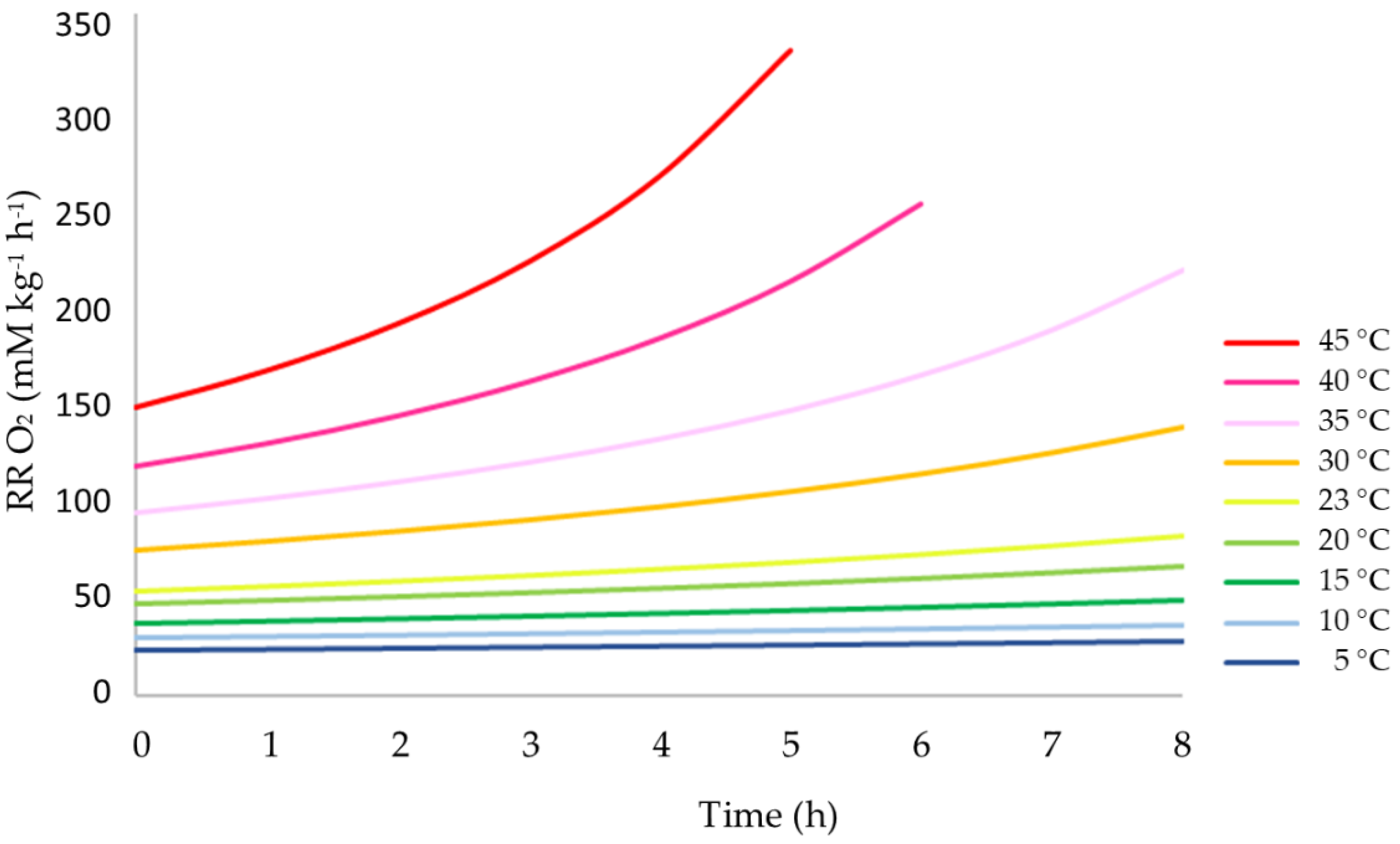
3.2. Influence of Temperature on the Respiration Rate
3.3. Heat Generated by Fruit Respiration
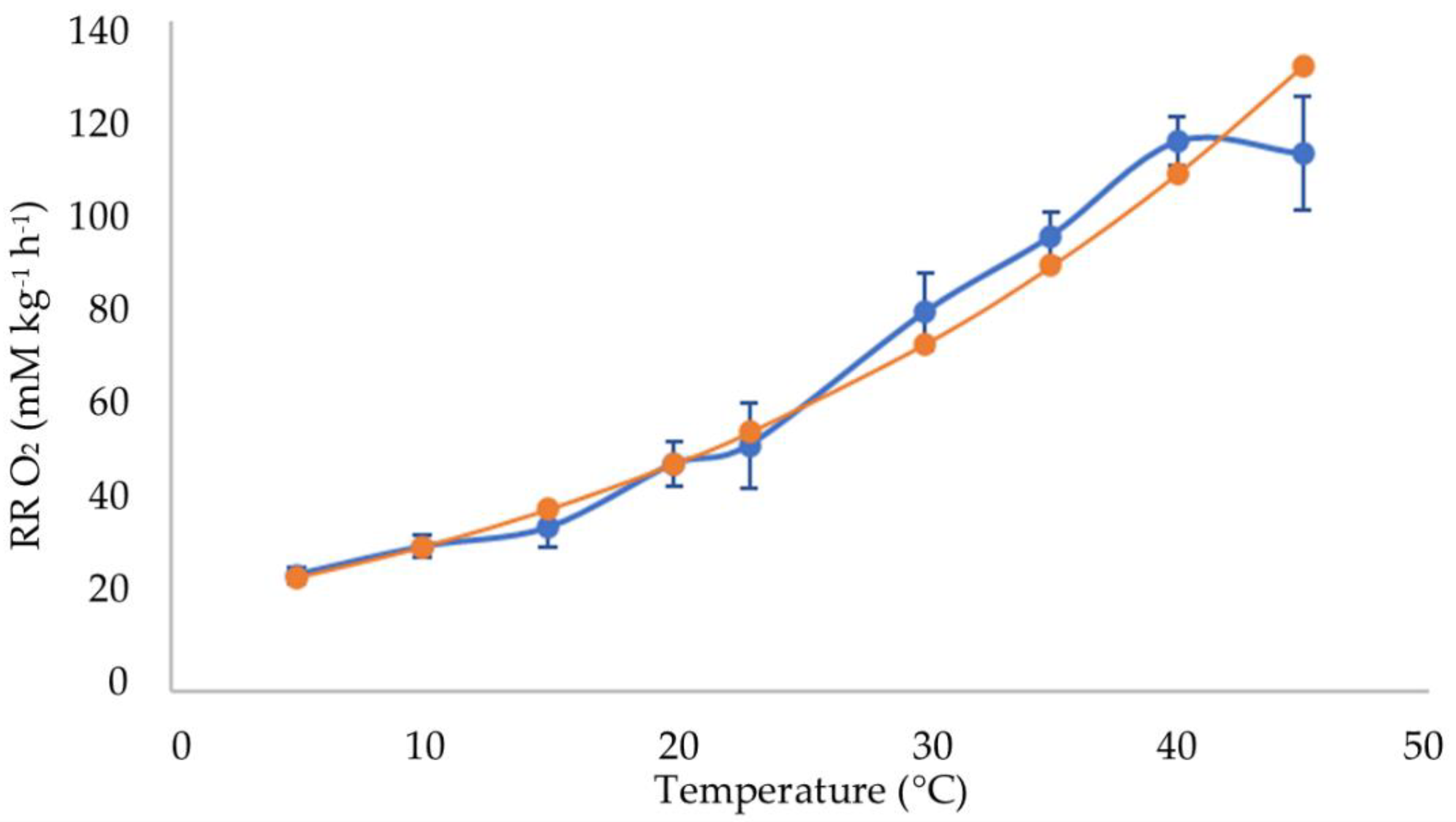
3.4. Consumption of O2 during Storage under Adiabatic Conditions
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Diez, C.M.; Moral, J.; Cabello, D.; Morello, P.; Rallo, L.; Barranco, D. Cultivar and tree density as key factors in the long-term performance of super high-density olive orchards. Front. Plant Sci. 2016, 7, 1–13. [Google Scholar] [CrossRef]
- Servicio de Estudios y Estadísticas de la Junta de Andalucía. Análisis de la Densidad en las Plantaciones de Olivar en Andalucía; Junta de Andalucía: Seville, Spain, 2019. [Google Scholar]
- Connor, D.J.; Gómez-del-Campo, M.; Rousseaux, M.C.; Searles, P.S. Structure, management and productivity of hedgerow olive orchards: A review. Sci. Hortic. 2014, 169, 71–93. [Google Scholar] [CrossRef]
- Rallo, L.; Díez, C.M.; Morales-Sillero, A.; Miho, H.; Priego-Capote, F.; Rallo, P. Quality of olives: A focus on agricultural preharvest factors. Sci. Hortic. 2018, 233, 491–509. [Google Scholar] [CrossRef]
- Fraga, H.; Pinto, J.G.; Santos, J.A. Climate change projections for chilling and heat forcing conditions in European vineyards and olive orchards: A multi-model assessment. Clim. Change 2019, 152, 179–193. [Google Scholar] [CrossRef]
- Arenas-Castro, S.; Gonçalves, J.F.; Moreno, M.; Villar, R. Projected climate changes are expected to decrease the suitability and production of olive varieties in southern Spain. Sci. Total Environ. 2020, 709, 136161. [Google Scholar] [CrossRef]
- Mihailescu, E.; Soares, M.B. The influence of climate on agricultural decisions for three European crops: A systematic review. Front. Sustain. Food Syst. 2020, 4, 64. [Google Scholar] [CrossRef]
- Sousa, A.A.R.; Barandica, J.M.; Aguilera, P.A.; Rescia, A.J. Examining potential environmental consequences of climate change and other driving forces on the sustainability of Spanish olive groves under a socio-ecological approach. Agriculture 2020, 10, 509. [Google Scholar] [CrossRef]
- Galán, C.; García-Mozo, H.; Vázquez, L.; Ruiz, L.; de La Guardia, C.D.; Trigo, M.M. Heat requirement for the onset of the Olea europaea L. pollen season in several sites in Andalusia and the effect of the expected future climate change. Int. J. Biometeorol. 2005, 49, 184–188. [Google Scholar] [CrossRef] [PubMed]
- Avolio, E.; Orlandi, F.; Bellecci, C.; Fornaciari, M.; Federico, S. Assessment of the impact of climate change on the olive flowering in Calabria (southern Italy). Theor. Appl. Climatol. 2012, 107, 531–540. [Google Scholar] [CrossRef]
- García-Mozo, H.; Oteros, J.; Galán, C. Phenological changes in olive (ola europaea l.) reproductive cycle in southern Spain due to climate change. Ann. Agric. Environ. Med. 2015, 22, 421–428. [Google Scholar] [CrossRef] [PubMed]
- Gabaldón-Leal, C.; Ruiz-Ramos, M.; de la Rosa, R.; León, L.; Belaj, A.; Rodríguez, A.; Santos, C.; Lorite, I.J. Impact of changes in mean and extreme temperatures caused by climate change on olive flowering in southern Spain. Int. J. Climatol. 2017, 37, 940–957. [Google Scholar] [CrossRef]
- Saltveit, M.E. Respiratory Metabolism. In The Commercial Storage of Fruits, Vegetables, and Florist and Nursery Stocks. Agriculture Handbook 66; Gross, K.C., Wang, C.Y., Saltveit, M., Eds.; United States Department of Agriculture: Washington, DC, USA, 2016; pp. 68–75. [Google Scholar]
- Fonseca, S.C.; Oliveira, F.A.R.; Brecht, J.K. Modelling respiration rate of fresh fruits and vegetables for modified atmosphere packages: A review. J. Food Eng. 2002, 52, 99–119. [Google Scholar] [CrossRef]
- Bendini, A.; Valli, E.; Cerretani, L.; Chiavaro, E.; Lercker, G. Study on the effects of heating of virgin olive oil blended with mildly deodorized olive oil: Focus on the hydrolytic and oxidative state. J. Agric. Food Chem. 2009, 57, 10055–10062. [Google Scholar] [CrossRef] [PubMed]
- Gómez-Coca, R.B.; Cruz-Hidalgo, R.; Fernandes, G.D.; Pérez-Camino, M.D.C.; Moreda, W. Analysis of methanol and ethanol in virgin olive oil. MethodsX 2014, 1, e207–e211. [Google Scholar] [CrossRef][Green Version]
- García-Vico, L.; Belaj, A.; León, L.; de la Rosa, R.; Sanz, C.; Pérez, A.G. A survey of ethanol content in virgin olive oil. Food Control 2018, 91, 248–253. [Google Scholar] [CrossRef]
- Beltran, G.; Sánchez, R.; Sánchez-Ortiz, A.; Aguilera, M.P.; Bejaoui, M.A.; Jimenez, A. How ‘ground-picked’ olive fruits affect virgin olive oil ethanol content, ethyl esters and quality. J. Sci. Food Agric. 2016, 96, 3801–3806. [Google Scholar] [CrossRef] [PubMed]
- Jabeur, H.; Zribi, A.; Abdelhedi, R.; Bouaziz, M. Effect of olive storage conditions on Chemlali olive oil quality and the effective role of fatty acids alkyl esters in checking olive oils authenticity. Food Chem. 2015, 169, 289–296. [Google Scholar] [CrossRef] [PubMed]
- Plasquy, E.; García Martos, J.M.; Florido, M.C.; Sola-guirado, R.R.; García Martín, J.F. Cold storage and temperature management of olive fruit: The impact on fruit physiology and olive oil quality—A review. Processes 2021, 9, 1543. [Google Scholar] [CrossRef]
- Beltran, G.; Hueso, A.; Bejaoui, M.A.; Gila, A.M.; Costales, R.; Sánchez-Ortiz, A.; Aguilera, M.P.; Jimenez, A. How olive washing and storage affect fruit ethanol and virgin olive oil ethanol, ethyl esters and composition. J. Sci. Food Agric. 2021, 101, 3714–3722. [Google Scholar] [CrossRef]
- García, J.M.; Streif, J. The effect of controlled atmosphere storage on fruit and oil quality of “Gordal” olives. Gartenbauwissenschaft 1991, 56, 233–238. [Google Scholar]
- Agar, I.T.; Hess-Pierce, B.; Sourour, M.M.; Kader, A.A. Quality of Fruit and Oil of Black-Ripe Olives Is Influenced by Cultivar and Storage Period. J. Agric. Food Chem. 1998, 46, 3415–3421. [Google Scholar] [CrossRef]
- Yousfi, K.; Cayuela, J.A.; García, J.M. Effect of Temperature, modified atmosphere and ethylene during olive storage on quality and bitterness level of the oil. JAOCS J. Am. Oil Chem. Soc. 2009, 86, 291–296. [Google Scholar] [CrossRef]
- Morales-Sillero, A.; Pérez, A.G.; Casanova, L.; García, J.M. Cold storage of ‘Manzanilla de Sevilla’ and ‘Manzanilla Cacereña’ mill olives from super-high density orchards. Food Chem. 2017, 237, 1216–1225. [Google Scholar] [CrossRef] [PubMed]
- Plasquy, E.; Blanco-Roldán, G.; Florido, M.C.; García, J.M. Effects of an integrated harvest system for small producers on the quality of the recollected olive fruit. Grasas y Aceites 2021, 72, e436. [Google Scholar]
- García, P.; Brenes, M.; Romero, C.; Garrido, A. Respiration and physicochemical changes in harvested olive fruits. J. Hortic. Sci. 1995, 70, 925–933. [Google Scholar] [CrossRef]
- Plasquy, E.; Garcia, J.M.; Florido, M.C.; Sola-guirado, R.R. Estimation of the cooling rate of six olive cultivars using thermal imaging. Agriculture 2021, 11, 164. [Google Scholar] [CrossRef]
- Plasquy, E.; García Martos, J.M.; Florido Fernández, M.D.C.; Sola-Guirado, R.R.; García Martín, J.F. Adjustment of olive fruit temperature before grinding for olive oil extraction. experimental study and pilot plant trials. Processes 2021, 9, 586. [Google Scholar] [CrossRef]
- Kader, A.A. Respiration and gas exchange of vegetables. In Postharvest Physiology of Vegetables; Weichmann, J., Ed.; Marcel Dekker: New York, NY, USA, 1987; pp. 25–43. [Google Scholar]
- Waghmare, R.B.; Mahajan, P.V.; Annapure, U.S. Modelling the effect of time and temperature on respiration rate of selected fresh-cut produce. Postharvest Biol. Technol. 2013, 80, 25–30. [Google Scholar] [CrossRef]
- Redding, G.P.; Yang, A.; Shim, Y.M.; Olatunji, J.; East, A. A review of the use and design of produce simulators for horticultural forced-air cooling studies. J. Food Eng. 2016, 190, 80–93. [Google Scholar] [CrossRef]
- Bower, J.H.; Jobling, J.J.; Patterson, B.D.; Ryan, D.J. A method for measuring the respiration rate and respiratory quotient of detached plant tissues. Postharvest Biol. Technol. 1998, 13, 263–270. [Google Scholar] [CrossRef]
- Bhande, S.D.; Ravindra, M.R.; Goswami, T.K. Respiration rate of banana fruit under aerobic conditions at different storage temperatures. J. Food Eng. 2008, 87, 116–123. [Google Scholar] [CrossRef]
- Caleb, O.J.; Mahajan, P.V.; Opara, U.L.; Witthuhn, C.R. Modelling the respiration rates of pomegranate fruit and arils. Postharvest Biol. Technol. 2012, 64, 49–54. [Google Scholar] [CrossRef]
- Iqbal, T.; Rodrigues, F.A.S.; Mahajan, P.V.; Kerry, J.P. Effect of Time, temperature, and slicing on respiration rate of mushrooms. J. Food Sci. 2009, 74, E298–E303. [Google Scholar] [CrossRef] [PubMed]
- Exama, A.; Arul, J.; Lencki, R.W.; Lee, I.Z.; Toupin, C. Suitability of plastic films for modified atmosphere packaging of fruits and vegetables. J. Food Sci. 1993, 58, 1365–1370. [Google Scholar] [CrossRef]
- Zagory, D.; Kader, A. Modified atmosphere packaging of fruits and vegetables. Food Technol. 1988, 42, 70–77. [Google Scholar]
- Fidler, J.C.; North, C.J. The effect of conditions of storage on the respiration of apples. I. The effects of temperature and concentrations of carbon dioxide and oxygen on the production of carbon dioxide and uptake of oxygen. J. Hortic. Sci. 1967, 42, 189–206. [Google Scholar] [CrossRef]
- García, J.M.; Yousfi, K. The postharvest of mill olives. Grasas y Aceites 2006, 57, 16–24. [Google Scholar] [CrossRef]
- Clodoveo, M.L.; Delcuratolo, D.; Gomes, T.; Colelli, G. Effect of different temperatures and storage atmospheres on Coratina olive oil quality. Food Chem. 2007, 102, 571–576. [Google Scholar] [CrossRef]
- Kalua, C.M.; Bedgood, D.R.; Bishop, A.G.; Prenzler, P.D. Changes in virgin olive oil quality during low-temperature fruit storage. J. Agric. Food Chem. 2008, 56, 2415–2422. [Google Scholar] [CrossRef]
- Tanner, D.J.; Cleland, A.C.; Opara, L.U.; Robertson, T.R. A generalised mathematical modelling methodology for design of horticultural food packages exposed to refrigerated conditions: Part 1, formulation. Int. J. Refrig. 2002, 25, 33–42. [Google Scholar] [CrossRef]
- Ferrua, M.J.; Singh, R.P. Modeling the forced-air cooling process of fresh strawberry packages, Part I: Numerical model. Int. J. Refrig. 2009, 32, 335–348. [Google Scholar] [CrossRef]
- Dehghannya, J.; Ngadi, M.; Vigneault, C. Mathematical Modeling Procedures for Airflow, Heat and Mass Transfer during Forced Convection Cooling of Produce: A Review. Food Eng. Rev. 2010, 2, 227–243. [Google Scholar] [CrossRef]
- Zhao, C.J.; Han, J.W.; Yang, X.T.; Qian, J.P.; Fan, B.L. A review of computational fluid dynamics for forced-air cooling process. Appl. Energy 2016, 168, 314–331. [Google Scholar] [CrossRef]
- Sola-Guirado, R.R.; Aragon-Rodriguez, F.; Castro-Garcia, S.; Gil-Ribes, J. The vibration behaviour of hedgerow olive trees in response to mechanical harvesting with straddle harvester. Biosyst. Eng. 2019, 184, 81–89. [Google Scholar] [CrossRef]


| Time | O2 (mg/golive) | ||||||||
| (h) | 5 °C | 10 °C | 15 °C | 20 °C | 23 °C | 30 °C | 35 °C | 40 °C | 45 °C |
| 0 | 0.55 ± 0.02 | 0.46 ± 0.00 | 0.50 ± 0.01 | 0.53 ± 0.02 | 0.53 ± 0.01 | 0.53 ± 0.02 | 0.48 ± 0.01 | 0.48 ± 0.01 | 0.40 ± 0.02 |
| 1 | 0.53 ± 0.02 | 0.42 ± 0.01 | 0.47 ± 0.01 | 0.49 ± 0.02 | 0.49 ± 0.04 | 0.42 ± 0.01 | 0.39 ± 0.01 | 0.36 ± 0.01 | 0.27 ± 0.02 |
| 2 | 0.51 ± 0.02 | 0.41 ± 0.00 | 0.44 ± 0.01 | 0.42 ± 0.02 | 0.45 ± 0.04 | 0.35 ± 0.01 | 0.27 ± 0.02 | 0.26 ± 0.03 | 0.19 ± 0.02 |
| 3 | 0.50 ± 0.02 | 0.39 ± 0.01 | 0.42 ± 0.01 | 0.37 ± 0.02 | 0.40 ± 0.03 | 0.30 ± 0.00 | 0.19 ± 0.03 | 0.16 ± 0.01 | 0.00 ± 0.00 |
| 4 | 0.50 ± 0.02 | 0.40 ± 0.01 | 0.39 ± 0.01 | 0.33 ± 0.01 | 0.36 ± 0.04 | 0.22 ± 0.00 | 0.15 ± 0.03 | 0.07 ± 0.02 | 0.00 ± 0.00 |
| 5 | 0.49 ± 0.02 | 0.37 ± 0.01 | 0.37 ± 0.02 | 0.28 ± 0.02 | 0.32 ± 0.04 | 0.16 ± 0.00 | 0.13 ± 0.03 | 0.04 ± 0.01 | 0.00 ± 0.00 |
| 6 | 0.47 ± 0.03 | 0.37 ± 0.01 | 0.35 ± 0.02 | 0.26 ± 0.02 | 0.28 ± 0.03 | 0.13 ± 0.00 | 0.12 ± 0.03 | 0.03 ± 0.01 | 0.00 ± 0.00 |
| 7 | 0.48 ± 0.02 | 0.35 ± 0.01 | 0.33 ± 0.02 | 0.24 ± 0.02 | 0.25 ± 0.03 | 0.11 ± 0.00 | 0.10 ± 0.03 | 0.04 ± 0.01 | 0.00 ± 0.00 |
| Time | CO2 (mg/golive) | ||||||||
| (h) | 5 °C | 10 °C | 15 °C | 20 °C | 23 °C | 30 °C | 35 °C | 40 °C | 45 °C |
| 0 | 0.01 ± 0.00 | 0.02 ± 0.00 | 0.01 ± 0.00 | 0.00 ± 0.00 | 0.01 ± 0.00 | 0.00 ± 0.00 | 0.02 ± 0.00 | 0.01 ± 0.00 | 0.01 ± 0.00 |
| 1 | 0.04 ± 0.00 | 0.06 ± 0.00 | 0.04 ± 0.00 | 0.04 ± 0.00 | 0.06 ± 0.00 | 0.15 ± 0.00 | 0.17 ± 0.00 | 0.20 ± 0.00 | 0.22 ± 0.00 |
| 2 | 0.04 ± 0.00 | 0.07 ± 0.00 | 0.07 ± 0.00 | 0.09 ± 0.00 | 0.11 ± 0.00 | 0.23 ± 0.00 | 0.35 ± 0.00 | 0.34 ± 0.00 | 0.53 ± 0.00 |
| 3 | 0.05 ± 0.00 | 0.09 ± 0.00 | 0.10 ± 0.00 | 0.13 ± 0.00 | 0.16 ± 0.00 | 0.29 ± 0.00 | 0.49 ± 0.00 | 0.48 ± 0.00 | 0.63 ± 0.00 |
| 4 | 0.05 ± 0.00 | 0.10 ± 0.00 | 0.13 ± 0.00 | 0.17 ± 0.00 | 0.21 ± 0.00 | 0.39 ± 0.00 | 0.60 ± 0.00 | 0.64 ± 0.00 | 0.63 ± 0.00 |
| 5 | 0.06 ± 0.00 | 0.11 ± 0.00 | 0.15 ± 0.00 | 0.20 ± 0.00 | 0.25 ± 0.00 | 0.46 ± 0.00 | 0.65 ± 0.00 | 0.73 ± 0.00 | 0.63 ± 0.00 |
| 6 | 0.06 ± 0.00 | 0.11 ± 0.00 | 0.17 ± 0.00 | 0.23 ± 0.00 | 0.28 ± 0.00 | 0.49 ± 0.00 | 0.68 ± 0.00 | 0.73 ± 0.00 | 0.63 ± 0.00 |
| 7 | 0.06 ± 0.00 | 0.12 ± 0.00 | 0.19 ± 0.00 | 0.24 ± 0.00 | 0.31 ± 0.00 | 0.52 ± 0.00 | 0.70 ± 0.00 | 0.74 ± 0.00 | 0.63 ± 0.00 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Plasquy, E.; Florido, M.C.; Sola-Guirado, R.R.; García Martos, J.M.; García Martín, J.F. Effect of Temperature and Time on Oxygen Consumption by Olive Fruit: Empirical Study and Simulation in a Non-Ventilated Container. Fermentation 2021, 7, 200. https://doi.org/10.3390/fermentation7040200
Plasquy E, Florido MC, Sola-Guirado RR, García Martos JM, García Martín JF. Effect of Temperature and Time on Oxygen Consumption by Olive Fruit: Empirical Study and Simulation in a Non-Ventilated Container. Fermentation. 2021; 7(4):200. https://doi.org/10.3390/fermentation7040200
Chicago/Turabian StylePlasquy, Eddy, María C. Florido, Rafael Rubén Sola-Guirado, José María García Martos, and Juan Francisco García Martín. 2021. "Effect of Temperature and Time on Oxygen Consumption by Olive Fruit: Empirical Study and Simulation in a Non-Ventilated Container" Fermentation 7, no. 4: 200. https://doi.org/10.3390/fermentation7040200
APA StylePlasquy, E., Florido, M. C., Sola-Guirado, R. R., García Martos, J. M., & García Martín, J. F. (2021). Effect of Temperature and Time on Oxygen Consumption by Olive Fruit: Empirical Study and Simulation in a Non-Ventilated Container. Fermentation, 7(4), 200. https://doi.org/10.3390/fermentation7040200










