Onset of Thermal Instabilities in the Plane Poiseuille Flow of Weakly Elastic Fluids: Viscous Dissipation Effects
Abstract
:1. Introduction
2. Governing Equations and Linear Stability Analysis
2.1. Transverse Rolls
2.2. Longitudinal Rolls
3. Discussion
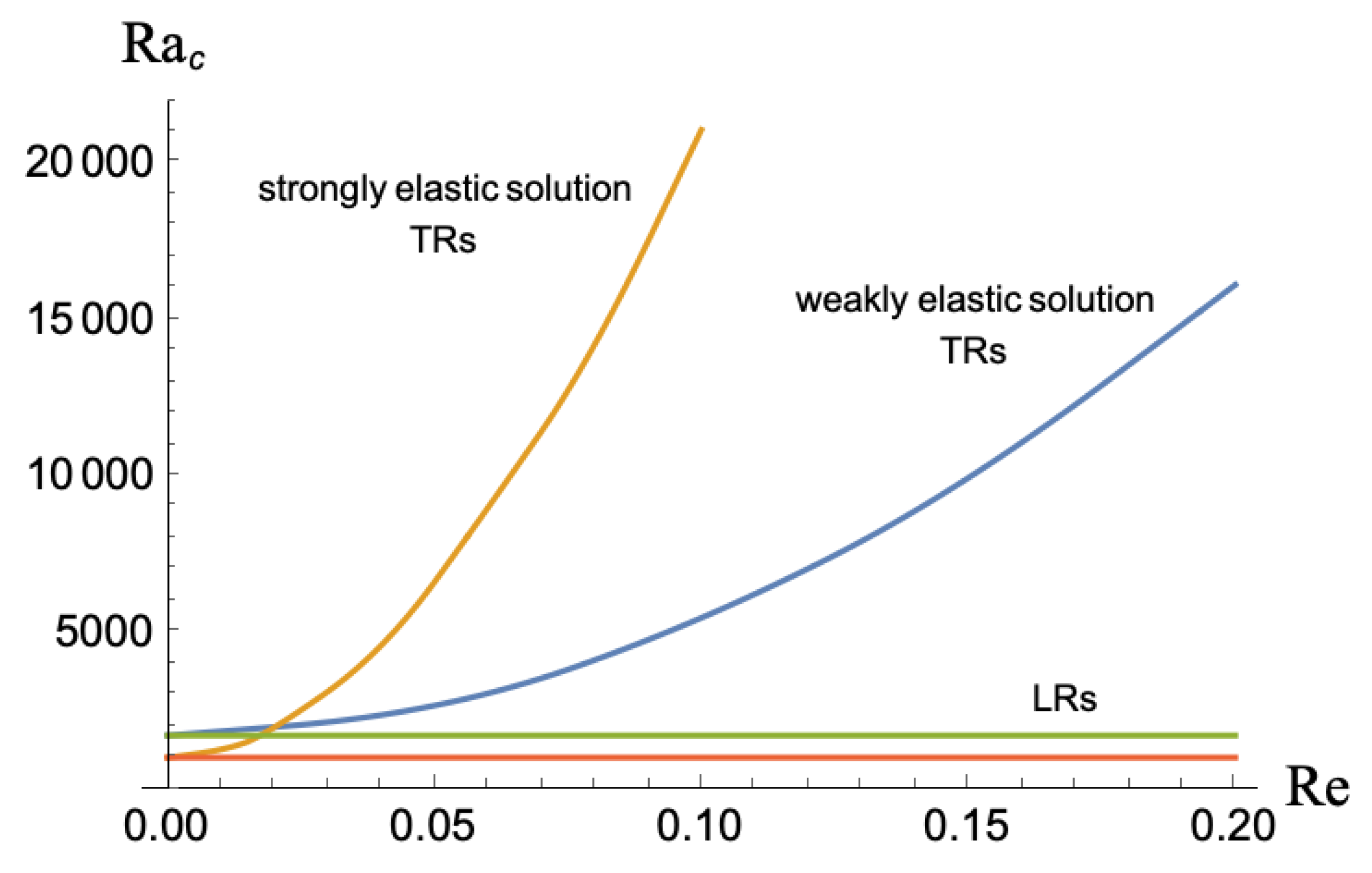
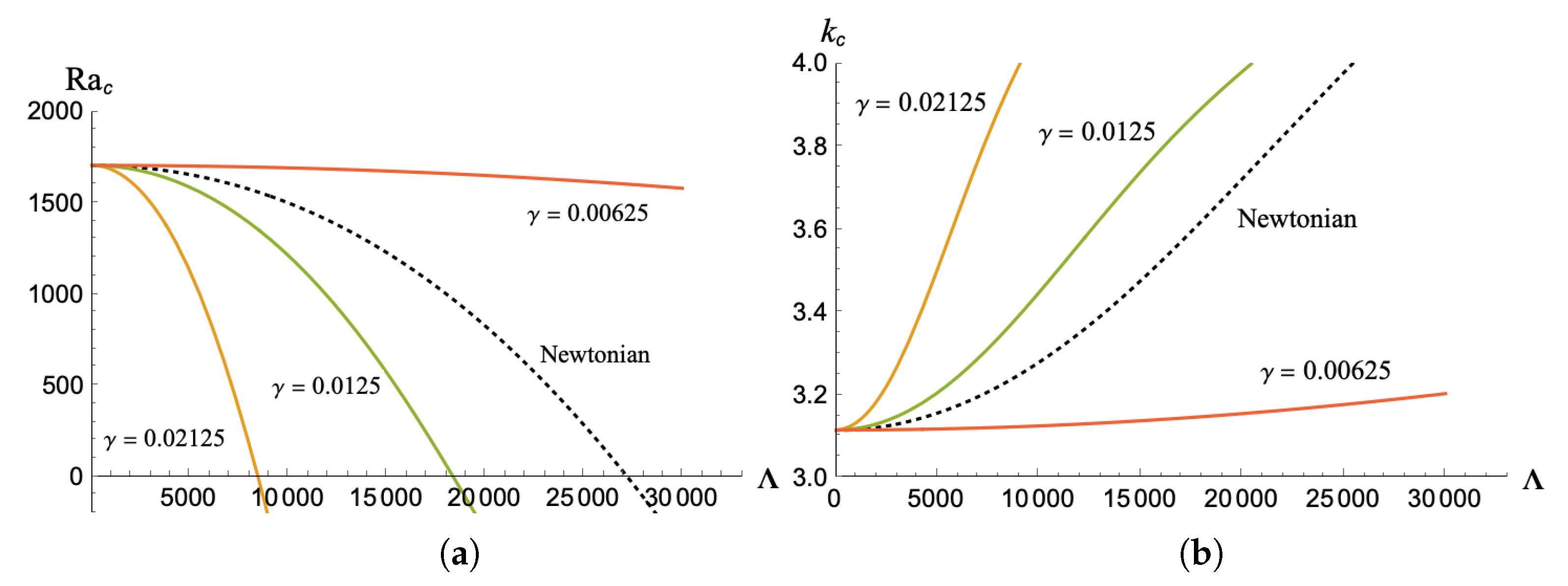
3.1. Effects of Viscous Dissipation and Viscoelasticity on Critical Parameters
3.2. Spatial Distribution of the Eigen-Fields
3.3. Relation to Hydrodynamic Instability
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| specific heat of the fluid at constant volume | |
| g | modulus of the gravitational acceleration |
| Gebhart number, Equation (12) | |
| h | fluid layer depth |
| k | dimensionless wave number, |
| dimensionless wave vector | |
| Peclet number, | |
| Prandtl number, Equation (11) | |
| Rayleigh number, Equation (13) | |
| Reynolds number, | |
| centerline velocity of the Poiseuille flow | |
| Greek symbols | |
| thermal expansion coefficient | |
| viscosity ratio, | |
| viscoelastic parameter, | |
| thermal diffusivity | |
| thermal conductivity | |
| dimensionless relaxation time, Equation (14) | |
| dimensionless retardation time, Equation (15) | |
| dissipation parameter, | |
| total dynamic viscosity, | |
| solvent dynamic viscosity | |
| polymeric solute dynamic viscosity | |
| total kinematic viscosity, | |
| dimensionless oscillation frequency | |
| reference mass density |
References
- Orszag, S.A. Accurate solution of the Orr-Sommerfeld stability equation. J. Fluid Mech. 1971, 50, 689–703. [Google Scholar] [CrossRef] [Green Version]
- Sadanandan, B.; Sureshkumar, R. Viscoelastic effects on the stability of wall-bounded shear flows. Phys. Fluids 2002, 14, 41–48. [Google Scholar] [CrossRef]
- Denn, M.M. Issues in Viscoelastic Fluid Mechanics. Annu. Rev. Fluid Mech. 1990, 22, 13–32. [Google Scholar] [CrossRef]
- Larson, R.G. Instabilities in viscoelastic flows. Rheol. Acta 1992, 31, 213–263. [Google Scholar] [CrossRef]
- Muller, H.W.; Lucke, M.; Kamps, M. Transversal convection patterns in horizontal shear flow. Phys. Rev. A 1992, 45, 3714–3726. [Google Scholar] [CrossRef] [PubMed]
- Carrière, P.; Monkewitz, P.A. Convective versus absolute instability in mixed Rayleigh-Bénard-Poiseuille convection. J. Fluid Mech. 1999, 384, 243–262. [Google Scholar] [CrossRef]
- Büchel, P.; Lücke, M. Influence of through-flow on binary fluid convection. Phys. Rev. E 2000, 61, 3793. [Google Scholar] [CrossRef]
- Hu, J.; Ben Hadid, H.; Henry, D. Linear stability analysis of Poiseuille-Rayleigh-Bénard flows in binary fluids with Soret effect. Phys. Fluids 2007, 19, 034101(1)–034101(17). [Google Scholar] [CrossRef]
- Delache, A.; Ouarzazi, M.N.; Combarnous, M. Spatio-temporal stability analysis of mixed convection flows in porous media heated from below: Comparison with experiments. Int. J. Heat Mass Transf. 2007, 50, 1485–1499. [Google Scholar] [CrossRef]
- Ouarzazi, M.N.; Mejni, F.; Delache, A.; Labrosse, G. Nonlinear global modes in inhomogeneous mixed convection flows in porous media. J. Fluid Mech. 2008, 595, 367–377. [Google Scholar] [CrossRef] [Green Version]
- Jerome, J.J.S.; Chomaz, J.M.; Huerre, P. Transient growth in Rayleigh-Benard-Poiseuille/Couette convection. Phys. Fluids 2012, 24, 044103. [Google Scholar] [CrossRef]
- Nouar, C.; Benaouda-Zouaoui, B.; Desaubry, C. Laminar mixed convection in a horizontal annular duct. case of thermodependent non-Newtonian fluid. Eur. J. Mech. B Fluids 2000, 19, 423–452. [Google Scholar] [CrossRef]
- Métivier, C.; Nouar, C. Linear stability of Rayleigh-Bénard Poiseuille flow for thermodependent viscoplastic fluids. J. Non-Newton. Fluid Mech. 2009, 163, 1–8. [Google Scholar] [CrossRef]
- Hirata, S.; Alves, L.S.d.B.; Delenda, N.; Ouarzazi, M. Convective and absolute instabilities in Rayleigh-Bénard-Poiseuille mixed convection for viscoelastic fluids. J. Fluid Mech. 2015, 765, 167–210. [Google Scholar] [CrossRef]
- Alves, L.S.d.B.; Hirata, S.; Ouarzazi, M. Linear onset of convective instability for Rayleigh-Bénard-Couette flows of viscoelastic fluids. J. Non-Newton. Fluid Mech. 2016, 231, 79–90. [Google Scholar] [CrossRef]
- Roy, K.; Ponalagusamy, R.; Murthy, P. The effects of double-diffusion and viscous dissipation on the oscillatory convection in a viscoelastic fluid saturated porous layer. Phys. Fluids 2020, 32, 094108. [Google Scholar] [CrossRef]
- Straughan, B. Nonlinear Stability for Thermal Convection in a Brinkman Porous Material with Viscous Dissipation. Transp. Porous Media 2020, 134, 303–314. [Google Scholar] [CrossRef]
- Norouzi, M.; Dorrani, S.; Shokri, H.; Anwar Beg, O. Effects of viscous dissipation on miscible thermo-viscous fingering instability in porous media. Int. J. Heat Mass Transf. 2019, 129, 212–223. [Google Scholar] [CrossRef]
- Miklavcic, M. Bistable fully developed mixed convection flow with viscous dissipation in a vertical channel. R. Soc. Open Sci. 2018, 5, 171880. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roy, K.; Murthy, P. Linear Stability of the Double-Diffusive Convection in a Horizontal Porous Layer with Open Top: Soret and Viscous Dissipation Effects. Transp. Porous Media 2018, 122, 693–712. [Google Scholar] [CrossRef]
- Celli, M.; Kuznetsov, A. A new mechanism for buoyancy driven convection in pulsating viscous flows: A theoretical study. Int. J. Heat Mass Transf. 2018, 118, 340–348. [Google Scholar] [CrossRef]
- Celli, M.; Barletta, A. Onset of convection in a non-Newtonian viscous flow through a horizontal porous channel. Int. J. Heat Mass Transf. 2018, 117, 1322–1330. [Google Scholar] [CrossRef]
- Barletta, A. On the thermal instability induced by viscous dissipation. Int. J. Therm. Sci. 2015, 88, 238–247. [Google Scholar] [CrossRef]
- Barletta, A.; Nield, D.A. Convection-dissipation instability in the horizontal plane Couette flow of a highly viscous fluid. J. Fluid Mech. 2010, 662, 475–492. [Google Scholar] [CrossRef]
- Barletta, A.; Celli, M.; Nield, D.A. On the onset of dissipation thermal instability for the Poiseuille flow of a highly viscous fluid in a horizontal channel. J. Fluid Mech. 2011, 681, 499–514. [Google Scholar] [CrossRef]
- Requilé, Y.; Hirata, S.C.; Ouarzazi, M.N.; Barletta, A. Weakly nonlinear analysis of viscous dissipation thermal instability in plane Poiseuille and plane Couette flows. J. Fluid Mech. 2020, 886, A26. [Google Scholar] [CrossRef]
- Requilé, Y.; Hirata, S.C.; Ouarzazi, M.N. Viscous dissipation effects on the linear stability of Rayleigh-Bénard-Poiseuille/Couette convection. Int. J. Heat Mass Transf. 2020, 146, 118834. [Google Scholar] [CrossRef]
- Bird, R.B.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids. Vol. 1: Fluid Mechanics; John Wiley and Sons Inc.: New York, NY, USA, 1987. [Google Scholar]
- Alves, L.D.B.; Hirata, S.C.; Schuabb, M.; Barletta, A. Identifying linear absolute instabilities from differential eigenvalue problems using sensitivity analysis. J. Fluid Mech. 2019, 870, 941–969. [Google Scholar] [CrossRef]
- Li, Z.; Khayat, R. Finite-amplitude Rayleigh-Bénard convection and pattern selection for viscoelastic fluids. J. Fluid Mech. 2005, 529, 221–251. [Google Scholar] [CrossRef]
- Sureshkumar, R.; Beris, A. Linear stability analysis of viscoelastic Poiseuille flow using an Arnoldi-based orthogonalization algorithm. J. Non-Newton. Fluid Mech. 1994, 56, 151–182. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hirata, S.C.; Ouarzazi, M.N. Onset of Thermal Instabilities in the Plane Poiseuille Flow of Weakly Elastic Fluids: Viscous Dissipation Effects. Fluids 2021, 6, 432. https://doi.org/10.3390/fluids6120432
Hirata SC, Ouarzazi MN. Onset of Thermal Instabilities in the Plane Poiseuille Flow of Weakly Elastic Fluids: Viscous Dissipation Effects. Fluids. 2021; 6(12):432. https://doi.org/10.3390/fluids6120432
Chicago/Turabian StyleHirata, Silvia C., and Mohamed Najib Ouarzazi. 2021. "Onset of Thermal Instabilities in the Plane Poiseuille Flow of Weakly Elastic Fluids: Viscous Dissipation Effects" Fluids 6, no. 12: 432. https://doi.org/10.3390/fluids6120432
APA StyleHirata, S. C., & Ouarzazi, M. N. (2021). Onset of Thermal Instabilities in the Plane Poiseuille Flow of Weakly Elastic Fluids: Viscous Dissipation Effects. Fluids, 6(12), 432. https://doi.org/10.3390/fluids6120432






