Stability of a Buoyant Oldroyd-B Flow Saturating a Vertical Porous Layer with Open Boundaries
Abstract
:1. Introduction
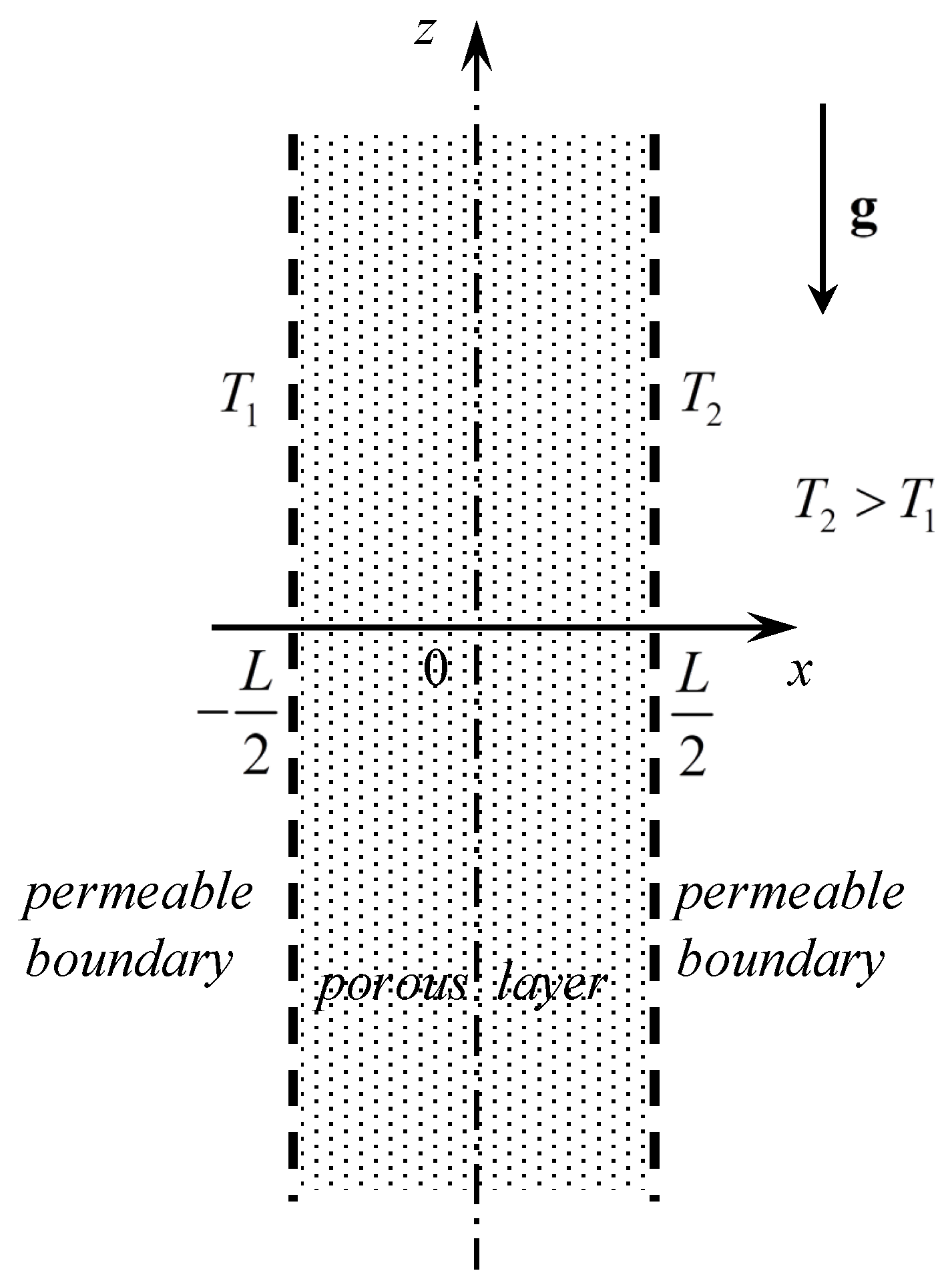
2. Mathematical Model
Basic State
3. Linear Stability Analysis
4. Numerical Solution and Discussion of the Results
4.1. Limiting Case of a Newtonian Fluid
4.2. Results for Viscoelastic Fluids
5. Conclusions
- The dimensionless governing parameters identifying the viscoelastic behaviour are the relaxation parameter, , and the ratio between the retardation time and the relaxation time, . The physically significant domain is one where . When , the Newtonian fluid behaviour is recovered: the critical value of the modified Darcy–Rayleigh number is 197.081 and the corresponding critical value of the wavenumber is 1.05950;
- The neutral stability threshold to the convective instability obtained for the Newtonian case always exists, whatever is the choice of and . However, in most cases, the Newtonian branch of neutral stability is not the lowest one. In these cases, the neutral stability condition is characterised by travelling modes, i.e., modes with a non-zero angular frequency. Thus, the effect of viscoelasticity is generally destabilising with respect to the Newtonian fluid case;
- There exist input values of , with , such that the critical conditions for the onset of the convective instability coincide with those for a Newtonian fluid or, equivalently, the Newtonian branch of neutral stability is the lowest one.
Author Contributions
Funding
Conflicts of Interest
References
- Gill, A.E. A proof that convection in a porous vertical slab is stable. J. Fluid Mech. 1969, 35, 545–547. [Google Scholar] [CrossRef]
- Rees, D.A.S. The stability of Prandtl–Darcy convection in a vertical porous layer. Int. J. Heat Mass Transf. 1988, 31, 1529–1534. [Google Scholar] [CrossRef]
- Straughan, B. A nonlinear analysis of convection in a porous vertical slab. Geophys. Astrophys. Fluid Dyn. 1988, 42, 269–275. [Google Scholar] [CrossRef]
- Lewis, S.; Bassom, A.P.; Rees, D.A.S. The stability of vertical thermal boundary–layer flow in a porous medium. Eur. J. Mech. B Fluids 1995, 14, 395–407. [Google Scholar]
- Rees, D.A.S.; Lage, J.L. The effect of thermal stratification on natural convection in a vertical porous insulation layer. Int. J. Heat Mass Transf. 1996, 40, 111–121. [Google Scholar] [CrossRef]
- Rees, D.A.S. The effect of local thermal nonequilibrium on the stability of convection in a vertical porous channel. Transp. Porous Media 2011, 87, 459–464. [Google Scholar] [CrossRef]
- Scott, N.L.; Straughan, B. A Nonlinear stability analysis of convection in a porous vertical channel including local thermal nonequilibrium. J. Math. Fluid Mech. 2013, 15, 171–178. [Google Scholar] [CrossRef]
- Barletta, A. A proof that convection in a porous vertical slab may be unstable. J. Fluid Mech. 2015, 770, 273–288. [Google Scholar] [CrossRef]
- Celli, M.; Barletta, A.; Rees, D.A.S. Local thermal non–equilibrium analysis of the instability in a vertical porous slab with permeable sidewalls. Transp. Porous Media 2017, 119, 539–553. [Google Scholar] [CrossRef]
- Barletta, A.; Celli, M.; Ouarzazi, M.N. Unstable buoyant flow in a vertical porous layer with convective boundary conditions. Int. J. Therm. Sci. 2017, 120, 427–436. [Google Scholar] [CrossRef]
- Barletta, A.; Rees, D.A.S. On the onset of convection in a highly permeable vertical porous layer with open boundaries. Phys. Fluids 2019, 31, 074106. [Google Scholar] [CrossRef]
- Barletta, A.; Celli, M. Anisotropy and the onset of the thermoconvective instability in a vertical porous layer. ASME J. Heat Transf. 2021, 143, 102601. [Google Scholar] [CrossRef]
- Barletta, A.; de Alves, L.S.B. On Gill’s stability problem for non–Newtonian Darcy’s flow. Int. J. Heat Mass Transf. 2014, 79, 759–768. [Google Scholar] [CrossRef]
- Shankar, B.M.; Shivakumara, I.S. On the stability of natural convection in a porous vertical slab saturated with an Oldroyd–B fluid. Theor. Comput. Fluid Dyn. 2017, 31, 221–231. [Google Scholar] [CrossRef]
- Shankar, B.M.; Shivakumara, I.S. Effect of local thermal nonequilibrium on the stability of natural convection in an Oldroyd–B fluid saturated vertical porous layer. ASME J. Heat Transf. 2017, 139, 041001. [Google Scholar] [CrossRef]
- Khuzhayorov, B.; Auriault, J.L.; Royer, P. Derivation of macroscopic filtration law for transient linear viscoelastic fluid flow in porous media. Int. J. Eng. Sci. 2000, 38, 487–504. [Google Scholar] [CrossRef] [Green Version]
- Hirata, S.C.; Ouarzazi, M.N. Three–dimensional absolute and convective instabilities in mixed convection of a viscoelastic fluid through a porous medium. Phys. Lett. A 2010, 374, 2661–2666. [Google Scholar] [CrossRef]
- de Alves, L.S.B.; Barletta, A.; Hirata, S.; Ouarzazi, M.N. Effects of viscous dissipation on the convective instability of viscoelastic mixed convection flows in porous media. Int. J. Heat Mass Transf. 2014, 70, 586–598. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 5th ed.; Springer: New York, NY, USA, 2017. [Google Scholar]
- Boger, D.V. Viscoelastic flows through contractions. Annu. Rev. Fluid Mech. 1987, 19, 157–182. [Google Scholar] [CrossRef]
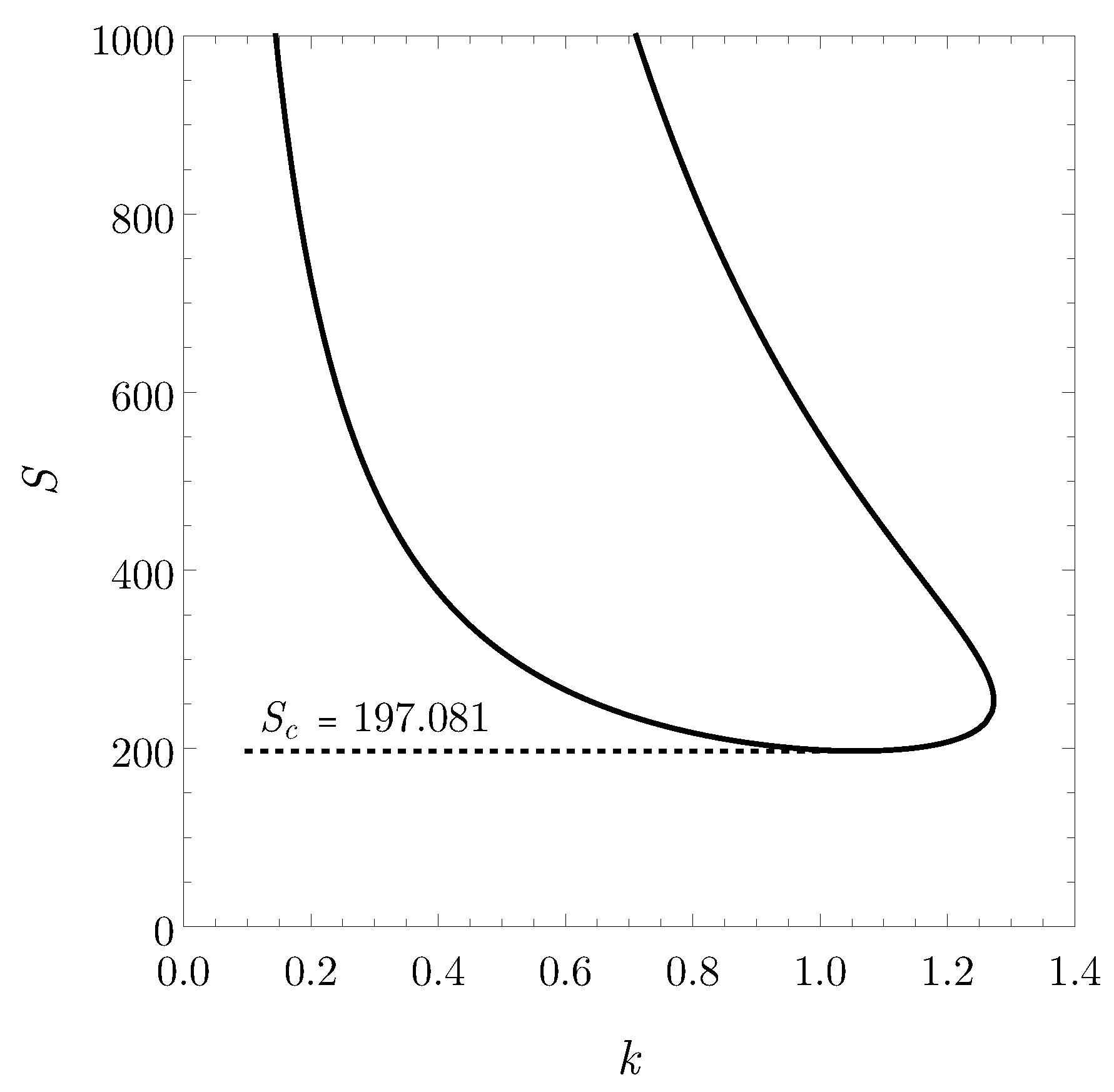

| 0.015 | 6.23219 | 125.630 | 301.680 | 4.35669 | 149.556 | 166.030 |
| 0.025 | 5.48899 | 92.0028 | 186.630 | 3.77358 | 114.751 | 95.0600 |
| 0.05 | 4.56254 | 59.4450 | 96.4569 | 3.24560 | 78.3898 | 47.4367 |
| 0.075 | 4.25166 | 45.9027 | 71.5633 | 3.11482 | 63.1441 | 35.6140 |
| 0.1 | 4.05828 | 38.3924 | 59.2173 | 3.03338 | 54.7411 | 29.7598 |
| 0.25 | 3.50236 | 23.0465 | 34.1704 | 2.77928 | 37.6406 | 17.7300 |
| 0.5 | 3.16277 | 17.0453 | 23.0225 | 2.61721 | 31.0081 | 12.2442 |
| 0.015 | 1.05950 | 197.081 | 0 | 1.05950 | 197.081 | 0 |
| 0.025 | 2.43604 | 183.662 | 53.9920 | 1.05950 | 197.081 | 0 |
| 0.05 | 2.21927 | 125.192 | 25.2047 | 1.05950 | 197.081 | 0 |
| 0.075 | 2.20008 | 102.173 | 19.2658 | 1.50760 | 183.721 | 8.72516 |
| 0.1 | 2.18714 | 90.0757 | 16.3564 | 1.53835 | 159.824 | 8.11327 |
| 0.25 | 2.12657 | 66.8434 | 10.1776 | 1.58390 | 118.328 | 5.94810 |
| 0.5 | 2.08008 | 58.4180 | 7.19023 | 1.59069 | 104.712 | 4.42120 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lazzari, S.; Celli, M.; Barletta, A. Stability of a Buoyant Oldroyd-B Flow Saturating a Vertical Porous Layer with Open Boundaries. Fluids 2021, 6, 375. https://doi.org/10.3390/fluids6110375
Lazzari S, Celli M, Barletta A. Stability of a Buoyant Oldroyd-B Flow Saturating a Vertical Porous Layer with Open Boundaries. Fluids. 2021; 6(11):375. https://doi.org/10.3390/fluids6110375
Chicago/Turabian StyleLazzari, Stefano, Michele Celli, and Antonio Barletta. 2021. "Stability of a Buoyant Oldroyd-B Flow Saturating a Vertical Porous Layer with Open Boundaries" Fluids 6, no. 11: 375. https://doi.org/10.3390/fluids6110375
APA StyleLazzari, S., Celli, M., & Barletta, A. (2021). Stability of a Buoyant Oldroyd-B Flow Saturating a Vertical Porous Layer with Open Boundaries. Fluids, 6(11), 375. https://doi.org/10.3390/fluids6110375








