The Influence of Spectral Filtering Bandwidth and Laser Gain on the Bound-State Pulse Formation Mechanism and Evolutionary Dynamics in the All-Fiber Mamyshev Oscillator
Abstract
1. Introduction
2. Theoretical Model
3. Influence of the Key Parameters on Multi-Pulse Dynamics
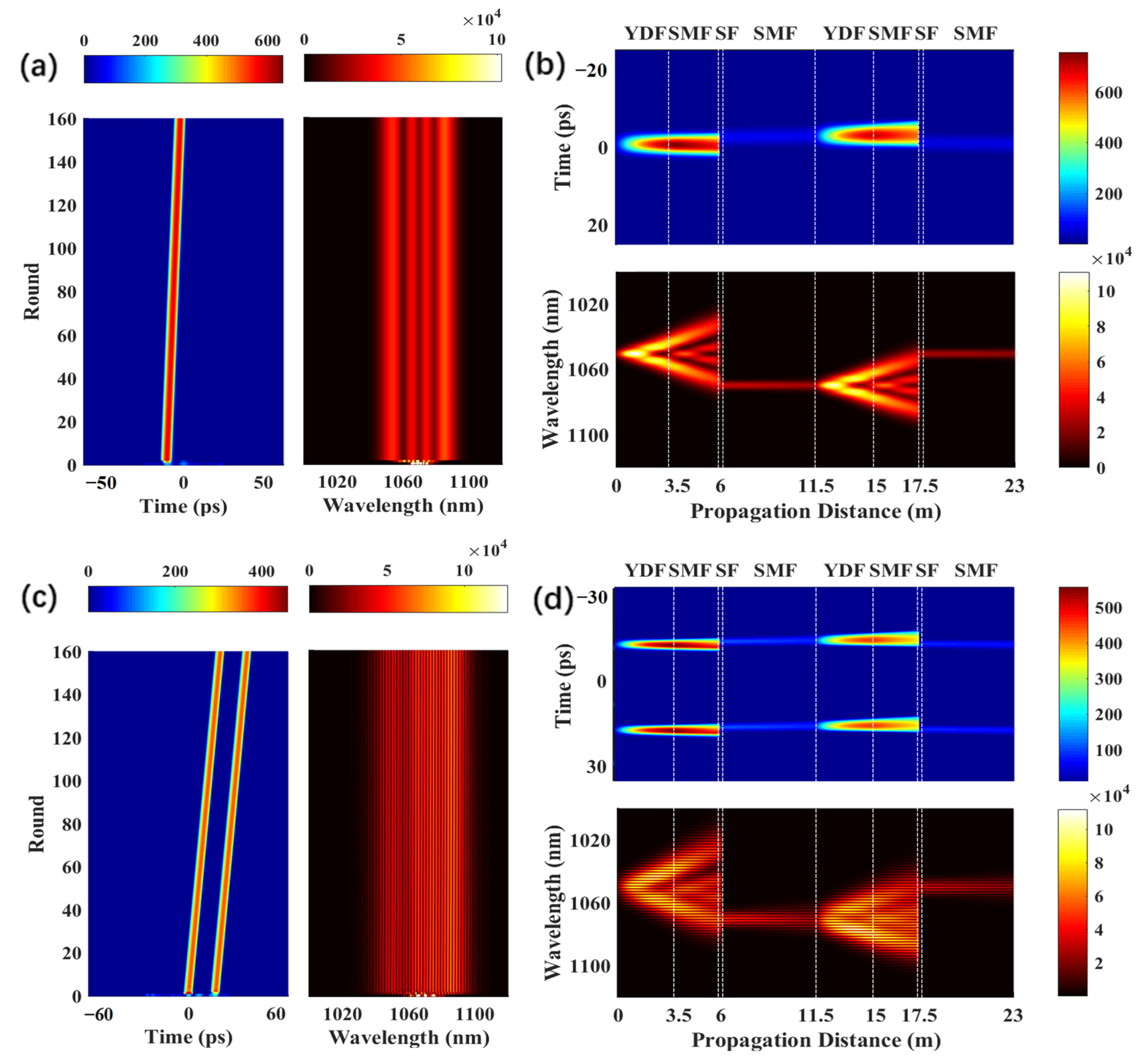
3.1. Influence of Filtering Bandwidth
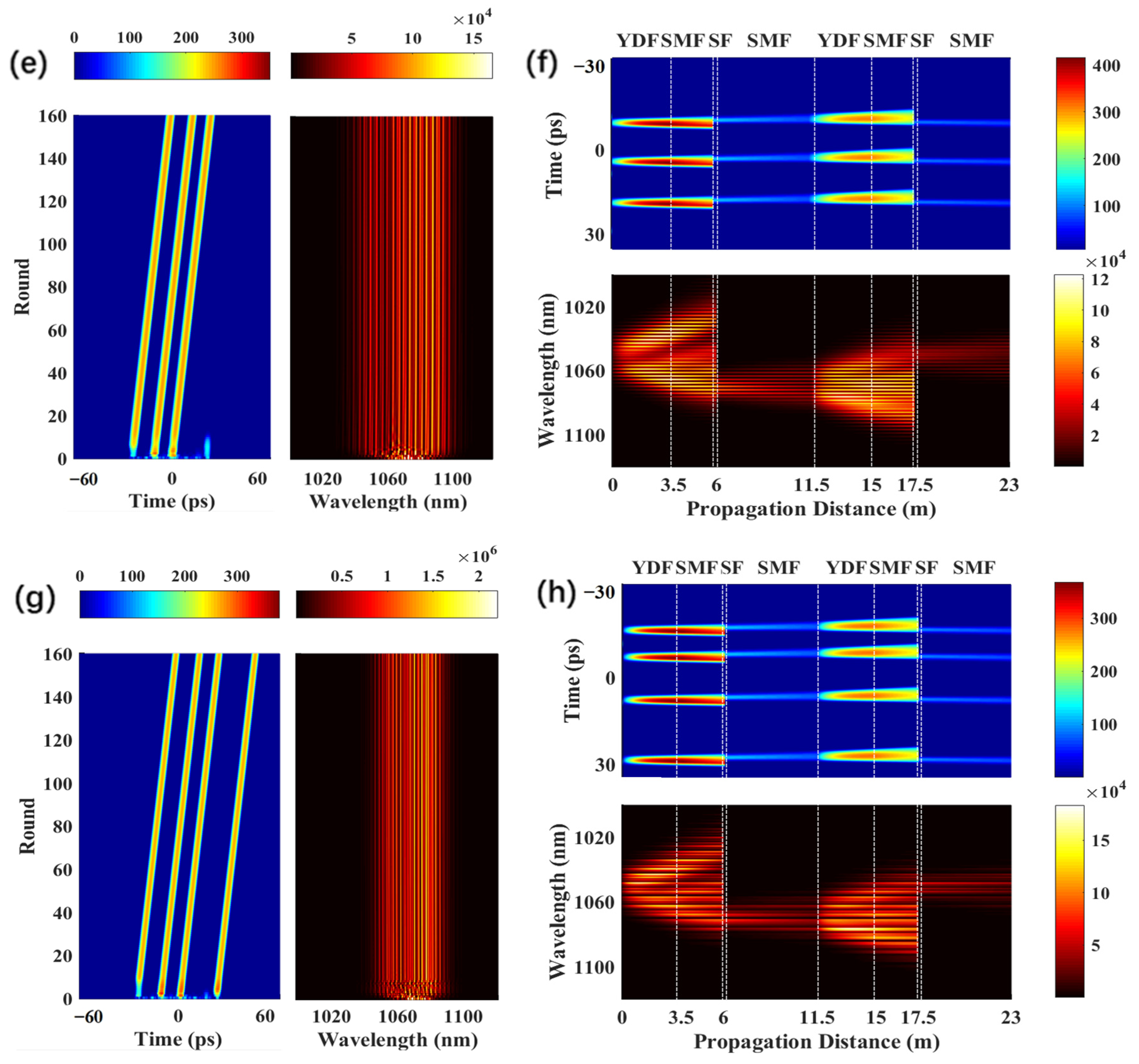
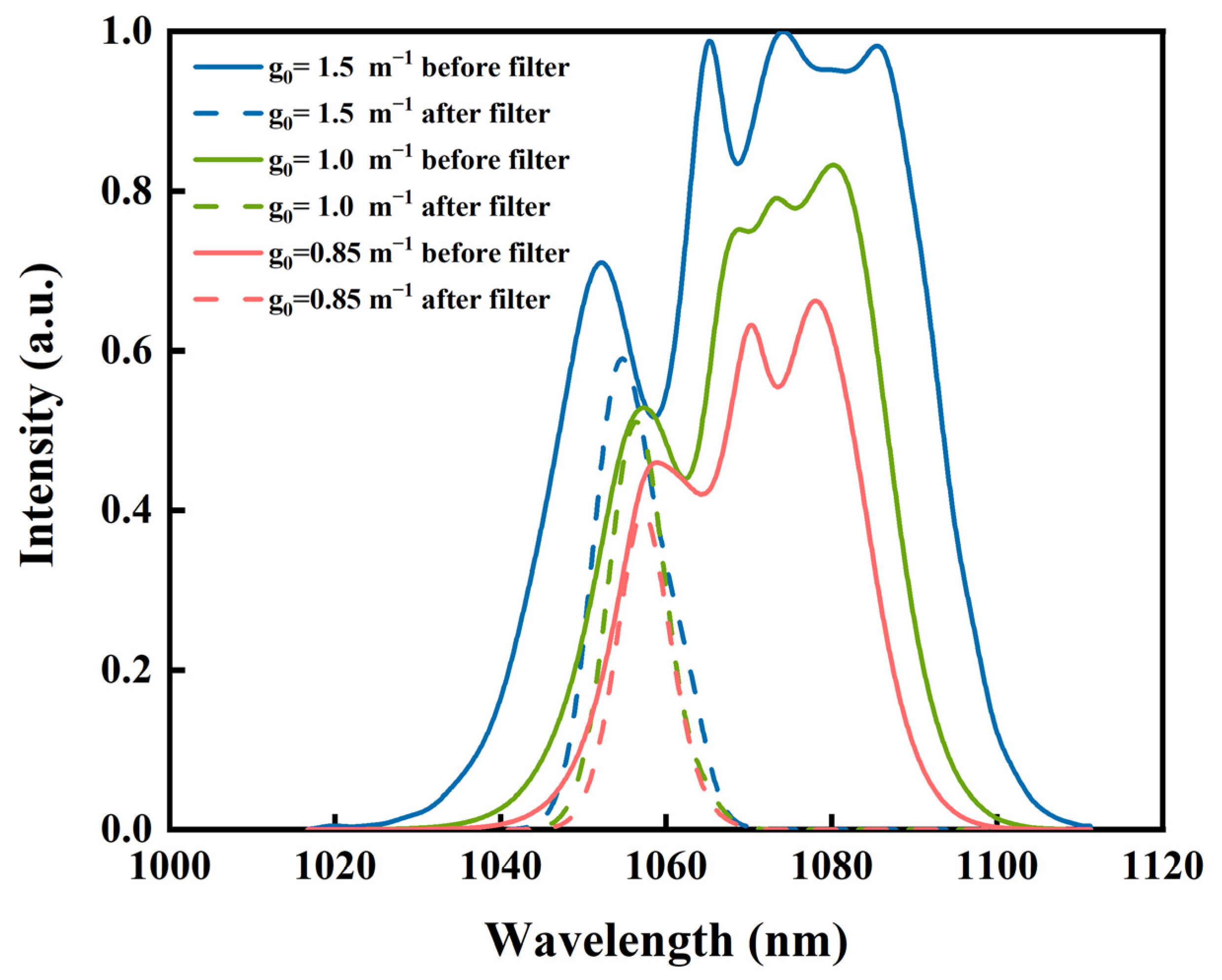
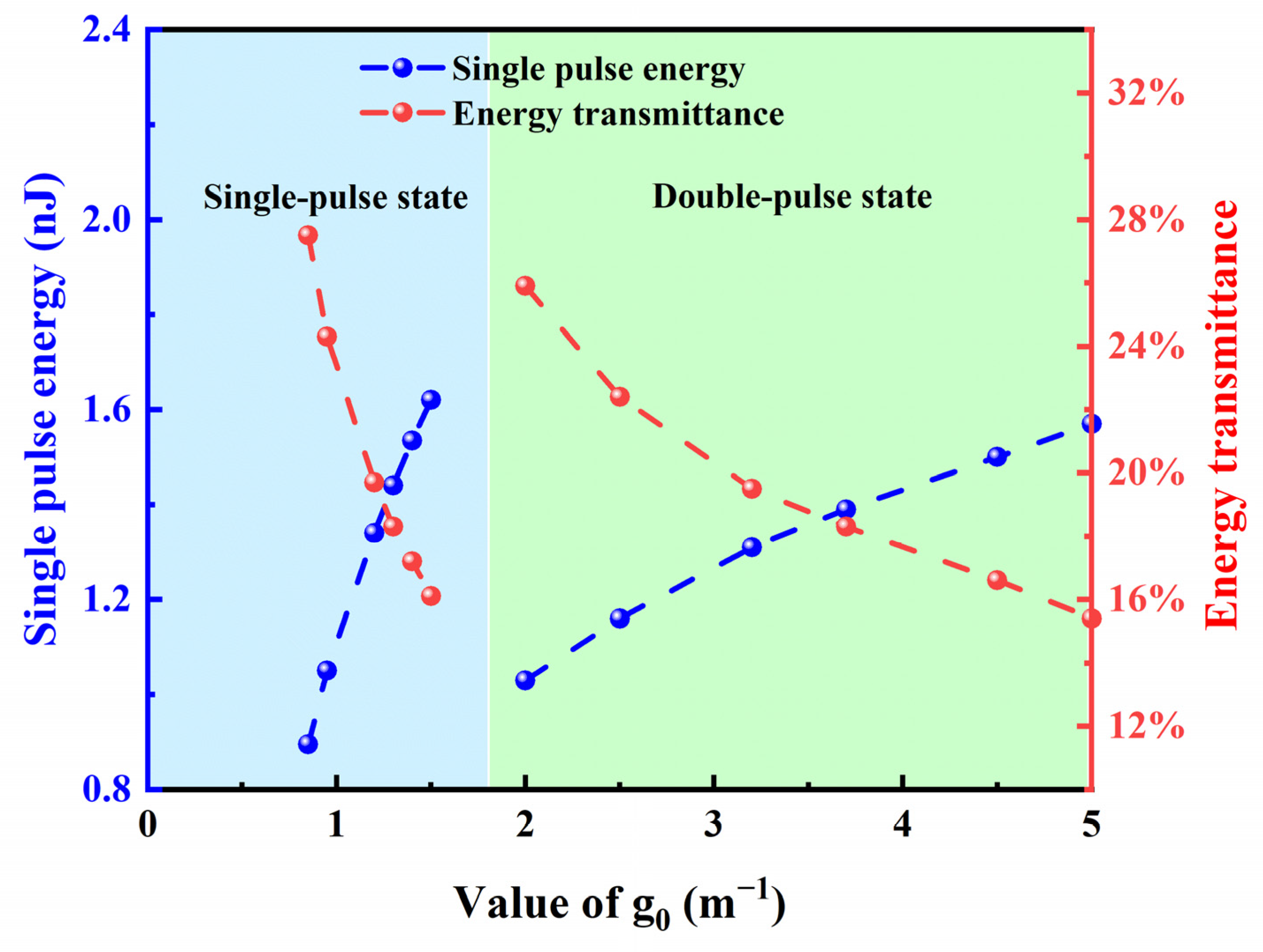
3.2. Influence of Small-Signal Gain
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, Y.; Lin, W.; Cheng, H.; Wang, W.; Yang, Z. Composite filtering effect in a SESAM mode-locked fiber laser with a 3.2-GHz fundamental repetition rate: Switchable states from single soliton to pulse bunch: Erratum. Opt. Express 2018, 26, 10842–10857. [Google Scholar] [CrossRef]
- Li, H.; Wang, Z.; Li, C.; Zhang, J.; Xu, S. Mode-locked Tm fiber laser using SMF-SIMF-GIMF-SMF fiber structure as a saturable absorber. Opt. Express 2017, 25, 26546–26553. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Z.; Yang, S.; He, C.; Lin, X. Vector pure-quartic soliton molecule fiber laser. Chaos Solitons Fractals 2023, 175, 113978. [Google Scholar] [CrossRef]
- Zhang, Y.; Qi, Y.; Sheng, Q.; Bai, Z.; Wang, Y.; Shi, W.; Lu, Z. Tunable vortex beams generation in visible band via Pr3+: YLF laser with a spot defect. Appl. Phys. Lett. 2023, 123, 251117. [Google Scholar] [CrossRef]
- Lin, D.; Feng, Y.; Ren, Z.; Richardson, D.J. The generation of femtosecond optical vortex beams with megawatt powers directly from a fiber based Mamyshev oscillator. Nanophotonics 2021, 11, 847–854. [Google Scholar] [CrossRef]
- Zheng, J.; Yang, S.; Zhu, Z.; Lau, K.; Li, L. 72-fs Er-doped Mamyshev oscillator. J. Light. Technol. 2021, 40, 2123–2127. [Google Scholar] [CrossRef]
- Olivier, M.; Boulanger, V.; Savary, F.G.; Sidorenko, P.; Wise, F.W. Femtosecond fiber Mamyshev oscillator at 1550 nm. Opt. Lett. 2019, 44, 851–854. [Google Scholar] [CrossRef] [PubMed]
- Piechal, B.; Szczepanek, J.; Kardas, T.M.; Stepanenko, Y. Mamyshev Oscillator with a Widely Tunable Repetition Rate. J. Light. Technol. 2020, 39, 574–581. [Google Scholar] [CrossRef]
- Rochette, M.; Chen, L.R.; Sun, K.; Hernandez-Cordero, J. Multiwavelength and Tunable Self-Pulsating Fiber Cavity Based on Regenerative SPM Spectral Broadening and Filtering. IEEE Photonics Technol. Lett. 2008, 20, 1497–1499. [Google Scholar] [CrossRef]
- Regelskis, K.; Želudevičius, J.; Viskontas, K.; Račiukaitis, G. Ytterbium-doped fiber ultrashort pulse generator based on self-phase modulation and alternating spectral filtering. Opt. Lett. 2015, 40, 5255–5258. [Google Scholar] [CrossRef]
- Perego, M.; Tarasov, N.; Churkin, V.; Turitsyn, K.; Staliunas, K. Pattern Generation by Dissipative Parametric Instability. Phys. Rev. Lett. 2016, 116, 028701. [Google Scholar] [CrossRef] [PubMed]
- Tarasov, N.; Perego, A.M.; Churkin, D.V.; Staliunas, K.; Turitsyn, S.K. Mode-locking via dissipative Faraday instability. Nat. Commun. 2016, 7, 12441. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Ziegler, Z.M.; Wright, L.G.; Wise, F.W. Megawatt peak power from a Mamyshev oscillator. Optica 2017, 4, 649–654. [Google Scholar] [CrossRef] [PubMed]
- Wu, L.; Liao, R.; Zhao, J.; Cui, J.; Hu, M. Femtosecond Mamyshev oscillator with 10-MW-level peak power. Optica 2019, 6, 194–197. [Google Scholar]
- Nie, M.; Wang, J.; Huang, S. Solid-state Mamyshev oscillator. Photonics Res. 2019, 7, 1175–1181. [Google Scholar] [CrossRef]
- Wang, T.; Ren, B.; Li, C.; Wu, J. Over 80 nJ Sub-100 fs All-Fiber Mamyshev Oscillator. IEEE J. Sel. Top. Quantum Electron. 2021, 27, 6. [Google Scholar] [CrossRef]
- Haig, H.; Sidorenko, P.; Thorne, R.; Wise, F.W. Megawatt pulses from an all-fiber and self-starting femtosecond oscillator. Opt. Lett. 2022, 47, 762–765. [Google Scholar] [CrossRef]
- Repgen, P.; Schuhbauer, B.; Hinkelmann, M.; Wandt, D. Mode-locked pulses from a thulium-doped fiber Mamyshev oscillator. Opt. Express 2020, 28, 13837–13844. [Google Scholar] [CrossRef]
- Repgen, P.; Wandt, D.; Morgner, U.; Neumann, J.; Kracht, D. Sub-50 fs, J-level pulses from a Mamyshev oscillator–amplifier system. Opt. Lett. 2019, 44, 5973–5976. [Google Scholar] [CrossRef]
- Zheng, J.; Yang, S.; Zhu, Z.; Lau, K.; Li, L. Low mode-locking threshold and sub-90 fs Er-doped Mamyshev oscillator. Opt. Commun. 2022, 508, 127711.1–127711.4. [Google Scholar] [CrossRef]
- Cao, B.; Zhao, K.; Gao, C.; Xiao, X.; Bao, C.; Yang, C. Observation of pulsating dissipative solitons in a Mamyshev oscillator. Phys. Rev. A 2021, 106, 023519. [Google Scholar] [CrossRef]
- Zhang, Y.; Dai, K.; Zhang, B.; Chen, D.; Guan, Z. Investigations on pulse dynamics and offset spectral filtering in Er-doped Mamyshev fiber oscillator. Opt. Commun. 2023, 529, 129103. [Google Scholar] [CrossRef]
- Perego, A.M. High-repetition-rate, multi-pulse all-normal-dispersion fiber laser. Opt. Lett. 2017, 42, 3574–3577. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Yao, S.; Grelu, P.; Xiao, X.; Yang, C. Pattern formation in 2-μm Tm Mamyshev oscillators associated with the dissipative Faraday instability. Photonics Res. 2019, 7, 1287–1295. [Google Scholar] [CrossRef]
- Luo, Z.; Liu, M.; Wei, Z.; Luo, A.; Xu, S. Multipulse dynamics in a Mamyshev oscillator. Opt. Lett. 2020, 45, 2620–2623. [Google Scholar]
- Yan, D.; Li, X.; Zhang, S.; Liu, J. Pulse dynamic patterns in a self-starting Mamyshev oscillator. Opt. Express 2021, 29, 9805–9815. [Google Scholar] [CrossRef] [PubMed]
- Mao, D.; Wang, H.; Zhang, H.; Zeng, C.; Du, Y.; He, Z.; Sun, Z.; Zhao, J. Synchronized multi-wavelength soliton fiber laser via intracavity group delay modulation. Nat. Commun. 2021, 12, 6712. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.; Zhu, Z.; Qi, Y.; Jin, L.; Li, L.; Lin, X. Internal motion within pulsating pure-quartic soliton molecules in a fiber laser. Chaos Solitons Fractals 2023, 172, 113544. [Google Scholar] [CrossRef]
- Agrawal, G.P. Applications of Nonlinear Fiber Optics; Academic Press: New York, NY, USA, 2007. [Google Scholar]
- Zhang, Z.; Luo, M.; Chen, J.; Chen, L.; Liu, M.; Luo, A.; Xu, W.; Luo, Z. Pulsating dynamics in a pure-quartic soliton fiber laser. Opt. Lett. 2022, 47, 1750–1753. [Google Scholar] [CrossRef]
- Pitois, S.; Finot, C.; Provost, L.; Richardson, D.J. Generation of localized pulses from incoherent wave in optical fiber lines made of concatenated Mamyshev regenerators. J. Opt. Soc. Am. B 2010, 25, 1537–1547. [Google Scholar] [CrossRef]
- Du, Y.; Shu, X. Pulse dynamics in all-normal dispersion ultrafast fiber lasers. J. Opt. Soc. Am. B 2017, 34, 553–558. [Google Scholar] [CrossRef]
- Lin, D.; Xu, D.; He, J.; Feng, Y. The Generation of 1.2 μJ Pulses from a Mamyshev Oscillator Based on a High Concentration, Large-Mode-Area Yb-Doped Fiber. J. Light. Technol. 2022, 40, 7175–7179. [Google Scholar] [CrossRef]
- Wang, J.; Qi, Y.; Bai, Z.; Ding, J.; Yan, B.; Wang, Y.; Lu, Z. Recent advance of high-energy ultrafast mode-locked oscillators based on Mamyshev mechanism with different starting modes. Opt. Eng. 2022, 61, 120901. [Google Scholar] [CrossRef]
- Zheng, J.; Yang, S.; Zhu, Z. Recent research progress of Mamyshev oscillator for high energy and ultrashort pulse generation. Opt. Fiber Technol. 2021, 67, 102691.1–102691.11. [Google Scholar] [CrossRef]




| Component | Saturation Energy (nJ) | |||
|---|---|---|---|---|
| SMF | 3.2 | 25 | −1.3 | 0 |
| YDF | 3.2 | 28 | −1.8 | 1.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, Y.; Zhang, T.; Bai, Z.; Ding, J.; Yan, B.; Wang, Y.; Lu, Z.; Yan, D. The Influence of Spectral Filtering Bandwidth and Laser Gain on the Bound-State Pulse Formation Mechanism and Evolutionary Dynamics in the All-Fiber Mamyshev Oscillator. Photonics 2024, 11, 139. https://doi.org/10.3390/photonics11020139
Qi Y, Zhang T, Bai Z, Ding J, Yan B, Wang Y, Lu Z, Yan D. The Influence of Spectral Filtering Bandwidth and Laser Gain on the Bound-State Pulse Formation Mechanism and Evolutionary Dynamics in the All-Fiber Mamyshev Oscillator. Photonics. 2024; 11(2):139. https://doi.org/10.3390/photonics11020139
Chicago/Turabian StyleQi, Yaoyao, Tianchen Zhang, Zhenxu Bai, Jie Ding, Bingzheng Yan, Yulei Wang, Zhiwei Lu, and Dapeng Yan. 2024. "The Influence of Spectral Filtering Bandwidth and Laser Gain on the Bound-State Pulse Formation Mechanism and Evolutionary Dynamics in the All-Fiber Mamyshev Oscillator" Photonics 11, no. 2: 139. https://doi.org/10.3390/photonics11020139
APA StyleQi, Y., Zhang, T., Bai, Z., Ding, J., Yan, B., Wang, Y., Lu, Z., & Yan, D. (2024). The Influence of Spectral Filtering Bandwidth and Laser Gain on the Bound-State Pulse Formation Mechanism and Evolutionary Dynamics in the All-Fiber Mamyshev Oscillator. Photonics, 11(2), 139. https://doi.org/10.3390/photonics11020139







