Numerical Simulation and Experimental Investigation of ps Pulsed Laser Modification inside 4H-SiC Material
Abstract
1. Introduction
2. Numerical Model Simulation
- (1)
- The incident laser’s light field distribution follows a Gaussian distribution.
- (2)
- The property parameters of 4H-SiC in the model remain constant with temperature variations.
- (3)
- Considering a pulse width of 10 ps for the excitation laser and an electron-to-ion transformation time scale of 10−12 to 10−10 s [25], the photoelectron interaction is negligible. In contrast, lattice relaxation is considered so that the heat conduction model is exclusively utilized.
- (4)
- The laser acts as an electromagnetic wave; its electric field is entirely absorbed in the focal zone, ignoring the absorption and ablation on the surface of 4H-SiC.
- (5)
- SiC material modification is usually accompanied by Si vapor generation [19]. Therefore, the modified threshold is set as 1687.15 K, which is the boiling point of silicon.
3. Materials and Methods
4. Results and Discussion
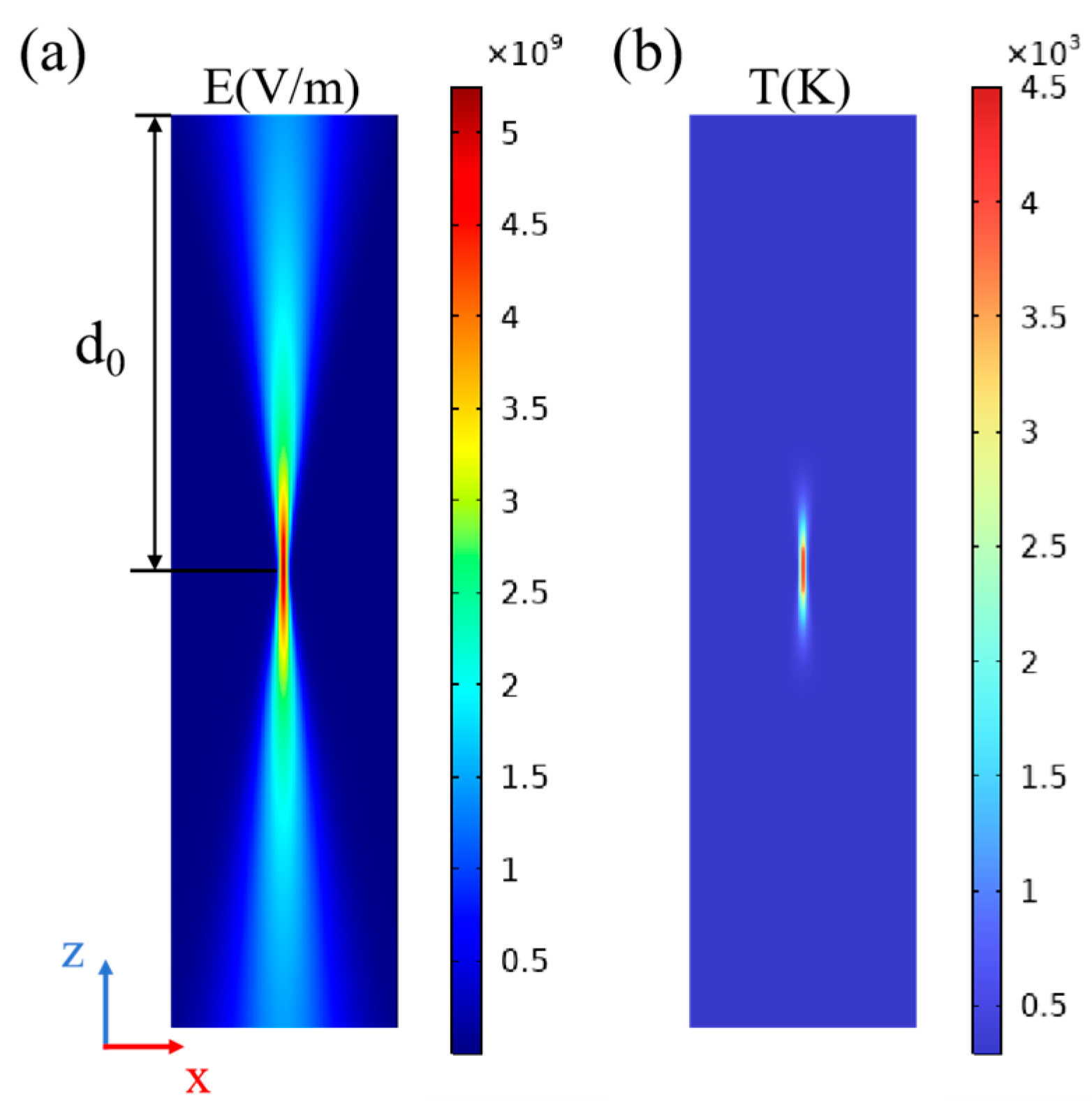
4.1. Electrical-Induced Temperature Field
4.2. Experimental Analysis
5. Conclusions
- (1)
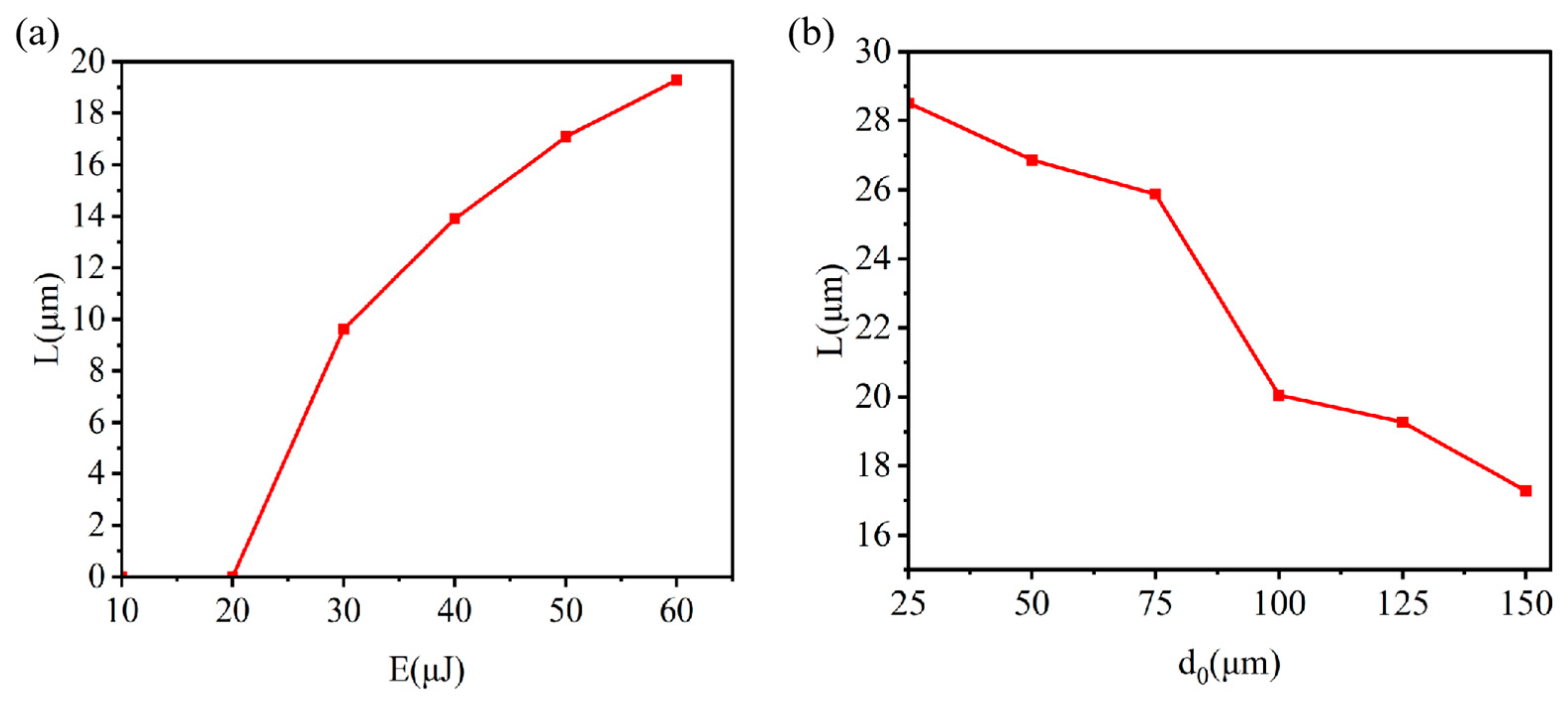
- The simulation method employed here provides a reasonable calculation process for the electrical-induced temperature field at 10 ps inside the 4H-SiC. It simulated the change of electrical-induced temperature field with the increasing of laser pulse energy and focusing depth. The simulation results indicate that the modification depth increases with the rise in laser pulse energy. However, a counter trend is observed as the increase of focusing depth. This means that to form a modified layer deep in the material, more energy is needed.
- (2)
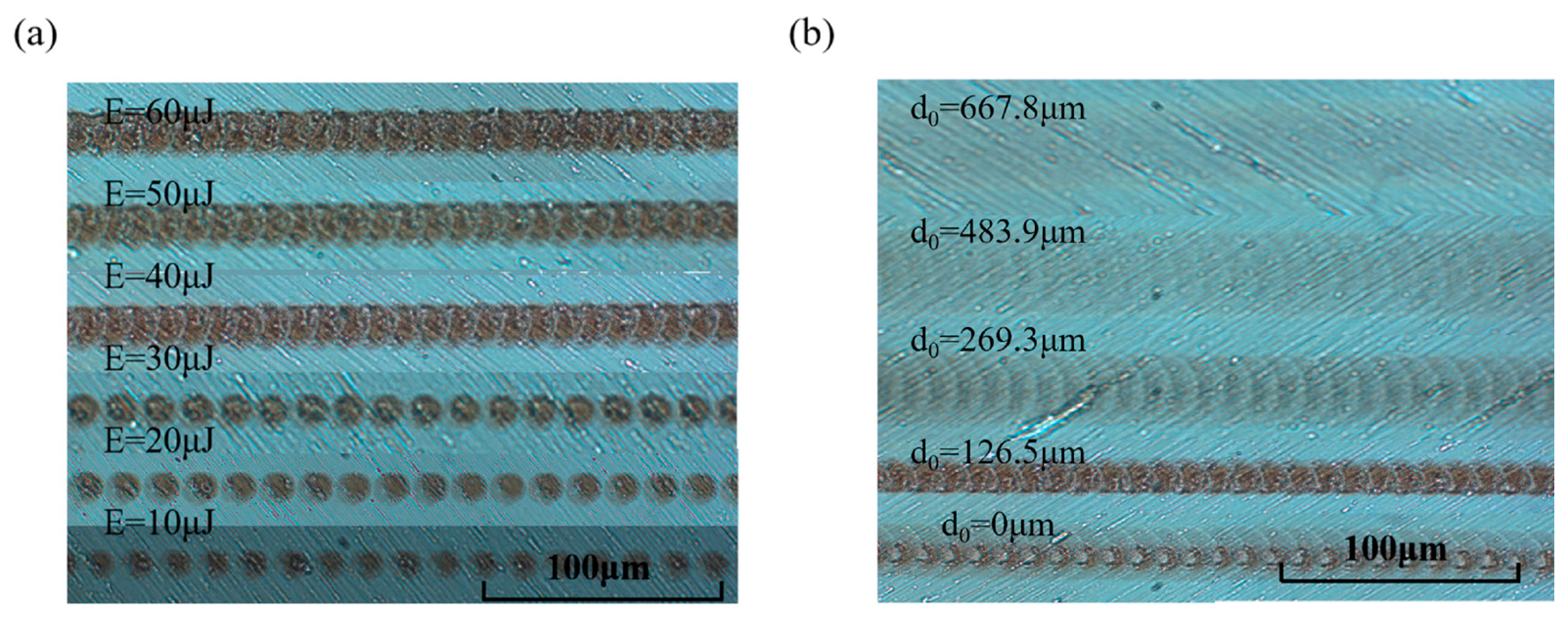
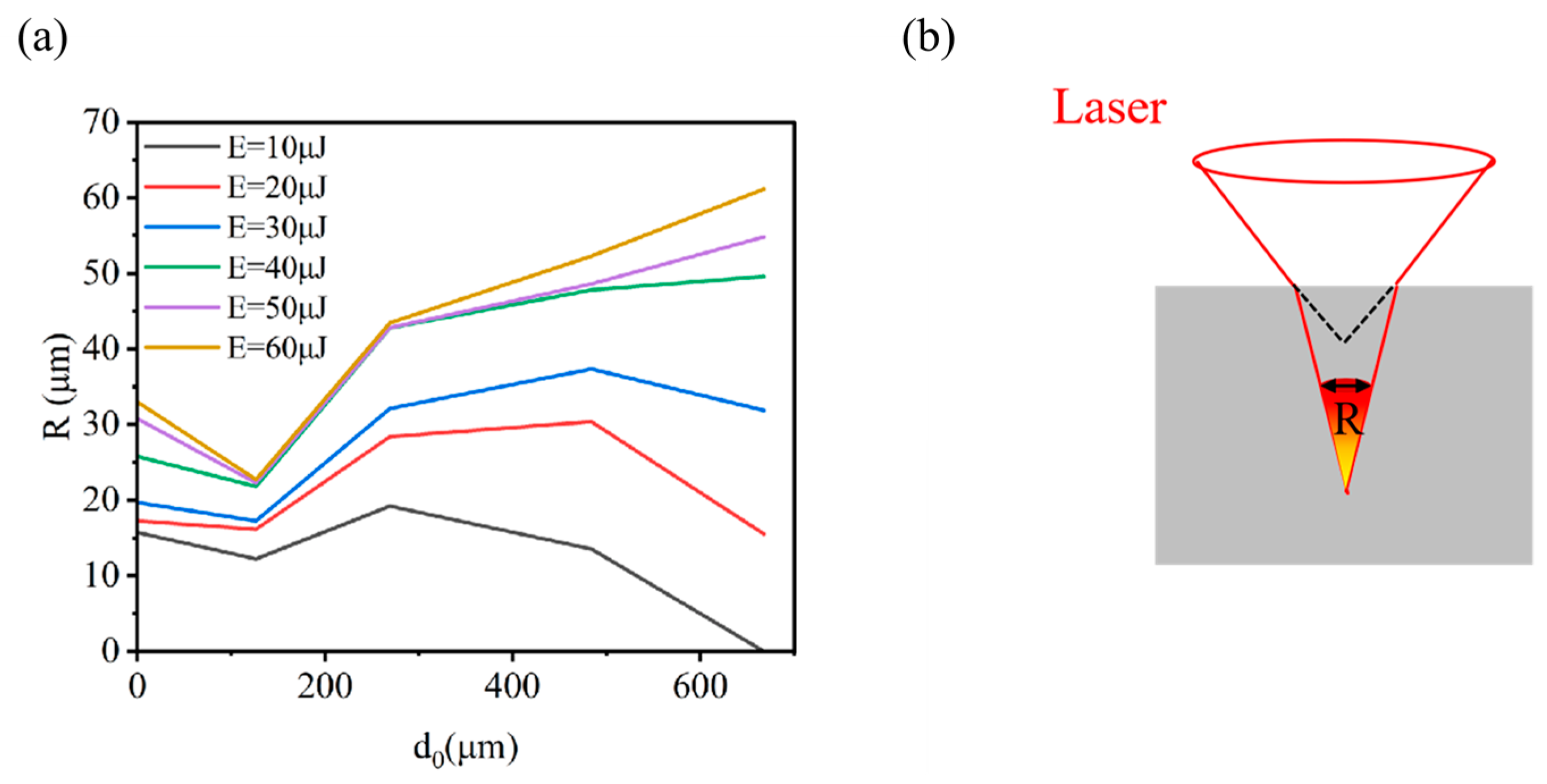
- In experiment, the increase of the modification radius is observed to correlate with an increase of laser pulse energy. Deeper layers of 4H-SiC exhibit a larger modification radius compared to shallow layers. This phenomenon is attributed to the laser beam being absorbed and fully heated by the material before reaching the designed focusing depth, contributing to the spot radius expansion, further enhancing the modification radius.
- (3)
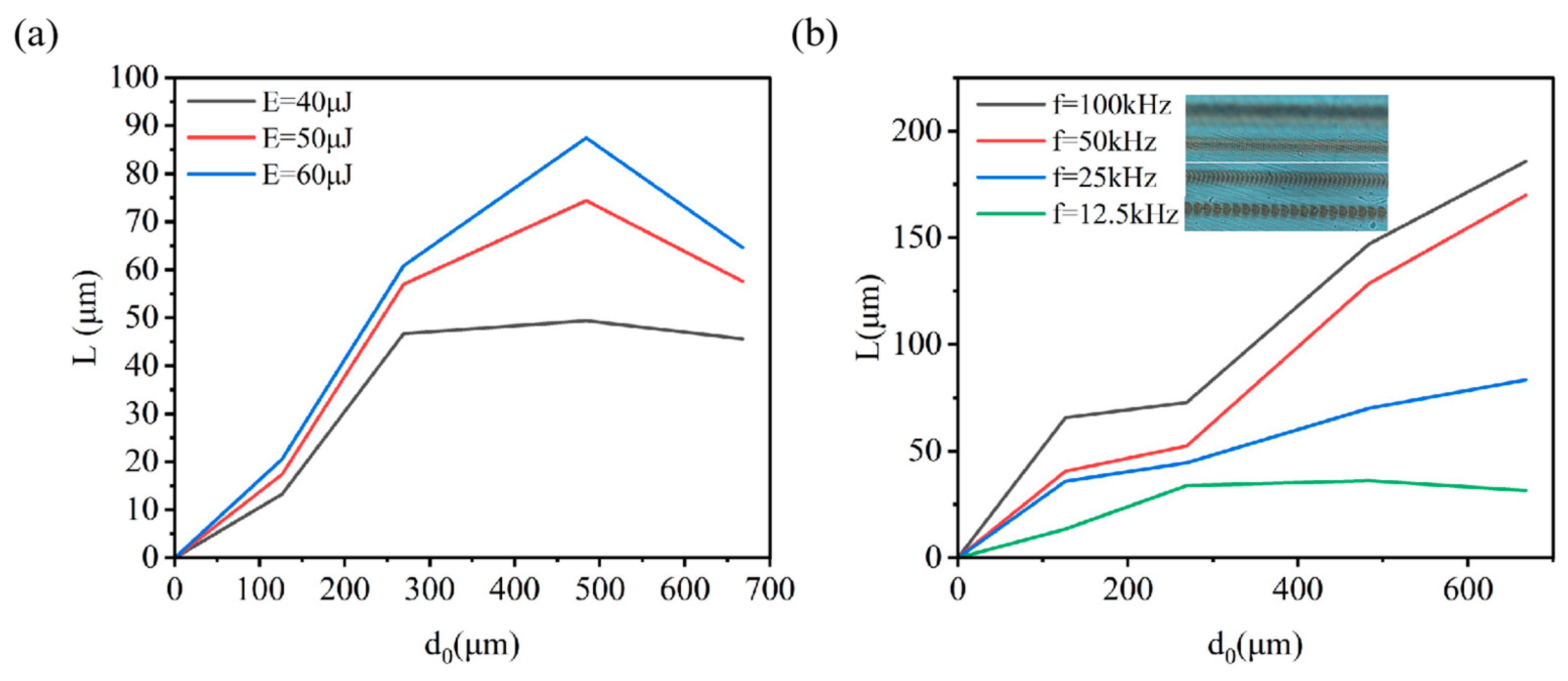
- In experiment, the depth of modification increases with the increase of laser pulse energy. Besides, as the focusing depth increases, this leads to a concurrent increase in the modification depth because of self-focusing within certain limits. However, it is crucial to note that beyond a certain point, the decreased energy density becomes a decisive factor. As the focusing depth further increases, the diminishing energy density contributes to a subsequent reduction in the extent of the modification depth.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mandal, K.C.; Kleppinger, J.W.; Chaudhuri, S.K. Advances in High-Resolution Radiation Detection Using 4H-SiC Epitaxial Layer Devices. Micromachines 2020, 11, 254. [Google Scholar] [CrossRef]
- Shi, X.; Zhang, J.; Fan, W.; Lu, Y.; Peng, N.; Rottwitt, K.; Ou, H. Compact low-birefringence polarization beam splitter using vertical-dual-slot waveguides in silicon carbide integrated platforms. Photonics Res. 2022, 10, A8–A13. [Google Scholar] [CrossRef]
- Zhou, X.; Lü, Y.; Guo, H.; Song, X.; Wang, Y.; Liang, S.; Bu, A.; Feng, Z. High-stability 4H-SiC avalanche photodiodes for UV detection at high temperatures. Chin. Opt. Lett. 2023, 21, 032502. [Google Scholar] [CrossRef]
- Cheng, J.; Peng, Z.; Zhang, W.; Shao, L. Metal-Free High-Overtone Bulk Acoustic Resonators with Outstanding Acoustic Match and Thermal Stability. IEEE Electron Device Lett. 2023, 44, 1877–1880. [Google Scholar] [CrossRef]
- Tang, Z.; Tang, X.; Zhang, Y.; Zhao, P.; Sun, Y.; Zhang, Y. 4H-SiC integrated circuits for high-temperature applications. J. Cryst. Growth 2023, 605, 127060. [Google Scholar] [CrossRef]
- Cao, X.; Li, B.; Wang, Y.; Fu, Y.; Yin, H.; Chen, Z. Experimental quantum e-commerce. Sci. Adv. 2024, 10, eadk3258. [Google Scholar] [CrossRef] [PubMed]
- Yin, H.; Fu, Y.; Li, C.; Weng, C.; Li, B.; Gu, J.; Lu, Y.; Huang, S.; Chen, Z. Experimental quantum secure network with digital signatures and encryption. Natl. Sci. Rev. 2022, 10, nwac228. [Google Scholar] [CrossRef] [PubMed]
- Wei, K.; Li, W.; Tan, H.; Li, Y.; Min, H.; Zhang, W.; Li, H.; You, L.; Wang, Z.; Jiang, X.; et al. High-Speed Measurement-Device-Independent Quantum Key Distribution with Integrated Silicon Photonics. Phys. Rev. X 2020, 10, 031030. [Google Scholar] [CrossRef]
- Hardin, C.W.; Qu, J.; Shih, A.J. Fixed Abrasive Diamond Wire Saw Slicing of Single-Crystal Silicon Carbide Wafers. Mater. Manuf. Process. 2004, 19, 355–367. [Google Scholar] [CrossRef]
- Shi, X.; Lu, Y.; Chaussende, D.; Rottwitt, K.; Ou, H. Wet-Oxidation-Assisted Chemical Mechanical Polishing and High-Temperature Thermal Annealing for Low-Loss 4H-SiC Integrated Photonic Devices. Materials 2023, 16, 2324. [Google Scholar] [CrossRef] [PubMed]
- Liao, Y.; Shin, S.; Kim, M. Ultraviolet antireflective porous nanoscale periodic hole array of 4H-SiC by Photon-Enhanced Metal-assisted chemical etching. Appl. Surf. Sci. 2022, 581, 152387. [Google Scholar] [CrossRef]
- Wu, S.; Wu, D.; Xu, J.; Hanada, Y.; Suganuma, R.; Wang, H.; Makimura, T.; Sugioka, K.; Midorikawa, K. Characterization and mechanism of glass microwelding by double-pulse ultrafast laser irradiation. Opt. Express 2012, 20, 28893–28905. [Google Scholar] [CrossRef] [PubMed]
- Zhao, S.; He, H.; Han, S.; Jiang, L.; Du, J.; Yu, H.; Lin, X.; Zhang, G. Research on key issues of laser splitting of transparent hard and brittle materials. Infrared Laser Eng. 2024, 53, 20230487. [Google Scholar]
- Qiu, J.; Kojima, K.; Miura, K.; Mitsuyu, T.; Hirao, K. Infrared femtosecond laser pulse–induced permanent reduction of Eu3+ to Eu2+ in a fluorozirconate glass. Opt. Lett. 1999, 24, 786–788. [Google Scholar] [CrossRef]
- Qiu, J.; Jiang, X.; Zhu, C.; Inouye, H.; Si, J.; Hirao, K. Optical properties of structurally modified glasses doped with gold ions. Opt. Lett. 2004, 29, 370–372. [Google Scholar] [CrossRef]
- Davis, K.M.; Miura, K.; Sugimoto, N.; Hirao, K. Writing waveguides in glass with a femtosecond laser. Opt. Lett. 1996, 21, 1729–1731. [Google Scholar] [CrossRef]
- Cai, Y.; Yang, L.; Zhang, H.; Wang, Y. Laser cutting silicon-glass double layer wafer with laser induced thermal-crack propagation. Opt. Lasers Eng. 2016, 82, 173–185. [Google Scholar] [CrossRef]
- Erwei, S. The Growth and Defects of Silicon Carbide Crystal; Science Press: Beijing, China, 2012. [Google Scholar]
- Han, S.; Yu, H.; He, C.; Zhao, S.; Ning, C.; Jiang, L.; Lin, X. Laser slicing of 4H-SiC wafers based on picosecond laser-induced micro-explosion via multiphoton processes. Opt. Laser Technol. 2022, 154, 108323. [Google Scholar] [CrossRef]
- Zhang, Y.; Xie, X.; Huang, Y.; Hu, W.; Long, J. Internal modified structure of silicon carbide prepared by ultrafast laser for wafer slicing. Ceram. Int. 2023, 49, 5249–5260. [Google Scholar] [CrossRef]
- Wang, H.; Chen, Q.; Yao, Y.; Che, L.; Zhang, B.; Nie, H.; Wang, R. Influence of Surface Preprocessing on 4H-SiC Wafer Slicing by Using Ultrafast Laser. Crystals 2023, 13, 15. [Google Scholar] [CrossRef]
- Mohammed, A.F.; Al-Jarwany, Q.A.; Clarke, A.J.; Amaral, T.M.; Lawrence, J.; Kemp, N.T.; Walton, C.D. Ablation threshold measurements and surface modifications of 193 nm laser irradiated 4H-SiC. Chem. Phys. Lett. 2018, 713, 194–202. [Google Scholar] [CrossRef]
- Huang, Y.; Zhou, Y.; Li, J.; Zhu, F. Femtosecond laser surface modification of 4H-SiC improves machinability. Appl. Surf. Sci. 2023, 615, 156436. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, C.; Liu, F.; Zheng, H.; Cheng, G.J. Ultrafast pulsed laser stealth dicing of 4H-SiC wafer: Structure evolution and defect generation. J. Manuf. Process. 2022, 81, 562–570. [Google Scholar] [CrossRef]
- Jiang, L.; Wang, A.-D.; Li, B.; Cui, T.-H.; Lu, Y.-F. Electrons dynamics control by shaping femtosecond laser pulses in micro/nanofabrication: Modeling, method, measurement and application. Light Sci. Appl. 2018, 7, 17134. [Google Scholar] [CrossRef]
- Sun, Q.; Jiang, H.; Liu, Y.; Zhou, Y.; Yang, H.; Gong, Q. Effect of spherical aberration on the propagation of a tightly focused femtosecond laser pulse inside fused silica. J. Opt. A Pure Appl. Opt. 2005, 7, 655. [Google Scholar] [CrossRef]
- Nirmala, K.S.; Ramana, M.S.; Tewari, S.P. Tuning of ring laser by varying the Gouy phase. Opt. Commun. 2011, 284, 2560–2564. [Google Scholar] [CrossRef]
- Halliday, D.; Resnick, R.; Walker, J. Fundamentals of Physics; Wiley: Hoboken, NJ, USA, 2002. [Google Scholar]
- Harish, D.V.N.; Bharatish, A.; Murthy, H.N.N.; Anand, B.; Subramanya, K.N. Investigation of thermal residual stresses during laser ablation of tantalum carbide coated graphite substrates using micro-Raman spectroscopy and COMSOL multiphysics. Ceram. Int. 2021, 47, 3498–3513. [Google Scholar] [CrossRef]
- Youngworth, K.; Brown, T. Focusing of high numerical aperture cylindrical-vector beams. Opt. Express 2000, 7, 77–87. [Google Scholar] [CrossRef]
- Gao, R.; Gao, S.; Fan, G.; Liu, J.; Wang, Q.; Zhao, H.; Qu, S. Enhancement of surface photoconductivityin 6H-SiC crystal modified by femtosecond laser pulse irradiation. Acta Phys. Sin. 2014, 63, 6. [Google Scholar] [CrossRef]





| Factor | Unit | Level | |||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | ||
| Laser pulse energy E | μJ | 10 | 20 | 30 | 40 | 50 | 60 |
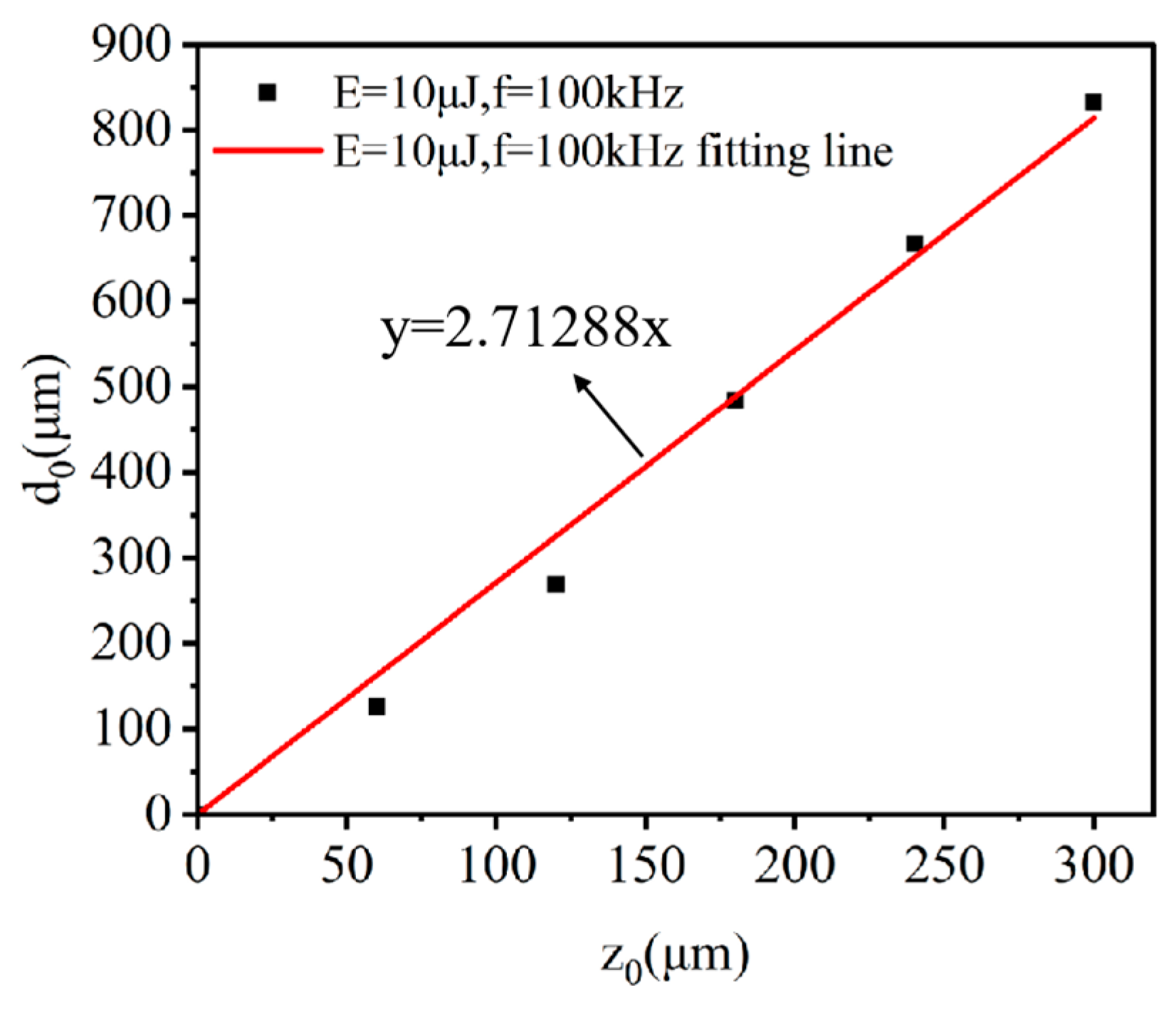
| Displacement of objective lens z0 | μm | 0 | 60 | 120 | 180 | 240 | none |
| Calculated focusing depth d0 | μm | 0 | 166 | 333 | 500 | 667 | none |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Zhao, S.; He, H.; Liang, H.; Dai, Z.; Lin, X.; Zhang, G. Numerical Simulation and Experimental Investigation of ps Pulsed Laser Modification inside 4H-SiC Material. Photonics 2024, 11, 189. https://doi.org/10.3390/photonics11020189
Song Y, Zhao S, He H, Liang H, Dai Z, Lin X, Zhang G. Numerical Simulation and Experimental Investigation of ps Pulsed Laser Modification inside 4H-SiC Material. Photonics. 2024; 11(2):189. https://doi.org/10.3390/photonics11020189
Chicago/Turabian StyleSong, Yiying, Shusen Zhao, Hongzhi He, Han Liang, Zhanfeng Dai, Xuechun Lin, and Guling Zhang. 2024. "Numerical Simulation and Experimental Investigation of ps Pulsed Laser Modification inside 4H-SiC Material" Photonics 11, no. 2: 189. https://doi.org/10.3390/photonics11020189
APA StyleSong, Y., Zhao, S., He, H., Liang, H., Dai, Z., Lin, X., & Zhang, G. (2024). Numerical Simulation and Experimental Investigation of ps Pulsed Laser Modification inside 4H-SiC Material. Photonics, 11(2), 189. https://doi.org/10.3390/photonics11020189





