Analysis of Metallic-to-Oxide Sputtering Mode Transition During Reactive Magnetron Deposition of Aluminum Oxide Coatings
Abstract
1. Introduction
2. Materials and Methods
3. Results
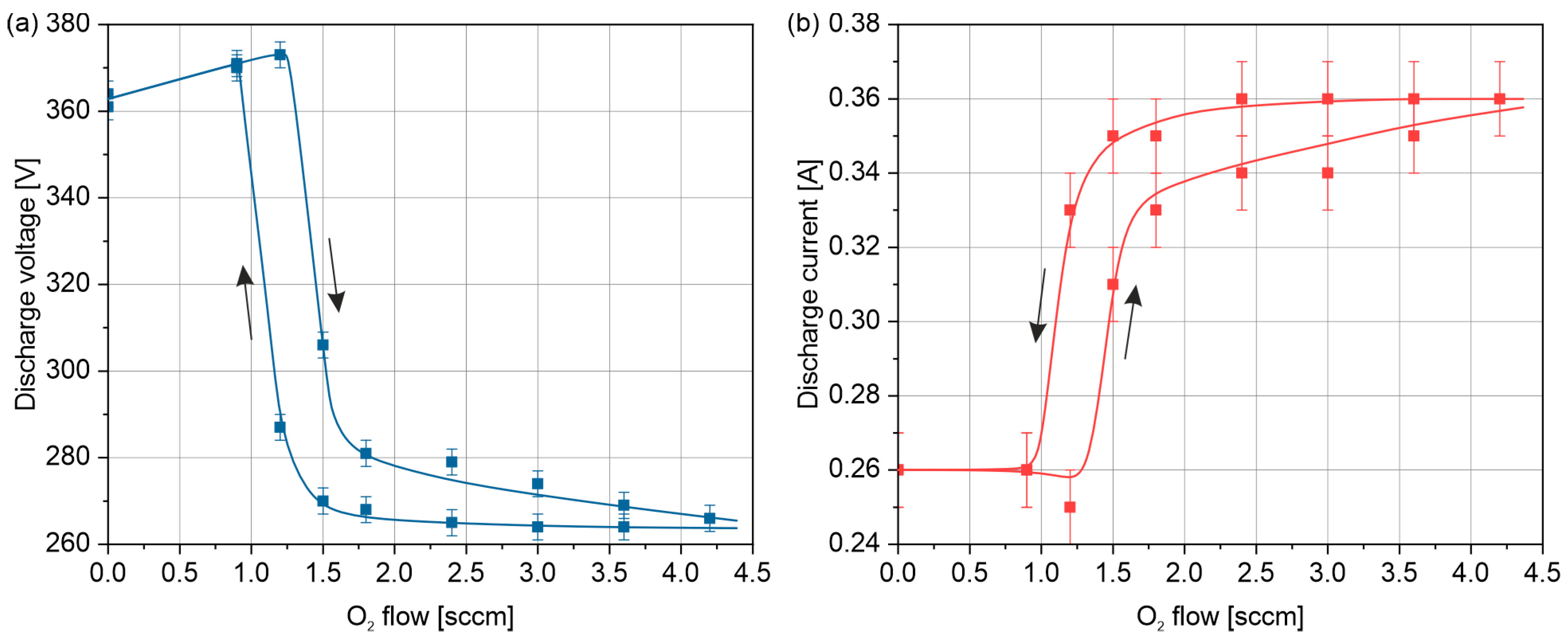
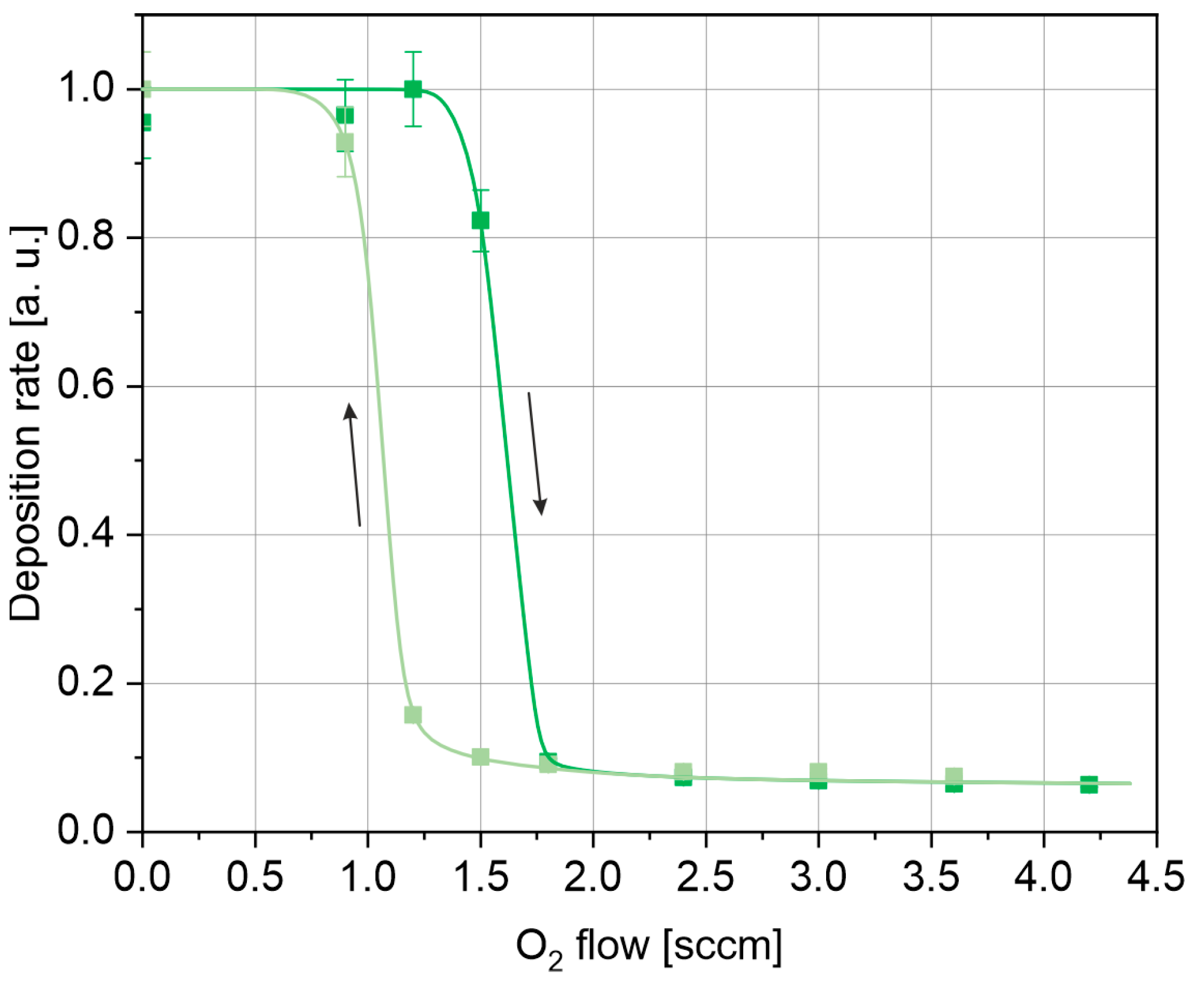
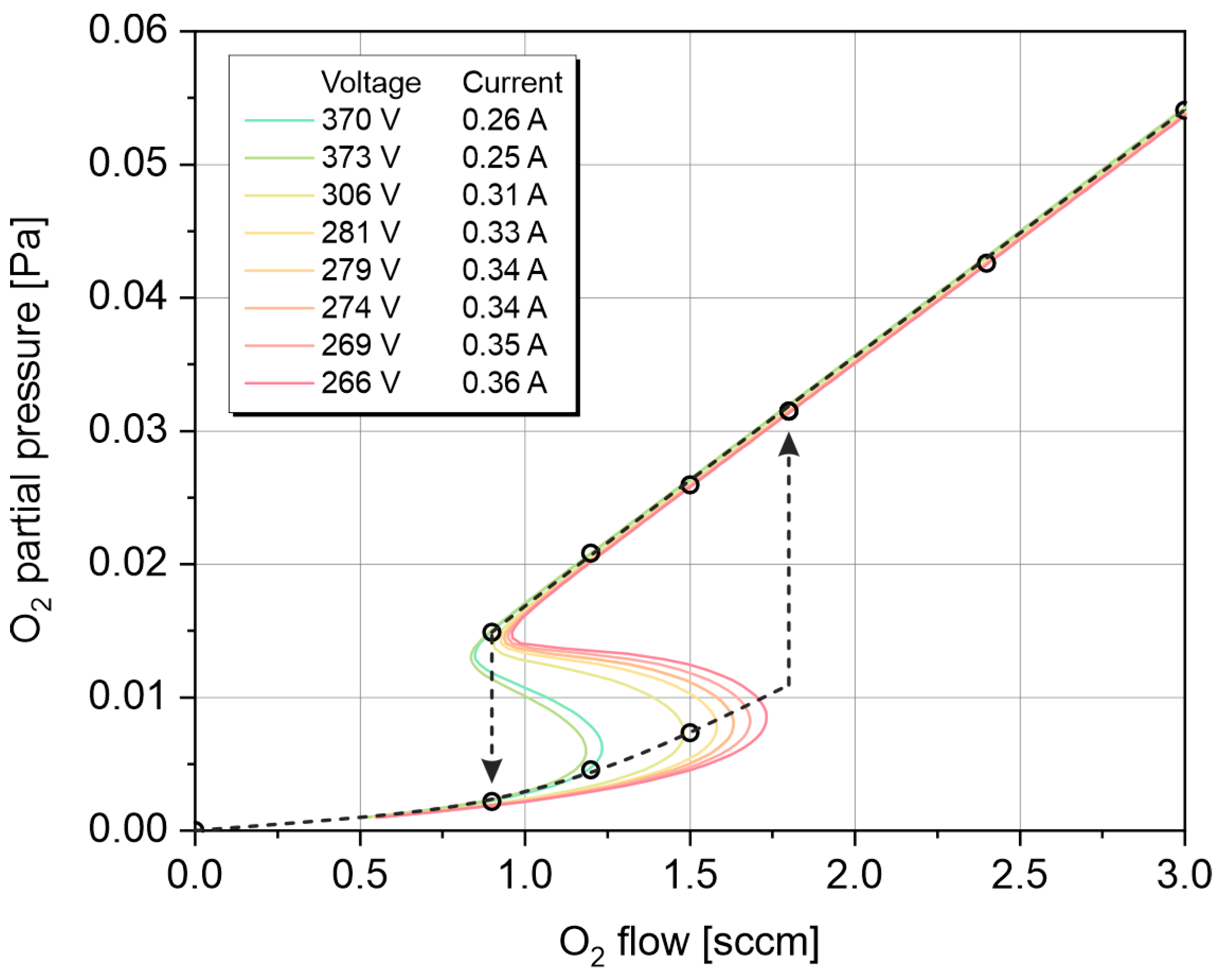
3.1. Electrical Parameters
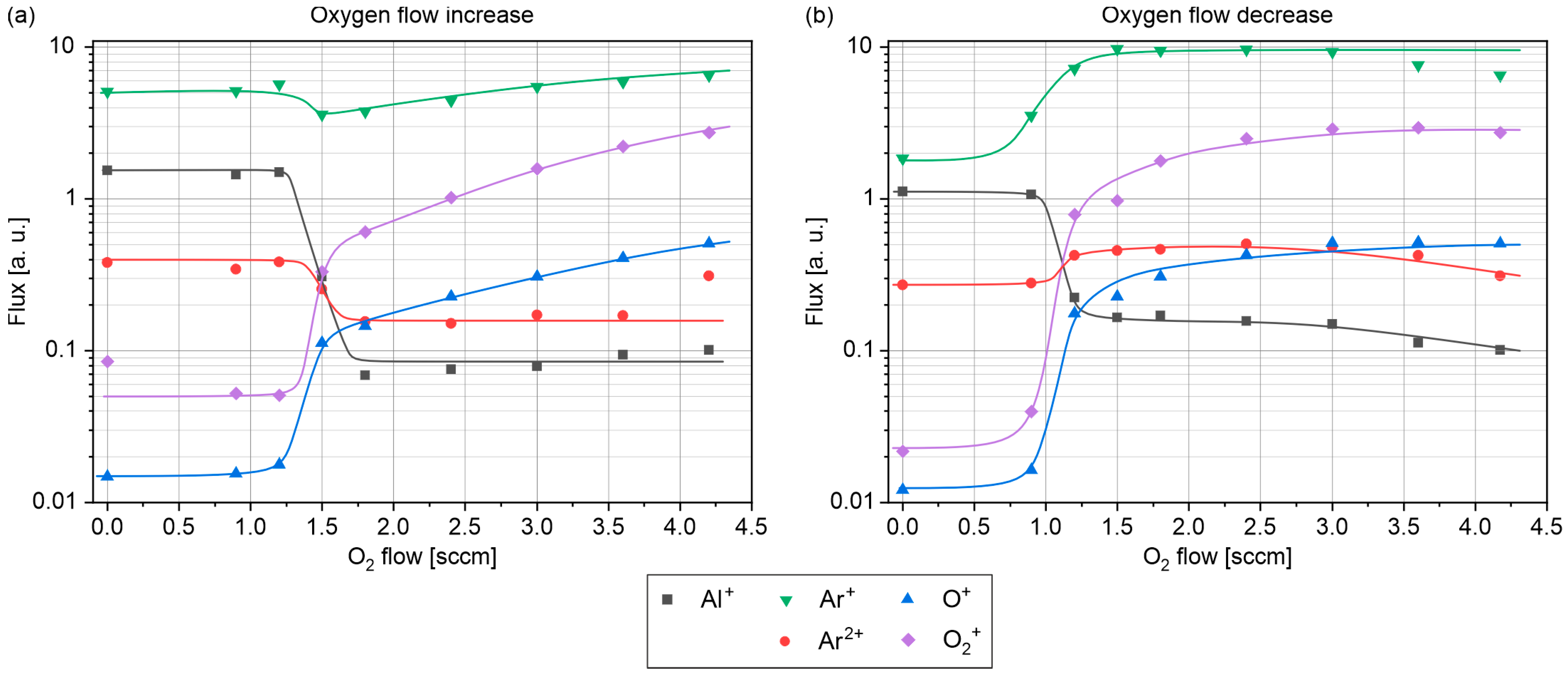
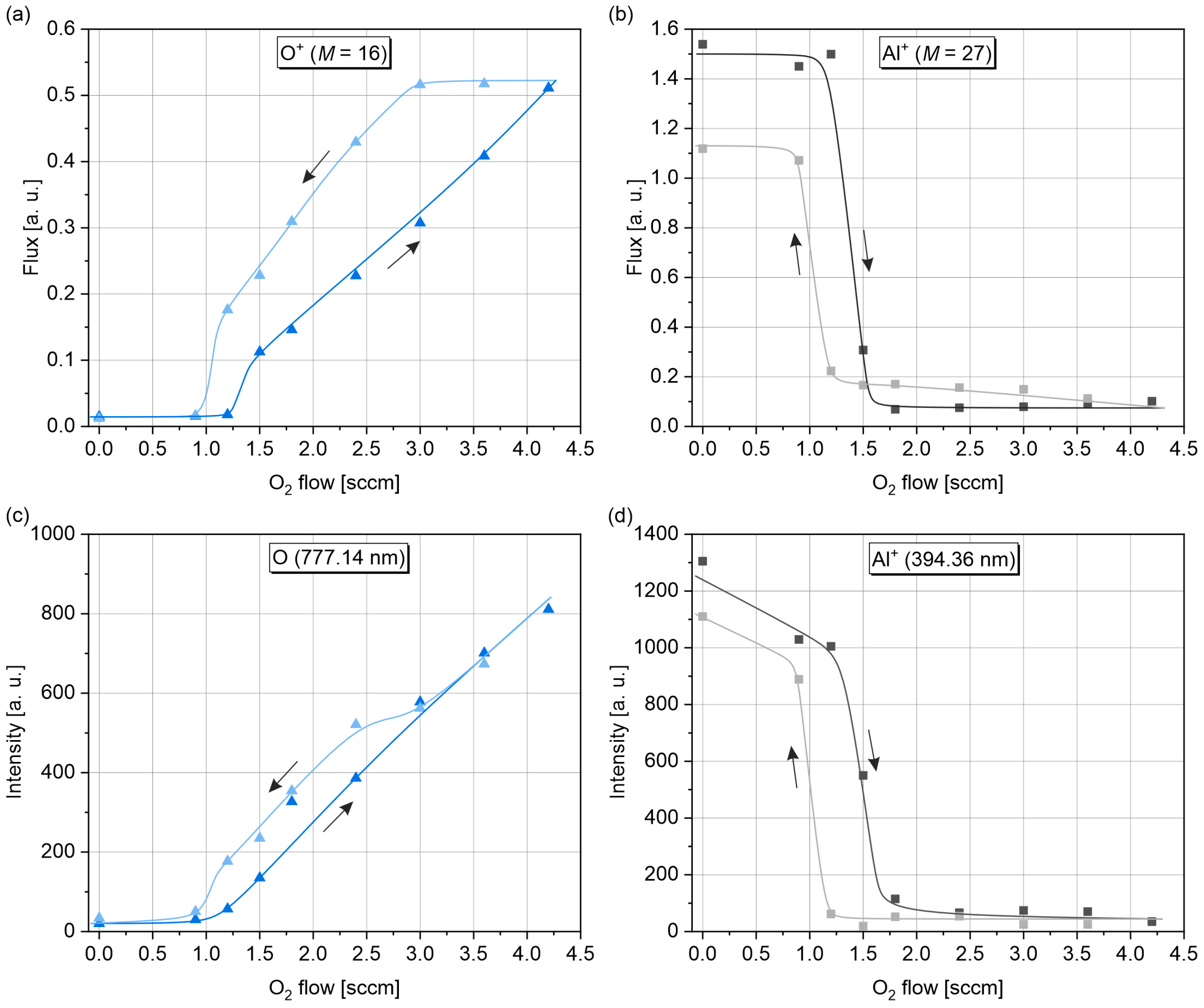
3.2. Ion Mass Spectrometry
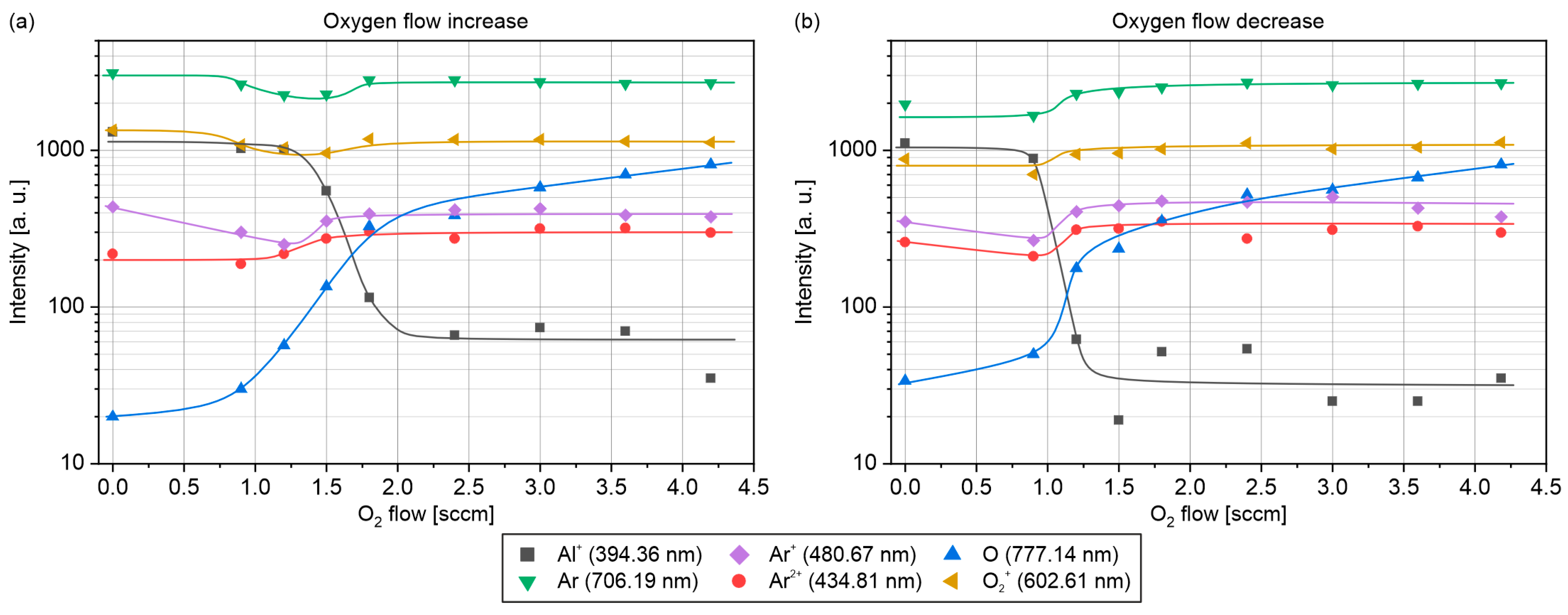
3.3. Optical Emission Spectroscopy
4. Discussion
[chemisorption] [sputtering]
[chemisorption] [oxide growth] [deposition of metal]
[balance of oxygen species in the chamber]
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, G.; Wang, L.; Wang, X.; Yu, Y.; Mutzke, A. Effect of Bias Voltage on Microstructure and Optical Properties of Al2O3 Thin Films Prepared by Twin Targets Reactive High Power Impulse Magnetron Sputtering. Vacuum 2019, 166, 88–96. [Google Scholar] [CrossRef]
- Zhou, G.; Wang, L.; Wang, X.; Yu, Y. Investigating the Plasma Parameters and Discharge Asymmetry in Dual Magnetron Reactive High Power Impulse Magnetron Sputtering Discharge with Al in Ar/O2 Mixture. Vacuum 2020, 175, 109253. [Google Scholar] [CrossRef]
- Tang, X.; Li, Z.; Liao, H.; Zhang, J. Growth of Ultrathin Al2O3 Films on N-InP Substrates as Insulating Layers by RF Magnetron Sputtering and Study on the Optical and Dielectric Properties. Coatings 2019, 9, 341. [Google Scholar] [CrossRef]
- Mensah, S.L.; Gordon, M.; Naseem, H.H. Investigating the Plasma Parameters of an Ar/O2 Discharge during the Sputtering of Al Targets in an Inverted Cylindrical Magnetron. Phys. Plasmas 2014, 21, 093510. [Google Scholar] [CrossRef]
- Lin, Y. Optimization of Deposition Parameters for α-Al2O3 Coatings by Double Glow Plasma Technique. Kem. u Ind. 2015, 64, 457–466. [Google Scholar] [CrossRef]
- Kohara, T.; Tamagaki, H.; Ikari, Y.; Fujii, H. Deposition of α-Al2O3 Hard Coatings by Reactive Magnetron Sputtering. Surf. Coat. Technol. 2004, 185, 166–171. [Google Scholar] [CrossRef]
- Gavrilov, N.V.; Kamenetskikh, A.S.; Trernikov, P.V.; Emlin, D.R.; Chukin, A.V.; Surkov, Y.S. Al2O3 Thin Films Deposition by Reactive Evaporation of Al in Anodic Arc with High Levels of Metal Ionization. Surf. Coat. Technol. 2019, 359, 117–124. [Google Scholar] [CrossRef]
- Engelhart, W.; Dreher, W.; Eibl, O.; Schier, V. Deposition of Alumina Thin Film by Dual Magnetron Sputtering: Is It γ-Al2O3? Acta Mater. 2011, 59, 7757–7767. [Google Scholar] [CrossRef]
- Angarita, G.; Palacio, C.; Trujillo, M.; Arroyave, M. Synthesis of Alumina Thin Films Using Reactive Magnetron Sputtering Method. J. Phys. Conf. Ser. 2017, 850, 012022. [Google Scholar] [CrossRef]
- Li, Q.; Yu, Y.-H.; Singh Bhatia, C.; Marks, L.D.; Lee, S.C.; Chung, Y.W. Low-Temperature Magnetron Sputter-Deposition, Hardness, and Electrical Resistivity of Amorphous and Crystalline Alumina Thin Films. J. Vac. Sci. Technol. A Vac. Surf. Film. 2000, 18, 2333–2338. [Google Scholar] [CrossRef]
- Depla, D.; Heirwegh, S.; Mahieu, S.; Haemers, J.; De Gryse, R. Understanding the Discharge Voltage Behavior during Reactive Sputtering of Oxides. J. Appl. Phys. 2007, 101, 013301. [Google Scholar] [CrossRef]
- Gudmundsson, J.T. Physics and Technology of Magnetron Sputtering Discharges. Plasma Sources Sci. Technol. 2020, 29, 113001. [Google Scholar] [CrossRef]
- Schulte, J.; Sobe, G. Magnetron Sputtering of Aluminium Using Oxygen or Nitrogen as Reactive Gas. Thin Solid Film. 1998, 324, 19–24. [Google Scholar] [CrossRef]
- Strijckmans, K.; Schelfhout, R.; Depla, D. Tutorial: Hysteresis during the Reactive Magnetron Sputtering Process. J. Appl. Phys. 2018, 124, 241101. [Google Scholar] [CrossRef]
- Belkind, A.; Freilich, A.; Lopez, J.; Zhao, Z.; Zhu, W.; Becker, K. Characterization of Pulsed Dc Magnetron Sputtering Plasmas. New J. Phys. 2005, 7, 90. [Google Scholar] [CrossRef]
- Kadlec, S.; Musil, J.; Vyskocil, H. Hysteresis Effect in Reactive Sputtering: A Problem of System Stability. J. Phys. D Appl. Phys. 1986, 19, L187–L190. [Google Scholar] [CrossRef]
- Kubart, T.; Kappertz, O.; Nyberg, T.; Berg, S. Dynamic Behaviour of the Reactive Sputtering Process. Thin Solid Film. 2006, 515, 421–424. [Google Scholar] [CrossRef]
- Sproul, W.D.; Christie, D.J.; Carter, D.C. Control of Reactive Sputtering Processes. Thin Solid Film. 2005, 491, 1–17. [Google Scholar] [CrossRef]
- Wang, Q.; Fang, L.; Liu, Q.; Chen, L.; Wang, Q.; Meng, X.; Xiao, H. Target Voltage Hysteresis Behavior and Control Point in the Preparation of Aluminum Oxide Thin Films by Medium Frequency Reactive Magnetron Sputtering. Coatings 2018, 8, 146. [Google Scholar] [CrossRef]
- Wiatrowski, A.; Patela, S.; Kunicki, P.; Posadowski, W. Effective Reactive Pulsed Magnetron Sputtering of Aluminium Oxide—Properties of Films Deposited Utilizing Automated Process Stabilizer. Vacuum 2016, 134, 54–62. [Google Scholar] [CrossRef]
- Strijckmans, K.; Moens, F.; Depla, D. Perspective: Is There a Hysteresis during Reactive High Power Impulse Magnetron Sputtering (R-HiPIMS)? J. Appl. Phys. 2017, 121, 080901. [Google Scholar] [CrossRef]
- Särhammar, E.; Strijckmans, K.; Nyberg, T.; Van Steenberge, S.; Berg, S.; Depla, D. A Study of the Process Pressure Influence in Reactive Sputtering Aiming at Hysteresis Elimination. Surf. Coat. Technol. 2013, 232, 357–361. [Google Scholar] [CrossRef]
- Madsen, N.D.; Christensen, B.H.; Louring, S.; Berthelsen, A.N.; Almtoft, K.P.; Nielsen, L.P.; Bøttiger, J. Controlling the Deposition Rate during Target Erosion in Reactive Pulsed DC Magnetron Sputter Deposition of Alumina. Surf. Coat. Technol. 2012, 206, 4850–4854. [Google Scholar] [CrossRef]
- Kaziev, A.V.; Kolodko, D.V.; Sergeev, N.S. Properties of Millisecond-Scale Modulated Pulsed Power Magnetron Discharge Applied for Reactive Sputtering of Zirconia. Plasma Sources Sci. Technol. 2021, 30, 055002. [Google Scholar] [CrossRef]
- Depla, D.; Haemers, J.; De Gryse, R. Influencing the Hysteresis during Reactive Magnetron Sputtering by Gas Separation. Surf. Coat. Technol. 2013, 235, 62–67. [Google Scholar] [CrossRef]
- Anders, A. Tutorial: Reactive High Power Impulse Magnetron Sputtering (R-HiPIMS). J. Appl. Phys. 2017, 121, 171101. [Google Scholar] [CrossRef]
- Schneider, J.M.; Sproul, W.D.; Chia, R.W.J.; Wong, M.-S.; Matthews, A. Very-High-Rate Reactive Sputtering of Alumina Hard Coatings. Surf. Coat. Technol. 1997, 96, 262–266. [Google Scholar] [CrossRef]
- Oskirko, V.O.; Zakharov, A.N.; Pavlov, A.P.; Solovyev, A.A.; Grenadyorov, A.S.; Semenov, V.A. Dual Mode of Deep Oscillation Magnetron Sputtering. Surf. Coat. Technol. 2020, 387, 125559. [Google Scholar] [CrossRef]
- Lin, J.; Sproul, W.D. Structure and Properties of Cr2O3 Coatings Deposited Using DCMS, PDCMS, and DOMS. Surf. Coat. Technol. 2015, 276, 70–76. [Google Scholar] [CrossRef]
- Kuschel, T.; von Keudell, A. Ion-Enhanced Oxidation of Aluminum as a Fundamental Surface Process during Target Poisoning in Reactive Magnetron Sputtering. J. Appl. Phys. 2010, 107, 103302. [Google Scholar] [CrossRef]
- Houska, J.; Kozak, T. Distribution of O Atoms on Partially Oxidized Metal Targets, and the Consequences for Reactive Sputtering of Individual Metal Oxides. Surf. Coat. Technol. 2020, 392, 125685. [Google Scholar] [CrossRef]
- Depla, D.; De Gryse, R. Target Poisoning during Reactive Magnetron Sputtering: Part II: The Influence of Chemisorption and Gettering. Surf. Coat. Technol. 2004, 183, 190–195. [Google Scholar] [CrossRef]
- Depla, D.; Colpaert, A.; Eufinger, K.; Segers, A.; Haemers, J.; De Gryse, R. Target Voltage Behaviour during DC Sputtering of Silicon in an Argon/Nitrogen Mixture. Vacuum 2002, 66, 9–17. [Google Scholar] [CrossRef]
- Depla, D. Note on the Low Deposition Rate during Reactive Magnetron Sputtering. Vacuum 2024, 228, 113546. [Google Scholar] [CrossRef]
- Novotný, M.; Bulíř, J.; Pokorný, P.; Bočan, J.; Fitl, P.; Lančok, J.; Musil, J. Optical Emission and Mass Spectroscopy of Plasma Processes in Reactive DC Pulsed Magnetron Sputtering of Aluminium Oxide. J. Optoelectron. Adv. Mater. 2010, 12, 697–700. [Google Scholar]
- Ries, S.; Bibinov, N.; Rudolph, M.; Schulze, J.; Mráz, S.; Schneider, J.M.; Awakowicz, P. Spatially Resolved Characterization of a Dc Magnetron Plasma Using Optical Emission Spectroscopy. Plasma Sources Sci. Technol. 2018, 27, 094001. [Google Scholar] [CrossRef]
- Vašina, P.; Fekete, M.; Hnilica, J.; Klein, P.; Dosoudilová, L.; Dvořák, P.; Navrátil, Z. Determination of Titanium Atom and Ion Densities in Sputter Deposition Plasmas by Optical Emission Spectroscopy. Plasma Sources Sci. Technol. 2015, 24, 065022. [Google Scholar] [CrossRef]
- Kolodko, D.V.; Ageychenkov, D.G.; Kaziev, A.V.; Leonova, K.A.; Kharkov, M.M.; Tumarkin, A.V. Diagnostics of Ion Fluxes in Low-Temperature Laboratory and Industrial Plasmas. J. Instrum. 2019, 14, P10005. [Google Scholar] [CrossRef]
- Schmidt, S.; Czigány, Z.; Greczynski, G.; Jensen, J.; Hultman, L. Ion Mass Spectrometry Investigations of the Discharge during Reactive High Power Pulsed and Direct Current Magnetron Sputtering of Carbon in Ar and Ar/N2. J. Appl. Phys. 2012, 112, 013305. [Google Scholar] [CrossRef]
- Pokorný, P.; Mišina, M.; Bulíř, J.; Lančok, J.; Fitl, P.; Musil, J.; Novotný, M. Investigation of the Negative Ions in Ar/O2 Plasma of Magnetron Sputtering Discharge with Al:Zn Target by Ion Mass Spectrometry. Plasma Process. Polym. 2011, 8, 459–464. [Google Scholar] [CrossRef]
- Pokorný, P.; Bulíř, J.; Lančok, J.; Musil, J.; Novotný, M. Generation of Positive and Negative Oxygen Ions in Magnetron Discharge During Reactive Sputtering of Alumina. Plasma Process. Polym. 2010, 7, 910–914. [Google Scholar] [CrossRef]
- Pokorný, P.; Musil, J.; Lančok, J.; Fitl, P.; Novotný, M.; Bulíř, J.; Vlček, J. Mass Spectrometry Investigation of Magnetron Sputtering Discharges. Vacuum 2017, 143, 438–443. [Google Scholar] [CrossRef]
- Mišina, M.; Shaginyan, L.R.; Maček, M.; Panjan, P. Energy Resolved Ion Mass Spectroscopy of the Plasma during Reactive Magnetron Sputtering. Surf. Coat. Technol. 2001, 142–144, 348–354. [Google Scholar] [CrossRef]
- Kolodko, D.V.; Ageychenkov, D.G.; Lisenkov, V.Y.; Kaziev, A. V Evidence of 1000 eV Positive Oxygen Ion Flux Generated in Reactive HiPIMS Plasma. Plasma Sources Sci. Technol. 2023, 32, 06LT01. [Google Scholar] [CrossRef]
- Bowes, M.; Poolcharuansin, P.; Bradley, J.W. Negative Ion Energy Distributions in Reactive HiPIMS. J. Phys. D Appl. Phys. 2013, 46, 045204. [Google Scholar] [CrossRef]
- Hippler, R.; Cada, M.; Hubicka, Z. Energy Distribution of Negatively and Positively Charged Ions in a Magnetron Sputtering Discharge with a Tungsten Cathode and a Positively Biased Anode in an Argon/Oxygen Gas Mixture. Plasma Sources Sci. Technol. 2024, 33, 115006. [Google Scholar] [CrossRef]
- Welzel, T.; Kleinhempel, R.; Dunger, T.; Richter, F. Ion Energy Distributions in Magnetron Sputtering of Zinc Aluminium Oxide. Plasma Process. Polym. 2009, 6, S331–S336. [Google Scholar] [CrossRef]
- Welzel, T.; Ellmer, K. Negative Ions in Reactive Magnetron Sputtering. Vak. Forsch. und Prax. 2013, 25, 52–56. [Google Scholar] [CrossRef]
- Welzel, T.; Ellmer, K. Negative Oxygen Ion Formation in Reactive Magnetron Sputtering Processes for Transparent Conductive Oxides. J. Vac. Sci. Technol. A Vac. Surf. Film. 2012, 30, 061306. [Google Scholar] [CrossRef]
- Richter, F.; Welzel, T.; Kleinhempel, R.; Dunger, T.; Knoth, T.; Dimer, M.; Milde, F. Ion Energy Distributions in AZO Magnetron Sputtering from Planar and Rotatable Magnetrons. Surf. Coat. Technol. 2009, 204, 845–849. [Google Scholar] [CrossRef]
- Berg, S.; Nyberg, T. Fundamental Understanding and Modeling of Reactive Sputtering Processes. Thin Solid Film. 2005, 476, 215–230. [Google Scholar] [CrossRef]
- Berg, S.; Blom, H.-O.; Larsson, T.; Nender, C. Modeling of Reactive Sputtering of Compound Materials. J. Vac. Sci. Technol. A Vac. Surf. Film. 1987, 5, 202–207. [Google Scholar] [CrossRef]
- Karnopp, J.; Sagás, J.C. Including Substrate Temperature in Berg Model for Reactive Sputtering. Thin Solid Film. 2020, 696, 137761. [Google Scholar] [CrossRef]
- Berg, S.; Särhammar, E.; Nyberg, T. Upgrading the “Berg-Model” for Reactive Sputtering Processes. Thin Solid Film. 2014, 565, 186–192. [Google Scholar] [CrossRef]
- Depla, D.; Heirwegh, S.; Mahieu, S.; Gryse, R. De Towards a More Complete Model for Reactive Magnetron Sputtering. J. Phys. D Appl. Phys. 2007, 40, 1957–1965. [Google Scholar] [CrossRef]
- Särhammar, E.; Nyberg, T.; Berg, S. Applying “the Upgraded Berg Model” to Predict Hysteresis Free Reactive Sputtering. Surf. Coat. Technol. 2016, 290, 34–38. [Google Scholar] [CrossRef]
- Strijckmans, K.; Leroy, W.P.; De Gryse, R.; Depla, D. Modeling Reactive Magnetron Sputtering: Fixing the Parameter Set. Surf. Coat. Technol. 2012, 206, 3666–3675. [Google Scholar] [CrossRef]
- Yagisawa, T.; Makabe, T. Modeling of Dc Magnetron Plasma for Sputtering: Transport of Sputtered Copper Atoms. J. Vac. Sci. Technol. A Vac. Surf. Film. 2006, 24, 908–913. [Google Scholar] [CrossRef]
- Strijckmans, K.; Depla, D. A Time-Dependent Model for Reactive Sputter Deposition. J. Phys. D Appl. Phys. 2014, 47, 235302. [Google Scholar] [CrossRef]
- Möller, W.; Güttler, D. Modeling of Plasma-Target Interaction during Reactive Magnetron Sputtering of TiN. J. Appl. Phys. 2007, 102, 094501. [Google Scholar] [CrossRef]
- Pflug, A.; Siemers, M.; Melzig, T.; Schäfer, L.; Bräuer, G. Simulation of Linear Magnetron Discharges in 2D and 3D. Surf. Coat. Technol. 2014, 260, 411–416. [Google Scholar] [CrossRef]
- Kaziev, A.V.; Kolodko, D.V.; Ageychenkov, D.G.; Tumarkin, A.V.; Kharkov, M.M.; Stepanova, T.V. Direct Ion Content Measurements in a Non-Sputtering Magnetron Discharge. J. Instrum. 2019, 14, C09004. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Ver. 5.12), [Online]. 2024. Available online: https://physics.nist.gov/asd (accessed on 3 March 2025).
- Krupenie, P.H. The Spectrum of Molecular Oxygen. J. Phys. Chem. Ref. Data 1972, 1, 423–534. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaziev, A.V.; Tumarkin, A.V.; Kolodko, D.V.; Kharkov, M.M.; Konaguru, R.; Ageychenkov, D.G.; Samotaev, N.N.; Oblov, K.Y. Analysis of Metallic-to-Oxide Sputtering Mode Transition During Reactive Magnetron Deposition of Aluminum Oxide Coatings. Appl. Sci. 2025, 15, 4305. https://doi.org/10.3390/app15084305
Kaziev AV, Tumarkin AV, Kolodko DV, Kharkov MM, Konaguru R, Ageychenkov DG, Samotaev NN, Oblov KY. Analysis of Metallic-to-Oxide Sputtering Mode Transition During Reactive Magnetron Deposition of Aluminum Oxide Coatings. Applied Sciences. 2025; 15(8):4305. https://doi.org/10.3390/app15084305
Chicago/Turabian StyleKaziev, Andrey V., Alexander V. Tumarkin, Dobrynya V. Kolodko, Maksim M. Kharkov, Raghavendra Konaguru, Dmitry G. Ageychenkov, Nikolay N. Samotaev, and Konstantin Yu. Oblov. 2025. "Analysis of Metallic-to-Oxide Sputtering Mode Transition During Reactive Magnetron Deposition of Aluminum Oxide Coatings" Applied Sciences 15, no. 8: 4305. https://doi.org/10.3390/app15084305
APA StyleKaziev, A. V., Tumarkin, A. V., Kolodko, D. V., Kharkov, M. M., Konaguru, R., Ageychenkov, D. G., Samotaev, N. N., & Oblov, K. Y. (2025). Analysis of Metallic-to-Oxide Sputtering Mode Transition During Reactive Magnetron Deposition of Aluminum Oxide Coatings. Applied Sciences, 15(8), 4305. https://doi.org/10.3390/app15084305






