Molecular Interaction and Magnetic Dipole Effects on Fully Developed Nanofluid Flowing via a Vertical Duct Applying Finite Volume Methodology
Abstract
1. Introduction
2. Governing Equations
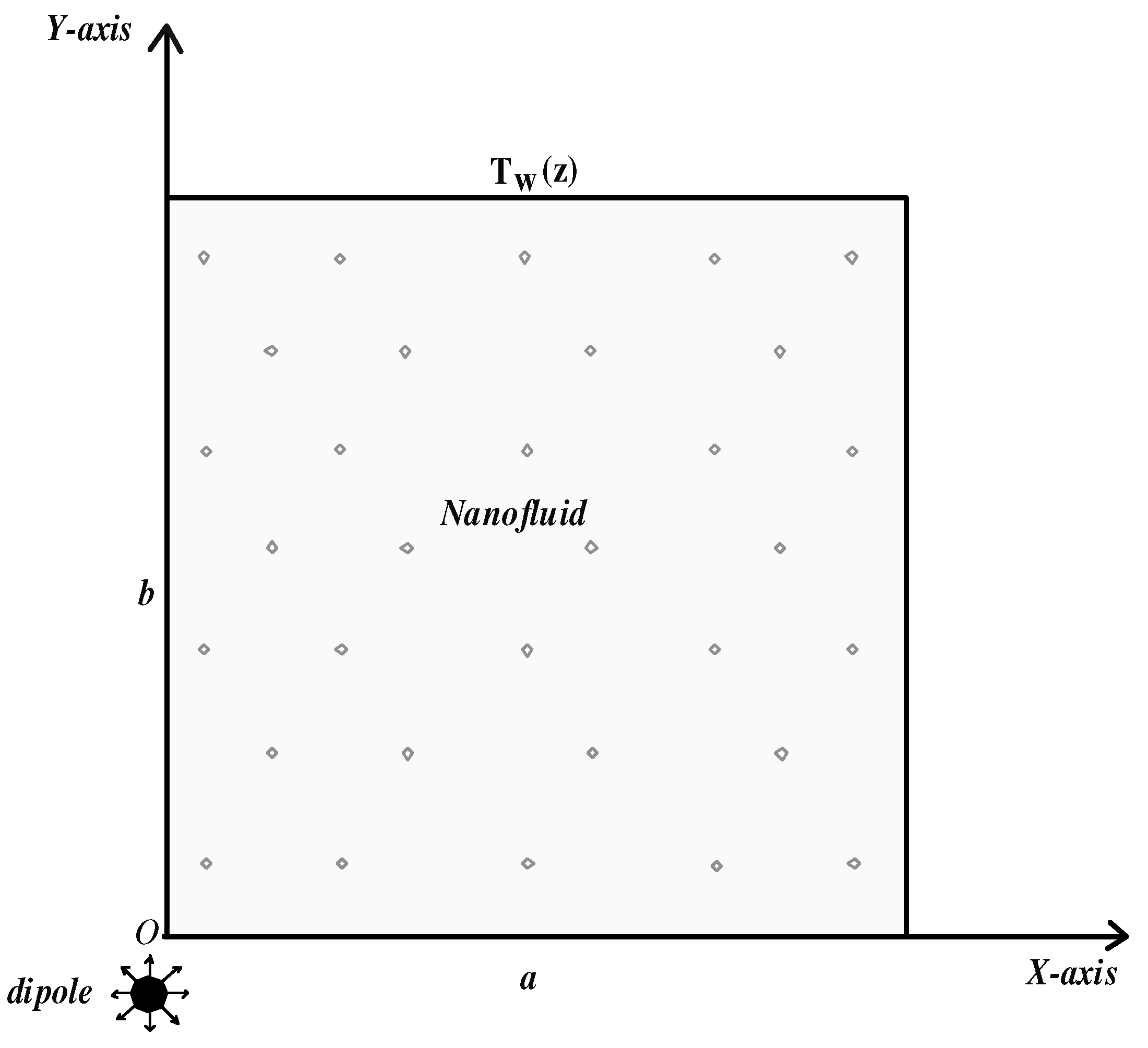
3. Numerical Approach: Finite Volume Method
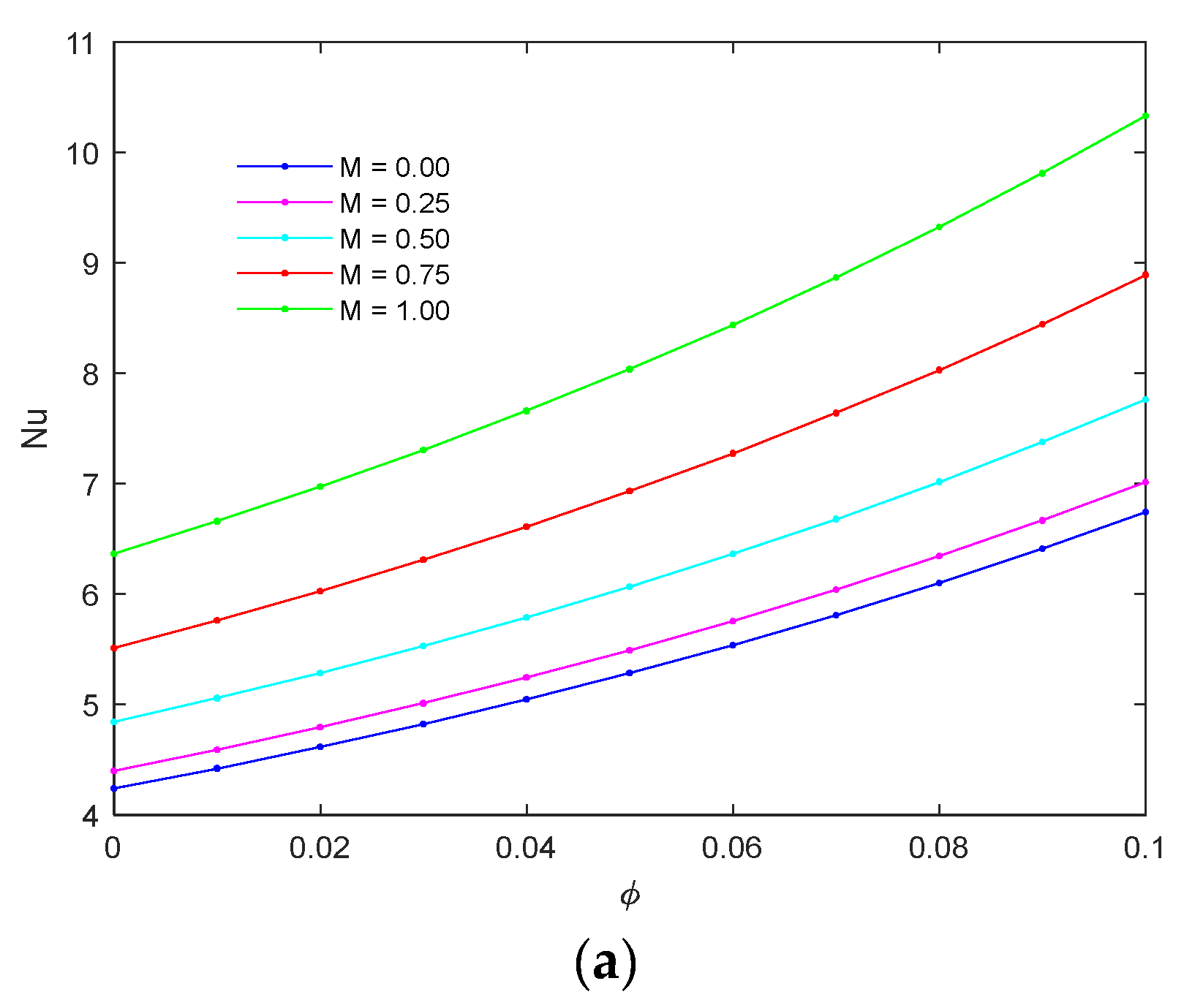
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| List of symbols | |
| magnetic field | |
| magnetic field intensity | |
| magnetic field induction | |
| magnetic field intensity at the origin | |
| ordinate of eastward located neighboring point of point P | |
| ordinate of general point P | |
| ordinate of westward located neighboring point of point P | |
| ordinate of northward located neighboring point of point P | |
| velocity at the eastward located point E | |
| velocity of general point P | |
| velocity at the westward located point W | |
| velocity at the northward located point N | |
| abscissa of general point P | |
| abscissa of the ordinate of southward located neighboring point of point P | |
| abscissa of the ordinate of northward located neighboring point of point P | |
| grid space along vertical direction | |
| grid space along horizontal direction | |
| value of the function at point | |
| coefficient for the spectral expression for u | |
| coefficient for the spectral expression for u | |
| magnetic parameter | |
| Nusselt number | |
| Reynolds number | |
| reference temperature | |
| hydraulic diameter of the duct | |
| constant peripherally averaged wall heat flux | |
| Greek letters | |
| dimensionless temperature | |
| nanoparticle volume fraction | |
| density for the solid particles | |
| density of the base fluids | |
| density of the nanofluids | |
| electrical diffusivity of the nanofluid | |
| corresponding location of the dipole | |
| aspect ratio of the duct | |
| pressure gradient | |
References
- Galanis, N.; Rashidi, M. Entropy generation in non-Newtonian fluids due to heat and mass transfer in the entrance region of ducts. Heat Mass Transf. 2012, 48, 1647–1662. [Google Scholar] [CrossRef]
- Barletta, A. Parallel and non-parallel laminar mixed convection flow in an inclined tube: The effect of the boundary conditions. Int. J. Heat Fluid Flow 2008, 29, 83–93. [Google Scholar] [CrossRef]
- Umavathi, J.C.; Ojjela, O.; Vajravelu, K. Numerical analysis of natural convective flow and heat transfer of nanofluids in a vertical rectangular duct using Darcy-Forchheimer-Brinkman model. Int. J. Therm. Sci. 2017, 111, 511–524. [Google Scholar] [CrossRef]
- Cheng, C.H.; Weng, C.J.; Aung, W. Buoyancy-assisted flow reversal and convective heat transfer in entrance region of a vertical rectangular duct. Int. J. Heat Fluid Flow 2000, 21, 403–411. [Google Scholar] [CrossRef]
- Barletta, A.; Magyari, E. Forced convection with viscous dissipation in the thermal entrance region of a circular duct with prescribed wall heat flux. Int. J. Heat Mass Transf. 2007, 50, 26–35. [Google Scholar] [CrossRef]
- Barletta, A.; Pulvirenti, B. Forced convection with slug flow and viscous dissipation in a rectangular duct. Int. J. Heat Mass Transf. 2000, 43, 725–740. [Google Scholar] [CrossRef]
- Mousavi, S.M.; Darzi, A.A.R.; Akbari, O.A.; Toghraie, D.; Marzban, A. Numerical study of biomagnetic fluid flow in a duct with a constriction affected by a magnetic field. J. Magn. Magn. Mater. 2019, 473, 42–50. [Google Scholar] [CrossRef]
- Öztop, H.F. Numerical study of flow and heat transfer in curvilinear ducts: Applications of elliptic grid generation. Appl. Math. Comput. 2005, 168, 1449–1460. [Google Scholar] [CrossRef]
- Abdellahoum, C.; Mataoui, A.; Öztop, H.F. Turbulent forced convection of nanofluid over a heated shallow cavity in a duct. Powder Technol. 2015, 277, 126–134. [Google Scholar] [CrossRef]
- Yang, G.; Wu, J.; Yan, L. Flow reversal and entropy generation due to buoyancy assisted mixed convection in the entrance region of a three dimensional vertical rectangular duct. Int. J. Heat Mass Transf. 2013, 67, 741–751. [Google Scholar] [CrossRef]
- Atashafrooz, M. The effects of buoyancy force on mixed convection heat transfer of MHD nanofluid flow and entropy generation in an inclined duct with separation considering Brownian motion effects. J. Therm. Anal. Calorim. 2019, 138, 3109–3126. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Jafaryar, M.; Li, Z. Second law analysis for nanofluid turbulent flow inside a circular duct in presence of twisted tape turbulators. J. Mol. Liq. 2018, 263, 489–500. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Jafaryar, M.; Li, Z. Nanofluid turbulent convective flow in a circular duct with helical turbulators considering CuO nanoparticles. Int. J. Heat Mass Transf. 2018, 124, 980–989. [Google Scholar] [CrossRef]
- Hussain, S.; Öztop, H.F.; Jamal, M.; Hamdeh, N.A. Double diffusive nanofluid flow in a duct with cavity heated from below. Int. J. Mech. Sci. 2017, 131, 535–545. [Google Scholar] [CrossRef]
- Morini, G.; Spiga, M. Transient laminar natural convection along rectangular ducts. Int. J. Heat Mass Transfer. 2001, 44, 4703–4710. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Combined effects of double rotating cones and magnetic field on the mixed convection of nanofluid in a porous 3D U-bend. Int. Commun. Heat Mass Transf. 2020, 116, 104703. [Google Scholar] [CrossRef]
- Ali, K.; Ahmad, S.; Ahmad, S.; Ashraf, M.; Asif, M. On the interaction between the external magnetic field and nanofluid inside a vertical square duct. AIP Adv. 2015, 5, 107120. [Google Scholar] [CrossRef]
- Barletta, A.; di Schio, E.R.; Zanchini, E. Combined forced and free flow in a vertical rectangular duct with prescribed wall heat flux. Int. J. Heat Fluid Flow 2003, 24, 874–887. [Google Scholar] [CrossRef]
- Li, Z.; Sheikholeslami, M.; Mittal, A.S.; Shafee, A.; Haq, R.U. Nanofluid heat transfer in a porous duct in the presence of Lorentz forces using the lattice Boltzmann method. Eur. Phys. J. Plus 2019, 134, 30. [Google Scholar] [CrossRef]
- Ahmad, S.; Cai, J.; Ali, K. Prediction of new vortices in single-phase nanofluid due to dipole interaction. J. Therm. Anal. Calorim. 2022, 147, 461–475. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Modeling and optimization of MHD mixed convection in a lid-driven trapezoidal cavity filled with alumina–water nanofluid: Effects of electrical conductivity models. Int. J. Mech. Sci. 2018, 136, 264–278. [Google Scholar] [CrossRef]
- Zanchini, E. Mixed convection with variable viscosity in a vertical annulus with uniform wall temperatures. Int. J. Heat Mass Transf. 2008, 51, 30–40. [Google Scholar] [CrossRef]
- el Hasadi, Y.M.F.; Busedra, A.A.; Rustum, I.M. Laminar mixed convection in the entrance region of horizontal semicircular ducts with the flat wall at the top. J. Heat Transf. 2007, 129, 1203–1211. [Google Scholar] [CrossRef]
- Barletta, A.; Nield, D. Mixed convection with viscous dissipation and pressure work in a lid-driven square enclosure. Int. J. Heat Mass Transf. 2009, 52, 4244–4253. [Google Scholar] [CrossRef]
- Barletta, A.; Magyari, E.; Lazzari, S.; Pop, I. Mixed convection with heating effects in a vertical porous annulus with a radially varying magnetic field. Int. J. Heat Mass Transf. 2008, 51, 5777–5784. [Google Scholar] [CrossRef]
- Orfi, J.; Galanis, N. Mixed convection with heat and mass transfer in horizontal tubes. Int. Commun. Heat Mass Transf. 2005, 32, 511–519. [Google Scholar] [CrossRef]
- Barletta, A. Fully developed mixed convection and flow reversal in a vertical rectangular duct with uniform wall heat flux. Int. J. Heat Mass Transf. 2002, 45, 641–654. [Google Scholar] [CrossRef]
- Barletta, A. On the existence of parallel flow for mixed convection in an inclined duct. Int. J. Heat Mass Transf. 2005, 48, 2042–2049. [Google Scholar] [CrossRef]
- Geridonmez, B.P.; Öztop, H. MHD natural convection in a cavity in the presence of cross partial magnetic fields and Al2O3-water nanofluid. Comput. Math. Appl. 2020, 80, 2796–2810. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, Y. Experimental study on enhanced heat transfer and flow performance of magnetic nanofluids under alternating magnetic field. Int. J. Therm. Sci. 2021, 164, 106897. [Google Scholar] [CrossRef]
- Umavathi, J.; Öztop, H.F. Investigation of MHD and applied electric field effects in a conduit cramed with nanofluids. Int. Commun. Heat Mass Transf. 2021, 121, 105097. [Google Scholar] [CrossRef]
- Abdellahoum, C.; Mataoui, A.; Öztop, H.F. Comparison of viscosity variation formulations for turbulent flow of Al2O3–water nanofluid over a heated cavity in a duct. Adv. Powder Technol. 2015, 26, 1210–1218. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, Y. Heat transfer and flow characteristics of Fe3O4-water nanofluids under magnetic excitation. Int. J. Therm. Sci. 2021, 163, 106826. [Google Scholar] [CrossRef]
- Ekiciler, R. Effects of novel hybrid nanofluid (TiO2–Cu/EG) and geometrical parameters of triangular rib mounted in a duct on heat transfer and flow characteristics. J. Therm. Anal. Calorim. 2021, 143, 1371–1387. [Google Scholar] [CrossRef]
- Umavathi, J.; Buonomo, B.; Manca, O.; Shereme, M. Double diffusion in a rectangular duct using metals or oxides suspended in a viscous fluid. Therm. Sci. Eng. Prog. 2021, 21, 100793. [Google Scholar] [CrossRef]
- Atashafrooz, M.; Sheikholeslami, M.; Sajjadi, H.; Delouei, A.A. Interaction effects of an inclined magnetic field and nanofluid on forced convection heat transfer and flow irreversibility in a duct with an abrupt contraction. J. Magn. Magn. Mater. 2019, 478, 216–226. [Google Scholar] [CrossRef]
- Mayeli, P.; Hesami, H.; Moghaddam, M.H.D.F. Numerical investigation of the MHD forced convection and entropy generation in a straight duct with sinusoidal walls containing water–Al2O3 nanofluid. Numer. Heat Transf. Part A Appl. 2017, 71, 1235–1250. [Google Scholar] [CrossRef]
- Ahmad, S.; Ali, K.; Ahmad, S.; Cai, J. Numerical Study of Lorentz Force Interaction with Micro Structure in Channel Flow. Energies 2021, 14, 4286. [Google Scholar] [CrossRef]
- Aidaoui, L.; Lasbet, Y.; Selimefendigil, F. Improvement of transfer phenomena rates in open chaotic flow of nanofluid under the effect of magnetic field: Application of a combined method. Int. J. Mech. Sci. 2020, 179, 105649. [Google Scholar] [CrossRef]
- Mandal, D.K.; Biswas, N.; Manna, N.K.; Gorla, R.S.R.; Chamkha, A.J. Magneto-hydrothermal performance of hybrid nanofluid flow through a non-Darcian porous complex wavy enclosure. Eur. Phys. J. Plus 2022, 231, 2695–2712. [Google Scholar] [CrossRef]
- Biswas, N.; Mondal, M.K.; Mandal, D.K.; Manna, N.K.; Gorla, R.S.R.; Chamkha, A.J. A narrative loom of hybrid nanofluid-filled wavy walled tilted porous enclosure imposing a partially active magnetic field. Int. J. Mech. Sci. 2022, 217, 107028. [Google Scholar] [CrossRef]
- Manna, N.K.; Mondal, M.K.; Biswas, N. A novel multi-banding application of magnetic field to convective transport system filled with porous medium and hybrid nanofluid. Phys. Scr. 2021, 96, 065001. [Google Scholar] [CrossRef]
- Mondal, M.K.; Biswas, N.; Datta, A.; Mandal, D.K.; Manna, N.K. Thermofluidic transport phenomena of hybrid nanofluid in a porous wavy enclosure imposing magnetic fields. Mater. Today Proc. 2022, 52, 505–512. [Google Scholar] [CrossRef]
- Afridi, M.I.; Ashraf, M.U.; Qasim, M.; Wakif, A. Numerical simulation of entropy transport in the oscillating fluid flow with transpiration and internal fluid heating by GGDQM. Waves Random Complex Media 2022, 1–19. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M.; Khan, N.A.; Makinde, O.D. Minimization of Entropy Generation in MHD Mixed Convection Flow with Energy Dissipation and Joule Heating: Utilization of Sparrow-Quack-Boerner Local Non-Similarity Method. Defect Diffus. Forum 2018, 387, 63–77. [Google Scholar] [CrossRef]
- Afridi, M.I.; Muhammad, I.; Qasim, M.; Shafie, S.; Makinde, O.D. Entropy generation analysis of spherical and non-spherical Ag-Water nanofluids in a porous medium with magnetic and porous dissipation. J. Nanofluids 2018, 7, 951–960. [Google Scholar] [CrossRef]
- Xuan, Y.; Roetzel, W. Conceptions for heat transfer correlation of nanofluids. Int. J. Heat Mass Transf. 2000, 43, 3701–3707. [Google Scholar] [CrossRef]
- Ali, K.; Ahmad, S.; Baluch, O.; Jamshed, W.; Mohamed, R.; Eid, M.R.; Pasha, A.A. Numerical study of magnetic field interaction with fully developed flow in a vertical duct. Alex. Eng. J. 2022, 61, 11351–11363. [Google Scholar] [CrossRef]
- Jamshed, W.; Aziz, A. Entropy Analysis of TiO2-Cu/EG Casson Hybrid Nanofluid via Cattaneo-Christov Heat Flux Model. Appl. Nanosci. 2018, 8, 1–14. [Google Scholar]
- Rasool, G.; Saeed, A.M.; Lare, A.I.; Abderrahmane, A.; Guedri, K.; Vaidya, H. Darcy-Forchheimer Flow of Water Conveying Multi-Walled Carbon Nanoparticles through a Vertical Cleveland Z-Staggered Cavity Subject to Entropy Generation. Micromachines 2022, 13, 744. [Google Scholar] [CrossRef]
- Shafiq, A.; Mebarek-Oudina, F.; Sindhu, T.N.; Rasool, G. Sensitivity analysis for Walters-B nanoliquid flow over a radiative Riga surface by RSM. Sci. Iran. 2022, 29, 1236–1249. [Google Scholar]
- Batool, S.; Rasool, G.; Alshammari, N.; Khan, I.; Kaneez, H.; Hamadneh, N. Numerical analysis of heat and mass transfer in micropolar nanofluids flow through lid driven cavity: Finite volume approach. Case Stud. Therm. Eng. 2022, 37, 102233. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Alqarni, M.S.; Wakif, A.; Khan, I.; Bhutta, M.S. Numerical Scrutinization of Darcy-Forchheimer Relation in Convective Magnetohydrodynamic Nanofluid Flow Bounded by Nonlinear Stretching Surface in the Perspective of Heat and Mass Transfer. Micromachines 2021, 12, 374. [Google Scholar] [CrossRef] [PubMed]
- Jamshed, W.; Nisar, K.S. Computational single phase comparative study of Williamson nanofluid in parabolic trough solar collector via Keller box method. Int. J. Energy Res. 2021, 45, 10696–10718. [Google Scholar] [CrossRef]
- Jamshed, W.; Devi, S.U.; Nisar, K.S. Single phase-based study of Ag-Cu/EO Williamson hybrid nanofluid flow over a stretching surface with shape factor. Phys. Scr. 2021, 96, 065202. [Google Scholar] [CrossRef]
- Jamshed, W.; Nisar, K.S.; Ibrahim, R.W.; Shahzad, F.; Eid, M.R. Thermal expansion optimization in solar aircraft using tangent hyperbolic hybrid nanofluid: A solar thermal application. J. Mater. Res. Technol. 2021, 14, 985–1006. [Google Scholar] [CrossRef]
- Jamshed, W.; Nisar, K.S.; Ibrahim, R.W.; Mukhtar, T.; Vijayakumar, V.; Ahmad, F. Computational frame work of Cattaneo-Christov heat flux effects on Engine Oil based Williamson hybrid nanofluids: A thermal case study. Case Stud. Therm. Eng. 2021, 26, 101179. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Hussain, S.; Zaydan, M.; Wakif, A.; Chamkha, A.J.; Bhutta, M.S. Significance of Rosseland’s Radiative Process on Reactive Maxwell Nanofluid Flows over an Isothermally Heated Stretching Sheet in the Presence of Darcy-Forchheimer and Lorentz Forces: Towards a New Perspective on Buongiorno’s Model. Micromachines 2022, 13, 368. [Google Scholar] [CrossRef]
- Pasha, A.; Islam, N.; Jamshed, W.; Alam, M.I.; Jameel, A.G.A.; Juhany, K.A.; Alsulami, R. Statistical analysis of viscous hybridized nanofluid flowing via Galerkin finite element technique. Int. Commun. Heat Mass Transf. 2022, 137, 106244. [Google Scholar] [CrossRef]
- Zari, I.; Shafiq, A.; Rasool, G.; Sindhu, T.N.; Khan, T.S. Double-stratified Marangoni boundary layer flow of Casson nanoliquid: Probable error application. J. Thermal Anal. Calorim. 2022, 147, 6913–6929. [Google Scholar] [CrossRef]
- Hussain, S.M.; Jamshed, W.; Pasha, A.A.; Adil, M.; Akram, M. Galerkin finite element solution for electromagnetic radiative impact on viscid Williamson two-phase nanofluid flow via extendable surface. Int. Commun. Heat Mass Transf. 2022, 137, 106243. [Google Scholar] [CrossRef]
- Rasool, G.; Shafiq, A.; Khan, I.; Baleanu, D.; Sooppy Nisar, K.; Shahzadi, G. Entropy Generation and Consequences of MHD in Darcy–Forchheimer Nanofluid Flow Bounded by Non-Linearly Stretching Surface. Symmetry 2020, 12, 652. [Google Scholar] [CrossRef]
- Sajid, T.; Ayub, A.; Shah, S.Z.H.; Jamshed, W.; Eid, M.R.; el Din, E.S.M.T.; Irfan, R.; Hussain, S.M. Trace of Chemical Reactions Accompanied with Arrhenius Energy on Ternary Hybridity Nanofluid Past a Wedge. Symmetry 2022, 14, 1850. [Google Scholar] [CrossRef]
- Sajid, T.; Jamshed, W.; Shahzad, F.; Ullah, I.; Ibrahim, R.W.; Eid, M.R.; Arshad, M.; Khalifa, H.A.E.; Alharbi, S.K.; el Din, M.E.S.T. Insightful into dynamics of magneto Reiner-Philippoff nanofluid flow induced by triple-diffusive convection with zero nanoparticle mass flux. Ain Shams Eng. J. 2022, 6, 101946. [Google Scholar] [CrossRef]
- Jafar, A.B.; Shafie, S.; Ullah, I.; Safdar, R.; Jamshed, W.; Pasha, A.A.; Rahman, M.M.; Hussain, S.M.; Rehman, A.; el Din, E.S.M.T.; et al. Mixed Convection Flow of an Electrically Conducting Viscoelastic Fluid past a Vertical Nonlinearly Stretching Sheet. Sci. Rep. 2022, 12, 14679. [Google Scholar] [CrossRef]
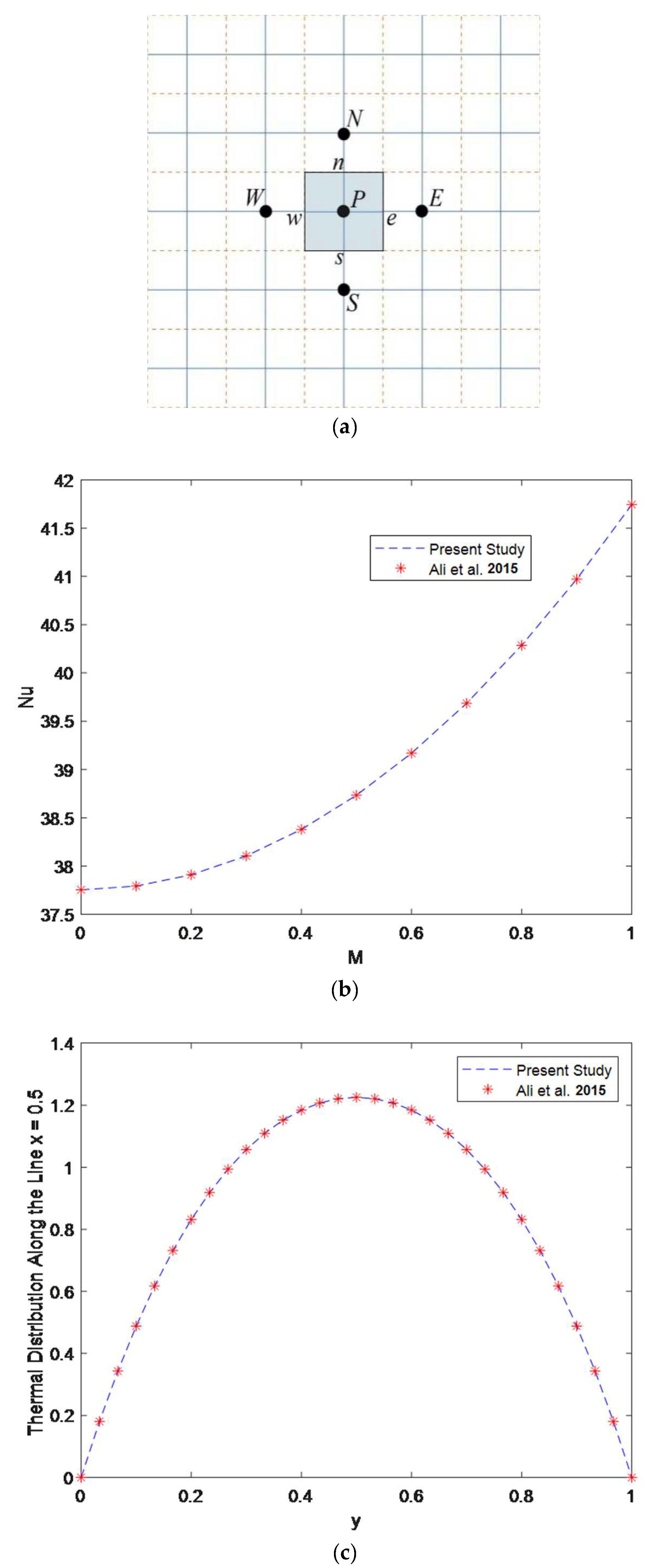
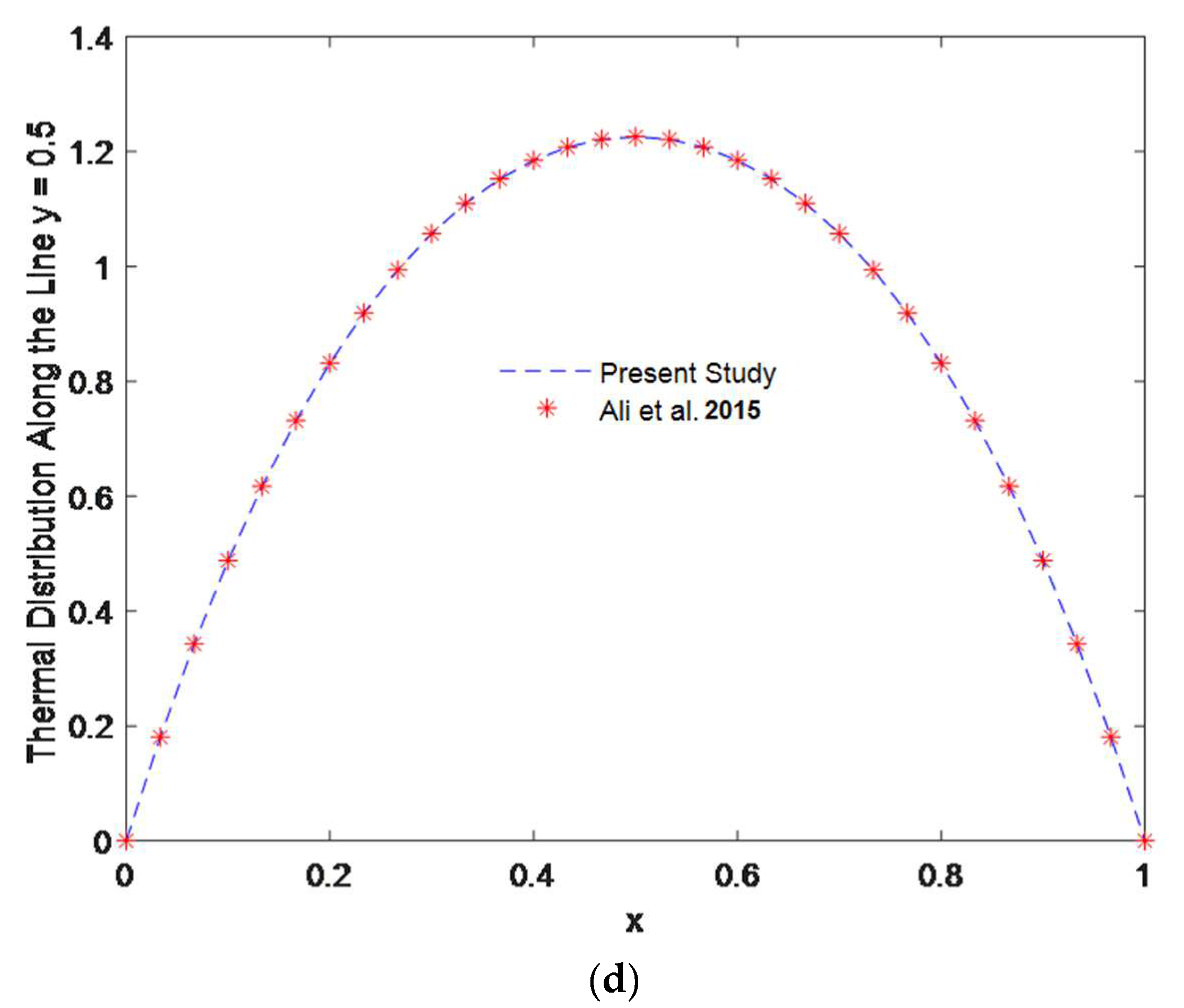
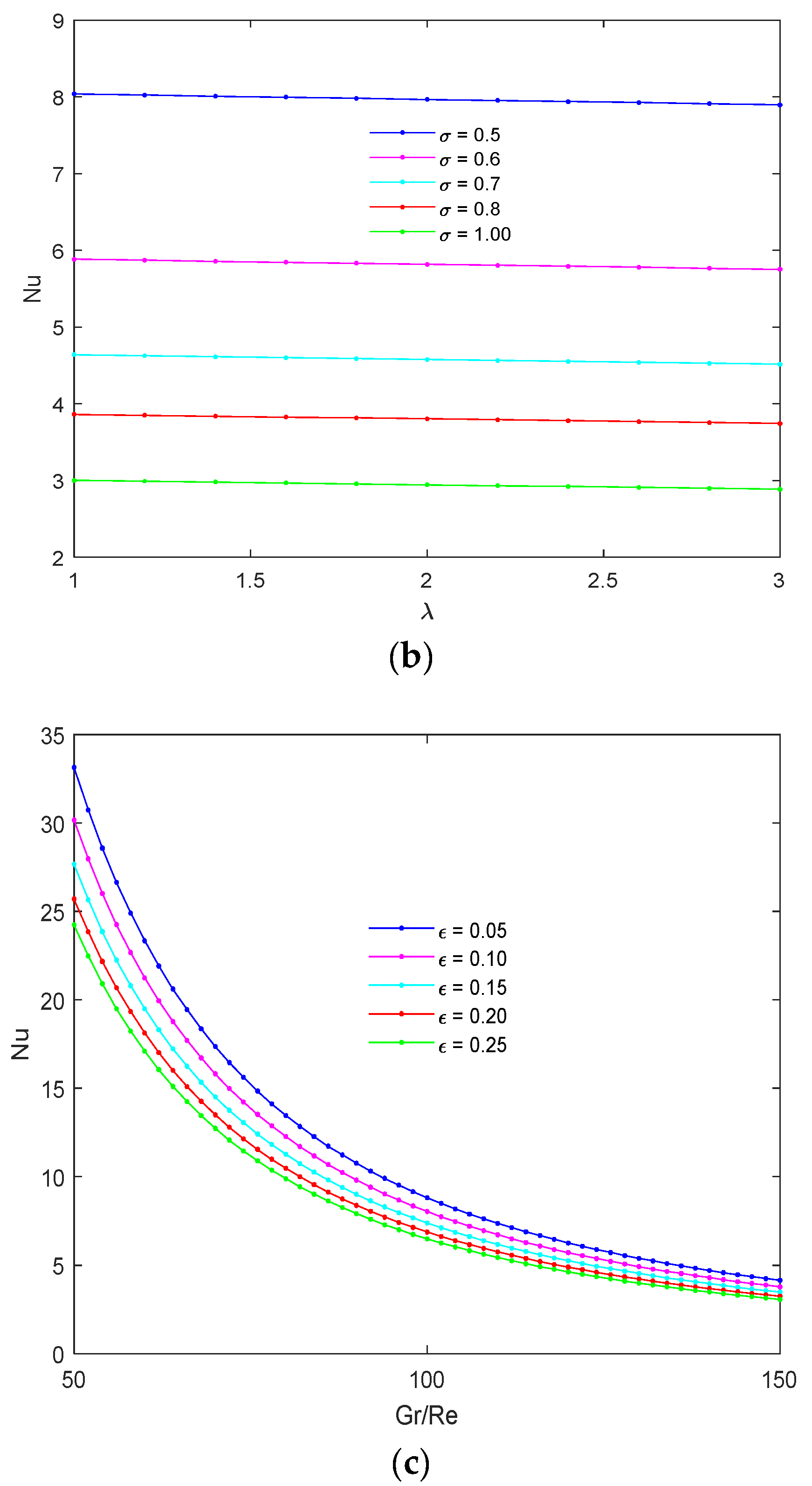


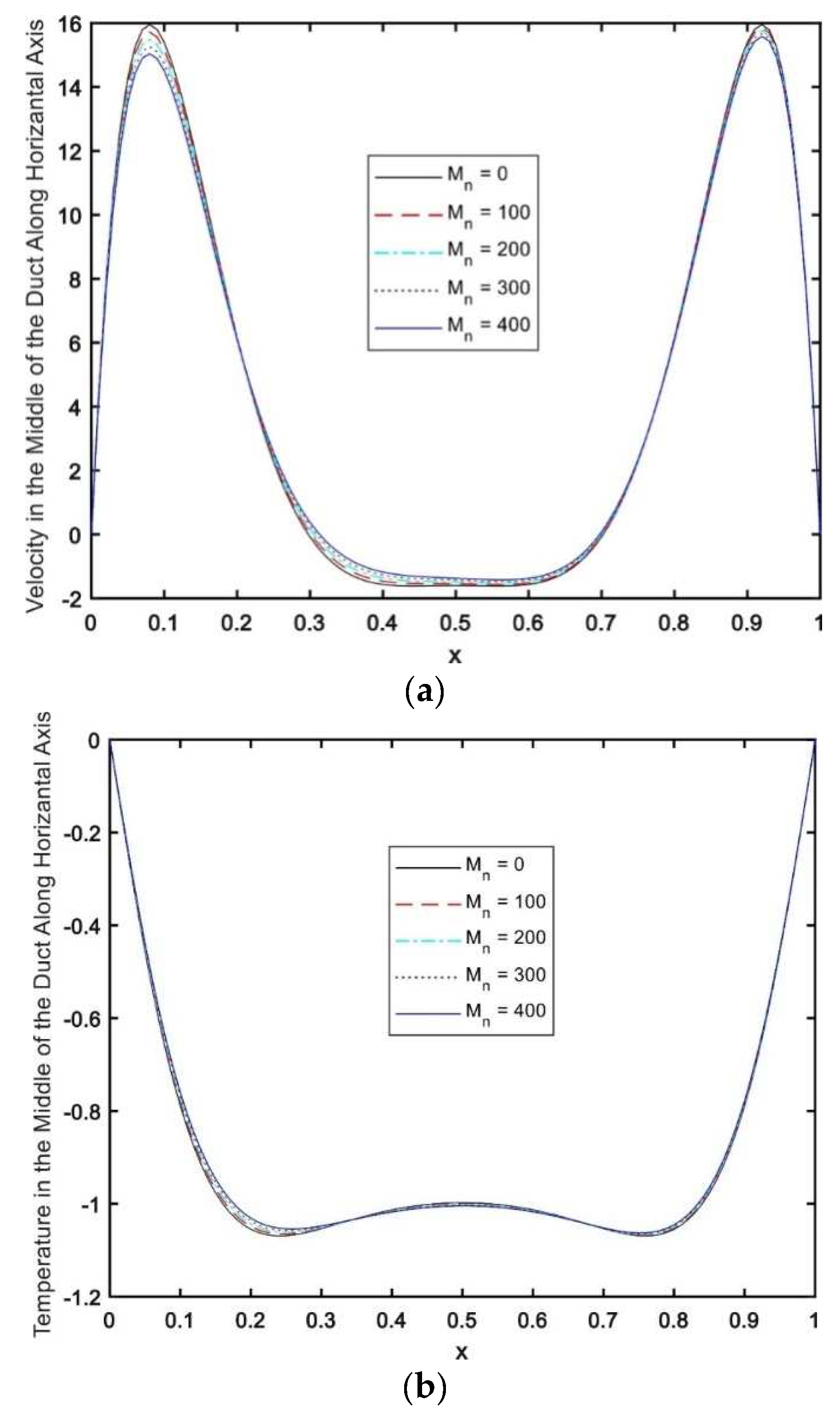
| Gr/Re | Nu (Present Method) | Nu (Spectral Method) |
|---|---|---|
| 1 | 1.1746 × 104 | 1.1745 × 104 |
| 10 | 1.2292 × 104 | 1.2291 × 104 |
| 100 | 1.8436 × 104 | 1.8433 × 104 |
| 1000 | 1.4612 × 104 | 1.4608 × 104 |
| 5000 | 1.9351 × 104 | 1.9343 × 104 |
| Thermo-Physical | ||||
|---|---|---|---|---|
| Water (H2O) | 997.1 | 4179 | 0.613 | 21 × 10−5 |
| Silver (Ag) | 10500 | 235 | 429 | 1.89 × 10−5 |
| Nu | |||||
|---|---|---|---|---|---|
| M = 0 | M = 50 | M = 100 | M = 150 | M = 200 | |
| 0.2 | 18.7016 | 19.8204 | 20.9379 | 22.0550 | 23.1728 |
| 0.4 | 1.2432 | 1.4441 | 1.6535 | 1.8710 | 2.0967 |
| 0.6 | 0.3056 | 0.3824 | 0.4654 | 0.5546 | 0.6497 |
| 0.8 | 0.1342 | 0.1753 | 0.2207 | 0.2701 | 0.3235 |
| 1.0 | 0.0808 | 0.1073 | 0.1367 | 0.1688 | 0.2037 |
| σ | Nu | ||||
|---|---|---|---|---|---|
| M = 0 | M = 50 | M = 100 | M = 150 | M = 200 | |
| 0.2 | 1.2608 | 1.3143 | 1.3677 | 1.4208 | 1.4737 |
| 0.4 | 0.5254 | 0.5767 | 0.6289 | 0.6819 | 0.7359 |
| 0.6 | 0.2800 | 0.3181 | 0.3576 | 0.3984 | 0.4406 |
| 0.8 | 0.1804 | 0.2088 | 0.2384 | 0.2693 | 0.3014 |
| 1.0 | 0.1318 | 0.1537 | 0.1766 | 0.2005 | 0.2254 |
| Nu | |||||
|---|---|---|---|---|---|
| M = 0 | M = 50 | M = 100 | M = 150 | M = 200 | |
| −100 | 2.3919 | 4.6080 | 7.4516 | 10.8838 | 14.8753 |
| −50 | 1.1507 | 1.5449 | 1.9836 | 2.4648 | 2.9868 |
| 0 | 0.5583 | 0.6756 | 0.8004 | 0.9325 | 1.0717 |
| 50 | 0.4289 | 0.4940 | 0.5619 | 0.6323 | 0.7053 |
| 100 | 0.3729 | 0.4173 | 0.4630 | 0.5099 | 0.5581 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, K.; Ahmad, S.; Ahmad, S.; Jamshed, W.; Hussain, S.M.; Tag El Din, E.S.M. Molecular Interaction and Magnetic Dipole Effects on Fully Developed Nanofluid Flowing via a Vertical Duct Applying Finite Volume Methodology. Symmetry 2022, 14, 2007. https://doi.org/10.3390/sym14102007
Ali K, Ahmad S, Ahmad S, Jamshed W, Hussain SM, Tag El Din ESM. Molecular Interaction and Magnetic Dipole Effects on Fully Developed Nanofluid Flowing via a Vertical Duct Applying Finite Volume Methodology. Symmetry. 2022; 14(10):2007. https://doi.org/10.3390/sym14102007
Chicago/Turabian StyleAli, Kashif, Shabbir Ahmad, Sohail Ahmad, Wasim Jamshed, Syed M. Hussain, and El Sayed M. Tag El Din. 2022. "Molecular Interaction and Magnetic Dipole Effects on Fully Developed Nanofluid Flowing via a Vertical Duct Applying Finite Volume Methodology" Symmetry 14, no. 10: 2007. https://doi.org/10.3390/sym14102007
APA StyleAli, K., Ahmad, S., Ahmad, S., Jamshed, W., Hussain, S. M., & Tag El Din, E. S. M. (2022). Molecular Interaction and Magnetic Dipole Effects on Fully Developed Nanofluid Flowing via a Vertical Duct Applying Finite Volume Methodology. Symmetry, 14(10), 2007. https://doi.org/10.3390/sym14102007







