A Nonlinear Five-Term System: Symmetry, Chaos, and Prediction
Abstract
1. Introduction
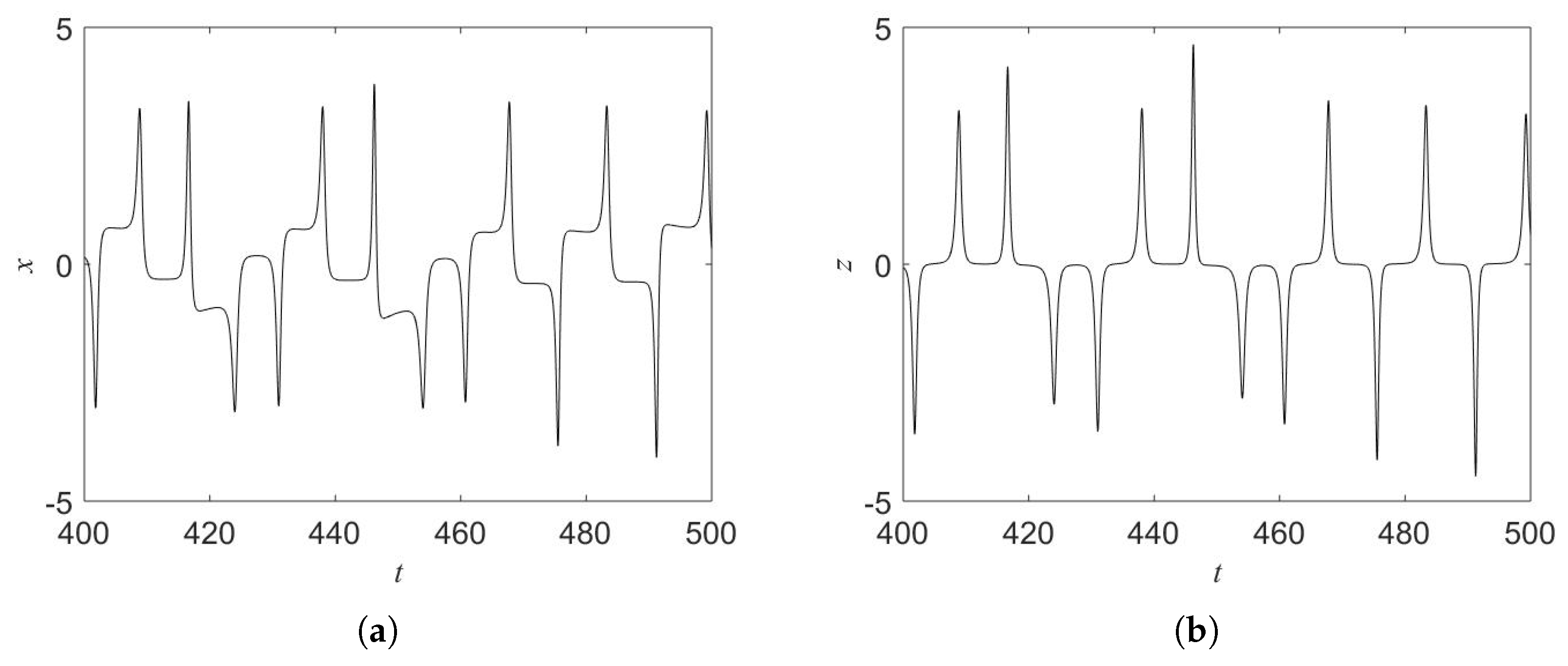
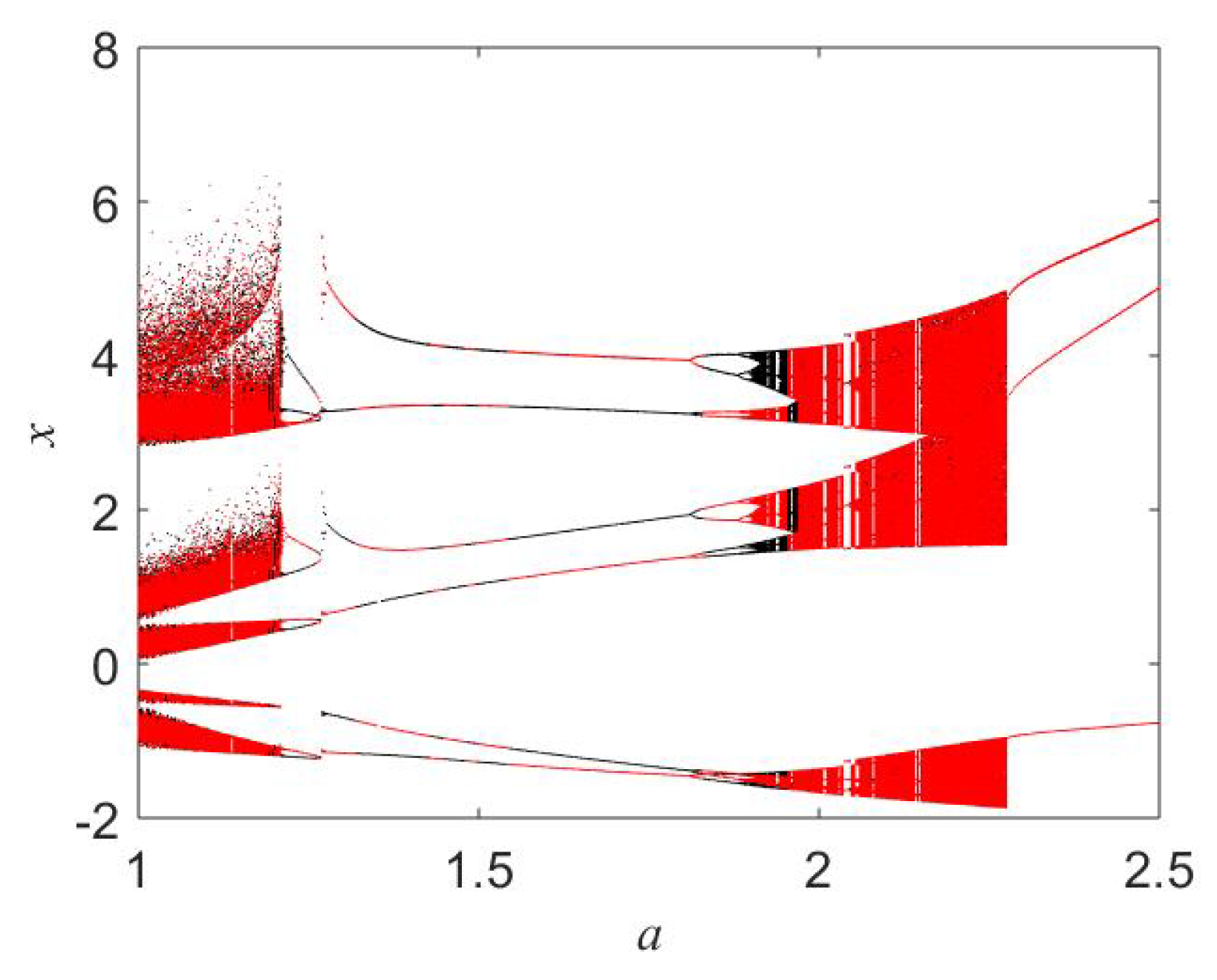
2. System without Linearity
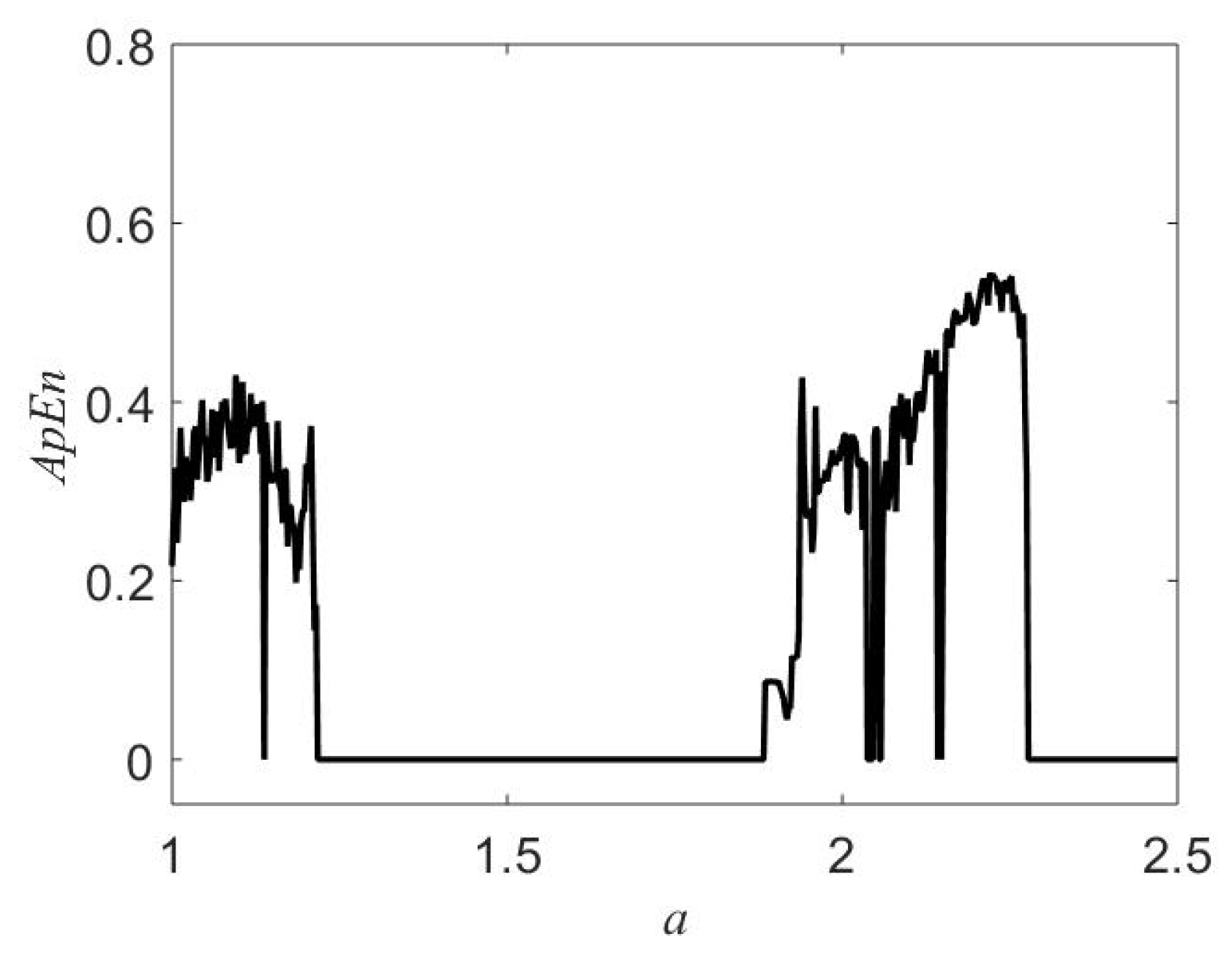
3. System’s Entropy
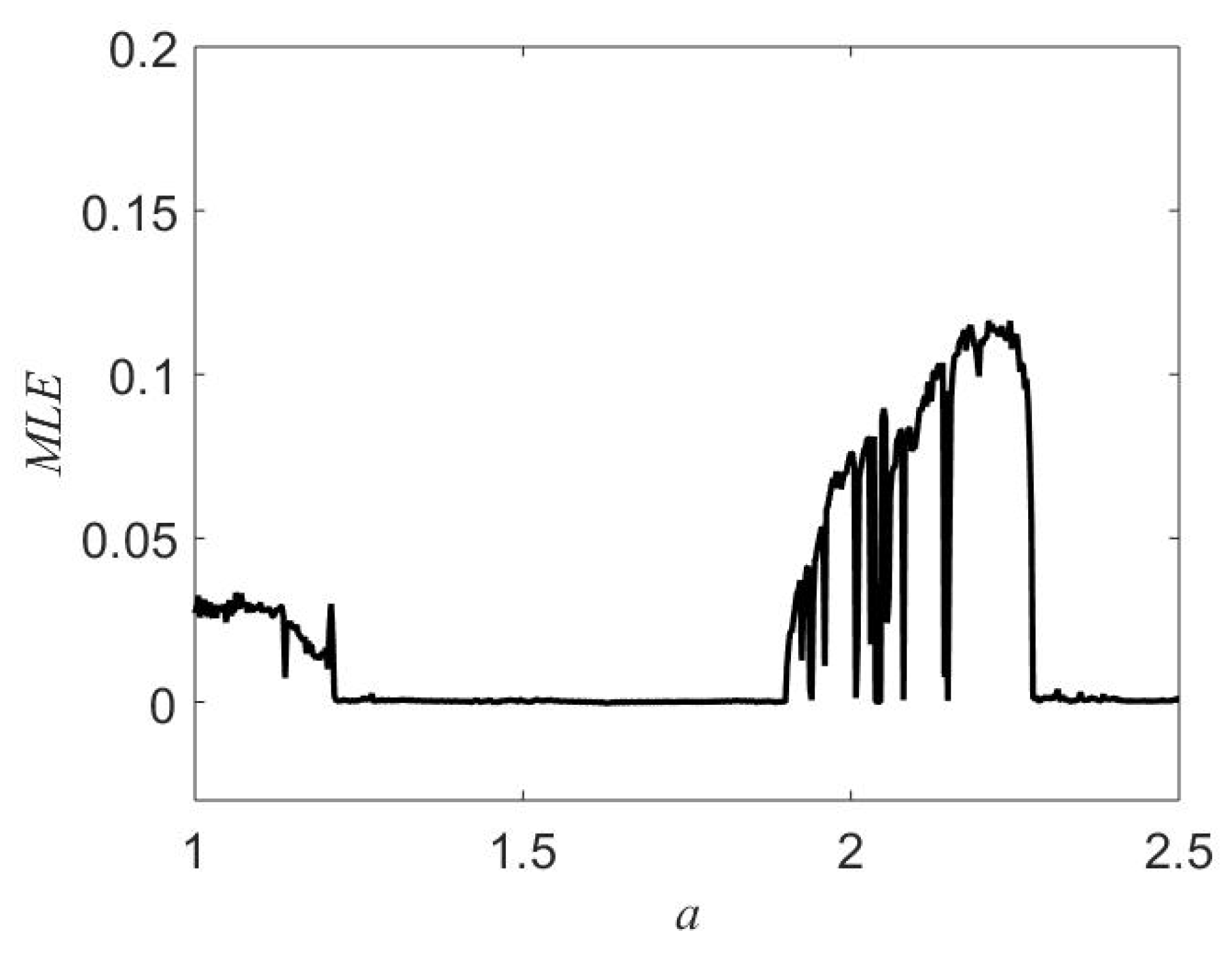
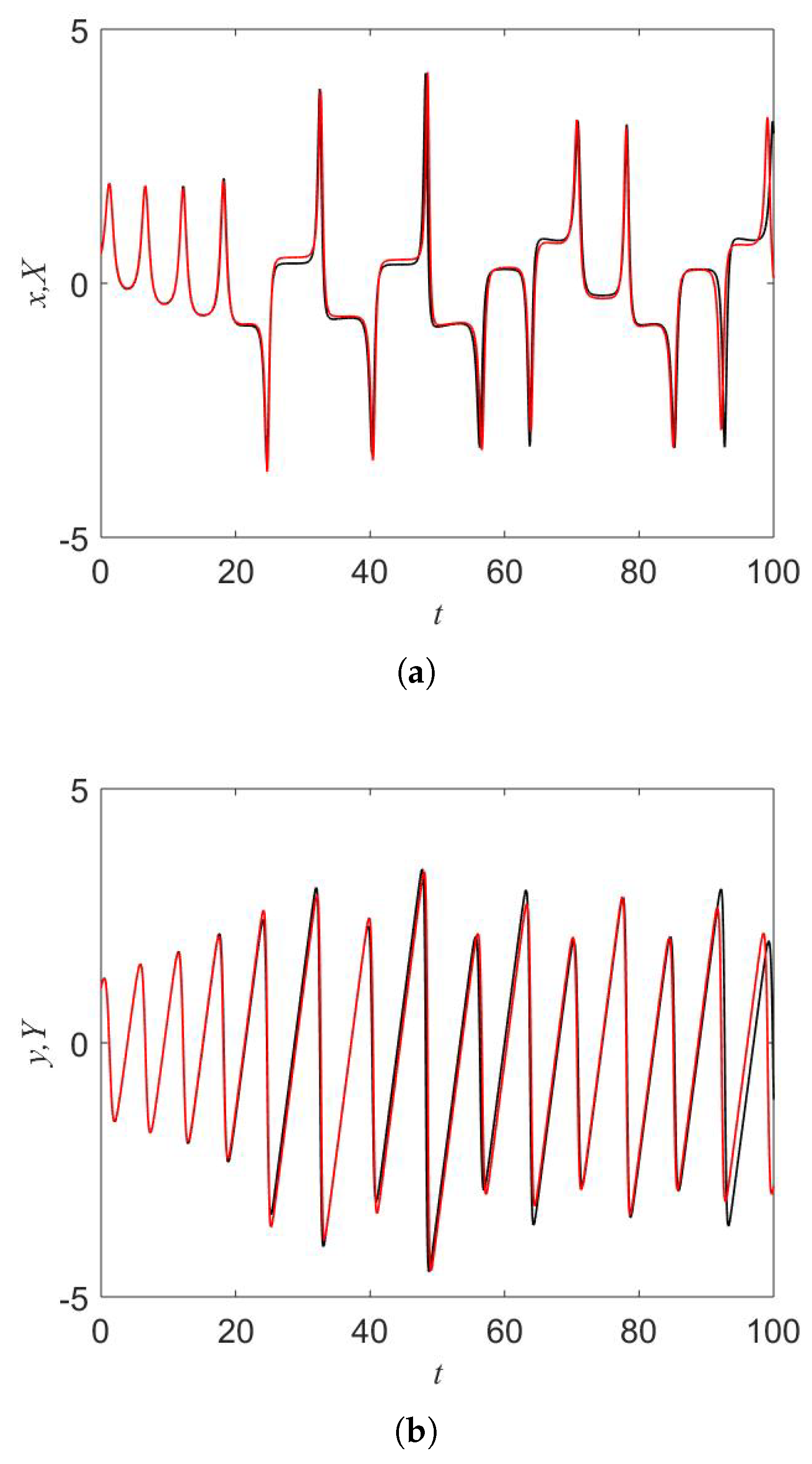
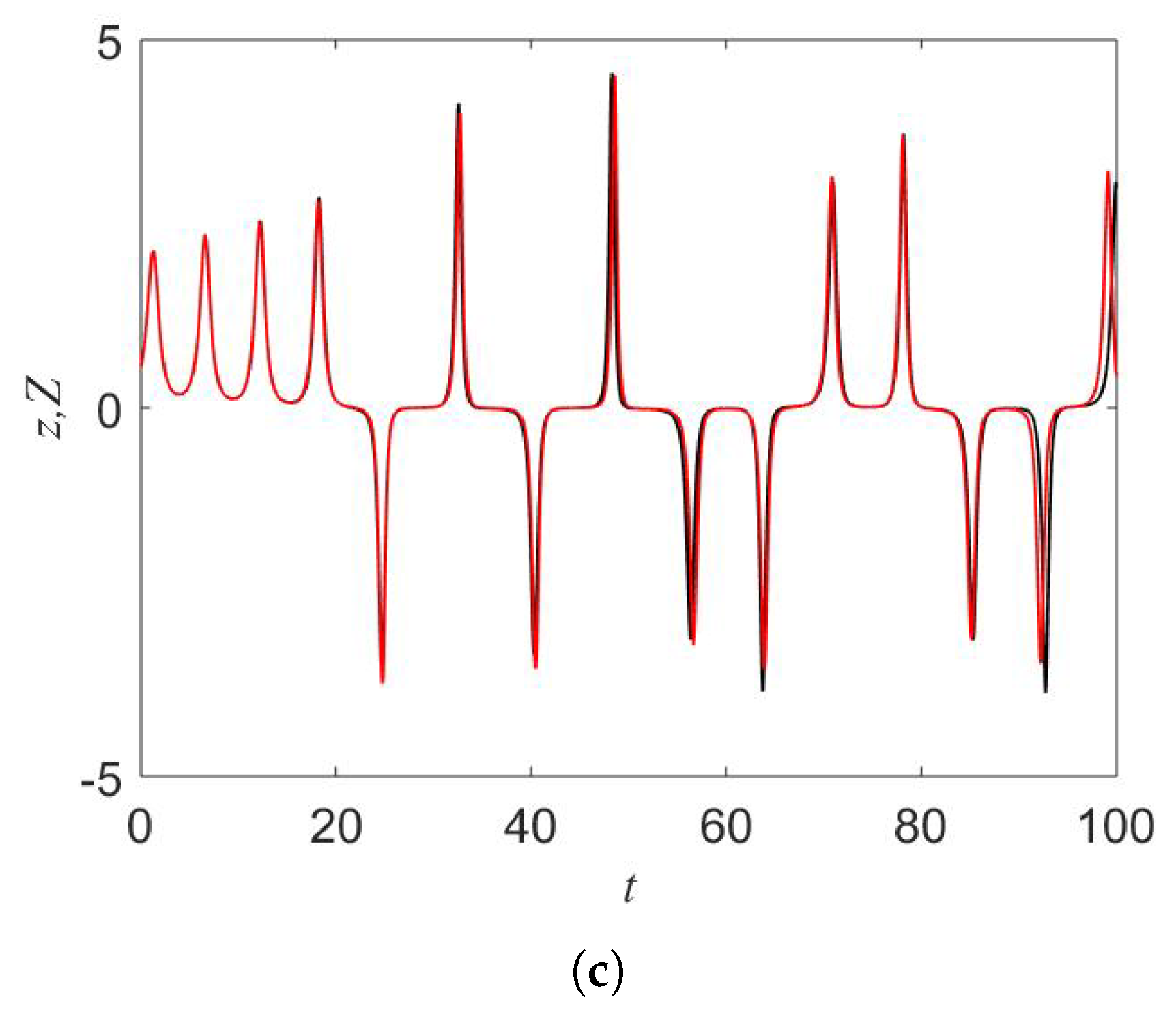
4. Chaos Prediction
5. Conclusions
Author Contributions
Conflicts of Interest
References
- Song, Y.; Yuan, F.; Li, Y. Coexisting attractors and multistability in a simple memristive Wien-Bridge chaotic circuit. Entropy 2019, 21, 678. [Google Scholar] [CrossRef]
- Azar, A.T.; Serrano, F.E. Stabilization of port Hamiltonian chaotic systems with hidden attractors by adaptive terminal sliding mode control. Entropy 2020, 22, 122. [Google Scholar] [CrossRef]
- Liu, L.; Du, C.; Liang, L.; Zhang, X. A high Spectral Entropy (SE) memristive hidden chaotic system with multi-type quasi-periodic and its circuit. Entropy 2019, 21, 1026. [Google Scholar] [CrossRef]
- Danca, M.F. Puu system of fractional order and its chaos suppression. Symmetry 2020, 12, 340. [Google Scholar] [CrossRef]
- Askar, S.S.; Al-khedhairi, A. Dynamic effects arise due to consumers’ preferences depending on past choices. Entropy 2020, 22, 173. [Google Scholar] [CrossRef]
- Ouannas, A.; Khennaoui, A.A.; Bendoukha, S.; Vo, T.P.; Pham, V.T.; Huynh, V.V. The fractional form of the Tinkerbell map is chaotic. Appl. Sci. 2018, 8, 2640. [Google Scholar] [CrossRef]
- Huynh, V.V.; Ouannas, A.; Wang, X.; Pham, V.T.; Nguyen, X.Q.; Alsaadi, F.E. Chaotic map with no fixed points: Entropy, implementation and control. Entropy 2019, 21, 279. [Google Scholar] [CrossRef]
- Ouannas, A.; Khennaoui, A.A.; Momani, S.; Grassi, G.; Pham, V.T. Chaos and control of a three-dimensional fractional order discrete-time system with no equilibrium and its synchronization. AIP Adv. 2020, 10, 045310. [Google Scholar] [CrossRef]
- Chen, L.; Nazarimehr, F.; Jafari, S.; Tlelo-Cuautle, E.; Hussain, I. Investigation of early warning indexes in a three-dimensional chaotic system with zero eigenvalues. Entropy 2020, 22, 341. [Google Scholar] [CrossRef]
- Wang, S.; Yousefpour, A.; Yusuf, A.; Jahanshahi, H.; Alcaraz, R.; He, S.; Munoz-Pacheco, J.M. Synchronization of a non-equilibrium four-dimensional chaotic system using a disturbance-observer-based adaptive terminal sliding mode control method. Entropy 2020, 22, 271. [Google Scholar] [CrossRef]
- Farhan, A.K.; Al-Saidi, N.M.; Maolood, A.T.; Nazarimehr, F.; Hussain, I. Entropy analysis and image encryption application based on a new chaotic system crossing a cylinder. Entropy 2019, 21, 958. [Google Scholar] [CrossRef]
- Xie, Y.; Yu, J.; Chen, X.; Ding, Q.; Wang, E. Low-element image restoration based on an out-of-order elimination algorithm. Entropy 2020, 22, 1192. [Google Scholar] [CrossRef]
- Petrzela, J. Fractional-order chaotic memory with wideband constant phase elements. Entropy 2020, 22, 422. [Google Scholar] [CrossRef]
- Xie, Y.; Yu, J.; Guo, S.; Ding, Q.; Wang, E. Image encryption scheme with compressed sensing based on new three-dimensional chaotic system. Entropy 2019, 21, 819. [Google Scholar] [CrossRef]
- Yu, J.; Guo, S.; Song, X.; Xie, Y.; Wang, E. Image parallel encryption technology based on sequence generator and chaotic measurement matrix. Entropy 2020, 22, 76. [Google Scholar] [CrossRef]
- Wang, X.; Akgul, A.; Cavusoglu, U.; Pham, V.T.; Hoang, D.V.; Nguyen, X.Q. A chaotic system with infinite equilibria and its S-Box constructing application. Appl. Sci. 2018, 8, 2132. [Google Scholar] [CrossRef]
- Wang, X.; Cavusoglu, U.; Kacar, S.; Akgul, A.; Pham, V.T.; Jafari, S.; Alsaadi, F.E.; Nguyen, X.Q. S-Box based image encryption application using a chaotic system without equilibrium. Appl. Sci. 2019, 9, 781. [Google Scholar] [CrossRef]
- Ouannas, A.; Debbouche, N.; Wang, X.; Pham, V.T.; Zehrour, O. Secure Multiple-Input Multiple-Output communications based on F-M synchronization of fractional-order chaotic systems with non-identical dimensions and orders. Appl. Sci. 2018, 8, 1746. [Google Scholar] [CrossRef]
- Zhang, X.; Li, C.; Lei, T.; Liu, Z.; Tao, C. A symmetric controllable hyperchaotic hidden attractor. Symmetry 2020, 12, 550. [Google Scholar] [CrossRef]
- Zhu, X.; Du, W.S. New chaotic systems with two closed curve equilibrium passing the same point: Chaotic behavior, bifurcations, and synchronization. Symmetry 2019, 11, 951. [Google Scholar] [CrossRef]
- Munoz-Pacheco, J.M.; García-Chávez, T.; Gonzalez-Diaz, V.R.; de La Fuente-Cortes, G.; del Carmen Gómez-Pavón, L. Two new asymmetric Boolean chaos oscillators with no dependence on incommensurate time-delays and their circuit implementation. Symmetry 2020, 12, 506. [Google Scholar] [CrossRef]
- Li, C.; Sun, J.; Lu, T.; Lei, T. Symmetry evolution in chaotic system. Symmetry 2020, 12, 574. [Google Scholar] [CrossRef]
- Artuǧer, F.; Özkaynak, F. A novel method for performance improvement of chaos-based substitution Boxes. Symmetry 2020, 12, 571. [Google Scholar] [CrossRef]
- Zhang, G.; Ding, W.; Li, L. Image encryption algorithm based on tent delay-sine cascade with logistic map. Symmetry 2020, 12, 355. [Google Scholar] [CrossRef]
- Stoyanov, B.; Nedzhibov, G. Symmetric key encryption based on rotation-translation equation. Symmetry 2020, 12, 73. [Google Scholar] [CrossRef]
- Sprott, J.C. Elegant Chaos Algebraically Simple Chaotic Flows; World Scientific: Singapore, 2010. [Google Scholar]
- Mobayen, S.; Kingni, S.T.; Pham, V.T.; Nazarimehr, F.; Jafari, S. Analysis, synchronisation and circuit design of a new highly nonlinear chaotic system. Int. J. Syst. Sci. 2018, 49, 617–630. [Google Scholar] [CrossRef]
- Xu, G.; Shekofteh, Y.; Akgul, A.; Li, C.; Panahi, S. A new chaotic system with a self-excited attractor: Entropy measurement, signal encryption, and parameter estimation. Entropy 2018, 20, 86. [Google Scholar] [CrossRef]
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Arena, P.; Caponetto, R.; Fortuna, L.; Manganaro, G. Cellular neural networks to explore complexity. Soft Comput. 1997, 1, 120–136. [Google Scholar] [CrossRef]
- Volos, C.K.; Jafari, S.; Kengne, J.; Munoz-Pacheco, J.M.; Rajagopal, K. Nonlinear dynamics and entropy of complex systems with hidden and self-excited attractors. Entropy 2019, 21, 370. [Google Scholar] [CrossRef]
- Liu, L.; Du, C.; Zhang, X.; Li, J.; Shi, S. Dynamics and entropy analysis for a new 4-D hyperchaotic system with coexisting hidden attractors. Entropy 2019, 21, 287. [Google Scholar] [CrossRef]
- Pincus, S.M. Approximate entropy as a measure of system complexity. Proc. Natl. Acad. Sci. USA 1991, 88, 2297–2301. [Google Scholar] [CrossRef] [PubMed]
- Lytton, W.W. From Computer to Brain: Foundations of Computational Neuroscience; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Haykin, S.O. Neural Networks and Learning Machines; Pearson: London, UK, 2008. [Google Scholar]
- Fausett, L.V. Fundamentals of Neural Networks: Architectures, Algorithms And Applications; Pearson: London, UK, 1993. [Google Scholar]
- Aggarwal, C.C. Neural Networks and Deep Learning: A Textbook; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Jaeger, H.; Haas, H. Harnessing nonlinearity: Predicting chaotic systems and saving energy in wireless communication. Science 2004, 304, 78–80. [Google Scholar] [CrossRef] [PubMed]
- Du, C.; Cai, F.; Zidan, M.A.; Ma, W.; Lee, S.H.; Lu, W.D. Reservoir computing using dynamic memristors for temporal information processing. Nat. Commun. 2017, 8, 2204. [Google Scholar] [CrossRef] [PubMed]
- Moon, J.; Ma, W.; Shin, J.H.; Cai, F.; Du, C.; Lee, S.H.; Lu, W.D. Temporal data classification and forecasting using a memristor-based reservoir computing system. Nat. Electron. 2019, 2, 480–487. [Google Scholar] [CrossRef]
- Goodfellow, I.; Bengio, Y.; Courville, A. Deep Learning; MIT Press: Cambridge, MA, USA, 2016. [Google Scholar]
- Du, K.L.; Swamy, M.N.S. Neural Networks and Statistical Learning; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Woolley, J.W.; Agarwal, P.K.; Baker, J. Modeling and prediction of chaotic systems with artificial neural networks. Int. J. Numer. Methods Fluids 2010, 63, 989–1004. [Google Scholar] [CrossRef]
- Lamamra, K.; Vaidyanathan, S.; Azar, A.T.; Salah, C.B. Chaotic System Modelling Using a Neural Network with Optimized Structure. In Fractional Order Control and Synchronization of Chaotic Systems; Azar, A.T., Vaidyanathan, S., Ouannas, A., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 833–856. [Google Scholar]
- Lellep, M.; Prexl, J.; Linkmann, M.; Eckhardt, B. Using machine learning to predict extreme events in the Hénon map. Chaos 2019, 30, 013113. [Google Scholar] [CrossRef]
- McDonough, J.M. Three-dimensional poor man’s Navier-Stokes equation: A discrete dynamical system exhibiting k−5/3 inertial subrange energy scaling. Phys. Rev. E 2009, 79, 065302. [Google Scholar] [CrossRef]
- Alberti, T.; Consolini, G.; Carbone, V. A discrete dynamical system: The poor man’s magnetohydrodynamic (PMMHD) equations. Chaos 2019, 29, 103107. [Google Scholar] [CrossRef]






| System | Linear Term | Nonlinear Term | Total Term |
|---|---|---|---|
| [17] | 6 | 4 | 10 |
| [1] | 6 | 2 | 8 |
| [20] | 1 | 7 | 8 |
| [27] | 0 | 8 | 8 |
| [16] | 3 | 4 | 7 |
| [28] | 2 | 5 | 7 |
| [11] | 2 | 4 | 6 |
| [9] | 2 | 3 | 5 |
| This work | 0 | 5 | 5 |
| Cases | a | ApEn |
|---|---|---|
| 1 | 1 | 0.2162 |
| 2 | 1.5 | |
| 3 | 2 | 0.3526 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thoai, V.P.; Kahkeshi, M.S.; Huynh, V.V.; Ouannas, A.; Pham, V.-T. A Nonlinear Five-Term System: Symmetry, Chaos, and Prediction. Symmetry 2020, 12, 865. https://doi.org/10.3390/sym12050865
Thoai VP, Kahkeshi MS, Huynh VV, Ouannas A, Pham V-T. A Nonlinear Five-Term System: Symmetry, Chaos, and Prediction. Symmetry. 2020; 12(5):865. https://doi.org/10.3390/sym12050865
Chicago/Turabian StyleThoai, Vo Phu, Maryam Shahriari Kahkeshi, Van Van Huynh, Adel Ouannas, and Viet-Thanh Pham. 2020. "A Nonlinear Five-Term System: Symmetry, Chaos, and Prediction" Symmetry 12, no. 5: 865. https://doi.org/10.3390/sym12050865
APA StyleThoai, V. P., Kahkeshi, M. S., Huynh, V. V., Ouannas, A., & Pham, V.-T. (2020). A Nonlinear Five-Term System: Symmetry, Chaos, and Prediction. Symmetry, 12(5), 865. https://doi.org/10.3390/sym12050865




