BDS Orbit Maneuver Detection Based on Epoch-Updated Orbits Estimated by SRIF
Abstract
1. Introduction
2. Mathematical Model
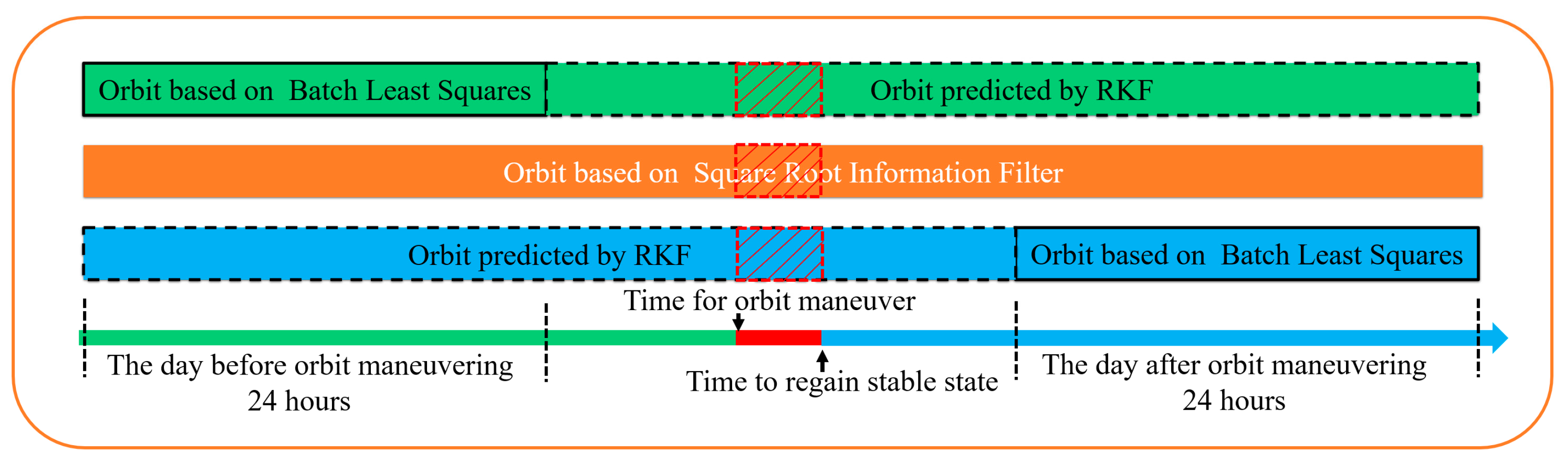
2.1. Epoch-Updated Orbit Estimation
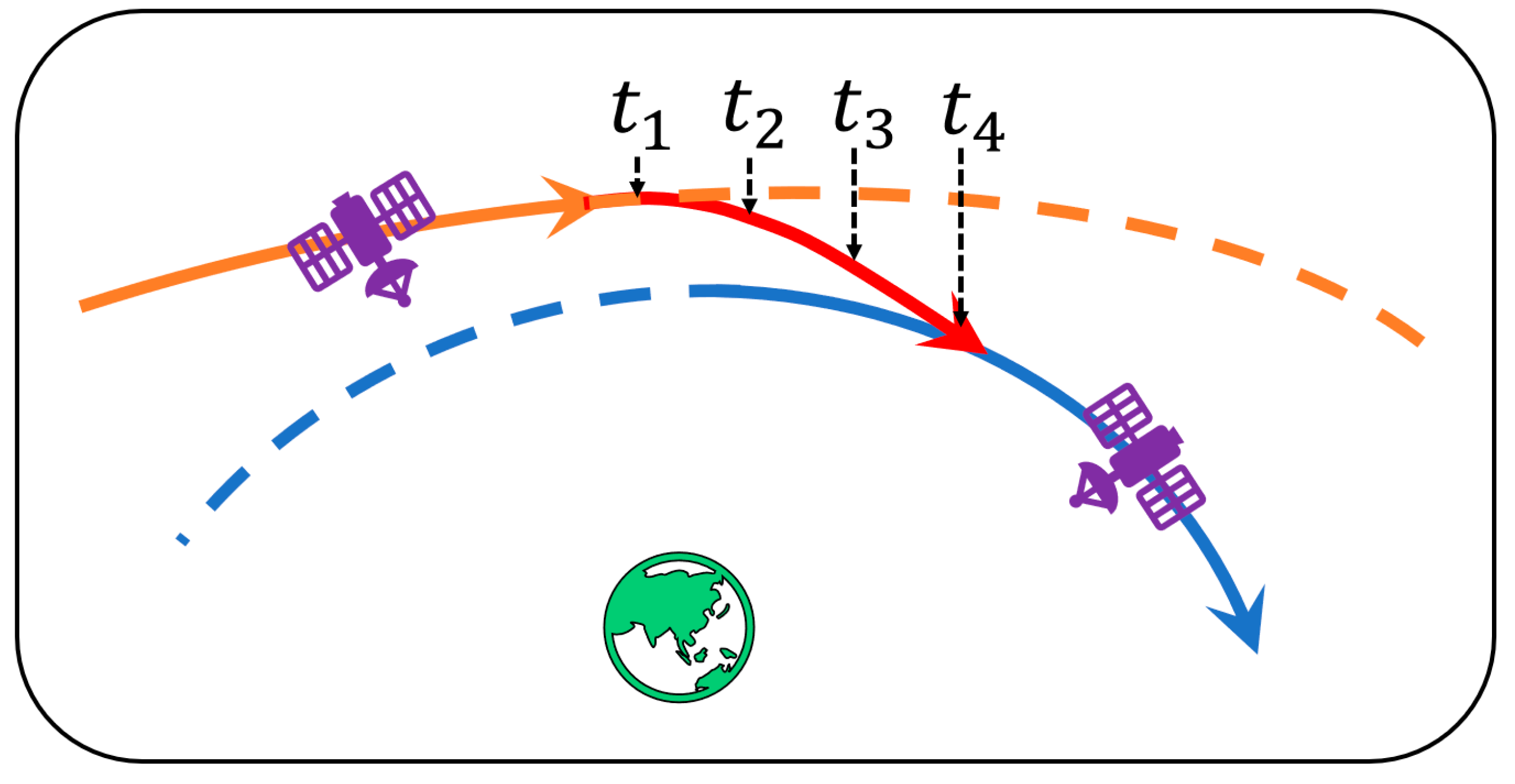
2.2. Orbit Maneuver Detection
3. Data Processing and Validation
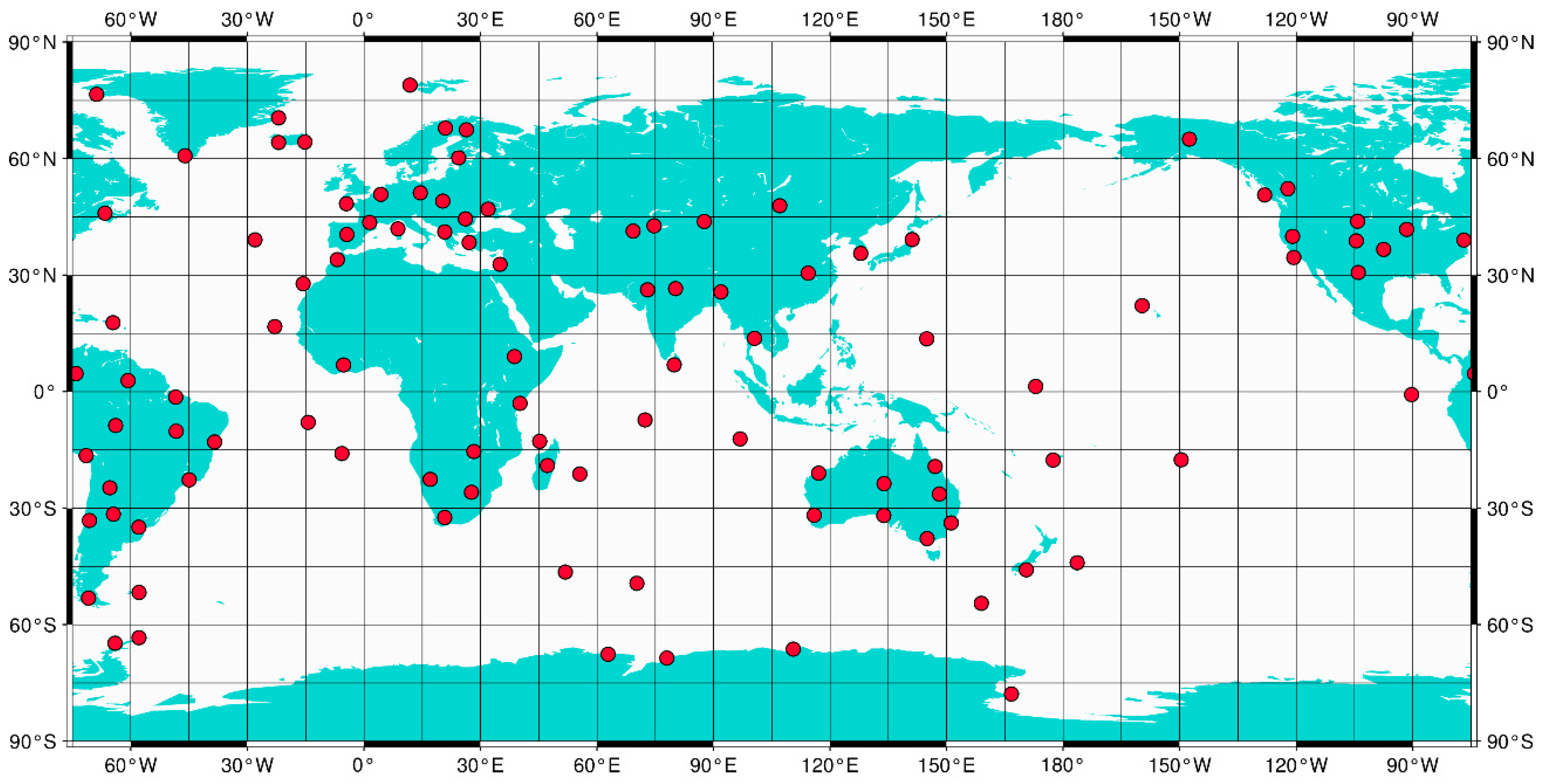
3.1. Network and Processing Strategy
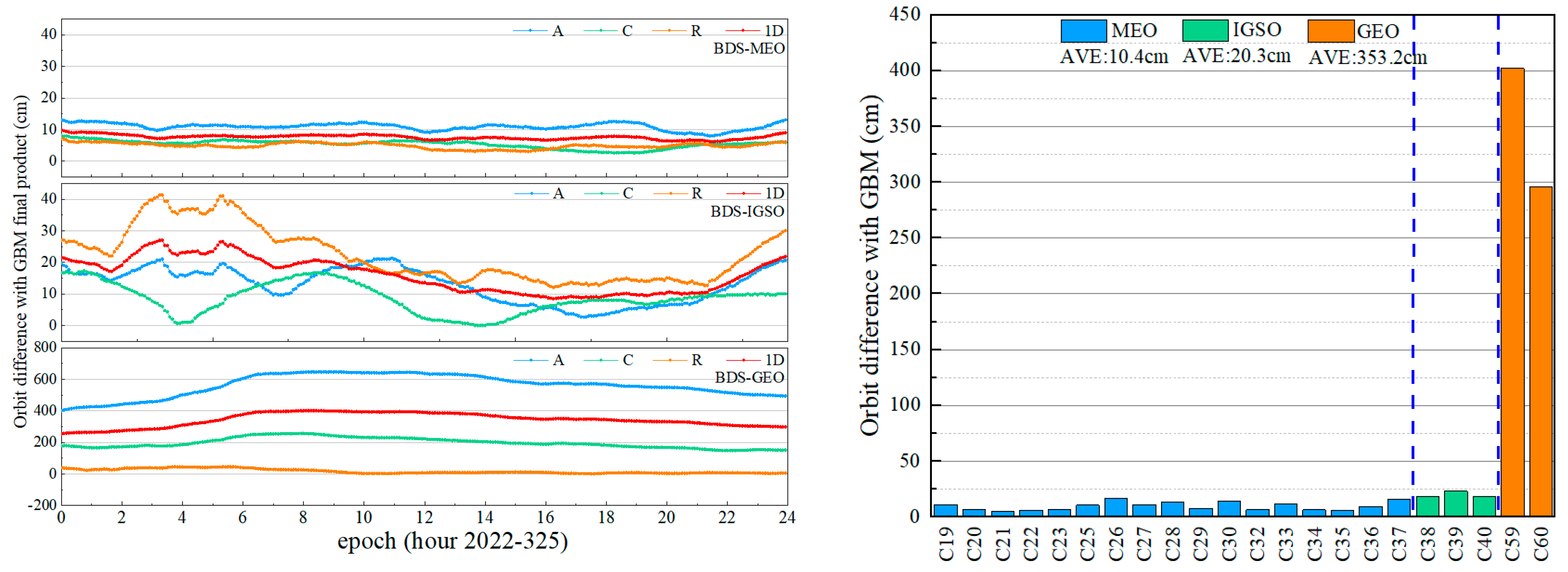
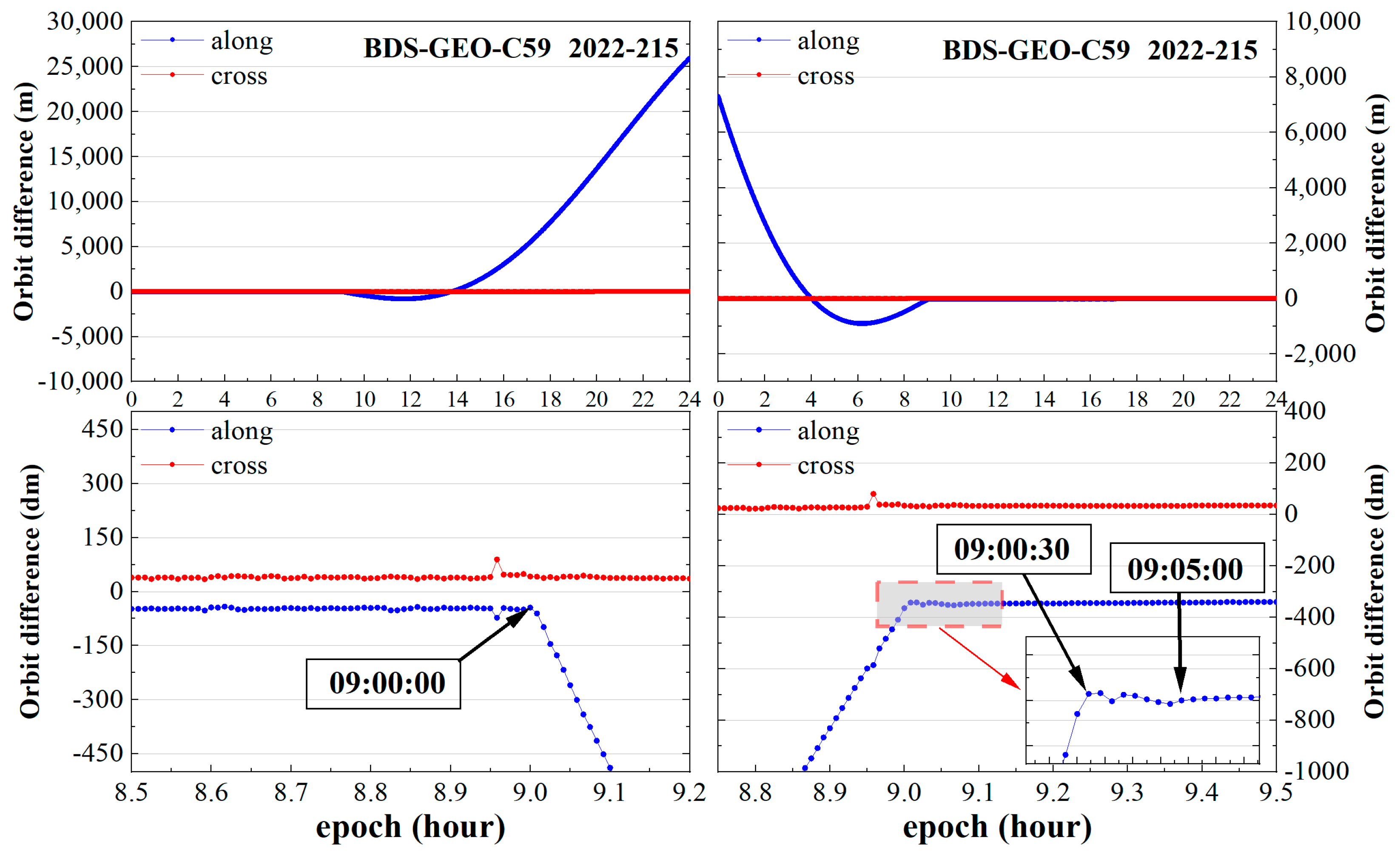
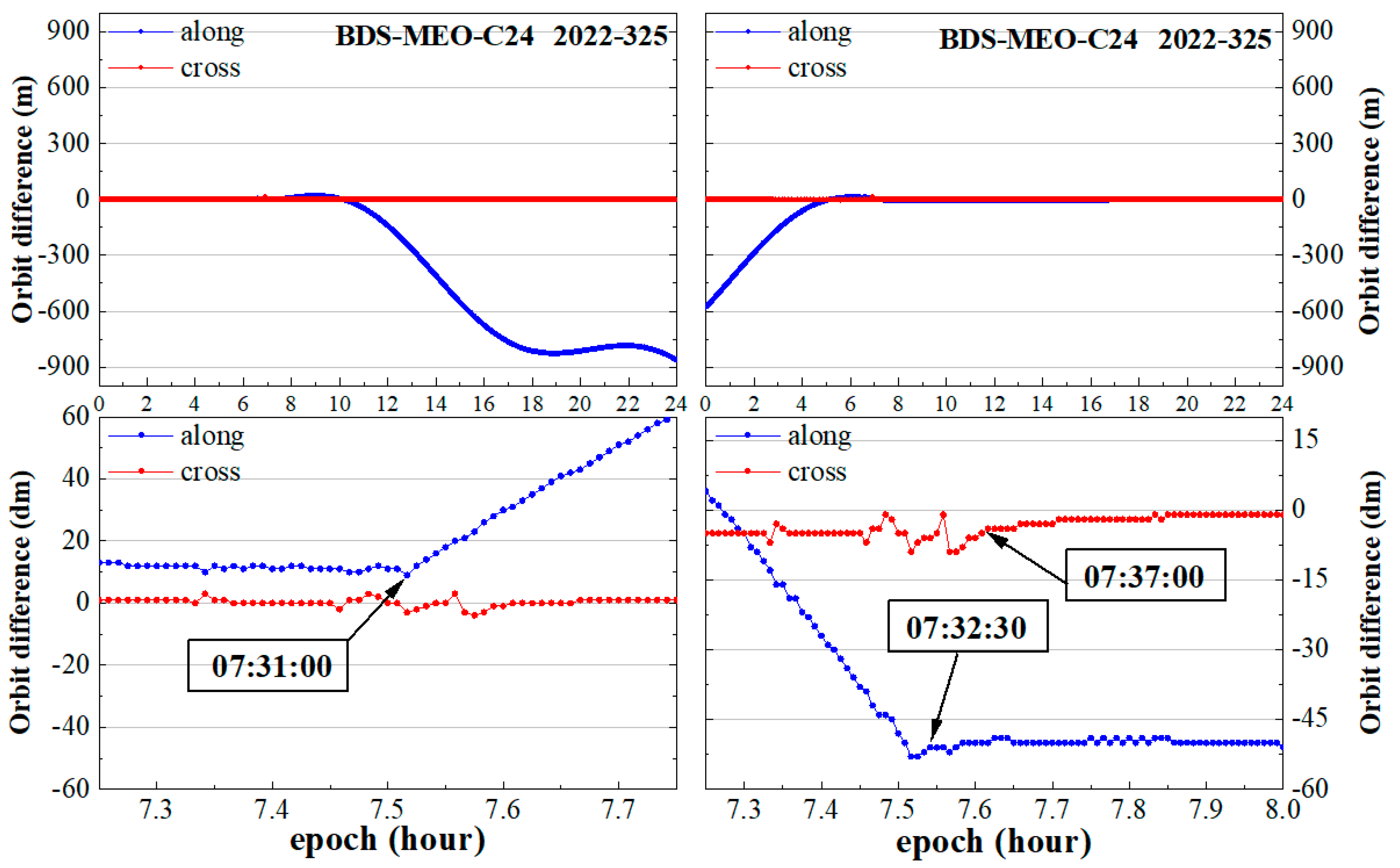
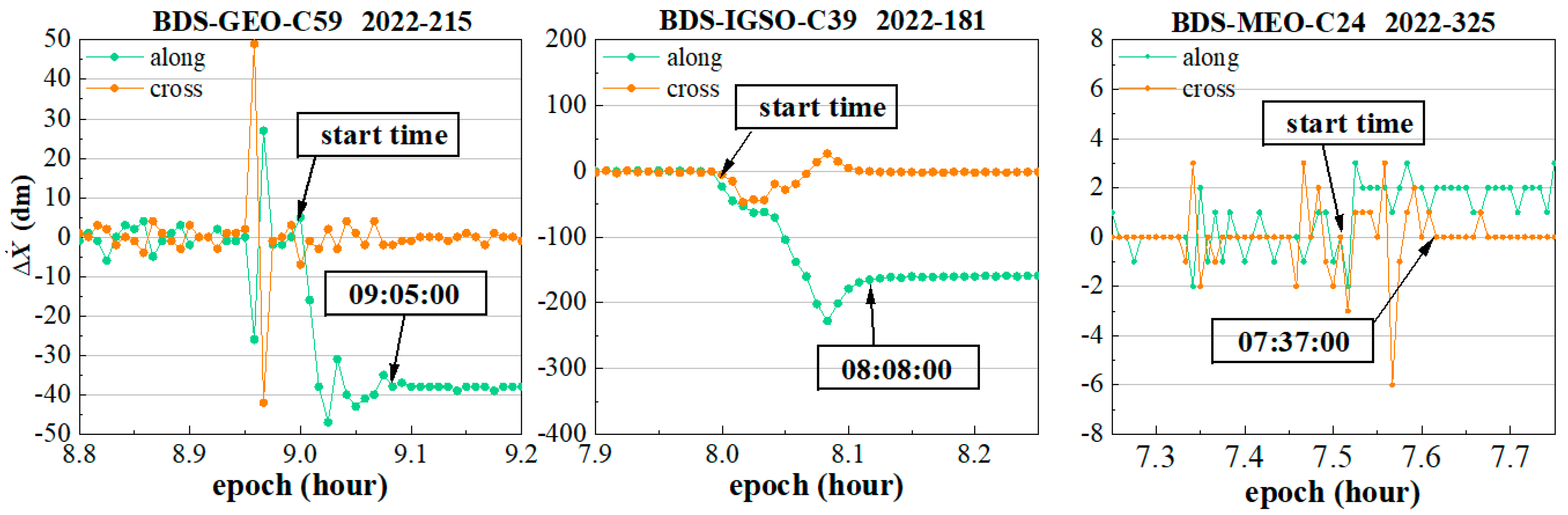
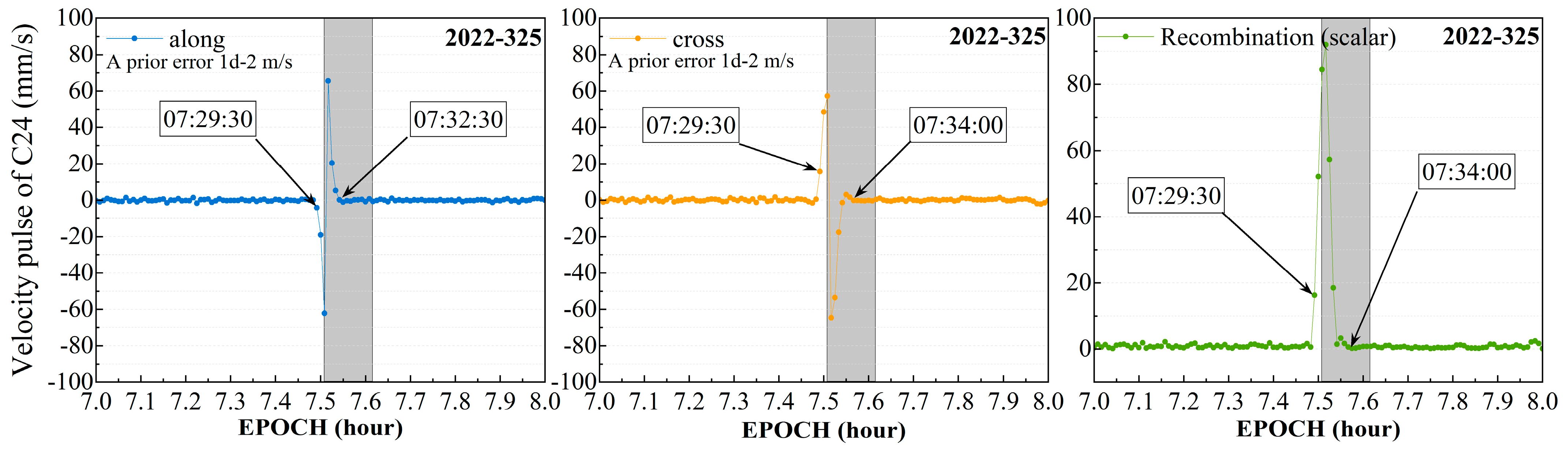
3.2. Experimental Results and Verification
4. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Steigenberger, P.; Hugentobler, U.; Hauschild, A.; Montenbruck, O. Orbit and clock analysis of Compass GEO and IGSO satellites. J. Geod. 2013, 87, 515–525. [Google Scholar] [CrossRef]
- Song, W.D.; Wang, R.L.; Wang, J. A simple and valid analysis method for orbit anomaly detection. Adv. Space Res. 2012, 49, 386–391. [Google Scholar] [CrossRef]
- Qin, Z.W.; Huang, G.W.; Zhang, Q.; Le, W.; Xie, S.C.; She, H.N.; Lai, W.; Wang, X.L. The analysis and detection of orbit maneuvers for the BeiDou satellites based on orbital elements. Acta Geod. Geophys. 2021, 56, 501–522. [Google Scholar] [CrossRef]
- Dach, R.; Brockmann, E.; Schaer, S.; Beutler, G.; Meindl, M.; Prange, L.; Bock, H.; Jaggi, A.; Ostini, L. GNSS processing at CODE: Status report. J. Geod. 2009, 83, 353–365. [Google Scholar] [CrossRef]
- Qiao, J.; Chen, W. Beidou satellite maneuver thrust force estimation for precise orbit determination. Gps Solut. 2018, 22, 1–12. [Google Scholar] [CrossRef]
- Qin, Z.W.; Huang, G.W.; Zhang, Q.; Wang, L.; Yan, X.Y.; Xie, S.C.; Cao, Y.; Wang, X.L. Precise Orbit Determination for BeiDou GEO/IGSO Satellites during Orbit Maneuvering with Pseudo-Stochastic Pulses. Remote Sens. 2019, 11, 2587. [Google Scholar] [CrossRef]
- Qin, Z.W.; Wang, L.; Huang, G.W.; Zhang, Q.; Yan, X.Y.; Xie, S.C.; She, H.N.; Yue, F.; Wang, X.L. Prediction of BeiDou Satellite Orbit Maneuvers to Improve the Reliability of Real-Time Navigation Products. Remote Sens. 2021, 13, 629. [Google Scholar] [CrossRef]
- Fan, L.H.; Tu, R.; Zhang, R.; Han, J.Q.; Zhang, P.F.; Wang, S.Y.; Hong, J.; Lu, X.C. An orbit maneuver detection method based on orbital elements for BeiDou GEO and IGSO satellites. Adv. Space Res. 2022, 69, 3644–3654. [Google Scholar] [CrossRef]
- Huang, G.; Qin, Z.; Zhang, Q.; Wang, L.; Yan, X.; Fan, L.; Wang, X. A Real-Time Robust Method to Detect BeiDou GEO/IGSO Orbital Maneuvers. Sensors 2017, 17, 2761. [Google Scholar] [CrossRef]
- Huang, G.; Qin, Z.; Zhang, Q.; Wang, L.; Yan, X.; Wang, X. An Optimized Method to Detect BDS Satellites’ Orbit Maneuvering and Anomalies in Real-Time. Sensors 2018, 18, 726. [Google Scholar] [CrossRef]
- Qin, Z.; Huang, G.; Zhang, Q.; Wang, L.; Yan, X.; Kang, Y.; Wang, X.; Xie, S. A Method to Determine BeiDou GEO/IGSO Orbital Maneuver Time Periods. Sensors 2019, 19, 2675. [Google Scholar] [CrossRef]
- Song, W.W.; Li, C.L.; Dai, X.L.; Lou, Y.D.; Xu, Y. BDS near real-time maneuver detection based on triple-differenced phase observations. Adv. Space Res. 2022, 69, 3032–3043. [Google Scholar] [CrossRef]
- Tu, R.; Zhang, R.; Fan, L.; Han, J.; Zhang, P.; Lu, X. Real-Time Detection of Orbital Maneuvers Using Epoch-Differenced Carrier Phase Observations and Broadcast Ephemeris Data: A Case Study of the BDS Dataset. Sensors 2020, 20, 4584. [Google Scholar] [CrossRef] [PubMed]
- Du, L.; Zhang, Z.; Li, X.; Wang, R.; Liu, L.; Guo, R. Station-keeping Maneuver Monitoring and Moving-window Ground Track Fitting of GEO Satellites. Acta Geod. Cartogr. Sin. 2014, 43, 233–239. [Google Scholar] [CrossRef]
- Ye, F.; Yuan, Y.; Tan, B.; Ou, J. A Robust Method to Detect BeiDou Navigation Satellite System Orbit Maneuvering/Anomalies and Its Applications to Precise Orbit Determination. Sensors 2017, 17, 1129. [Google Scholar] [CrossRef]
- Bierman, G.J. Factorization Methods for Discrete Sequential Estimation; Dover Publications, Inc.: Mineola, NY, USA, 1977; Volume 128. [Google Scholar]
- Bierman, G.J. Sequential square root filtering and smoothing of discrete linear systems. Automatica 1974, 10, 147–158. [Google Scholar] [CrossRef]
- Bierman, G.J. The treatment of bias in the square-root information filter/smoother. J. Optim. Theory Appl. 1975, 16, 165–178. [Google Scholar] [CrossRef]
- Householder, A.S. Unitary Triangularization of a Nonsymmetric Matrix. J. ACM 1958, 5, 339–342. [Google Scholar] [CrossRef]
- Zuo, X.; Jiang, X.; Li, P.; Wang, J.; Ge, M.; Schuh, H. A square root information filter for multi-GNSS real-time precise clock estimation. Satell. Navig. 2021, 2, 1–14. [Google Scholar] [CrossRef]
- Dai, X.L.; Lou, Y.D.; Dai, Z.Q.; Qing, Y.; Li, M.; Shi, C. Real-time precise orbit determination for BDS satellites using the square root information filter. Gps Solut. 2019, 23, 1–14. [Google Scholar] [CrossRef]
- Dai, X.L.; Gong, X.P.; Li, C.L.; Qing, Y.; Gu, S.F.; Lou, Y.D. Real-time precise orbit and clock estimation of multi-GNSS satellites with undifferenced ambiguity resolution. J. Geod. 2022, 96, 73. [Google Scholar] [CrossRef]
- Duan, B.B.; Hugentobler, U.; Chen, J.P.; Selmke, I.; Wang, J.X. Prediction versus real-time orbit determination for GNSS satellites. Gps Solut. 2019, 23, 1–10. [Google Scholar] [CrossRef]
- Hairer, E. A Runge-Kutta Method of Order 10. IMA J. Appl. Math. 1978, 21, 47–59. [Google Scholar] [CrossRef]
- Montenbruck, O.; Gill, E. Satellite Orbits, Models, Methods and Applications. Appl. Mech. Rev. 2000, 55, B27–B28. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Prange, L.; Deng, Z.; Zhao, Q.; Perosanz, F.; Romero, I.; Noll, C.; Stürze, A.; Weber, G.; et al. The Multi-GNSS Experiment (MGEX) of the International GNSS Service (IGS)–Achievements, prospects and challenges. Adv. Space Res. 2017, 59, 1671–1697. [Google Scholar] [CrossRef]
- Jing-nan, L.; Mao-rong, G. PANDA software and its preliminary result of positioning and orbit determination. Wuhan Univ. J. Nat. Sci. 2003, 8, 603–609. [Google Scholar] [CrossRef]
- Schmid, R.; Dach, R.; Collilieux, X.; Jäggi, A.; Schmitz, M.; Dilssner, F. Absolute IGS antenna phase center model igs08.atx: Status and potential improvements. J. Geod. 2015, 90, 343–364. [Google Scholar] [CrossRef]
- Wu, J.; Wu, S.C.; Hajj, G.A.; Bertiger, W.I.; Lichten, S.M. Effects of antenna orientation on GPS carrier phase. In Proceedings of the AAS/AIAA Astrodynamics Conference, Durango, CO, USA, 19–22 August 1992; pp. 1647–1660. [Google Scholar]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, 25546. [Google Scholar] [CrossRef]
- Luzum, B.; Petit, G. The IERS Conventions (2010): Reference systems and new models. Proc. Int. Astron. Union 2015, 10, 227–228. [Google Scholar] [CrossRef]
- Pavlis, N.K.; Holmes, S.A.; Kenyon, S.C.; Factor, J.K. The development and evaluation of the Earth Gravitational Model 2008 (EGM2008). J Geophys. Res.-Sol. Ea 2012, 117, 8916. [Google Scholar] [CrossRef]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean. Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Arnold, D.; Meindl, M.; Beutler, G.; Dach, R.; Schaer, S.; Lutz, S.; Prange, L.; Sosnica, K.; Mervart, L.; Jaggi, A. CODE’s new solar radiation pressure model for GNSS orbit determination. J. Geod. 2015, 89, 775–791. [Google Scholar] [CrossRef]
- Wang, C.; Guo, J.; Zhao, Q.L.; Ge, M.R. Improving the Orbits of the BDS-2 IGSO and MEO Satellites with Compensating Thermal Radiation Pressure Parameters. Remote Sens. 2022, 14, 641. [Google Scholar] [CrossRef]



| Item | Applied Models |
|---|---|
| Observation modelling | |
| Observation | BDS ionosphere-free combinations of code and phase measurements. |
| Sample rate | 30 s. |
| Elevation mask | 7°. |
| Weighting | A priori precision of 0.02 cycles and 1.5 m for raw phase and code, respectively. 1 for E > 30, otherwise 2sin(E) where E means the elevation angle. |
| Corrections | |
| Satellite phase center | igs14.atx [28]. |
| Receiver phase center | igs14.atx [28]. |
| Phase wind up | Corrected [29]. |
| Troposphere a priori model | Saastamoinen model for wet and dry hydrostatic delay with Global Mapping Function (GMF) mapping functions without gradient model [30] |
| Station displacements | Solid Earth tides, pole tides, and ocean tides are corrected according to IERS Conventions 2010 [31]. |
| Parameterization | |
| Adjustment method | SRIF. |
| Station coordinate | Tightly constrained to the IGS weekly combined solution (weekly SINEX product). |
| Receiver clocks | White noise. |
| Satellite clocks | White noise. |
| Ambiguity fixing | NO. |
| Tropospheric delay | Estimated for each station as random walk. |
| Orbit state constraints | 1d-5 km (position), 1d-6 km/s (velocity), and 1d-5 μm (solar radiation pressure). |
| Dynamical models | |
| Earth gravity | EGM2008 up to 12 × 12 [32]. |
| M-body gravity | Sun, Moon, Mercury, Venus, Mars, Jupiter, Saturn, Uranus, Neptune, and Pluto. |
| Tidal displacement | Solid Earth, pole: IERS conventions 2010 [31]. Ocean tide: FES2004 [33]. |
| Solar radiation pressure | ECOM2 + EMP [34,35]. |
| PRN | DOY | Start Time (GPST) | End Time (GPST) | Duration (Minute) |
|---|---|---|---|---|
| C24 | 325 | 07:31:00 | 07:37:00 | 6 |
| C38 | 029 | 19:19:00 | 19:26:00 | 7 |
| 191 | 22:44:30 | 22:53:00 | 8.5 | |
| C39 | 017 | 00:59:30 | 01:07:30 | 8 |
| 181 | 07:59:30 | 08:07:00 | 7.5 | |
| 356 | 00:29:30 | 00:32:00 | 3.5 | |
| C40 | 026 | 01:29:00 | 01:38:30 | 9.5 |
| 188 | 16:29:00 | 16:38:00 | 9 | |
| C59 | 008 | 15:30:00 | - | - |
| 009 | - | - | - | |
| 040 | 01:30:00 | 01:33:00 | 3 | |
| 070 | 01:30:00 | 01:35:00 | 5 | |
| 098 | 08:30:00 | 08:32:30 | 2.5 | |
| 127 | 09:00:00 | 09:05:00 | 5 | |
| 154 | 08:30:00 | 08:32:30 | 2.5 | |
| 183 | 00:30:00 | 00:35:00 | 5 | |
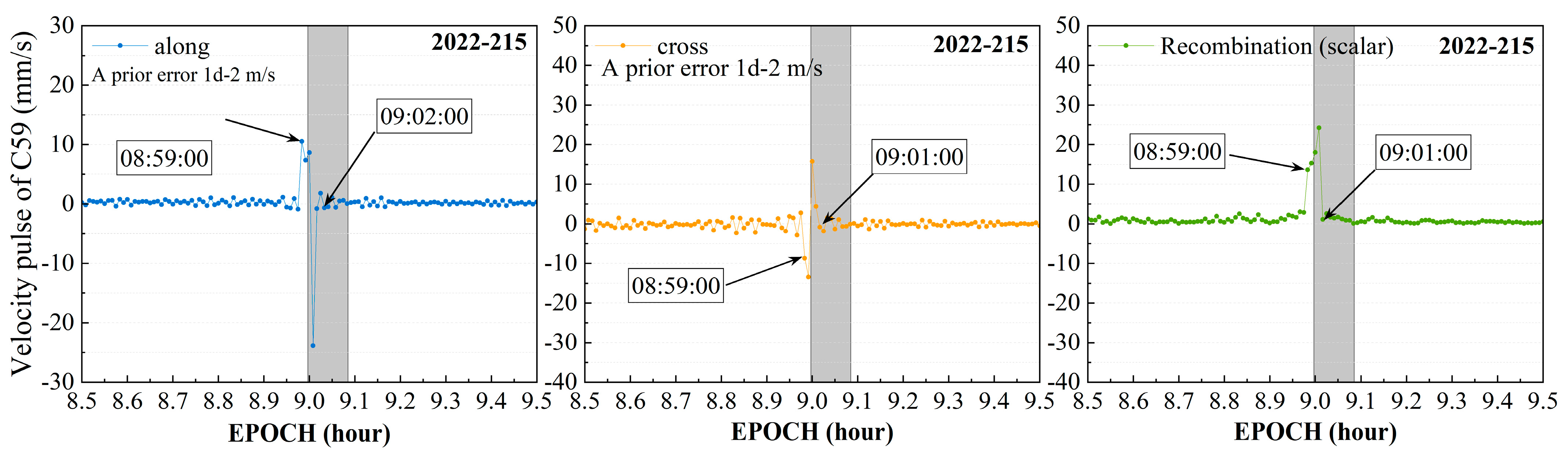
| 215 | 09:00:00 | 09:05:00 | 5 | |
| 248 | 01:00:00 | 01:04:00 | 4 | |
| 281 | 09:00:00 | 09:05:00 | 5 | |
| 311 | 09:00:00 | 09:07:00 | 7 | |
| 341 | 03:25:00 | - | - | |
| 342 | - | - | - | |
| C60 | 028 | 00:30:00 | 00:33:30 | 3.5 |
| 058 | 09:00:00 | 09:05:00 | 5 | |
| 085 | 09:00:00 | 09:12:00 | 12 | |
| 105 | 09:00:00 | 09:02:00 | 2 | |
| 129 | 09:00:00 | 09:02:00 | 2 | |
| 147 | 01:30:00 | 01:32:00 | 2 | |
| 168 | 09:00:00 | 09:02:30 | 2.5 | |
| 198 | 15:30:00 | 15:32:00 | 2 | |
| 225 | 03:00:00 | 03:05:00 | 5 | |
| 245 | 03:00:00 | 03:05:00 | 5 | |
| 271 | 01:00:00 | 01:03:00 | 3 | |
| 291 | 01:00:00 | 01:04:00 | 4 | |
| 329 | 00:25:30 | 00:32:00 | 6.5 | |
| 361 | 00:30:00 | 00:35:00 | 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, Z.; Zhang, Q.; Huang, G.; Tang, L.; Wang, J.; Wang, X. BDS Orbit Maneuver Detection Based on Epoch-Updated Orbits Estimated by SRIF. Remote Sens. 2023, 15, 2558. https://doi.org/10.3390/rs15102558
Qin Z, Zhang Q, Huang G, Tang L, Wang J, Wang X. BDS Orbit Maneuver Detection Based on Epoch-Updated Orbits Estimated by SRIF. Remote Sensing. 2023; 15(10):2558. https://doi.org/10.3390/rs15102558
Chicago/Turabian StyleQin, Zhiwei, Qin Zhang, Guanwen Huang, Longjiang Tang, Jungang Wang, and Xiaolei Wang. 2023. "BDS Orbit Maneuver Detection Based on Epoch-Updated Orbits Estimated by SRIF" Remote Sensing 15, no. 10: 2558. https://doi.org/10.3390/rs15102558
APA StyleQin, Z., Zhang, Q., Huang, G., Tang, L., Wang, J., & Wang, X. (2023). BDS Orbit Maneuver Detection Based on Epoch-Updated Orbits Estimated by SRIF. Remote Sensing, 15(10), 2558. https://doi.org/10.3390/rs15102558







