Trapezoidal-Type Inequalities for Strongly Convex and Quasi-Convex Functions via Post-Quantum Calculus
Abstract
:1. Introduction and Preliminaries
1.1. q-Derivatives and Integrals
- 1.
- ;
- 2.
- ,
1.2. (p,q)-Derivatives and Integrals
2. Trapezoidal Type Inequalities for (p,q)-Quantum Integrals
3. Examples

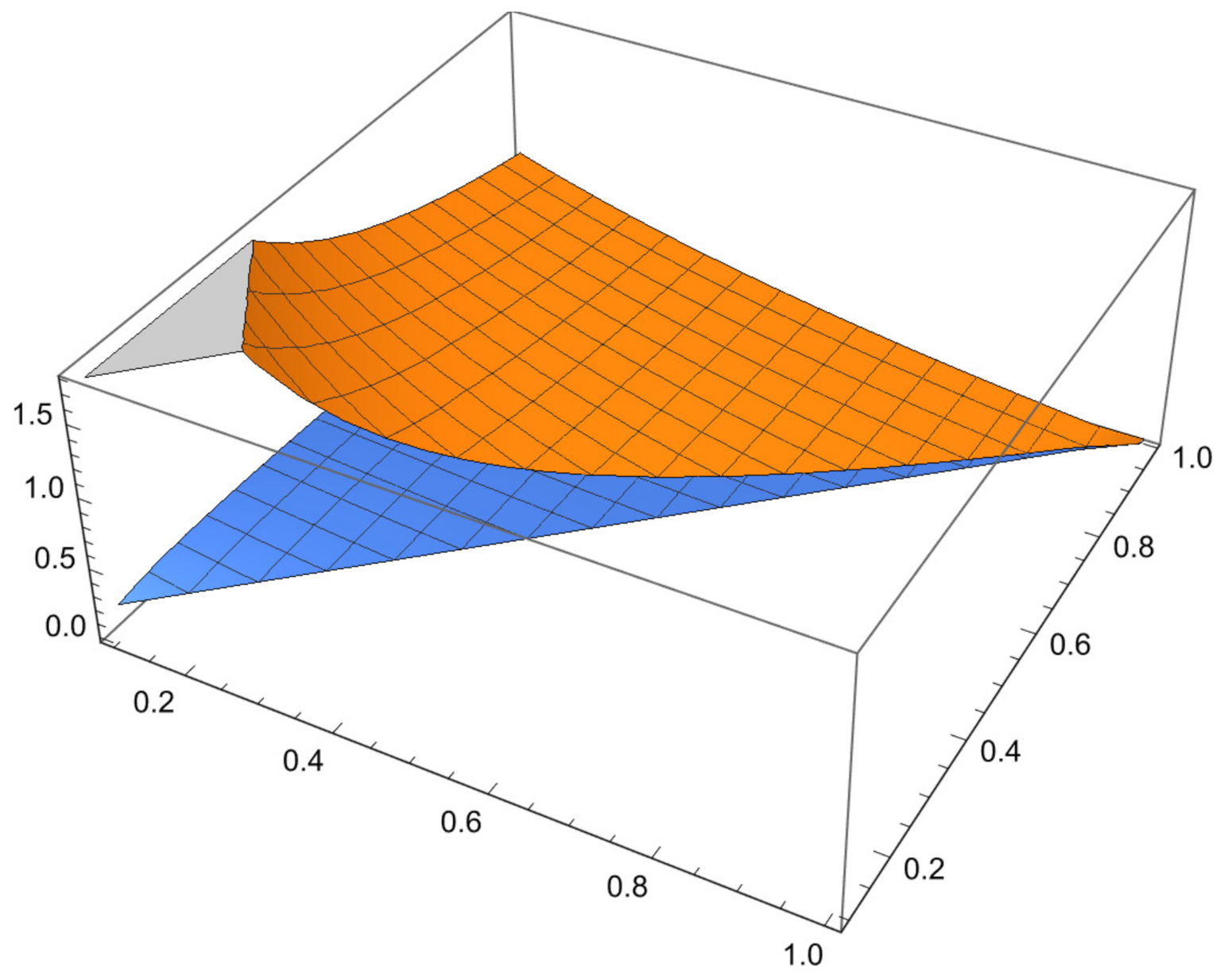

4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jackson, F.H. On a q-definite integrals. Quarterly J. Pure Appl. Math. 1910, 41, 193–203. [Google Scholar]
- Ernst, T. A Comprehensive Treatment of q-Calculus; Springer: Basel, Switzerland, 2012. [Google Scholar]
- Gauchman, H. Integral inequalities in q-calculus. Comput. Math. Appl. 2004, 47, 281–300. [Google Scholar] [CrossRef] [Green Version]
- Kac, V.; Cheung, P. Quantum Calculus, Universitext; Springer: New York, NY, USA, 2002. [Google Scholar]
- Tariboon, J.; Ntouyas, S.K. Quantum calculus on finite intervals and applications to impulsive difference equations. Adv. Differ. Equ. 2013, 2013, 19. [Google Scholar] [CrossRef] [Green Version]
- Noor, M.A.; Noor, K.I.; Awan, M.U. Some quantum integral inequalities via preinvex functions. Appl. Math. Comput. 2015, 269, 242–251. [Google Scholar] [CrossRef]
- Sudsutad, W.; Ntouyas, S.K.; Tariboon, J. Quantum integral inequalities for convex functions. J. Math. Inequal. 2015, 9, 781–793. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Du, T.-S.; Wang, H.; Shen, Y.-J. Different types of quantum integral inequalities via (α,m)-convexity. J. Inequal. Appl. 2018, 2018, 264. [Google Scholar] [CrossRef] [PubMed]
- Alp, N.; Kaya, M.Z.S.; Kunt, M.; İşcan, İ. q-Hermite-Hadamard inequalities and quantum estimates for midpoint type inequalities via convex and quasi-convex functions. J. King Saud Univ. Sci. 2018, 30, 193–203. [Google Scholar] [CrossRef] [Green Version]
- Khan, M.A.; Mohammad, N.; Nwaeze, E.R.; Chu, Y.-M. Quantum Hermite-Hadamard inequality by means of a Green function. Adv. Differ. Equ. 2020, 2020, 99. [Google Scholar] [CrossRef]
- Kalsoom, H.; Rashid, S.; Idrees, M.; Baleanu, D.; Chu, Y.M. Two-variable quantum integral inequalities of Simpson-type based on higher-order generalized strongly preinvex and quasi-preinvex functions. Symmetry 2020, 12, 51. [Google Scholar] [CrossRef] [Green Version]
- Deng, Y.; Kalsoom, H.; Wu, S. Some new quantum Hermite–Hadamard-type estimates within a class of generalized (s, m)-preinvex functions. Symmetry 2019, 1, 1283. [Google Scholar] [CrossRef] [Green Version]
- Kalsoom, H.; Wu, J.D.; Hussain, S.; Latif, M.A. Simpson’s type inequalities for co-ordinated convex functions on quantum calculus. Symmetry 2019, 11, 768. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Kalsoom, H.; Budak, H.; Idrees, M. q-Hermite–Hadamard Inequalities for Generalized Exponentially (s, m, η)-Preinvex Functions. J. Math. 2021, 2021, 5577340. [Google Scholar] [CrossRef]
- Bermudo, S.; Kórus, P.; Valdés, J.N. On q-Hermite–Hadamard inequalities for general convex functions. Acta Math. Hung. 2020, 162, 364–374. [Google Scholar] [CrossRef]
- Kalsoom, H.; Idrees, M.; Baleanu, D.; Chu, Y.M. New Estimates of q1q2-Ostrowski-Type Inequalities within a Class of-Polynomial Prevexity of Functions. J. Funct. Spaces 2020, 2020, 3720798. [Google Scholar] [CrossRef]
- You, X.; Kara, H.; Budak, H.; Kalsoom, H. Quantum Inequalities of Hermite—Hadamard Type for r-Convex Functions. J. Math. 2021, 2021, 6634614. [Google Scholar] [CrossRef]
- Vivas-Cortez, M.; Ali, M.A.; Budak, H.; Kalsoom, H.; Agarwal, P. Some New Hermite–Hadamard and Related Inequalities for Convex Functions via (p, q)-Integral. Entropy 2021, 23, 828. [Google Scholar] [CrossRef]
- Chu, H.; Kalsoom, H.; Rashid, S.; Idrees, M.; Safdar, F.; Chu, Y.M.; Baleanu, D. Quantum Analogs of Ostrowski-Type Inequalities for Raina’s Function correlated with Coordinated Generalized Φ-Convex Functions. Symmetry 2020, 12, 308. [Google Scholar] [CrossRef] [Green Version]
- Tunç, M.; Göv, E. Some integral inequalities via (p,q)-calculus on finite intervals. RGMIA Res. Rep. Coll. 2016, 19, 1–12. [Google Scholar]
- Kunt, M.; İ.şcan, I.; Alp, N.; Sarikaya, M.Z. (p,q)-Hermite-Hadamard inequalities and (p,q)-estimates for midpoint type inequalities via convex and quasi-convex functions. Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Mat. RACSAM 2018, 112, 969–992. [Google Scholar] [CrossRef]
- Latif, M.A.; Kunt, M.; Dragomir, S.S. Post-quantum trapezoid type inequalities. Aims Math. 2020, 5, 4011–4026. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Awan, M.U.; Talib, S.; Noor, M.A.; Noor, K.I. New post quantum analogues of Ostrowski-type inequalities using new definitions of left–right (p,q)-derivatives and definite integrals. Adv. Differ. Equ. 2020, 634, 1–15. [Google Scholar] [CrossRef]
- Prabseang, J.; Nonlaopon, K.; Tariboon, J.; Ntouyas, S.K. Refinements of Hermite-Hadamard Inequalities for Continuous Convex Functions via (p,q)-Calculus. Mathematics 2021, 9, 446. [Google Scholar] [CrossRef]
- Klasoom, H.; Minhyung, C. Trapezoidal (p,q)-Integral Inequalities Related to (η1,η2)-convex Functions with Applications. Int. J. Theor. Phys. 2021, 60, 2627–2641. [Google Scholar] [CrossRef]
- Kalsoom, H.; Amer, M.; Junjua, M.U.; Hussain, S.; Shahzadi, G. Some (p,q)-estimates of Hermite-Hadamard-type inequalities for coordinated convex and quasi-convex functions. Mathematics 2019, 7, 683. [Google Scholar] [CrossRef] [Green Version]
- Kalsoom, H.; Latif, M.A.; Rashid, S.; Baleanu, D.; Chu, Y.M. New (p,q)-estimates for different types of integral inequalities via (α,m)-convex mappings. Open Math. 2020, 18, 1830–1854. [Google Scholar] [CrossRef]
- Kalsoom, H.; Idrees, M.; Kashuri, A.; Awan, M.U.; Chu, Y.M. Some new (p1p2,q1q2)-estimates of Ostrowski-type integral inequalities via n-polynomials s-type convexity. AIMS Math 2020, 5, 7122–7144. [Google Scholar] [CrossRef]
- Usman, T.; Saif, M.; Choi, J. Certain identities associated with (p,q)-binomial coefficients and (p,q)-Stirling Polynomials of the second kind. Symmetry 2020, 12, 1436. [Google Scholar] [CrossRef]
- Kalsoom, H.; Ali, M.A.; Idrees, M.; Agarwal, P.; Arif, M. New Post Quantum Analogues of Hermite–Hadamard Type Inequalities for Interval-Valued Convex Functions. Math. Probl. Eng. 2021, 17, 5529650. [Google Scholar]
- Hermite, C. Sur deux limites d’une intégrale dé finie. Mathesis 1883, 3, 82. [Google Scholar]
- Hadamard, J. Etude sur les propri etes des fonctions enteres et en particulier dune fonction Consideree par Riemann. J. Math. Pures Appl. 1893, 58, 171–215. [Google Scholar]
- Dragomir, S.S.; Agarwal, R.P. Two inequalities for differentiable mappings and applications to special means of real numbers and to trapezoidal formula. Appl. Math. Lett. 1998, 11, 91–95. [Google Scholar] [CrossRef] [Green Version]
- Kalsoom, H.; Hussain, S.; Rashid, S. Hermite-Hadamard type integral inequalities for functions whose mixed partial derivatives are co-ordinated preinvex. Punjab Univ. J. Math. 2020, 52, 63–76. [Google Scholar]
- Kalsoom, H.; Hussain, S. Some Hermite-Hadamard type integral inequalities whose n-times differentiable functions are s-logarithmically convex functions. Punjab Univ. J. Math. 2019, 2019, 65–75. [Google Scholar]
- Polyak, B.T. Existence theorems and convergence of minimizing sequences in extremum problems with restrictions, Soviet mathematics. Doklady 1966, 166, 72–75. [Google Scholar]
- Nikodem, K.; Pales, Z.S. Characterizations of inner product spaces by strongly convex functions. Banach J. Math. Anal. 2011, 1, 83–87. [Google Scholar] [CrossRef]
- Ion, D.A. Some estimates on the Hermite-Hadamard inequality through quasi-convex functions. Ann. Univ. Craiova Ser. Mat. Inform. 2007, 34, 83–88. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalsoom, H.; Vivas-Cortez, M.; Latif, M.A. Trapezoidal-Type Inequalities for Strongly Convex and Quasi-Convex Functions via Post-Quantum Calculus. Entropy 2021, 23, 1238. https://doi.org/10.3390/e23101238
Kalsoom H, Vivas-Cortez M, Latif MA. Trapezoidal-Type Inequalities for Strongly Convex and Quasi-Convex Functions via Post-Quantum Calculus. Entropy. 2021; 23(10):1238. https://doi.org/10.3390/e23101238
Chicago/Turabian StyleKalsoom, Humaira, Miguel Vivas-Cortez, and Muhammad Amer Latif. 2021. "Trapezoidal-Type Inequalities for Strongly Convex and Quasi-Convex Functions via Post-Quantum Calculus" Entropy 23, no. 10: 1238. https://doi.org/10.3390/e23101238
APA StyleKalsoom, H., Vivas-Cortez, M., & Latif, M. A. (2021). Trapezoidal-Type Inequalities for Strongly Convex and Quasi-Convex Functions via Post-Quantum Calculus. Entropy, 23(10), 1238. https://doi.org/10.3390/e23101238






