An Analytical Technique, Based on Natural Transform to Solve Fractional-Order Parabolic Equations
Abstract
1. Introduction
2. Preliminaries
3. Idea of FNTM
4. Numerical Implementation
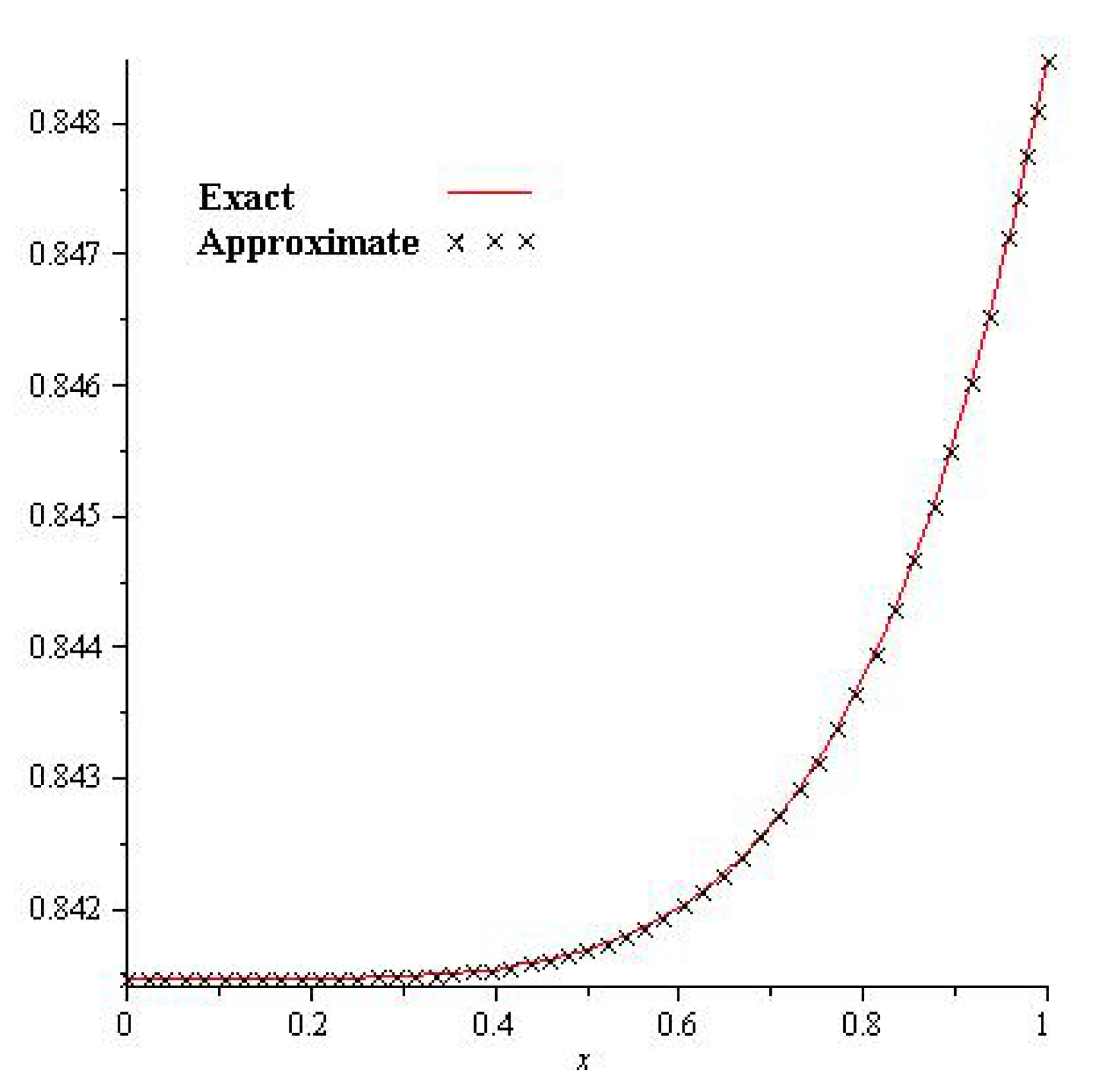
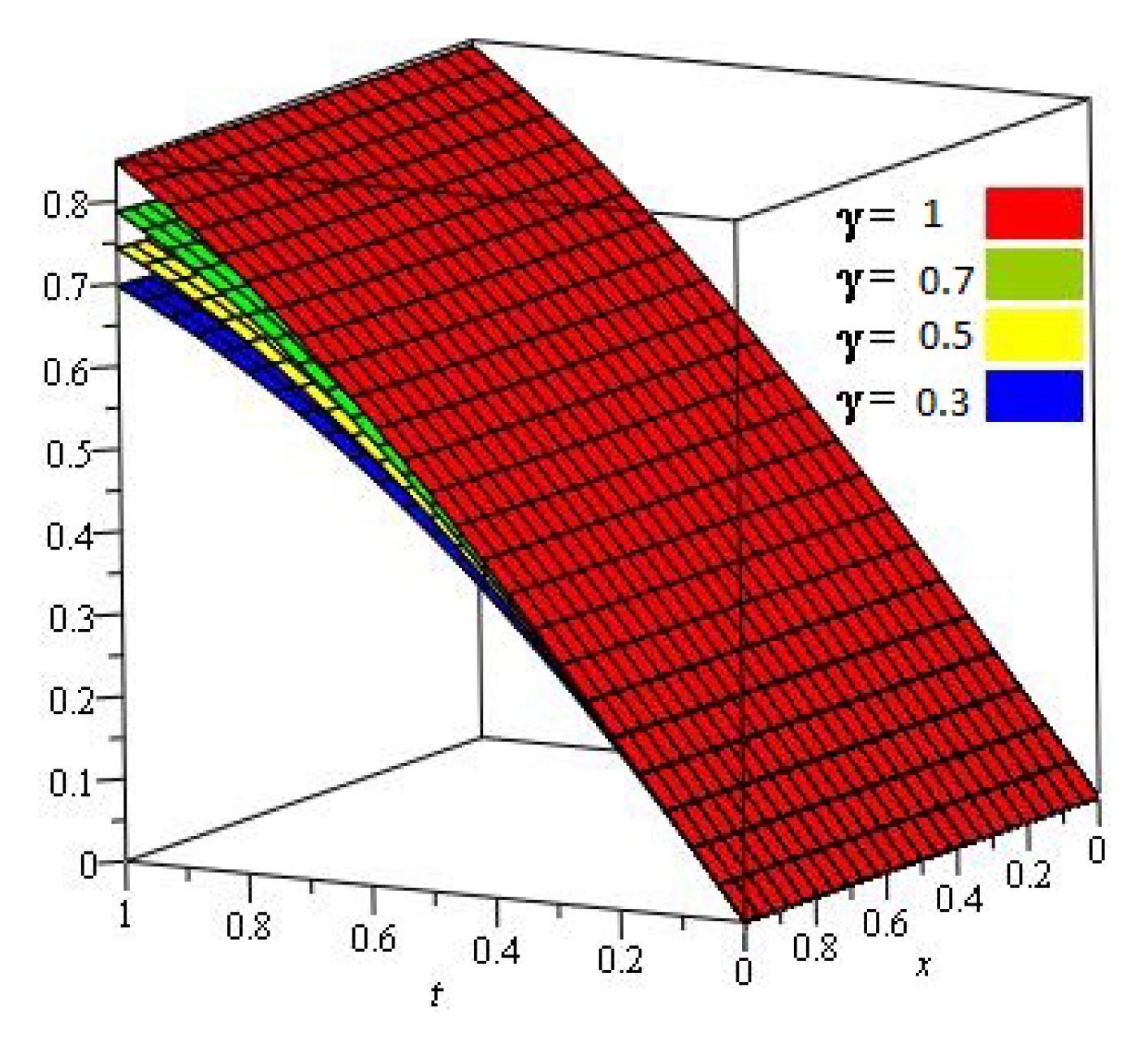
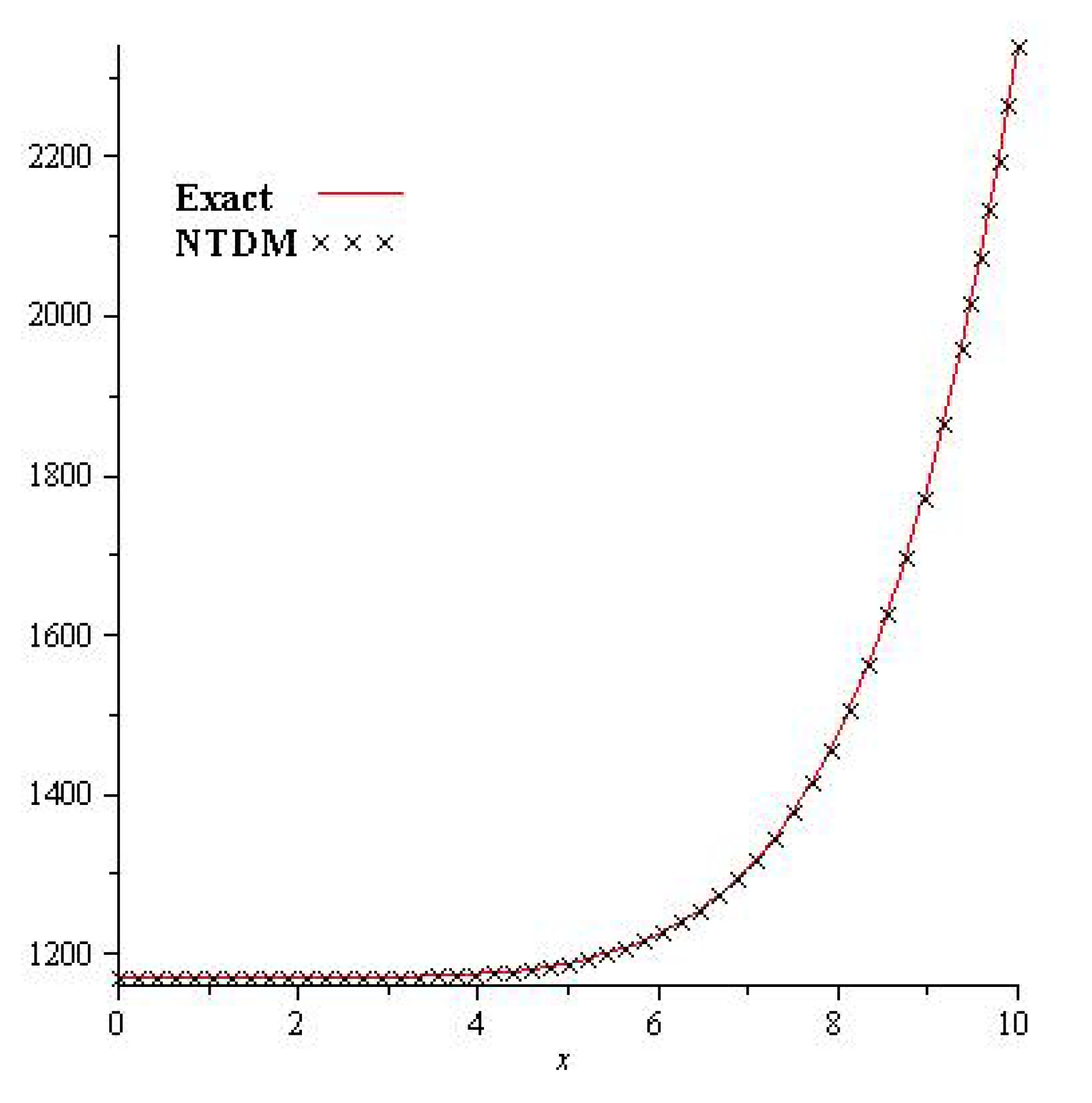
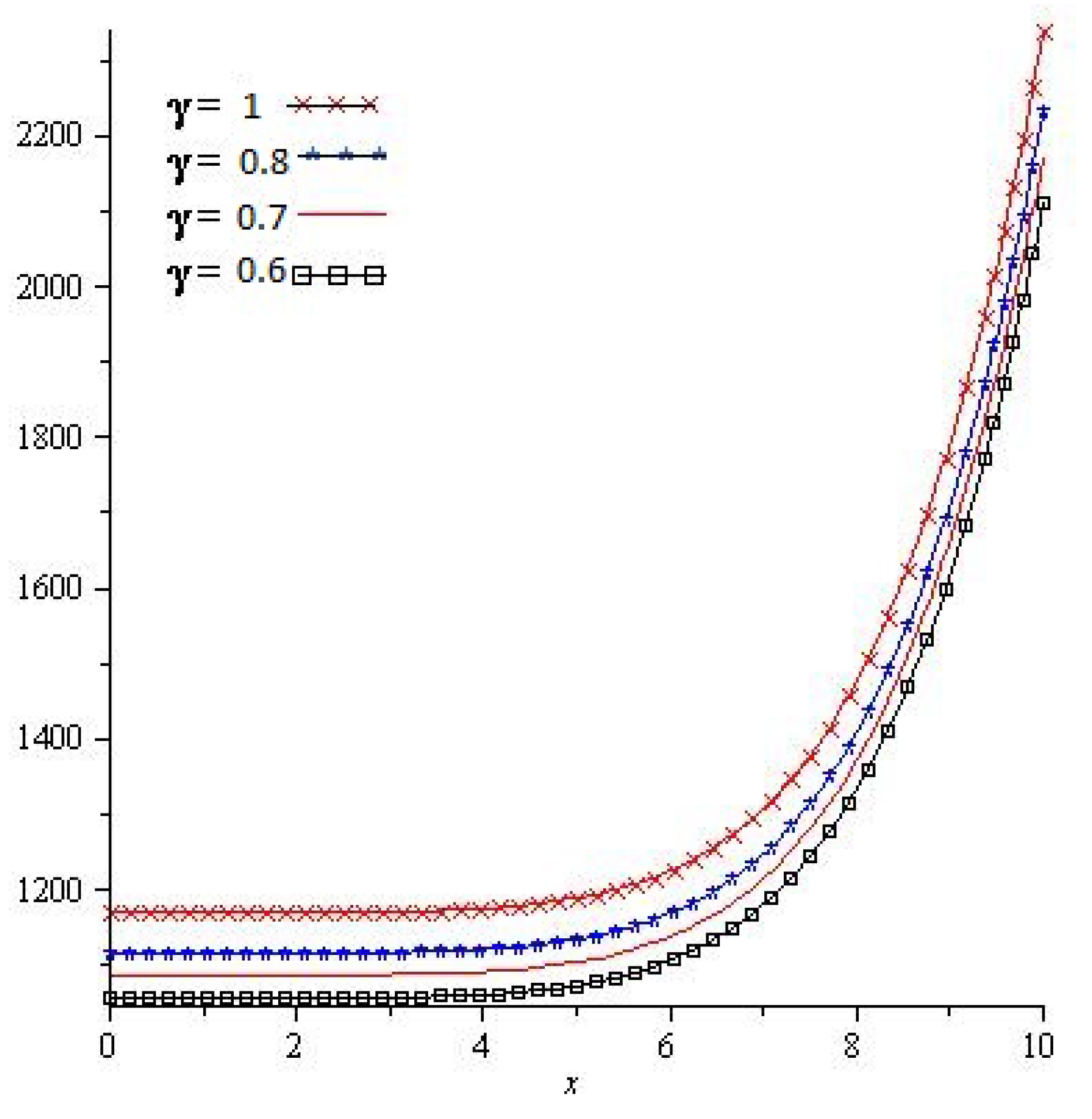
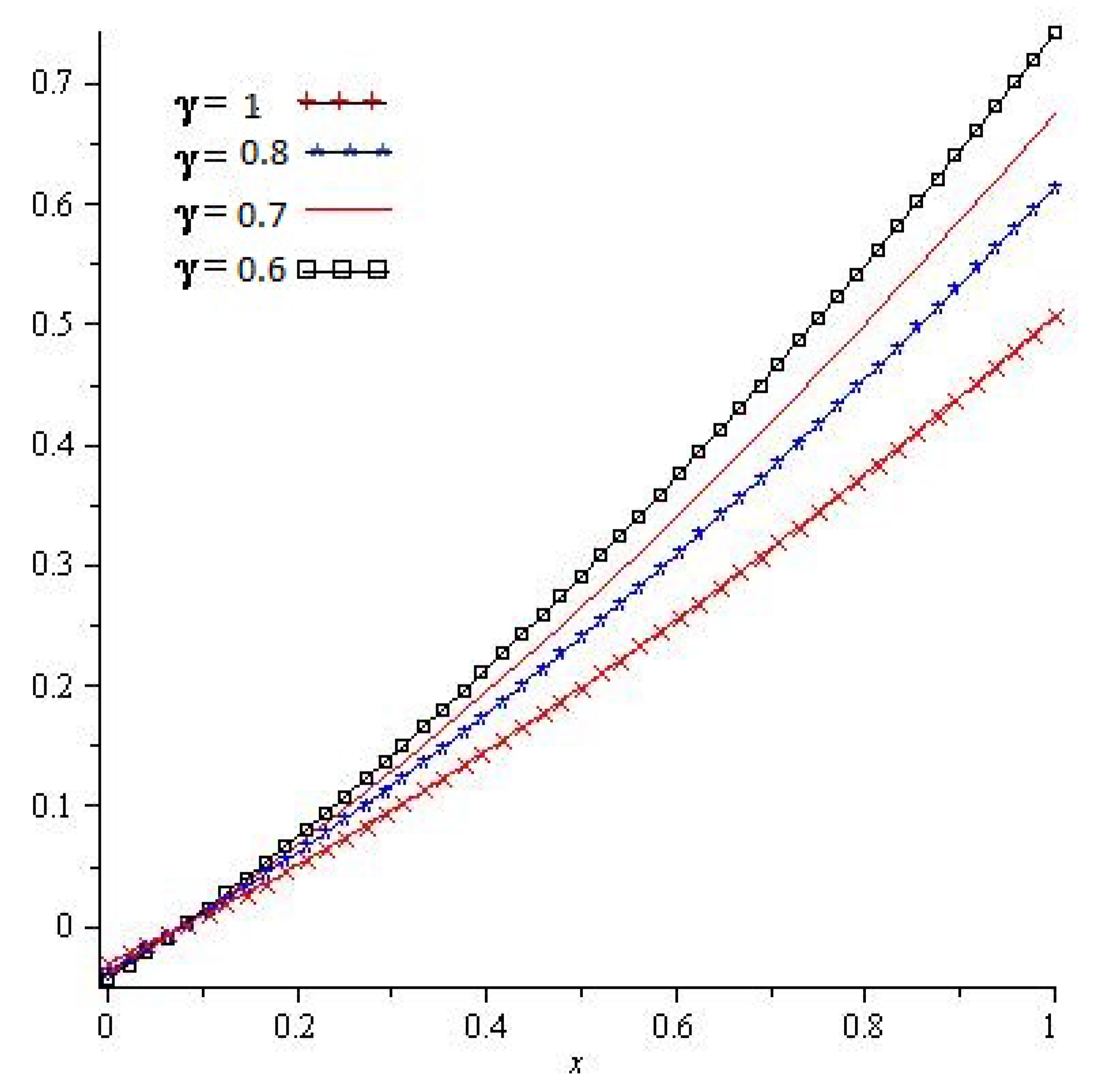
4.1. Problem
4.2. Problem
4.3. Problem
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Khaliq, A.Q.M.; Twizell, E.H. A family of second order methods for variable coefficient fourth order parabolic partial differential equations. Int. J. Comput. Math. 1987, 23, 63–76. [Google Scholar] [CrossRef]
- Gorman, D.J. Free Vibration Analysisi of Beams and Shafts(Book); Research supported by the National Research Council of Canada; Wiley-Interscience: New York, NY, USA, 1975; 395p. [Google Scholar]
- Andrade, C.; McKee, S. High accuracy ADI methods for fourth order parabolic equations with variable coefficients. J. Comput. Appl. Math. 1977, 3, 11–14. [Google Scholar] [CrossRef][Green Version]
- Conte, S.D. A stable implicit finite difference approximation to a fourth order parabolic equation. J. ACM 1957, 4, 18–23. [Google Scholar] [CrossRef]
- Royster, W.C.; Conte, S.D. Convergence of finite difference solutions to a solution of the equation of the vibrating rod. Proc. Am. Math. Soc. 1956, 7, 742–749. [Google Scholar] [CrossRef]
- Evans, D.J. A stable explicit method for the finite-difference solution of a fourth-order parabolic partial differential equation. Comput. J. 1965, 8, 280–287. [Google Scholar] [CrossRef][Green Version]
- Evans, D.J.; Yousif, W.S. A note on solving the fourth order parabolic equation by the AGE method. Int. J. Comput. Math. 1991, 40, 93–97. [Google Scholar] [CrossRef]
- Wazwaz, A.-M. On the solution of the fourth order parabolic equation by the decomposition method. Int. J. Comput. Math. 1995, 57, 213–217. [Google Scholar] [CrossRef]
- Liao, W.; Zhu, J.; Khaliq, A.Q.M. An efficient high-order algorithm for solving systems of reaction-diffusion equations. Numer. Methods Part. Differ. Equ. 2002, 18, 340–354. [Google Scholar] [CrossRef]
- Jain, M.K.; Iyengar, S.R.K.; Lone, A.G. Higher order difference formulas for a fourth order parabolic partial differential equation. Int. J. Numer. Methods Eng. 1976, 10, 1357–1367. [Google Scholar] [CrossRef]
- Caglar, H.; Caglar, N. Fifth-degree B-spline solution for a fourth-order parabolic partial differential equations. Appl. Math. Comput. 2008, 201, 597–603. [Google Scholar] [CrossRef]
- Wazwaz, A.-M. Analytic treatment for variable coefficient fourth-order parabolic partial differential equations. Appl. Math. Comput. 2001, 123, 219–227. [Google Scholar] [CrossRef]
- Rashidinia, J.; Mohammadi, R. Sextic spline solution of variable coefficient fourth-order parabolic equations. Int. J. Comput. Math. 2010, 87, 3443–3454. [Google Scholar] [CrossRef]
- Aziz, T.; Khan, A.; Rashidinia, J. Spline methods for the solution of fourth-order parabolic partial differential equations. Appl. Math. Comput. 2005, 167, 153–166. [Google Scholar] [CrossRef]
- Biazar, J.; Ghazvini, H. Hes variational iteration method for fourth-order parabolic equations. Comput. Math. Appl. 2007, 54, 1047–1054. [Google Scholar] [CrossRef]
- Noor, M.A.; Noor, K.I.; Mohyud-Din, S.T. Modified variational iteration technique for solving singular fourth-order parabolic partial differential equations. Nonlinear Anal. Theory Methods Appl. 2009, 71, e630–e640. [Google Scholar] [CrossRef]
- Dehghan, M.; Manafian, J. The solution of the variable coefficients fourth-order parabolic partial differential equations by the homotopy perturbation method. Z. Naturforschung A 2009, 64, 420–430. [Google Scholar] [CrossRef]
- Nadeem, M.; Li, F.; Ahmad, H. Modified Laplace variational iteration method for solving fourth-order parabolic partial differential equation with variable coefficients. Comput. Math. Appl. 2019, 78, 2052–2062. [Google Scholar] [CrossRef]
- Rawashdeh, M.; Maitama, S. Finding exact solutions of nonlinear PDEs using the natural decomposition method. Math. Methods Appl. Sci. 2017, 40, 223–236. [Google Scholar] [CrossRef]
- Baskonus, H.M.; Bulut, H.; Pandir, Y. The natural transform decomposition method for linear and nonlinear partial differential equations. Math. Eng. Sci. Aerosp. (MESA) 2014, 5, 111–126. [Google Scholar]
- Adomian, G. A new approach to nonlinear partial differential equations. J. Math. Anal. Appl. 1984, 102, 420–434. [Google Scholar] [CrossRef]
- Belgacem, F.B.M.; Silambarasan, R. Theory of natural transform. J. MESA 2012, 3, 99–124. [Google Scholar]
- Khan, H.; Shah, R.; Kumam, P.; Arif, M. Analytical Solutions of Fractional-Order Heat and Wave Equations by the Natural Transform Decomposition Method. Entropy 2019, 21, 597. [Google Scholar] [CrossRef] [PubMed]
- Shah, R.; Khan, H.; Kumam, P.; Arif, M.; Baleanu, D. Natural Transform Decomposition Method for Solving Fractional-Order Partial Differential Equations with Proportional Delay. Mathematics 2019, 7, 532. [Google Scholar] [CrossRef]
- Shah, R.; Khan, H.; Mustafa, S.; Kumam, P.; Arif, M. Analytical Solutions of Fractional-Order Diffusion Equations by Natural Transform Decomposition Method. Entropy 2019, 21, 557. [Google Scholar] [CrossRef]
- Rawashdeh, M.S.; Al-Jammal, H. New approximate solutions to fractional nonlinear systems of partial differential equations using the FNDM. Adv. Differ. Equ. 2016, 1, 235. [Google Scholar] [CrossRef]
- Eltayeb, H.; Abdalla, Y.T.; Bachar, I.; Khabir, M.H. Fractional telegraph equation and its solution by natural transform decomposition method. Symmetry 2019, 11, 334. [Google Scholar] [CrossRef]
- Prakasha, D.G.; Veeresha, P.; Rawashdeh, M.S. Numerical solution for (2+1)-dimensional time-fractional coupled Burger equations using fractional natural decomposition method. Math. Methods Appl. Sci. 2019, 42, 3409–3427. [Google Scholar] [CrossRef]
- Belgacem, F.B.M.; Silambarasan, R. Advances in the natural transform. AIP Conf. Proc. 2012, 1493, 106–110. [Google Scholar]
- Khan, Z.H.; Khan, W.A. N-transform properties and applications. NUST J. Eng. Sci. 2008, 1, 127–133. [Google Scholar]
- Hilfer, R. Applications of Fractional Calculus in Physics; World Sci. Publishing: River Edge, NJ, USA, 2000. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Elsevier: Amsterdam, The Netherlands, 1998; Volume 198. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations, 1st ed.; Wiley: Hoboken, NJ, USA, 1993. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agarwal, R.P.; Mofarreh, F.; Shah, R.; Luangboon, W.; Nonlaopon, K. An Analytical Technique, Based on Natural Transform to Solve Fractional-Order Parabolic Equations. Entropy 2021, 23, 1086. https://doi.org/10.3390/e23081086
Agarwal RP, Mofarreh F, Shah R, Luangboon W, Nonlaopon K. An Analytical Technique, Based on Natural Transform to Solve Fractional-Order Parabolic Equations. Entropy. 2021; 23(8):1086. https://doi.org/10.3390/e23081086
Chicago/Turabian StyleAgarwal, Ravi P., Fatemah Mofarreh, Rasool Shah, Waewta Luangboon, and Kamsing Nonlaopon. 2021. "An Analytical Technique, Based on Natural Transform to Solve Fractional-Order Parabolic Equations" Entropy 23, no. 8: 1086. https://doi.org/10.3390/e23081086
APA StyleAgarwal, R. P., Mofarreh, F., Shah, R., Luangboon, W., & Nonlaopon, K. (2021). An Analytical Technique, Based on Natural Transform to Solve Fractional-Order Parabolic Equations. Entropy, 23(8), 1086. https://doi.org/10.3390/e23081086







