Microstructure Evolution by Thermomechanical Processing in the Fe-10Al-12V Superalloy
Abstract
1. Introduction
2. Materials and Methods
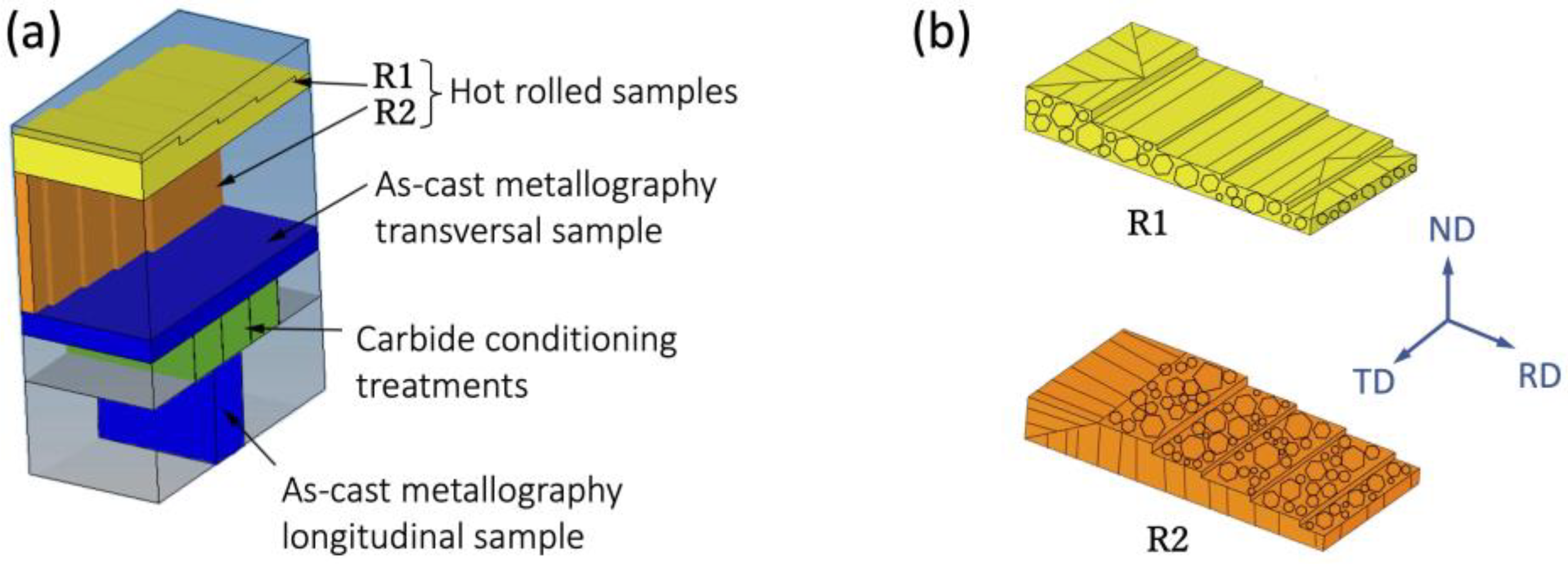
2.1. Alloy Production and Processing
2.1.1. Carbide Conditioning Treatments Exploration
2.1.2. Prismatic Ingot Processing
2.1.3. Cylindrical Ingot Processing
2.2. Characterization
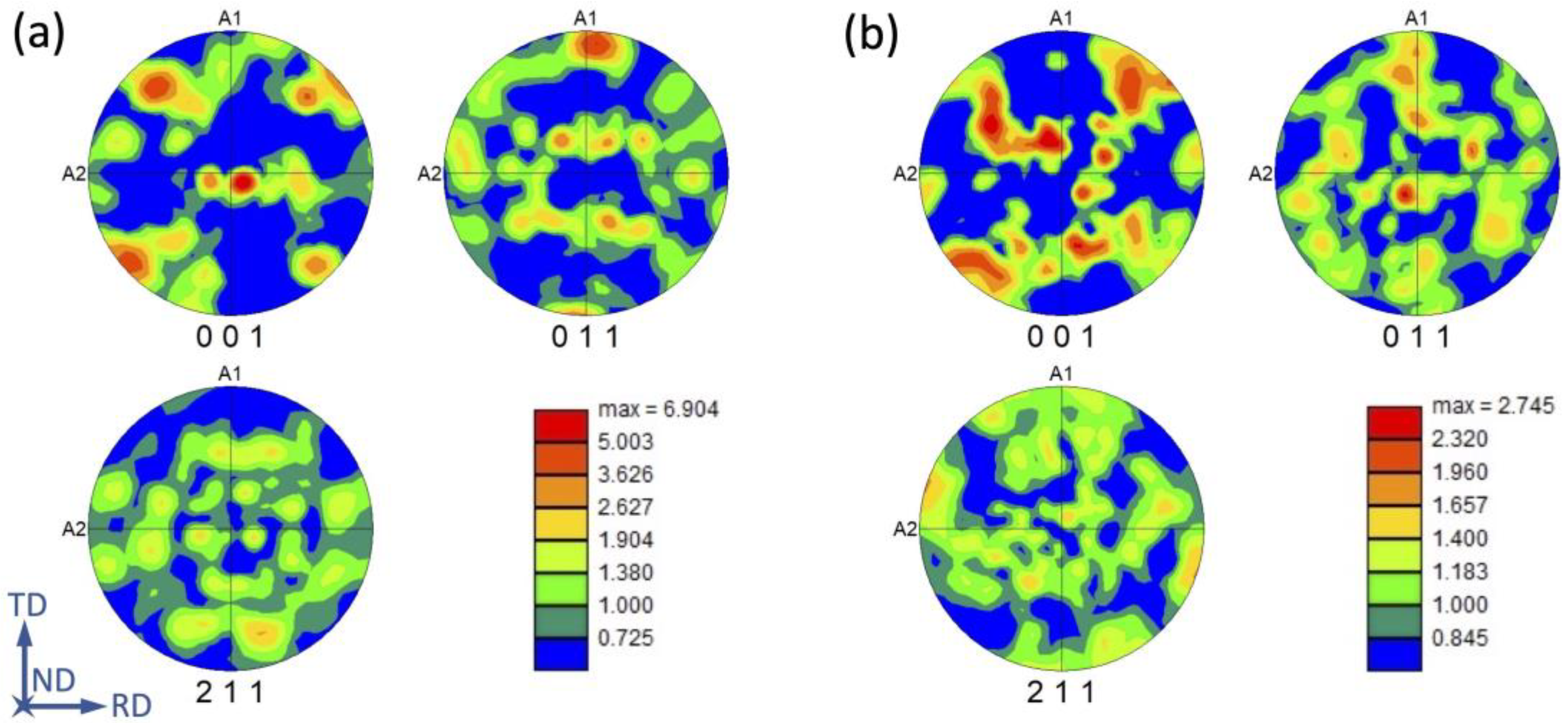
3. Results and Discussion
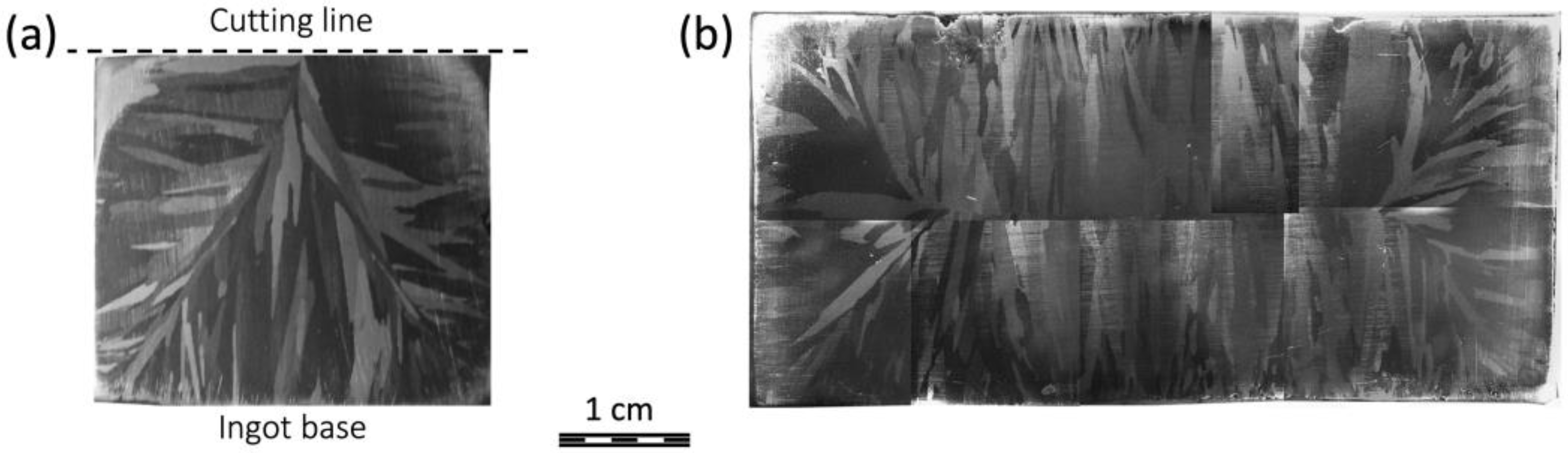
3.1. As-Cast Microstructure of Prismatic Ingot
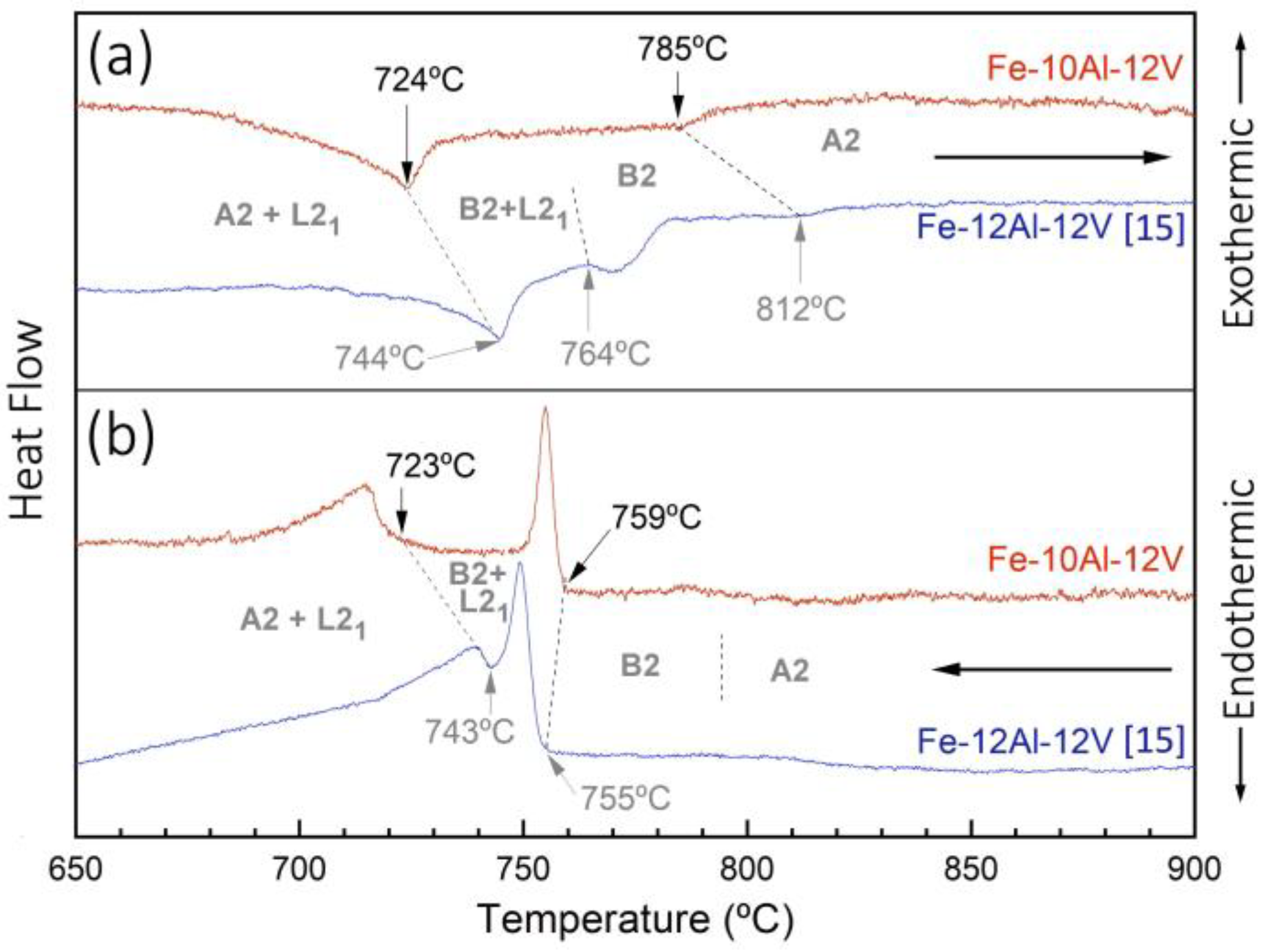
3.2. Phase Transitions
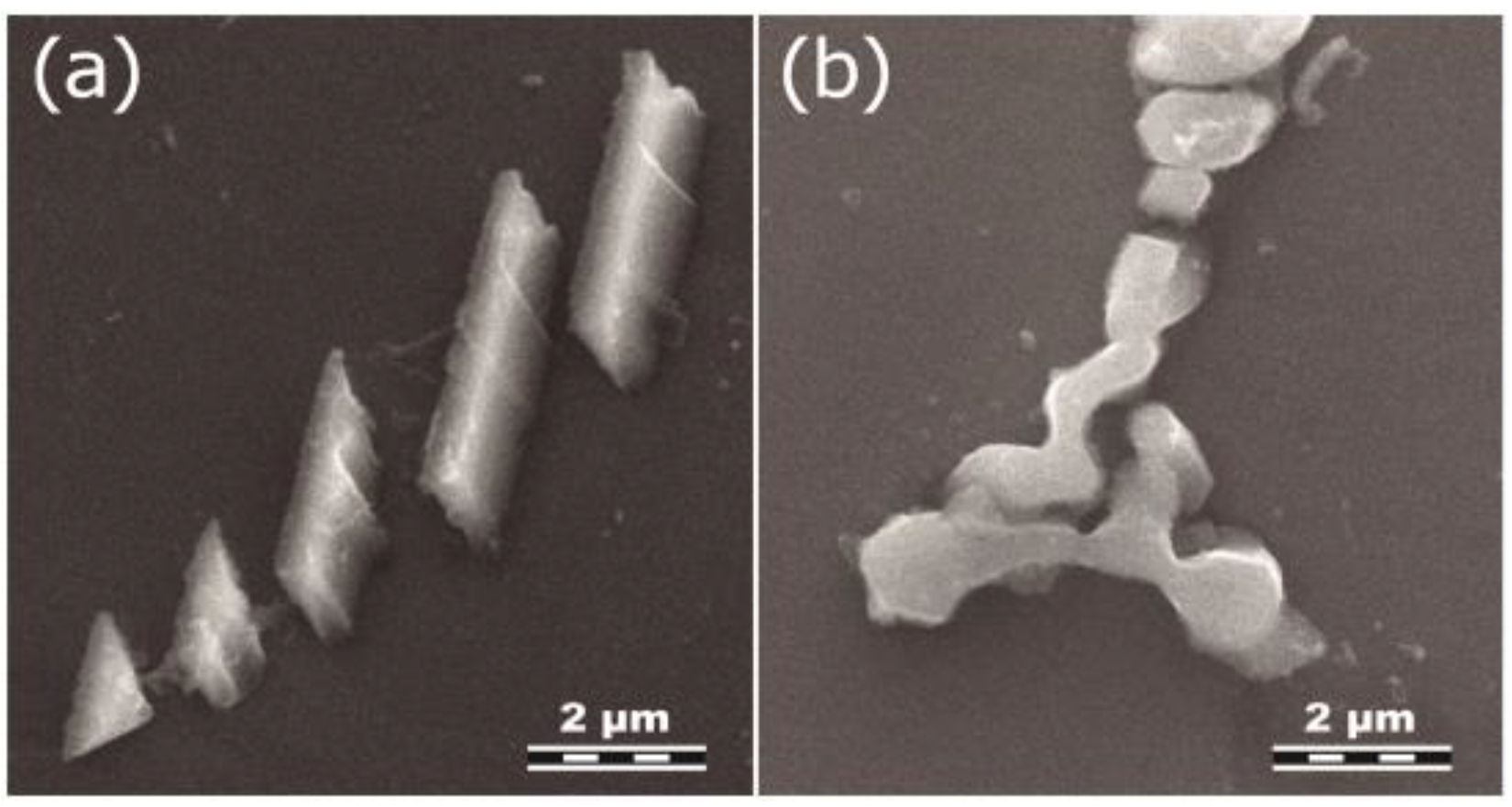
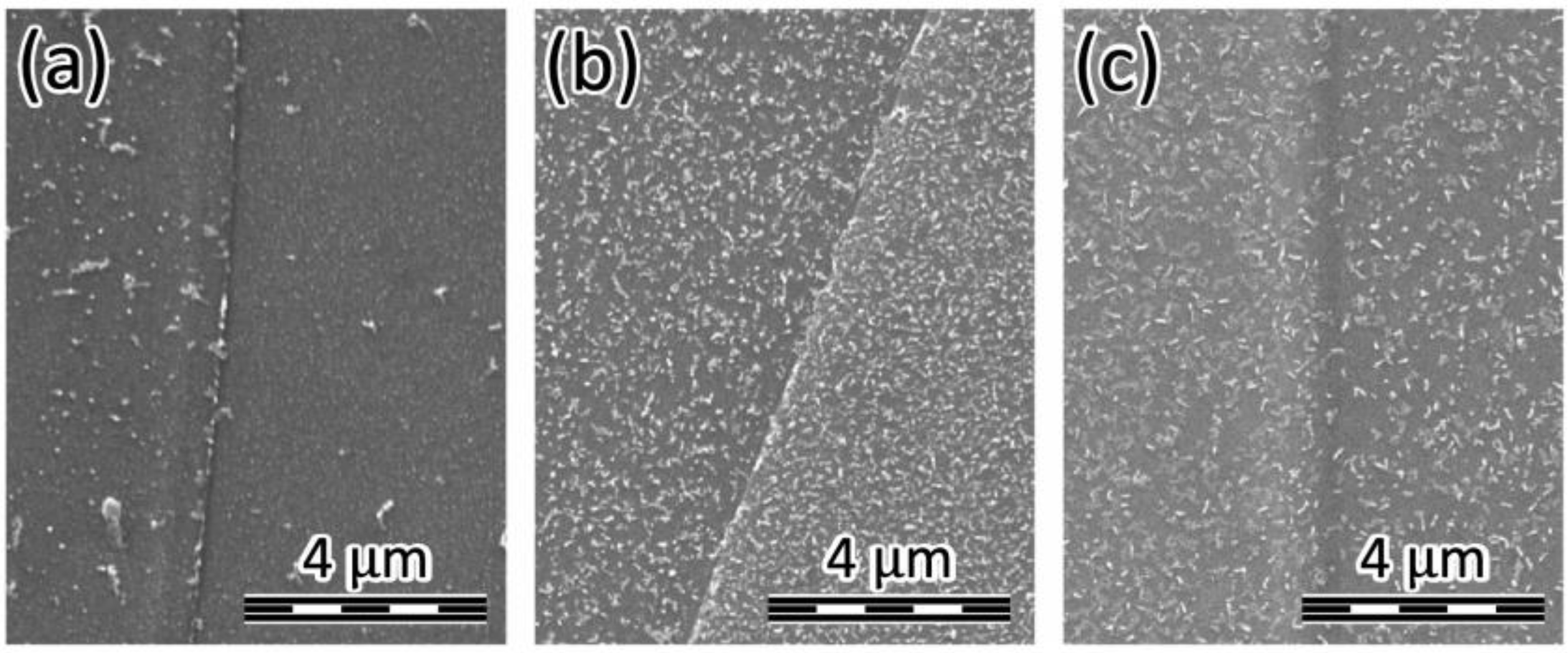
3.3. Carbide Conditioning Treatments
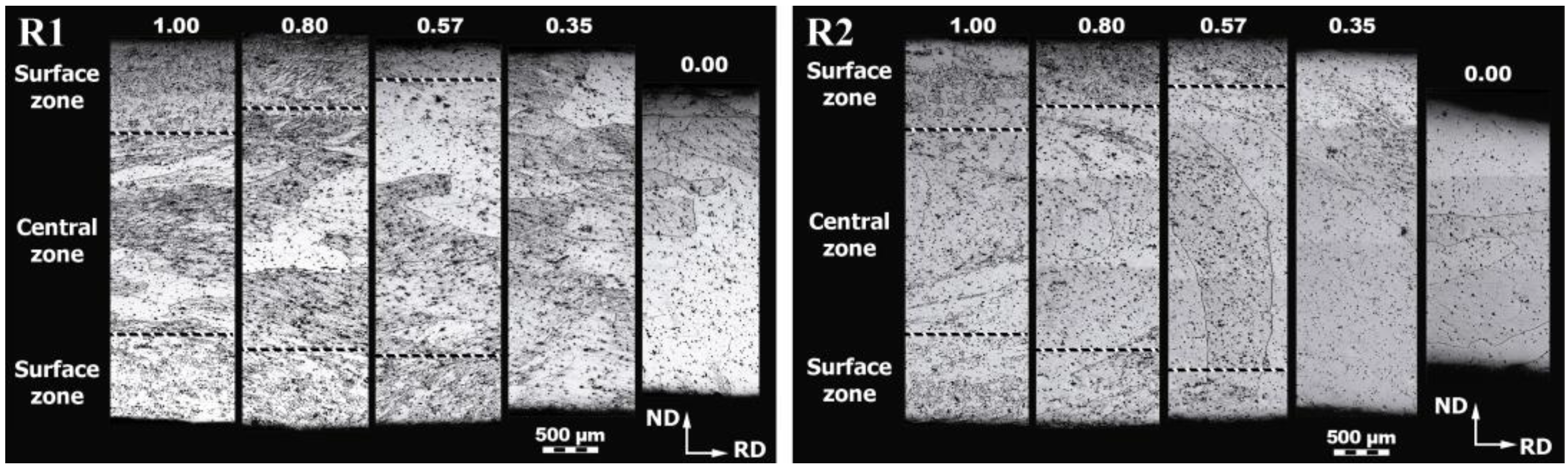
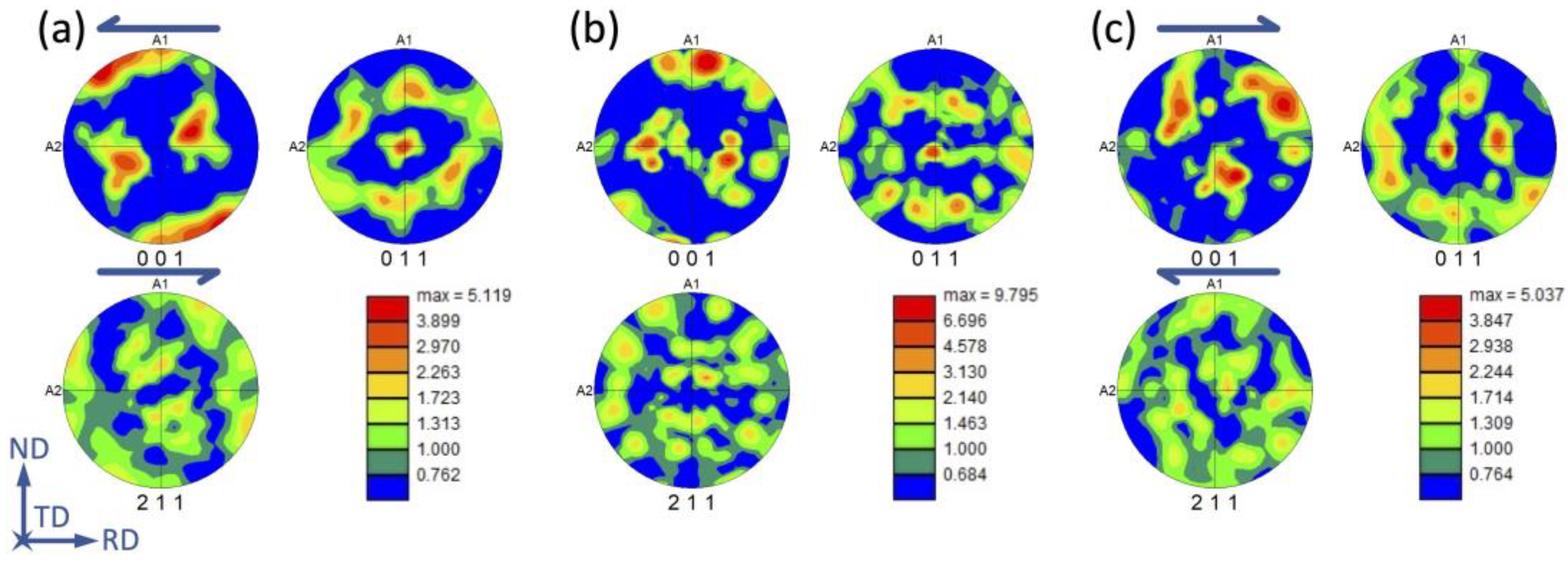
3.4. Hot Rolling and Annealing of Stepped Samples
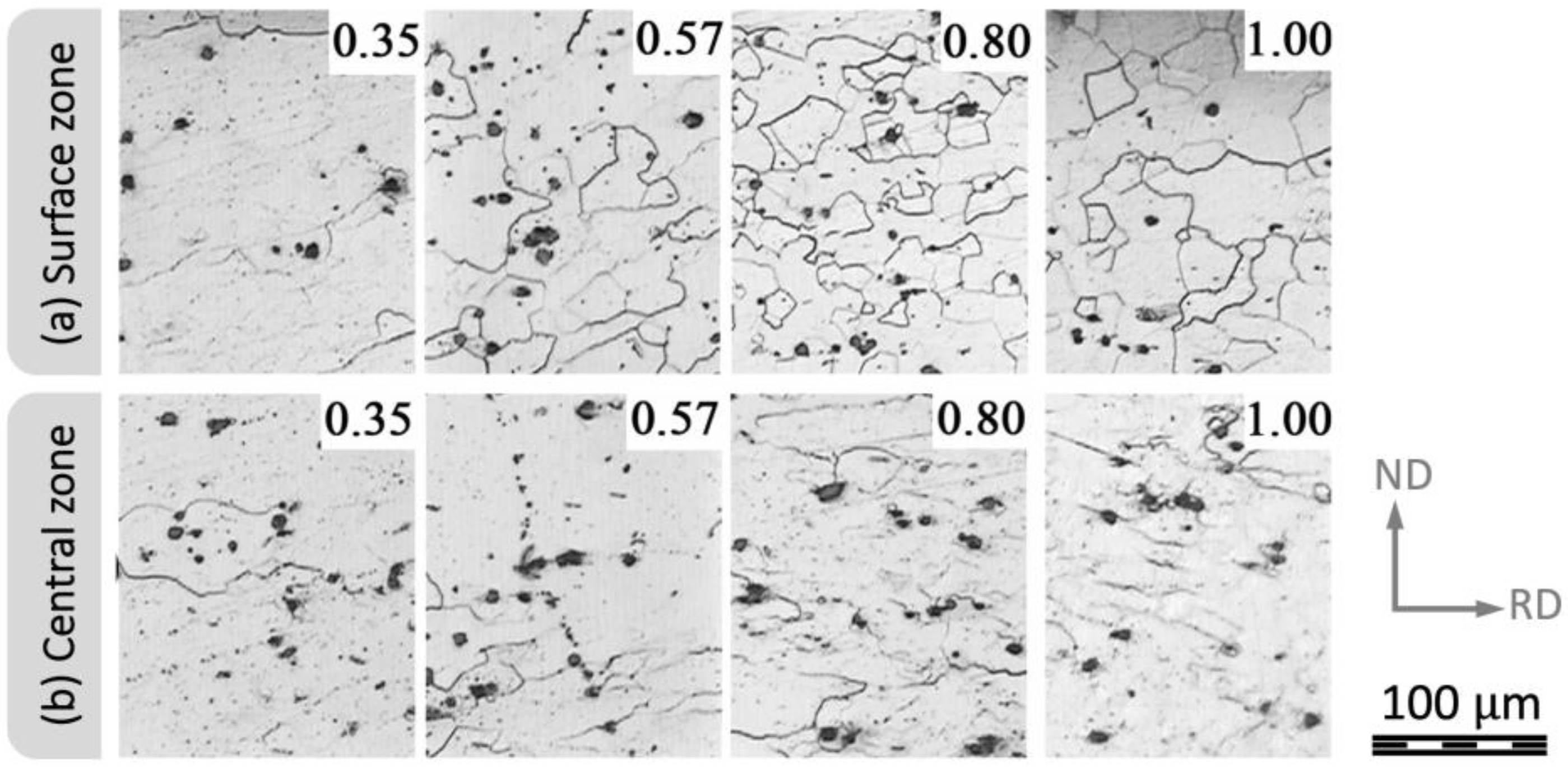
3.5. Grain Distribution and Restoration Mechanisms
3.6. Thermomechanical Processing in the Cylindrical Ingot
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Reed, R.C.; Rae, C.M.F. 22—Physical Metallurgy of the Nickel-Based Superalloys. In Physical Metallurgy, 5th ed.; Laughlin, D.E., Hono, K., Eds.; Elsevier: Oxford, UK, 2014; pp. 2215–2290. ISBN 978-0-444-53770-6. [Google Scholar]
- Knowles, A.J.; Dye, D.; Dodds, R.J.; Watson, A.; Hardie, C.D.; Humphry-Baker, S.A. Tungsten-Based Bcc-Superalloys. Appl. Mater. Today 2021, 23, 101014. [Google Scholar] [CrossRef]
- Morales, L.A.; Luo, N.; Li, K.; Zenk, C.H.; Körner, C. On Stabilizing an α/α′/α″ Microstructure in Ferritic Superalloys. J. Alloys Compd. 2022, 911, 164996. [Google Scholar] [CrossRef]
- Pickering, E.J.; Christofidou, K.A.; Stone, H.J.; Jones, N.G. On the Design and Feasibility of Tantalum-Base Superalloys. J. Alloys Compd. 2019, 804, 314–321. [Google Scholar] [CrossRef]
- Greer, J.R.; Weinberger, C.R.; Cai, W. Comparing the Strength of f.c.c. and b.c.c. Sub-Micrometer Pillars: Compression Experiments and Dislocation Dynamics Simulations. Mater. Sci. Eng. A 2008, 493, 21–25. [Google Scholar] [CrossRef]
- Ryu, I.; Nix, W.D.; Cai, W. Plasticity of Bcc Micropillars Controlled by Competition between Dislocation Multiplication and Depletion. Acta Mater. 2013, 61, 3233–3241. [Google Scholar] [CrossRef]
- Aragon, N.K.; Yin, S.; Lim, H.; Ryu, I. Temperature Dependent Plasticity in BCC Micropillars. Materialia 2021, 19, 101181. [Google Scholar] [CrossRef]
- Han, J.J.; Wang, C.P.; Liu, X.J. A Modified Model to Predict Self-Diffusion Coefficients in Metastable Fcc, Bcc and Hcp Structures. J. Phase Equilib. Diffus. 2013, 34, 17–24. [Google Scholar] [CrossRef]
- Miracle, D.B.; Tsai, M.-H.; Senkov, O.N.; Soni, V.; Banerjee, R. Refractory High Entropy Superalloys (RSAs). Scr. Mater. 2020, 187, 445–452. [Google Scholar] [CrossRef]
- Couzinié, J.-P.; Heczko, M.; Mazánová, V.; Senkov, O.N.; Ghazisaeidi, M.; Banerjee, R.; Mills, M.J. High-Temperature Deformation Mechanisms in a BCC+B2 Refractory Complex Concentrated Alloy. Acta Mater. 2022, 233, 117995. [Google Scholar] [CrossRef]
- Li, X.; Li, W.; Irving, D.L.; Varga, L.K.; Vitos, L.; Schönecker, S. Ductile and Brittle Crack-Tip Response in Equimolar Refractory High-Entropy Alloys. Acta Mater. 2020, 189, 174–187. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Gargano, P.H.; Bozzano, P.B.; Troiani, H.E.; Baruj, A.; Rubiolo, G.H. Characterization of Microstructures and Age Hardening of Fe1−2xAlxVx Alloys. Intermetallics 2014, 50, 65–78. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Rubiolo, G.H. Coarsening Process and Precipitation Hardening in Fe2AlV-Strengthened Ferritic Fe76Al12V12 Alloy. Mater. Sci. Eng. A 2017, 684, 394–405. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Rubiolo, G.H. A Whole Pattern Iterative Refinement Method for Powder X-Ray Diffraction Spectra of Two-Phase Coherent Alloys. J. Mater. Sci. 2018, 53, 2802–2811. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Rubiolo, G.H. Effect of Ti Additions on Phase Transitions, Lattice Misfit, Coarsening, and Hardening Mechanisms in a Fe2AlV-Strengthened Ferritic Alloy. J. Alloys Compd. 2019, 806, 683–697. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Gomez, G.R.; Rubiolo, G.H. Impact Toughness Transition Temperature of Ferritic Fe-Al-V Alloy with Strengthening Fe2AlV Precipitates. Mater. Sci. Eng. A 2017, 706, 136–141. [Google Scholar] [CrossRef]
- Cahn, R.W. CHAPTER 28—Recovery and Recrystallization. In Physical Metallurgy, 4th ed.; Cahn, R.W., Haasen, P., Eds.; North-Holland: Oxford, UK, 1996; pp. 2399–2500. ISBN 978-0-444-89875-3. [Google Scholar]
- Murty, S.V.S.N.; Torizuka, S.; Nagai, K.; Kitai, T.; Kogo, Y. Dynamic Recrystallization of Ferrite during Warm Deformation of Ultrafine Grained Ultra-Low Carbon Steel. Scr. Mater. 2005, 53, 763–768. [Google Scholar] [CrossRef]
- Doherty, R.D.; Hughes, D.A.; Humphreys, F.J.; Jonas, J.J.; Jensen, D.J.; Kassner, M.E.; King, W.E.; McNelley, T.R.; McQueen, H.J.; Rollett, A.D. Current Issues in Recrystallization: A Review. Mater. Sci. Eng. A 1997, 238, 219–274. [Google Scholar] [CrossRef]
- Lombry, R.; Rossard, C.; Thomas, B.J. Étude des mécanismes de déformation et d’adoucissement dynamique des aciers ferritiques Fe-Cr au cours de la déformation à chaud. Rev. Met. Paris 1981, 78, 975–988. [Google Scholar] [CrossRef]
- Belyakov, A.; Kimura, Y.; Tsuzaki, K. Recovery and Recrystallization in Ferritic Stainless Steel after Large Strain Deformation. Mater. Sci. Eng. A 2005, 403, 249–259. [Google Scholar] [CrossRef]
- Castan, C.; Montheillet, F.; Perlade, A. Dynamic Recrystallization Mechanisms of an Fe–8% Al Low Density Steel under Hot Rolling Conditions. Scr. Mater. 2013, 68, 360–364. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Sterin, U.A.; Alonso, P.R.; Knowles, A.J.; Rubiolo, G.H. Influence of Precipitate and Grain Sizes on the Brittle-to-Ductile Transition in Fe–Al–V Bcc-L21 Ferritic Superalloys. Mater. Sci. Eng. A 2022, 856, 144031. [Google Scholar] [CrossRef]
- Ferreirós, P.A. Propiedades Mecánicas de La Aleación Ferrítica Fe76Al12V12 Endurecida Por Precipitación Coherente Fe2AlV (L21). Ph.D. Thesis, Instituto Sabato, Buenos Aires, Argentina, 2016. [Google Scholar]
- Holman, J.P. Heat Transfer, 10th ed.; McGraw-Hill: New York, NY, USA, 2009. [Google Scholar]
- Properties and Selection: Irons, Steels, and High-Performance Alloys, 10th ed.; ASM Handbook; ASM International: Materials Park, OH, USA, 1990; Volume 1, ISBN 978-0-87170-377-4.
- Qi, J.B.; Kang, K.; Hu, Z.Y.; Jin, Z.L.; Ren, H.P. Application of Transient Nonlinear Thermal Analysis on Quenching of Hot Die Steel. IOP Conf. Ser. Mater. Sci. Eng. 2019, 657, 012073. [Google Scholar] [CrossRef]
- Ventola, L.; Chiavazzo, E.; Calignano, F.; Manfredi, D.; Asinari, P. Heat Transfer Enhancement by Finned Heat Sinks with Micro-Structured Roughness. J. Phys. Conf. Ser. 2014, 494, 012009. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Gargano, P.H.; Bozzano, P.B.; Troiani, H.E.; Rubiolo, G.H. Phase Transformations in Fe1-2xAlxVx (X ≤ 0.15) Alloys. Procedia Mater. Sci. 2015, 8, 994–1003. [Google Scholar] [CrossRef]
- Deevi, S.C.; Sikka, V.K. Nickel and Iron Aluminides: An Overview on Properties, Processing, and Applications. Intermetallics 1996, 4, 357–375. [Google Scholar] [CrossRef]
- Peng, L.-Z.; Jiang, Z.-H.; Geng, X. Reaction Mechanisms between Molten CaF2-Based Slags and Molten 9CrMoCoB Steel. Int. J. Miner. Metall. Mater. 2020, 27, 611–619. [Google Scholar] [CrossRef]
- Rao, V.S. Some Observations on the Hydrogen Embrittlement of Fe3Al–Fe3AlC Intermetallic Compounds. Mater. Res. Bull. 2004, 39, 169–174. [Google Scholar] [CrossRef]
- Ghosh, A. Segregation in Cast Products. Sadhana 2001, 26, 5–24. [Google Scholar] [CrossRef]
- Maehara, Y.; Nakai, K.; Yasumoto, K.; Mishima, T. Hot Cracking of Low Alloy Steels in Simulated Continuous Casting-Direct Rolling Process. Trans. Iron Steel Inst. Jpn. 1988, 28, 1021–1027. [Google Scholar] [CrossRef]
- Yoo, K.B.; Kim, J.H.; Heo, N.H. Impurities Segregation to Grain Boundary Carbide Interfaces and Grain Boundaries and the Mechanism of Elevated Temperature Intergranular Cracking in Heat-Resistant Steel. ISIJ Int. 2010, 50, 1702–1706. [Google Scholar] [CrossRef]
- Joseph, C.; Hörnqvist, M.; Brommesson, R.; Persson, C. Influence of Carbide Distribution on Ductility of Haynes®282® Forgings. In 13th International Symposium on Superalloys, SUPERALLOYS 2016, United States; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2016; pp. 523–529. [Google Scholar] [CrossRef]
- Sakai, T.; Saito, Y.; Hirano, K.; Kato, K. Deformation and Recrystallization Behavior of Low Carbon Steel in High Speed Hot Rolling. Trans. Iron Steel Inst. Jpn. 1988, 28, 1028–1035. [Google Scholar] [CrossRef]
- Wang, R.; Bao, Y.; Yan, Z.; Li, D.; Kang, Y. Comparison between the Surface Defects Caused by Al2O3 and TiN Inclusions in Interstitial-Free Steel Auto Sheets. Int. J. Min. Met. Mater. 2019, 26, 178–185. [Google Scholar] [CrossRef]
- Wang, R.; Bao, Y.; Li, Y.; Yan, Z.; Li, D.; Kang, Y. Influence of Metallurgical Processing Parameters on Defects in Cold-Rolled Steel Sheet Caused by Inclusions. Int. J. Min. Met. Mater. 2019, 26, 440–446. [Google Scholar] [CrossRef]
- Wang, H.; Bao, Y.; Zhao, M.; Wang, M.; Yuan, X.; Gao, S. Effect of Ce on the Cleanliness, Microstructure and Mechanical Properties of High Strength Low Alloy Steel Q690E in Industrial Production Process. Int. J. Min. Met. Mater. 2019, 26, 1372–1384. [Google Scholar] [CrossRef]
- Yang, L.; Cheng, G. Characteristics of Al2O3, MnS, and TiN Inclusions in the Remelting Process of Bearing Steel. Int. J. Min. Met. Mater. 2017, 24, 869–875. [Google Scholar] [CrossRef]
- Bai, X.; Sun, Y.; Chen, R.; Zhang, Y.; Cai, Y. Formation and Thermodynamics of CaS-Bearing Inclusions during Ca Treatment in Oil Casting Steels. Int. J. Min. Met. Mater. 2019, 26, 573–587. [Google Scholar] [CrossRef]
- Couchet, C.; Allain, S.Y.P.; Geandier, G.; Teixeira, J.; Gaudez, S.; Macchi, J.; Lamari, M.; Bonnet, F. Recovery of Severely Deformed Ferrite Studied by in Situ High Energy X-Ray Diffraction. Mater. Charact. 2021, 179, 111378. [Google Scholar] [CrossRef]
- Bergmann, R.B.; Bill, A. On the Origin of Logarithmic-Normal Distributions: An Analytical Derivation, and Its Application to Nucleation and Growth Processes. J. Cryst. Growth 2008, 310, 3135–3138. [Google Scholar] [CrossRef]
- Pande, C.S.; Dantsker, E. On a Stochastic Theory of Grain Growth—II. Acta Metall. Mater. 1990, 38, 945–951. [Google Scholar] [CrossRef]
- Wang, C.; Liu, G. On the Stability of Grain Structure with Initial Weibull Grain Size Distribution. Mater. Lett. 2003, 57, 4424–4428. [Google Scholar] [CrossRef]
- Fayad, W.; Thompson, C.V.; Frost, H.J. Steady-State Grain-Size Distributions Resulting from Grain Growth in Two Dimensions. Scr. Mater. 1999, 40, 1199–1204. [Google Scholar] [CrossRef]
- Thompson, C.V. Grain Growth and Evolution of Other Cellular Structures. In Solid State Physics; Ehrenreich, H., Spaepen, F., Eds.; Academic Press: Cambridge, MA, USA, 2001; Volume 55, pp. 269–314. [Google Scholar]
- Pande, C.S. A Possible Model of Grain Size Distribution during Primary Recrystallization. Model. Simul. Mater. Sci. Eng. 2015, 23, 035009. [Google Scholar] [CrossRef]
- Humphreys, F.J. Review Grain and Subgrain Characterisation by Electron Backscatter Diffraction. J. Mater. Sci. 2001, 36, 3833–3854. [Google Scholar] [CrossRef]
- Dziaszyk, S.; Payton, E.J.; Friedel, F.; Marx, V.; Eggeler, G. On the Characterization of Recrystallized Fraction Using Electron Backscatter Diffraction: A Direct Comparison to Local Hardness in an IF Steel Using Nanoindentation. Mater. Sci. Eng. A 2010, 527, 7854–7864. [Google Scholar] [CrossRef]
- Cruz-Gandarilla, F.; Bolmaro, R.E.; Mendoza-León, H.F.; Salcedo-Garrido, A.M.; Cabañas-Moreno, J.G. Study of Recovery and First Recrystallisation Kinetics in CGO Fe3%Si Steels Using Misorientation-Derived Parameters (EBSD). J. Microsc. 2019, 275, 133–148. [Google Scholar] [CrossRef] [PubMed]
- Mirzadeh, H.; Cabrera, J.M.; Najafizadeh, A.; Calvillo, P.R. EBSD Study of a Hot Deformed Austenitic Stainless Steel. Mater. Sci. Eng. A 2012, 538, 236–245. [Google Scholar] [CrossRef]
- Mccabe, R.J.; Teter, D.F. Analysis of Recrystallized Volume Fractions in Uranium Using Electron Backscatter Diffraction. J. Microsc. 2006, 223, 33–39. [Google Scholar] [CrossRef]
- Winning, M.; Rollett, A.D.; Gottstein, G.; Srolovitz, D.J.; Lim, A.; Shvindlerman, L.S. Mobility of Low-Angle Grain Boundaries in Pure Metals. Philos. Mag. 2010, 90, 3107–3128. [Google Scholar] [CrossRef]
- Ferreirós, P.A.; Alonso, P.R.; Rubiolo, G.H. Hardening and Coherent Precipitates Size Evolution with Aging Fe-12Al-12V Alloy. Procedia Mater. Sci. 2015, 9, 213–220. [Google Scholar] [CrossRef]
- Chamanfar, A.; Valberg, H.S.; Templin, B.; Plumeri, J.E.; Misiolek, W.Z. Development and Validation of a Finite-Element Model for Isothermal Forging of a Nickel-Base Superalloy. Materialia 2019, 6, 100319. [Google Scholar] [CrossRef]
- Wang, M.; Li, D.; Wang, F.; Zang, X.; Li, X.; Xiao, H.; Du, F.; Zhang, F.C.; Jiang, Z. Analysis of Laminated Crack Defect in the Upsetting Process of Heavy Disk-Shaped Forgings. Eng. Fail. Anal. 2016, 59, 197–210. [Google Scholar] [CrossRef]




| Step | Initial Thickness (mm) | Rolled Thickness (mm) | ε | γ (s-1) |
|---|---|---|---|---|
| 1 | 2.50 | 2.50 | 0 | 0 |
| 2 | 4.54 | 3.20 | 0.35 | 20.24 |
| 3 | 5.65 | 3.20 | 0.57 | 24.31 |
| 4 | 7.08 | 3.20 | 0.80 | 26.98 |
| 5 | 8.75 | 3.20 | 1.00 | 28.57 |
| Ingot | Fe | Al | V | Si | Mn | C |
|---|---|---|---|---|---|---|
| Prismatic | 77.1 ± 0.3 | 10.2 ± 0.2 | 12.1 ± 0.1 | 0.38 ± 0.01 | 0.30 ± 0.01 | 0.26 ± 0.03 |
| Cylindrical | 77.0 ± 0.8 | 10.0 ± 0.5 | 12.2 ± 0.5 | 0.42 ± 0.01 | 0.39 ± 0.01 | 0.27 ± 0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferreirós, P.A.; Becerra, A.A.; Sterin, U.A.; Ávalos, M.C.; Bolmaro, R.E.; Rubiolo, G.H. Microstructure Evolution by Thermomechanical Processing in the Fe-10Al-12V Superalloy. Alloys 2023, 2, 29-43. https://doi.org/10.3390/alloys2010002
Ferreirós PA, Becerra AA, Sterin UA, Ávalos MC, Bolmaro RE, Rubiolo GH. Microstructure Evolution by Thermomechanical Processing in the Fe-10Al-12V Superalloy. Alloys. 2023; 2(1):29-43. https://doi.org/10.3390/alloys2010002
Chicago/Turabian StyleFerreirós, Pedro A., Abraham A. Becerra, Uriel A. Sterin, Martina C. Ávalos, Raúl E. Bolmaro, and Gerardo H. Rubiolo. 2023. "Microstructure Evolution by Thermomechanical Processing in the Fe-10Al-12V Superalloy" Alloys 2, no. 1: 29-43. https://doi.org/10.3390/alloys2010002
APA StyleFerreirós, P. A., Becerra, A. A., Sterin, U. A., Ávalos, M. C., Bolmaro, R. E., & Rubiolo, G. H. (2023). Microstructure Evolution by Thermomechanical Processing in the Fe-10Al-12V Superalloy. Alloys, 2(1), 29-43. https://doi.org/10.3390/alloys2010002