Identification of MEMS Geometric Uncertainties through Homogenization
Abstract
:1. Introduction
2. Asymptotic Homogenization
3. MEMS Filter
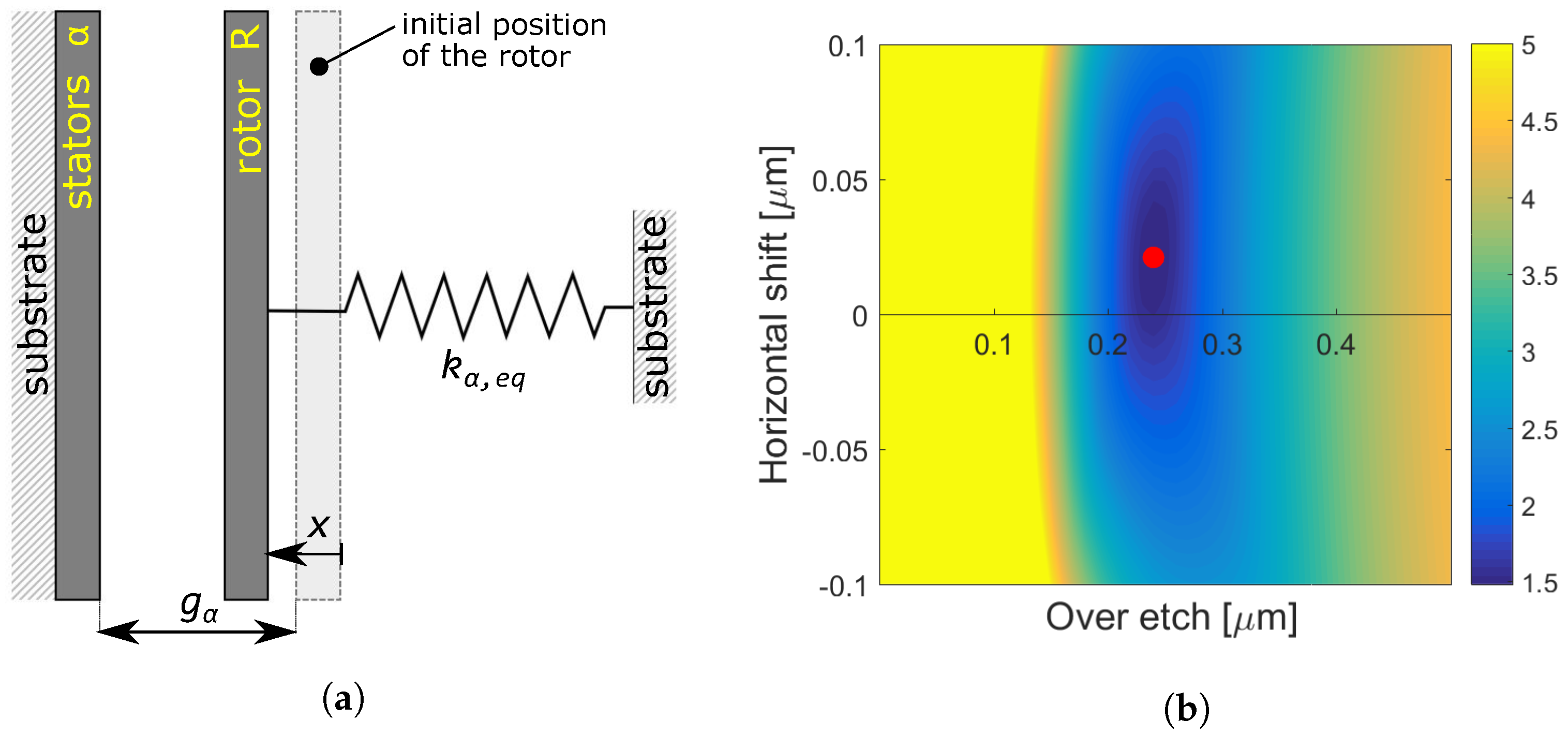
3.1. Mechanical Design
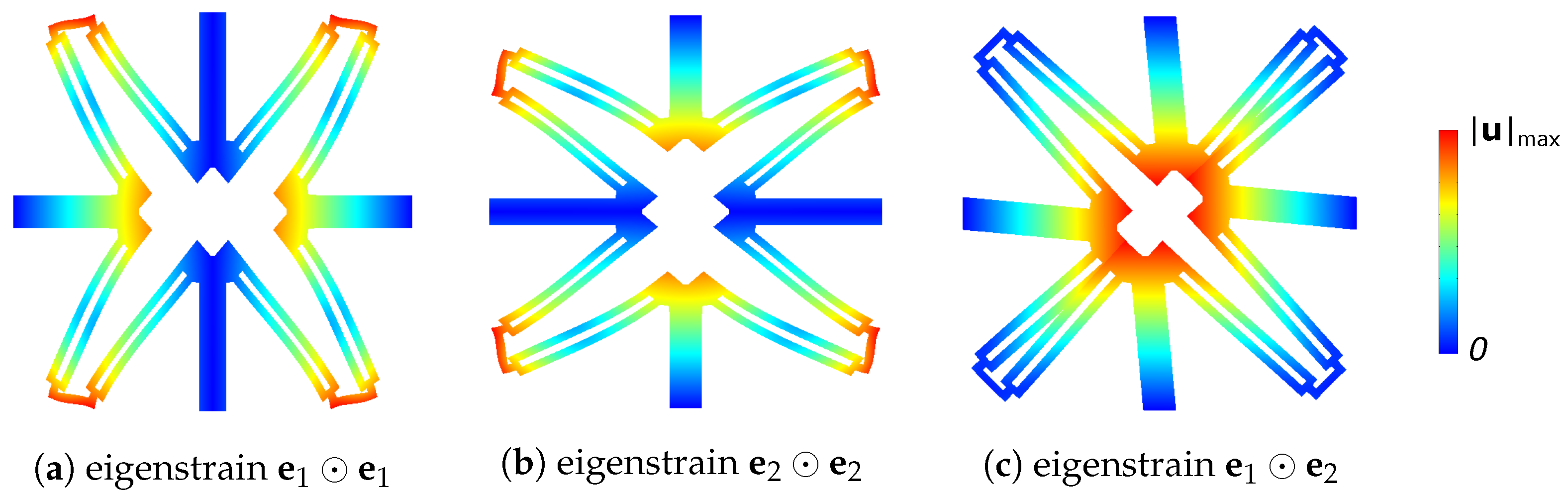
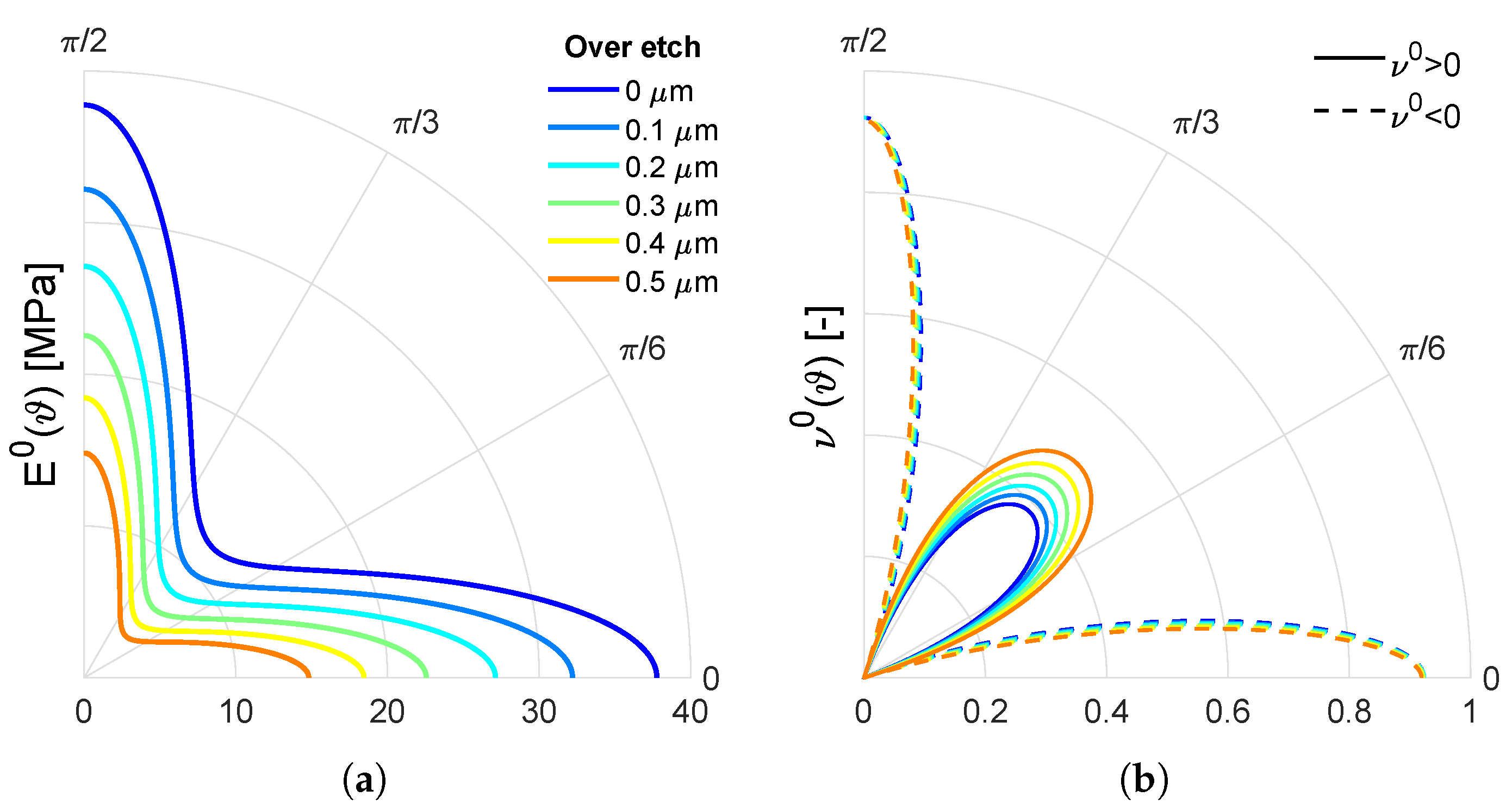
3.2. Homogenization of the Auxetic Core
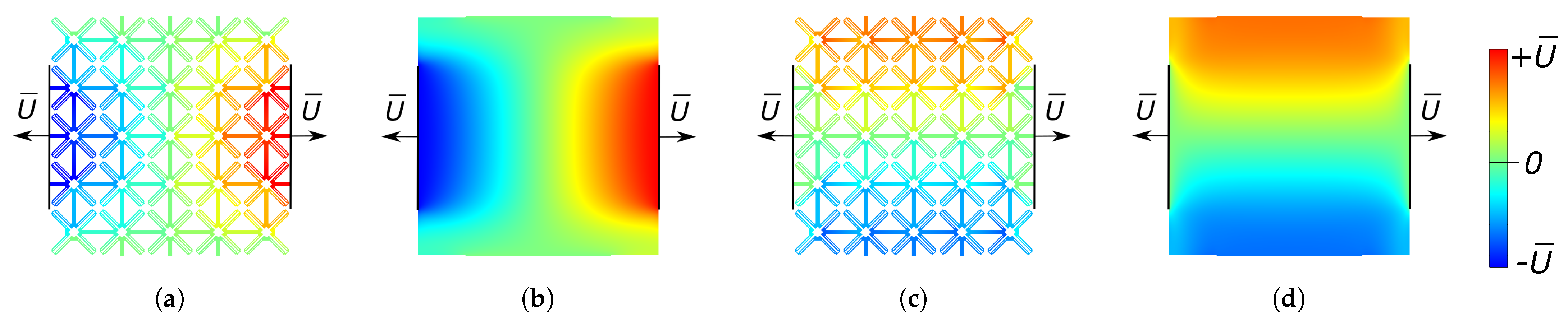
3.3. Validation of Effective Properties
4. Identification of Geometric Uncertainties
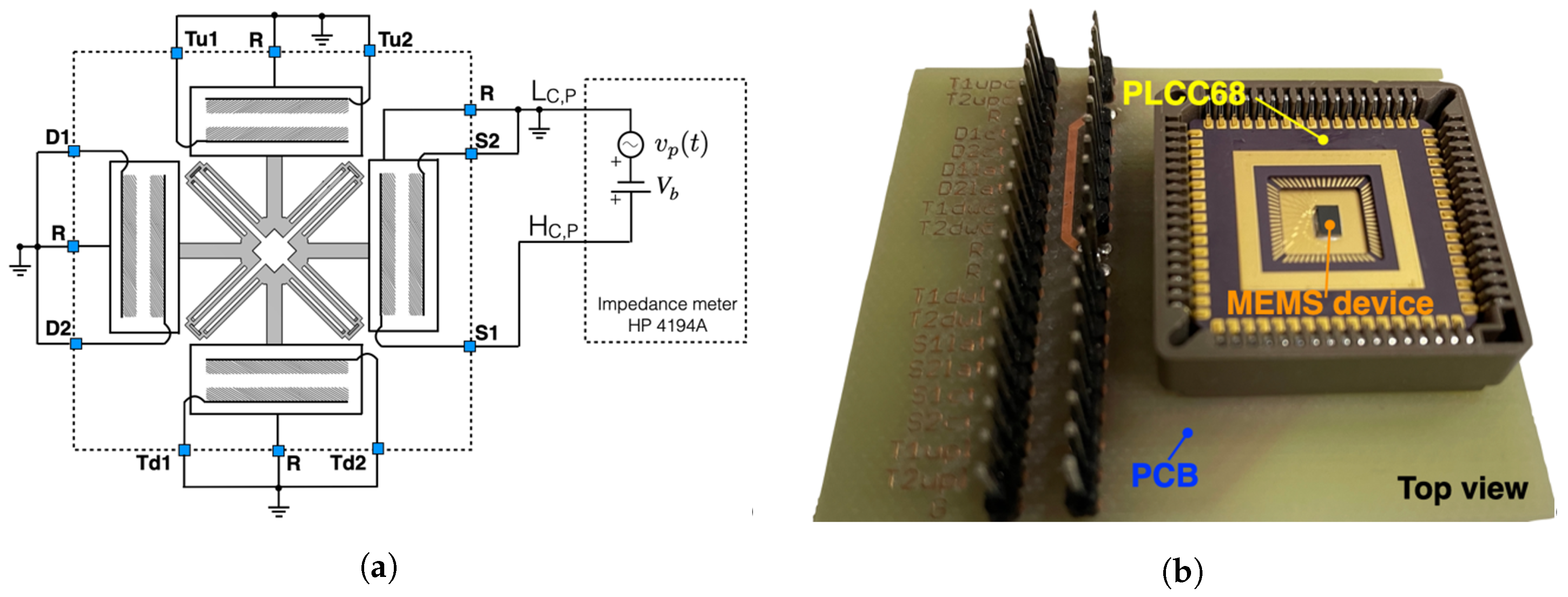
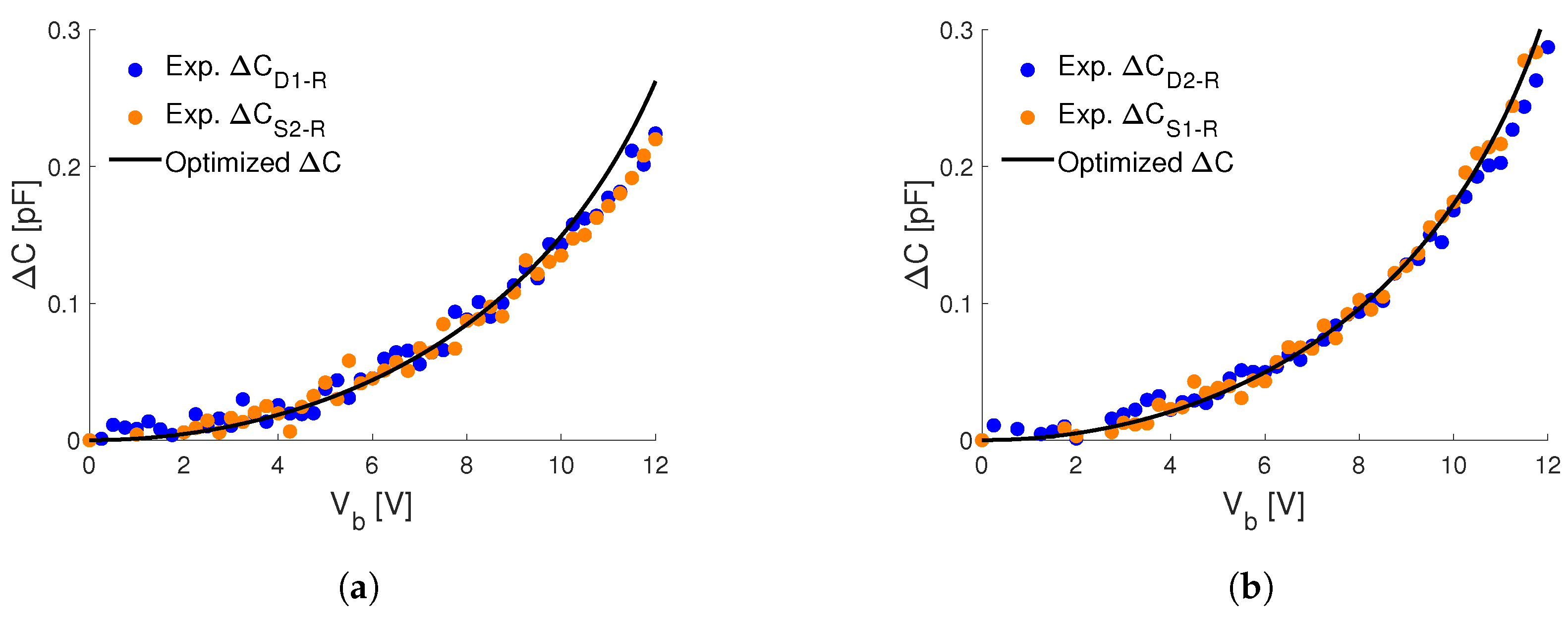
4.1. Experimental Results
4.2. Optimization Procedure
4.3. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FEA | Finite Element Analysis |
| MEMS | Micro-Electro-Mechanical Systems |
| PCB | Printed Circuit Board |
| PLCC68 | 68-Pin Plastic-Leaded-Chip-Carrier |
| SEM | Scanning Electron Microscopy |
References
- Choudhary, V.; Iniewski, K. Mems: Fundamental Technology and Applications; CRC Press: New York, NY, USA, 2017. [Google Scholar]
- Corigliano, A.; Ardito, R.; Comi, C.; Frangi, A.; Ghisi, A.; Mariani, S. Mechanics of Microsystems; Wiley: New York, NY, USA, 2018; ISBN 978-1-119-05383-5. [Google Scholar]
- Vigna, B.; Ferrari, P.; Villa, F.F.; Lasalandra, E.; Zerbini, S. Silicon Sensors and Actuators; Springer: New York, NY, USA, 2022. [Google Scholar]
- Tseng, S.H. CMOS MEMS Design and Fabrication Platform. Front. Mech. Eng. 2022, 8, 894484. [Google Scholar] [CrossRef]
- Williams, K.; Gupta, K.; Wasilik, M. Etch rates for micromachining processing-Part II. J. Microelectromech. Syst. 2003, 12, 761–778. [Google Scholar] [CrossRef]
- Williams, K.; Muller, R. Etch rates for micromachining processing. J. Microelectromech. Syst. 1996, 5, 256–269. [Google Scholar] [CrossRef]
- Hartzell, A.L.; da Silva, M.G.; Shea, H.R. MEMS Reliability; Springer: New York, NY, USA, 2011. [Google Scholar]
- Ghisi, A.; Mariani, S. Effect of Imperfections Due to Material Heterogeneity on the Offset of Polysilicon MEMS Structures. Sensors 2019, 19, 3256. [Google Scholar] [CrossRef]
- Codreanu, I.; Martowicz, A.; Gallina, A.; Pieczonka, L.; Uhl, T. Study of the effect of process induced uncertainties on the performance of a micro-comb resonator. In Proceedings of the 4th Conference Mechatronic Systems and Materials 2008—MSM 2008, Beijing, China, 12–15 October 2008. [Google Scholar]
- Vatanparvar, D.; Shkel, A.M. Effect of fabrication imperfections on energy loss through mechanical mode coupling in MEMS. In Proceedings of the 2018 IEEE International Symposium on Inertial Sensors and Systems (INERTIAL), Moltrasio, Italy, 26–29 March 2018; pp. 1–2. [Google Scholar]
- Karmakar, A.; Biswas, B.; Chauhan, A. Investigation of Various Commonly Associated Imperfections in Radiofrequency Micro-Electro-Mechanical System Devices and its Empirical Modeling. IETE J. Res. 2021, 0, 1–10. [Google Scholar] [CrossRef]
- Uhl, T.; Martowicz, A.; Codreanu, I.; Klepka, A. Analysis of uncertainties in MEMS and their influence on dynamic properties. Arch. Mech. 2009, 61, 349–370. [Google Scholar]
- Nyyssonen, D.; Larrabee, R.D. Submicrometer linewidth metrology in the optical microscope. J. Res. Natl. Bur. Stand. 1987, 92, 187. [Google Scholar] [CrossRef]
- Gennat, M.; Meinig, M.; Shaporin, A.; Kurth, S.; Rembe, C.; Tibken, B. Determination of parameters with uncertainties for quality control in MEMS fabrication. J. Microelectromech. Syst. 2013, 22, 613–624. [Google Scholar] [CrossRef]
- Poreddy, S.; Pai, P.F.; Feng, Z.C. Design Compensation for Fabrication Imperfections of Tuning-Fork Gyroscopes. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2006, 220, 97–103. [Google Scholar] [CrossRef]
- Liu, M.; Maute, K.; Frangopol, D.M. Multi-objective design optimization of electrostatically actuated microbeam resonators with and without parameter uncertainty. Reliab. Eng. Syst. Saf. 2007, 92, 1333–1343. [Google Scholar] [CrossRef]
- Pugno, N.; Peng, B.; Espinosa, H. Predictions of strength in MEMS components with defects—A novel experimental–theoretical approach. Int. J. Solids Struct. 2005, 42, 647–661. [Google Scholar] [CrossRef]
- Fang, X.S.; Myung, N.V.; Nobe, K.; Judy, J.W. Modeling the effect of etch holes on ferromagnetic MEMS. IEEE Trans. Magn. 2001, 37, 2637–2639. [Google Scholar] [CrossRef]
- Ghisi, A.; Geninazzi, M.V.; Mariani, S. Polysilicon MEMS Sensors: Sensitivity to Sub-Micron Imperfections. Proceedings 2019, 4, 35. [Google Scholar]
- Mirzazadeh, R.; Eftekhar Azam, S.; Mariani, S. Micromechanical characterization of polysilicon films through on-chip tests. Sensors 2016, 16, 1191. [Google Scholar] [CrossRef]
- Mirzazadeh, R.; Eftekhar Azam, S.; Mariani, S. Mechanical Characterization of Polysilicon MEMS: A Hybrid TMCMC/POD-Kriging Approach. Sensors 2018, 18, 1243. [Google Scholar] [CrossRef]
- Bakhvalov, N.S.; Panasenko, G. Homogenisation: Averaging Processes in Periodic Media; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1989. [Google Scholar]
- Bensoussan, A.; Lions, J.L.; Papanicolaou, G. Asymptotic Analysis for Periodic Structures; North-Holland Publishing Company: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Auriault, J.L.; Bonnet, G. Dynamique des composites élastiques périodiques. Arch. Mech. 1985, 37, 269–284. [Google Scholar]
- Craster, R.V.; Kaplunov, J.; Pichugin, A.V. High-frequency homogenization for periodic media. Proc. R. Soc. A 2010, 466, 2341–2362. [Google Scholar] [CrossRef]
- Comi, C.; Marigo, J.J. Homogenization Approach and Bloch-Floquet Theory for Band-Gap Prediction in 2D Locally Resonant Metamaterials. J. Elast. 2020, 139, 61–90. [Google Scholar] [CrossRef]
- Faraci, D.; Comi, C.; Marigo, J.J. Band Gaps in Metamaterial Plates: Asymptotic Homogenization and Bloch-Floquet Approaches. J. Elast. 2022, 148, 55–79. [Google Scholar] [CrossRef]
- Zega, V.; Nastro, A.; Ferrari, M.; Ardito, R.; Ferrari, V.; Corigliano, A. An Innovative Auxetic Electrically-Tunable Mems Mechanical Filter. In Proceedings of the 2022 IEEE 35th International Conference on Micro Electro Mechanical Systems Conference (MEMS), Tokyo, Japan, 9–13 January 2022; pp. 539–542. [Google Scholar]
- Zega, V.; Nastro, A.; Ferrari, M.; Ardito, R.; Ferrari, V.; Corigliano, A. Design, fabrication and experimental validation of a MEMS periodic auxetic structure. Smart Mater. Struct. 2019, 28, 095011. [Google Scholar] [CrossRef]
- Nordmann, J.; Aßmus, M.; Altenbach, H. Visualising elastic anisotropy: Theoretical background and computational implementation. Contin. Mech. Thermodyn. 2018, 30, 689–708. [Google Scholar] [CrossRef]
- Faraci, D.; Nastro, A.; Zega, V.; Comi, C. Two-scale asymptotic homogenization in a MEMS auxetic structure for over etch identification. In Proceedings of the ECCOMAS 2022, Oslo, Norway, 5–9 June 2022; pp. 1–10. [Google Scholar]
- Corigliano, A.; De Masi, B.; Frangi, A.; Comi, C.; Villa, A.; Marchi, M. Mechanical characterization of polysilicon through on-chip tensile tests. J. Microelectromech. Syst. 2004, 13, 200–219. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Faraci, D.; Zega, V.; Nastro, A.; Comi, C. Identification of MEMS Geometric Uncertainties through Homogenization. Micro 2022, 2, 564-574. https://doi.org/10.3390/micro2040037
Faraci D, Zega V, Nastro A, Comi C. Identification of MEMS Geometric Uncertainties through Homogenization. Micro. 2022; 2(4):564-574. https://doi.org/10.3390/micro2040037
Chicago/Turabian StyleFaraci, David, Valentina Zega, Alessandro Nastro, and Claudia Comi. 2022. "Identification of MEMS Geometric Uncertainties through Homogenization" Micro 2, no. 4: 564-574. https://doi.org/10.3390/micro2040037
APA StyleFaraci, D., Zega, V., Nastro, A., & Comi, C. (2022). Identification of MEMS Geometric Uncertainties through Homogenization. Micro, 2(4), 564-574. https://doi.org/10.3390/micro2040037







