FE-Simulation and Experimental Characterisation of Environmental Effects on the Diffusion and Mechanical Performance of Hyperelastic Adhesive Joints
Abstract
:1. Introduction
2. Materials and Methods
2.1. Adhesives and Substrates
- Polyurethane adhesives (PUs);
- Silane modified polymers (SMPs).
2.2. Adhesive Joints
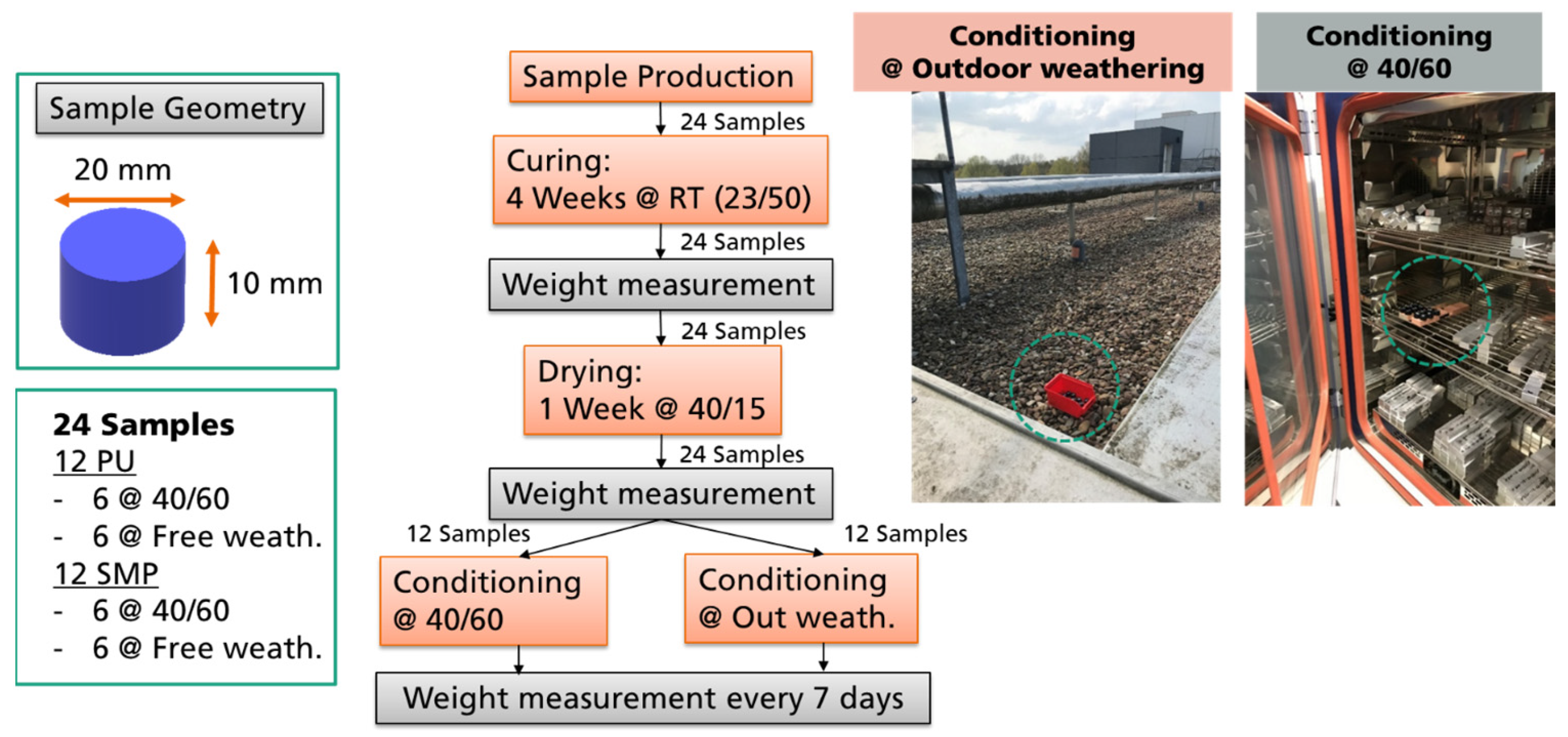
2.3. Sample Manufacturing
2.4. Geometry of Samples
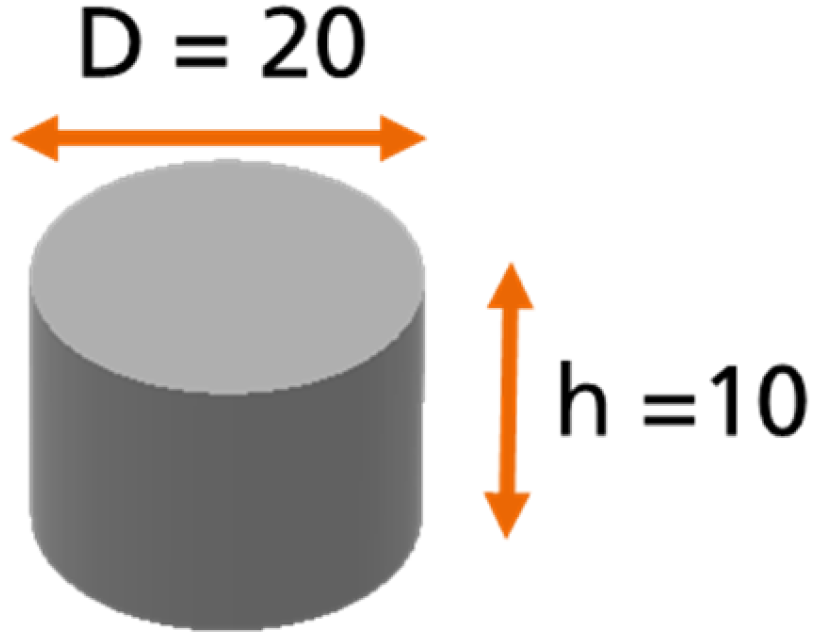
2.4.1. Pure Adhesive Geometry-Coin Sample
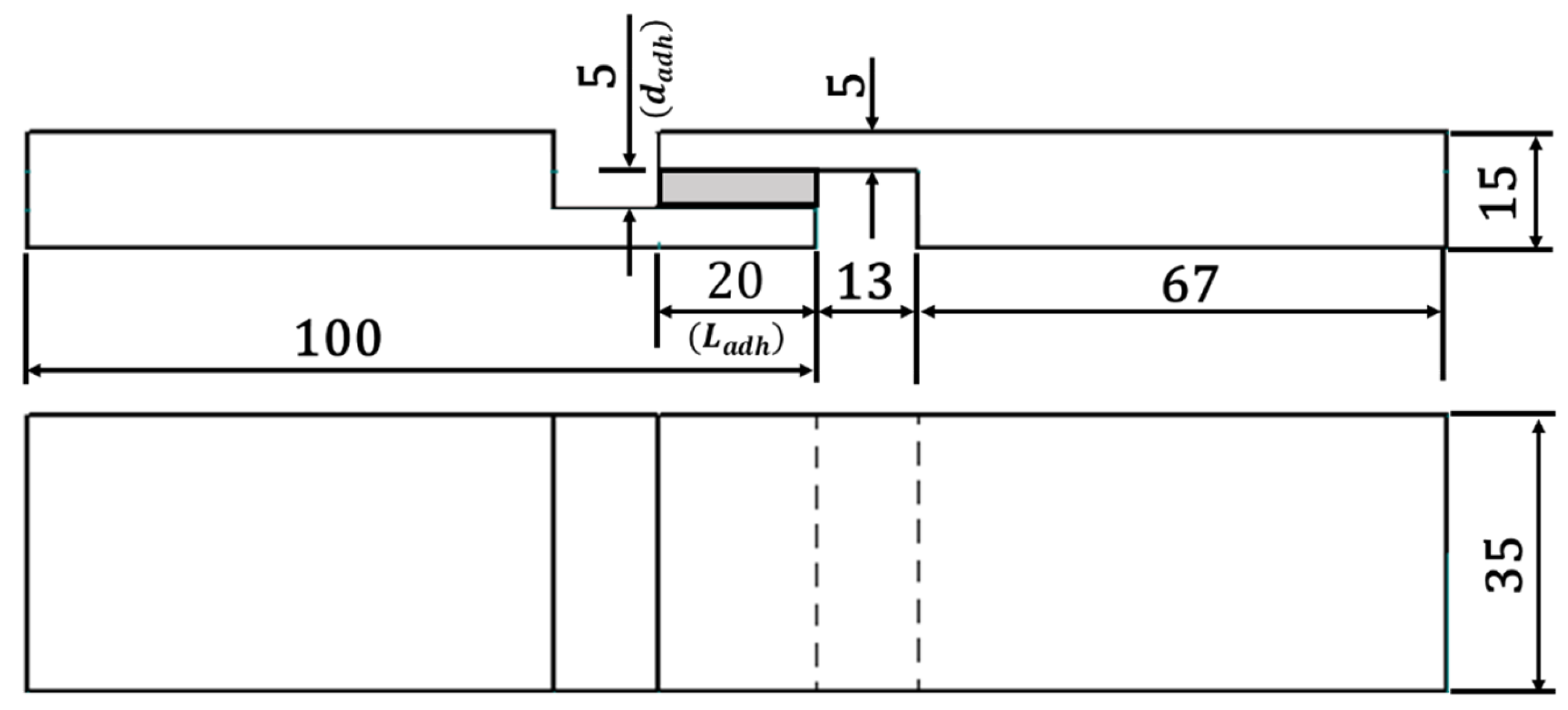
2.4.2. Adhesively Bonded Joint Geometry—TAST Sample
2.5. Diffusion Experiments
2.6. Mechanical Characterisation of Environmental Effects
3. Finite Element Analysis—Diffusion and Weight
3.1. Implementation
3.2. Simulation Parameters of Conditioning in the Climatic Chamber
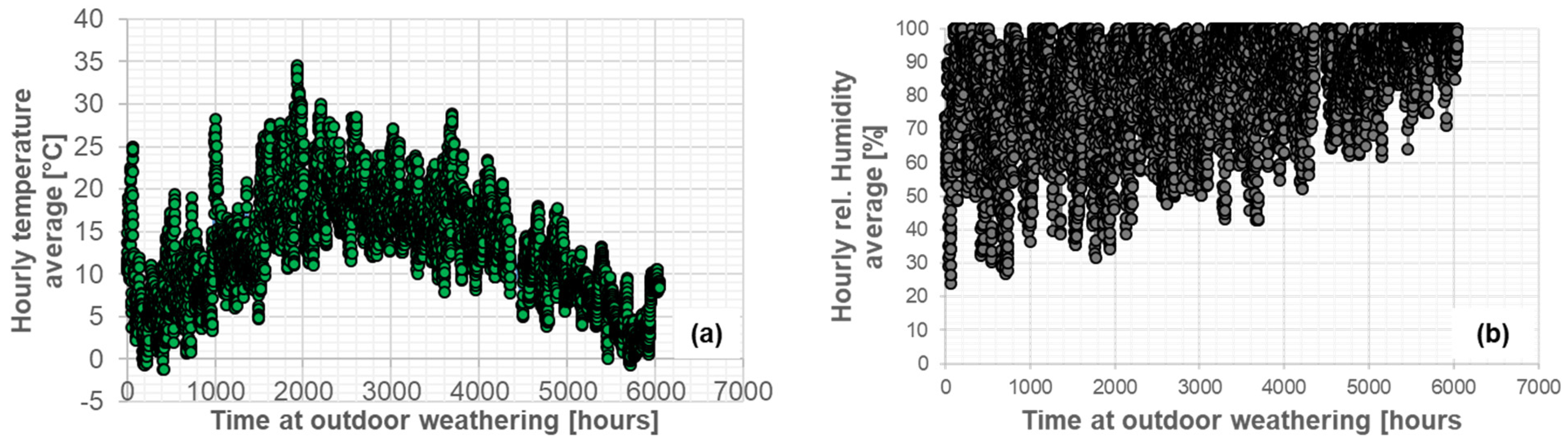
3.3. Simulation Parameters of Conditioning under Outdoor Weathering
4. Diffusion Results
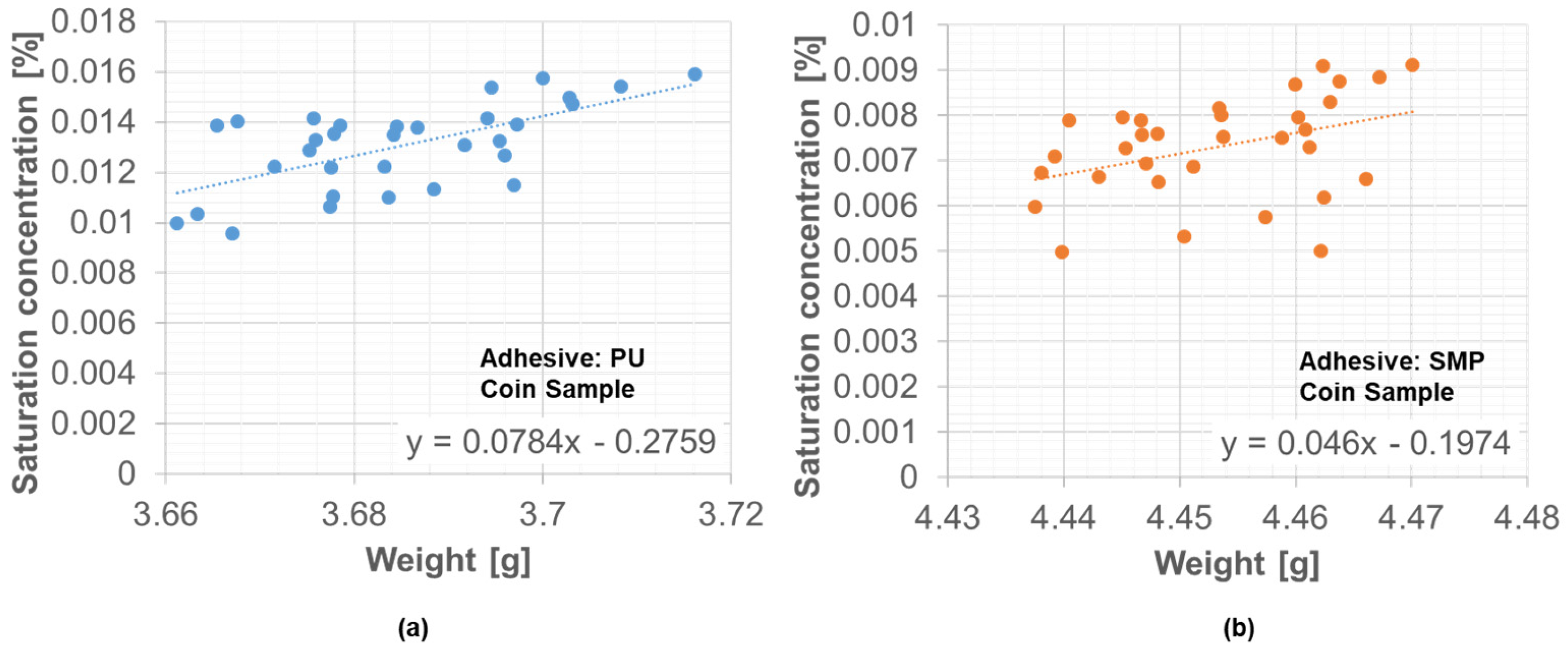
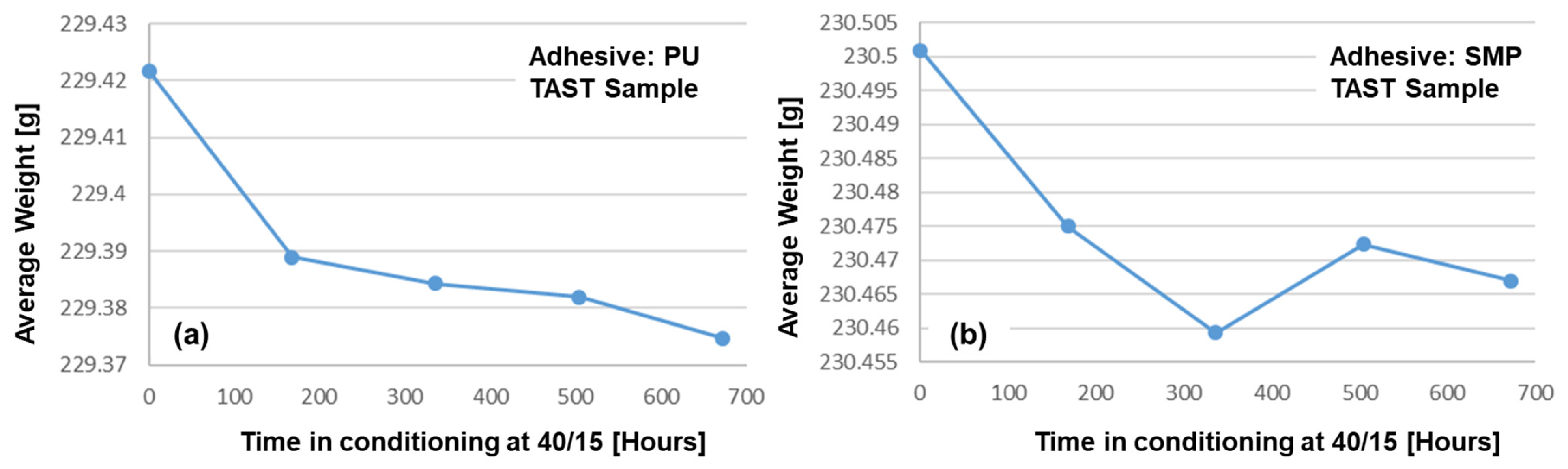
4.1. Weight Measurements
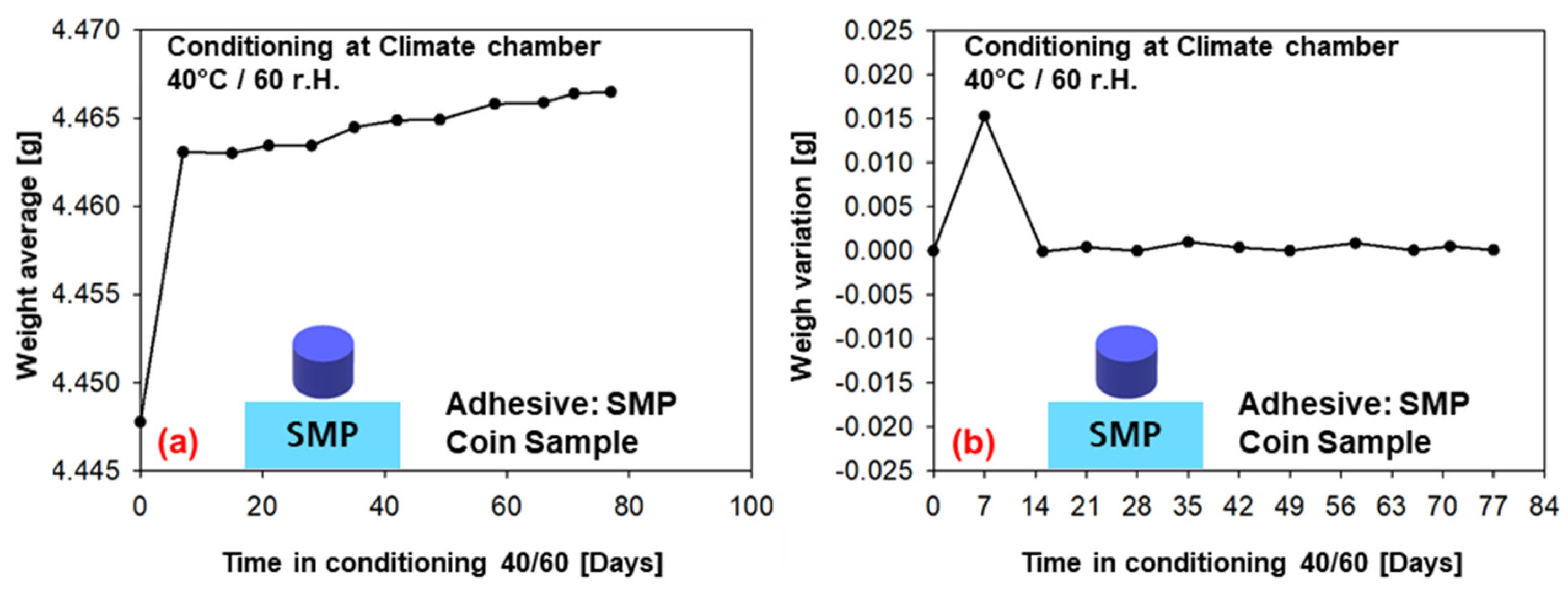
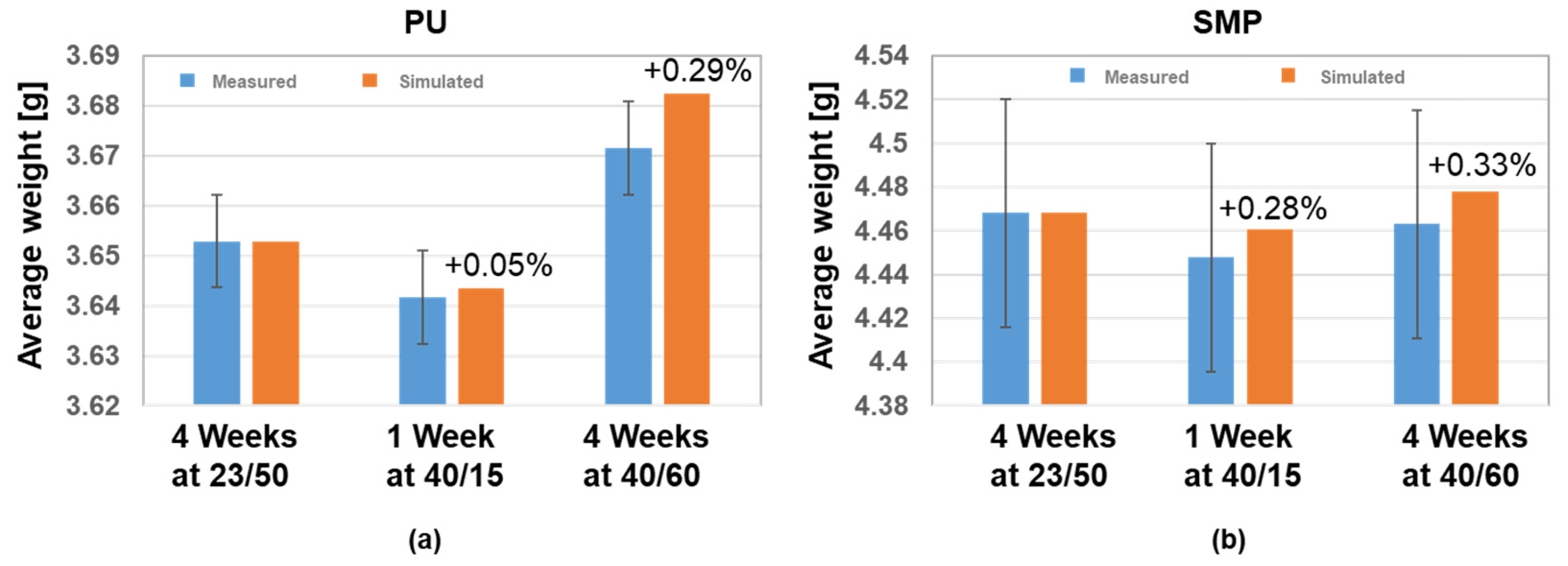
4.1.1. Measurements for Conditioning in the Climatic Chamber
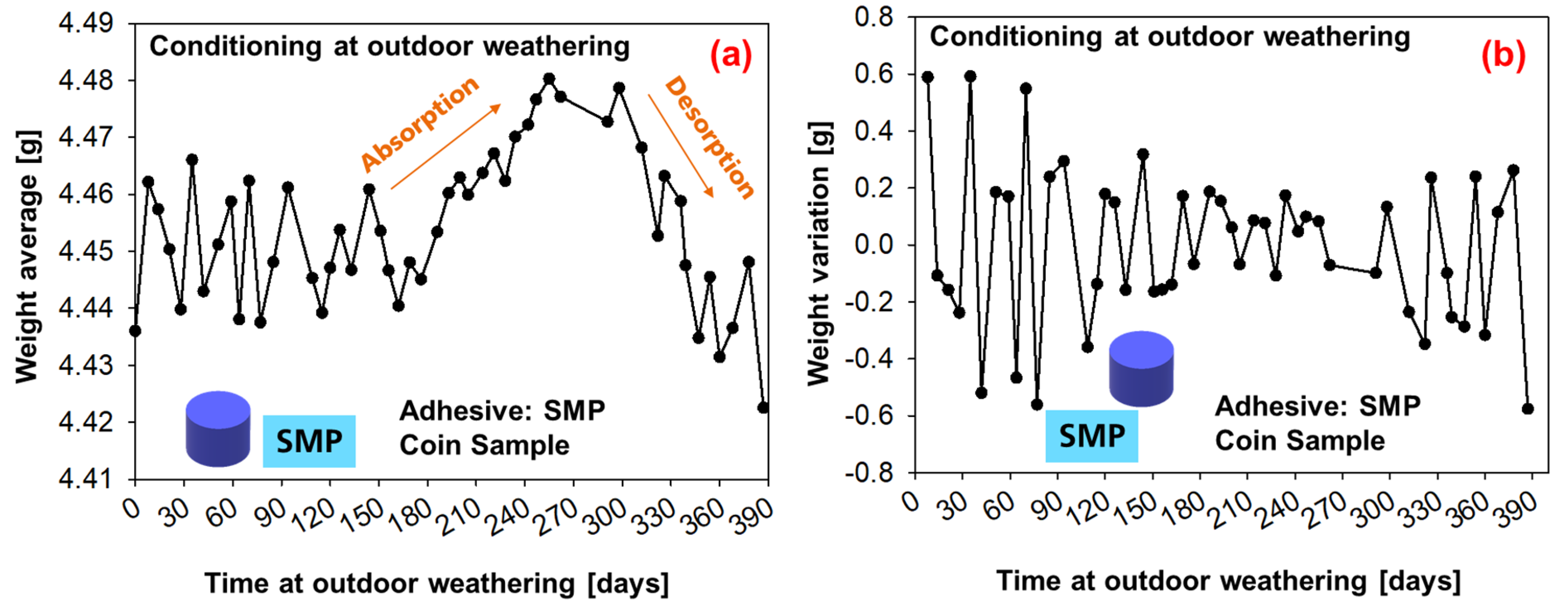
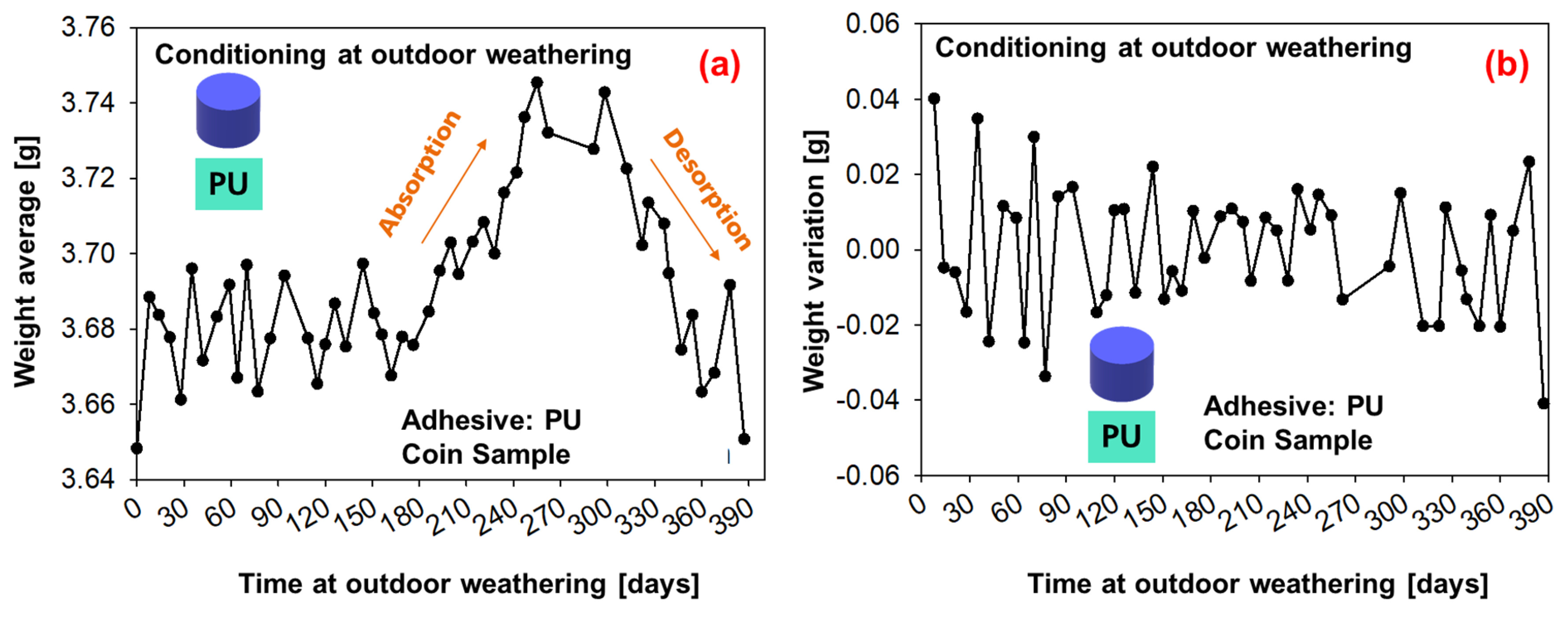
4.1.2. Measurements for Conditioning under Outdoor Weathering
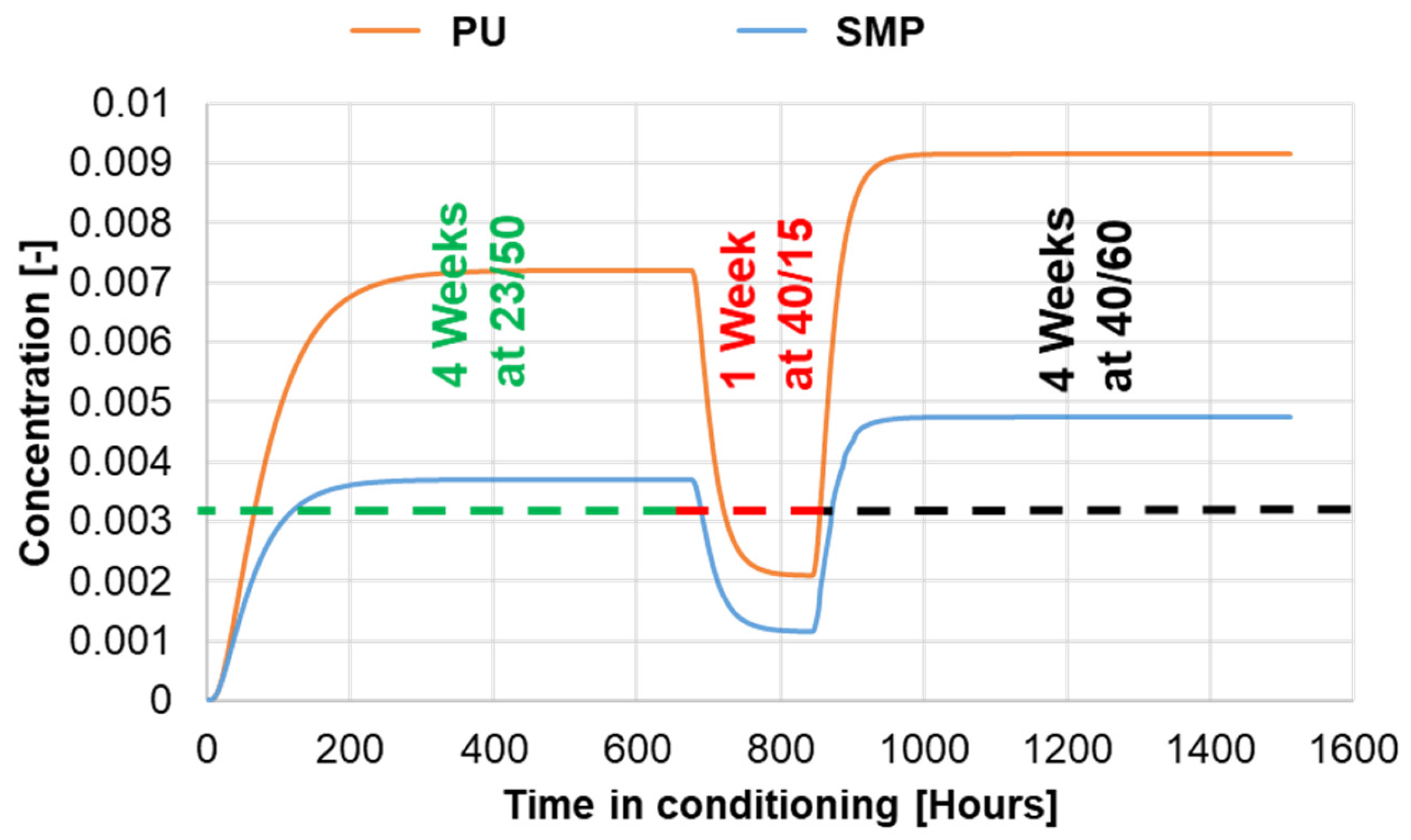
4.2. Simulations Results of Conditioning in the Climatic Chamber
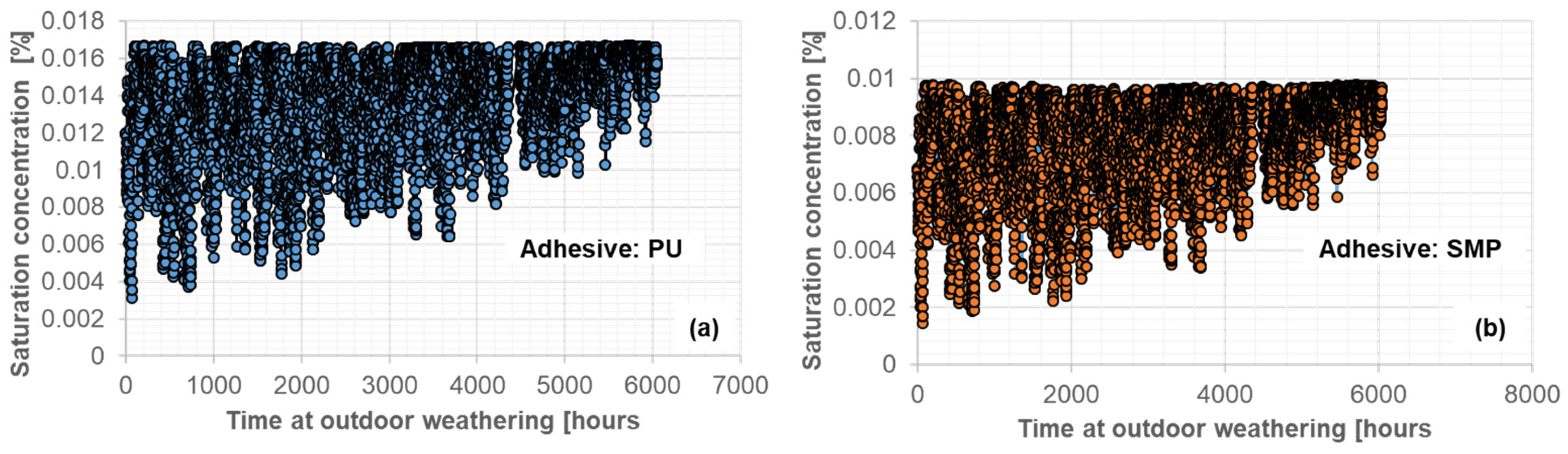
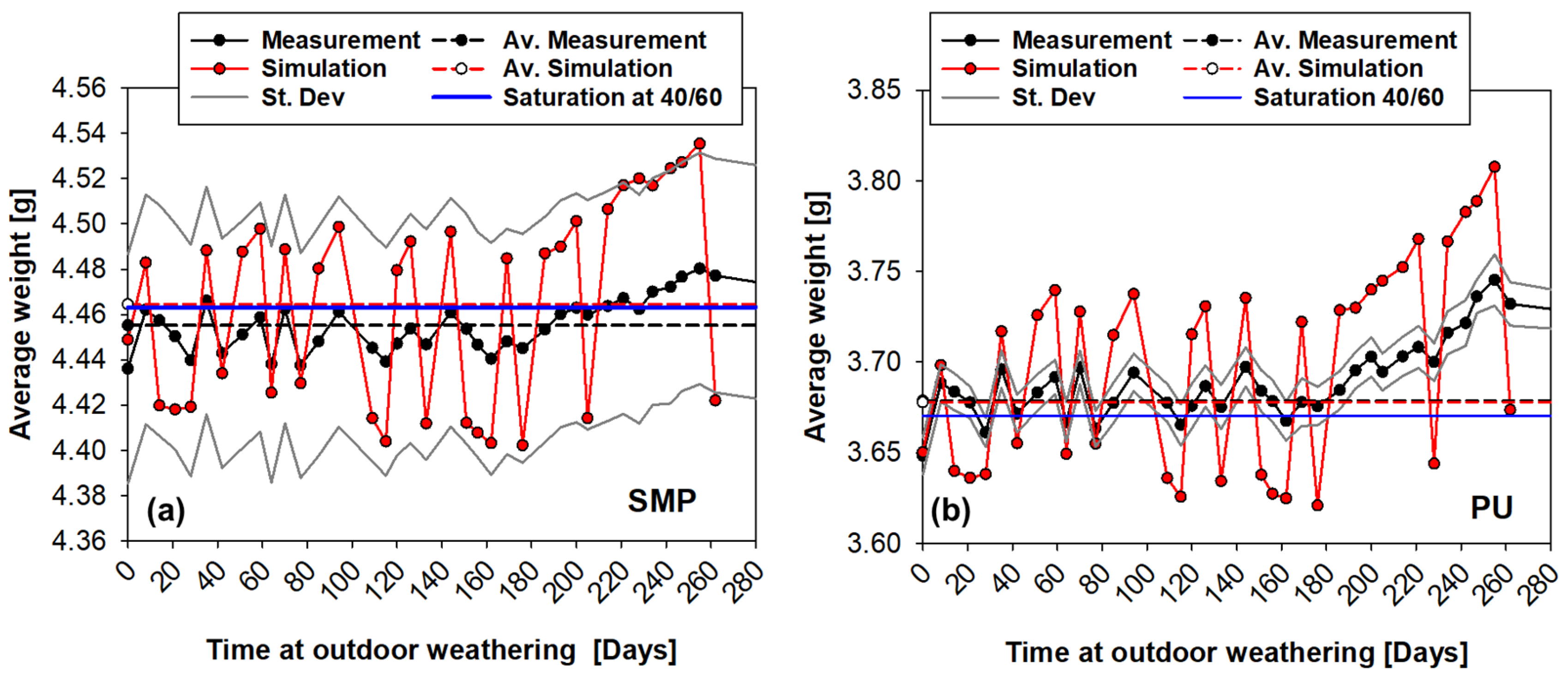
4.3. Simulations Results of Conditioning under Outdoor Weathering
5. Mechanical Characterisation Results
5.1. Verification
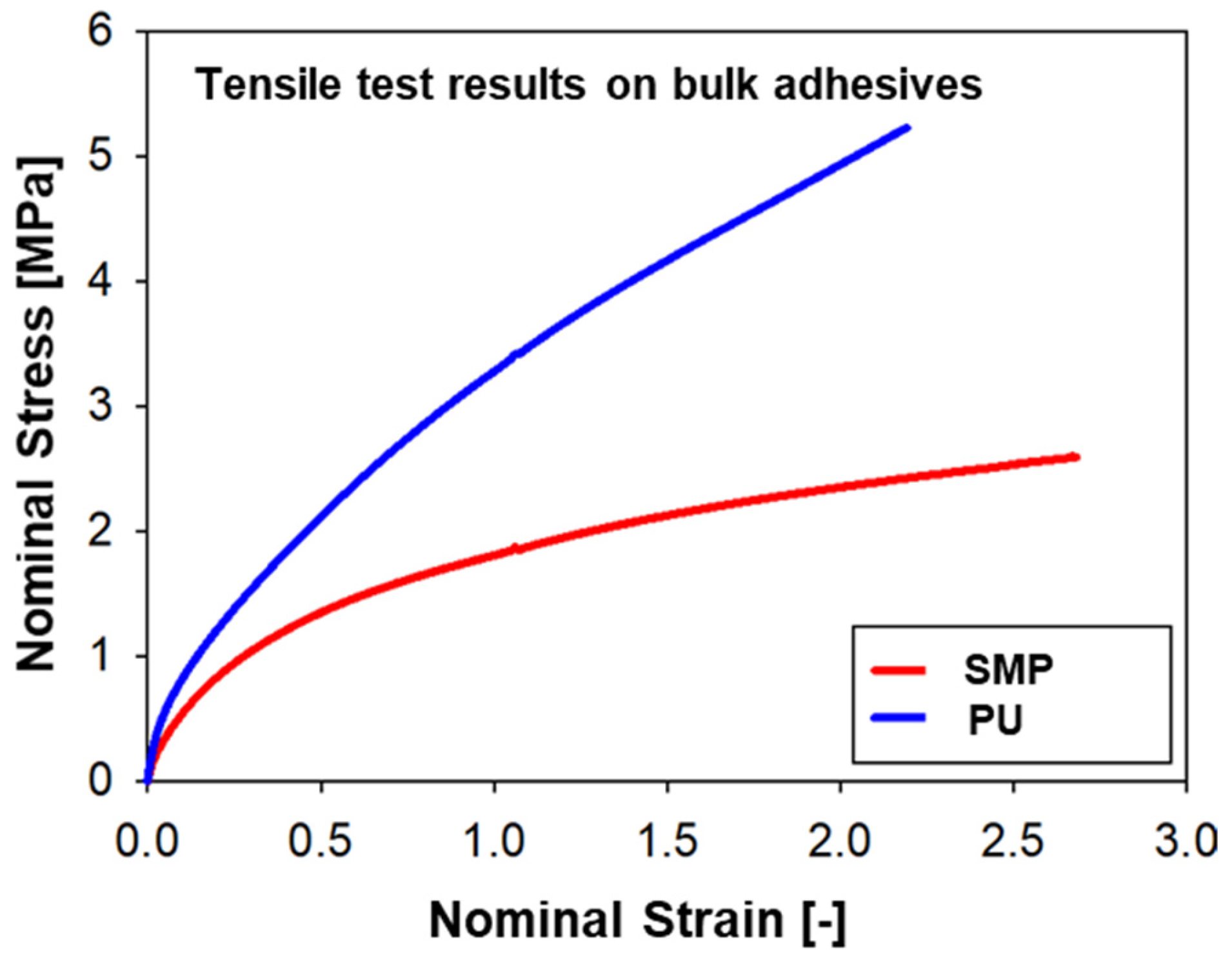
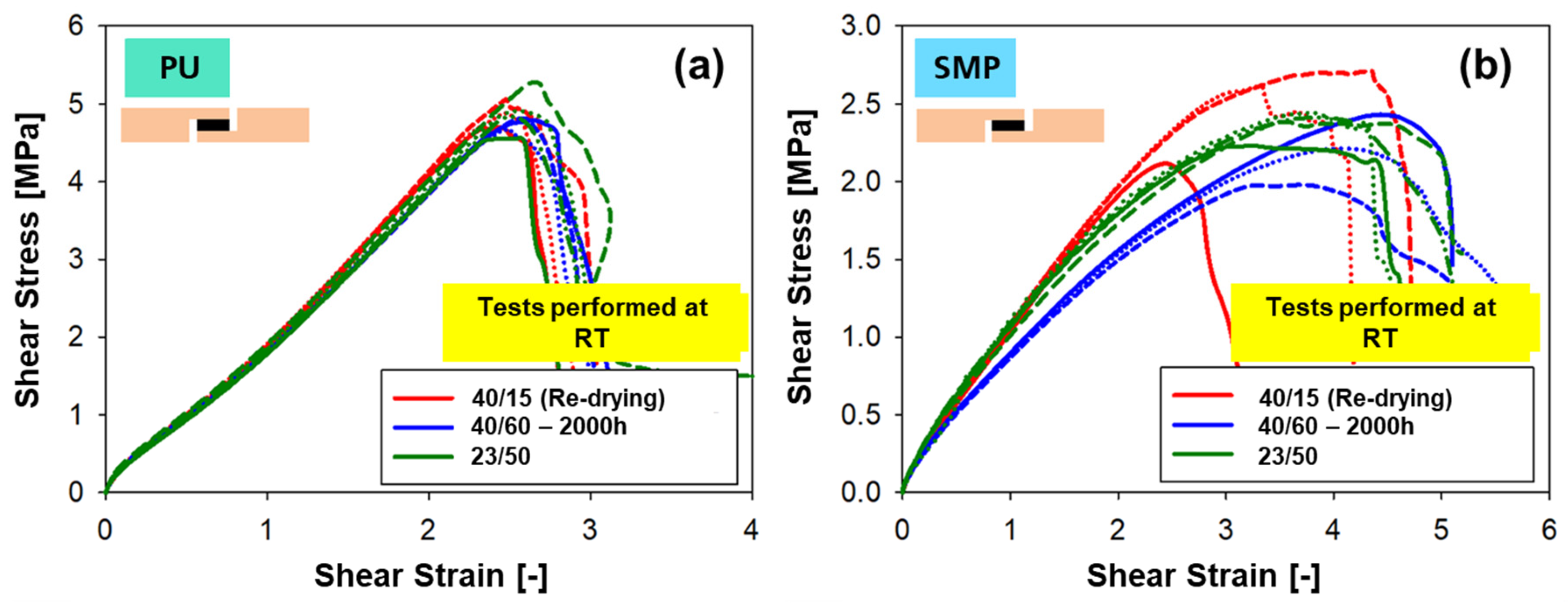
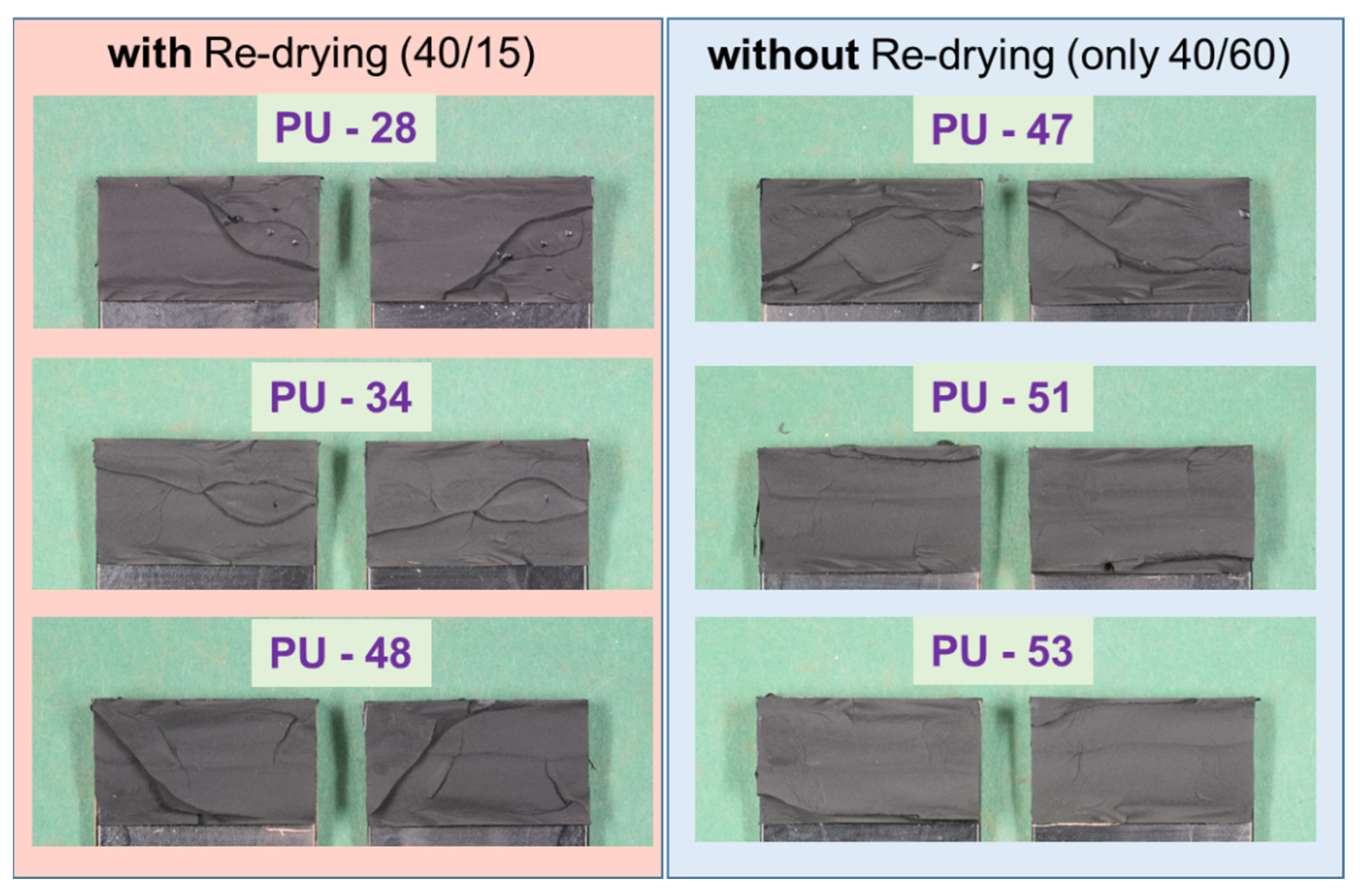
5.2. Quasi-Static Testing
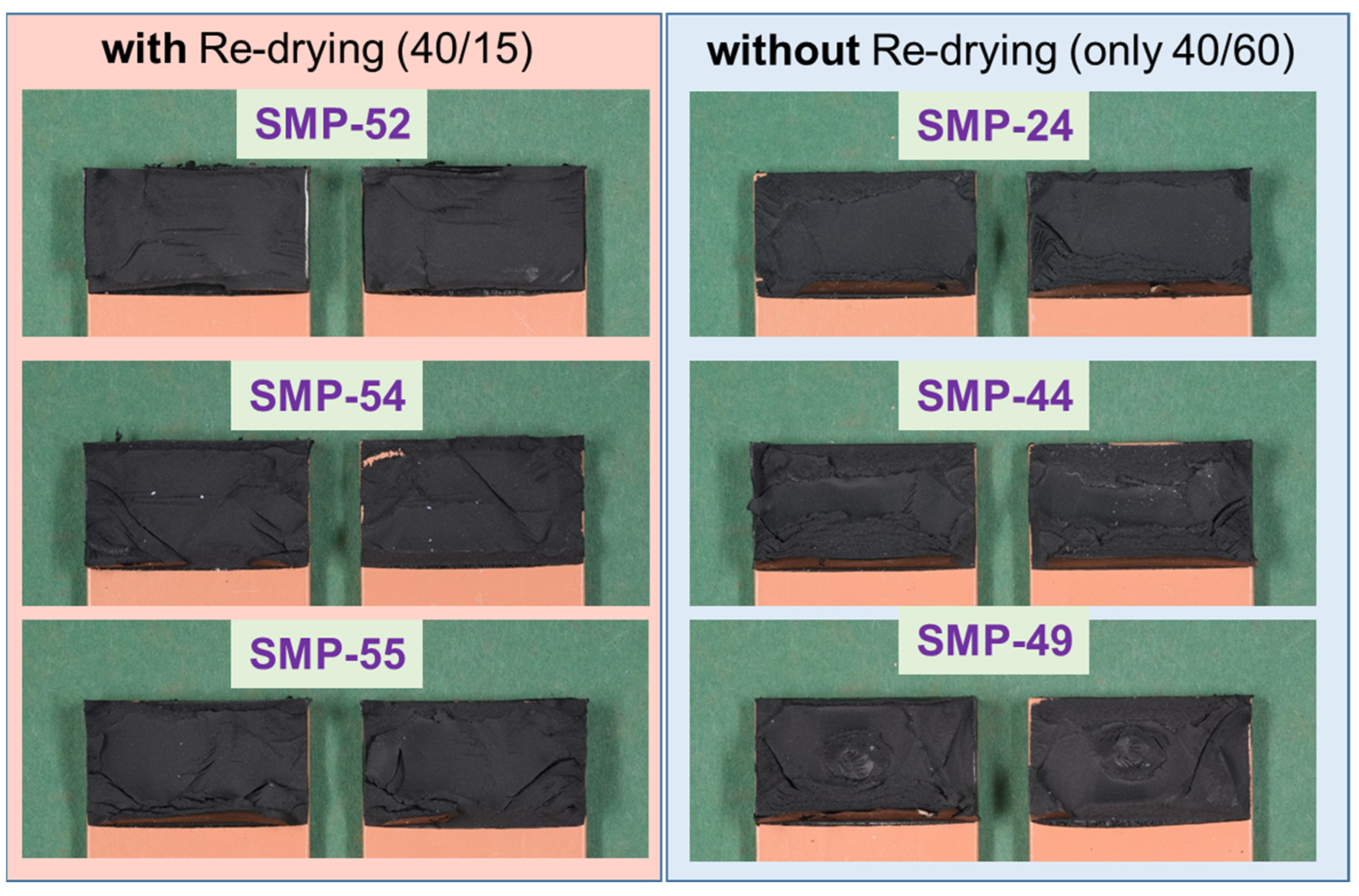
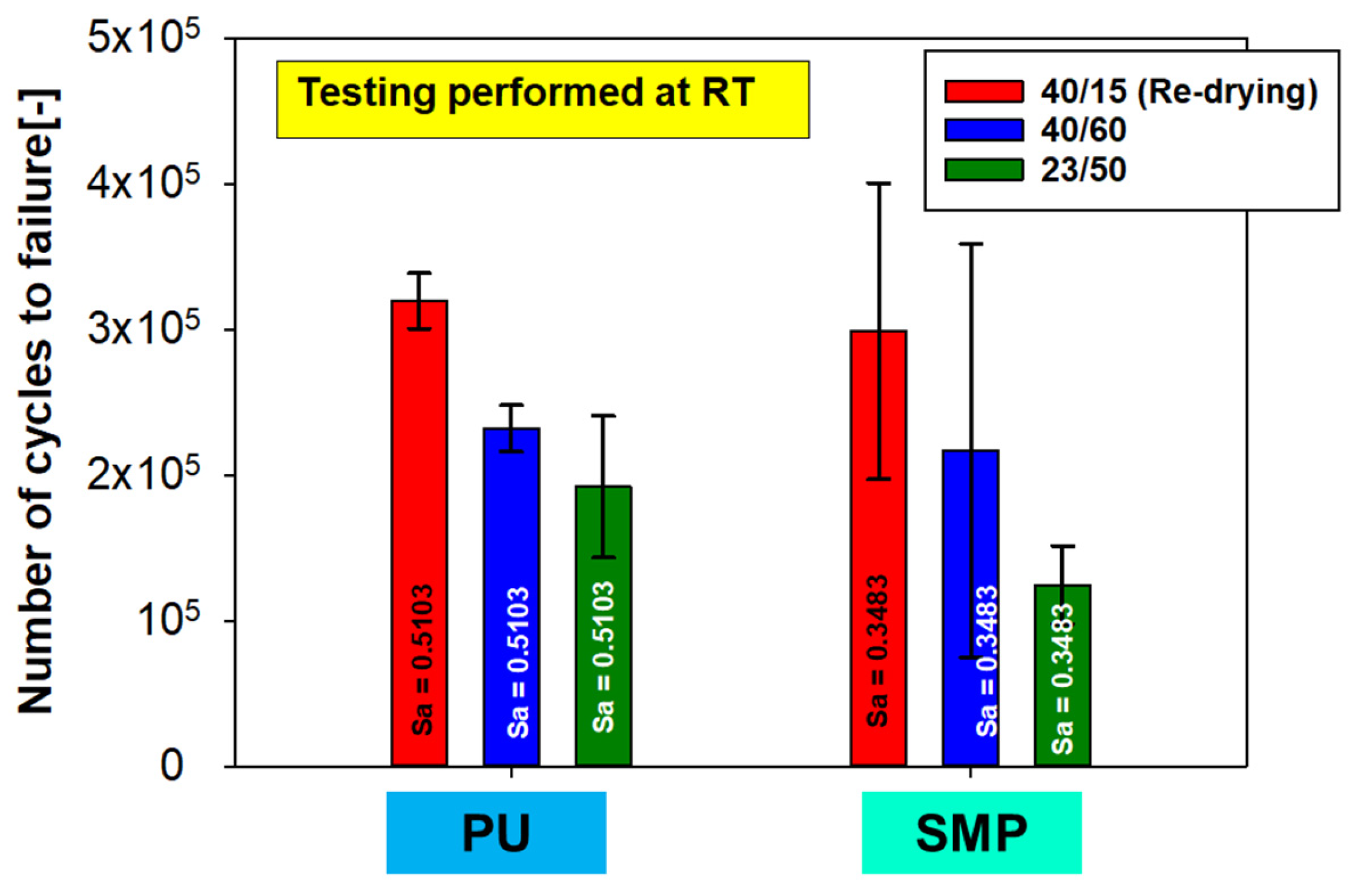
5.3. Fatigue Testing
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviation
| Symbol | Meaning |
| A | surface |
| b | sample width |
| average concentration | |
| , | amount of solute at each concentration point |
| adhesive layer thickness | |
| D | diameter |
| f | frequency |
| F | force |
| G | shear modulus |
| integration point | |
| initial gauge length | |
| overlap length | |
| mass change due diffusion | |
| PU | polyurethane |
| r.h | relative humidity |
| R | stress ratio |
| RT | room temperature |
| s | displacement |
| SMP | silicon-modified polymer |
| TAST | thick adherend shear test |
| volume of each integration point | |
| shear stress | |
| shear strain | |
| density |
References
- van Amerongen, G.J. Diffusion in Elastomers. Rubber Chem. Technol. 1964, 37, 1065–1152. [Google Scholar] [CrossRef]
- Costa, M.; Viana, G.; da Silva, L.F.M.; Campilho, R.D.S.G. Environmental effect on the fatigue degradation of adhesive joints: A review. J. Adhes. 2017, 93, 127–146. [Google Scholar] [CrossRef]
- Lai, M.; Botsis, J.; Cugnoni, J.; Coric, D. An experimental–numerical study of moisture absorption in an epoxy. Compos. Part A Appl. Sci. Manuf. 2012, 43, 1053–1060. [Google Scholar] [CrossRef]
- Possart, W.; Brede, M. (Eds.) Adhesive Joints: Ageing and Durability of Epoxies and Polyurethanes; Wiley-VCH: Weinheim, Germany, 2019. [Google Scholar]
- Pethrick, R.A. Design and ageing of adhesives for structural adhesive bonding—A review. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2015, 229, 349–379. [Google Scholar] [CrossRef]
- da Silva, L.F.M.; Öchsner, A.; Adams, R.D. Handbook of Adhesion Technology; Springer International Publishing: Cham, Switherland, 2017. [Google Scholar]
- Zhai, J.; Zhao, H.; Guo, X.; Li, X.; Song, T. Influence of Temperature on Mechanical Properties of P(BAMO-r-THF) Elastomer. Polymers 2020, 12, 2507. [Google Scholar] [CrossRef] [PubMed]
- DIN 6701-3: 2015-12; Adhesive Bonding of Railway Vehicles and Parts—Part 3: Guideline for Construction Design and Verification of Bonds on Railway Vehicles. Beuth Verlag GmbH: Berlin, Germany, 2015.
- Wulf, A.; Hesebeck, O.; Baumert, M.; Brede, M. Nachweisführung für die Beanspruchbarkeit von hyperelastischen Klebverbindungen unter betriebsrelevanten Bedingungen; Final Report of the Project; IGF-Vorhaben Nr. 18173 N; DVS Forschungsvereinigung, Band: 341; DVS-Media GmbH: Düsseldorf, Germany, 2018. [Google Scholar]
- da Silva, L.F.M.; Sato, C. Design of Adhesive Joints Under Humid Conditions; Springer Berlin Heidelberg: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Huacuja-Sánchez, J.E.; Müller, K.; Possart, W. Water diffusion in a crosslinked polyether-based polyurethane adhesive. Int. J. Adhes. Adhes. 2016, 66, 167–175. [Google Scholar] [CrossRef]
- Guo, Y.C.; Wang, X.; Sun, K.W. Finite Element Analysis on Moisture Distribution within RAC under Standard Drying Conditions. AMR 2011, 261–263, 356–360. [Google Scholar] [CrossRef]
- Das, A.; Mahanwar, P. A brief discussion on advances in polyurethane applications. Adv. Ind. Eng. Polym. Res. 2020, 3, 93–101. [Google Scholar] [CrossRef]
- Sheikh, N.; Mirzadeh, H.; Katbab, A.A.; Salehian, P.; Daliri, M.; Amanpour, S. Isocyanate-terminated urethane prepolymer as bioadhesive material: Evaluation of bioadhesion and biocompatibility, in vitro and in vivo assays. J. Biomater. Sci. Polym. Ed. 2001, 12, 707–719. [Google Scholar] [CrossRef] [PubMed]
- Gadhave, R.V.; Gadhave, C.R.; Dhawale, P.V. Silane Terminated Prepolymers: An Alternative to Silicones and Polyurethanes. Open J. Polym. Chem. 2021, 11, 31–54. [Google Scholar] [CrossRef]
- Berzins, R.; Merijs-Meri, R.; Zicans, J. Comparison of Two-Component Silyl-Terminated Polyether/Epoxy Resin Model and Complete Systems and Evaluation of Their Mechanical, Rheological and Adhesive Properties. Polymers 2022, 14, 2421. [Google Scholar] [CrossRef] [PubMed]
- Hertz, D.L. Elastomers: An engineering material. Rubber Chem. Tech. 2007, 80, 520–532. [Google Scholar] [CrossRef]
- DVS 1608; Richtlinie DVS 1608-1 (02/2022): Gestaltung und Festigkeitsbewertung von Schweißkonstruktionen aus Aluminiumlegierungen im Schienenfahrzeugbau. Beuth Verlag GmbH: Berlin, Germany, 2022.
- Shen, C.-H.; Springer, G.S. Moisture Absorption and Desorption of Composite Materials. J. Compos. Mater. 1976, 10, 2–20. [Google Scholar] [CrossRef] [Green Version]
- Dassault Systèmes Simulia. Abaqus Users’ Manual, Version 2019; Dassault Systèmes Simulia: Providence, RI, USA, 2019. [Google Scholar]
- Crank, J. The Mathematics of Diffusion, 2nd ed.; Oxford University Press: London, UK, 1975. [Google Scholar]
- Driemeier, C.; Mendes, F.M.; Oliveira, M.M. Dynamic vapor sorption and thermoporometry to probe water in celluloses. Cellulose 2012, 19, 1051–1063. [Google Scholar] [CrossRef] [Green Version]
- Hosseinpourpia, R.; Adamopoulos, S.; Mai, C. Dynamic vapour sorption of wood and holocellulose modified with thermosetting resins. Wood Sci. Technol. 2016, 50, 165–178. [Google Scholar] [CrossRef]
- Eslami, S.; Honarbakhsh-Raouf, A.; Eslami, S. Effects of moisture absorption on degradation of E-glass fiber reinforced Vinyl Ester composite pipes and modelling of transient moisture diffusion using finite element analysis. Corros. Sci. 2015, 90, 168–175. [Google Scholar] [CrossRef]
- Bonilla Mora, V.; Mieloszyk, M.; Ostachowicz, W. Model of moisture absorption by adhesive joint. Mech. Syst. Signal Processs. 2018, 99, 534–549. [Google Scholar] [CrossRef]








| E [GPa] | Tensile Strength [Mpa] | Yield Point [Mpa] | Elongation at Rupture [%] |
|---|---|---|---|
| 70 | 310 | 260 | 6 |
| T [°C] | Diffusivity PU–Adhesive [mm2/s] | Diffusivity SMP–Adhesive [mm2/s] |
|---|---|---|
| 0 | 0.02462 | 0.056361603 |
| 5 | 0.03523 | 0.070434496 |
| 10 | 0.04978 | 0.08733106 |
| 15 | 0.0695 | 0.107475942 |
| 20 | 0.09594 | 0.131334501 |
| 23 | 0.1158 | 0.147641408 |
| 25 | 0.131 | 0.159413882 |
| 30 | 0.17705 | 0.19226387 |
| 35 | 0.23697 | 0.230477522 |
| 40 | 0.31422 | 0.274691558 |
| 45 | 0.41297 | 0.325586527 |
| 50 | 0.5382 | 0.383886745 |
| Conditioning | ||
|---|---|---|
| 23/50 | 0.00369983 | 0.00719743 |
| 40/60 | 0.00475 | 0.00915 |
| 40/15 | 0.00114375 | 0.00208125 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernandes, P.H.E.; Wulf, A.; Nagel, C.; Beber, V.C. FE-Simulation and Experimental Characterisation of Environmental Effects on the Diffusion and Mechanical Performance of Hyperelastic Adhesive Joints. Eng 2023, 4, 2122-2140. https://doi.org/10.3390/eng4030121
Fernandes PHE, Wulf A, Nagel C, Beber VC. FE-Simulation and Experimental Characterisation of Environmental Effects on the Diffusion and Mechanical Performance of Hyperelastic Adhesive Joints. Eng. 2023; 4(3):2122-2140. https://doi.org/10.3390/eng4030121
Chicago/Turabian StyleFernandes, Pedro H. E., Andreas Wulf, Christof Nagel, and Vinicius C. Beber. 2023. "FE-Simulation and Experimental Characterisation of Environmental Effects on the Diffusion and Mechanical Performance of Hyperelastic Adhesive Joints" Eng 4, no. 3: 2122-2140. https://doi.org/10.3390/eng4030121
APA StyleFernandes, P. H. E., Wulf, A., Nagel, C., & Beber, V. C. (2023). FE-Simulation and Experimental Characterisation of Environmental Effects on the Diffusion and Mechanical Performance of Hyperelastic Adhesive Joints. Eng, 4(3), 2122-2140. https://doi.org/10.3390/eng4030121










