All Acoustical Excitation of Spin Waves in High Overtone Bulk Acoustic Resonator
Abstract
1. Introduction
2. Materials and Methods
2.1. HBAR Structure and Basic Properties
2.2. Theoretical Description
2.3. Experimental Methods
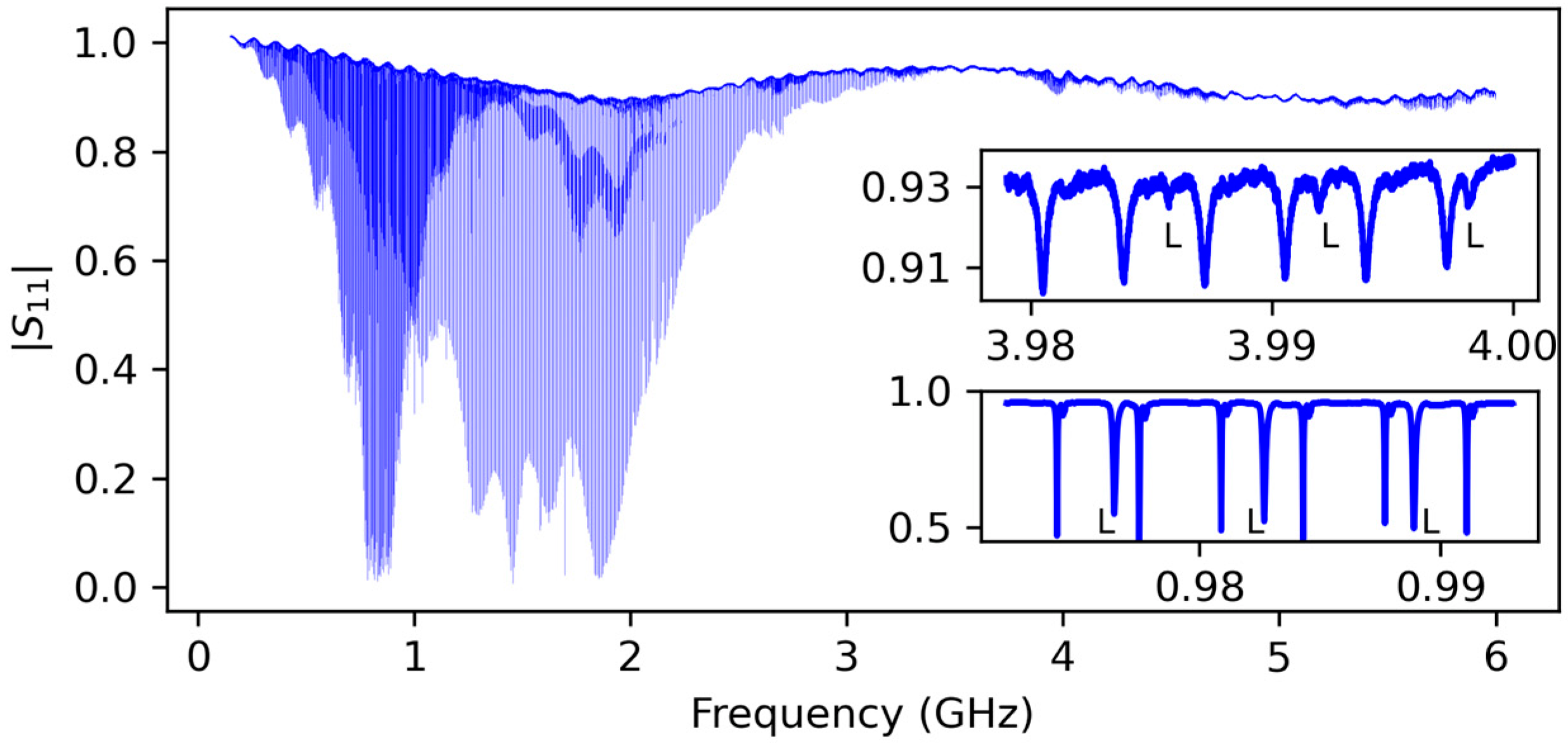
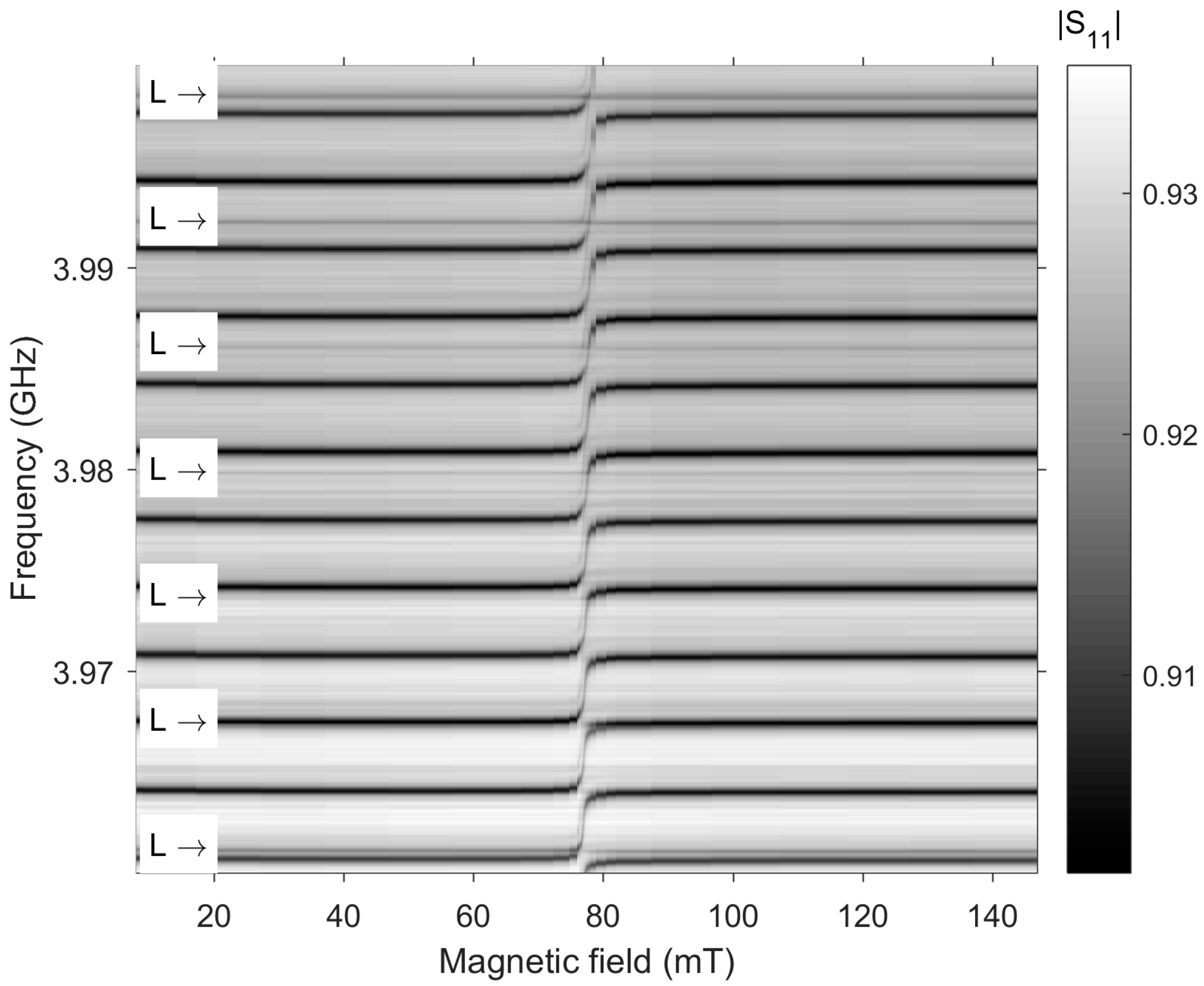
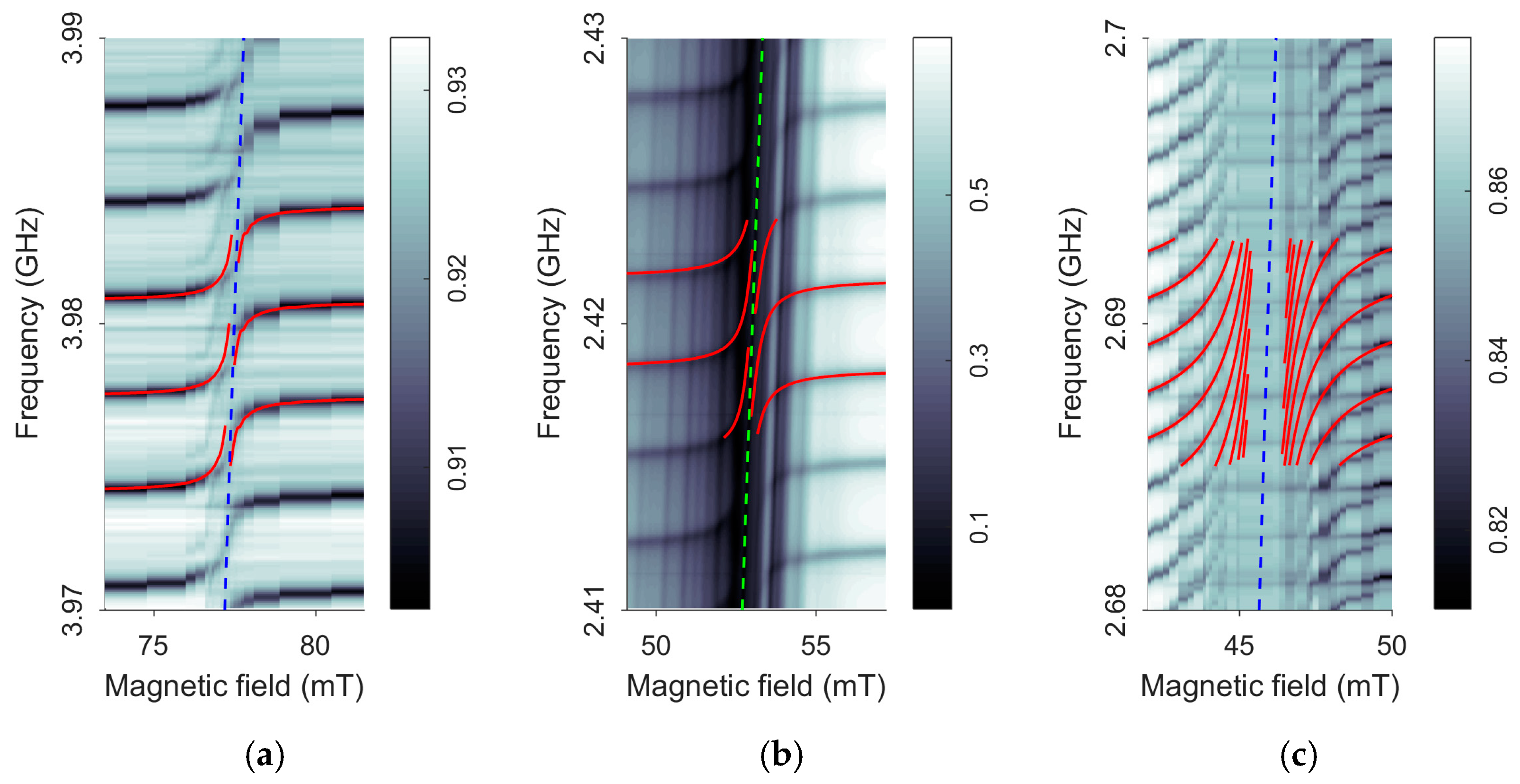
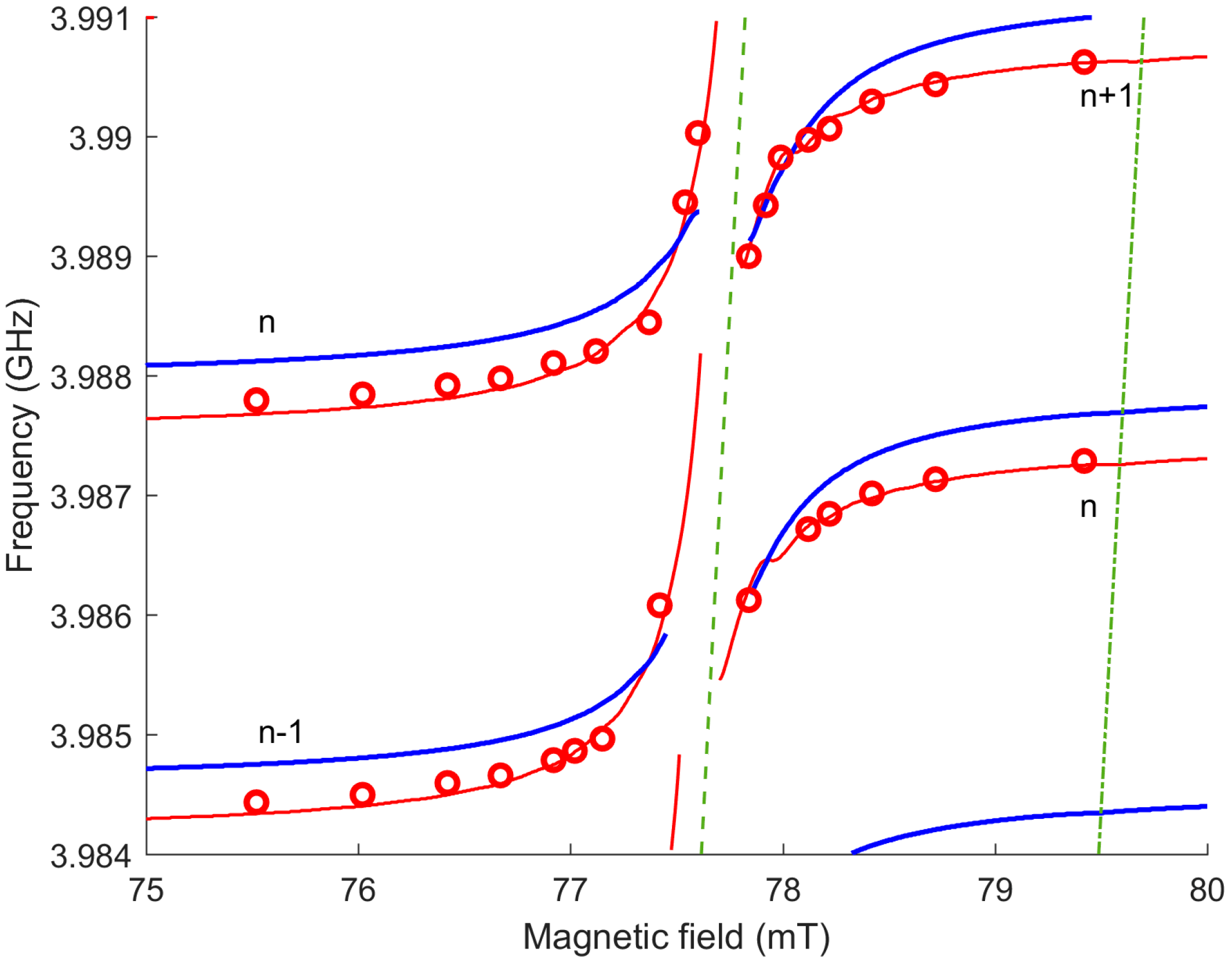
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Awschalom, D.D.; Du, C.R.; He, R.; Heremans, F.J.; Hoffmann, A.; Hou, J.; Kurebayashi, H.; Li, Y.; Liu, L.; Novosad, V.; et al. Quantum Engineering with Hybrid Magnonic Systems and Materials (Invited Paper). IEEE Trans. Quantum Eng. 2021, 2, 1–36. [Google Scholar] [CrossRef]
- Delsing, P.; Cleland, A.N.; Schuetz, M.J.A.; Knörzer, J.; Giedke, G.; Cirac, J.I.; Srinivasan, K.; Wu, M.; Balram, K.C.; Bäuerle, C.; et al. The 2019 Surface Acoustic Waves Roadmap. J. Phys. D Appl. Phys. 2019, 52, 353001. [Google Scholar] [CrossRef]
- Barman, A.; Gubbiotti, G.; Ladak, S.; Adeyeye, A.O.; Krawczyk, M.; Gräfe, J.; Adelmann, C.; Cotofana, S.; Naeemi, A.; Vasyuchka, V.I.; et al. The 2021 Magnonics Roadmap. J. Phys. Condens. Matter 2021, 33, 413001. [Google Scholar] [CrossRef] [PubMed]
- Puebla, J.; Hwang, Y.; Maekawa, S.; Otani, Y. Perspectives on Spintronics with Surface Acoustic Waves. Appl. Phys. Lett. 2022, 120, 220502. [Google Scholar] [CrossRef]
- Latcham, O.S.; Gusieva, Y.I.; Shytov, A.V.; Gorobets, O.Y.; Kruglyak, V.V. Controlling Acoustic Waves Using Magneto-Elastic Fano Resonances. Appl. Phys. Lett. 2019, 115, 082403. [Google Scholar] [CrossRef]
- An, K.; Litvinenko, A.N.; Kohno, R.; Fuad, A.A.; Naletov, V.V.; Vila, L.; Ebels, U.; de Loubens, G.; Hurdequint, H.; Beaulieu, N.; et al. Coherent Long-Range Transfer of Angular Momentum between Magnon Kittel Modes by Phonons. Phys. Rev. B 2020, 101, 060407. [Google Scholar] [CrossRef]
- Litvinenko, A.; Khymyn, R.; Tyberkevych, V.; Tikhonov, V.; Slavin, A.; Nikitov, S. Tunable Magnetoacoustic Oscillator with Low Phase Noise. Phys. Rev. Appl. 2021, 15, 034057. [Google Scholar] [CrossRef]
- Yang, W.-G.; Schmidt, H. Acoustic Control of Magnetism toward Energy-Efficient Applications. Appl. Phys. Rev. 2021, 8, 021304. [Google Scholar] [CrossRef]
- Kittel, C. Interaction of Spin Waves and Ultrasonic Waves in Ferromagnetic Crystals. Phys. Rev. 1958, 110, 836–841. [Google Scholar] [CrossRef]
- Cherepov, S.; Khalili Amiri, P.; Alzate, J.G.; Wong, K.; Lewis, M.; Upadhyaya, P.; Nath, J.; Bao, M.; Bur, A.; Wu, T.; et al. Electric-Field-Induced Spin Wave Generation Using Multiferroic Magnetoelectric Cells. Appl. Phys. Lett. 2014, 104, 082403. [Google Scholar] [CrossRef]
- Geilen, M.; Nicoloiu, A.; Narducci, D.; Mohseni, M.; Bechberger, M.; Ender, M.; Ciubotaru, F.; Hillebrands, B.; Müller, A.; Adelmann, C.; et al. Fully Resonant Magneto-Elastic Spin-Wave Excitation by Surface Acoustic Waves under Conservation of Energy and Linear Momentum. Appl. Phys. Lett. 2022, 120, 242404. [Google Scholar] [CrossRef]
- Küß, M.; Heigl, M.; Flacke, L.; Hefele, A.; Hörner, A.; Weiler, M.; Albrecht, M.; Wixforth, A. Symmetry of the Magnetoelastic Interaction of Rayleigh and Shear Horizontal Magnetoacoustic Waves in Nickel Thin Films on LiTaO3. Phys. Rev. Appl. 2021, 15, 034046. [Google Scholar] [CrossRef]
- Uchida, K.; An, T.; Kajiwara, Y.; Toda, M.; Saitoh, E. Surface-Acoustic-Wave-Driven Spin Pumping in Y3Fe5O12/Pt Hybrid Structure. Appl. Phys. Lett. 2011, 99, 212501. [Google Scholar] [CrossRef]
- Weiler, M.; Huebl, H.; Goerg, F.S.; Czeschka, F.D.; Gross, R.; Goennenwein, S.T.B. Spin Pumping with Coherent Elastic Waves. Phys. Rev. Lett. 2012, 108, 176601. [Google Scholar] [CrossRef] [PubMed]
- Driscoll, M.M.; Jelen, R.A.; Matthews, N. Extremely Low Phase Noise UHF Oscillators Utilizing High-Overtone, Bulk-Acoustic Resonators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1992, 39, 774–779. [Google Scholar] [CrossRef] [PubMed]
- Lakin, K.M. Thin Film Resonator Technology. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2005, 52, 707–716. [Google Scholar] [CrossRef]
- Mansfeld, G.D.; Alekseev, S.G.; Polzikova, N.I. Unique Properties of HBAR Characteristics. In Proceedings of the 2008 IEEE Ultrasonics Symposium, Beijing, China, 2–5 November 2008; pp. 439–442. [Google Scholar]
- Sorokin, B.P.; Kvashnin, G.M.; Volkov, A.P.; Bormashov, V.S.; Aksenenkov, V.V.; Kuznetsov, M.S.; Gordeev, G.I.; Telichko, A.V. AlN/Single Crystalline Diamond Piezoelectric Structure as a High Overtone Bulk Acoustic Resonator. Appl. Phys. Lett. 2013, 102, 113507. [Google Scholar] [CrossRef]
- Sorokin, B.P.; Asafiev, N.O.; Kvashnin, G.M.; Scherbakov, D.A.; Terentiev, S.A.; Blank, V.D. Toward 40 GHz Excitation of Diamond-Based HBAR. Appl. Phys. Lett. 2021, 118, 083501. [Google Scholar] [CrossRef]
- Gokhale, V.J.; Downey, B.P.; Katzer, D.S.; Nepal, N.; Lang, A.C.; Stroud, R.M.; Meyer, D.J. Epitaxial Bulk Acoustic Wave Resonators as Highly Coherent Multi-Phonon Sources for Quantum Acoustodynamics. Nat. Commun. 2020, 11, 2314. [Google Scholar] [CrossRef]
- Daugey, T.; Friedt, J.-M.; Martin, G.; Boudot, R. A High-Overtone Bulk Acoustic Wave Resonator-Oscillator-Based 4.596 GHz Frequency Source: Application to a Coherent Population Trapping Cs Vapor Cell Atomic Clock. Rev. Sci. Instrum. 2015, 86, 114703. [Google Scholar] [CrossRef]
- Tian, H.; Liu, J.; Dong, B.; Skehan, J.C.; Zervas, M.; Kippenberg, T.J.; Bhave, S.A. Hybrid Integrated Photonics Using Bulk Acoustic Resonators. Nat. Commun. 2020, 11, 3073. [Google Scholar] [CrossRef] [PubMed]
- Chowdhury, P.; Jander, A.; Dhagat., P. Nondegenerate Parametric Pumping of Spin Waves by Acoustic Waves. IEEE Magn. Lett. 2017, 8, 3108204. [Google Scholar] [CrossRef]
- Fung, T.C.; Karenowska, A.D.; Gregg, J.F. Broadband phonon to magnon conversion in yttrium iron garnet. Mater. Quantum Technol. 2021, 1, 011003. [Google Scholar] [CrossRef]
- Polzikova, N.; Alekseev, S.; Kotelyanskii, I.; Raevskiy, A.; Fetisov, Y. Magnetic Field Tunable Acoustic Resonator with Ferromagnetic-Ferroelectric Layered Structure. J. Appl. Phys. 2013, 113, 17C704. [Google Scholar] [CrossRef]
- Polzikova, N.I.; Alekseev, S.G.; Raevskii, A.O. Parametric Pumping of Magnons in a Hybrid Magnon–Phonon Resonator. J. Commun. Technol. Electron. 2021, 66, 1296–1301. [Google Scholar] [CrossRef]
- Alekseev, S.G.; Dizhur, S.E.; Polzikova, N.I.; Luzanov, V.A.; Raevskiy, A.O.; Orlov, A.P.; Kotov, V.A.; Nikitov, S.A. Magnons Parametric Pumping in Bulk Acoustic Waves Resonator. Appl. Phys. Lett. 2020, 117, 072408. [Google Scholar] [CrossRef]
- Polzikova, N.I.; Alekseev, S.G.; Luzanov, V.A.; Raevskiy, A.O. Electroacoustic Excitation of Spin Waves and Their Detection Due to the Inverse Spin Hall Effect. Phys. Solid State 2018, 60, 2211–2217. [Google Scholar] [CrossRef]
- Polzikova, N.I.; Alekseev, S.G.; Pyataikin, I.I.; Luzanov, V.A.; Raevskiy, A.O.; Kotov, V.A. Frequency and Magnetic Field Mapping of Magnetoelastic Spin Pumping in High Overtone Bulk Acoustic Wave Resonator. AIP Adv. 2018, 8, 056128. [Google Scholar] [CrossRef]
- Polzikova, N.I.; Alekseev, S.G.; Luzanov, V.A.; Raevskiy, A.O. Acoustic Excitation and Electrical Detection of Spin Waves and Spin Currents in Hypersonic Bulk Waves Resonator with YIG/Pt System. J. Magn. Magn. Mater. 2019, 479, 38–42. [Google Scholar] [CrossRef]
- Luzanov, V.A.; Alekseev, S.G.; Polzikova, N.I. Deposition Process Optimization of Zinc Oxide Films with Inclined Texture Axis. J. Commun. Technol. Electron. 2018, 63, 1076–1079. [Google Scholar] [CrossRef]
- Alekseev, S.G.; Luzanov, V.A.; Polzikova, N.I. Study of Spatial Distribution of Piezoelectric Properties of ZnO Films by Acoustic Resonator Spectroscopy. J. Commun. Technol. Electron. 2020, 65, 1339–1344. [Google Scholar] [CrossRef]
- Alekseev, S.G.; Mansfeld, G.D.; Polzikova, N.I. Trapping of Acoustic Energy in Composite Resonators Based on Cubic Crystals. J. Commun. Technol. Electron. 2006, 51, 925–931. [Google Scholar] [CrossRef]
- Strauss, W. Magnetoelastic properties of yttrium iron garnet. In Physical Acoustics; Mason, W.P., Ed.; Academic Press: New York, NY, USA, 1968; Volume IV(B), pp. 211–268. [Google Scholar]
- Zhang, Y.; Wang, Z.; Cheeke, J.D.N. Resonant Spectrum Method to Characterize Piezoelectric Films in Composite Resonators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2003, 50, 321–333. [Google Scholar] [CrossRef] [PubMed]
- Alekseev, S.G.; Kotelyanskii, I.M.; Polzikova, N.I.; Mansfel’d, G.D. Study of Layered Structures Using Modified Acoustic Resonator Spectroscopy. J. Commun. Technol. Electron. 2015, 60, 300–307. [Google Scholar] [CrossRef]
- Alekseev, S.G.; Polzikova, N.I.; Raevskiy, A.O. Yttrium Iron Garnet Thickness Influence on the Spin Pumping in the Bulk Acoustic Wave Resonator. J. Commun. Technol. Electron. 2019, 64, 1318–1322. [Google Scholar] [CrossRef]
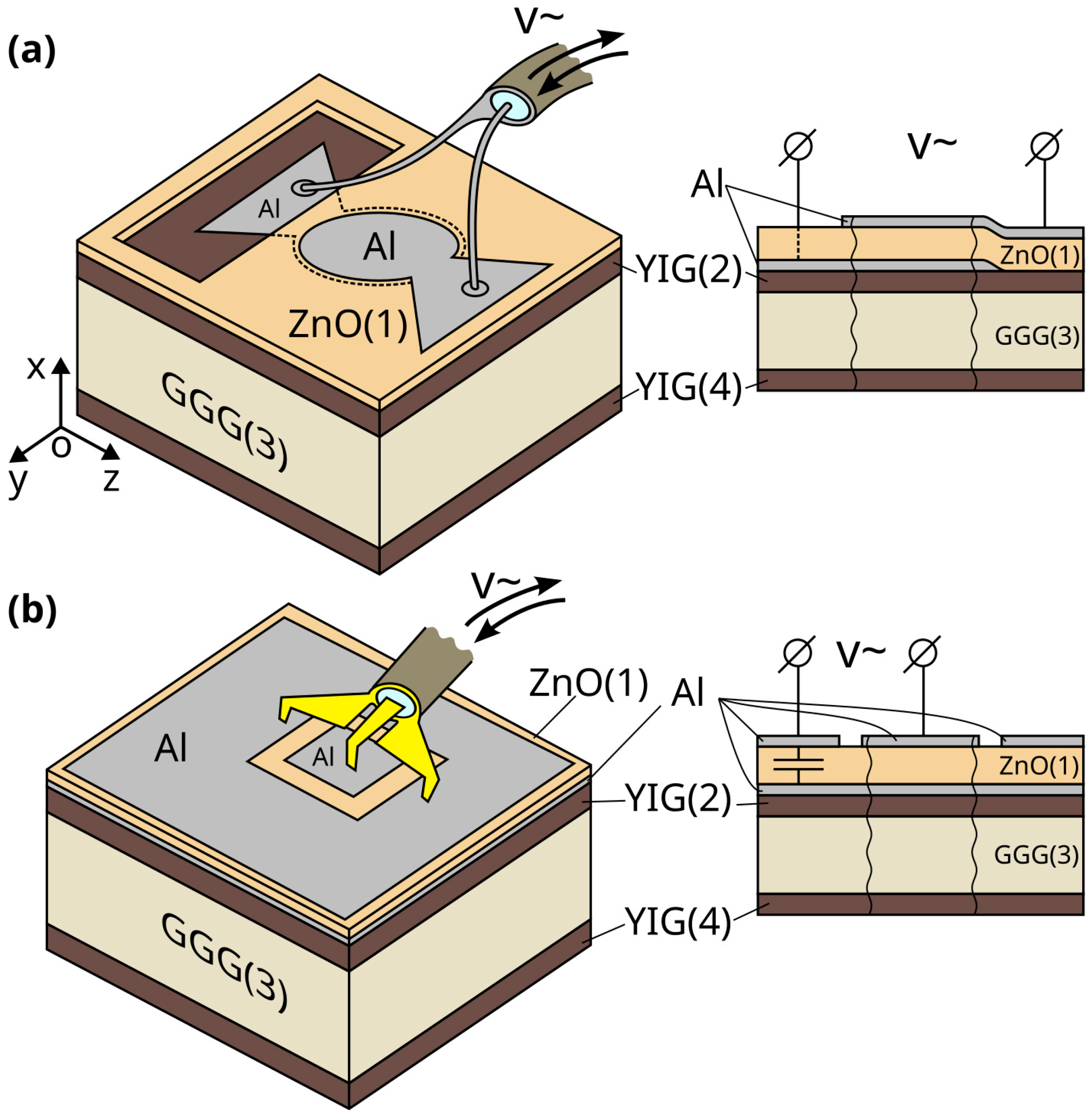
| No. | Transducers, Figure 1 | YIG | Thickness, of YIG, μm | Thickness, of GGG, μm | μ0M0, mT | b, MJ/m3 |
|---|---|---|---|---|---|---|
| 1 | (b) | 2 films, pure | 15 | 500 | 175 | 0.38 |
| 2 | (a) | 2 films, doped | 31 | 500 | 86 | 0.31 |
| 3 | (a) | 1 plate, pure | 1180 | 0 | 151 | 0.48 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alekseev, S.; Polzikova, N.; Luzanov, V. All Acoustical Excitation of Spin Waves in High Overtone Bulk Acoustic Resonator. Acoustics 2023, 5, 268-279. https://doi.org/10.3390/acoustics5010016
Alekseev S, Polzikova N, Luzanov V. All Acoustical Excitation of Spin Waves in High Overtone Bulk Acoustic Resonator. Acoustics. 2023; 5(1):268-279. https://doi.org/10.3390/acoustics5010016
Chicago/Turabian StyleAlekseev, Sergey, Natalia Polzikova, and Valery Luzanov. 2023. "All Acoustical Excitation of Spin Waves in High Overtone Bulk Acoustic Resonator" Acoustics 5, no. 1: 268-279. https://doi.org/10.3390/acoustics5010016
APA StyleAlekseev, S., Polzikova, N., & Luzanov, V. (2023). All Acoustical Excitation of Spin Waves in High Overtone Bulk Acoustic Resonator. Acoustics, 5(1), 268-279. https://doi.org/10.3390/acoustics5010016








