Nonlinear Distortions and Parametric Amplification Generate Otoacoustic Emissions and Increased Hearing Sensitivity
Abstract
1. Introduction
2. Methods
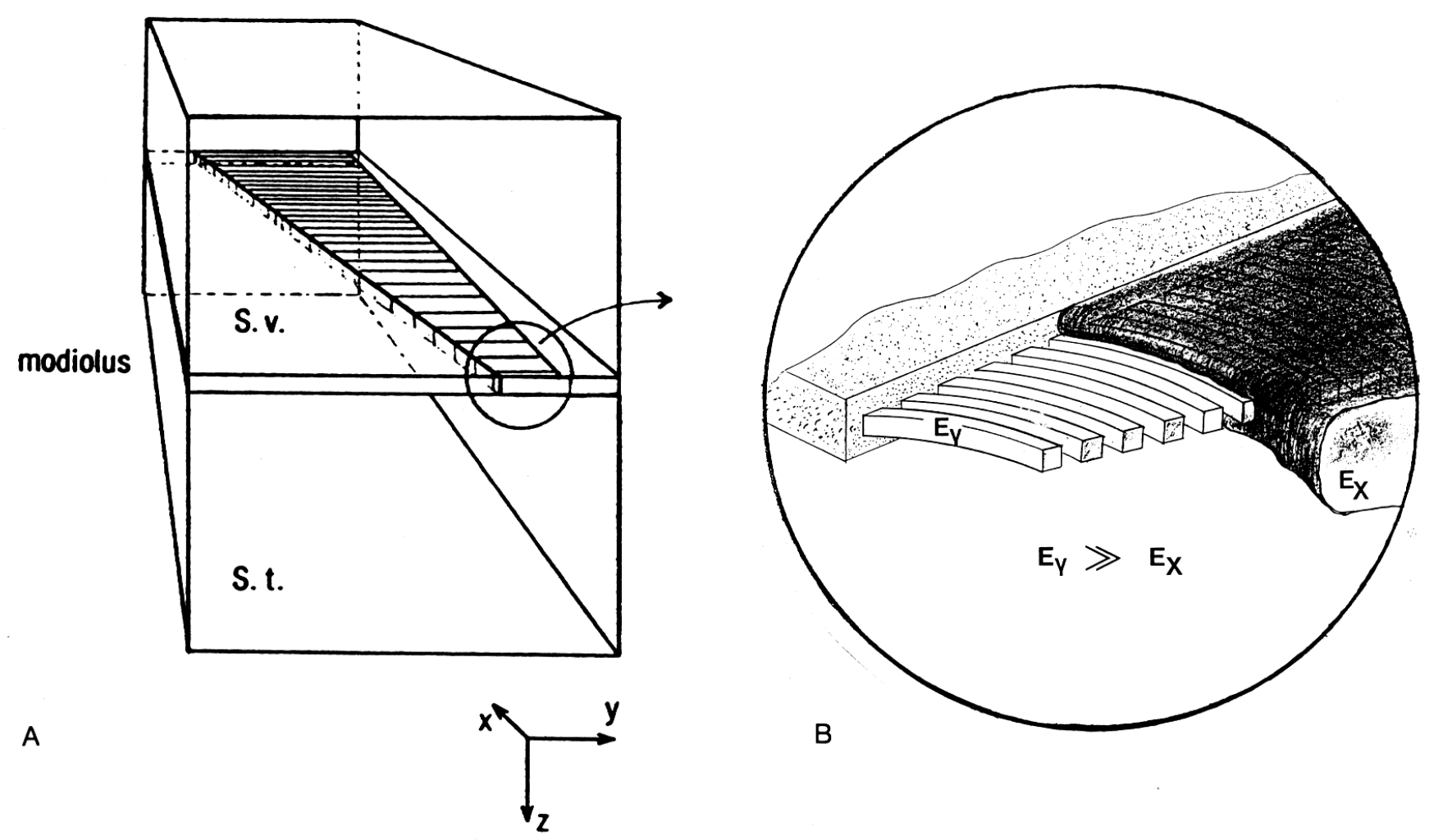
2.1. Partial Differential Equation (Parametric Traveling-Wave Amplifier)
2.2. Finite Difference Scheme (Damped Wave Equation)
2.3. Nonlinear Stiffness Function of Outer Hair Cells
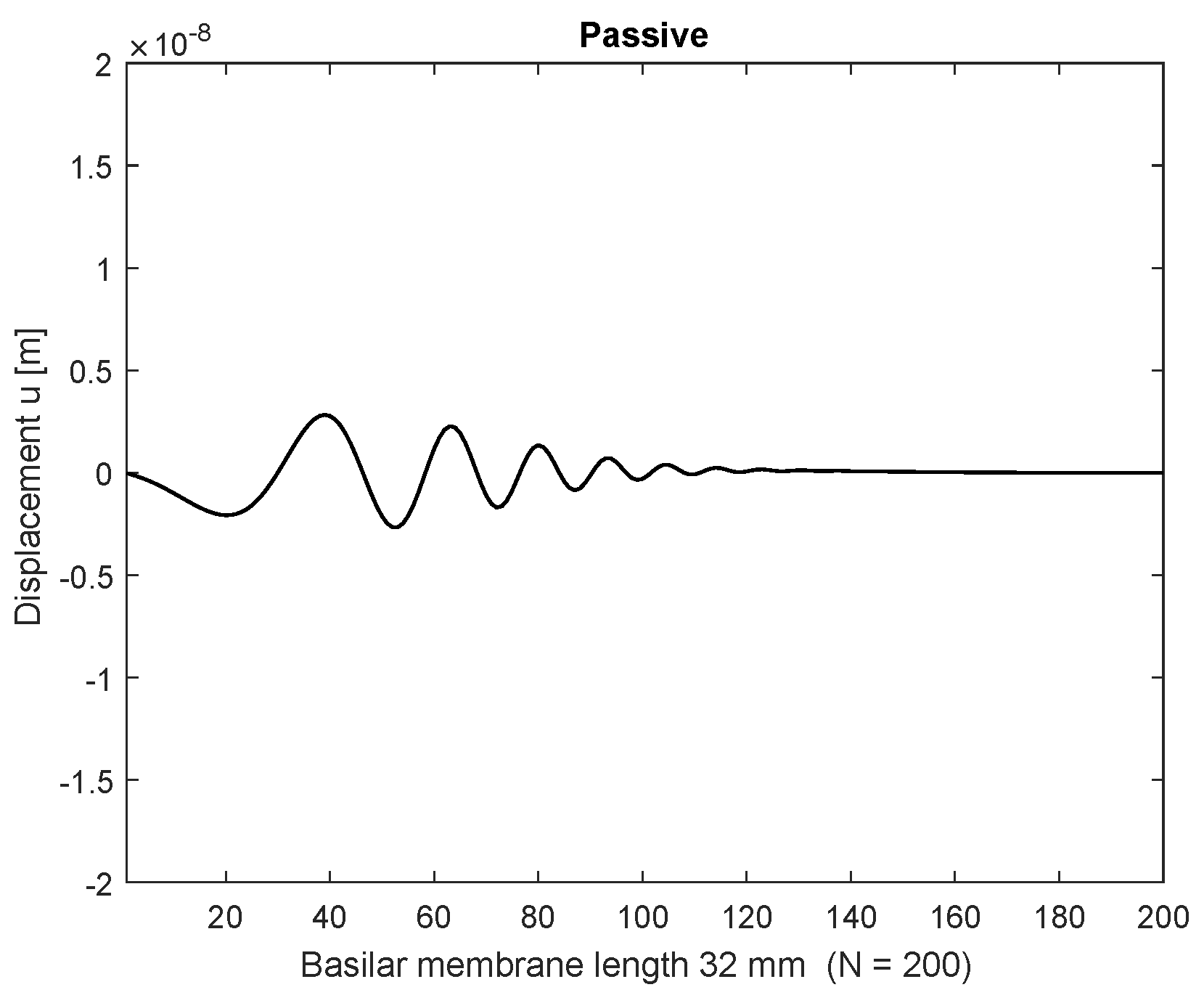
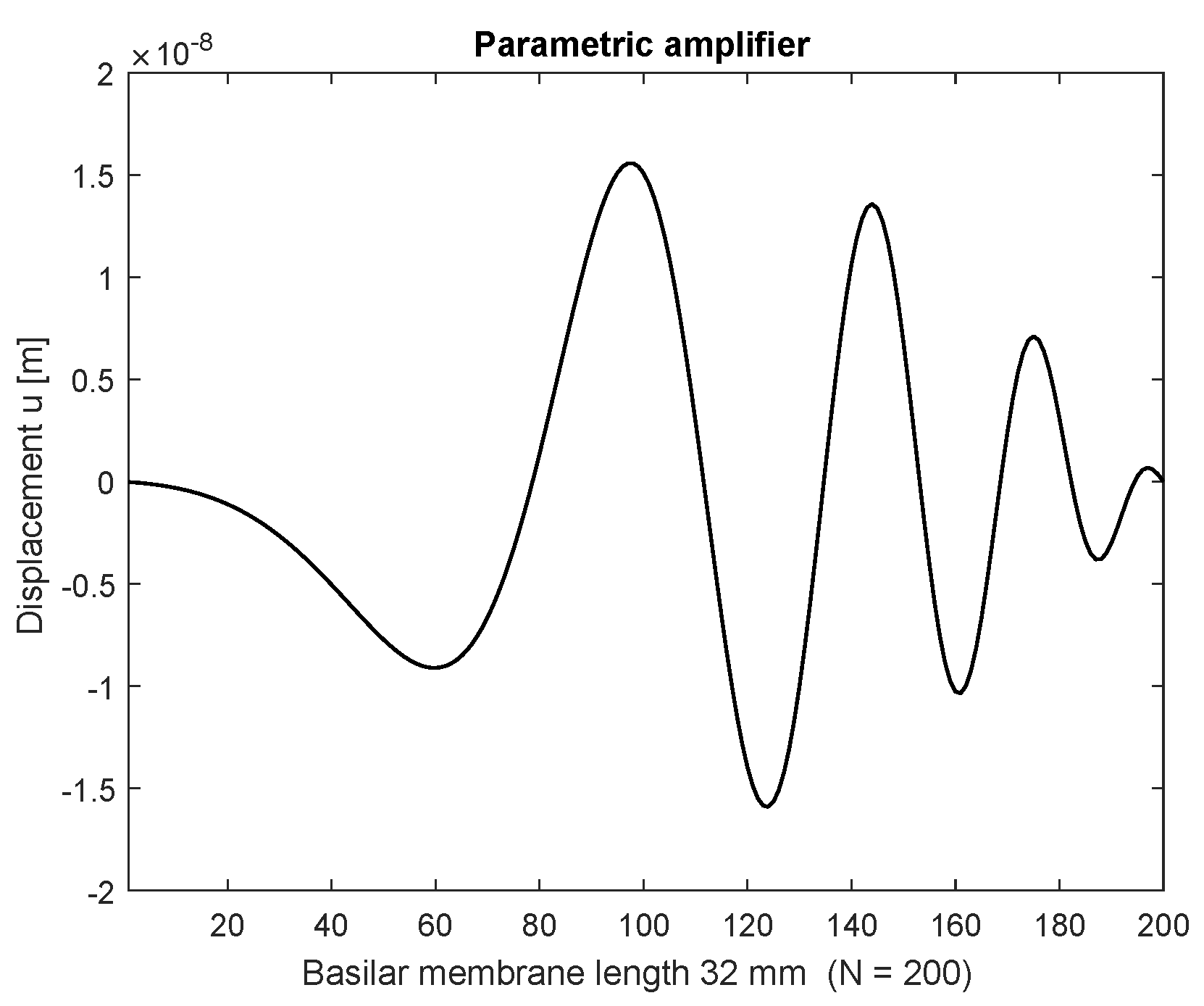
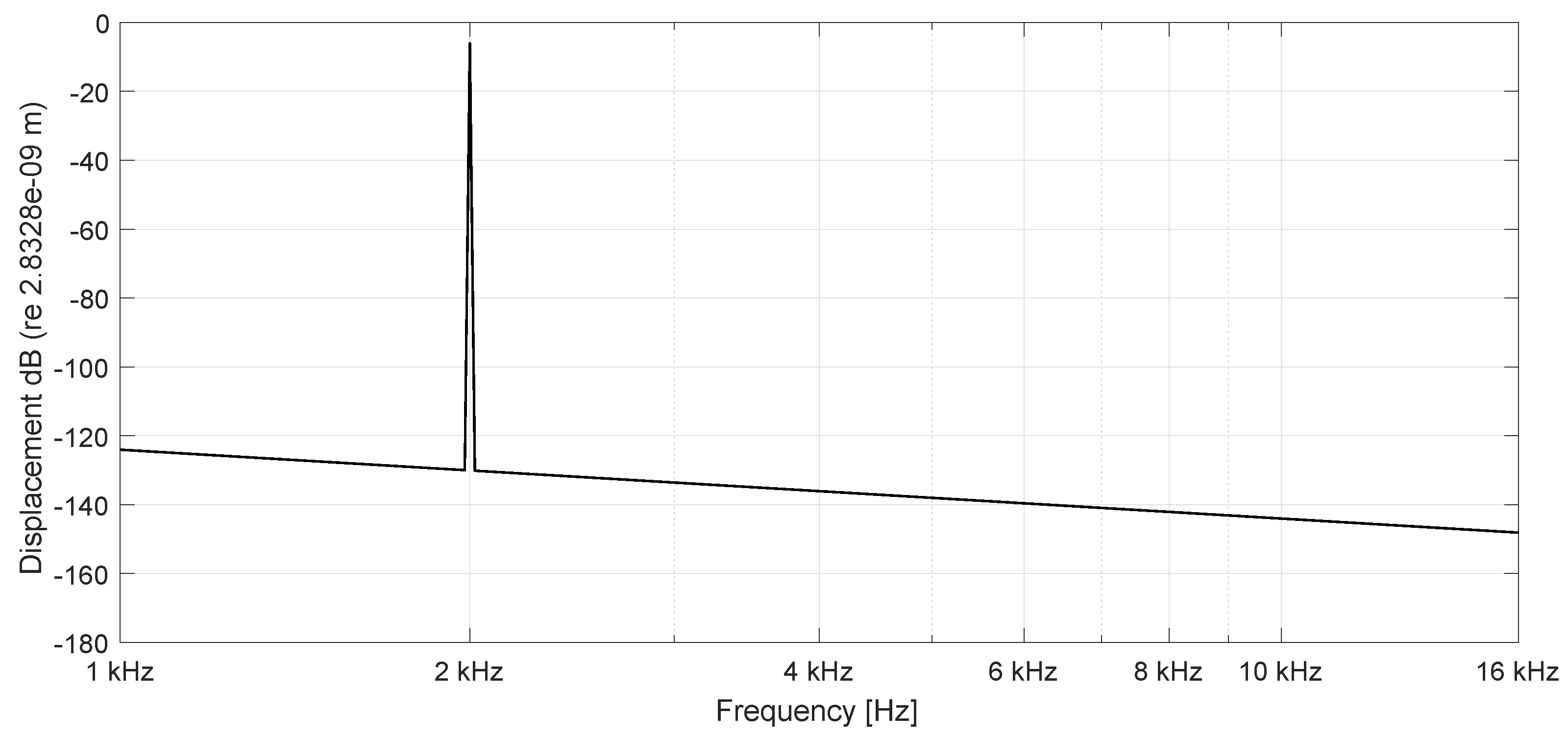
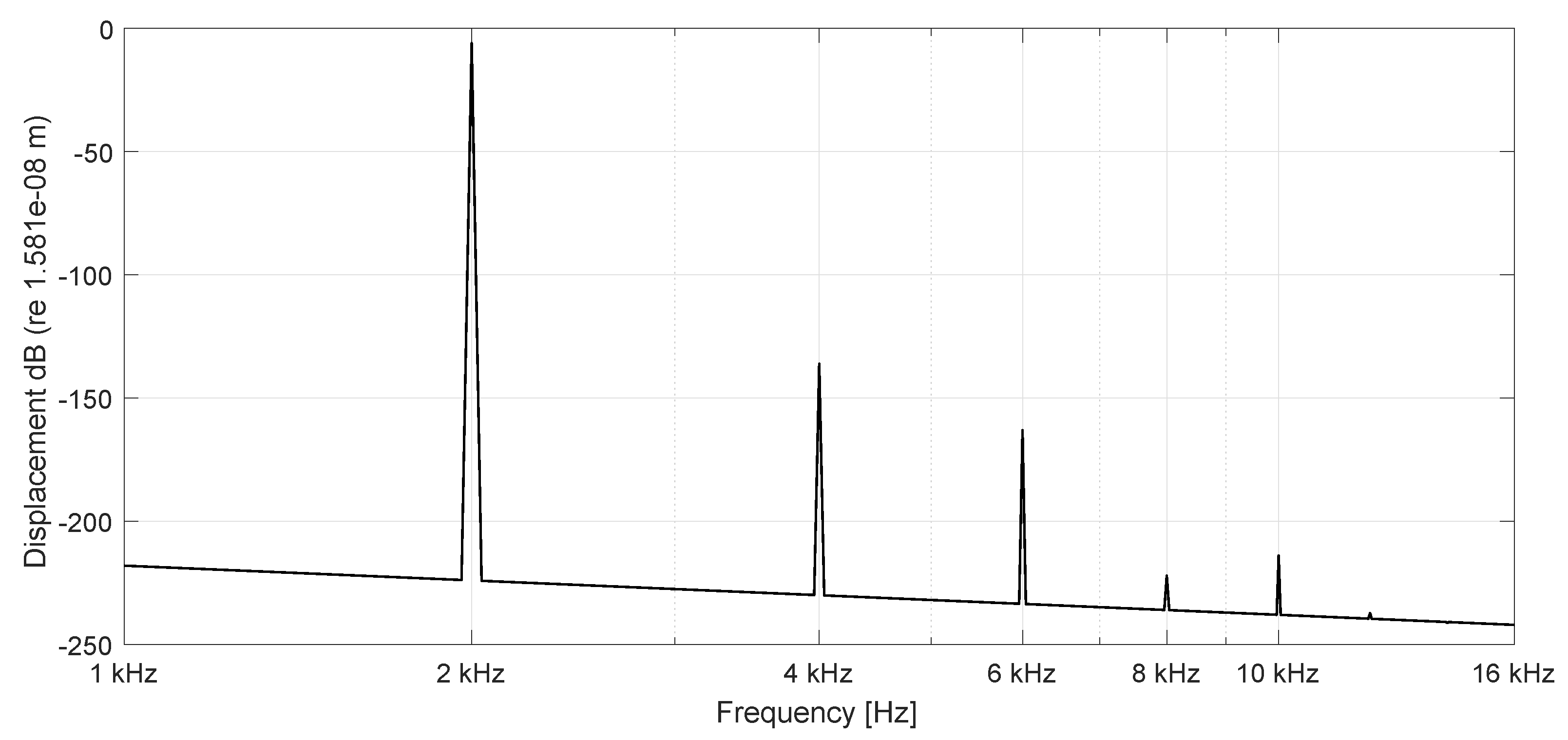
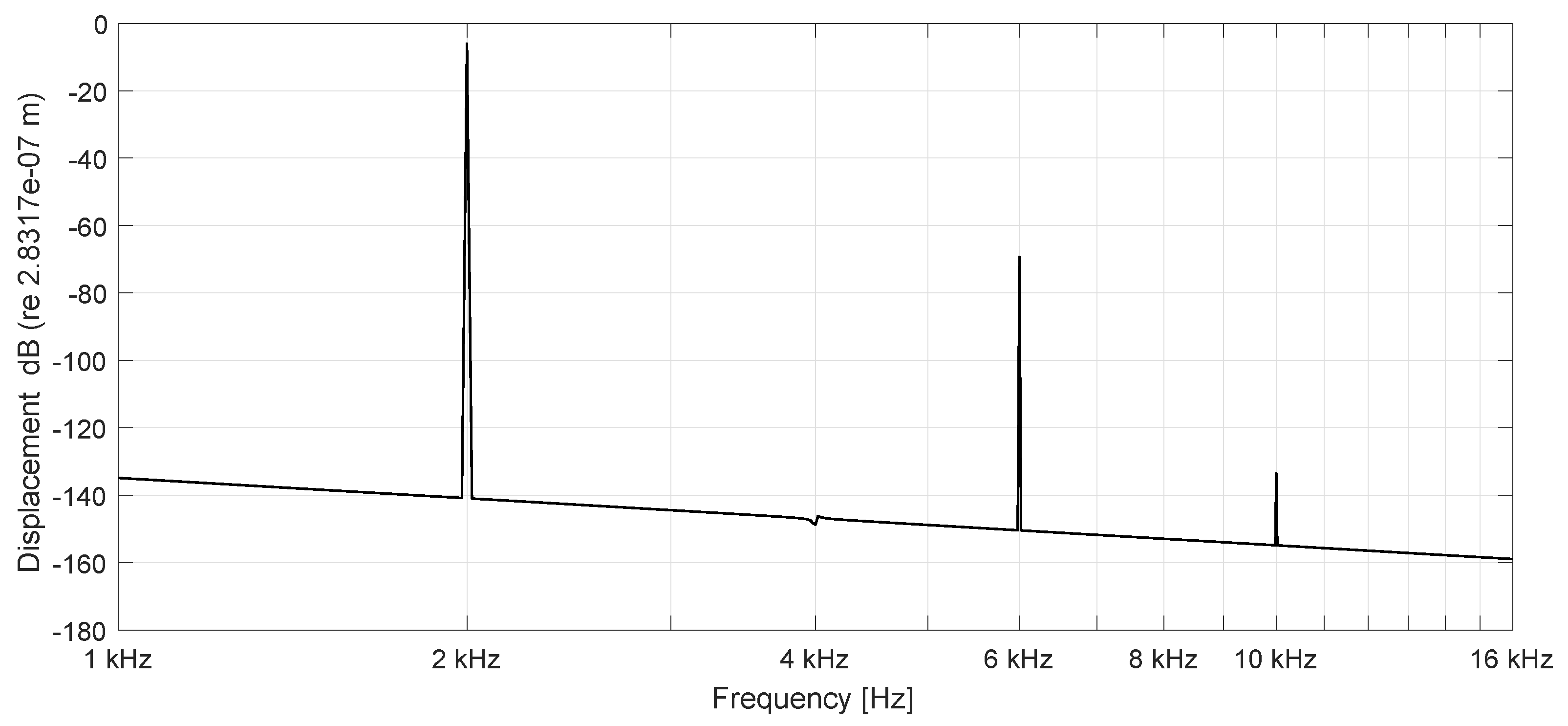
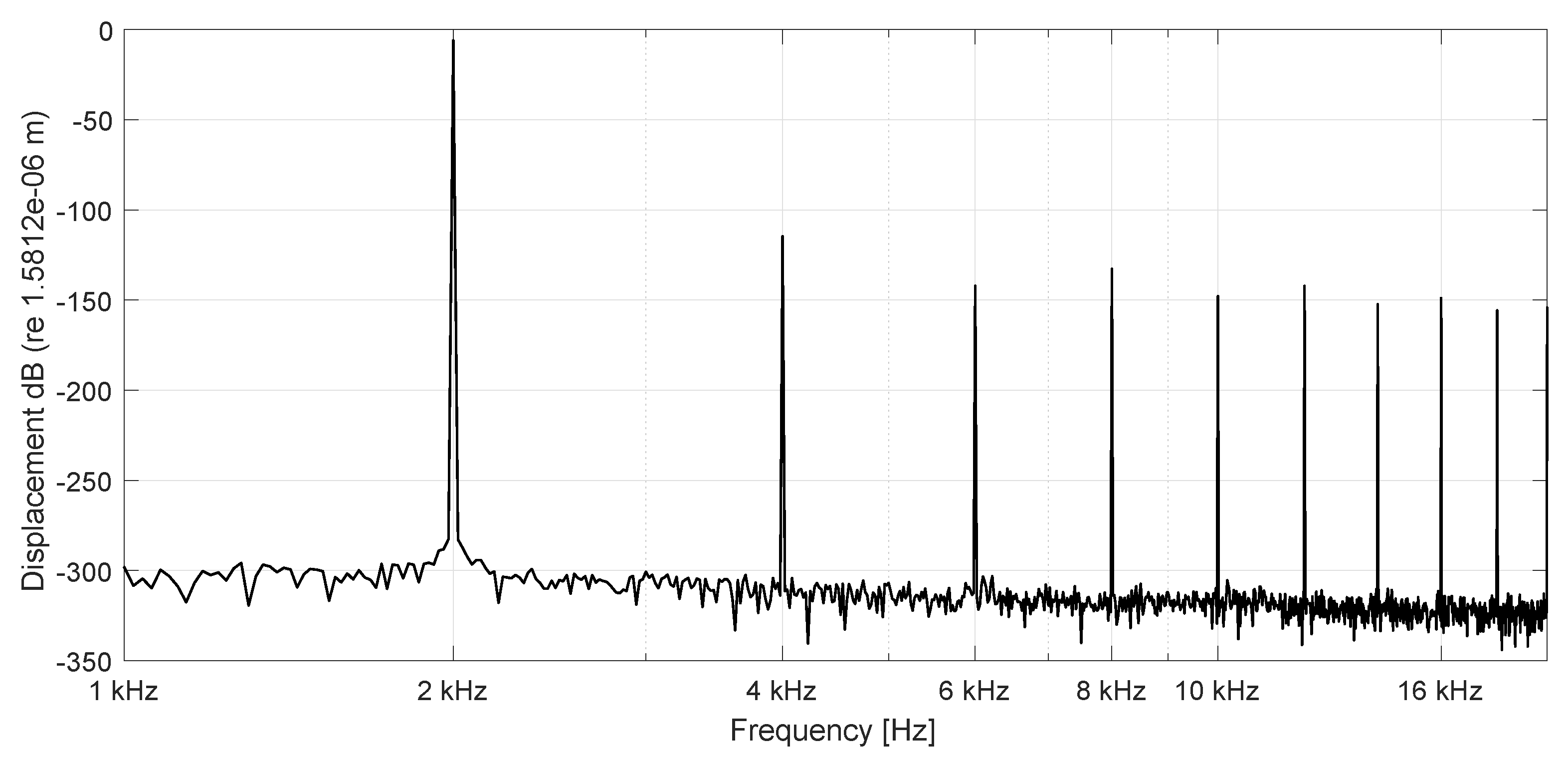
3. Results
4. Discussion and Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Melde, F. Über die Erregung stehender Wellen eines fadenförmigen Körpers [On the excitation of standing waves on a string]. Annalen der Physik und Chemie (Ser. 2) 1859, 109, 193–215. [Google Scholar]
- Cullen, A.L. Theory of the travelling-wave parametric amplifier. Proc. IEE-Part B 1959, 107, 101–107. [Google Scholar] [CrossRef][Green Version]
- Morse, P.M.; Ingard, K.U. Theoretical Acoustics; Princeton University Press: Princeton, NJ, USA, 1986. [Google Scholar]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; Pure & Applied Mathematics; John Wiley & Sons: New York, NY, USA, 1979. [Google Scholar]
- Cheng, X.; Blanchard, A.; Tan, C.A.; Lu, H.; Bergman, L.A.; McFarland, D.M.; Vakakis, A.F. Separation of traveling and standing waves in a finite dispersive string with partial or continuous viscoelastic foundation. J. Sound Vibr. 2017, 411, 193–209. [Google Scholar] [CrossRef]
- Tan, C.A.; Yang, B.; Mote, C.D., Jr. More On the vibration of a translating string coupled to hydrodynamic bearings. J. Vib. Acoust. 1990, 112, 337–345. [Google Scholar] [CrossRef]
- Huang, F.Y.; Mote, C.D., Jr. On the translating damping caused by a thin viscous fluid layer between a translating string and a translating rigid surface. J. Vib. Acoust. 1995, 181, 251–260. [Google Scholar] [CrossRef]
- Böhnke, F.; Janssen, T.; Steinhoff, H.-J.; Zimmermann, P. Structural Cochlear Model including the Coupling of Basilar Membrane Fibers in the Longitudinal Direction. Il Valsalva 1989, 54 (Suppl. 1), 10–16. [Google Scholar]
- Elliott, S.J. Wave propagation in a constrained fluid layer bounded by an elastic half-space and its relevance in cochlear micromechanics. J. Sound Vib. 2007, 305, 918–924. [Google Scholar] [CrossRef]
- Böhnke, F.; Semmelbauer, S. Acoustic boundary layer attenuation in ducts with rigid and elastic walls applied to cochlear mechanics. J. Fluids Eng. 2017, 139, 101202. [Google Scholar] [CrossRef]
- Najafi, H.S.; Izadi, F. Comparison of two finite-difference methods for solving the damped wave equation. Int. J. Math. Eng. Sci. 2014, 3, 35–49. [Google Scholar]
- Preyer, S.; Gummer, A.W. Nonlinearity of mechanoelectrical transduction of outer hair cells as the source of nonlinear basilar-membrane motion and loudness recruitment. Audiol. Neuro-Otol. 1996, 1, 3–11. [Google Scholar] [CrossRef]
- He, D.Z.; Dallos, P. Somatic stiffness of cochlear outer hair cells is voltage-dependent. Proc. Natl. Acad. Sci. USA 1999, 96, 8223–8228. [Google Scholar] [CrossRef]
- Deo, N.; Grosh, K. Two-State Model for Outer Hair Cell Stiffness. Biophys. J. 2004, 86, 3519–3528. [Google Scholar] [CrossRef] [PubMed]
- Rhode, W.S.; Robles, L. Evidence from Mössbauer experiments for nonlinear vibration in the cochlea. J. Acoust. Soc. Am. 1974, 55, 588–596. [Google Scholar] [CrossRef] [PubMed]
- Ko, W.; Stockie, J.M. An immersed boundary model of the cochlea with parametric forcing. SIAM J. Appl. Math. 2015, 75, 1065–1089. [Google Scholar] [CrossRef][Green Version]
- Kielczynski, P. Power amplification and selectivity in the cochlear amplifier. Arch. Acoust. 2013, 38, 83–92. [Google Scholar] [CrossRef]
- Böhnke, F.; Arnold, W. Mechanics of the Organ of Corti Caused by Deiters Cells. IEEE Trans. Biomed. Eng. 1998, 45, 1227–1233. [Google Scholar] [CrossRef] [PubMed]
- von Békésy, G. Zur Theorie des Hörens, Die Schwingungsform der Basilarmembran. Physikalische Zeitschrift 1928, 29, 793–810. [Google Scholar]
- Dong, W. Simultaneous intracochlear pressure measurements from two cochlear locations: Propagation of distortion products in gerbil. J. Assoc. Res. Otolaryngol. 2017, 18, 209–225. [Google Scholar] [CrossRef][Green Version]
- Kemp, D.T. Evidence of mechanical nonlinearity and frequency selective wave amplification in the cochlea. Arch. Otorhinolaryngol. 1979, 224, 37–45. [Google Scholar] [CrossRef]
- Zwicker, E. A hardware cochlear nonlinear preprocessing model with active feedback. J. Acoust. Soc. Am. 1986, 80, 146–153. [Google Scholar] [CrossRef]
- Verhulst, S.; Dau, T.; Shera, C.A. Nonlinear time-domain cochlear model for transient stimulation and human otoacoustic emission. J. Acoust. Soc. Am. 2012, 132, 3842–3848. [Google Scholar] [CrossRef] [PubMed]
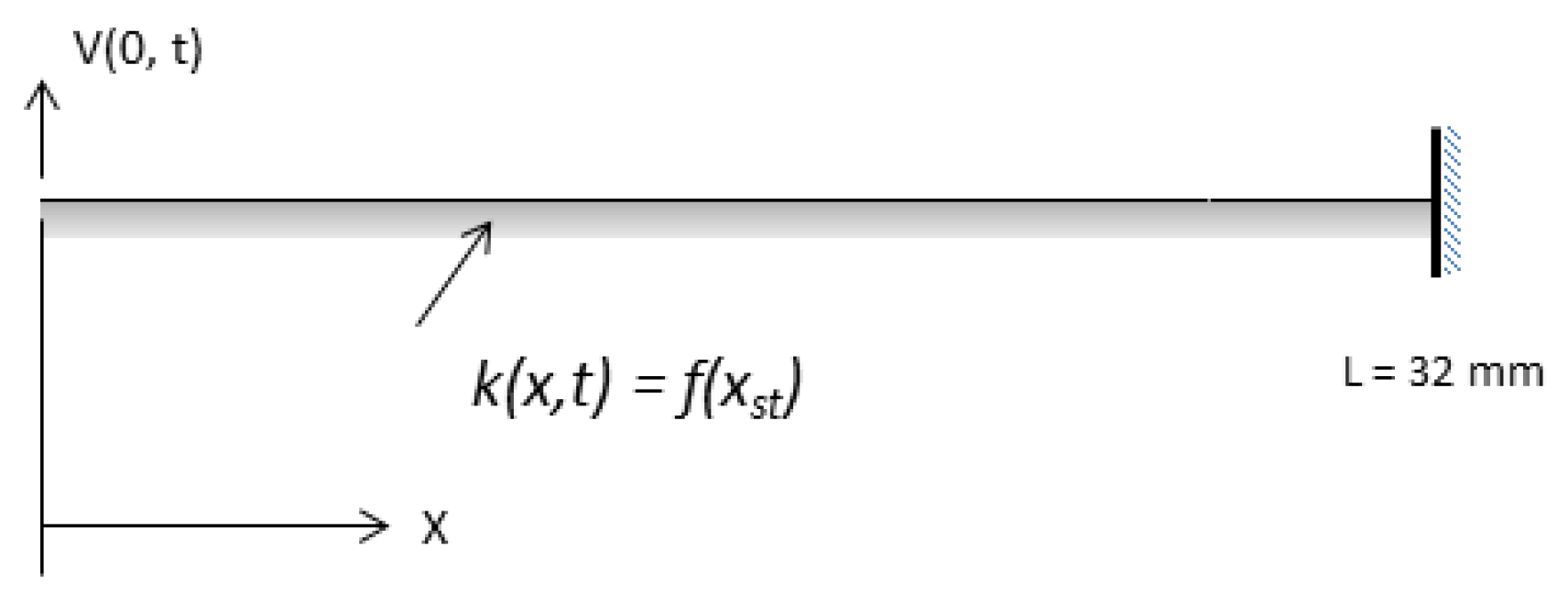
| Symbol | Denotation | Value Unit |
|---|---|---|
| fluid density per unit area | 1000 kg m | |
| b(x) | BM width | 0.1–0.5 mm |
| u(x,t) | BM displacement | m |
| damping constant | 4000 kg m s | |
| cubic nonlinearity constant | kg m | |
| transverse Young’s modulus | 100 GPa | |
| variable Young’s modulus | Pa | |
| h | BM thickness | 10 m |
| S | cross section area of canal | 1 mm |
| L | BM length | 32 mm |
| Symbol | Denotation | Value Unit |
|---|---|---|
| OHC membrane potential | mV | |
| OHC membrane potential for maximum hyperpolarization | mV | |
| force factors | 60 fN, 120 fN | |
| displacements | 56.8 nm, 27.3 nm | |
| Boltzmann-constant temperature (energy) | J | |
| stereocilia displacement | m | |
| OHC stiffness | 0.001 Nm | |
| constants | ||
| constants | ||
| factor |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Böhnke, F. Nonlinear Distortions and Parametric Amplification Generate Otoacoustic Emissions and Increased Hearing Sensitivity. Acoustics 2019, 1, 608-617. https://doi.org/10.3390/acoustics1030036
Böhnke F. Nonlinear Distortions and Parametric Amplification Generate Otoacoustic Emissions and Increased Hearing Sensitivity. Acoustics. 2019; 1(3):608-617. https://doi.org/10.3390/acoustics1030036
Chicago/Turabian StyleBöhnke, Frank. 2019. "Nonlinear Distortions and Parametric Amplification Generate Otoacoustic Emissions and Increased Hearing Sensitivity" Acoustics 1, no. 3: 608-617. https://doi.org/10.3390/acoustics1030036
APA StyleBöhnke, F. (2019). Nonlinear Distortions and Parametric Amplification Generate Otoacoustic Emissions and Increased Hearing Sensitivity. Acoustics, 1(3), 608-617. https://doi.org/10.3390/acoustics1030036





