Numerical Simulations of the Nonlinear Interaction of a Bubble Cloud and a High Intensity Focused Ultrasound Field
Abstract
1. Introduction
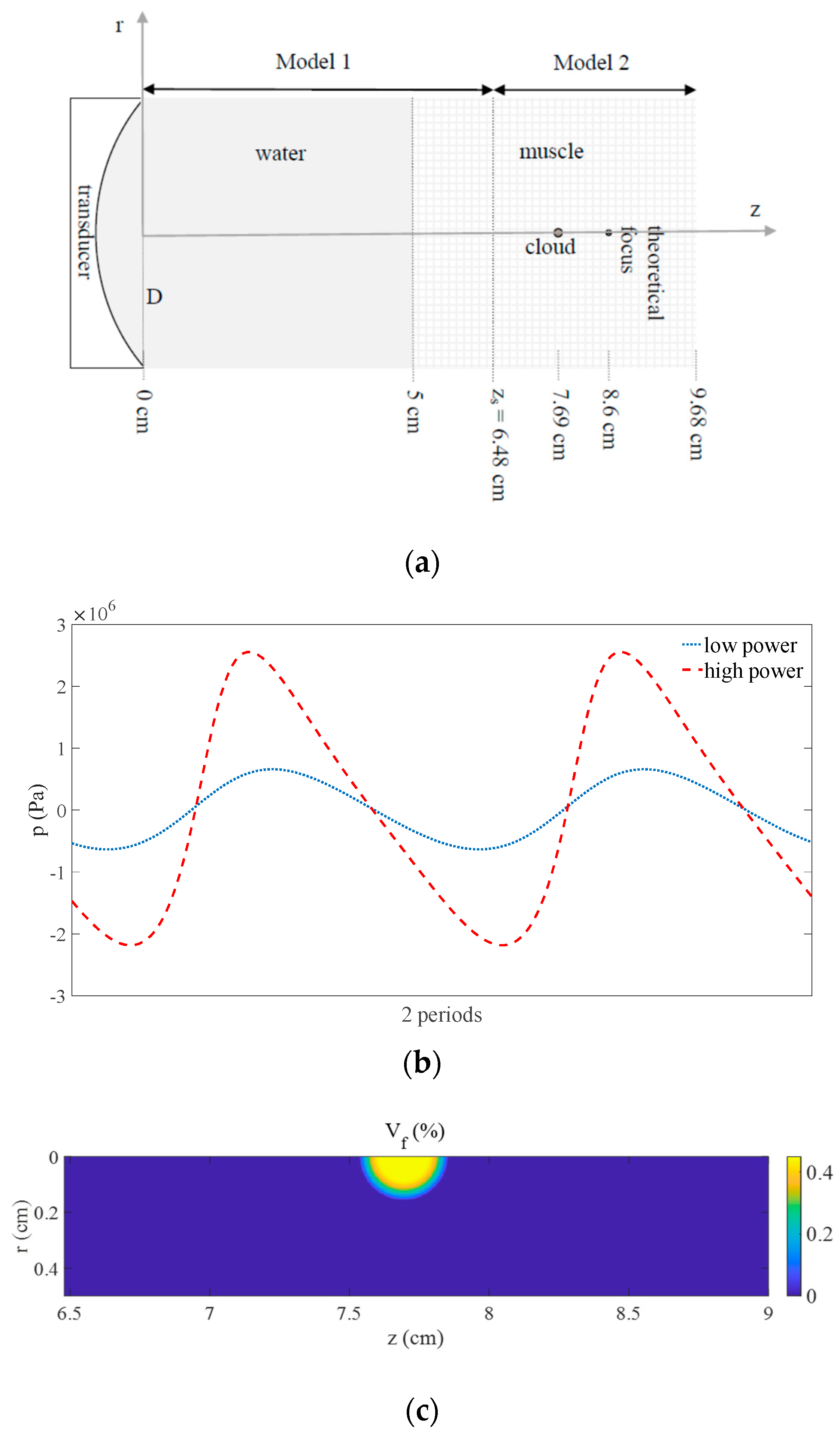
2. Materials and Methods
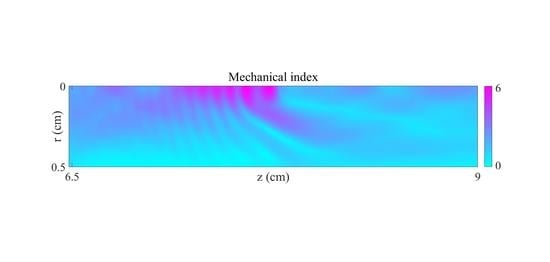
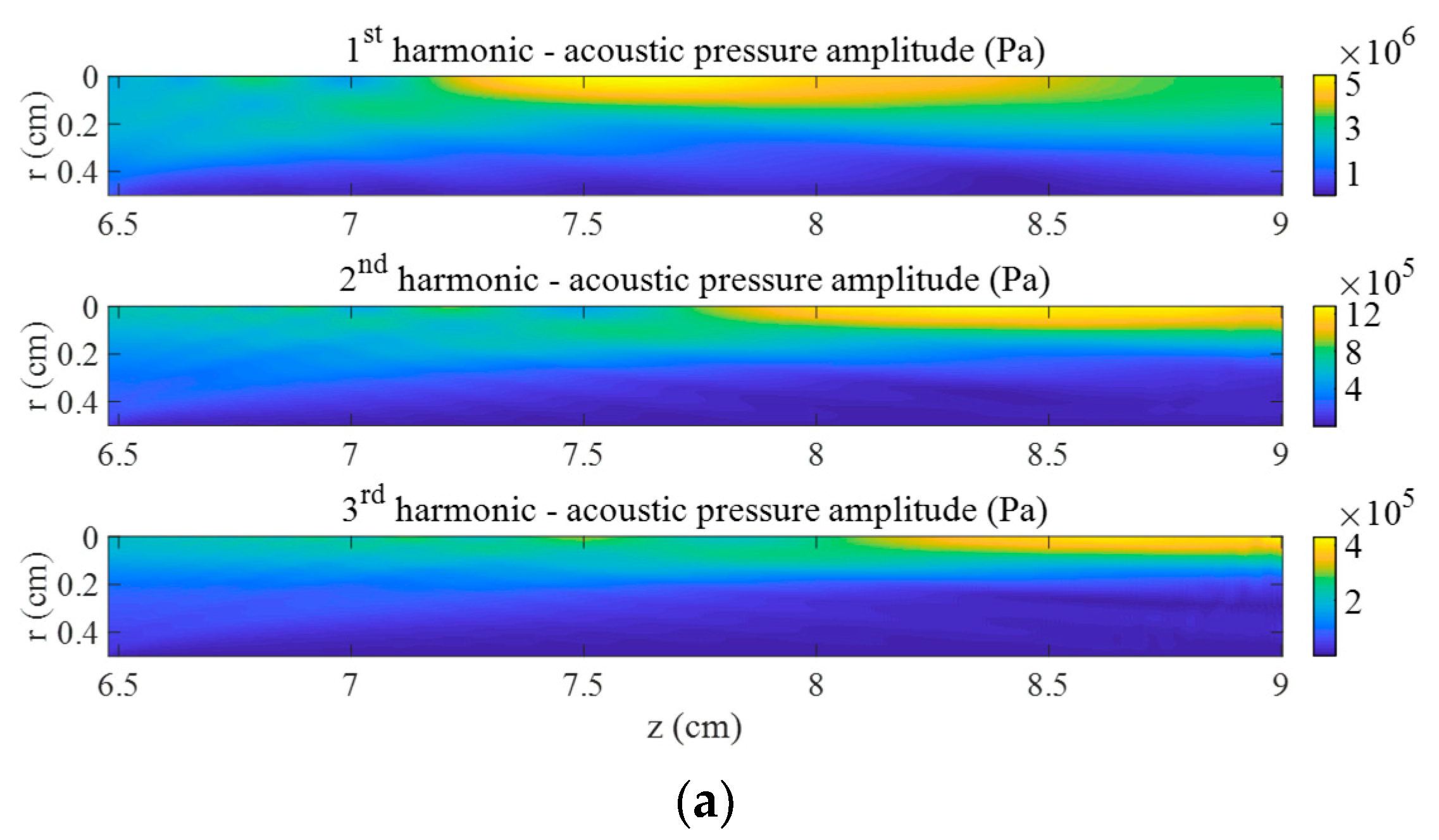
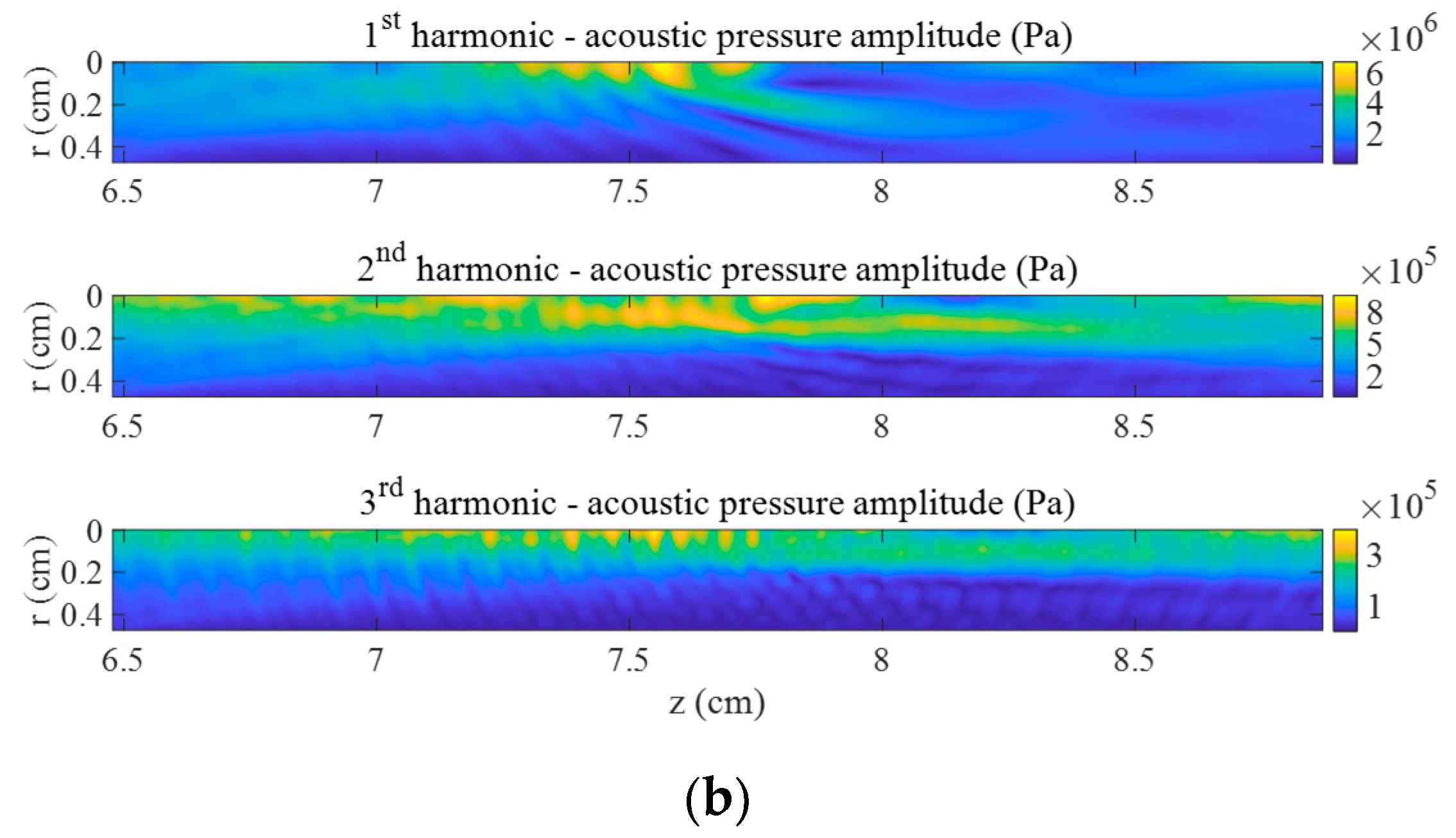
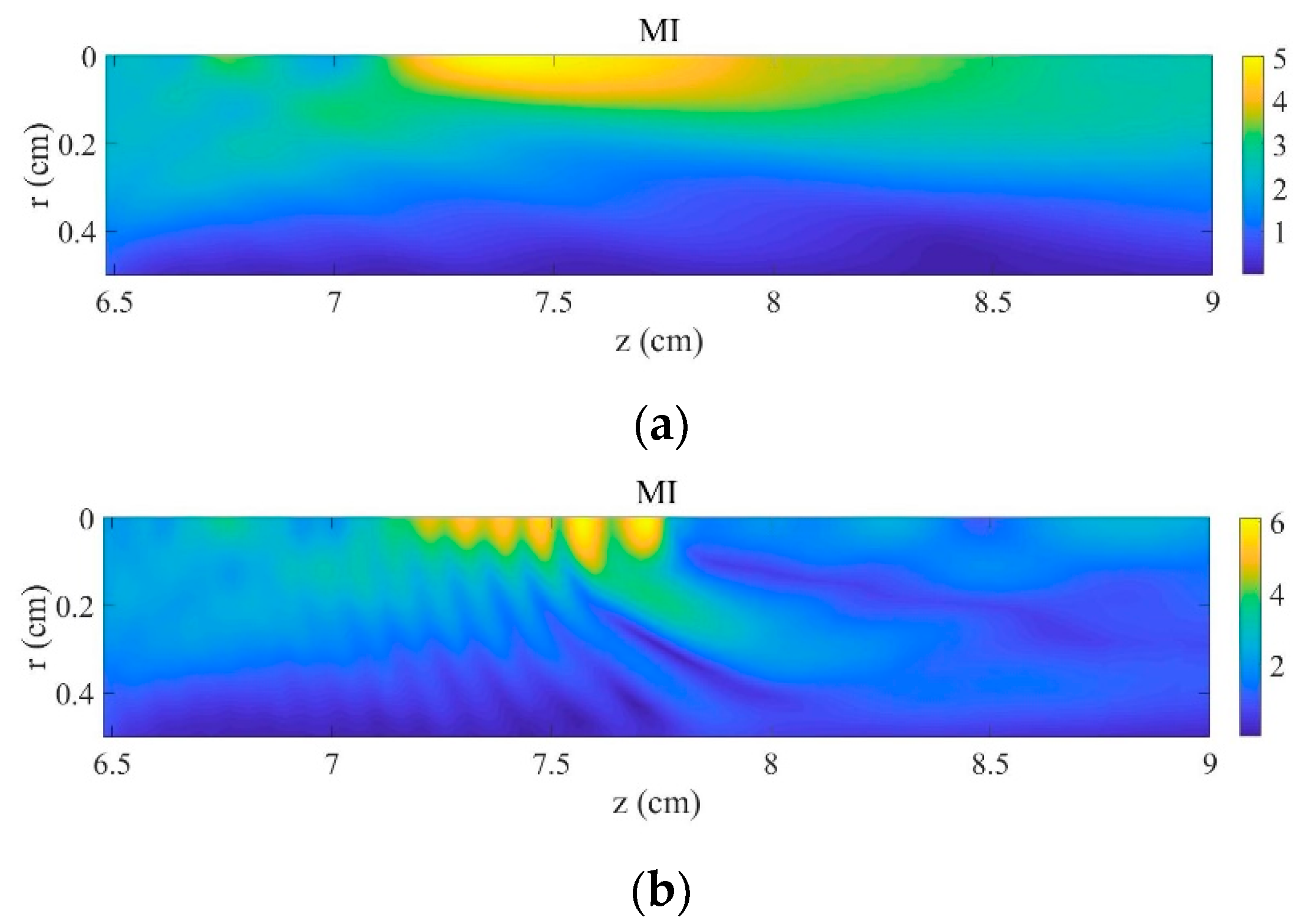
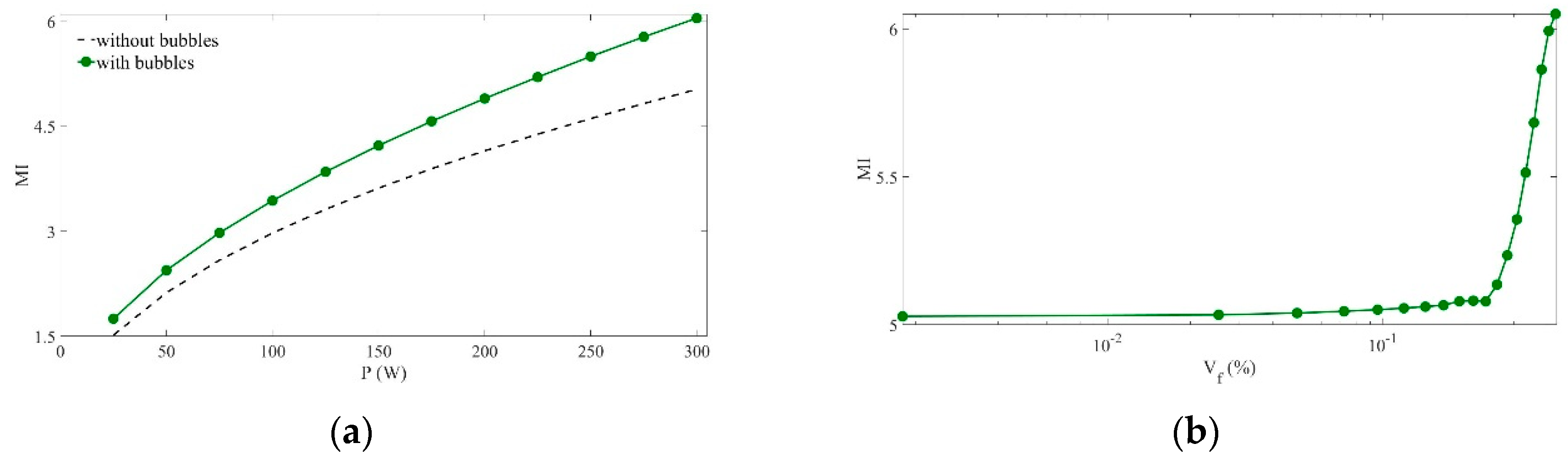
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tempany, C.M.C.; Stewart, E.A.; McDannold, N.; Quade, B.J.; Jolesz, F.A.; Hynynen, K. MR imaging-guided focused ultrasound surgery of uterine leiomyomas: A feasibility study. Radiology 2003, 226, 897–905. [Google Scholar] [CrossRef] [PubMed]
- Chapman, A.; ter Haar, G. Thermal ablation of uterine fibroids using MR-guided focused ultrasound: A truly non-invasive treatment modality. Eur. Radiol. 2007, 17, 2505–2511. [Google Scholar] [CrossRef] [PubMed]
- Gianfelice, D.G.; Gupta, C.; Kucharczyk, W.; Bret, P.; Havill, D.; Clemons, M. Palliative treatment of painful bone metastases with MR imaging-guided focused ultrasound. Radiology 2008, 249, 355–363. [Google Scholar] [CrossRef] [PubMed]
- Maestroni, U.; Ziveri, M.; Azzolini, N.; Dinale, F.; Ziglioli, F.; Campaniello, G.; Frattini, A.; Ferretti, S. High intensity focused ultrasound (HIFU): A useful alternative choice in prostate cancer treatment. Preliminary results. Acta Biomed. 2008, 79, 211–216. [Google Scholar]
- Furusawa, H.; Namba, K.; Nakahara, H.; Tanaka, C.; Yasuda, Y.; Hirabara, E.; Imahariyama, M.; Komaki, K. The evolving non-surgical ablation of breast cancer: MR guided focused ultrasound (MRgFUS). Breast Cancer 2007, 14, 55–58. [Google Scholar] [CrossRef]
- Jeanmonod, D.; Werner, B.; Morel, A.; Michels, L.; Zadicario, E.; Schiff, G.; Martin, E. Transcranial magnetic resonance imaging-guided focused ultrasound: noninvasive central lateral thalamotomy for chronic neuropathic pain. Neurosurg. Focus 2012, 32, E1. [Google Scholar] [CrossRef]
- Elias, W.J.; Huss, D.; Voss, T.; Loomba, J.; Khaled, M.; Zadicario, E.; Frysinger, R.C.; Sperling, S.A.; Wylie, S.; Monteith, S.J.; et al. A pilot study of focused ultrasound thalamotomy for essential tremor. N. Engl. J. Med. 2013, 369, 640–648. [Google Scholar] [CrossRef]
- Lipsman, N.; Schwartz, M.L.; Huang, Y.; Lee, L.; Sankar, T.; Chapman, M.; Hynynen, K.; Lozano, A.M. MR-guided focused ultrasound thalamotomy for essential tremor: A proof-of-concept study. Lancet Neurol. 2013, 12, 462–468. [Google Scholar] [CrossRef]
- Chung, A.H.; Hynynen, K.; Colucci, V.; Oshio, K.; Cline, H.E.; Jolesz, F.A. Optimization of spoiled gradient-echo phase imaging for in vivo localization of a focused ultrasound beam. Magn. Reson. Med. 1996, 36, 745–752. [Google Scholar] [CrossRef]
- Young, F.R. Cavitation; McGraw-Hill: London, UK, 1989; Available online: https://www.worldcat.org/title/cavitation/oclc/19521628 (accessed on 20 September 2019).
- Bai, L.; Xu, W.; Deng, J.; Li, C.; Xu, D.; Gao, Y. Generation and control of acoustic cavitation structure. Ultrason. Sonochem. 2014, 21, 1696–1706. [Google Scholar] [CrossRef]
- Zhao, L.-Y.; Zou, J.Z.; Chen, Z.-G.; Liu, S.; Jiao, J.; Wu, F. Acoustic cavitation enhances focused ultrasound ablation with phase-shift inorganic perfluorohexane nanoemulsions: An in vitro study using a clinical device. Biomed. Research Int. 2016, 2016, 7936902. [Google Scholar] [CrossRef][Green Version]
- Hi, M.; Zhong, Z.; Li, X.; Gong, X.; Wang, Z.; Li, F. Effects of different hydrostatic pressure on lesions in ex vivo bovine livers induced by high intensity focused ultrasound. Ultrason. Sonochem. 2017, 36, 36–41. [Google Scholar]
- Wang, M.; Lei, Y.; Zhou, Y. High-intensity focused ultrasound (HIFU) ablation by the frequency chirps: Enhanced thermal field and cavitation at the focus. Ultrasonics 2019, 91, 134–149. [Google Scholar] [CrossRef]
- Qiao, Y.; Yin, H.; Chang, N.; Wan, M. Phase-shift nano-emulsions induced cavitation and ablation during high intensity focused ultrasound exposure, Proceeding from the 41th International Symposium on Therapeutic Ultrasound. AIP Conf. Proc. 2017, 1821, 040001. [Google Scholar]
- Pishchalnikov, Y.A.; Williams, J.C., Jr.; McAteer, J.A. Bubble proliferation in the cavitation field of a shock wave lithotripter. J. Acoust. Soc. Am. 2011, 130, EL87–EL93. [Google Scholar] [CrossRef]
- Gyöngy, M.; Coussios, C.C. Passive cavitation mapping for localization and tracking of bubble dynamics. J. Acoust. Soc. Am. 2010, 128, EL175–EL180. [Google Scholar] [CrossRef]
- Lo, A.H.; Kripfgans, O.D.; Carson, P.L.; Fowlkes, J.B. Spatial control of gas bubbles and their effects on acoustic fields. Ultrasound Med. Biol. 2006, 32, 95–106. [Google Scholar] [CrossRef]
- Zhang, S.; Li, C.; Zhou, F.; Wan, M.; Wang, S. Enhanced lesion-to-bubble ratio on ultrasonic Nakagami imaging for monitoring of high-intensity focused ultrasound. J. Ultrasound Med. 2014, 33, 959–970. [Google Scholar] [CrossRef]
- Elbes, D.; Denost, Q.; Robert, B.; Köhler, M.O.; Tanter, M.; Bruno, Q. Magnetic resonance imaging for the exploitation of bubble-enhanced heating by high-intensity focused ultrasound: a feasibility study. Ultrasound Med. Biol. 2014, 40, 956–964. [Google Scholar] [CrossRef]
- Hosseini, S.H.R.; Zheng, X.; Vaezy, S. Effects of gas pockets on high-intensity focused ultrasound field. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2011, 58, 1203–1210. [Google Scholar] [CrossRef]
- Sapareto, S.; Dewey, W. Thermal dose determination in cancer therapy. Int. J. Radiat. Oncol. Biol. Phys. 1984, 10, 787–800. [Google Scholar] [CrossRef]
- Williams, R.; Cherin, E.; Lam, T.Y.J.; Tavakkoli, J.; Zemp, R.J.; Foster, F.S. Nonlinear ultrasound propagation through layered liquid and tissue-equivalent media: computational and experimental results at high frequency. Phys. Med. Biol. 2006, 51, 5809–5824. [Google Scholar] [CrossRef]
- Canney, M.S.; Bailey, M.R.; Crum, L.A.; Khokhlova, V.A.; Sapozhnikov, O.A. Acoustic characterization of high intensity focused ultrasound fields: a combined measurement and modeling approach. J. Acoust. Soc. Am. 2008, 124, 2406–2420. [Google Scholar] [CrossRef]
- Khokhlova, T.D.; Canney, M.S.; Lee, D.; Marro, K.I.; Crum, L.A.; Khokhlova, V.A.; Bailey, M.R. Magnetic resonance imaging of boiling induced by high intensity focused ultrasound. J. Acoust. Soc. Am. 2009, 125, 2420–2431. [Google Scholar] [CrossRef]
- Tavakkoli, J.; Cathignol, D.; Souchon, R.; Sapozhnikov, O.A. Modeling of pulsed finite-amplitude focused sound beams in time domain. J. Acoust. Soc. Am. 1998, 104, 2061–2072. [Google Scholar] [CrossRef]
- Liu, X.; Li, J.; Gong, X.; Zhang, D. Nonlinear absorption in biological tissue for high intensity focused ultrasound. Ultrasonics 2006, 44, e27–e30. [Google Scholar] [CrossRef]
- Zemp, R.J.; Tavakkoli, J.; Cobbold, R.S.C. Modeling of nonlinear ultrasound propagation in tissue from array transducers. J. Acoust. Soc. Am. 2003, 113, 139–152. [Google Scholar] [CrossRef]
- Zeng, X.; McGough, R.J. Evaluation of the angular spectrum approach for simulations of near-field pressures. J. Acoust. Soc. Am. 2008, 123, 68–76. [Google Scholar] [CrossRef]
- Jing, Y.; Cleveland, R.O. Modeling the propagation of nonlinear three dimensional acoustic beams in inhomogeneous media. J. Acoust. Soc. Am. 2007, 122, 1352–1364. [Google Scholar] [CrossRef]
- Yang, X.; Cleveland, R.O. Time domain simulation of nonlinear acoustic beams generated by rectangular pistons with application to harmonic imaging. J. Acoust. Soc. Am. 2005, 117, 113–123. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Hynynen, K. Computational aspects in high intensity ultrasonic surgery planning. Computerized Med. Imaging Graphics 2010, 34, 69–78. [Google Scholar] [CrossRef]
- Filnonenko, E.A.; Khokhlova, V.A. Effect of acoustic nonlinearity on heating of biological tissue by high-intensity focused ultrasound. Acoust. Phys. 2001, 47, 468–475. [Google Scholar] [CrossRef]
- Khokhlova, V.A.; Bailey, M.R.; Reed, J.A.; Cunitz, B.W.; Kaczkowski, P.J.; Crum, L.A. Effects of nonlinear propagation, cavitation, and boiling in lesion formation by high intensity focused ultrasound in a gel phantom. J. Acoust. Soc. Am. 2006, 119, 1834–1848. [Google Scholar] [CrossRef]
- Matula, T.J.; Cordry, S.M.; Roy, R.A.; Crum, L.A. Bjerknes force and bubble levitation under single-bubble sonoluminescence conditions. J. Acoust. Soc. Am. 1997, 102, 1522–1527. [Google Scholar] [CrossRef]
- Bakhtiari-Nejad, M.; Shahab, S. Effects of nonlinear propagation of focused ultrasound on the stable cavitation of a single bubble. Acoustics 2019, 1, 14–34. [Google Scholar] [CrossRef]
- Karpov, S.; Prosperetti, A.; Ostrovsky, L. Nonlinear oscillations of bubble layers. J. Acoust. Soc. Am. 2003, 113, 1304–1316. [Google Scholar] [CrossRef]
- Servant, G.; Laborde, J.L.; Hita, A.; Caltagirone, J.P.; Gérard, A. On the interaction between ultrasound waves and bubble clouds in mono- and dual-frequency sonoreactors. Ultrason. Sonochem. 2003, 10, 347–355. [Google Scholar] [CrossRef]
- Vanhille, C.; Campos-Pozuelo, C. Nonlinear ultrasonic propagation in bubbly liquids: a numerical model. Ultrasound Med. Biol. 2008, 34, 792–808. [Google Scholar] [CrossRef]
- Vanhille, C.; Campos-Pozuelo, C. Nonlinear ultrasonic waves in bubbly liquids with nonhomogeneous bubble distribution: numerical experiments. Ultrason. Sonochem. 2009, 16, 669–685. [Google Scholar] [CrossRef]
- Vanhille, C.; Campos-Pozuelo, C. Numerical simulations of three-dimensional nonlinear acoustic waves in bubbly liquids. Ultrason. Sonochem. 2013, 20, 963–969. [Google Scholar] [CrossRef]
- Hamilton, M.F.; Il’Inskii, Y.A.; Zabolotskaya, E.A. Dispersion. In Nonlinear Acoustics; Hamilton, M.F., Blackstock, D.T., Eds.; Academic Press: San Diego, CA, USA, 1998; pp. 151–175. [Google Scholar]
- Saito, S. Ultrasound field and bubbles. In Sonochemistry and the Acoustic Bubble; Grieser, F., Choi, P.K., Enomoto, N., Harada, H., Okitsu, K., Yasui, K., Eds.; Elsevier: Amsterdam, The Netherlands, 2015; pp. 11–39. [Google Scholar]
- Vanhille, C.; Pulkkinen, A.; Hynynen, K.; Campos-Pozuelo, C. Efectos de una nube de burbujas en un campo HIFU. In Proceedings of the Congress on Numerical Methods in Engineering, Bilbao, Spain, 25–28 June 2013; Universidad del País Vasco: Bilbao, Spain. [Google Scholar]
- Blackstock, D.T. Fundamentals of Physical Acoustics; John Wiley and Sons: New York, NY, USA, 2000. [Google Scholar]
- Vanhille, C.; Campos-Pozuelo, C. Nonlinear interaction of air bubbles and ultrasonic field: an analysis of some physical aspects. In Recent Development in Nonlinear Acoustics, AIP Conference Proceedings 1685; Blanc-Benon, P., Sparrow, V.W., Dragna, D., Eds.; American Institute of Physics Publishing: Melville, NY, USA, 2015; p. 050008. [Google Scholar]
- Verweij, M.D.; Huijssen, J. Computational methods for nonlinear acoustic wavefields in homogeneous media. In Computational Methods in Nonlinear Acoustics: Current Trends; Vanhille, C., Campos-Pozuelo, C., Eds.; Research Signpost: Kerala, India, 2011; pp. 1–19. [Google Scholar]
- Naugolnykh, K.; Ostrovsky, L. Nonlinear Wave Processes in Acoustics; Cambridge University Press: New York, NY, USA, 1998. [Google Scholar]
- Zabolotskaya, E.A.; Soluyan, S.I. Emission of harmonic and combination-frequency waves by air bubbles. Sov. Phys. Acoust. 1973, 18, 396–398. [Google Scholar]
- Wu, J.; Nyborg, W.L. Emerging Therapeutic Ultrasound; World Scientific: Hackensack, NJ, USA, 2006. [Google Scholar]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vanhille, C.; Hynynen, K. Numerical Simulations of the Nonlinear Interaction of a Bubble Cloud and a High Intensity Focused Ultrasound Field. Acoustics 2019, 1, 825-836. https://doi.org/10.3390/acoustics1040049
Vanhille C, Hynynen K. Numerical Simulations of the Nonlinear Interaction of a Bubble Cloud and a High Intensity Focused Ultrasound Field. Acoustics. 2019; 1(4):825-836. https://doi.org/10.3390/acoustics1040049
Chicago/Turabian StyleVanhille, Christian, and Kullervo Hynynen. 2019. "Numerical Simulations of the Nonlinear Interaction of a Bubble Cloud and a High Intensity Focused Ultrasound Field" Acoustics 1, no. 4: 825-836. https://doi.org/10.3390/acoustics1040049
APA StyleVanhille, C., & Hynynen, K. (2019). Numerical Simulations of the Nonlinear Interaction of a Bubble Cloud and a High Intensity Focused Ultrasound Field. Acoustics, 1(4), 825-836. https://doi.org/10.3390/acoustics1040049