Experience-Based Physico-Chemical Models for Long-Term Reinforcement Corrosion

Abstract

The long-term corrosion progression of steel reinforcement is important for estimating the life of reinforced concrete infrastructure. Reviews of field experience and results from recent controlled long-term experiments show that the development of reinforcement corrosion is much more complex than the classical empirical Tuutti model. A new, comprehensive model is proposed, referencing observations and inferences from many field and laboratory observations and built on the bi-modal model for the corrosion of steel. It includes the critical roles of air-voids in the concrete at the concrete-steel interface and the effect of long-term alkali leaching as accelerated by the presence of chlorides. Both are affected by compaction and concrete permeability. The role of chlorides in the early stages is confined to pitting within air-voids. These are critical for allowing initiation to occur, while their size influences the severity of early corrosion. Empirical data show that for seawater with an average water temperature in the range of 10–20 °C, the corresponding rate of long-term corrosion r_a is in the range of 0.012–0.015 mm/y.

1. Introduction

Reinforcement corrosion of marine structures can be a major problem for structural safety and serviceability. Despite much research attention over many years, the causes of such corrosion remain unclear: “after more than half a century of research on the issue of steel corrosion in concrete, many questions remain open” [1]. Seldom mentioned in these overviews is that a considerable amount of practical experience over many years has shown, repeatedly, that for many reinforced concrete structures exposed for decades in high chloride environments, reinforcement corrosion has not occurred or is negligible despite very high concentrations of chlorides at the reinforcement bars [2,3,4]. One example of this type of behavior is the set of some 900 driven reinforced concrete piles, constructed during the 1930s that were found, on extraction from their foundations in 2012, to show almost no evidence of reinforcement corrosion. During that time they had been exposed, continuously, to the immersion, tidal, splash, and atmospheric zones of the coastal Pacific Ocean [5]. The state of the reinforcement and the lack of corrosion for most of the surfaces of the reinforcing bars were verified by breaking open randomly selected piles. This showed the high density of the concrete, the lack of air-voids within the concrete and at the steel interface surfaces, and that the rusts that were present were very thin and of the type generated under very low oxygen conditions. Very high chloride concentrations were observed inside the concrete, including immediately adjacent to the steel reinforcement bars. The concrete cross-sections yielded pH values everywhere around 12 other than the pH around 7 in the 2–3 mm outer edges. This indicated that much of the concrete cross-sections still contained high levels of calcium hydroxide (Ca(OH)₂) even after about 80 years of exposure. There were some exceptions to this trend. The most notable was for one pile that showed very severe localized corrosion at the point where it was inferred that the pile had been deeply cracked in flexure at the time of construction [5].

Similar findings have been made recently [6] for the massive reinforced concrete Phoenix caissons hastily produced during WW2 and now lying abandoned along the coast of Normandy (F). Those that could be inspected directly or through aerial photography showed little or no obvious corrosion of reinforcement [7], despite having been exposed in the chloride-rich immersion, tidal, splash, and marine atmospheric zones since 1944. Reinforcement corrosion was evident mostly only where early structural damage (through a major storm event in 1944) had occurred or at poor construction joints. Parallel findings are available for a range of other practical reinforced concrete structures [3,8,9,10,11]. However, the conventional wisdom, based largely on laboratory research, appears focused primarily on cases of reinforced (and prestressed) concrete structures and laboratory samples that showed early initiation of reinforcement corrosion and relatively fast development of some type of structural damage.

The reasons for the poor performance of some practical reinforced concretes have become clearer as a result of recent long-term experimental findings [12,13]. These are reviewed briefly in the next section. They provide a background for critical aspects of initiation of reinforcement corrosion in marine conditions and for the subsequent rate of its progression. A new model for reinforcement corrosion progression as a function of longer-term exposures is then introduced, extended from an earlier empirical model [14] that was based on the empirical analysis of data from actual structures and on modern understanding of the development of corrosion of bare steel in seawater. The extended model proposed herein accounts for the current and new understanding of the relevant physico-chemical mechanisms and criteria.

As a first step in the calibration of the proposed model, it is compared with data from experiments conducted during the 1950s on a range of model reinforced concretes covering different water-cement and aggregate-cement ratios. Comments are made about the principal factors that govern the model, including the important aspect of the interfacial zone between the steel and concrete. The roles of the depth of concrete cracking, of fractures, and of poor construction joints are discussed, including the likely rates of very localized corrosion. Some comments about practical implications are made throughout the paper.

At this point it is noted that apart from empirical and physico-chemical modeling approaches, the literature, as reviewed by Raupach [15], also contains some models based on interpretations from electro-chemical testing. Although they have been advocated for many years (cf. [16]), these are not used herein. The reason is that the results obtained are known to be problematic when compared to physical observations of corrosion and pitting in actual seawater conditions (e.g., [17]) and this also has been noted repeatedly for reinforcement corrosion (e.g., [18,19,20,21,22,23]). This confirms the need for calibration and validation of electrochemical test results against empirical field data [24]. The approach herein is to work directly with the available empirical data for calibration of the physico-chemical model.

2. Background

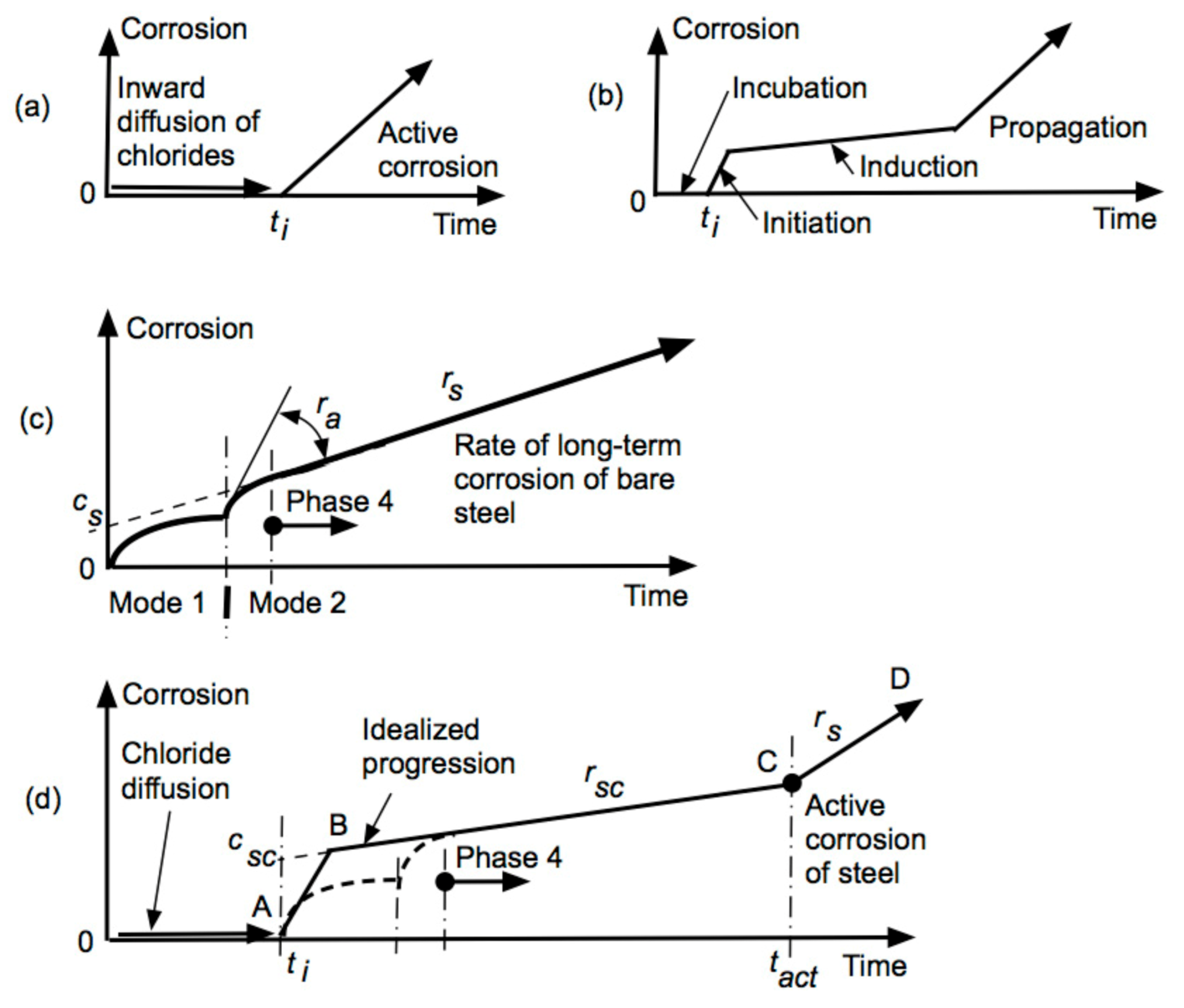
The classical, entirely empirical model for the initiation and progression of reinforcement corrosion is attributed to Tuutti [25] although there was a similar antecedent [26]. The Tuutti model provides a period during which chlorides permeate through the concrete cover to eventually reach the reinforcement. Then, it is assumed that when a sufficiently high concentration of chlorides is reached at reinforcement, corrosion initiates, followed by a steady increase in corrosion with time (Figure 1a).

To allow for cracking of the concrete as might be caused by tensile flexural stresses in the concrete François et al. [27] proposed, on the basis of their own test results, the phenomenological model shown in Figure 1b (cf. [28]). However, a practical assessment of test conditions indicates that the constant load applied for the beams is considerably more than the beams of the proportions used, which would sustain in normal service. While the stated maximum moment that was applied to the beams is realistic for the nominal working load capacity used in design, typically the “sustained” loading, that is the loading applicable for most of the operational life of a beam, is some 10–20% of the design load [29]. It follows that the crack sizes in the experiments are some 5–10 times greater than those that would be expected under normal service conditions. In fact, most beams in practice show no signs of flexural cracking. In practice, if severe cracking does occur, it almost invariably is the result of overloading or poor design. It follows that the model is unduly conservative for realistic structures (Figure 1b).

In the model of Figure 1b, t_i is the time at which initiation occurs. Due to the large crack sizes in the experimental work, the initiation of corrosion will occur relatively early in the life of the structure, presumably as a result of chlorides (and likely oxygen) being able to reach the reinforcement relatively quickly. The model assumes that shortly after t_i the rate of corrosion drops to a very low value, attributed to the rate-controlling reaction stated as then being cathodic oxygen reduction. The reason for the large reduction in the corrosion rate is considered to be a build-up of corrosion products. Eventually, at t_prop, the model enters the “propagation” phase that has a damaging rate of reinforcement corrosion.

One difficulty with both the Tuutti and the François et al. models is in the role they assign to chlorides. As noted already by Foley [30] and as evident in results from carefully controlled experiments by Heyn and Bauer [31] and Mercer and Lumbard [32] in zero velocity conditions (such as inside concretes), the chloride concentration has very little effect on the rate of corrosion (although it can affect the propensity for pitting). Potentially, this is the reason the much-studied critical chloride concentration, at which t_i is assumed to occur, has proved so elusive, with very wide variability in the experimental results (e.g., [33]). It also may explain why some actual reinforced concretes have very high chloride concentrations inside the concrete but little or no evidence of reinforcement corrosion (cf. [4]). Despite these observations, the concept of chloride as the critical factor for initiating reinforcement corrosion in marine environments appears still firmly entrenched [34,35,36], although the modern terminology has become “chloride-induced” corrosion. However, the precise meaning of this term remains uncertain.

A second difficulty with these models is the assumption that the oxidization of the steel in the presence of water is always through the cathodic oxygen reduction reaction (ORR): O₂ + 2H₂O + 4e⁻ → 4OH⁻. This has also been assumed as the case for extended exposure periods, such as over decades (e.g., [37]), even though the reinforcement has already corroded significantly and there has been a considerable build-up of rusts. According to the bi-modal model for the corrosion of steel [38], a considerable build-up of rusts should produce predominantly anaerobic corrosion conditions after only a few years of exposure. For reinforcement corrosion in concrete, direct evidence of anaerobic corrosion is available for concrete structures exposed in marine conditions since WW2 (see Figure 1c in [39]).

A model that accounts for these factors and which is consistent with long-term corrosion behavior for steel in marine environments, was proposed by Melchers and Li [14]. Rather than assuming the corrosion of steel is a linear function of time as in the models of Tuutti and François et al., it was built on the more accurate bi-modal model for the corrosion of steel (Figure 1c). That model has been verified for a wide range of environments including soils and also a variety of steels and other alloys [40]. Hence, it can be expected to be valid also in concrete. The model for reinforcement corrosion is shown in Figure 1d. As in the Tuutti and François et al. models, it has a period of initiation (0–t_i) during which inward diffusion of chloride ions is likely to occur. Reinforcement corrosion commences at t_i (Figure 1d). However, as described further below, the conditions under which this occurs are more complex than the mere achievement of a “critical chloride concentration”. After initiation, corrosion progresses initially in Mode 1, governed, as explained further below, by the availability of oxygen (and water) in air-voids in the concrete at the concrete-steel interface. As the oxygen is depleted and corrosion products build-up, the corrosion of the reinforcement transitions into Mode 2 with a corresponding relatively fast increase in reinforcement corrosion loss (Figure 1c). The overall effect is shown as (A-B) in Figure 1d. Thereafter, the reinforcement corrosion is the rate controlled predominantly by the cathodic hydrogen evolution reaction. This relatively slow reaction accounts for the plateau-effect (B-C) in Figure 1d. Eventually, at t_act, new conditions arise that permit a relatively fast and damaging corrosion (C-D)—these also are considered further below.

In wet oxygenated environments, the corrosion of steels in Mode 1 is predominantly under aerobic conditions (Figure 1c). The corresponding cathodic oxygen reduction reaction (ORR) is rate-controlled by oxygen diffusion from the external environment. As rusts build-up, the environment at the steel-rust interface changes predominantly under anaerobic conditions, for which corrosion occurs essentially by pitting under very low pH values [41]. The usual anodic reaction Fe → Fe²⁺ + 2e⁻ still applies but the process is now rate-controlled by the cathodic hydrogen evolution reaction (HER): 2H₂O + 2e⁻ → H₂ ↑ + 2OH⁻. The dissolution of water provides the hydroxide ions necessary to form rusts.

While oxygen is not directly involved in the HER, oxygen is not entirely excluded from the overall longer-term corrosion behavior. For atmospheric and for immersion corrosion, that is without the presence of concrete, oxidation may occur at the external rust layers [42], releasing ferrous ions and thus diminishing the overall rust layer [43]. The net effect of this is that the long-term corrosion rate r_s depends both on the rate of loss of external rust by oxidation and on the build-up of rusts by anaerobic processes at the metal-rust interface. In effect, oxygen is still the ultimate electron acceptor but the process is more convoluted. It is clear that the concentration or availability of oxygen at the external rust surface can exert some influence over the rate of long-term corrosion r_s and also that both oxygen availability and r_s can be affected by encasing the bar in concrete.

Before proceeding, it is noted that field data [44] show that, closely enough for practical purposes, the longer-term part of the process, denoted as phase 4 in Mode 2 (Figure 1c), can be considered a linear function in time. It may be represented in a simplified manner by parameters c_s and r_s. It is certainly not the usual “corrosion rate”—this is a linear function passing through the origin and driven at the metal-rust interface region by the oxygen reduction reaction and the availability of oxygen.

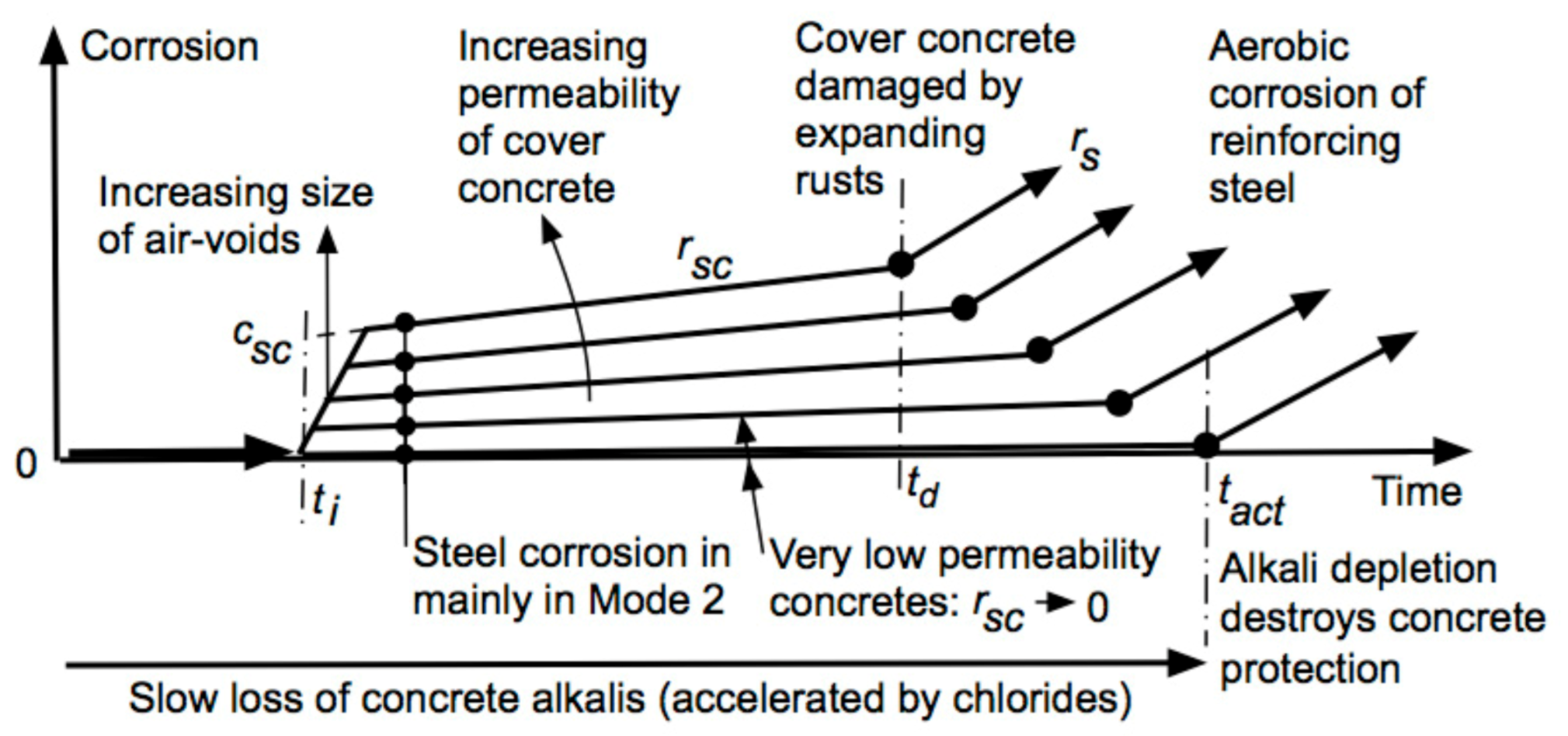
It is reasonable to assume that for steel bars inside concrete, the progression of the corrosion process will follow a pattern of behavior similar to that for the corrosion of steels in other environments. It is likely that for the steel encased in concrete relatively impermeable to oxygen Mode 1 will be rather short in duration. In this sense, encasement in concrete would have an effect essentially similar to a lower oxygen concentration in the external environment [45]. Therefore, encasement would also tend to depress the rate of oxidation of the external rust layers in phase 4 and reduce the net value of r_s (Figure 1c). Let this reduced value, due to concrete encasement, be denoted r_sc noting that it is likely to also depend on factors such as concrete over thickness, concrete compaction, and the permeability of that concrete potentially as affected by the wetness of the concrete. When the cover concrete is very dense and of very low permeability oxygen diffusion to the external rust layers will be much inhibited and, in the limit, r_sc → 0. This is consistent with observations of essentially no corrosion in very dense, low permeability concretes even after more than 80 years of exposure [5]. However, such a scenario is unlikely to continue ad-infinitum. Other mechanisms are likely to intervene, shown in Figure 1d as commencing at t_act. Originally proposed purely empirically [14], this has recently been shown to be caused by the gradual, long-term loss of concrete alkali such that at t_act the concrete will have lost so much material that it has greatly increased pore spaces and much greater pore connectivity. This permits a high level of local oxygen diffusion and thus much increased corrosion by direct oxidation [13].

On the other hand, for concretes with high permeability (and cracking) access of oxygen from the external environment and thus the exterior oxidation of the rust layers is likely to be somewhat easier. The result will be that rusts are permeated into the concrete pre spaces immediately surrounding corroded steel bars. However, the effect of greater oxygen permeation through the concrete cover on the rate of corrosion r_s is likely to be slight, since r_s depends mainly on the rate of the cathodic HER at the metal-rust interface. The situation changes dramatically, however, if there is significant damage, such as from widespread concrete cover cracking and spalling.

The corrosion behavior denoted schematically by (A-B) in Figure 1d arises from two aspects. One, as noted above, is the transition from Mode 1 to Mode 2 for the corrosion of steel (Figure 1c). The other, as will be seen, and the more important effect is that from air-voids and similar imperfections in the concrete matrix at the concrete-steel interface. The overall corrosion loss is shown idealized by parameter c_sc and occurs in the relative short-time period immediately after initiation at t_i.

Corrosion initiation for general (or uniform) corrosion at the usual potentials for iron in water is possible only for a local pH below about 9, dictated by thermodynamic conditions (Gibbs free energy or Pourbaix). This is irrespective of chloride concentration. It tends to rule out the initiation of general corrosion inside concretes and concrete pore waters with their usually high pH. The situation for pitting corrosion is rather different. Pitting corrosion involves a higher (more active) potential, and is thermodynamically possible, even at elevated pH environments when the chloride concentration is sufficiently high [46]. This possibility directly permits the initiation of reinforcement corrosion at chloride-rich wet air-voids in the concrete adjacent to the reinforcement steel.

The severity of corrosion associated with an air-void depends on the amount of oxygen in the air-void and the local availability of pore water. It has been shown to commence as differential aeration, localized at the edges of the air-voids [1] that then causes localized (pitting) corrosion of the adjacent steel [12]. Once initiated, such localized corrosion is only very mildly inhibited by diffusion considerations and will increase rapidly until eventually limited by the availability of oxygen or water. The net result is an almost step-wise increase in corrosion loss just after t_i, idealized as (A-B) and c_sc in Figure 1d. This type of behavior also has been observed for near-full-scale beams, for example, by Yu et al. [28] who attributed it purely to corrosion products inhibiting oxygen diffusion.

The size and distribution of the air-voids in the concrete matrix at the steel surface reflect the degree of concrete compaction achieved prior to concrete setting. Moreover, they are likely to be functions of the composition of the concrete and properties such as water-cement ratio and aggregate-cement ratios. All these tend to have a degree of statistical uncertainty and this is likely reflected in the amount of corrosion at the air-voids, i.e., in c_sc in Figure 1d.

Collecting together the various factors noted above provides the overall schematic model shown in Figure 2. It can be seen that after t_i the amount of corrosion is governed not just by the rate of progression of corrosion, that is by r_sc, but also by the volume of the air-voids, as these govern c_sc. Estimates for the values of the parameters (c_sc and r_sc) for a longer-term corrosion, based on physical tests, are given in the next section.

3. Reinforcement Corrosion after Initiation (Parameters c_sc and r_sc)

There are few longer-term experimental programs covering a range of concrete mixes for which both reinforcement corrosion initiation and progression were observed and which were sufficiently detailed to observe the bi-modal corrosion behavior of the steel. One of these is the program reported by Shalon and Raphael [47]. It used multiple model concrete specimens each 40 mm × 40 mm × 140 mm long made from local (limestone) aggregates and commercial cement without additives. Each specimen was provided with a longitudinal, centrally-placed 6 mm diam. mild steel bar. The mixing water consisted of local natural seawater. As a result, chlorides were present at a high concentration in the concrete matrix from the outset. Thus, the initiation period (0–t_i) can be considered negligible. This is a valid experimental technique to accelerate the overall process [48]. A range of aggregate-cement and water-cement ratios was used for the concrete specimens. They were cast horizontally in steel molds.

There is no information on concrete compaction other than the fact that the bars were “inserted” and “embedded” in the concrete of each specimen, apparently after the molds were filled with concrete [49]. All the specimens were stored in a laboratory fog-room at about RH 98% and average air temperature about 25 °C until required for examination. At 3, 6, 12, 24, and 48 months, one or two specimens from each concrete mix was broken up and the surface condition of the bars examined. Any rusts on the bars were removed using a protocol generally similar to that currently specified for reinforcement bar cleaning. The cleaned bars were then weighed and the masses compared with the original masses. The original paper only provides percentage mass losses. For the present analysis, these were converted to corrosion losses (in mm) using the reported nominal diameter and the typical density of steel (7800 kg/m³).

A parallel project using specimens of the same size and with comparable water-cement and aggregate-cement ratios with exposures extending over more than 12 years has been reported recently [12,13]. This program used low-heat as well as blended commercial cement. Some mixes were made with calcareous aggregates. Unlike the Shalon and Raphael [47] experiments, the parallel project found no or negligible corrosion losses even over the 12 years of exposure, which was insufficient to obtain accurate quantitative results. The major difference was that a high degree of compaction had been carried out. Only microscopic voids were visible in the concrete at the steel interface.

A completely different project has yielded information on reinforcement corrosion and its progression over some 28 years of exposure. Three-meter long reinforced concrete beams (36 in total) were exposed to artificial chloride-rich wet and dry cyclic laboratory conditions in ambient temperatures between about 5 and 20 °C [50]. After only a few months of exposure, some initial corrosion was reported but after the first few years (about 4–5) the corrosion rate declined significantly [28]. This was assumed to be due to rust products and calcite blocking oxygen access through the cracks. More severe general and pitting corrosion of the steel reinforcement bars was observed after 14, 23, 26, and 28 years of exposure, together with concrete cracking and damage [34]. However, even after 28 years of exposure, the corrosion of the reinforcement was considered very mild and highly erratic along the steel bars, with some longitudinal sections still showing no obvious corrosion, despite the low concrete cover in some beams (10 mm). In all cases, most of the corrosion occurred along the bottom of the bars (of the horizontally-cast beams).

The corrosion losses were reported as a loss of the cross-sectional area of the main reinforcement bars, sampled at numerous locations along the bars. The cross-section area loss for the two 12 mm diam. main reinforcement bars in each beam tested show considerable variation but mostly in a range of 20–25 mm². Converting this to corrosion loss produces an average (radial) corrosion loss of 0.32 mm after 28 years. The corrosion losses at the shorter exposure periods show a linear trend from negligible corrosion at 4.5 years [34] which, taken together, are equivalent to a long-term rate of reinforcement corrosion r_sc = 0.014 mm/y (Figure 14). Since only one concrete mix was used throughout, with a similar workmanship for all the specimen beams, there is no information on the potential effects of concrete mix design or concrete compaction.

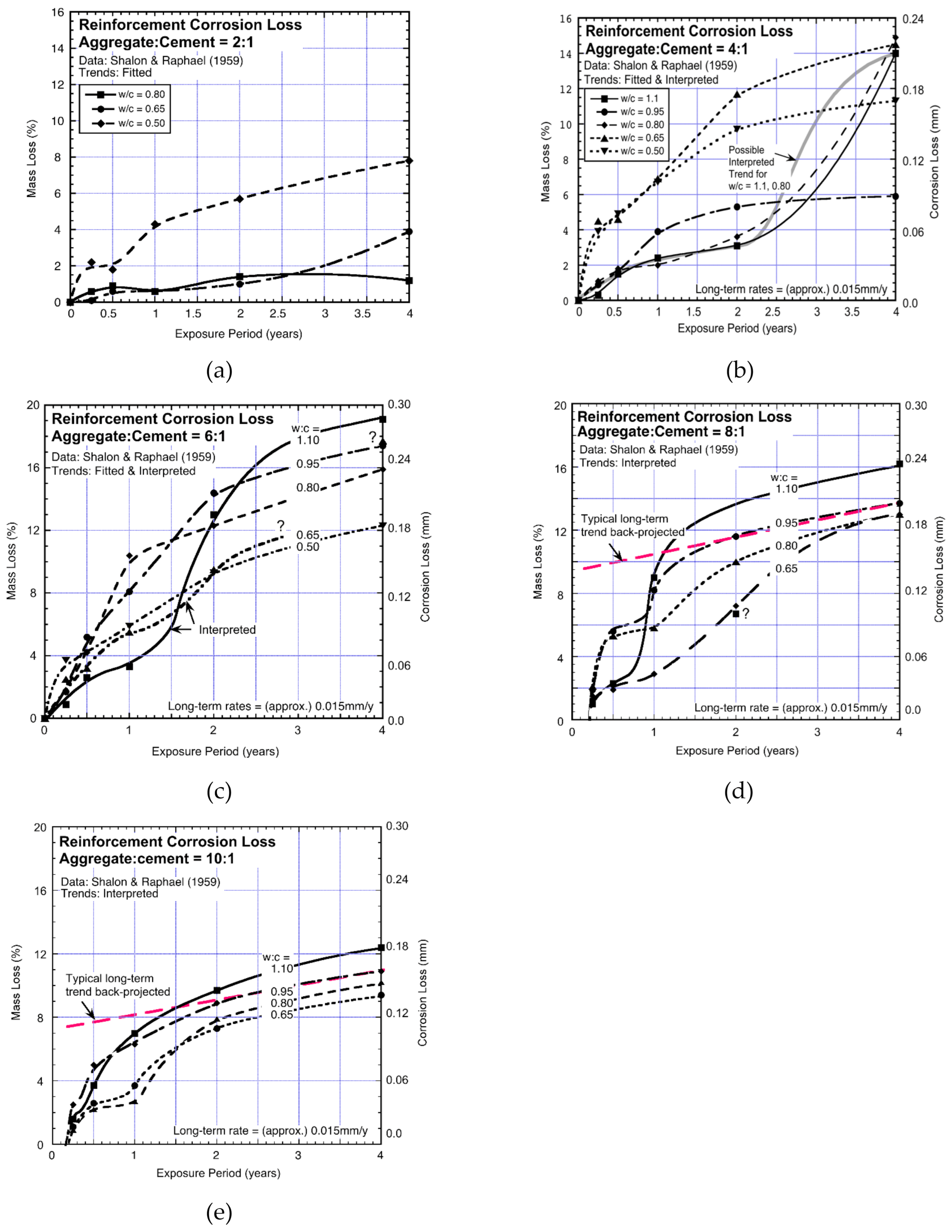
Returning now to the experimental results reported by Shalon and Raphael [47], Figure 3 summarizes their observations of corrosion losses as functions of water-cement (w/c) and aggregate-cement (a/c) ratios. For the combinations shown, trends have been added through the data points, in most cases fitted using the Stineman [51] non-linear “best-fit” function. In a few cases interpreted trends are shown. These are based on the data points but in between build on the expected overall consistency with the majority of the best-fit trends.

Remarkably, throughout all the plots, the bi-modal corrosion loss trend for the corrosion of the reinforcement steel is clearly evident (Figure 3). It occurs within the first 1–2 years of exposure. Remarkably also, the rate of longer-term corrosion r_sc is highly consistent, in all cases around 0.015 mm/y for the whole of the (wide) ranges of aggregate-cement and water-cement ratios. Due to the inverse relationship between the concrete strength and concrete permeability [52], this result can be interpreted immediately since showing r_sc is not strongly dependent on concrete permeability.

The majority of the trends in Figure 3 show that after the first 2–3 years the trends tend to be linear at a rate r_sc ≈ 0.015 mm/y. This appears almost independent of the precise proportions of the concrete mixes. In Figure 3a,b, some concrete mixes with high water-cement ratios (i.e., very wet mixes) show very little corrosion, at least for the first 3–4 years, followed by corrosion losses that are more consistent with the other data sets. Although the exposure periods are not sufficiently long to confirm, the data trends do suggest that for these cases, too, the pattern is the same as the others, albeit delayed in time.

For the cement-rich trends in Figure 3a, it is seen that corrosion losses are relatively low, and one case, at least, shows r_sc approaching zero. This is consistent with the expectations noted above for high impermeability concretes. It also is consistent with practical observations even after periods of marine exposure exceeding 80 years [5].

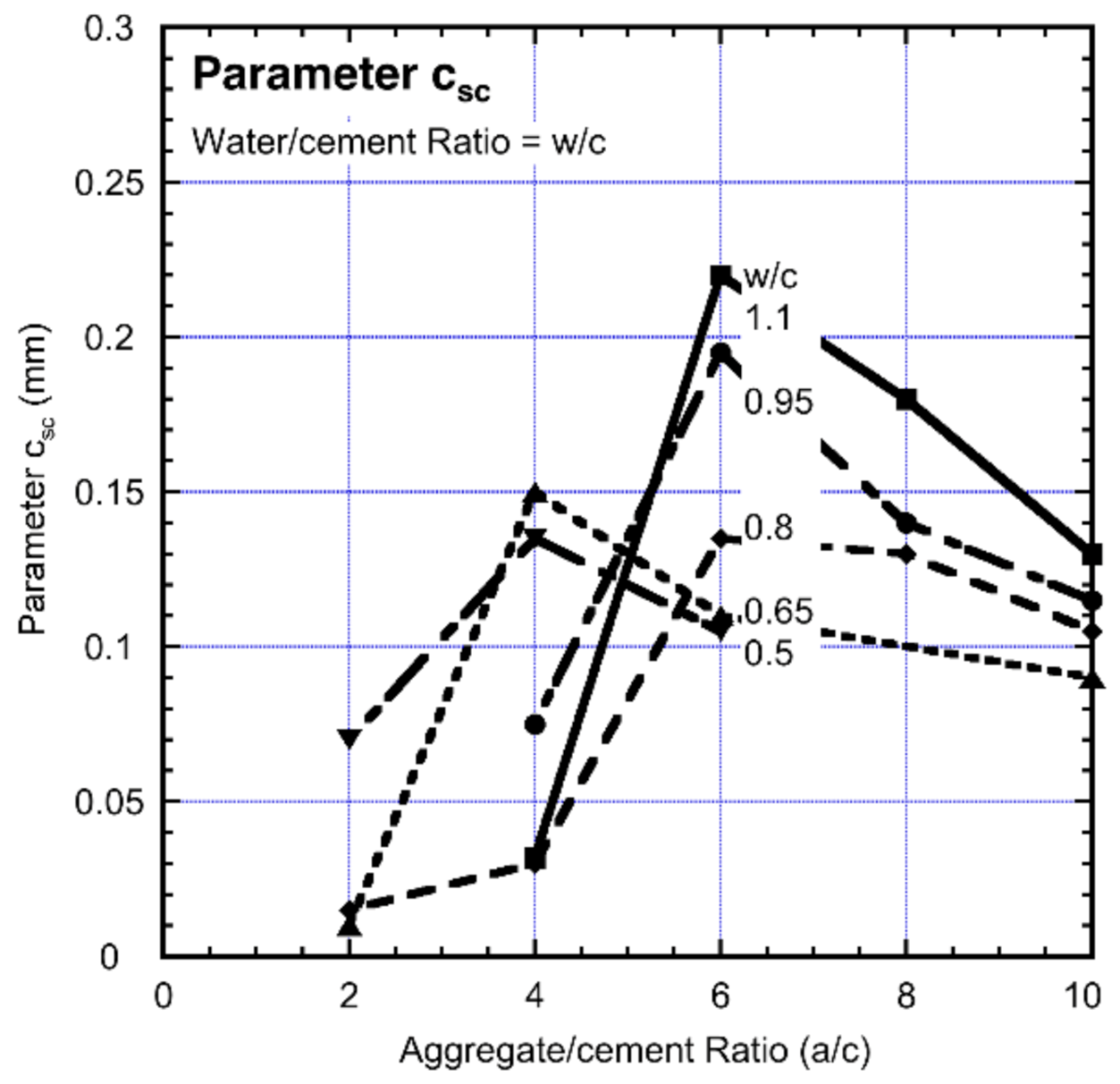
The plots in Figure 3 allow the parameter c_sc to be extracted. The results are summarized in Figure 4 as functions of the w/c and a/c ratios. Evidently, c_sc increases with increased water-cement (w/c) ratio and then declines for the further increase in w/c. This trending for c_sc is slightly later and also higher for concretes with high w/c ratios.

In interpreting the results in Figure 4, it is reasonable to assume that the lack of compaction of the concrete once the bars had been “placed” in them [47] would have left air-voids at the steel-concrete interface. These air-voids can be expected, after concrete setting, to be greater for the concretes with higher w/c ratios as a result of greater shrinkage with higher water content. This is likely the reason for greater values of c_sc with increased w/c ratio. As shown in Figure 4, initially the higher a/c ratio produced higher values of c_sc but this is not the case for a/c greater than about 4–6, for which c_s declines with a/c. As noted, this behavior is likely to be a result of the permeability of the concrete. Comparisons with other scenarios are of interest. For example, for sand particle-steel interfaces, permeability and voids are known to influence localized corrosion [53]. This holds also for spherical glass beads on metal surfaces [54] and for poorly compacted clays [55]. In each case, the observations can be attributed to the effect of voids on differential aeration at the void-space and also on their size (volume).

For the more permeable concretes, i.e., those with a/c ratios > 2, the overall long-term corrosion loss c(t) as a function of continuous exposure time t is given by:

c(t) = c_sc + 0.015 (t − t_i)   for t > t_i

(1)

where t is the actual elapsed time, t_i > 0 is the (estimated) time to initiation, and c_sc is obtained from Figure 4. For a/c ratios < 2 Equation (1) overestimates c(t). There is insufficient data to be quantitatively more definitive but it is clear from Figure 2 and the above discussion that for such cases c_sc → 0 and r_s → 0.

While there appears to be no data in the literature for the parameter c_sc (rightly, since this parameter has only recently been identified), some information is available from which to make estimates for r_s. Beaton et al. [56] reported that typical corrosion rates equivalent to about 0.012 mm/y for RC piles above the mudline, and up to 0.18 mm/y elsewhere, both for 37 years of exposure, are sufficiently long to be taken as estimating r_s. Stewart and Rosowsky [57] proposed long-term corrosion rates in the range 0.011–0.23 mm/y, based on current density measurements for superficially sound concretes [58,59]. Moreover, Andrade and Alonso [60] and Sagüés et al. [10] reported longer-term rates around 0.01 mm/y based on electrochemical measurements. These estimates bracket the rate derived from the experiments in Figure 3.

4. Commencement of Corrosion at t_act

The lower trend line shown in Figure 2 represents the practical observations that high quality, very low permeability concretes with no discernable air-voids show almost no corrosion. Figure 5 shows an example, for marine concretes recovered from bridge piles more than 80 years old [5]. As noted, for these there was no observable corrosion so that both c_sc and r_s → 0. Under these conditions, some other mechanism must come into play if reinforcement corrosion is to become possible. Recent experimental observations have shown that this involves the loss, through dissolution, of calcium hydroxide (Ca(OH)₂) in the concrete surrounding the reinforcing bars, leaving behind a concrete matrix with pH around 7–8 [13]. Usually the dissolution process is very slow but it is accelerated proportionally to the concentration of chlorides in the solution [61]. The experimental results showed that the dissolution process leaves behind a permeable concrete matrix and clear evidence that oxygen can readily permeate through it to oxidize the reinforcement [13]. Thus, not only the lowering of concrete pH at the reinforcement bars but also the greater permeability for oxygen leads to the severe rate of corrosion after t_act (Figure 2).

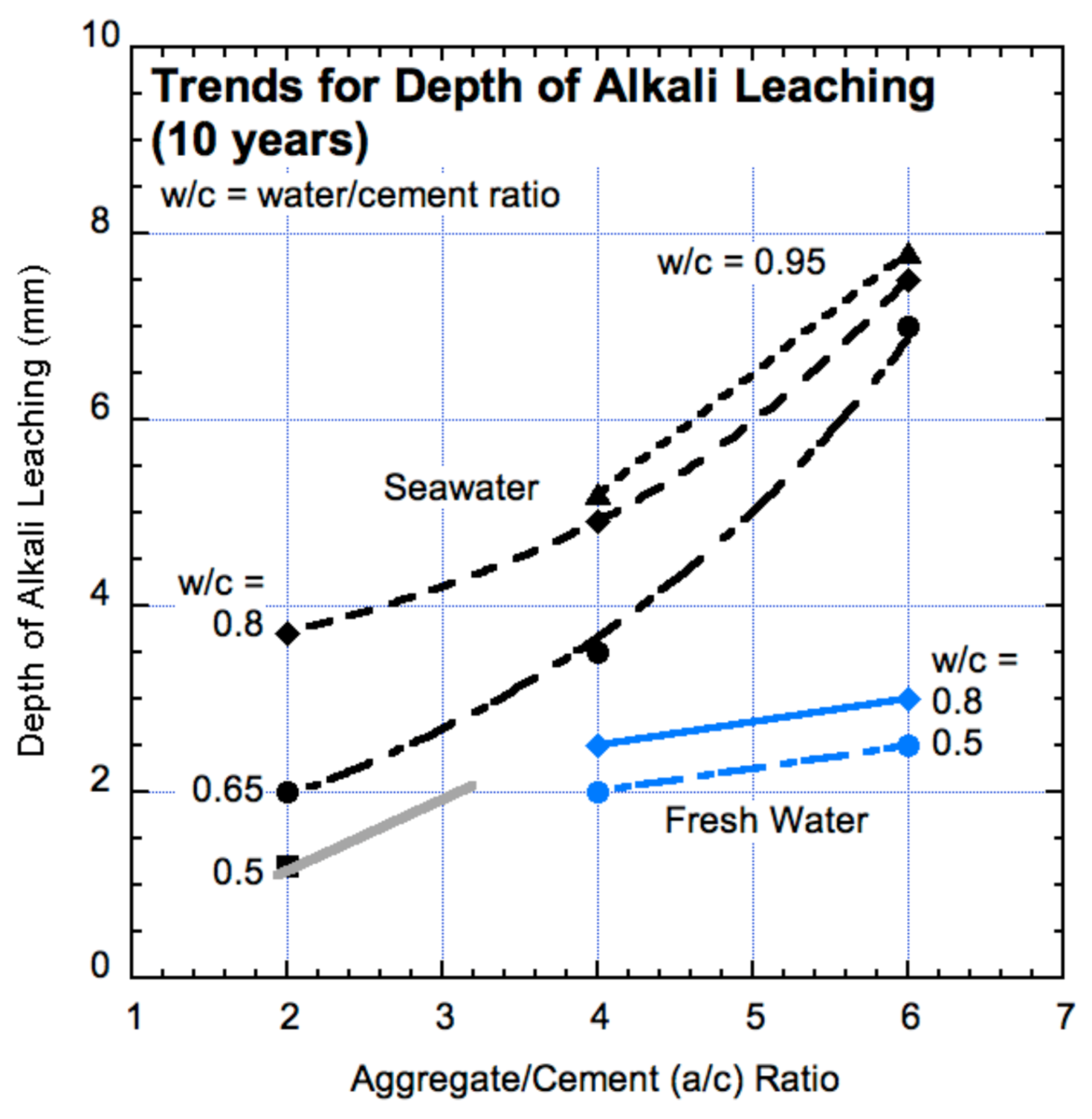
The rate of loss of alkalis for high quality concrete may be estimated, to a first approximation, by assuming a constant rate of loss of Ca(OH)₂ from first exposure onwards. Figure 6 shows a summary of the loss of Ca(OH)₂ over a period of 10 years for different water-cement and aggregate-cement ratios and for concretes made with seawater and with freshwater [13].

The trends in Figure 6 may be used to estimate the expected time before a complete loss of alkali material and thus, the local development of a concrete matrix permeable to oxygen. By way of example, for an (uncracked) concrete made with freshwater with (moderate) water cement ratio of 0.5 and an aggregate cement ratio of 4:1, Figure 6 indicates that the depth of alkali dissolution is about 2 mm in 10 years or 0.2 mm/y. For a concrete made with seawater the corresponding rate is about 0.3 mm/y. Thus, a concrete structure with a cover of 50 mm would commence with active corrosion caused by the loss of alkalis after t_act = 250 and 165 years, respectively. For a leaner concrete, say a/c = 5:1, the respective depths of loss of Ca(OH)₂ are greater and the rates are higher (about 0.23 and 0.45 mm/y), and the expected times shorter, 220 and 110 years, respectively. These comparative times demonstrate the significant effect of chlorides on the rate of alkali dissolution. They also demonstrate the importance of aggregate-cement ratio, which is the importance of cement content relative to the aggregate content.

In both examples the time estimates appear to be high, but they are not unrealistic when compared, for example, with observations for reinforced concrete piles exposed to Pacific Ocean immersion, tidal and splash conditions for over 80 years [5]. Full-sized (380 mm × 460 mm) cross-sectional samples of these showed concrete cross-section pH readings around 12, except for the outer 2–3 mm, despite very high chloride concentrations—around ten times the normally accepted threshold. Importantly, the cement content for these piles was high, with a/c ratios estimated around 4.5:1. Similar to the specimens in the Shalon and Raphael [47] experiments, these piles were all uncracked. The effect of cracks is considered in the next section.

5. Corrosion at Deep (Hairline) Cracks and Other Imperfections

The conventional wisdom, for example, as codified in standard specifications, is that cracks in the concrete of less than about 0.3 mm across are of negligible importance. Tracing the origin of this criterion shows that it is derived from short-term laboratory experiments [62]. However, other reports have discounted crack width as the important parameter in favor of the “existence” of a crack [50,63], while several practical reports have noted severe localized reinforcement corrosion for very narrow (i.e., hairline) cracks that extended to the reinforcement [10,64,65]. Similar observations have been made in other practical cases.

Figure 7 shows an example of very severe, so-called “tunneling” corrosion of a 6 mm diam. steel bar after 65 years of exposure in marine atmospheric conditions along the North Sea [66,67]. In this case, corrosion had penetrated along the bar axis for about 6–8 mm but had left a “sleeve” at the outer surface of the bar. Figure 8 shows the very considerable localized reinforcement corrosion of two of four 32 mm diam. steel bars together with watery-looking rust stains located at a cracked cross-section exposed in Pacific Ocean tidal conditions for about 85 years [5]. In both cases the cracks were “hairline” in width. In neither case were rust deposits or rust stains visible on the exterior surfaces of the concretes, including at or near the hairline cracks.

Two questions arise immediately from these cases: (a) What were the corrosion mechanism(s) and (b) where did the corroded steel go, and how? In both cases, a critical observation is that there was a (hairline) crack from the exterior concrete surface into the concrete and deeper than the location of the reinforcing bars. While initially the hairline crack could permit some access of atmospheric or dissolved oxygen to the reinforcement bar, any build-up of rusts would soon convert local conditions to predominantly anaerobic, and move the local steel corrosion process into Mode 2, governed by the cathodic HER with the generation of pits and acidic ferric chlorides (Figure 1c) [41]. Being water-soluble, the ferrous chlorides are able to leach easily from the corrosion site via the hairline cracking. Taken together, these aspects allow a mechanism to be postulated to explain the observations in Figure 7 and Figure 8.

At the commencement of Mode 2, corrosion will be, as noted, predominantly by pitting under anaerobic conditions at a corrosion rate r_a (Figure 1c). As corrosion develops it will be through successive pitting, each pit depth step restrained in depth, followed by sideways growth of the pits with amalgamation of adjacent pits, followed by further pitting [68]. This pattern leads to the sequential development of corrosion by pitting as shown in Figure 9. In the earlier stages, corrosion of the steel bar is from the concrete crack inwards with, through radial amalgamations of pits, the development of (for a circular bar) an annular ring of corrosion. Eventually, the center of the steel bar will be reached, leaving an annular grove around the bar. One-half of this forms the sharp-pointed bar geometry shown in Figure 8. Further corrosion is possible only for the remaining exposed metal of the annular ring, attacking each side independently. Corrosion will progress along the axis of the bar, more severely along the center as this is the predominant source of iron and also the location of impurities that arise from hot-rolling, which are known to increase the rate of corrosion slightly (Figure 9) [69,70]. The eventual effect is to produce the tunneling corrosion seen in Figure 7. The fact that the tunneling shown in Figure 7 occurred at about 65 years of exposure, some 20 years earlier than that shown in Figure 8, is largely due to the considerable difference in bar diameter—6 mm compared with 32 mm.

Much of the corrosion development shown in Figure 9 is governed by anaerobic, high chloride conditions, producing, as noted, highly soluble FeCl₂ that can move easily through the (hairline) crack to the external environment, leaving little or no trace of rust deposits such as red-brown rust spots (Figure 8). On reaching the external environment the FeCl₂ will be oxidized to FeOOH or to essentially similar insoluble rusts [71]. These may leave characteristic rust stains on the concrete or more likely are washed away, by rainwater or seawater, and thus leaving little or no trace.

Since there is no deposition of corrosion products within the crack to inhibit the rate of the corrosion reaction, r_a remains the governing corrosion rate. It can be considered to act perpendicular to all corroding surfaces, including at the deepest penetration (i.e., perpendicular to the longitudinal axis of the reinforcing bar) (Figure 9). It follows that a first estimate of the loss of bar radius, Δr, over a time interval (t − t₀) is given by:

Δr(t) = r_a (t − t₀)

(2)

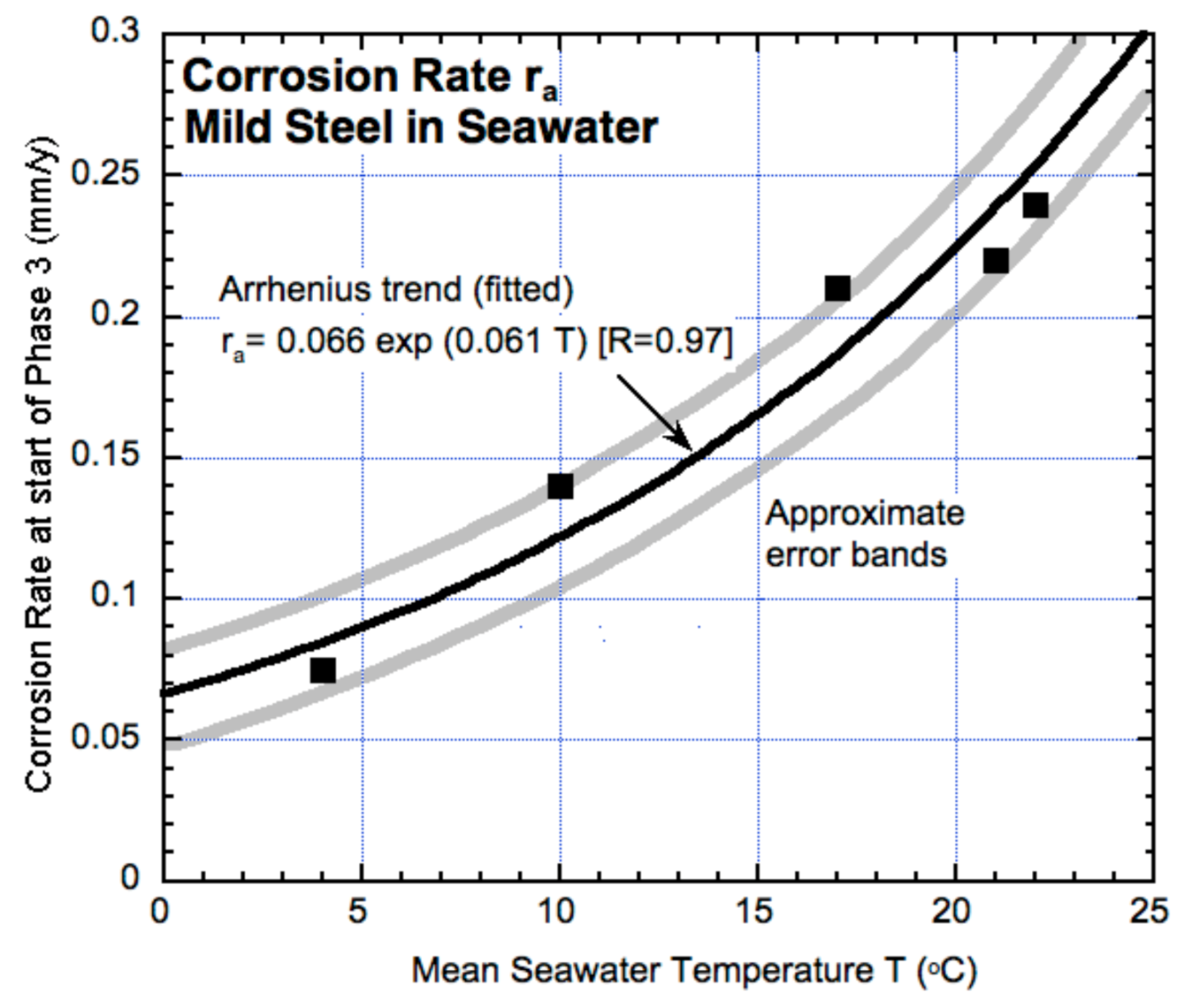
Here, t₀ represents the time period prior to the commencement of the above corrosion process. In most cases, this will be approximately t₀ = 0. The rate r_a may be extracted from earlier work that considered the commencement of anaerobic corrosion for steel in seawater [38]. Figure 10 shows the results of field observations at different average seawater temperatures for exposure sites in different parts of the world.

Assuming no obstructions or rust products develop to inhibit the free movement of FeCl₂, Equation (2) can be expected to also apply to the rate of tunneling corrosion once the centerline of the reinforcing bar is reached and the only available steel for corrosion is the steel bar cross-section. In essence, this means that Relationship (2) also applies for corrosion that goes “around corners”.

Predictions from Equation (2) may be compared with observations, such as for reinforced concrete handrail elements along the North Sea at Arbroath, Scotland [66,67]. According to Figure 10, r_a = 0.11–0.14 mm/y for an annual average temperature of 10 °C. For 60 years of exposure and with r_a = 0.11 mm/y it would take 27 years to fully penetrate a 3 mm radius bar, leaving 33 years to produce a tunneling depth of 3.6 mm. If the rate r_a = 0.14 mm/y, these figures become 21 years and 5.4 mm of tunneling depth. The latter is consistent with physical observations (Figure 7).

For the Hornibrook bridge case (Figure 8), the average water temperature is about 22 °C, so that r_a is in the range of 0.22–0.27 mm/y, with, over 85 years, an estimated penetration of about 19–23 mm. This estimate is somewhat greater than the physical radius of the bars (16 mm) but is not inconsistent with the observations. Better consistency can be derived if t₀ is in the range of 15–25 years—not unreasonable for a concrete that had maintained pH around 12 after 85 years of exposure [5].

Corrosion in hairline flexural cracks in beams is likely to follow a pattern similar to that outlined above. Unfortunately, the flexural cracks observed in the full-scale laboratory tests of François et al. [27] and Zhu et al. [34] are much wider than the hairline cracks and thus, more exposed to the environment. As noted, such cracks are not typical of those in actual structures under normal service (“sustained”) load conditions.

6. Discussion

The analyses presented above permits the development of practical models for initiation and for progression of corrosion of steel reinforcement in concretes—models based on practical observations and on interpretations from fundamental theory. Most empirical models in the literature do not reference back, even partially, to fundamentals. Predominantly, they rely on the assumption that chlorides are the main driver for reinforcement corrosion. This is despite many practical cases having provided empirical contrary evidence. Recent detailed experimental observations, coupled with observations discounting the role of chlorides for general corrosion in quiescent conditions have demonstrated the important role of wet air-voids in the concrete matrix at the steel surface as reservoirs for oxygen and water and that only when these are present do chlorides advance the potential for corrosion, and specifically for pitting corrosion [39]. The experimental work showed that it is pitting corrosion that ultimately drives the initiation process, even in high pH environments. Remarkably, the role of chlorides in relation to the pitting corrosion potential has been known for many years [46]. However, it has almost always been ignored in discussions of the initiation of corrosion in concretes with their normally high alkalinity. Moreover, while some studies have reported the presence of air-voids, very few have mentioned any related pitting and its possible influence on initiation [33]. Failure to account for these effects may explain (a) the wide variation in the “critical chloride concentration” derived from various studies and (b) the apparent conundrum in establishing a relationship between the concentration of chlorides and corrosion initiation in practical structures. Without considering air-voids to supply oxygen (and water) there can be no corrosion, irrespective of the concentration of chlorides at the reinforcement steel.

The size of the air-voids where the concrete interfaces with the steel is important. As follows directly from theoretical considerations, and as shown in practical cases, it governs the amount of corrosion that can occur after initiation and is reflected in the parameter c_sc (Figure 2). Void-size is related to permeability but more importantly to the degree of concrete compaction. Where compaction of the concrete around the bars is poor, larger air-voids are likely both throughout the concrete matrix and against the formwork and at reinforcement bars. For the latter, air-voids are often observed on the underside of horizontal reinforcement bars, irrespective of whether they are “top” bars or bars in the lower parts of a beam or slab [72]. This has been observed also for laboratory specimens [12,73,74]. These observations provide one explanation to why spalling is mostly from the underside of beams rather than from the sides, even for a similar concrete cover and when exposure to seawater or seawater spray is similar.

For well-made, well-compacted, impermeable concretes air-voids tend to be very small or negligible (Figure 5). Practical experience shows that the initiation of reinforcement corrosion, if it occurs at all, is then not a significant issue. Experiments that observe reinforcement corrosion over extended time periods show that for such concretes reinforcement corrosion effectively ceases after a relatively short time (cf. AB in Figure 2). There is little corrosion product and negligible or no structural damage. As the size of air-voids increases, corrosion at initiation tends to increase also (Figure 2). Moreover, in this scenario concrete permeability (for oxygen) is likely to be greater. This will result in a somewhat greater longer-term rate r_s and an earlier time t_act to serious damaging corrosion (Figure 2).

Shalon and Raphael [47] interpreted their data along conventional lines at the time. Their observations led others to make a conclusion that concretes should not be made with seawaters due to their high chloride content (even though the use of seawater had been a standard practice in many locations for many years). The analysis given herein shows that the Shalon and Raphael data can be interpreted in an entirely different way when using the recently exposed importance of air-voids in the concrete, as well as when the bi-modal model for the progression of steel corrosion are taken into account. The analysis given above shows that air-voids, with chlorides present to allow pitting corrosion to be thermodynamically feasible, drive the amount of corrosion soon after initiation. Reinforcement corrosion then follows the bi-modal trending and, since the rate of oxygen availability is inhibited by the concrete cover, reinforcement corrosion soon reaches linear trending, idealized as BC in Figure 2. It corresponds to the predominantly anaerobic (Mode 2) part of the bi-modal model.

The data reported by Shalon and Raphael [47] clearly show the effect of concrete mix design, with concretes having higher a/c ratios (i.e., lean concretes) and having linear trending for much longer than for the other concrete mixes (Figure 3c,d). However, the effect on r_s is small, while the effect on c_sc is consistent with the parameter (aggregate-cement ratio) having the most effect on permeability (Figure 4). The overall consistency seen in Figure 3 and Figure 4 adds a degree of confidence to the proposed model.

The concrete cover thickness was not considered by Shalon and Raphael [47] but from experience it is known to be an important parameter, even in non-chloride environments [52]. Depending on the exposure environment, it normally is assigned the role of inhibiting diffusion of oxygen, chloride, and carbon dioxide to the reinforcement. However, in terms of the present exposition, the concrete envelope around reinforcement also is important. It retards the loss of ferrous ions from the external oxidation of the rusts [43] and, in the earlier stages, it tends to keep the rusts in place, protecting them from oxidation, abrasion, erosion or velocity effects as typical in some other environments. Again, this interpretation provides a completely different perspective to the conventional view that the rate of supply of oxygen governs the rate of oxidation at the concrete-steel interface. Such behavior would not result in the long-term linear trends seen in the experimental data.

The oxidation of the external rust layers likely accounts also for the practical observations of concrete cover spalling and high degrees of reinforcement corrosion when the cover thickness is small. Usually, such spalling is attributed to an overall expansion of the rust envelope around a reinforcement bar. This may be exacerbated by excessive (e.g., atmospheric) temperature variations damaging the concrete material. However, the concept of oxidation of the external rust layers is likely to be just as damaging, but has the advantage of being consistent with theory.

One question about the data from the Shalon and Raphael [47] experiments is whether their use of calcareous aggregates had an effect on the results obtained. This should be seen in the context of the pH for concrete in many actual structures being still around 12 after many years of exposure [13]. Where serious alkali leaching has begun to occur the presence of calcareous material may delay the drop in pH of the concrete, keeping it at around 9 by virtue of the calcareous material. This was noted, for example, for 65 year-old concretes exposed to marine atmospheres [66]. In addition, a survey of many reinforced concrete structures and the likely aggregates used for their concretes indicated that those made with calcareous aggregates tended to have longer effective lives [75]. For these, the time to initiation was much more difficult to estimate ex post. However, the practical experience suggested that the use of calcareous aggregates is not a critical issue for initiation or for r_sc. Instead, it appears to have the effect of maintaining somewhat longer the alkalinity necessary to maintain a concrete pH above 9. It is an area that has had little investigation and could benefit from further research.

A second question about the data from the Shalon and Raphael [47] experiments is whether the permeability of the concrete, and the air-voids at the steel-concrete interface, is reflected properly by their aggregate-cement and water-cement ratios. Both are often associated with permeability (e.g., [52]) but whether these parameters provide realistic representations of permeability for actual concretes is an open question.

As noted, in the Shalon and Raphael experiments the steel bars were placed into the concretes. There is no information on compaction. Air-voids were not mentioned in the published paper. It is reasonable to assume that if they had been observed they were unlikely to have been considered important and therefore were not measured. More broadly, it appears that since the availability of vibrators from the 1940s onwards it has been assumed that the vibrators produce adequate concrete compaction. It is unclear whether this was ever assessed in terms of air-voids around, and in particular under, reinforcement bars. It is also unclear whether mechanical vibration has been assessed relative to the earlier techniques of hand-rodding and hand-tamping. In view of the above discussion about the importance of air-voids, a further investigation appears warranted, preferably using realistic concretes and realistic compaction techniques.

Overall, more regard should be paid to the actual experience of actual structures, particularly those with highly repetitive, but individually made elements since these could be considered examples of very large experiments. They certainly are realistic, much more so than any laboratory concretes and even more so than electrochemical tests. There are also related experiences, not for reinforced concrete but for systems with a steel-particle contact, and without high pH conditions, and in some cases with seawater present. Despite these apparently adverse conditions, the evidence is compelling. It has long been recognized that bare steel piles driven into sands and muds in seawater conditions show essentially no corrosion even after many decades of exposure except at the sand/mud-seawater interface zone [76]. Similarly, ferrous iron pipes buried in extremely well compacted clay soils with acidic soil pH around 5–7 show almost no corrosion, again over many decades, simply since oxygen is excluded, particularly from the external rust surfaces [77]. The lessons from these observations are obvious and it is clear that the pre-occupation with chloride-induced corrosion for already high pH concretes does not sit well with these observations. It also does not sit well with the practice over many years of permitting concretes to be made with seawater [8], even though this practice was banned in many countries in the 1960s. The ban has been attributed by some authors (e.g., [52]) in part to the very paper used herein—namely Shalon and Raphael [47]. The present paper, and the earlier work [4,44], indicate that the problems with many reinforced concrete structures are not so much with chlorides but with the conditions (poor concrete permeability, permeable perhaps thin concrete cover, deep and possibly hairline cracking, and damage to the concrete matrix and cover from material issues such as alkali-aggregate reactions [78]) that permit such corrosion to progress after initiation. For atmospheric exposures, there is also the issue of thin concrete covers deteriorating under high temperature fluctuations. These are all potentially important matters of detail that may affect the progression of reinforcement corrosion.

Finally, the present developments show that provided the above matters of detail are properly considered, reinforced concrete structures can have extended service lives, even in high chloride environments. This is provided the concretes are well-made and have no or negligible air-voids in the concrete at the steel-concrete interface. The model proposed herein allows for some degree of corrosion after initiation, as caused by the volume of air-voids and as subsequently increased at a rate of about 0.015 mm/y, influenced only a small amount by the permeability of the exterior (cover) concrete. In this model it is not necessary to consider the imposition of concrete flexural cracking since in practice most concrete structures show little or negligible degrees of concrete flexural or other cracking. Therefore, it is inappropriate to use data for the corrosion of reinforcement in concrete structures when these have induced crack sizes much larger than occur under normal sustained loadings. The present results and proposed model show that it is possible to design and make reinforced concretes that avoid reinforcement corrosion or reduce it to negligible levels, as has been shown by experience to be feasible in practical concrete structures. As noted, this requires low permeability and very well compacted concretes to ensure there are minimal air-voids at the reinforcement, in particular under horizontal bars. Such concretes will also delay the loss of concrete alkalinity, a rate that can also be reduced through an adequate cement content so as to add the acid-buffering capacity and thereby delay the long-term development of alkali-leached concrete permeability, which will permit a much greater rate of oxidation commencing at t_act. The fundamentals for achieving good quality durable concretes have been well-known in the industry for many decades, but the experiences have not been placed in quite the context outlined herein. The present analyses provide the theoretical support for such experiences. It also makes clear where attention must be focused to achieve long-term durable reinforced concrete structures.

7. Conclusions

The following conclusions may be drawn from the material presented herein.

1. The development of reinforcement corrosion after initiation can be represented by a relatively fast increase to c_sc followed by a slow rate of corrosion defined by r_sc, where c_sc depends on the aggregate-cement ratio and water-cement ratio of the concrete and r_sc is about 0.015 mm/y of the general corrosion for most concrete mixes. Concretes with extremely low permeability are associated with very low values of r_sc.

2. For aggregate-cement ratios less than about 4, the parameter c_sc increases with the aggregate-cement ratio but decreases for higher aggregate/cement ratios, in both cases more so for higher water-cement ratios. This is attributed to the effect of the water-cement ratio on concrete permeability.

3. The slow loss of the low solubility concrete alkali calcium hydroxide leads, for extended exposures, to a much reduced concrete pH and to a porous concrete matrix that permits the entry of atmospheric or dissolved oxygen, which then causes oxidation of the revealed reinforcement bars. Since the solubility of calcium hydroxide is accelerated in the presence of seawater, corrosion will likely occur earlier for otherwise similar conditions.

4. In some conditions, severe corrosion may occur at deep hairline cracks intersecting the reinforcement and in the presence of chlorides, without necessarily leaving “tell-tale” rust stains. The process involves water-soluble ferrous chloride formed in corrosion pits leaching to the external environment, eventually leaving a characteristic tunneling type of corroded bar. Empirical data show that for seawater with an average water temperature in the range of 10–20 °C, the corresponding rate of corrosion r_a is in the range of 0.22–0.27 mm/y.