Modeling Salt Behavior with ECOS/RUNSALT: Terminology, Methodology, Limitations, and Solutions
Abstract
1. Introduction
2. Models and Theory
3. Terminology for Mixed Salt Systems and Methodology for Using RUNSALT
- A.
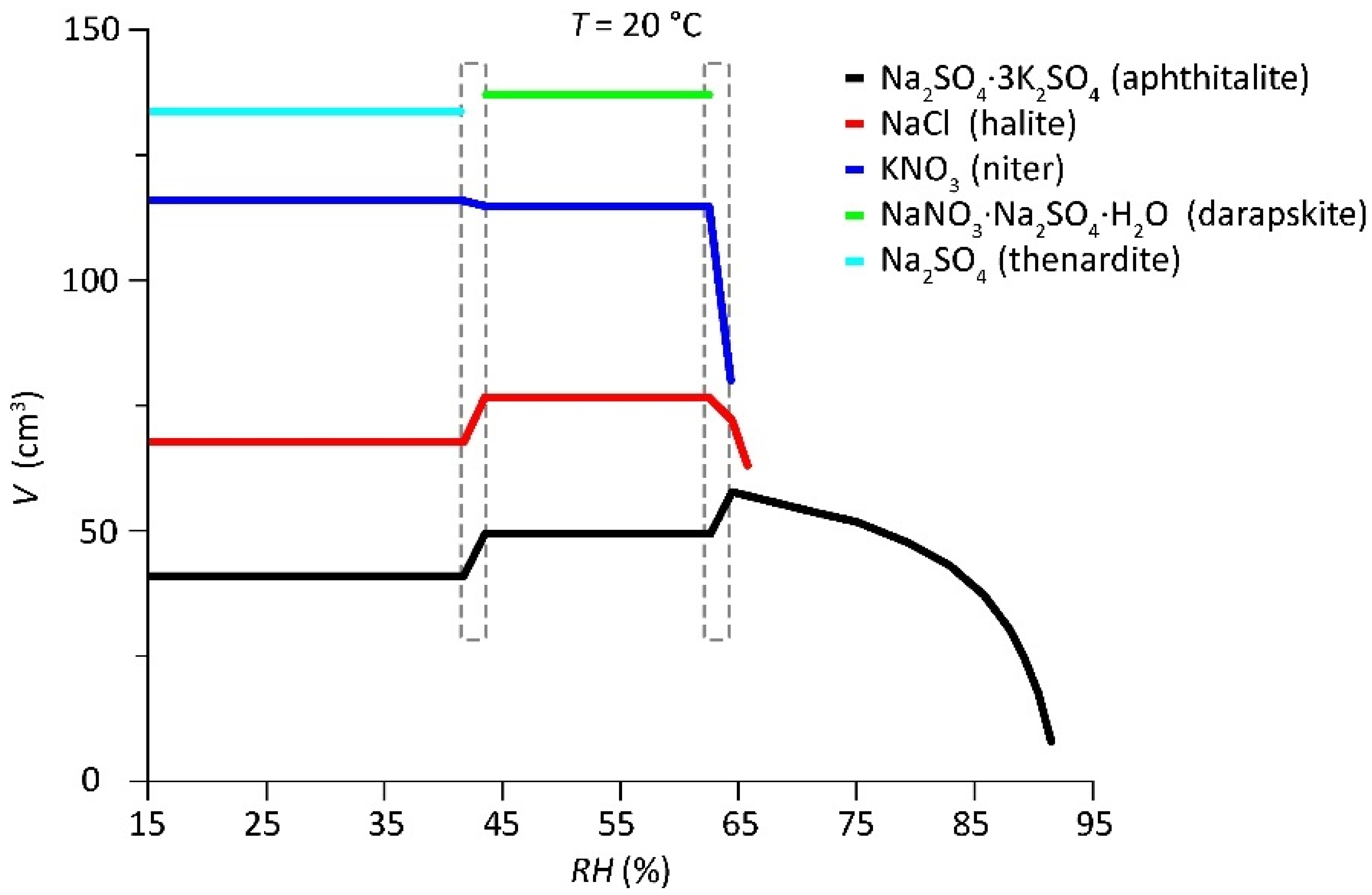
- The first mutual crystallization relative humidity of the mixture () represents the RH at which crystallization initiates for the first solid that appears under drying conditions (aphthitalite here, and thus ). The solution is saturated with respect to aphthitalite, and above this RH all solids are dissolved.
- B.
- The mutual crystallization relative humidity of all the following solids that crystallize from the solution in the mixture, and thus is the RH at which crystallization first begins for .
- C.
- The mutual dissolution relative humidity of all solids in the mixture when solution becomes available is equal to the RH points when a crystal starts to dissolve for . B and C are often at the same RH; here, the resolution of the plot distorts the position for halite and niter, a phenomenon explained further on.
- D.
- The mutual deliquescence relative humidity of the mixture is the RH determined by the solids in the mixture at which the first dissolution starts to occur and solution becomes available; here, also equals the dissolution relative humidity of .
- E.
- The mutual transition relative humidity . Here, under drying conditions, thenardite is formed and the amount of niter increases from the decomposition of darapskite and aphthitalite in a solid-state reaction.
- F.
- Plot stacking artifact caused by transition reactions, herein identified by chloride that is not available in other solids.
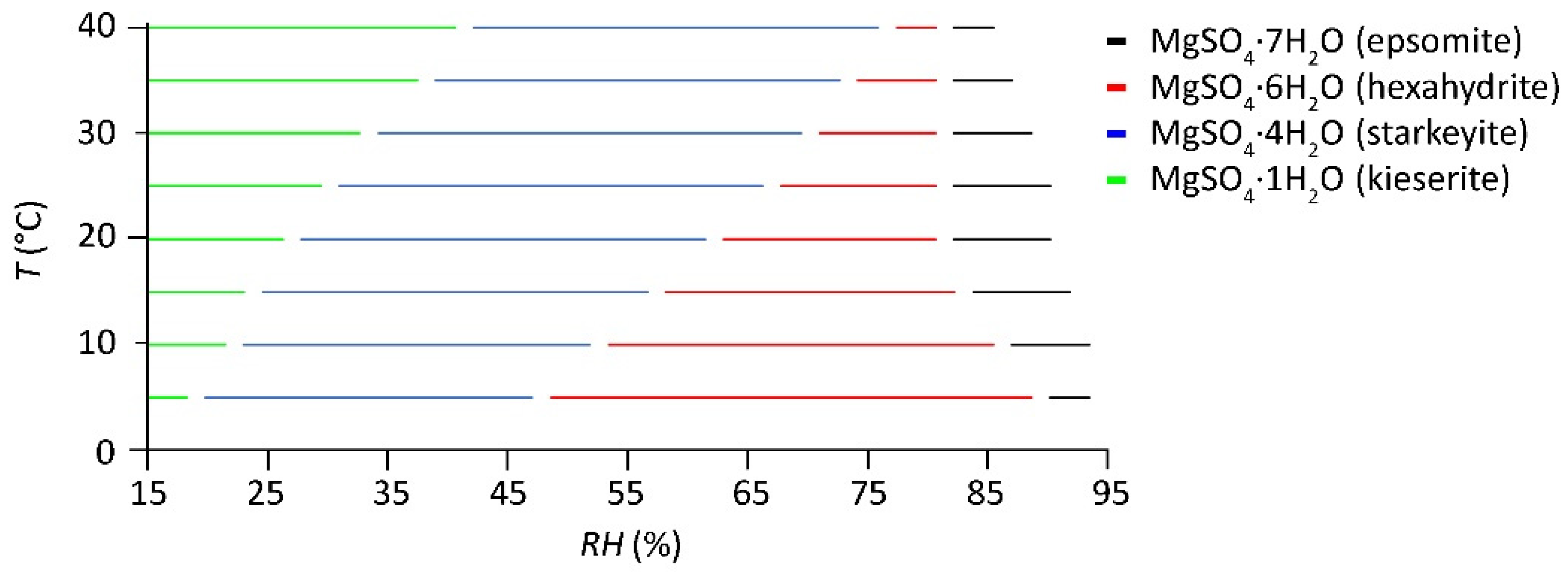
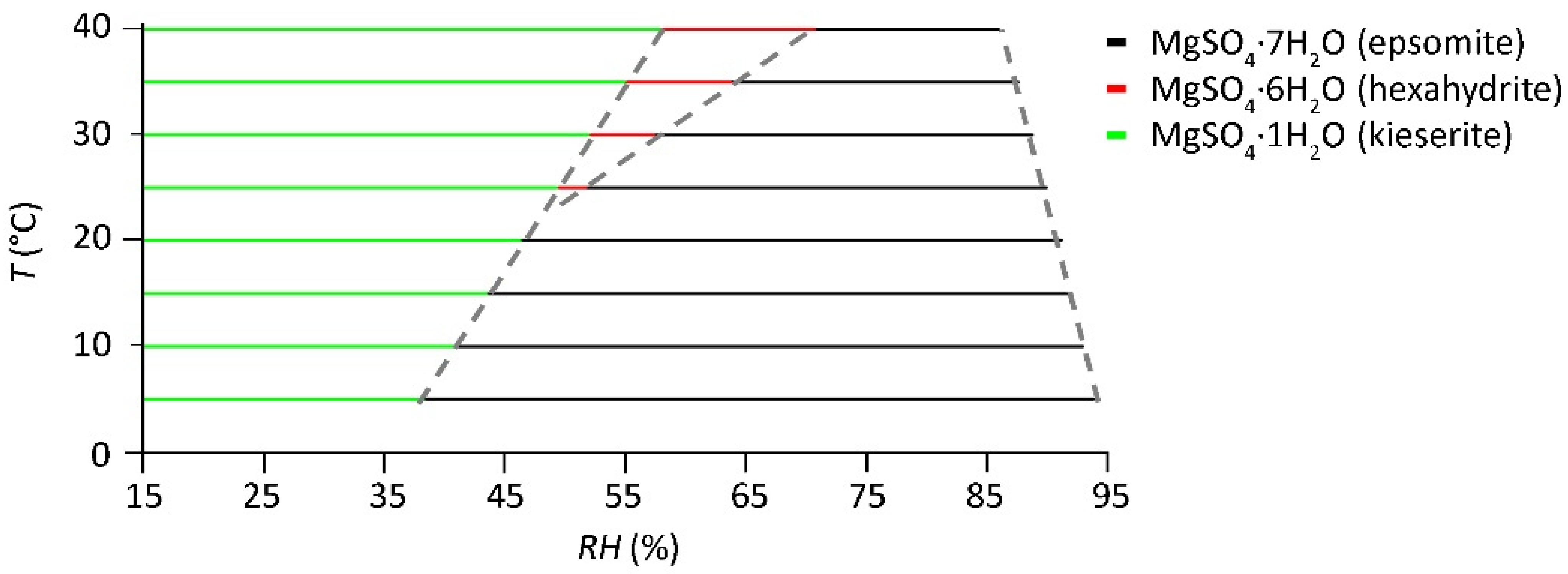
4. ECOS/RUNSALT Limitations and Solutions
5. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Evans, I.S. Salt Crystallization and Rock Weathering: A Review. Rev. Geomorphol. Dyn. 1970, 19, 153–177. [Google Scholar]
- Goudie, A.; Viles, H. Salt Weathering Hazards; John Wiley & Sons: Chichester, UK, 1997; ISBN 0-471-95842-5. [Google Scholar]
- Doehne, E. Salt Weathering: A Selective Review. Geol. Soc. Spec. Publ. 2002, 205, 51–64. [Google Scholar] [CrossRef]
- Doehne, E.; Price, C.A. Stone Conservation: An Overview of Current Research, 2nd ed.; Research in Conservation; Getty Conservation Institute: Los Angeles, CA, USA, 2010; ISBN 978-1-60606-046-9. [Google Scholar]
- Snethlage, R.; Sterflinger, K. Stone Conservation. In Stone in Architecture; Siegesmund, S., Snethlage, R., Eds.; Springer Berlin Heidelberg: Berlin/Heidelberg, Germany, 2011; pp. 411–544. ISBN 978-3-642-14474-5. [Google Scholar]
- Oguchi, C.T.; Yu, S. A Review of Theoretical Salt Weathering Studies for Stone Heritage. Prog. Earth Planet. Sci. 2021, 8, 1–23. [Google Scholar] [CrossRef]
- Godts, S.; Steiger, M.; Orr, S.A.; De Kock, T.; Desarnaud, J.; De Clercq, H.; Cnudde, V. Charge Balance Calculations for Mixed Salt Systems Applied to a Large Dataset from the Built Environment. Sci. Data 2022, 9, 324. [Google Scholar] [CrossRef]
- Price, C.; Brimblecombe, P. Preventing Salt Damage in Porous Materials. Stud. Conserv. 1994, 39, 90–93. [Google Scholar] [CrossRef]
- Espinosa, R.M.; Franke, L.; Deckelmann, G. Model for the Mechanical Stress Due to the Salt Crystallization in Porous Materials. Constr. Build. Mater. 2008, 22, 1350–1367. [Google Scholar] [CrossRef]
- Espinosa, R.M.; Franke, L.; Deckelmann, G. Phase Changes of Salts in Porous Materials: Crystallization, Hydration and Deliquescence. Constr. Build. Mater. 2008, 22, 1758–1773. [Google Scholar] [CrossRef]
- Naillon, A.; Joseph, P.; Prat, M. Ion Transport and Precipitation Kinetics as Key Aspects of Stress Generation on Pore Walls Induced by Salt Crystallization. Phys. Rev. Lett. 2018, 120, 034502. [Google Scholar] [CrossRef]
- Li, L.; Kohler, F.; Dziadkowiec, J.; Røyne, A.; Espinosa Marzal, R.M.; Bresme, F.; Jettestuen, E.; Dysthe, D.K. Limits to Crystallization Pressure. Langmuir 2022, 38, 11265–11273. [Google Scholar] [CrossRef]
- Espinosa-Marzal, R.M.; Scherer, G.W. Advances in Understanding Damage by Salt Crystallization. Acc. Chem. Res. 2010, 43, 897–905. [Google Scholar] [CrossRef]
- Flatt, R.; Aly Mohamed, N.; Caruso, F.; Derluyn, H.; Desarnaud, J.; Lubelli, B.; Espinosa Marzal, R.M.; Pel, L.; Rodriguez-Navarro, C.; Scherer, G.W.; et al. Predicting Salt Damage in Practice: A Theoretical Insight into Laboratory Tests. RILEM Tech. Lett. 2017, 2, 108–118. [Google Scholar] [CrossRef]
- Espinosa-Marzal, R.M.; Scherer, G.W. Impact of In-Pore Salt Crystallization on Transport Properties. Environ. Earth Sci. 2013, 69, 2657–2669. [Google Scholar] [CrossRef]
- Price, C. An Expert Chemical Model for Determining the Environmental Conditions Needed to Prevent Salt Damage in Porous Materials: Protection and Conservation of the European Cultural Heritage; Project ENV4-CT95-0135 (1996–2000) Final Report; Protection and conservation of the European Cultural Heritage; Archetype: London, UK, 2000; ISBN 978-1-873132-52-4. [Google Scholar]
- Bionda, D. RUNSALT—A Graphical User Interface to the ECOS Thermodynamic Model for the Prediction of the Behaviour of Salt Mixtures under Changing Climate Conditions (2005). Available online: Http://Science.Sdf-Eu.Org/Runsalt/ (accessed on 9 October 2022).
- Bionda, D.; Storemyr, P. Modelling the Behaviour of Salts Mixtures in Walls: A Case Study from Tenaille von Fersen Building, Suomenlinna, Finland. In Proceedings of the Study of Salt Deterioration Mechanisms. Decay of Brick Walls Influenced by Interior Climate Changes; von Konow, T., Ed.; Suomenlinnan Hoitokunta: Suomenlinna, Finland, 2002; pp. 95–101. [Google Scholar]
- Bionda, D. Methodology for the Preventive Conservation of Sensitive Monuments: Microclimate and Salt Activity in a Church. In Proceedings of the 10th International Congress on Deterioration and Conservation of Stone, Stockholm, Sweden, 27 June–2 July 2004; Kwiatkowski, D., Löfvendahl, R., Eds.; ICOMOS Sweden: Stockholm, Sweden, 2004; pp. 627–634. [Google Scholar]
- Klenz Larsen, P. The Salt Decay of Medieval Bricks at a Vault in Brarup Church, Denmark. Environ. Geol. 2007, 52, 375–383. [Google Scholar] [CrossRef]
- Prokos, P. Equilibrium Conditions of Marine Originated Salt Mixtures: An ECOS Application at the Archaeological Site of Delos, Greece. In Proceedings of the SWBSS, Salt Weathering of Buildings and Stone Sculptures, Copenhagen, Denmark, 22–24 October 2008; Ottosen, L.M., Ed.; Technical University of Denmark: Lyngby, Denmark, 2008. [Google Scholar]
- Eklund, S. Stone Weathering in the Monastic Building Complex on Mountain of St Aaron in Petra, Jordan; University of Helsinki: Helsinki, Jordan, 2008. [Google Scholar]
- Franzen, C.; Mirwald, P.W. Moisture Sorption Behaviour of Salt Mixtures in Porous Stone. Geochemistry 2009, 69, 91–98. [Google Scholar] [CrossRef]
- Maguregui, M.; Sarmiento, A.; Martínez-Arkarazo, I.; Angulo, M.; Castro, K.; Arana, G.; Etxebarria, N.; Madariaga, J.M. Analytical Diagnosis Methodology to Evaluate Nitrate Impact on Historical Building Materials. Anal. Bioanal. Chem. 2008, 391, 1361–1370. [Google Scholar] [CrossRef] [PubMed]
- Maguregui, M.; Knuutinen, U.; Castro, K.; Madariaga, J.M. Raman Spectroscopy as a Tool to Diagnose the Impact and Conservation State of Pompeian Second and Fourth Style Wall Paintings Exposed to Diverse Environments (House of Marcus Lucretius). J. Raman Spectrosc. 2010, 41, 1400–1409. [Google Scholar] [CrossRef]
- Price, C.A. Predicting Environmental Conditions to Minimise Salt Damage at the Tower of London: A Comparison of Two Approaches. Environ. Geol. 2007, 52, 369–374. [Google Scholar] [CrossRef]
- Sawdy, A.; Heritage, A. Evaluating the Influence of Mixture Composition on the Kinetics of Salt Damage in Wall Paintings Using Time Lapse Video Imaging with Direct Data Annotation. Environ. Geol. 2007, 52, 303–315. [Google Scholar] [CrossRef]
- Sawdy, A.; Price, C. Salt Damage at Cleeve Abbey, England. J. Cult. Herit. 2005, 6, 125–135. [Google Scholar] [CrossRef]
- Steiger, M. Modellierung von Phasengleichgewichten. In Proceedings of the DBU Workshops im Februar 2008 in Osnabrück, Salzschäden an Kulturgütern Stand des Wissens und Forschungsdefizite; Schwarz, H.-J., Steiger, M., Eds.; Deutsche Bundesstiftung Umwelt: Hannover, Germany, 2009; pp. 80–99. [Google Scholar]
- Zehnder, K.; Schoch, O. Efflorescence of Mirabilite, Epsomite and Gypsum Traced by Automated Monitoring on-Site. J. Cult. Herit. 2009, 10, 319–330. [Google Scholar] [CrossRef]
- Sawdy, A. The Kinetics of Salt Weathering of Porous Materials; Institute of Archaeology University College London: London, UK, 2001. [Google Scholar]
- Bionda, D. RUNSALT. Available online: http://science.sdf-eu.org/runsalt/ (accessed on 6 January 2022).
- Menéndez, B. Estimation of Salt Mixture Damage on Built Cultural Heritage from Environmental Conditions Using ECOS-RUNSALT Model. J. Cult. Herit. 2017, 24, 22–30. [Google Scholar] [CrossRef]
- Scatigno, C.; Prieto-Taboada, N.; Festa, G.; Madariaga, J.M. Soluble Salts Quantitative Characterization and Thermodynamic Modeling on Roman Bricks to Assess the Origin of Their Formation. Molecules 2021, 26, 2866. [Google Scholar] [CrossRef] [PubMed]
- Godts, S.; Hayen, R.; De Clercq, H. Investigating Salt Decay of Stone Materials Related to the Environment, a Case Study in the St. James Church in Liège, Belgium. Stud. Conserv. 2017, 62, 329–342. [Google Scholar] [CrossRef]
- Gómez-Laserna, O.; Cardiano, P.; Diez-Garcia, M.; Prieto-Taboada, N.; Kortazar, L.; Olazabal, M.Á.; Madariaga, J.M. Multi-Analytical Methodology to Diagnose the Environmental Impact Suffered by Building Materials in Coastal Areas. Environ. Sci. Pollut. Res. 2018, 25, 4371–4386. [Google Scholar] [CrossRef]
- Gibeaux, S.; Thomachot-Schneider, C.; Eyssautier-Chuine, S.; Marin, B.; Vazquez, P. Simulation of Acid Weathering on Natural and Artificial Building Stones According to the Current Atmospheric SO2/NOx Rate. Environ. Earth Sci. 2018, 77, 327. [Google Scholar] [CrossRef]
- Heinrichs, K.; Azzam, R. Quantitative Analysis of Salt Crystallization–Dissolution Processes on Rock-Cut Monuments in Petra/Jordan. In Engineering Geology for Society and Territory—Volume 8; Lollino, G., Giordan, D., Marunteanu, C., Christaras, B., Yoshinori, I., Margottini, C., Eds.; Springer International Publishing: Cham, Sweden, 2015; pp. 507–510. ISBN 978-3-319-09407-6. [Google Scholar]
- Crevals, V.; Godts, S.; Desarnaud, J. Salt Problems and Climate Control in the Case of the Church of Sint Aldegondis in Mespelare, Belgium, an ECOS/Runsalt Approach. In Proceedings of the Fifth International Conference on Salt Weathering of Buildings and Stone Sculptures, Delft, The Netherlands, 22–24 September 2021; Lubelli, B., Kamat, A.A., Quist, W.J., Eds.; TU Delft Open: Delft, The Netherlands, 2021; pp. 13–20. [Google Scholar]
- Godts, S.; Hayen, R.; Clercq, H.D. Common Salt Mixtures Database: A Tool to Identify Research Needs. In Proceedings of the 3rd International Conference on Salt Weathering of Buildings and Stone Sculptures, Brussels, Belgium, 14–16 October 2014; KIK-IRPA: Brussels, Belgium, 2014; p. 14. [Google Scholar]
- Godts, S.; Hayen, R. Onderzoek en preventie van vocht- en zoutschade gerelateerd aan het klimaat in de crypte van de Sint-Baafskathedraal te Gent. In Proceedings of the WTA-PRECOM3OS Studiedag: Preventieve Conservatie: Van klimaat- en schademonitoring naar een geïntegreerde benadering, Leuven, Belgium, 5 April 2019; Verstynge, E., van Bommel, B., Vernimme, N., van Hees, R., Eds.; TUDelft—KULeuven: Leuven, Belgium, 2019. [Google Scholar]
- Godts, S.; Clercq, C.D. Analysis of the Salt Content during Water Bath Desalination of a Polychrome Limestone Relief. In Proceedings of the 14th International Congress on the Deterioration and Conservation of Stone, Monument Future: Decay and Conservation of Stone, Göttingen, Germany, 7–12 September 2020; Siegesmund, S., Middendorf, B., Eds.; Mitteldeutscher Verlag: Göttingen, Germany, 2020; pp. 853–858. [Google Scholar]
- Godts, S.; Orr, S.A.; Desarnaud, J.; Steiger, M.; Wilhelm, K.; De Clercq, H.; Cnudde, V.; De Kock, T. NaCl-Related Weathering of Stone: The Importance of Kinetics and Salt Mixtures in Environmental Risk Assessment. Herit. Sci. 2021, 9, 1–13. [Google Scholar] [CrossRef]
- Godts, S.; Clercq, H.D.; Hayen, R.; Roy, J.D. Risk Assessment and Conservation Strategy of a Salt Laden Limestone Mausoleum and the Surrounding Funeral Chapel in Boussu, Belgium. In Proceedings of the 12th International Congress on the Deterioration and Conservation of Stone, New York, NY, USA, 22–26 October 2012; Columbia University: New York, NY, USA, 2012. [Google Scholar]
- De Clercq, H.; Godts, S. Rehabilitation of Farmhouses and Barns: Limits of Salt Content. Appl. Phys. A 2016, 122, 831. [Google Scholar] [CrossRef]
- De Clercq, H.; Godts, S.; Hayen, R. Impact of the Indoor Climate on the Performance of Building Materials Contaminated with Salt Mixtures. Conserv. Manag. Archaeol. Sites 2014, 16, 39–55. [Google Scholar] [CrossRef]
- Menéndez, B. Estimators of the Impact of Climate Change in Salt Weathering of Cultural Heritage. Geosciences 2018, 8, 401. [Google Scholar] [CrossRef]
- Laue, S.; Poerschke, D.; Hübner, B. Investigation and Conservation of Salt Damaged Epitaphs in the Church of Werben (Saxony-Anhalt, Germany). SWBSS 2017 Fourth Int Conf Salt Weather Build Stone Sculpt. In Proceedings of the Fourth Int Conf salt Weather Build Stone Sculpt, Potsdam, Germany, 20–22 September 2017; Laue, S., Ed.; Verlag der Fachhochschule: Potsdam, Germany, 2017. [Google Scholar]
- Chabas, A.; Kloppmann, W.; Sizun, J.-P.; Wille, G.; Coman, A.; Petitmangin, A.; Nowak, S.; Martin, E.; Jurgens, M.-A. Sources and Chronology of Soluble Salt Formation in a Medieval Dovecote Caught up in Urbanization: A Resilience Story? Environ. Sci. Pollut. Res. 2022. preprint. [Google Scholar] [CrossRef]
- Pintér, F. The Combined Use of Ion Chromatography and Scanning Electron Microscopy to Assess Salt-Affected Mineral Materials in Cultural Heritage. J. Am. Inst. Conserv. 2022, 61, 85–99. [Google Scholar] [CrossRef]
- Godts, S.; Orr, S.A.; Steiger, M.; De Kock, T. Mixed Salt Systems in the Built Environment—Charge Balance Calculations [Data Set]. Available online: https://zenodo.org/record/6280617#.Y32dBX1BxPY (accessed on 22 September 2022).
- Richards, J.; Brimblecombe, P. The Transfer of Heritage Modelling from Research to Practice. Herit. Sci. 2022, 10. [Google Scholar] [CrossRef]
- Marion, G.M.; Mironenko, M.V.; Roberts, M.W. FREZCHEM: A Geochemical Model for Cold Aqueous Solutions. Comput. Geosci. 2010, 36, 10–15. [Google Scholar] [CrossRef]
- Wexler, A.S. Atmospheric Aerosol Models for Systems Including the Ions H+, NH4+, Na+, SO42−, NO3−, Cl−, Br−, and H2O. J. Geophys. Res. 2002, 107, 4207. [Google Scholar] [CrossRef]
- Clegg, S.L.; Pitzer, K.S.; Brimbiecombet, P. Thermodynamics of Multicomponent, Miscible, Ionic Solutions. 2. Mixtures Includlng Unsymmetrical Electrolytes. J Phys Chem 1992, 96, 9470–9479. [Google Scholar] [CrossRef]
- Gruszkiewicz, M.S.; Palmer, D.A.; Springer, R.D.; Wang, P.; Anderko, A. Phase Behavior of Aqueous Na–K–Mg–Ca–Cl–NO3 Mixtures: Isopiestic Measurements and Thermodynamic Modeling. J. Solut. Chem. 2007, 36, 723–765. [Google Scholar] [CrossRef]
- Thomsen, K.; Rasmussen, P.; Gani, R. Correlation and Prediction of Thermal Properties and Phase Behaviour for a Class of Aqueous Electrolyte Systems. Chem. Eng. Sci. 1996, 51, 3675–3683. [Google Scholar] [CrossRef]
- Wang, P.; Anderko, A.; Young, R.D. A Speciation-Based Model for Mixed-Solvent Electrolyte Systems. Fluid Phase Equilibria 2002, 203, 141–176. [Google Scholar] [CrossRef]
- Wang, P.; Springer, R.D.; Anderko, A.; Young, R.D. Modeling Phase Equilibria and Speciation in Mixed-Solvent Electrolyte Systems. Fluid Phase Equilibria 2004, 222, 11–17. [Google Scholar] [CrossRef]
- Wang, P.; Anderko, A.; Springer, R.D.; Young, R.D. Modeling Phase Equilibria and Speciation in Mixed-Solvent Electrolyte Systems: II. Liquid–Liquid Equilibria and Properties of Associating Electrolyte Solutions. J. Mol. Liq. 2006, 125, 37–44. [Google Scholar] [CrossRef]
- Hingerl, F.F.; Wagner, T.; Kulik, D.A.; Kosakowski, G.; Driesner, T.; Institut, P.S.; Hingerl, F. Development of a New Activity Model for Complex Mixed-Salt Solutions from Ambient to Geothermal Conditions. In Proceedings of the Geophysical Research Abstracts, Vienna, Austria, 22–27 April 2012; Volume 14, p. 5332. [Google Scholar]
- Parkhurst, D.L.; Appelo, C.A.J. Description of Input and Examples for PHREEQC Version 3: A Computer Program for Speciation, Batch-Reaction, One-Dimensional Transport, and Inverse Geochemical Calculations; Techniques and Methods; U.S. Geological Survey: Reston, VA, USA, 2013; Volume 6-A43, p. 519. [Google Scholar]
- Benavente, D.; Brimblecombe, P.; Grossi, C.M. Thermodynamic Calculations for the Salt Crystallisation Damage in Porous Built Heritage Using PHREEQC. Environ. Earth Sci. 2015, 74, 2297–2313. [Google Scholar] [CrossRef]
- Pérez-Diez, S.; Fernández-Menéndez, L.J.; Morillas, H.; Martellone, A.; De Nigris, B.; Osanna, M.; Bordel, N.; Caruso, F.; Madariaga, J.M.; Maguregui, M. Elucidation of the Chemical Role of the Pyroclastic Materials on the State of Conservation of Mural Paintings from Pompeii. Angew. Chem. Int. Ed. 2021, 60, 3028–3036. [Google Scholar] [CrossRef] [PubMed]
- Marcilla, A.; Reyes-Labarta, J.A.; Olaya, M.M. Should We Trust All the Published LLE Correlation Parameters in Phase Equilibria? Necessity of Their Assessment Prior to Publication. Fluid Phase Equilibria 2017, 433, 243–252. [Google Scholar] [CrossRef]
- Pitzer, K.S. Ion Interaction Approach: Theory and Data Correlation. In Activity coefficients in electrolyte solutions; CRC Press: Boca Raton, FL, USA, 1991; pp. 75–153. [Google Scholar]
- Clegg, S.L.; Pitzer, K.S. Thermodynamics of Multicomponent, Miscible, Ionic Solutions: Generalized Equations for Symmetrical Electrolytes. J. Phys. Chem. 1992, 96, 3513–3520. [Google Scholar] [CrossRef]
- Pitzer, K.S.; Simonson, J.M. Thermodynamics of Multicomponent, Miscible, Ionic Systems: Theory and Equations. J. Phys. Chem. 1986, 90, 3005–3009. [Google Scholar] [CrossRef]
- Pitzer, K.S. The Treatment of Ionic Solutions over the Entire Miscibility Range. Berichte Bunsenges. Für Phys. Chem. 1981, 85, 952–959. [Google Scholar] [CrossRef]
- Clegg, S.L. Personal Communication about the ECOS/Runsalt Model and Backend Calculations; University of East Anglia: Norwich, UK, 2021. [Google Scholar]
- Clegg, S.L.; Brimblecombe, P. Pitzer Model of Electrolyte Solutions. In An Expert Chemical Model for Determining the Environmental Conditions Needed to Prevent Salt Damage in Porous Materials: Project ENV4-CT95-0135 (1996-2000) Final Report; Protection and conservation of the European Cultural Heritage; Price, C.A., Ed.; Archetype: London, UK, 2000; pp. 13–18. ISBN 978-1-873132-52-4. [Google Scholar]
- Steiger, M.; Kiekbusch, J.; Nicolai, A. An Improved Model Incorporating Pitzer’s Equations for Calculation of Thermodynamic Properties of Pore Solutions Implemented into an Efficient Program Code. Constr. Build. Mater. 2008, 22, 1841–1850. [Google Scholar] [CrossRef]
- Pitzer, K.S.; Mayorga, G. Thermodynamics of Electrolytes. III. Activity and Osmotic Coefficients for 2–2 Electrolytes. J. Solut. Chem. 1974, 3, 539–546. [Google Scholar] [CrossRef]
- Steiger, M.; Asmussen, S. Crystallization of Sodium Sulfate Phases in Porous Materials: The Phase Diagram Na2SO4–H2O and the Generation of Stress. Geochim. Cosmochim. Acta 2008, 72, 4291–4306. [Google Scholar] [CrossRef]
- Steiger, M. Salts in Porous Materials: Thermodynamics of Phase Transitions, Modeling and Preventive Conservation. Restor. Build. Monum. 2005, 11, 419–432. [Google Scholar] [CrossRef]
- Lindström, N.; Talreja, T.; Linnow, K.; Stahlbuhk, A.; Steiger, M. Crystallization Behavior of Na2SO4–MgSO4 Salt Mixtures in Sandstone and Comparison to Single Salt Behavior. Appl. Geochem. 2016, 69, 50–70. [Google Scholar] [CrossRef]
- Shen, Y.; Linnow, K.; Steiger, M. Crystallization Behavior and Damage Potential of Na2SO4–NaCl Mixtures in Porous Building Materials. Cryst. Growth Des. 2020, 20, 5974–5985. [Google Scholar] [CrossRef]
- Steiger, M.; Charola, A.E.; Sterflinger, K. Weathering and Deterioration. In Stone in Architecture; Siegesmund, S., Snethlage, R., Eds.; Springer Berlin Heidelberg: Berlin/Heidelberg, Germany, 2014; pp. 225–316. ISBN 978-3-642-45154-6. [Google Scholar]
- Steiger, M.; Heritage, A. Modelling the Crystallization Behaviour of Mixed Salt Systems: Input Data Requirements. In Proceedings of the 12th International Congress on the Deterioration and Conservation of Stone, New York, NY, USA, 22–26 October 2012. [Google Scholar]
- Arnold, A.; Zehnder, K. Monitoring Wall Paintings Affected by Soluble Salts; Cather, S., Ed.; The Courtauld Institute of Fine Art and The Getty Conservation Institute: London, UK, 1987; pp. 103–135. [Google Scholar]
- Costa, F.M.d.C.; Henriques Rosa, M.A.N.; Canetto, M.; Sobral da Fonseca, M.J. The Degradation of the “Study Room” (Convent of Christ, Tomar, Portugal), from a Preliminary Analysis towards a Sustainable Maintenance. Ge-conservacion 2022, 21, 95–107. [Google Scholar] [CrossRef]
- Gulotta, D.; Godts, S.; De Kock, T.; Steiger, M. Comparative Estimation of the Pore Filling of Single Salts in Natural Stone. In Proceedings of the Fifth International Conference on Salt Weathering of Buildings and Stone Sculptures, Delft, The Netherlands, 22–24 September 2021; Lubelli, B., Kamat, A.A., Quist, W.J., Eds.; TU Delft Open: Delft, The Netherlands, 2021. [Google Scholar]
- Rörig-Dalgaard, I. Direct Measurements of the Deliquescence Relative Humidity in Salt Mixtures Including the Contribution from Metastable Phases. ACS Omega 2021, 6, 16297–16306. [Google Scholar] [CrossRef] [PubMed]
- Steiger, M.; Linnow, K.; Ehrhardt, D.; Rohde, M. Decomposition Reactions of Magnesium Sulfate Hydrates and Phase Equilibria in the MgSO4–H2O and Na+–Mg2+–Cl−–SO42−–H2O Systems with Implications for Mars. Geochim. Cosmochim. Acta 2011, 75, 3600–3626. [Google Scholar] [CrossRef]
- Archer, D.G.; Kirklin, D.R. Enthalpies of Solution of Sodium Chloride and Potassium Sulfate in Water. Thermodynamic Properties of the Potassium Sulfate + Water System. J. Chem. Eng. Data 2002, 47, 33–46. [Google Scholar] [CrossRef]
- Flatt, R.; Bocherens, P. Sur Le Système Ternaire Ca++_K+_NO3-_H2O. Helv Chim Acta 1962, 45, 187–195. [Google Scholar] [CrossRef]
- Siedel, H. Salt Efflorescence as Indicator for Sources of Damaging Salts on Historic Buildings and Monuments: A Statistical Approach. Environ. Earth Sci. 2018, 77, 572. [Google Scholar] [CrossRef]
- Morillas, H.; Maguregui, M.; Paris, C.; Bellot-Gurlet, L.; Colomban, P.; Madariaga, J.M. The Role of Marine Aerosol in the Formation of (Double) Sulfate/Nitrate Salts in Plasters. Microchem. J. 2015, 123, 148–157. [Google Scholar] [CrossRef][Green Version]
- Benavente, D.; de Jongh, M.; Cañaveras, J.C. Weathering Processes and Mechanisms Caused by Capillary Waters and Pigeon Droppings on Porous Limestones. Minerals 2020, 11, 18. [Google Scholar] [CrossRef]
- Von Konow, T. Test Results. The Study of Salt Deterioration Mechanisms. Decay of Brick Walls Influenced by Interior Climate Changes; von Konow, T., Ed.; Suomenlinnan Hoitokunta: Helsinki, Finland, 2002. [Google Scholar]
- Charola, A.; Lewin, S. Efflorescence on Building Stones—SEM in the Characterization and Elucidation of the Mechanism of Formation. Scan Electron Microsc 1979, 79, 379–387. [Google Scholar]
- Arnold, A.; Küng, A. Crystallization and Habit of Salt Efflorescences on Walls I. In Proceedings of the 5th International Congress on Deterioration and Conservation of Stone, Lausanne, Switzerland, 25–27 September 1985; Félix, G., Ed.; Presses Romandes: Lausanne, Switzerland, 1985; pp. 255–267. [Google Scholar]
- Bionda, D. Modelling Indoor Climate and Salt Behaviour in Historical Buildings; Swiss Federal Institute of Technology: Zurich, Switzerland, 2006. [Google Scholar]




| Meaning | Base Symbol | Species-Specific Symbol 1 | |
|---|---|---|---|
| Explanation following RUNSALT plots (example Figure 1) | |||
| 1. | Mutual crystallization relative humidity | ||
| RH point at the onset of any line shown in a plot corresponding to the start of crystallization; the number shown in the specific symbol refers to the species/solid in order of appearance from a humid to a dry environment. The use of the number (e.g., 1) in relation to the solids can be useful to understand the sequence of crystallization. The solution at this point is saturated with respect to a specific solid. When available, the first letters of the mineral name or chemical formula can used to replace the number, e.g., is aphthitalite = aph and thus (letter A in Figure 1). Aphthitalite is the first that crystallizes in the mixture and the same base symbol is used for the mutual crystallization relative humidity of all solids that crystallize (indicated with the letter B in Figure 1). This is only relevant when solution is still available before crystallization takes place (reactions in solution in Table 2). | |||
| 2. | Mutual dissolution relative humidity | ||
| RH point at the end of a horizontal line in a plot, looking from a dry to a humid environment, equals the start of dissolution; e.g., in Figure 1 this is illustrated by the RH points indicated with the letter C, and thus when solution becomes available. | |||
| 3. | Mutual deliquescence relative humidity | ||
| RH point at the end of a horizontal line in a plot when no more solution is available, looking from a dry to a humid environment, e.g., indicated as letter D in Figure 1. Here, the last solid that crystallizes is darapskite, and afterwards no more solution is available. Thus, , as further illustrated by reaction number 2 shown in Table 2. | |||
| 4. | Mutual transition relative humidity | ||
| RH point at which salt transitions occur. The numbers refer to the solids involved in the transition, starting with solids before the dash (e.g., 3 in [3–5]) at more humid conditions transitioning to solids after the dash (e.g., 5 in [3–5]) at dryer conditions. Either a phase change (hydration, dehydration), decomposition, or the formation (addition) of solids occur under both wetting and drying conditions. For example, the transition of mirabilite to thenardite is , or is more complicated, as shown by reaction 3 in Table 2 (letter E in Figure 1). | |||
| Additional terms that are useful when calculating water activities or concentrations. Values that are not included in the RUNSALT output data yet could be derived from the ECOS calculations. | |||
| 5. | Mutual equilibrium relative humidity | ||
| Any RH point at which a solution is in equilibrium with its environment = water activity at any concentration if solution is available, e.g., in Figure 1 any RH point above D, and thus . | |||
| 6. | Mutual saturation relative humidity | ||
| Any RH point at which a solution is saturated (points on the curves, e.g., in Figure 1, all RH points between A and C on the curve of aphthitalite crystallization), equal to the points during crystallization (when solid and solution are available). | |||
| Start Composition of the Solution (mol): 2Na+ + 2K+ + 1Cl− + 1NO3− + 1SO42− | |
|---|---|
| # | Reactions in solution |
| 1. | 2Na+ + 6K+ + 4SO42− → Na2SO4∙3K2SO4 (cr) |
| 2. | Na2SO4∙3K2SO4 (cr) + 11Na+ + Cl− + 10NO3− + 4H2O → NaCl (cr) + 6KNO3 (cr) + 4NaNO3∙Na2SO4∙H2O (cr) |
| Solid-state reactions | |
| 3. | 6NaNO3∙Na2SO4∙H2O (cr) + Na2K SO4)4 (cr) → 6KNO3 (cr) + 10Na2SO4 (cr) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Godts, S.; Steiger, M.; Orr, S.A.; Stahlbuhk, A.; Desarnaud, J.; De Clercq, H.; Cnudde, V.; De Kock, T. Modeling Salt Behavior with ECOS/RUNSALT: Terminology, Methodology, Limitations, and Solutions. Heritage 2022, 5, 3648-3663. https://doi.org/10.3390/heritage5040190
Godts S, Steiger M, Orr SA, Stahlbuhk A, Desarnaud J, De Clercq H, Cnudde V, De Kock T. Modeling Salt Behavior with ECOS/RUNSALT: Terminology, Methodology, Limitations, and Solutions. Heritage. 2022; 5(4):3648-3663. https://doi.org/10.3390/heritage5040190
Chicago/Turabian StyleGodts, Sebastiaan, Michael Steiger, Scott Allan Orr, Amelie Stahlbuhk, Julie Desarnaud, Hilde De Clercq, Veerle Cnudde, and Tim De Kock. 2022. "Modeling Salt Behavior with ECOS/RUNSALT: Terminology, Methodology, Limitations, and Solutions" Heritage 5, no. 4: 3648-3663. https://doi.org/10.3390/heritage5040190
APA StyleGodts, S., Steiger, M., Orr, S. A., Stahlbuhk, A., Desarnaud, J., De Clercq, H., Cnudde, V., & De Kock, T. (2022). Modeling Salt Behavior with ECOS/RUNSALT: Terminology, Methodology, Limitations, and Solutions. Heritage, 5(4), 3648-3663. https://doi.org/10.3390/heritage5040190







