The Physical Characterization and Terminal Velocities of Aluminium, Iron and Plastic Bottle Caps in a Water Environment
Abstract
:1. Introduction
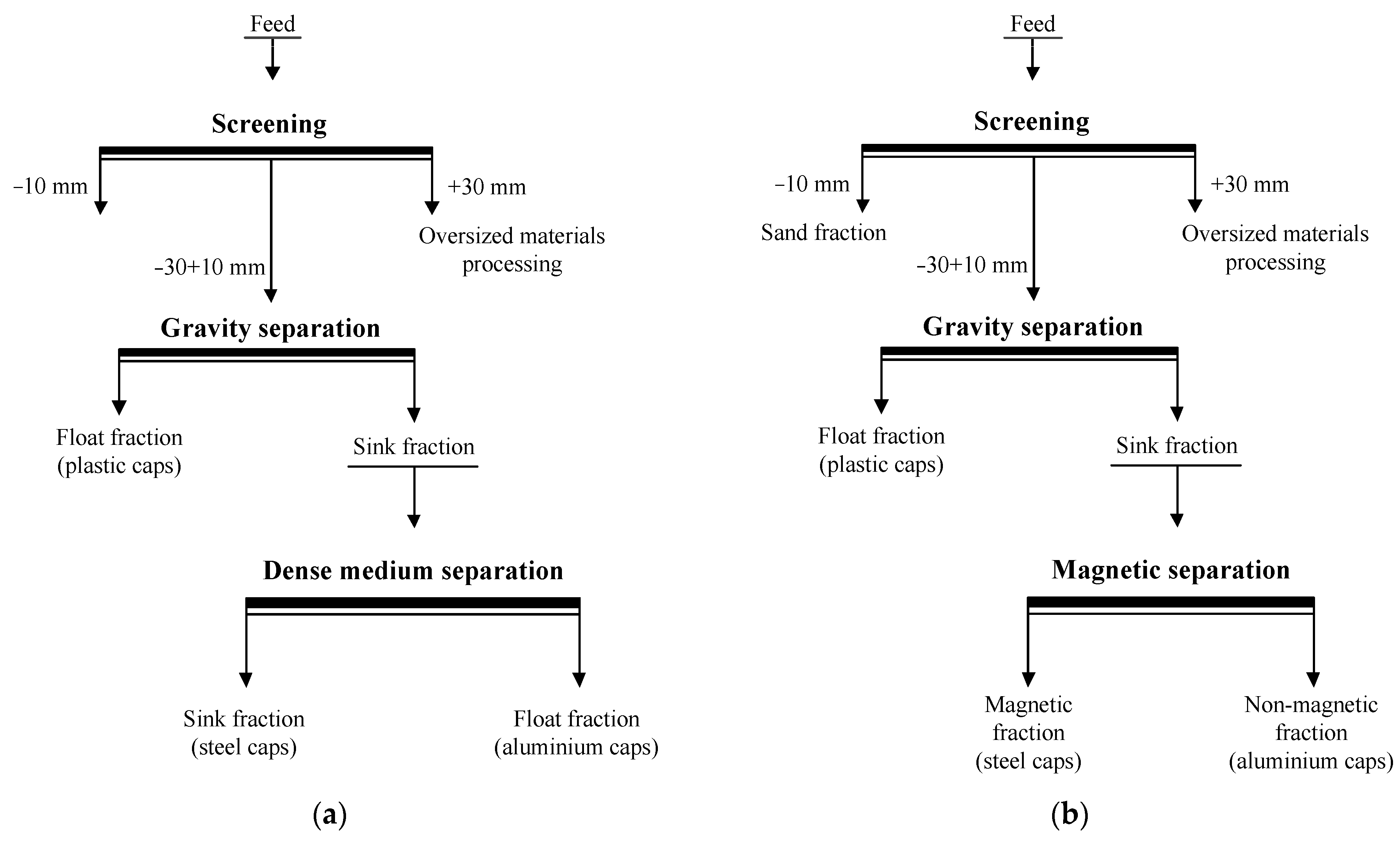
2. Materials and Methods
2.1. Physical Properties Measurements Techniques
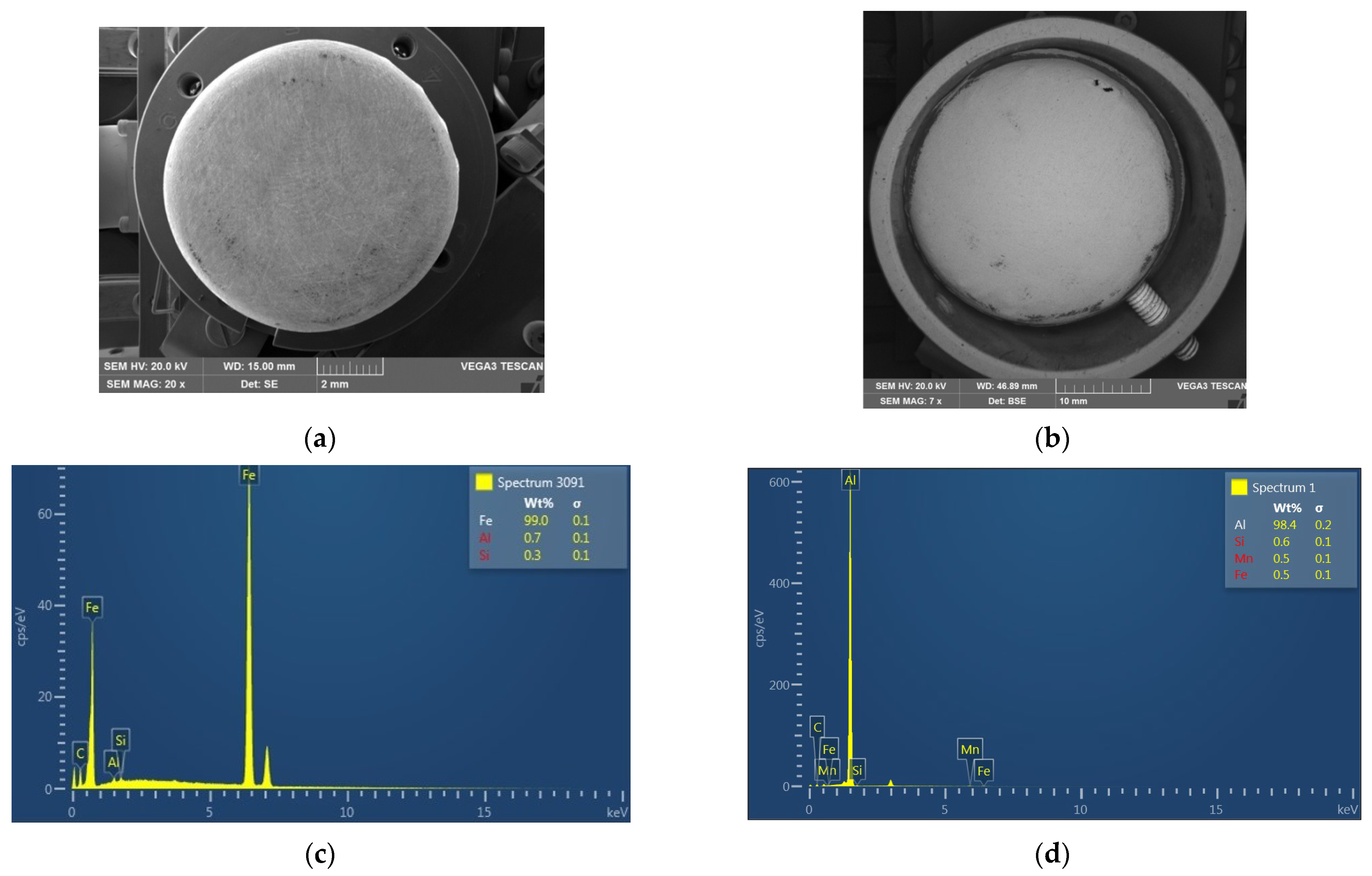
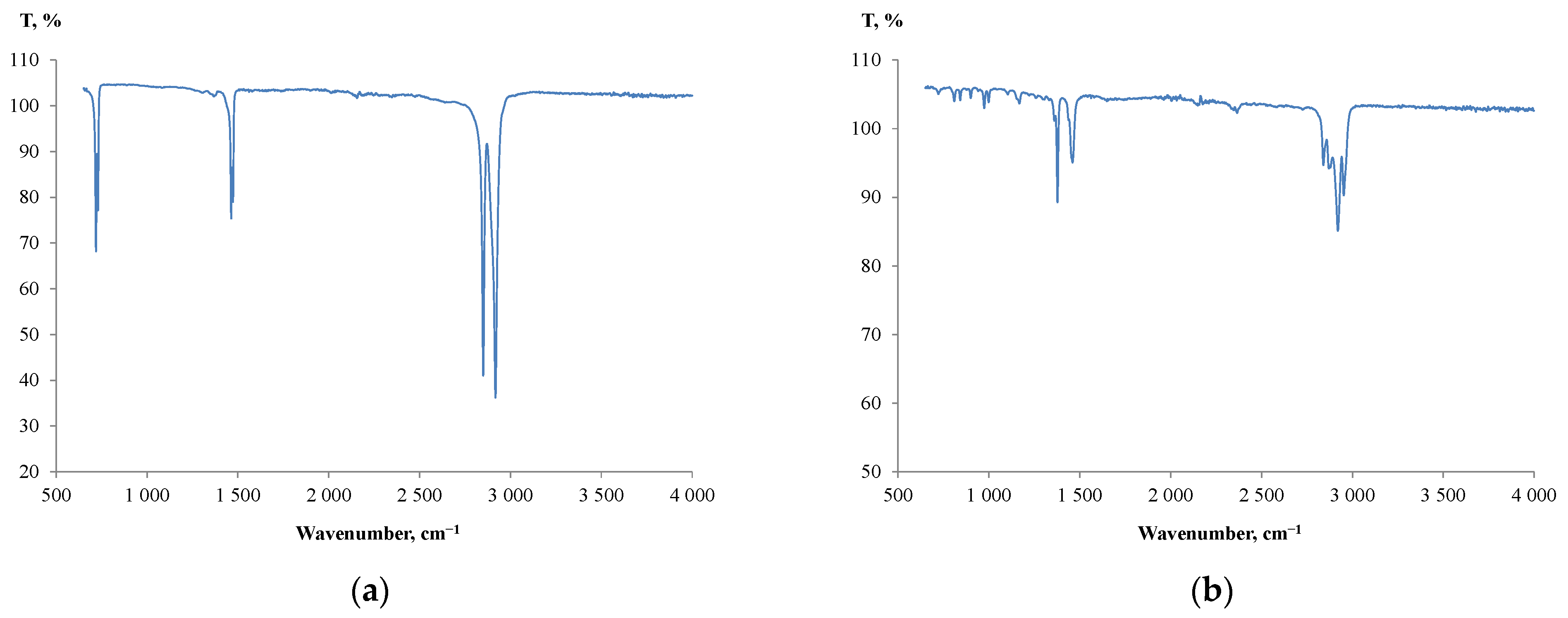
2.2. SEM Analysis and FTIR Spectroscopy
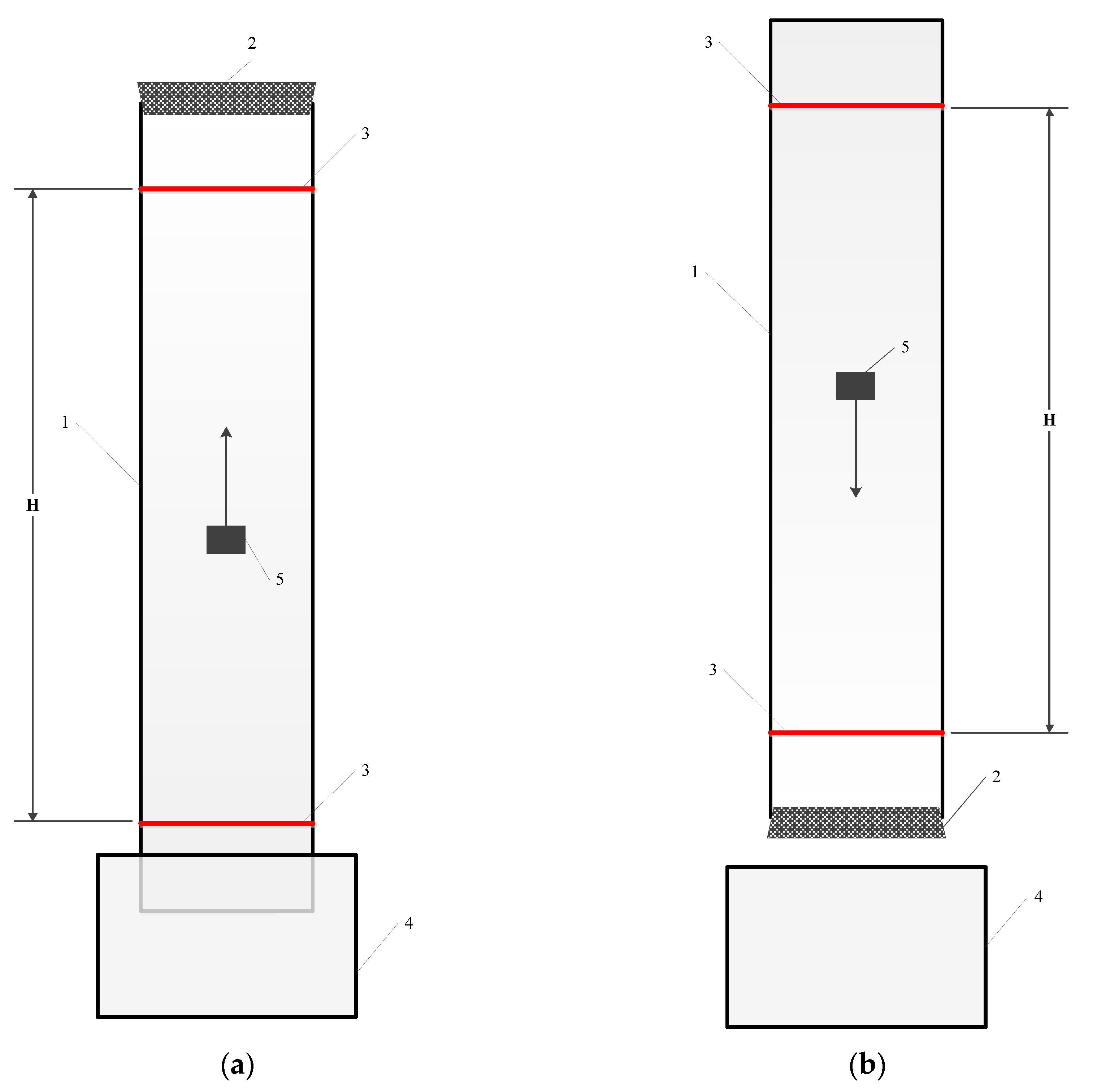
2.3. Settling and Rising Tests
3. Results and Discussion
3.1. Physical Characteristics of the Particles
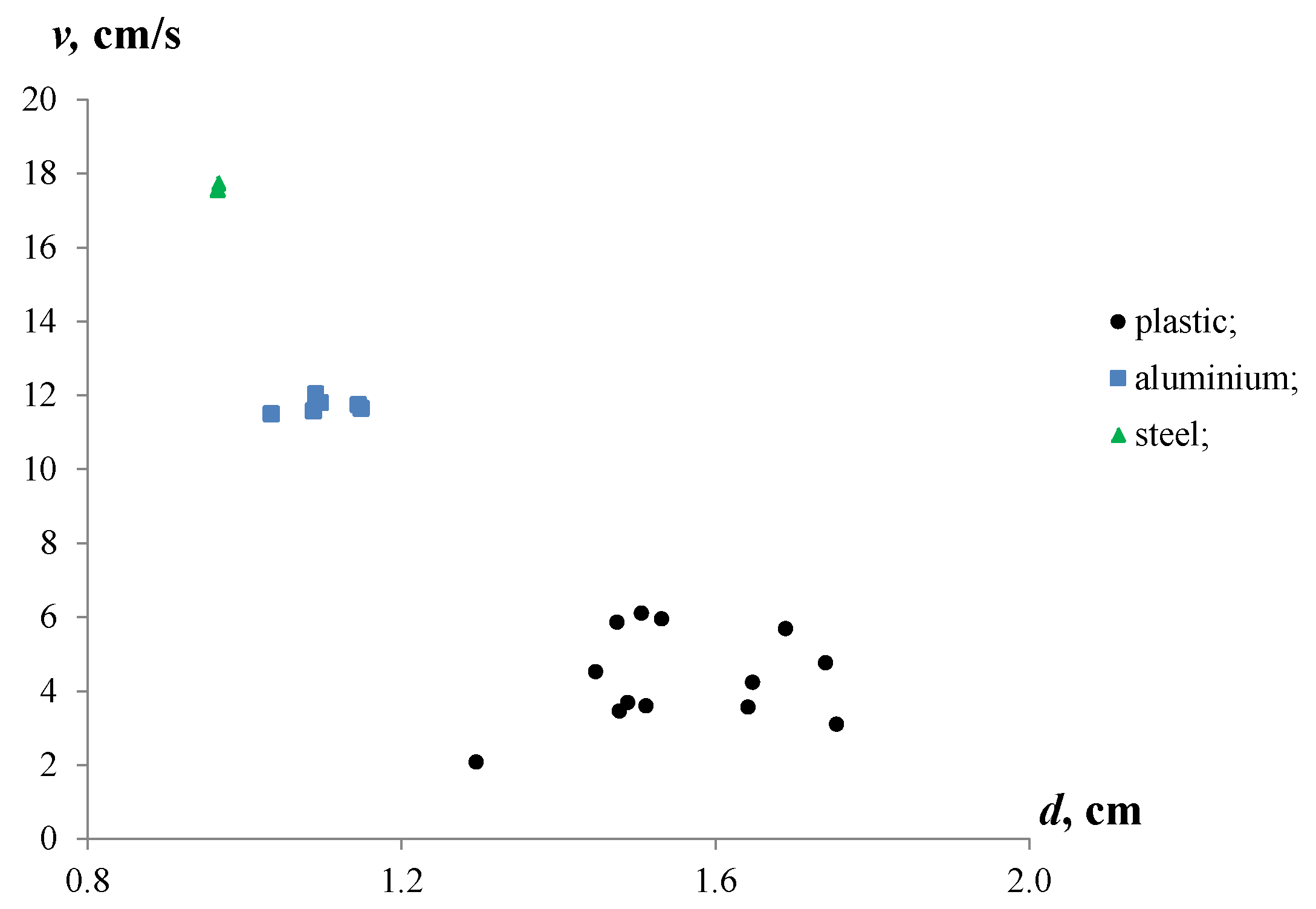
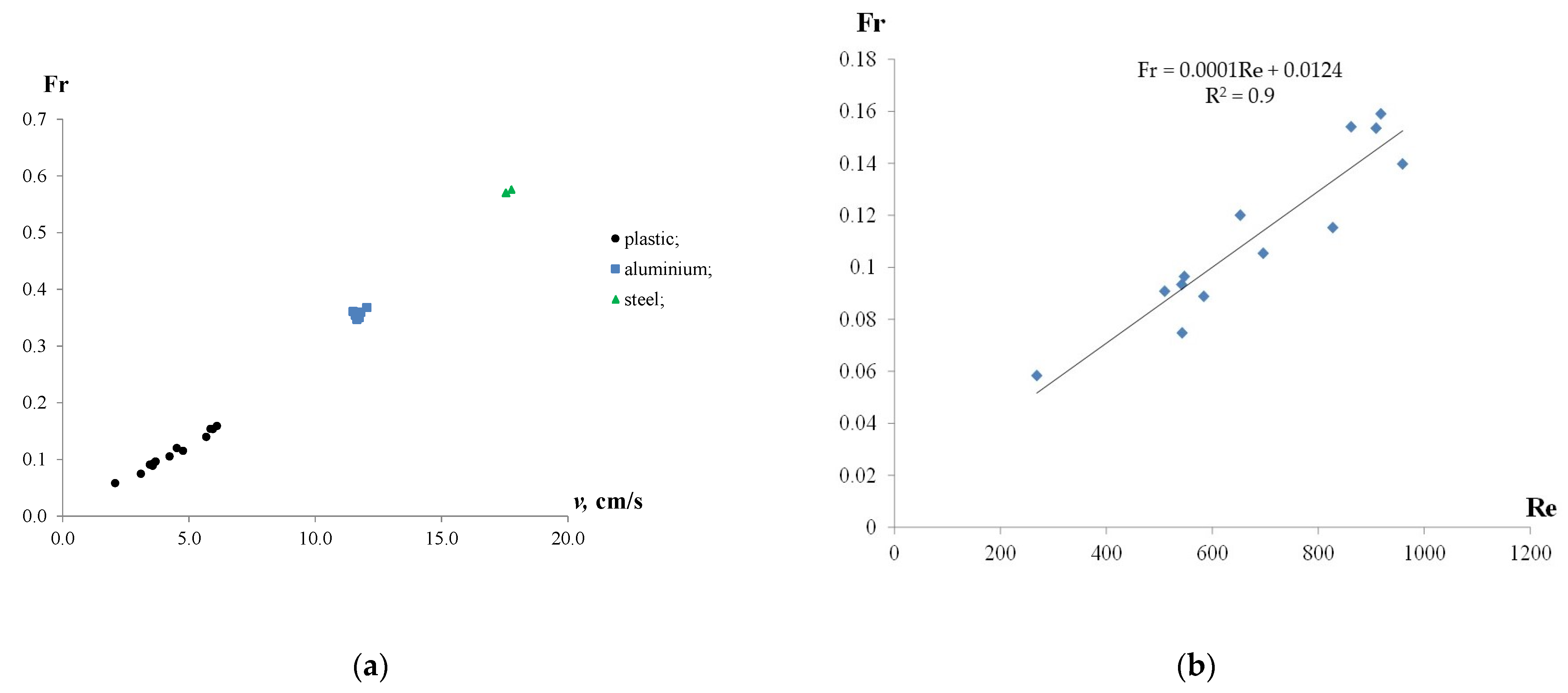
3.2. Particle Settling Velocity
3.3. Particle Rising Velocity
3.4. Analysis of the Results of Particles Settling and Rising in Water
4. Conclusions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Grumezescu, A.M.; Holban, A.M. Bottled and packaged water. In Science of Beverages; Woodhead Publishing: Thorston, UK, 2019; Volume 4, p. 488. [Google Scholar]
- World Steel Association. Steel Statistical Yearbook. 2018. Available online: https://www.worldsteel.org/en/dam/jcr:e5a8eda5-4b46-4892-856b-00908b5ab492/SSY_2018.pdf (accessed on 8 May 2021).
- Non-Alcoholic Beverage Market Size, Share & Trends Analysis Report By Product (CSD, Fruit Beverages, Bottled Water, Functional Beverages, Sports Drinks), By Distribution Channel, And Segment Forecasts, 2018–2025. Available online: https://www.grandviewresearch.com/industry-analysis/nonalcoholic-beverage-market (accessed on 8 May 2021).
- Bleich, S.N.; Wang, Y.C.; Wang, Y.; Gortmaker, S.L. Increasing consumption of sugar-sweetened beverages among US adults: 1988–1994 to 1999–2001. Am. J. Clin. Nutr. 2009, 89, 372–381. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Henckens, M.L.C.M.; van Ierland, E.C.; Driessen, P.P.J.; Worrell, E. Mineral resources: Geological scarcity, market price trends, and future generations. Resour. Policy 2016, 49, 102–111. [Google Scholar] [CrossRef] [Green Version]
- The Global Aluminium Recycling Committee. International Aluminum Institute Global Aluminium Recycling: A Cornerstone of Sustainable Development; International Aluminium Institute: London, UK, 2009. [Google Scholar]
- Paraskevas, D.; Kellens, K.; Dewulf, W.; Duflou, J.R. Environmental modelling of aluminium recycling: A Life Cycle Assessment tool for sustainable metal management. J. Clean. Prod. 2015, 105, 357–370. [Google Scholar] [CrossRef]
- Jacobsen, R.; Willeghems, G.; Gellynck, X.; Buysse, J. Increasing the quantity of separated post-consumer plastics for reducing combustible household waste: The case of rigid plastics in Flanders. Waste Manag. 2018, 78, 708–716. [Google Scholar] [CrossRef]
- Agarski, B.; Vukelic, D.; Micunovic, M.I.; Budak, I. Evaluation of the environmental impact of plastic cap production, packaging, and disposal. J. Environ. Manag. 2019, 245, 55–65. [Google Scholar] [CrossRef]
- Gu, F.; Guo, J.; Zhang, W.; Summers, P.A.; Hall, P. From waste plastics to industrial raw materials: A life cycle assessment of mechanical plastic recycling practice based on a real-world case study. Sci. Total Environ. 2017, 601–602, 1192–1207. [Google Scholar] [CrossRef]
- Jambeck, J.R.; Geyer, R.; Wilcox, C.; Siegler, T.R.; Perryman, M.; Andrady, A.; Narayan, R.; Law, K.L. Plastic waste inputs from land into the ocean. Science 2015, 347, 768–771. [Google Scholar] [CrossRef]
- Jiang, Q.; Izumi, T.; Yoshida, H.; Dilixiati, D.; Leeabai, N.; Suzuki, S.; Takahashi, F. The effect of recycling bin design on PET bottle collection performance. Waste Manag. 2019, 95, 32–42. [Google Scholar] [CrossRef]
- Boonstra, M.; van Hest, F. The Plastic Bottle Cap Report. Findings of the First Survey into Plastic Bottle Cap Pollution on Beaches in The Netherlands. 2017. Available online: https://www.bottlebill.org/images/PDF/Dutch%20study%20on%20caps_Doppenrapport_EN_2017_DEF_small.pdf (accessed on 8 May 2021).
- De Frond, H.L.; Van Sebille, E.; Parnis, J.M.; Diamond, M.L.; Mallos, N.; Kingsbury, T.; Rochman, C.M. Estimating the Mass of Chemicals Associated with Ocean Plastic Pollution to Inform Mitigation Efforts. Integr. Environ. Assess. Manag. 2019, 15, 596–606. [Google Scholar] [CrossRef]
- Abelouah, M.R.; Ben-Haddad, M.; Alla, A.A.; Rangel-Buitrago, N. Marine litter in the central Atlantic coast of Morocco. Ocean Coast. Manag. 2021, 214, 105940. [Google Scholar] [CrossRef]
- Simeonova, A.; Chuturkova, R. Marine litter accumulation along the Bulgarian Black Sea coast: Categories and predominance. Waste Manag. 2019, 84, 182–193. [Google Scholar] [CrossRef] [PubMed]
- Gall, M.; Schweighuber, A.; Buchberger, W.; Lang, R.W. Plastic Bottle Cap Recycling—Characterization of Recyclate Composition and Opportunities for Design for Circularity. Sustainability 2020, 12, 10378. [Google Scholar] [CrossRef]
- Kaya, M. Recovery of metals and nonmetals from electronic waste by physical and chemical recycling processes. Waste Manag. 2016, 57, 64–90. [Google Scholar] [CrossRef] [PubMed]
- Ziouzios, D.; Baras, N.; Balafas, V.; Dasygenis, M.; Stimoniaris, A. Intelligent and Real-Time Detection and Classification Algorithm for Recycled Materials Using Convolutional Neural Networks. Recycling 2022, 7, 9. [Google Scholar] [CrossRef]
- Martey, S.; Hendren, K.; Farfaras, N.; Kelly, J.C.; Newsome, M.; Ciesielska-Wrobel, I.; Sobkowicz, M.J.; Chen, W.-T. Recycling of Pretreated Polyolefin-Based Ocean-Bound Plastic Waste by Incorporating Clay and Rubber. Recycling 2022, 7, 25. [Google Scholar] [CrossRef]
- Lubongo, C.; Alexandridis, P. Assessment of Performance and Challenges in Use of Commercial Automated Sorting Technology for Plastic Waste. Recycling 2022, 7, 11. [Google Scholar] [CrossRef]
- Balakrishnan, P.; Sreekala, M.S. Recycling of Plastics. In Recycling of Polymers: Methods, Characterization and Applications; Wiley-VCH: Weinheim, Germany, 2016. [Google Scholar]
- Wills, B.A.; Finch, J.A. Gravity Concentration. In Wills’ Mineral Processing Technology; Butterworth-Heinemann: Oxford, UK, 2016. [Google Scholar]
- Ruj, B.; Pandey, V.; Jash, P.; Srivastava, V.K. Sorting of plastic waste for effective recycling. Int. J. Appl. Sci. Eng. Res. 2015, 4, 564–571. [Google Scholar] [CrossRef]
- Kumar, A.; Holuszko, M.E.; Janke, T. Assessing the Applicability of Gravity Separation for Recycling of Non-Metal Fraction from Waste Printed Circuit Boards. Adv. Sustain. Syst. 2022, 6, 2000231. [Google Scholar] [CrossRef]
- Lowes, C.; Zhou, J.; McGrath, T.; Eksteen, J.; Galvin, K. Characterisation and Modelling of Gravity Pre-Concentration Amenability Using LST Fluidisation in a REFLUX™ classifier. Minerals 2020, 10, 545. [Google Scholar] [CrossRef]
- Whitworth, A.J.; Forbes, E.; Verster, I.; Jokovic, V.; Awatey, B.; Parbhakar-Fox, A. Review on advances in mineral processing technologies suitable for critical metal recovery from mining and processing wastes. Clean. Eng. Technol. 2022, 7, 100451. [Google Scholar] [CrossRef]
- Flemmer, R.L.C.; Banks, C.L. On the drag coefficient of a sphere. Powder Technol. 1986, 48, 217–221. [Google Scholar] [CrossRef]
- Brown, P.P.; Lawler, D.F. Sphere Drag and Settling Velocity Revisited. J. Environ. Eng. 2003, 129, 222–231. [Google Scholar] [CrossRef]
- Cheng, N.-S. Comparison of formulas for drag coefficient and settling velocity of spherical particles. Powder Technol. 2009, 189, 395–398. [Google Scholar] [CrossRef]
- Terfous, A.; Hazzab, A.; Ghenaim, A. Predicting the drag coefficient and settling velocity of spherical particles. Powder Technol. 2013, 239, 12–20. [Google Scholar] [CrossRef]
- Mikhailov, M.D.; Freire, A.P.S. The drag coefficient of a sphere: An approximation using Shanks transform. Powder Technol. 2013, 237, 432–435. [Google Scholar] [CrossRef]
- Barati, R.; Neyshabouri, S.A.A.S.; Ahmadi, G. Development of empirical models with high accuracy for estimation of drag coefficient of flow around a smooth sphere: An evolutionary approach. Powder Technol. 2014, 257, 11–19. [Google Scholar] [CrossRef]
- Song, X.; Xu, Z.; Li, G.; Pang, Z.; Zhu, Z. A new model for predicting drag coefficient and settling velocity of spherical and non-spherical particle in Newtonian fluid. Powder Technol. 2017, 321, 242–250. [Google Scholar] [CrossRef]
- Goossens, W.R.A. Review of the empirical correlations for the drag coefficient of rigid spheres. Powder Technol. 2019, 352, 350–359. [Google Scholar] [CrossRef]
- Tran-Cong, S.; Gay, M.; Michaelides, E.E. Drag coefficients of irregularly shaped particles. Powder Technol. 2004, 139, 21–32. [Google Scholar] [CrossRef]
- Loth, E. Drag of non-spherical solid particles of regular and irregular shape. Powder Technol. 2008, 182, 342–353. [Google Scholar] [CrossRef]
- Gabitto, J.; Tsouris, C. Drag coefficient and settling velocity for particles of cylindrical shape. Powder Technol. 2008, 183, 314–322. [Google Scholar] [CrossRef]
- Bagheri, G.H.; Bonadonna, C.; Manzella, I.; Vonlanthen, P. On the characterization of size and shape of irregular particles. Powder Technol. 2015, 270, 141–153. [Google Scholar] [CrossRef]
- Bagheri, G.; Bonadonna, C. On the drag of freely falling non-spherical particles. Powder Technol. 2016, 301, 526–544. [Google Scholar] [CrossRef] [Green Version]
- Zhu, X.; Zeng, Y.H.; Huai, W.X. Settling velocity of non-spherical hydrochorous seeds. Adv. Water Resour. 2017, 103, 99–107. [Google Scholar] [CrossRef]
- Breakey, D.E.S.; Vaezi, G.F.; Masliyah, J.H.; Sanders, R.S. Side-view-only determination of drag coefficient and settling velocity for non-spherical particles. Powder Technol. 2018, 339, 182–191. [Google Scholar] [CrossRef]
- Gerhardter, H.; Prieler, R.; Mayr, B.; Knoll, M.; Mühlböck, M.; Tomazic, P.; Hochenauer, C. Evaluation of drag models for particles and powders with non-uniform size and shape. Powder Technol. 2018, 330, 152–163. [Google Scholar] [CrossRef]
- Esteban, L.B.; Shrimpton, J.; Ganapathisubramani, B. Study of the circularity effect on drag of disk-like particles. Int. J. Multiph. Flow 2019, 110, 189–197. [Google Scholar] [CrossRef] [Green Version]
- Hayashi, K.; Tomiyama, A. A drag correlation of fluid particles rising through stagnant liquids in vertical pipes at intermediate Reynolds numbers. Chem. Eng. Sci. 2009, 64, 3019–3028. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.; Stevens, G.W.; Fei, W. An experimental study on single drop rising in a low interfacial tension liquid–liquid system. Chem. Eng. Res. Des. 2019, 148, 349–360. [Google Scholar] [CrossRef]
- Yan, X.; Jia, Y.; Wang, L.; Cao, Y. Drag coefficient fluctuation prediction of a single bubble rising in water. Chem. Eng. J. 2017, 316, 553–562. [Google Scholar] [CrossRef]
- Liu, L.; Tang, H.; Quan, S. Shapes and terminal velocities of a drop rising in stagnant liquids. Comput. Fluids 2013, 81, 17–25. [Google Scholar] [CrossRef]
- van Hout, R.; Gulitski, A.; Barnea, D.; Shemer, L. Experimental investigation of the velocity field induced by a Taylor bubble rising in stagnant water. Int. J. Multiph. Flow 2002, 28, 579–596. [Google Scholar] [CrossRef] [Green Version]
- Churyumov, A.V.; Bazlov, A.I.; Zadorozhnyy, V.Y.; Solonin, A.N.; Caron, A.; Louzguine-Luzgin, D.V. Phase transformations in Zr-based bulk metallic glass cyclically loaded before plastic yielding. Mater. Sci. Eng. A 2012, 550, 358–362. [Google Scholar] [CrossRef]
- Pozdniakov, A.V.; Lotfy, A.; Qadir, A.; Shalaby, E.; Khomutov, M.G.; Churyumov, A.Y.; Zolotorevskiy, V.S. Development of Al-5Cu/B4C composites with low coefficient of thermal expansion for automotive application. Mater. Sci. Eng. A 2017, 688, 1–8. [Google Scholar] [CrossRef]
- Amelinckx, S.; Van Dyck, D.; Van Landuyt, J.; Van Tendeloo, G. Electron Microscopy: Principles and Fundamentals; Wiley: Weinheim, Germany, 2007; ISBN 9783527614561. [Google Scholar]
- Khvan, A.V.; Dinsdale, A.T.; Cheverikin, V.V.; Kudashov, D.; Stepanov, P.P.; Watson, A.; Kondratiev, A. Oxide formation during electric resistance welding of low carbon steels. Mater. Sci. Technol. 2016, 32, 556–567. [Google Scholar] [CrossRef]
- Jung, M.R.; Horgen, F.D.; Orski, S.V.; Rodriguez, C.V.; Beers, K.L.; Balazs, G.H.; Jones, T.T.; Work, T.M.; Brignac, K.C.; Royer, S.-J.; et al. Validation of ATR FT-IR to identify polymers of plastic marine debris, including those ingested by marine organisms. Mar. Pollut. Bull. 2018, 127, 704–716. [Google Scholar] [CrossRef] [PubMed]
- Asensio, R.C.; San Andrés Moya, M.; De La Roja, J.M.; Gómez, M. Analytical characterization of polymers used in conservation and restoration by ATR-FTIR spectroscopy. Anal. Bioanal. Chem. 2009, 395, 2081–2096. [Google Scholar] [CrossRef]
- Kim, Y.T.; Min, B.; Kim, K.W. General Characteristics of Packaging Materials for Food System. In Innovations in Food Packaging, 2nd ed.; Academic Press: Cambridge, MA, USA, 2014; ISBN 9780123946010. [Google Scholar] [CrossRef]
- Shokhin, V.N.; Lopatin, A.G. Gravitatsionnye Metody Obogashcheniya; Nauka: Moskva, Russia, 1980. [Google Scholar]
- White, F.M. Fluid Mechanics; McGraw-Hill: New York, NY, USA, 1991. [Google Scholar]
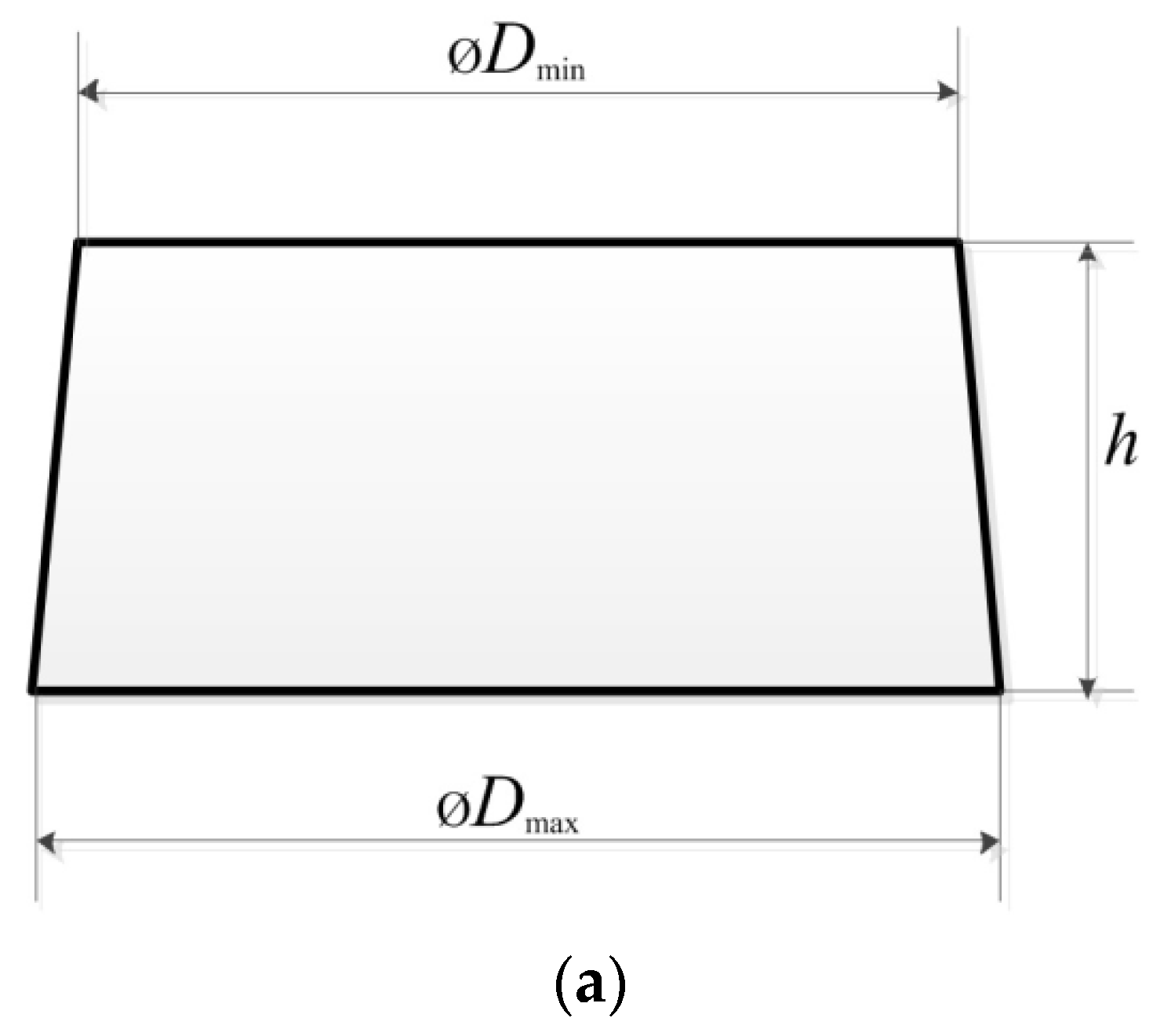
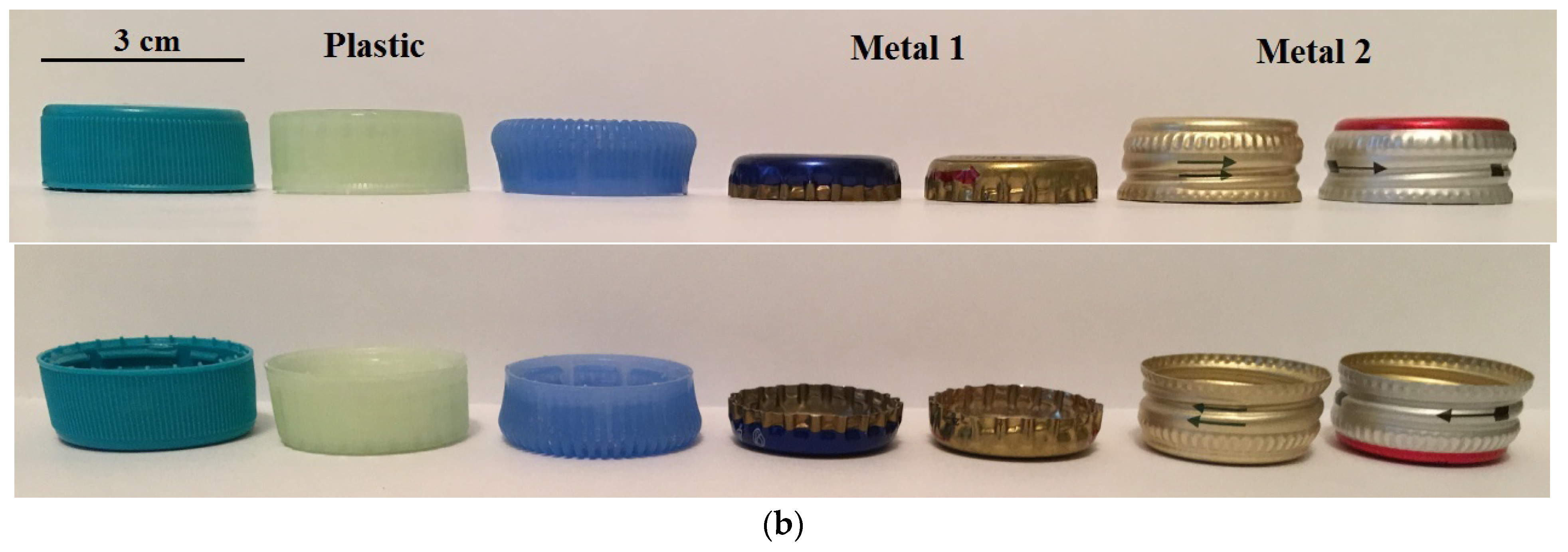

| Particle | Material | Dmin, cm | Dmax, cm | h, cm | r | dm, cm | V1, cm3 | ρp, g/cm3 | de, cm |
|---|---|---|---|---|---|---|---|---|---|
| Particle 1 | Plastic | 2.8 | 3.0 | 1.2 | 2.5 | 2.33 | 1.69 | 0.94 | 1.48 |
| Particle 2 | Plastic | 2.8 | 3.1 | 1.2 | 2.6 | 2.37 | 2.52 | 0.904 | 1.69 |
| Particle 3 | Plastic | 2.7 | 3.1 | 1.5 | 2.1 | 2.43 | 2.76 | 0.946 | 1.74 |
| Particle 4 | Plastic | 2.8 | 3.1 | 1.1 | 2.8 | 2.33 | 2.34 | 0.936 | 1.65 |
| Particle 5 | Plastic | 2.6 | 2.7 | 1.2 | 2.3 | 2.17 | 2.32 | 0.611 | 1.64 |
| Particle 6 | Plastic | 2.8 | 3.1 | 1.2 | 2.6 | 2.37 | 1.81 | 0.930 | 1.51 |
| Particle 7 | Plastic | 3.2 | 3.0 | 1.1 | 2.7 | 2.43 | 2.83 | 0.752 | 1.75 |
| Particle 8 | Plastic | 2.8 | 3.0 | 1.1 | 2.7 | 2.30 | 1.73 | 0.934 | 1.49 |
| Particle 9 | Plastic | 2.1 | 2.4 | 1.3 | 1.8 | 1.93 | 1.59 | 0.956 | 1.45 |
| Particle 10 | Plastic | 2.2 | 2.5 | 1.3 | 1.9 | 2.00 | 1.88 | 0.944 | 1.53 |
| Particle 11 | Plastic | 2.3 | 2.3 | 1.2 | 1.9 | 1.93 | 1.67 | 0.953 | 1.47 |
| Particle 12 | Plastic | 2.4 | 2.5 | 1.2 | 2.1 | 2.03 | 1.13 | 0.916 | 1.29 |
| Particle 13 | Plastic | 2.0 | 2.4 | 1.3 | 1.8 | 1.90 | 1.79 | 0.937 | 1.51 |
| Particle 14 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 15 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 16 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 17 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 18 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 19 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 20 | Metal 1 | 2.6 | 2.8 | 0.6 | 4.7 | 2.00 | 0.48 | 4.43 | 0.97 |
| Particle 21 | Metal 2 | 2.5 | 2.9 | 1.3 | 2.2 | 2.23 | 0.69 | 1.77 | 1.10 |
| Particle 22 | Metal 2 | 2.5 | 2.9 | 1.3 | 2.2 | 2.23 | 0.67 | 1.80 | 1.09 |
| Particle 23 | Metal 2 | 2.5 | 2.9 | 1.3 | 2.2 | 2.23 | 0.68 | 1.80 | 1.09 |
| Particle 24 | Metal 2 | 2.5 | 2.9 | 1.3 | 2.2 | 2.23 | 0.79 | 1.66 | 1.15 |
| Particle 25 | Metal 2 | 2.5 | 2.9 | 1.3 | 2.2 | 2.23 | 0.78 | 1.67 | 1.14 |
| Particle 26 | Metal 2 | 2.5 | 2.9 | 1.2 | 2.4 | 2.20 | 0.58 | 1.97 | 1.03 |
| Particles | ρp, g/cm3 | de, cm | v, cm/s | ψ | Re | Fr | |
|---|---|---|---|---|---|---|---|
| Rising | Settling | ||||||
| Plastic | 0.752–0.956 | 1.29–1.75 | 2.1–6.1 | – | 1.0–4.2 1 | 543–959 | 0.06–0.16 |
| Aluminium | 1.66–1.97 | 1.03–1.15 | – | 11.5–12.0 | 2.9–3.9 | 1186–1342 | 0.35–0.37 |
| Iron | 4.43 | 0.97–1.1 | – | 17.6–17.7 | 5.4–5.5 | 1692–1711 | 0.57–0.58 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nikolaev, A.A. The Physical Characterization and Terminal Velocities of Aluminium, Iron and Plastic Bottle Caps in a Water Environment. Recycling 2022, 7, 28. https://doi.org/10.3390/recycling7030028
Nikolaev AA. The Physical Characterization and Terminal Velocities of Aluminium, Iron and Plastic Bottle Caps in a Water Environment. Recycling. 2022; 7(3):28. https://doi.org/10.3390/recycling7030028
Chicago/Turabian StyleNikolaev, Alexander A. 2022. "The Physical Characterization and Terminal Velocities of Aluminium, Iron and Plastic Bottle Caps in a Water Environment" Recycling 7, no. 3: 28. https://doi.org/10.3390/recycling7030028
APA StyleNikolaev, A. A. (2022). The Physical Characterization and Terminal Velocities of Aluminium, Iron and Plastic Bottle Caps in a Water Environment. Recycling, 7(3), 28. https://doi.org/10.3390/recycling7030028





