Hydrodynamics of Supersonic Steam Jets Injected into Cross-Flowing Water
Abstract
:1. Introduction
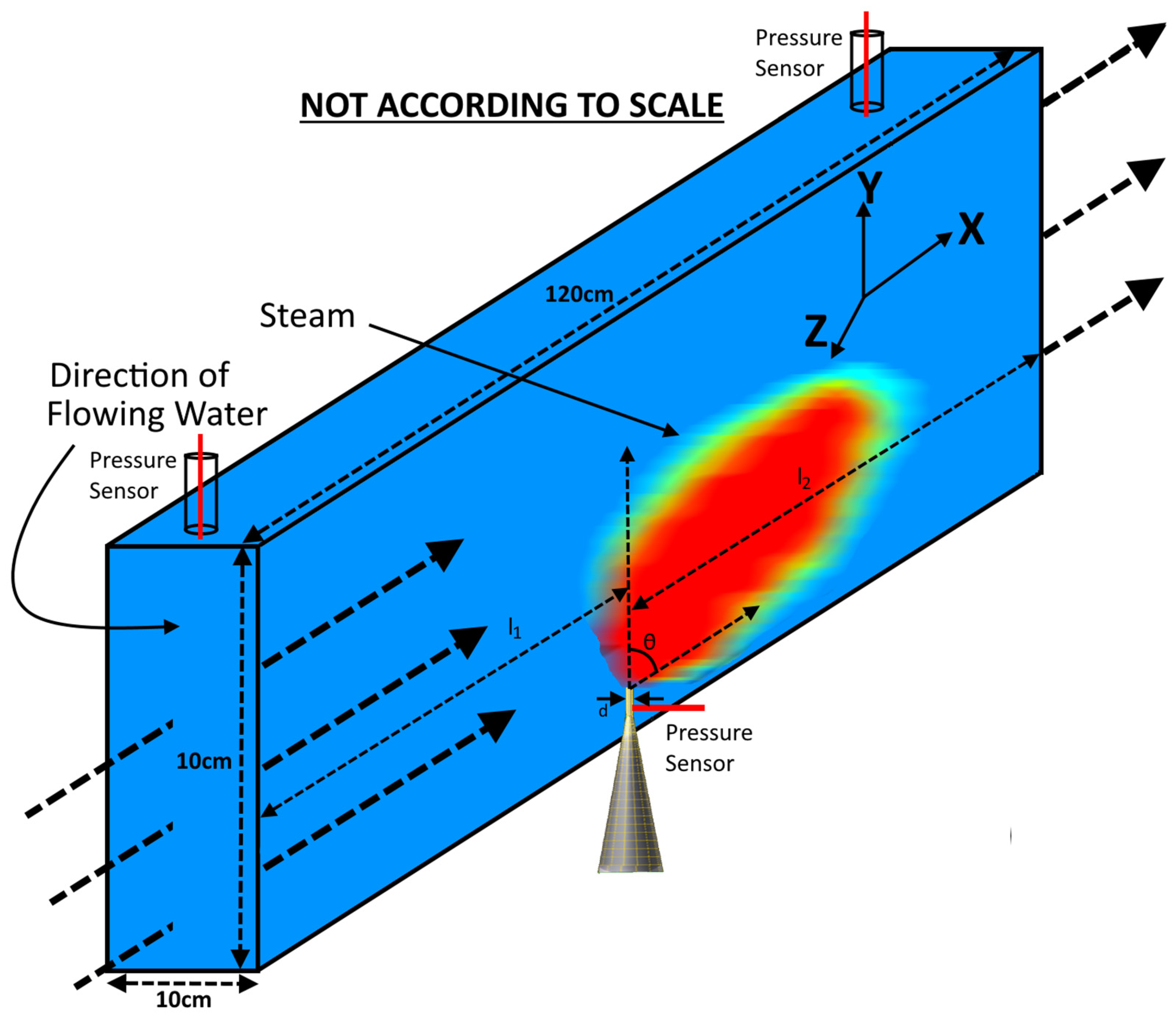
2. Experimental Setup
3. Results and Discussion
3.1. Hydrodynamics of the Supersonic Steam Jet into the Cross-Flowing Water
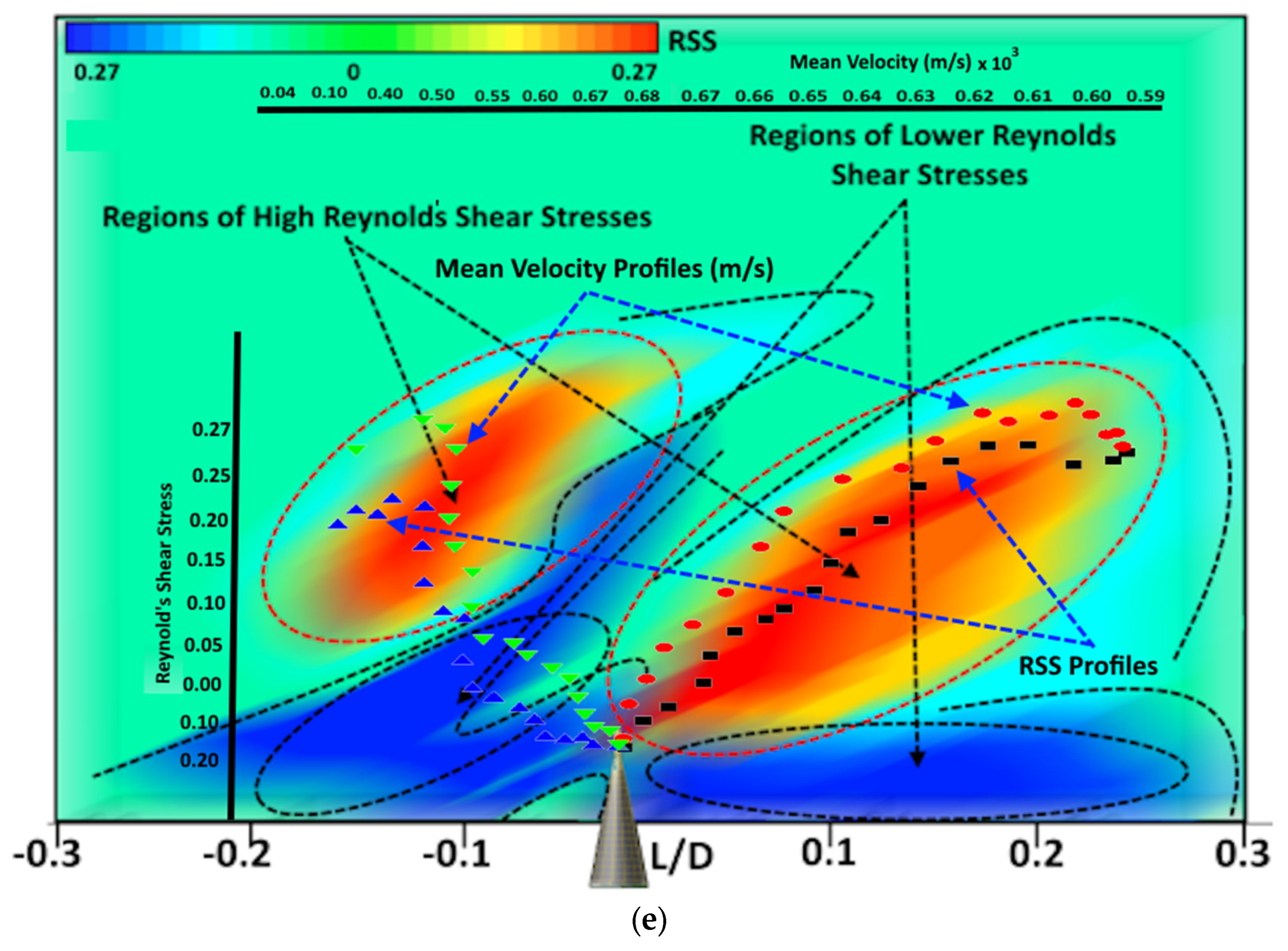
3.2. Shear Layer-Driven Instabilities
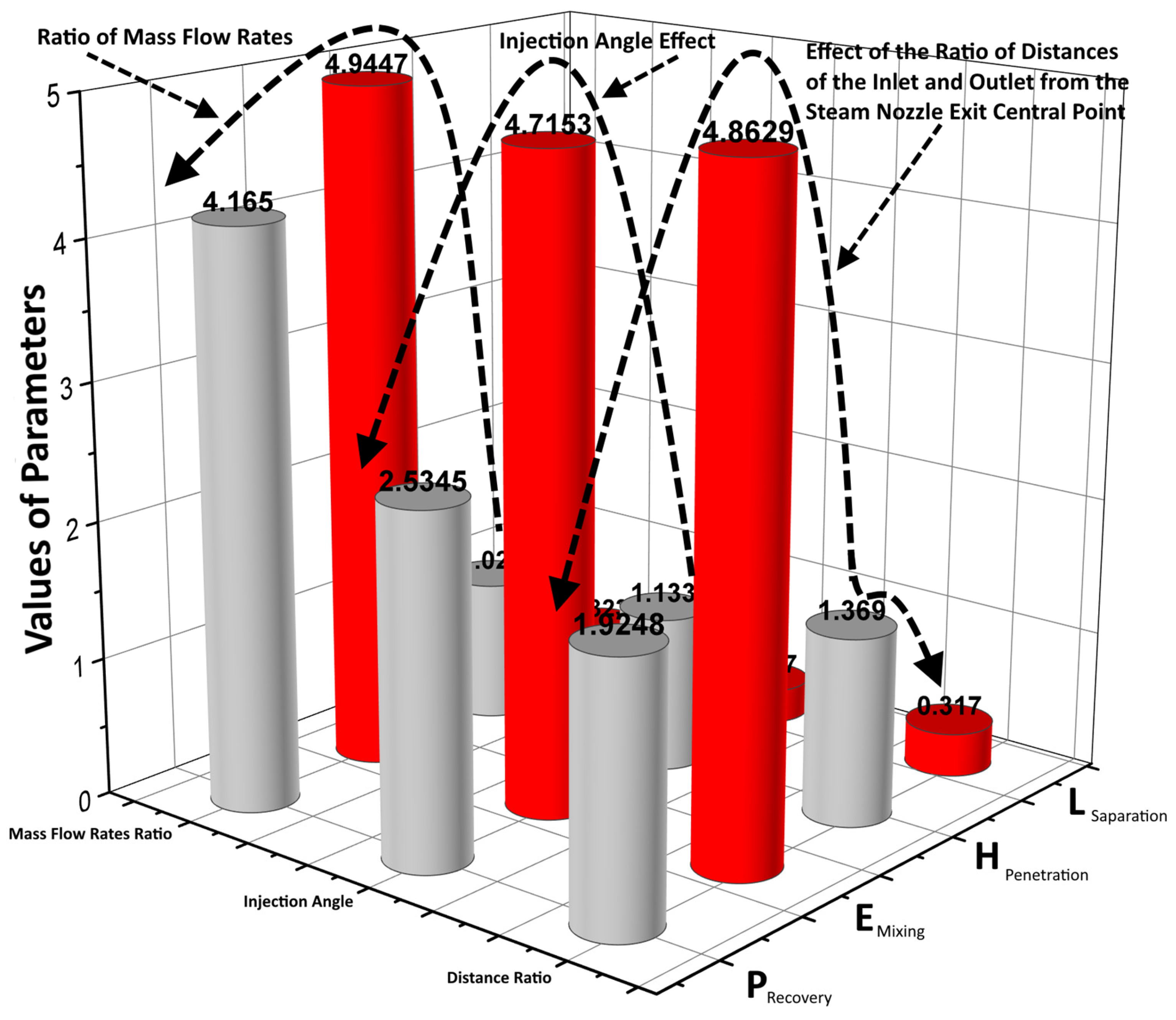
3.3. Effects of Operating Conditions on the Mixing
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cooper, H.B.H.; Tang, R.E.; Degling, D.E.; Ewan, T.K.; Ewan, S.M. Process and Apparatus for Carbon Capture and Elimination of Multi-Pollutants in Flue Gas from Hydrocarbon Fuel Sources and Recovery of Multiple by-Products. US7842264B2, 2 April 2008. Available online: https://patents.google.com/patent/US7842264B2/en (accessed on 1 July 2020).
- Ben-Yakar, A.; Mungal, M.G.; Hanson, R.K. Time evolution and mixing characteristics of hydrogen and ethylene transverse jets in supersonic crossflows. Phys. Fluids 2006, 18, 026101. [Google Scholar] [CrossRef]
- Gruber, M.R.; Nejad, A.S.; Chen, T.H.; Dutton, J.C. Mixing and Penetration Studies of Sonic Jets in a Mach 2 Freestream. J. Propuls. Power 1995, 11, 315–323. [Google Scholar] [CrossRef]
- Zukoski, E.E.; Spaid, F.W. Secondary injection of gases into a supersonic flow. AIAA J. 1964, 2, 1689–1696. [Google Scholar] [CrossRef]
- Zakkay, V.; Calarese, W.; Sakell, L. An experimental investigation of the interaction between a transverse sonic jet and a hypersonic stream. AIAA J. 1971, 9, 674–682. [Google Scholar] [CrossRef]
- Kaufman, L.G. Hypersonic flows past transverse jets. J. Spacecr. Rocket. 1967, 4, 1230–1235. [Google Scholar] [CrossRef]
- Vanierschot, M.; Persoons, T.; Bulck, E.V.D. A new method for annular jet control based on cross-flow injection. Phys. Fluids 2009, 21, 025103. [Google Scholar] [CrossRef]
- Sarno, R.L.; Franke, M.E. Suppression of flow-induced pressure oscillations in cavities. J. Aircr. 1994, 31, 90–96. [Google Scholar] [CrossRef]
- Ukeiley, L.S.; Ponton, M.K.; Seiner, J.M.; Jansen, B. Suppression of Pressure Loads in Cavity Flows. AIAA J. 2004, 42, 70–79. [Google Scholar] [CrossRef]
- Vakili, A.D.; Gauthier, C. Control of cavity flow by upstream mass-injection. J. Aircr. 1994, 31, 169–174. [Google Scholar] [CrossRef]
- Rowley, C.W.; Williams, D.R. Dynamics and control of high-reynolds-number flow over open cavities. Annu. Rev. Fluid Mech. 2006, 38, 251–276. [Google Scholar] [CrossRef]
- Cattafesta, L.; Alvi, F.; Williams, D.; Rowley, C. Review of Active Control of Flow-Induced Cavity Oscillations (Invited). In Proceedings of the 33rd AIAA Fluid Dynamics Conference and Exhibit, Orlando, FL, USA, 23–26 June 2003. [Google Scholar] [CrossRef]
- Larson, W.J.; Henry, G.N.; Humble, R.W. Space Propulsion Analysis and Design; McGraw-Hill: New York, NY, USA, 1995. [Google Scholar]
- Ko, H.; Yoon, W.-S. Performance Analysis of Secondary Gas Injection into a Conical Rocket Nozzle. J. Propuls. Power 2002, 18, 585–591. [Google Scholar] [CrossRef]
- Schramm, J.M.; Karl, S.; Hannemann, K.; Steelant, J. Steelant, Ground testing of the HyShot II scramjet configuration in HEG. In Proceedings of the 15th AIAA International Space Planes and Hypersonic Systems and Technologies Conference, Dayton, OH, USA, 28 April–1 May 2008. [Google Scholar] [CrossRef]
- Davidenko, D.; Gökalp, I.; Dufour, E.; Magre, P. Numerical simulation of hydrogen supersonic combustion and validation of computational approach. In Proceedings of the 12th AIAA International Space Planes and Hypersonic Systems and Technologies, Norfolk, VA, USA, 15–19 December 2003. [Google Scholar] [CrossRef]
- Gardner, A.D.; Hannemann, K.; Paull, A.; Steelant, J. Ground testing of the HyShot supersonic combustion flight experiment in HEG. In Shock Waves; Springer: Berlin/Heidelberg, Germany, 2005; pp. 329–334. [Google Scholar] [CrossRef]
- Furudate, M.; Lee, B.-J.; Jeung, I.-S. Computation of HyShot scramjet flows in the T4 experiments. In Proceedings of the 13th AIAA/CIRA International Space Planes and Hypersonic Systems and Technologies Conference, Capua, Italy, 16–20 May 2005; pp. 1419–1428. [Google Scholar] [CrossRef]
- Spaid, F.W. A Study of Secondary Injection of Gases into a Supersonic Flow—CaltechTHESIS, (n.d.). Available online: https://thesis.library.caltech.edu/4336/ (accessed on 1 July 2020).
- Cohen, L.S.; Coulter, L.J.; Egan, W.J. Penetration and mixing of multiple gas jets subjected to a cross flow. AIAA J. 1971, 9, 718–724. [Google Scholar] [CrossRef]
- Choo, Y.J.; Song, C.-H. PIV measurements of turbulent jet and pool mixing produced by a steam jet discharge in a subcooled water pool. Nucl. Eng. Des. 2010, 240, 2215–2224. [Google Scholar] [CrossRef]
- Abramovich, G.N.; Girshovich, T.A.; Krasheninnikov, S.I.; Sekundov, A.N.; Smirnova, I.P. The theory of turbulent jets. Mosc. Izd. Nauka 1984. [Google Scholar]
- Orth, R.C.; Funk, J.A. An experimental and comparative study of jet penetration in supersonic flow. J. Spacecr. Rocket. 1967, 4, 1236–1242. [Google Scholar] [CrossRef]
- Khan, A.; Sanaullah, K.; Takriff, M.S.; Hussain, A.; Shah, A.; Chughtai, I.R. Void fraction of supersonic steam jet in subcooled water. Flow Meas. Instrum. 2016, 47, 35–44. [Google Scholar] [CrossRef]
- Khan, A.; Sanaullah, K.; Takriff, M.S.; Zen, H.; Rigit, A.R.H.; Shah, A.; Chughtai, I.R. Numerical and experimental investigations on the physical characteristics of supersonic steam jet induced hydrodynamic instabilities. Asia-Pac. J. Chem. Eng. 2016, 11, 271–283. [Google Scholar] [CrossRef]
- Santiago, J.G.; Dutton, J.C. Velocity Measurements of a Jet Injected into a Supersonic Crossflow. J. Propuls. Power 1997, 13, 264–273. [Google Scholar] [CrossRef]
- Kawai, S.; Lele, S. Large-eddy simulation of jet mixing in a supersonic turbulent crossflow. In Proceedings of the 19th AIAA Computational Fluid Dynamics Conference, San Antonio, TX, USA, 22–25 June 2009. [Google Scholar] [CrossRef]
- Viti, V.; Neel, R.; Schetz, J.A. Detailed flow physics of the supersonic jet interaction flow field. Phys. Fluids 2009, 21, 046101. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, H.; Jin, X. Efficient image decolorization with a multimodal contrast-preserving measure. Comput. Graph. 2018, 70, 251–260. [Google Scholar] [CrossRef]
- Matlab, Find Edges in Intensity Image—MATLAB Edge, (n.d.). Available online: https://www.mathworks.com/help/images/ref/edge.html (accessed on 8 July 2020).
- Kelso, R.M.; Lim, T.T.; Perry, A.E. An experimental study of round jets in cross-flow. J. Fluid Mech. 1996, 306, 111–144. [Google Scholar] [CrossRef]
- Perry, A.E.; Lim, T.T. Coherent structures in coflowing jets and wakes. J. Fluid Mech. 1978, 88, 451–463. [Google Scholar] [CrossRef]
- Moussa, Z.M.; Trischka, J.W.; Eskinazi, S. The near field in the mixing of a round jet with a cross-stream. J. Fluid Mech. 1977, 80, 49–80. [Google Scholar] [CrossRef]
- Andreopoulos, J. On the structure of jets in a crossflow. J. Fluid Mech. 1985, 157, 163–197. [Google Scholar] [CrossRef]
- Sykes, R.I.; Lewellen, W.S.; Parker, S.F. On the vorticity dynamics of a turbulent jet in a crossflow. J. Fluid Mech. 1986, 168, 393–413. [Google Scholar] [CrossRef]
- Huang, W.; Yang, J.; Yan, L. Multi-objective design optimization of the transverse gaseous jet in supersonic flows. Acta Astronaut. 2014, 93, 13–22. [Google Scholar] [CrossRef]
- Huang, W.; Liu, W.-D.; Li, S.-B.; Xia, Z.-X.; Liu, J.; Wang, Z.-G. Influences of the turbulence model and the slot width on the transverse slot injection flow field in supersonic flows. Acta Astronaut. 2012, 73, 1–9. [Google Scholar] [CrossRef]
- Huang, W.; Li, X.S.; Wu, X.Y.; Wang, Z.G. Configuration Effect Analysis of Scramjet Combustor Based on the Integral Balanceable Method. J. Astronaut. 2009, 30, 282–286. Available online: http://en.cnki.com.cn/Article_en/CJFDTotal-YHXB200901049.htm (accessed on 1 July 2020).



| Serial No. | Experimental Phases | Steam Inlet Pressure (Bars) | Water Volumetric Flow Rate (m3/s) | Mach Number |
|---|---|---|---|---|
| 1 | 1 | 3.0 | 1.0 | 2 |
| 2 | 2 | 3.0 | 1.5 | 2 |
| 3 | 3 | 3.0 | 2.0 | 2 |
| 4 | 4 | 3.0 | 2.5 | 2 |
| 5 | 5 | 3.0 | 3.0 | 2 |
| Experimental Phases | Parameter | Values |
|---|---|---|
| 1 | Ratio of Volumetric flow rates of Steam and Water (Fsteam/Fwater) | 2.46 |
| 2 | 2.13 | |
| 3 | 1.91 | |
| 4 | 1.74 | |
| 5 | 1.60 | |
| 6 | Angle of steam injection (θ) | 90 |
| 7 | 88 | |
| 8 | 86 | |
| 9 | 84 | |
| 10 | 82 | |
| 11 | Ratio of distances from the inlet to the steam nozzle exit/the outlet to the steam nozzle exit (l1/l2) | 1 |
| 12 | 1.2 | |
| 13 | 1.3 | |
| 14 | 1.4 | |
| 15 | 1.5 |
| Experimental Phases | Pressure Recovery (P_recovery) | Mixing Efficiency (E_mixing) | Penetration Height (H_penetration) = h/D | Separation Length (L_saparation) = L/D |
|---|---|---|---|---|
| 1 | 77.38% | 98.12% | 27.2 | 5.3 |
| 2 | 79.12% | 98.68% | 23.7 | 5.9 |
| 3 | 84.19% | 99.12% | 22.4 | 6.4 |
| 4 | 86.48% | 99.24% | 17.9 | 6.9 |
| 5 | 89.33% | 99.31% | 11.7 | 7.8 |
| 6 | 56.28% | 94.58% | 26.8 | 5.7 |
| 7 | 54.21% | 94.47% | 24.8 | 5.4 |
| 8 | 51.19% | 94.31% | 21.9 | 5.0 |
| 9 | 47.33% | 94.28% | 20.1 | 4.5 |
| 10 | 44.44% | 93.89% | 19.7 | 4.1 |
| 11 | 43.18% | 97.33% | 26.7 | 5.4 |
| 12 | 40.79% | 97.31% | 26.9 | 5.9 |
| 13 | 39.64% | 97.27% | 27.4 | 6.4 |
| 14 | 36.66% | 97.21% | 27.7 | 6.8 |
| 15 | 32.21% | 97.17% | 28.2 | 7.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghazwani, H.A.; Sanaullah, K.; Khan, A. Hydrodynamics of Supersonic Steam Jets Injected into Cross-Flowing Water. Fluids 2023, 8, 250. https://doi.org/10.3390/fluids8090250
Ghazwani HA, Sanaullah K, Khan A. Hydrodynamics of Supersonic Steam Jets Injected into Cross-Flowing Water. Fluids. 2023; 8(9):250. https://doi.org/10.3390/fluids8090250
Chicago/Turabian StyleGhazwani, Hassan Ali, Khairuddin Sanaullah, and Afrasyab Khan. 2023. "Hydrodynamics of Supersonic Steam Jets Injected into Cross-Flowing Water" Fluids 8, no. 9: 250. https://doi.org/10.3390/fluids8090250
APA StyleGhazwani, H. A., Sanaullah, K., & Khan, A. (2023). Hydrodynamics of Supersonic Steam Jets Injected into Cross-Flowing Water. Fluids, 8(9), 250. https://doi.org/10.3390/fluids8090250








