A CFD Comparative Study of Bubbling Fluidized Bed Behavior with Thermal Effects Using the Open-Source Platforms MFiX and OpenFOAM
Abstract
:1. Introduction
2. Computational Model
2.1. Continuity Equations
2.2. Momentum Balance
2.3. Granular Rheology
2.4. Internal Energy Balance
2.5. Numerical Method
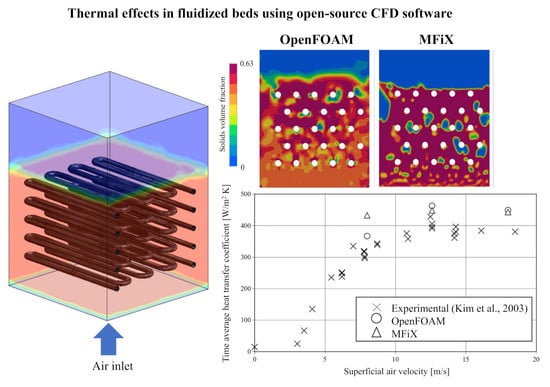
3. Results and Discussion
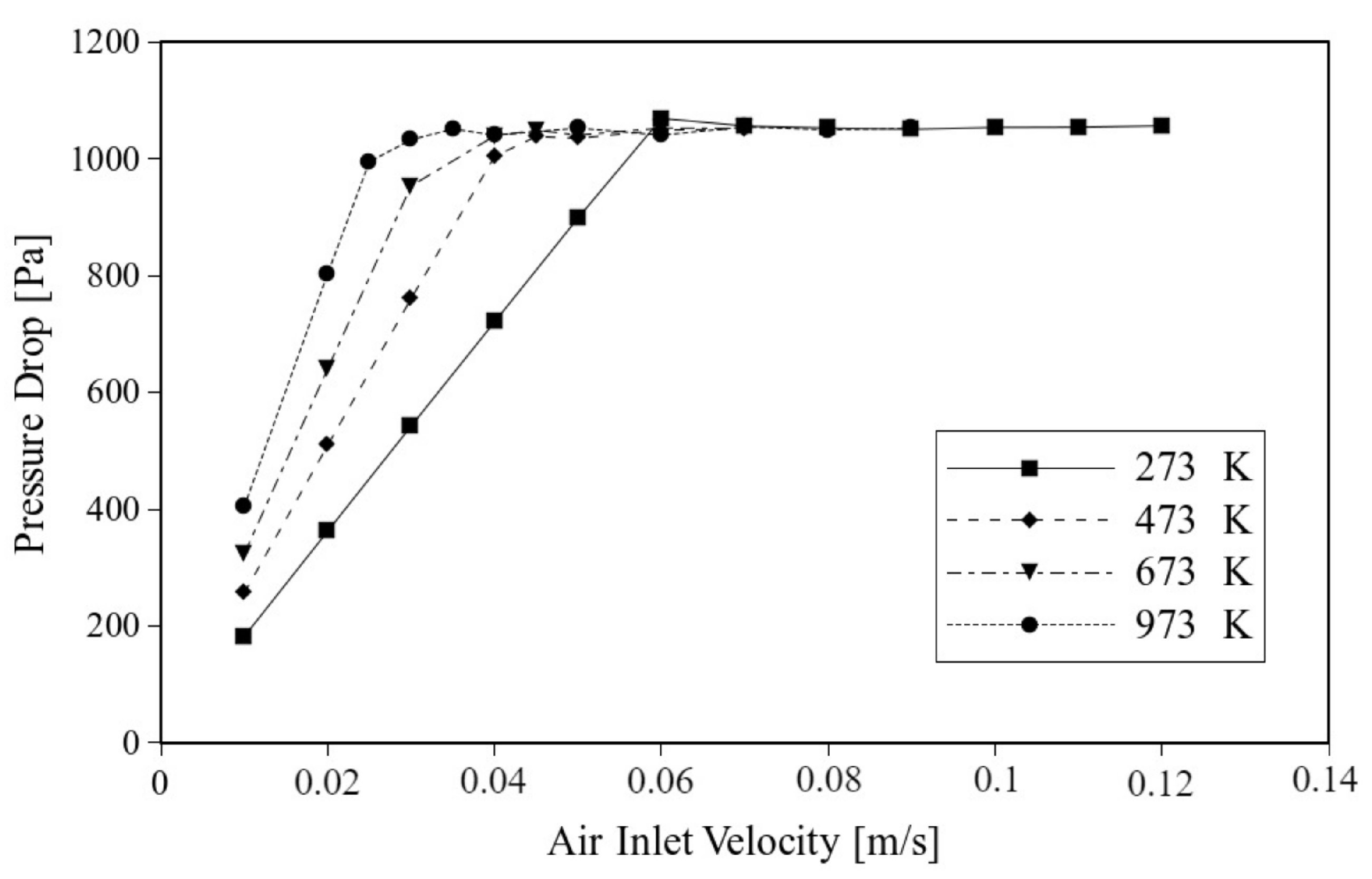
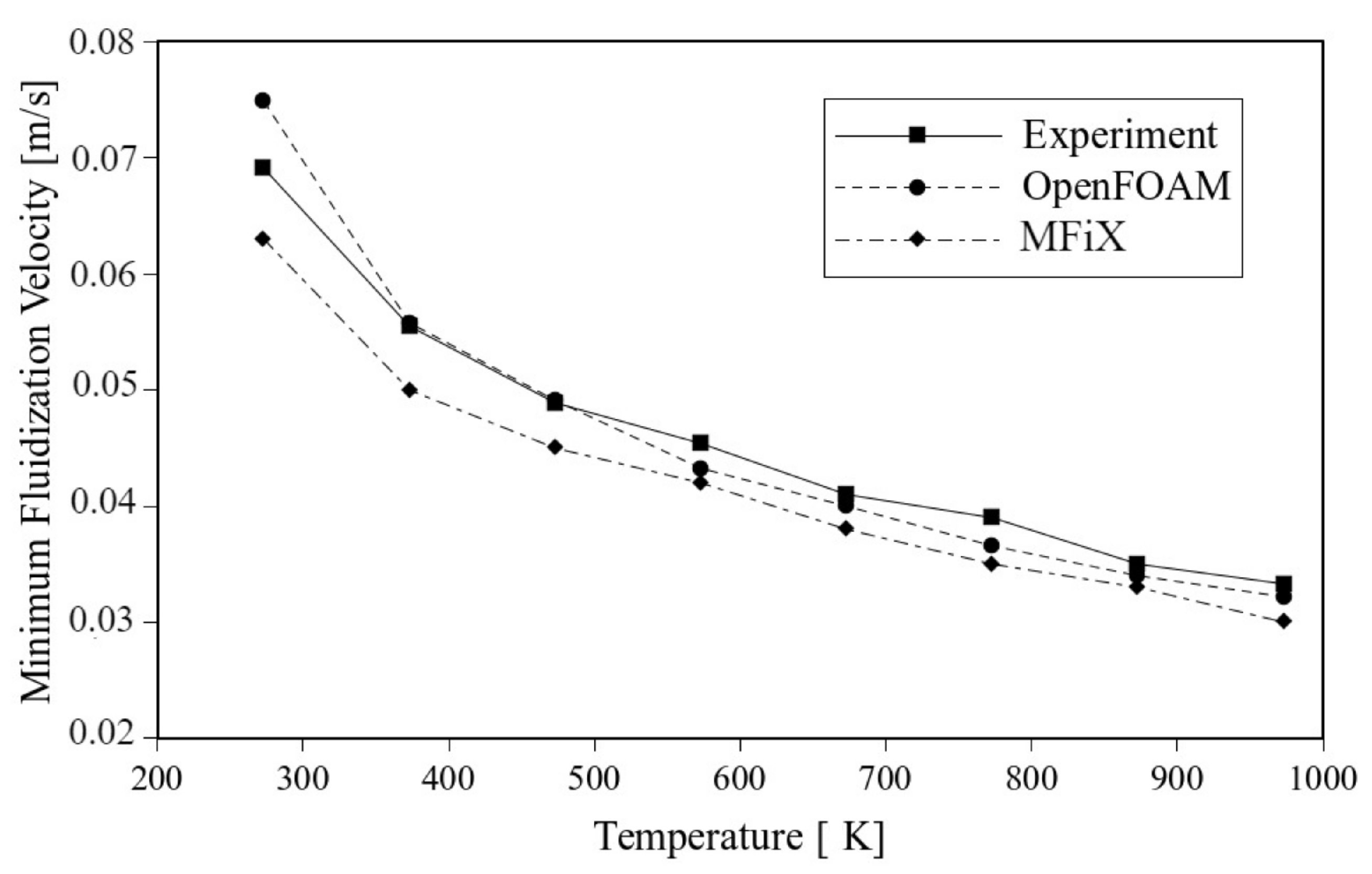
3.1. Test 1: Minimum Fluidization Velocity
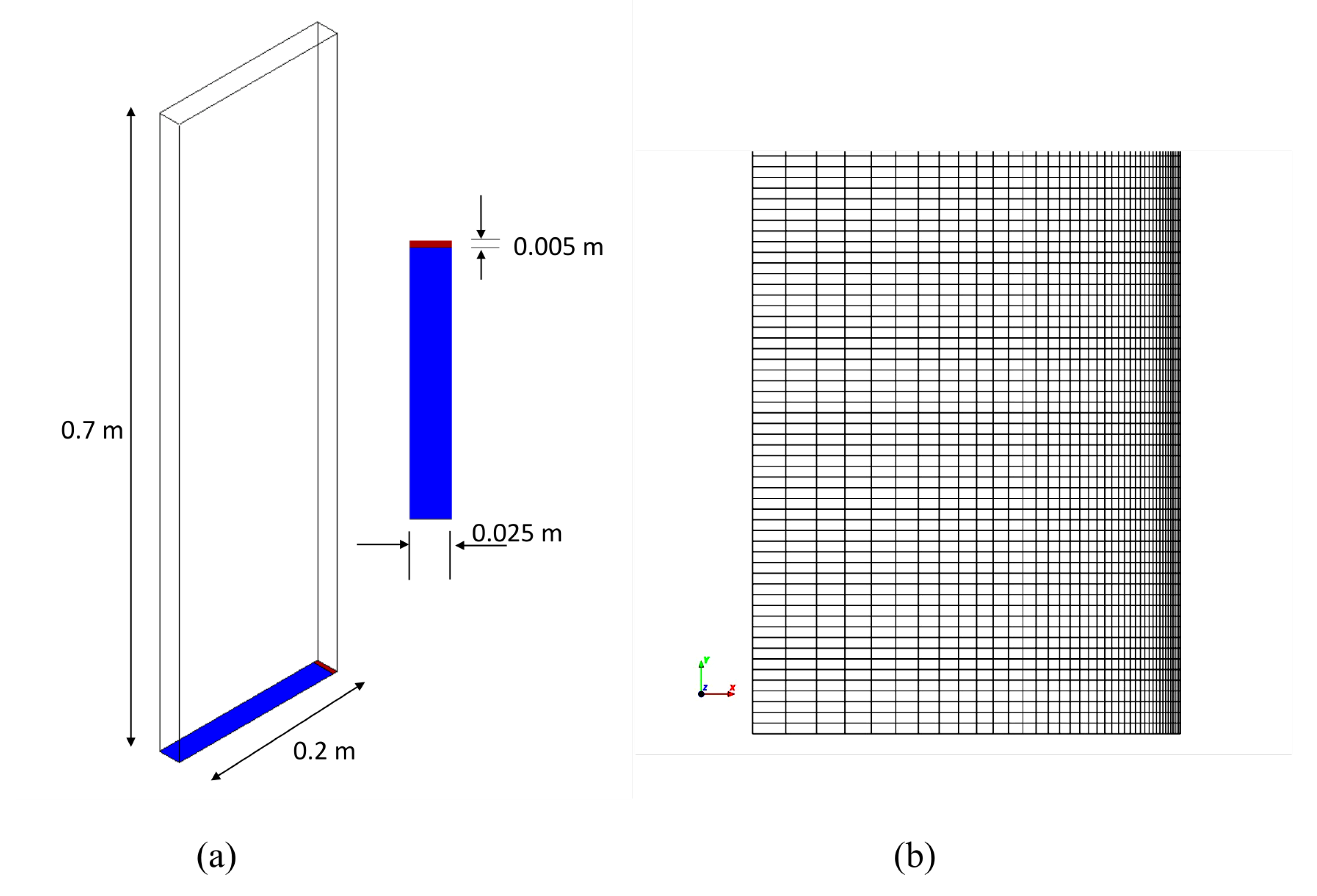
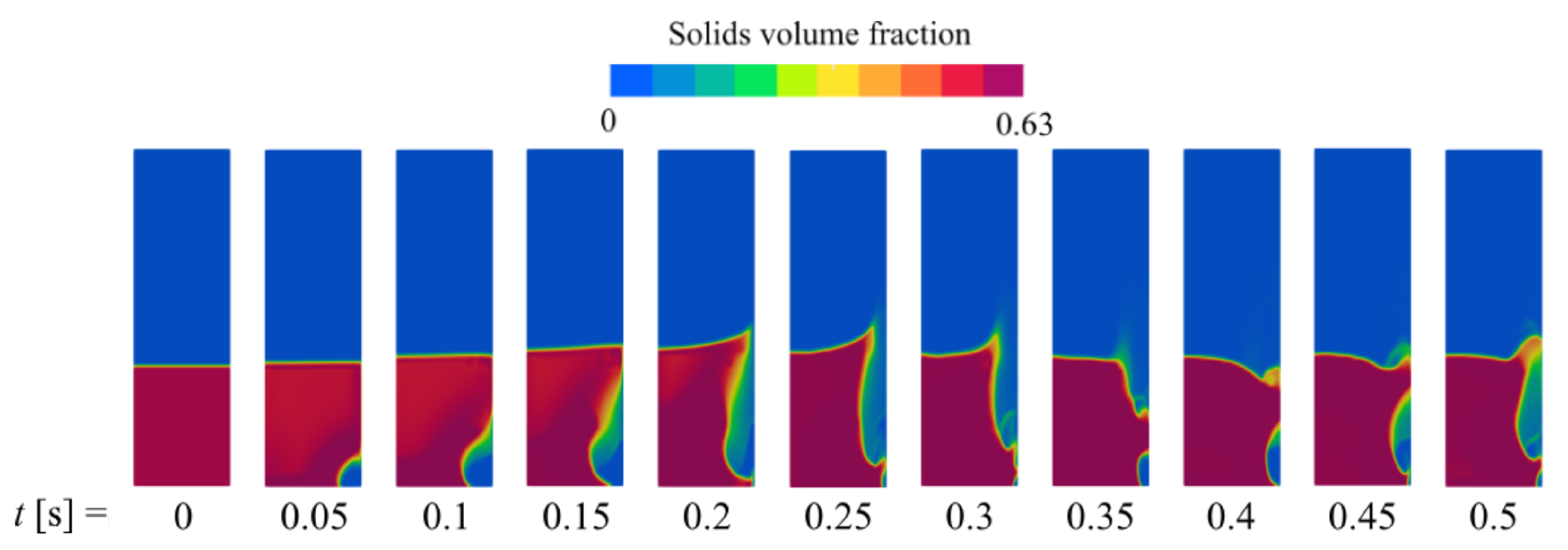
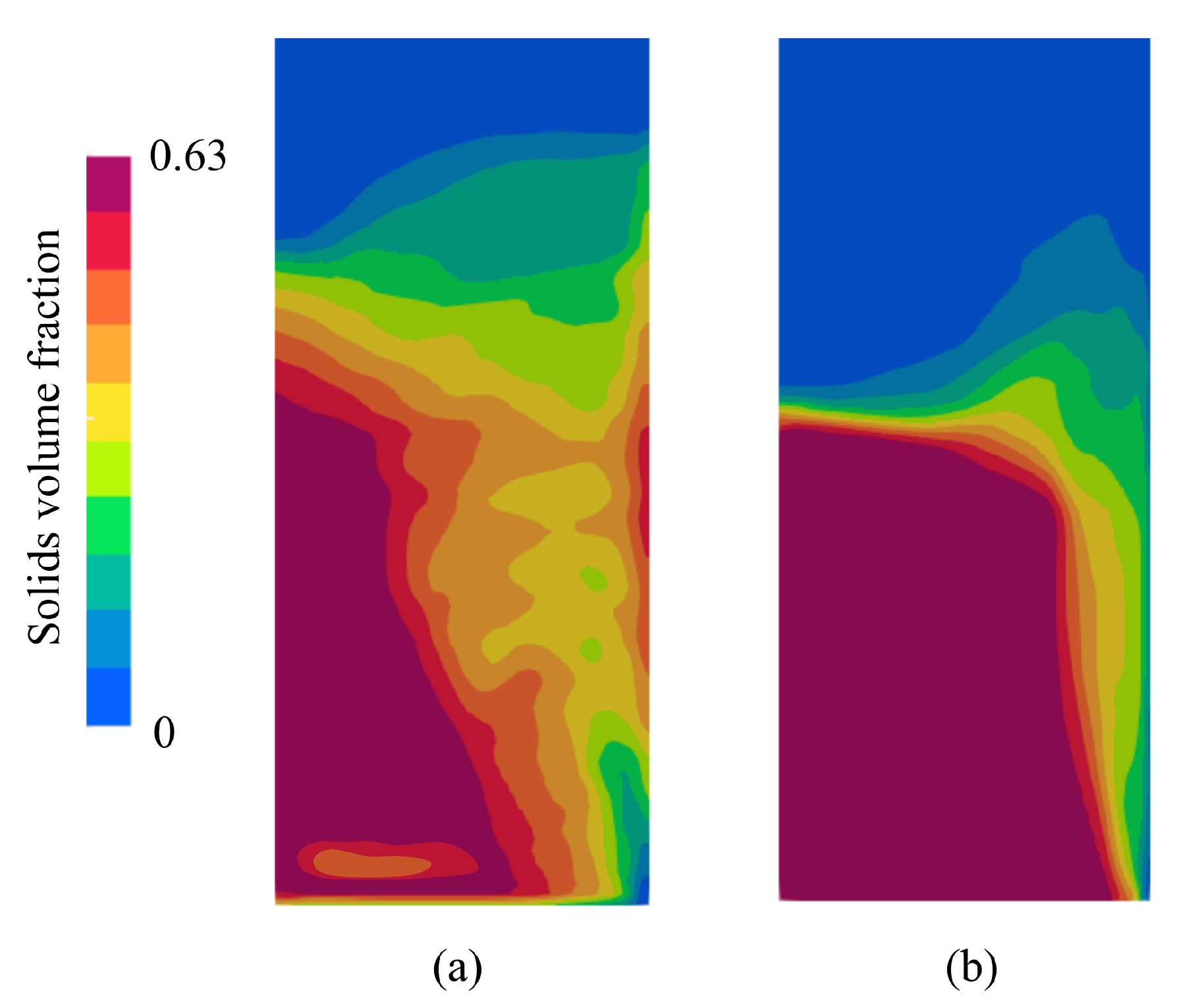
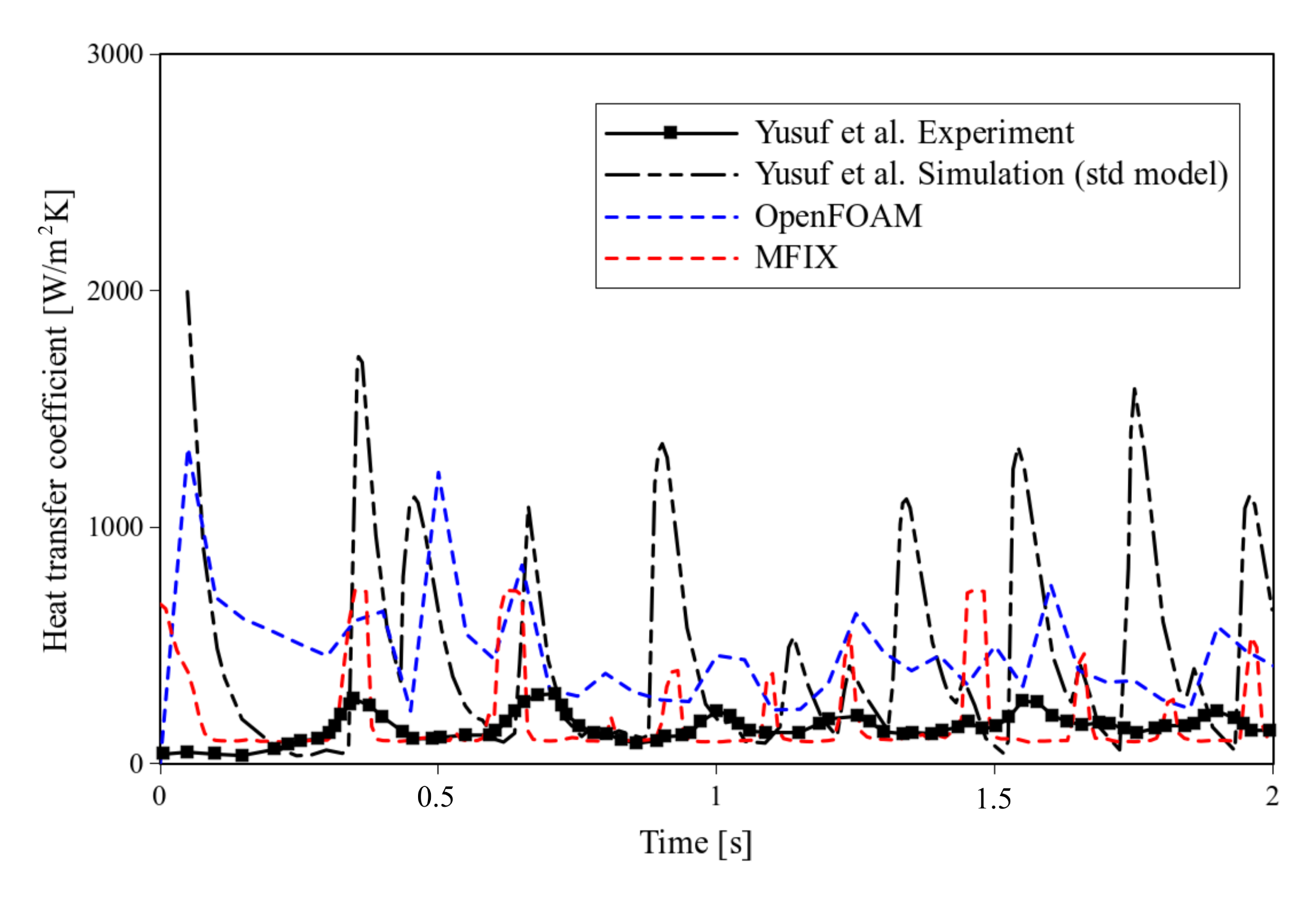
3.2. Test 2: Heat Transfer from a Vertical Wall
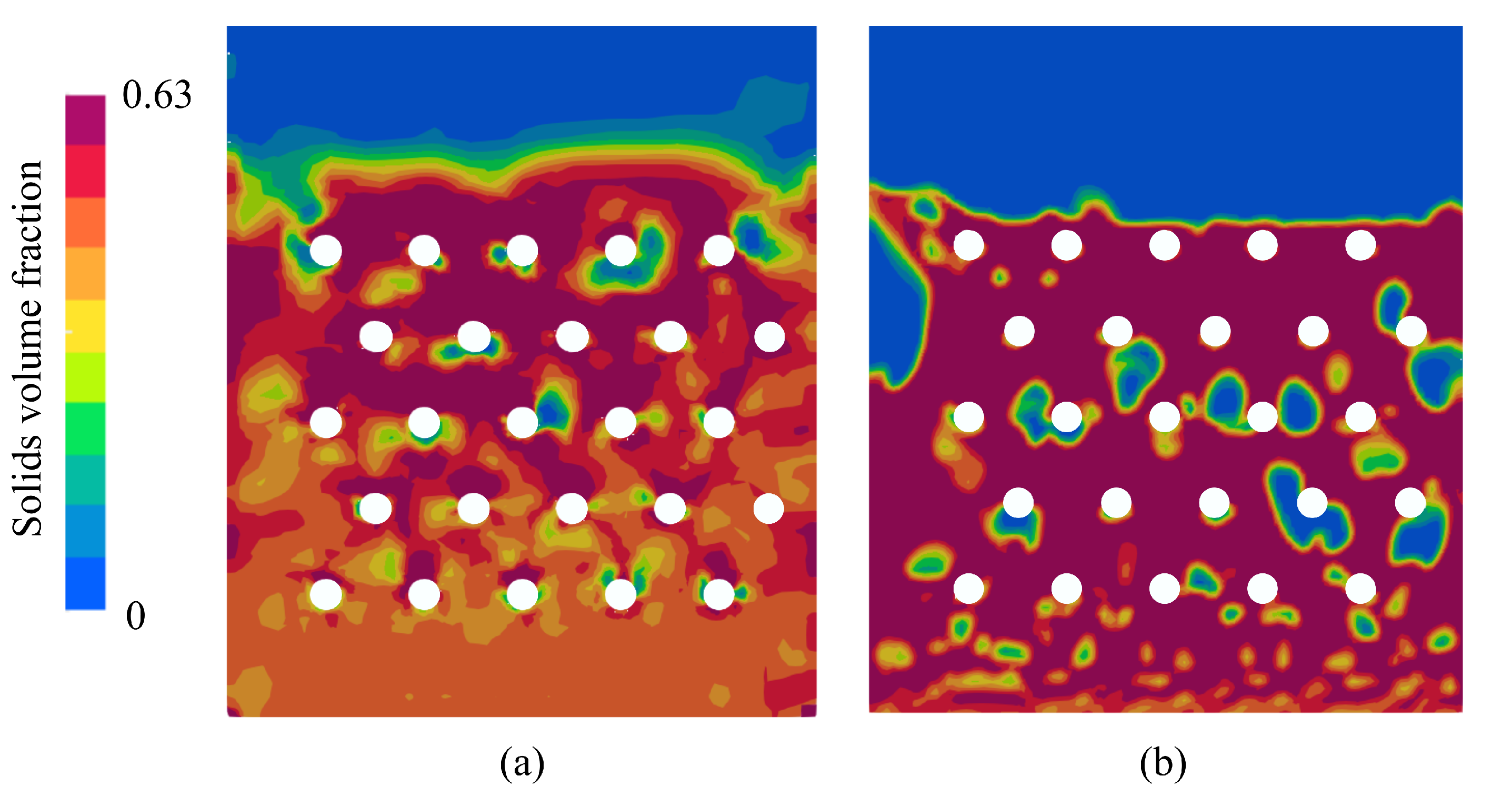
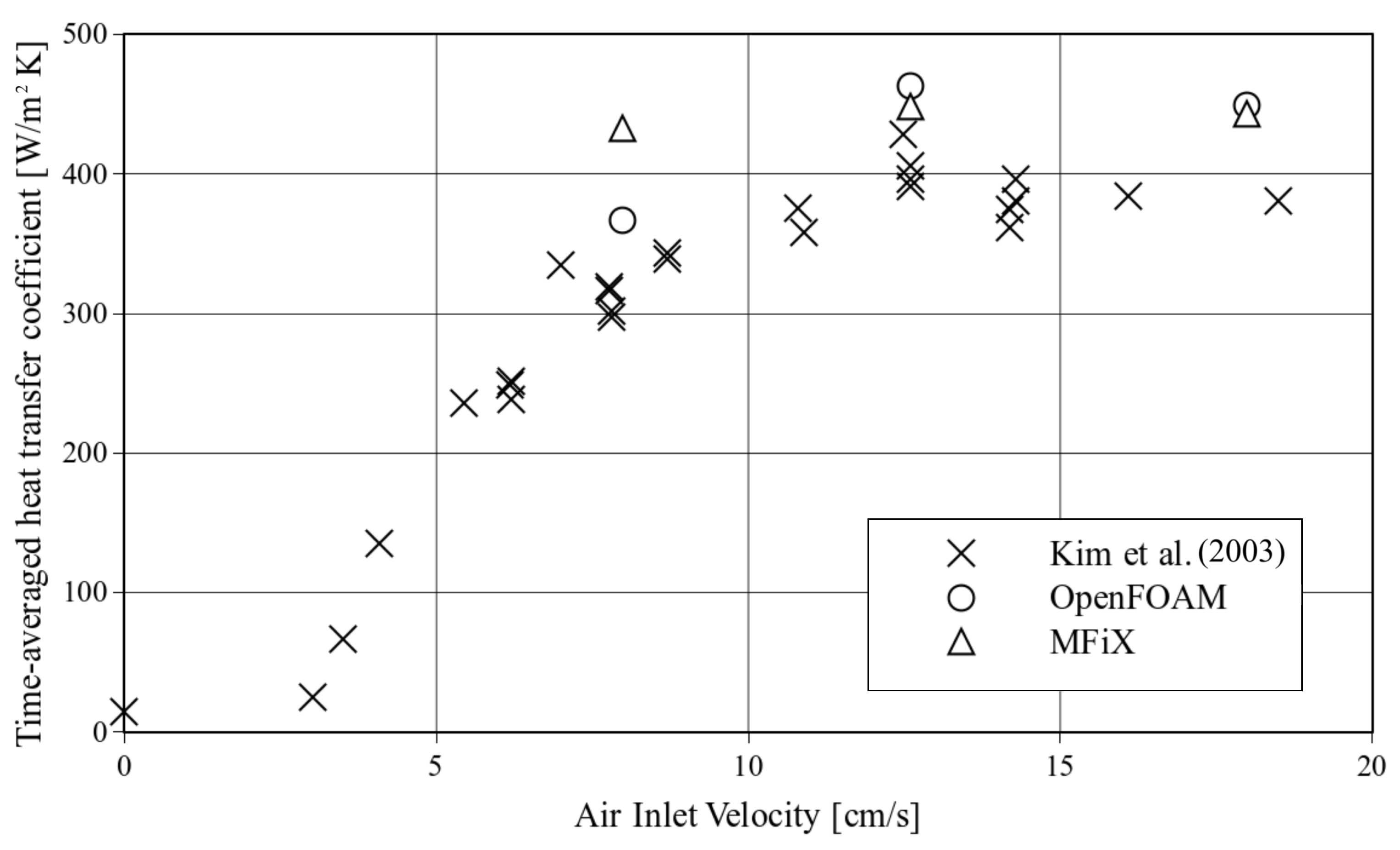
3.3. Test 3: Heat Transfer from Submerged Tubes
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Description |
| Phase density [Kg/m] | |
| Phase volume fraction | |
| Maximum packing | |
| Minimum volume fraction for frictional effects | |
| Phase velocity [m/s] | |
| p | Pressure [Pa] |
| Shear stress tensor [N/m] | |
| Acceleration of gravity [m/s] | |
| Drag coefficient [Kg/m3s] | |
| Dynamic viscosity [Pa s] | |
| Bulk viscosity [Pa s] | |
| Particles diameter [m] | |
| Sphericity factor | |
| Particle Reynolds number () | |
| Granular temperature [m/s] | |
| Granular conductivity [Kg/m s] | |
| Dissipation of granular energy due to particle collisions [Kg/m s] | |
| Dissipation of granular energy due to viscous damping [Kg/m s] | |
| Production of granular energy due to slip between phases [Kg/m s] | |
| e | Restitution coefficient |
| Radial distribution | |
| Frictional pressure coefficient () [Pa] | |
| Frictional exponent () | |
| Second deviatoric of the stress tensor | |
| Angle of internal friction | |
| Minimum fluidization velocity [m/s] | |
| H | Phase enthalpy [J/Kg] |
| Heat transfer coefficient between phases [W/m K] | |
| Thermal conductivity of the material [W/m K] | |
| Phase effective thermal conductivity [W/m K] | |
| Bulk thermal conductivity [W/m K] | |
| Prandtl number () | |
| s | Subindex for solid phase |
| g | Subindex for gas phase |
References
- Wu, S.; Baeyens, J. Effect of operating temperature on minimum fluidization velocity. Powder Technol. 1991, 67, 217–220. [Google Scholar] [CrossRef]
- Shao, Y.; Gu, J.; Zhong, W.; Yu, A. Determination of minimum fluidization velocity in fluidized bed at elevated pressures and temperatures using CFD simulations. Powder Technol. 2019, 350, 81–90. [Google Scholar] [CrossRef]
- Kozanoglu, B.; Solis, J.; De Lucio, L.; Tlapaltotoli, V.; Guerrero-Beltrán, J.; Welti-Chanes, J. Hydrodynamics of reduced pressure fluidization employing particles with variable density. Dry. Technol. 2012, 30, 342–350. [Google Scholar] [CrossRef]
- Eder, C.; Hofer, G.; Proll, T. Wall-to-Bed Heat Transfer in Bubbling Fluidized Bed Reactors with an Immersed Heat Exchanger and Continuous Particle Exchange. Ind. Eng. Chem. Res. 2021, 60, 7417–7428. [Google Scholar] [CrossRef]
- Kim, S.W.; Ahn, J.Y.; Kim, S.D.; Lee, D.H. Heat transfer and bubble characteristics in a fluidized bed with immersed horizontal tube bundle. Int. J. Heat Mass Transf. 2003, 46, 399–409. [Google Scholar] [CrossRef]
- Pattipati, R.R.; Wen, C. Minimum fluidization velocity at high temperatures. Ind. Eng. Chem. Process. Des. Dev. 1981, 20, 705–707. [Google Scholar] [CrossRef]
- Wen, C.; Yu, Y. A generalized method for predicting the minimum fluidization velocity. AIChE J. 1966, 12, 610–612. [Google Scholar] [CrossRef]
- Gosavi, S.; Kulkarni, N.; Mathpati, C.; Mandal, D. CFD modeling to determine the minimum fluidization velocity of particles in gas-solid fluidized bed at different temperatures. Powder Technol. 2018, 327, 109–119. [Google Scholar] [CrossRef]
- Geldart, D. Types of gas fluidization. Powder Technol. 1973, 7, 285–292. [Google Scholar] [CrossRef]
- Soria, J.M.; Zambon, M.T.; Mazza, G.D. Computational fluid dynamics simulations of heat transfer between the dense-phase of a high-temperature fluidized bed and an immersed surface. AIChE J. 2012, 58, 412–426. [Google Scholar] [CrossRef]
- Mazza, G.; Mariani, N.; Barreto, G. Evaluation of overall heat transfer rates between bubbling fluidized beds and immersed surfaces. Chem. Eng. Commun. 1997, 162, 125–149. [Google Scholar] [CrossRef]
- Mazza, G.; Mariani, N.; Barreto, G. Evaluation of conductive heat transfer mechanisms between an immersed surface and the adjacent layer of particles in bubbling fluidized beds. Chem. Eng. Commun. 1997, 162, 93–123. [Google Scholar] [CrossRef]
- Ali, O.S.A.E.K. Hydrodynamic and Heat Transfer Simulation of Fluidized Bed Using CFD. In Nuclear Reactor Thermal Hydraulics and Other Applications; IntechOpen: London, UK, 2013; pp. 155–192. [Google Scholar]
- Reyes-Urrutia, A.; Benoit, H.; Zambon, M.; Gauthier, D.; Flamant, G.; Mazza, G. Simulation of the behavior of a dense SiC particle suspension as an energy transporting vector using computational fluid dynamics (CFD). Chem. Eng. Res. Des. 2016, 106, 141–154. [Google Scholar] [CrossRef]
- Zehner, P.; Schlünder, E. Thermal conductivity of packings at moderate temperatures. Chem. Ing. Tech. 1970, 42, 933. [Google Scholar] [CrossRef]
- Schmidt, A.; Renz, U. Numerical prediction of heat transfer in fluidized beds by a kinetic theory of granular flows. Int. J. Therm. Sci. 2000, 39, 871–885. [Google Scholar] [CrossRef]
- Syamlal, M.; Gidaspow, D. Hydrodynamics of fluidization: Prediction of wall to bed heat transfer coefficients. AIChE J. 1985, 31, 127–135. [Google Scholar] [CrossRef]
- Kuipers, J.; Prins, W.; Van Swaaij, W.P.M. Numerical calculation of wall-to-bed heat-transfer coefficients in gas-fluidized beds. AIChE J. 1992, 38, 1079–1091. [Google Scholar] [CrossRef] [Green Version]
- Syamlal, M.; Rogers, W.; OBrien, T.J. MFIX Documentation Theory Guide; Technical Report; USDOE Morgantown Energy Technology Center: Morgantown, WV, USA, 1993.
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Physics 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Musser, J.M.; Carney, J.E. Theoretical Review of the MFIX Fluid and Two-Fluid Models; DOE/NETL-2020/2100 Technical Report Series; National Energy Technology Laboratory (NETL): Pittsburgh, PA, USA; Morgantown, WV, USA; Albany, OR, USA, 2020.
- Passalacqua, A.; Fox, R.O. Implementation of an iterative solution procedure for multi-fluid gas–particle flow models on unstructured grids. Powder Technol. 2011, 213, 174–187. [Google Scholar] [CrossRef]
- Venier, C.M.; Damian, S.M.; Nigro, N.M. Numerical aspects of Eulerian gas–particles flow formulations. Comput. Fluids 2016, 133, 151–169. [Google Scholar] [CrossRef]
- Venier, C.M. Resolución Computacional de Flujos Multifásicos Granulares por Métodos Eulerianos. Ph.D. Thesis, Universidad Nacional del Litoral, Santa Fe, Argentina, 2018. [Google Scholar]
- Oliveira, P.J.; Issa, R.I. On the numerical treatment of interphase forces in two-phase flow. ASME-PUBLICATIONS-FED 1994, 185, 131. [Google Scholar]
- Ishii, M. Thermo-fluid dynamic theory of two-phase flow. NASA Sti/Recon Tech. Rep. A 1975, 75, 29657. [Google Scholar]
- Lun, C.; Savage, S.; Jeffrey, D.; Chepurniy, N. Kinetic theories for granular flow: Inelastic particles in Couette flow and slightly inelastic particles in a general flowfield. J. Fluid Mech. 1984, 140, 223–256. [Google Scholar] [CrossRef]
- Gidaspow, D. Multiphase Flow and Fluidization: Continuum and Kinetic Theory Descriptions; Academic Press: San Diego, CA, USA, 1994. [Google Scholar]
- Sinclair, J.; Jackson, R. Gas-particle flow in a vertical pipe with particle-particle interactions. AIChE J. 1989, 35, 1473–1486. [Google Scholar] [CrossRef]
- Johnson, P.C.; Jackson, R. Frictional–collisional constitutive relations for granular materials, with application to plane shearing. J. Fluid Mech. 1987, 176, 67–93. [Google Scholar] [CrossRef]
- Schaeffer, D.G. Instability in the evolution equations describing incompressible granular flow. J. Differ. Equations 1987, 66, 19–50. [Google Scholar] [CrossRef] [Green Version]
- Syamlal, M.; Rogers, W.; O’Brien, T.J. MFIX documentation: Theory guide. In National Energy Technology Laboratory, Department of Energy, Technical Note DOE/METC-95/1013 and NTIS/DE95000031; USDOE Morgantown Energy Technology Center: Morgantown, WV, USA, 1993. [Google Scholar]
- Bauer, R.; Schlünder, E. Effective radial thermal conductivity of packings in gas flow. Part II Thermal conductivity of the packing fraction without gas flow. Int. Chem. Eng. 1978, 18, 189–204. [Google Scholar]
- Gunn, D. Transfer of heat or mass to particles in fixed and fluidised beds. Int. J. Heat Mass Transf. 1978, 21, 467–476. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Peric, M. Computational Methods for Fluid Dynamics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Jasak, H. Error Analysis and Estimation for the Finite Volume Method with Applications to Fluid Flows. Ph.D. Thesis, Imperial College of Science Technology and Medicine, London, UK, 1996. [Google Scholar]
- Patankar, S.V.; Spalding, D.B. A calculation procedure for heat, mass and momentum transfer in three-dimensional parabolic flows. Int. J. Heat Mass Transf. 1972, 15, 1787–1806. [Google Scholar] [CrossRef]
- Issa, R.I. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Spalding, D. Numerical computation of multi-phase fluid flow and heat transfer. Recent Adv. Numer. Methods Fluids 1980, 1, 139–167. [Google Scholar]
- Oliveira, P.J.; Issa, R.I. Numerical aspects of an algorithm for the Eulerian simulation of two-phase flows. Int. J. Numer. Methods Fluids 2003, 43, 1177–1198. [Google Scholar] [CrossRef]
- Subramani, H.J.; Balaiyya, M.M.; Miranda, L.R. Minimum fluidization velocity at elevated temperatures for Geldart’s group-B powders. Exp. Therm. Fluid Sci. 2007, 32, 166–173. [Google Scholar] [CrossRef]
- Yusuf, R.; Halvorsen, B.; Melaaen, M.C. An experimental and computational study of wall to bed heat transfer in a bubbling gas–solid fluidized bed. Int. J. Multiph. Flow 2012, 42, 9–23. [Google Scholar] [CrossRef]


| Experiment | Particles | Density [Kg/m] | Diameter [μm] | H0 bed [m] |
|---|---|---|---|---|
| Subramani et al. [41] | Ilmenite | 4690 | 200 | 0.04 |
| Yusuf et al. [42] | Glass | 2485 | 491 | 0.25 |
| Kim et al. [5] | Silica sand | 2582 | 240 | 0.37 |
| Setup | Description |
|---|---|
| Total simulated time | 10 s (Test 1), 2 s (Test 2), 10 s (Test 3) |
| Maximum packing | 0.63 |
| Minimum fraction for frictional effects | 0.61 |
| Restitution coefficient | 0.9 |
| Maximum residuals | 1 × 10 |
| Time step | 1 × 10 s |
| Time discretization | Second-order implicit |
| Advection schemes | TVD |
| Mesh Number | Stretch Value | Heat Transfer Coefficient [W/m K] |
|---|---|---|
| mesh 1 | 5 | 50.1 |
| mesh 2 | 1 | 92.3 |
| mesh 3 | 0.5 | 165.4 |
| mesh 4 | 0.05 | 166.1 |
| Exp. (Yusuf et al. [42]) | CFD (Yusuf et al. [42]) | CFD (OpenFOAM) | CFD (MFiX) |
|---|---|---|---|
| 169.9 | 550.4 | 398.5 | 165.4 |
| Mesh Number | Total Number of Cells | Heat Transfer Coef. [W/m K] |
|---|---|---|
| MFiX | ||
| mesh 1 | 783,360 | 155.1 |
| mesh 2 | 2,176,000 | 377.3 |
| mesh 3 | 3,133,440 | 446.7 |
| mesh 4 | 4,896,000 | 451.2 |
| OpenFOAM | ||
| mesh 1 | 96,105 | 330.3 |
| mesh 2 | 330,152 | 461.2 |
| mesh 3 | 502,240 | 459.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reyes-Urrutia, A.; Venier, C.; Mariani, N.J.; Nigro, N.; Rodriguez, R.; Mazza, G. A CFD Comparative Study of Bubbling Fluidized Bed Behavior with Thermal Effects Using the Open-Source Platforms MFiX and OpenFOAM. Fluids 2022, 7, 1. https://doi.org/10.3390/fluids7010001
Reyes-Urrutia A, Venier C, Mariani NJ, Nigro N, Rodriguez R, Mazza G. A CFD Comparative Study of Bubbling Fluidized Bed Behavior with Thermal Effects Using the Open-Source Platforms MFiX and OpenFOAM. Fluids. 2022; 7(1):1. https://doi.org/10.3390/fluids7010001
Chicago/Turabian StyleReyes-Urrutia, Andrés, Cesar Venier, Néstor Javier Mariani, Norberto Nigro, Rosa Rodriguez, and Germán Mazza. 2022. "A CFD Comparative Study of Bubbling Fluidized Bed Behavior with Thermal Effects Using the Open-Source Platforms MFiX and OpenFOAM" Fluids 7, no. 1: 1. https://doi.org/10.3390/fluids7010001
APA StyleReyes-Urrutia, A., Venier, C., Mariani, N. J., Nigro, N., Rodriguez, R., & Mazza, G. (2022). A CFD Comparative Study of Bubbling Fluidized Bed Behavior with Thermal Effects Using the Open-Source Platforms MFiX and OpenFOAM. Fluids, 7(1), 1. https://doi.org/10.3390/fluids7010001