Correlation between Large-Scale Streamwise Velocity Features and the Height of Coherent Vortices in a Turbulent Boundary Layer
Abstract
:1. Introduction
2. Methods
2.1. Data Source
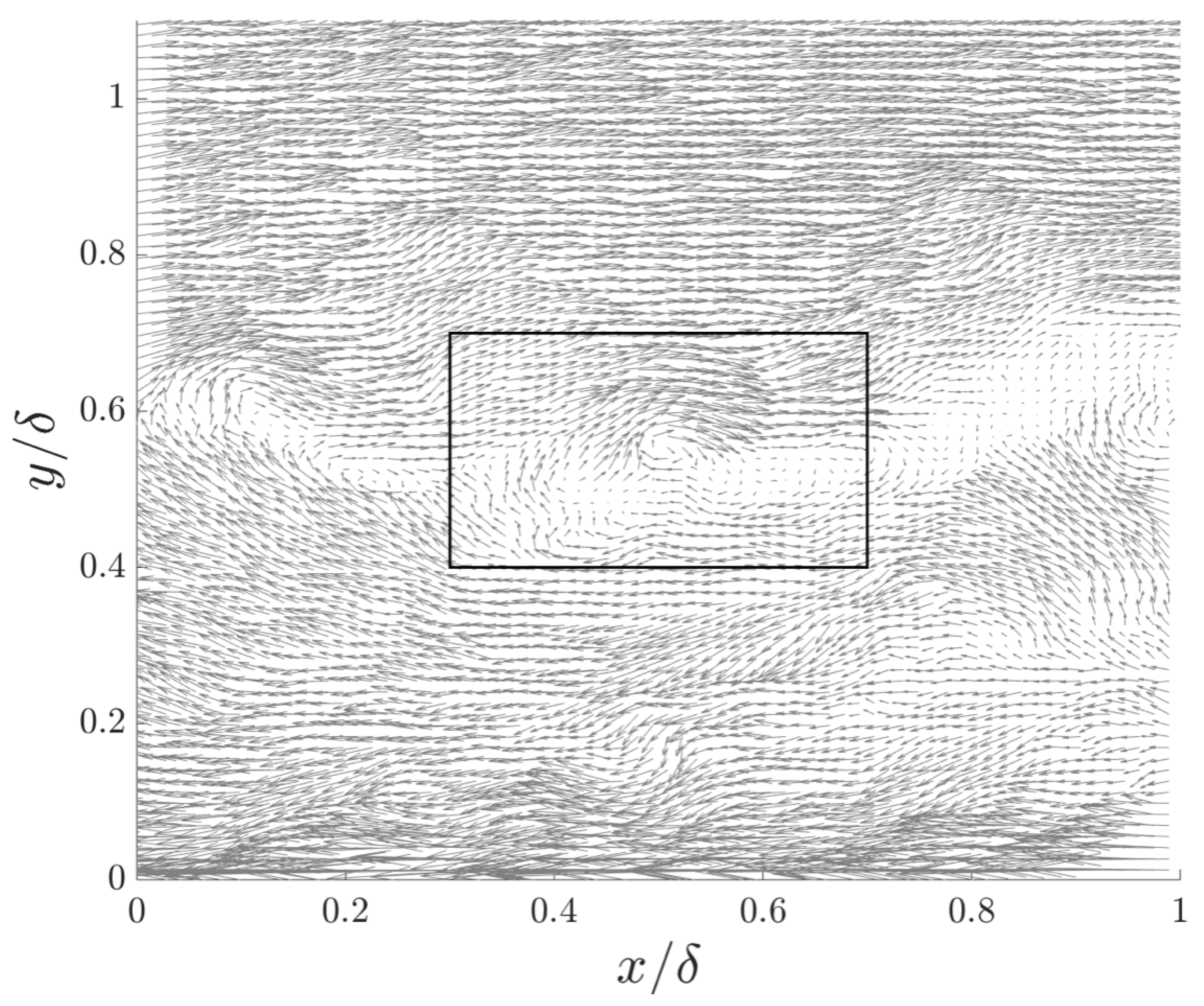
2.2. Vortex Identification
2.3. Filtering
2.4. Relationship between Coherent Vortices and Small-Scale Velocity Features
3. Results
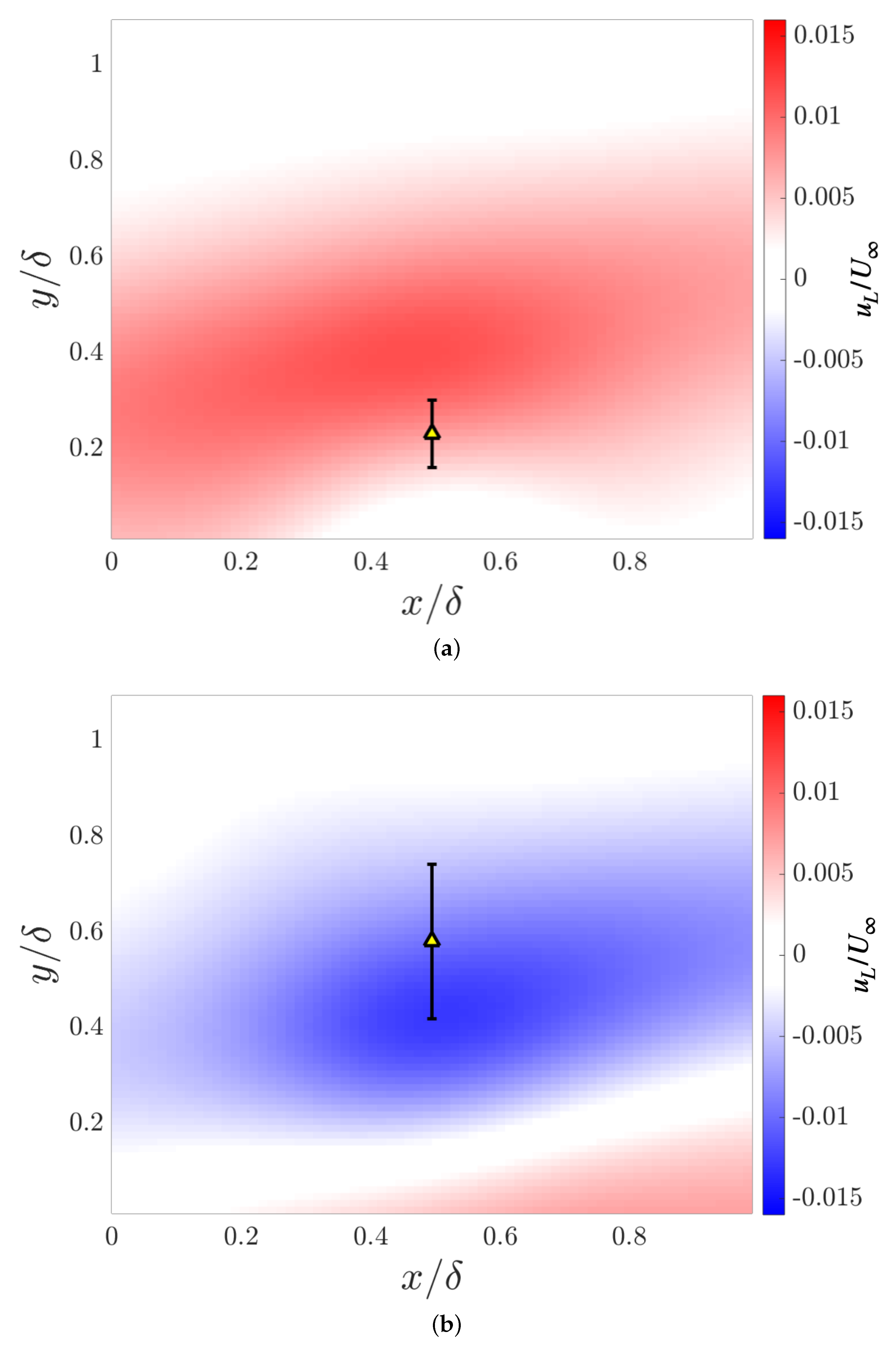
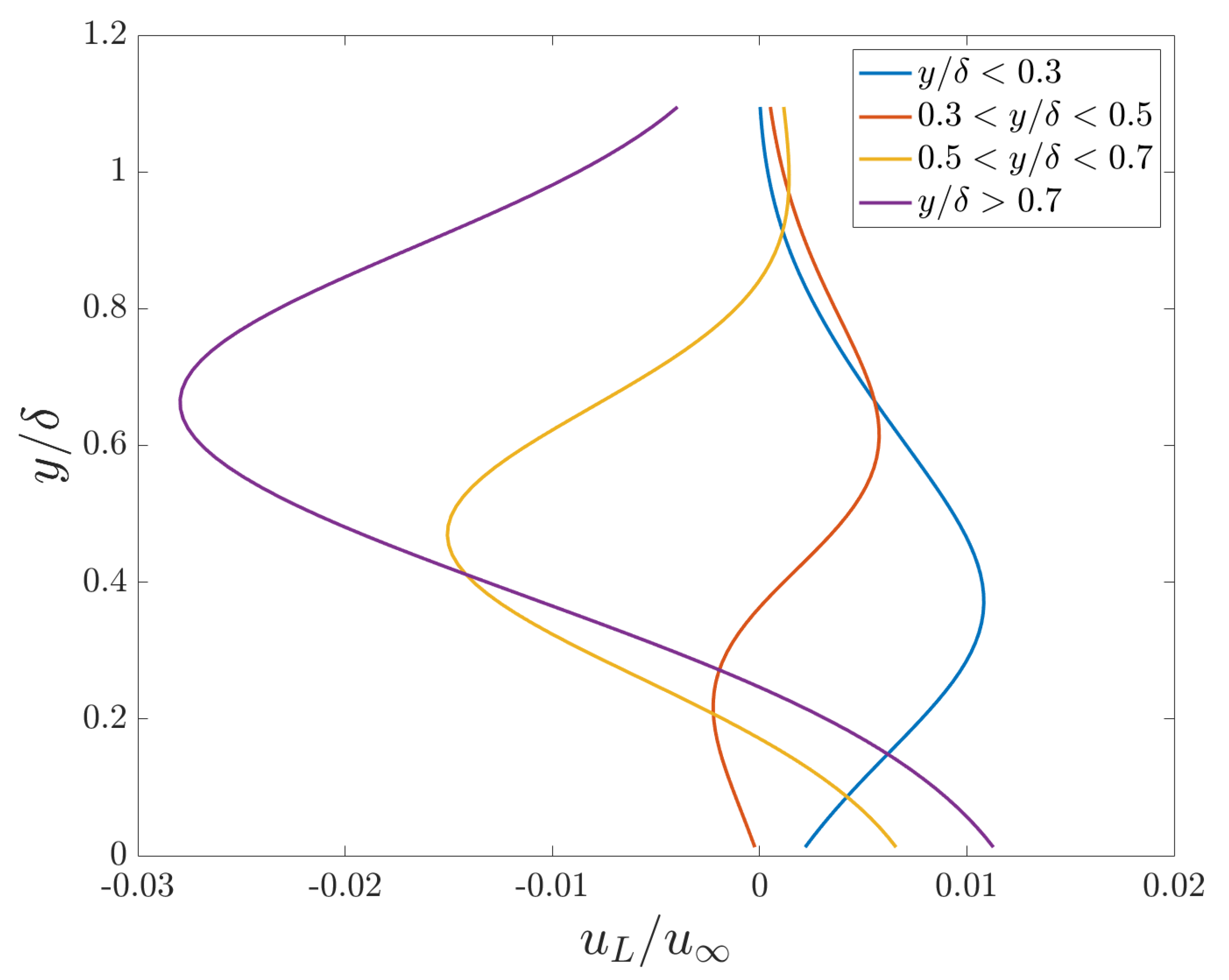
3.1. Conditional Averaging
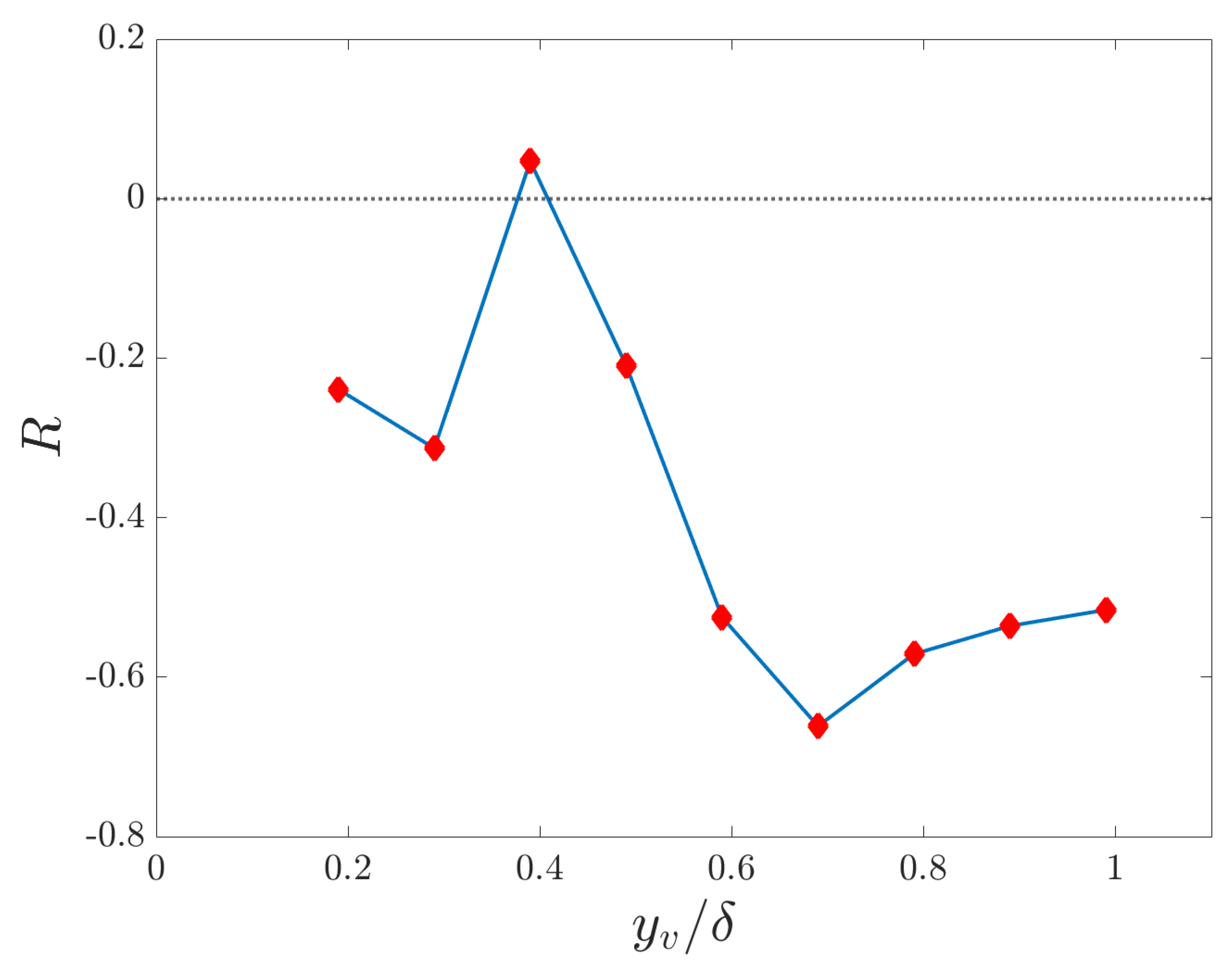
3.2. Correlation Coefficient
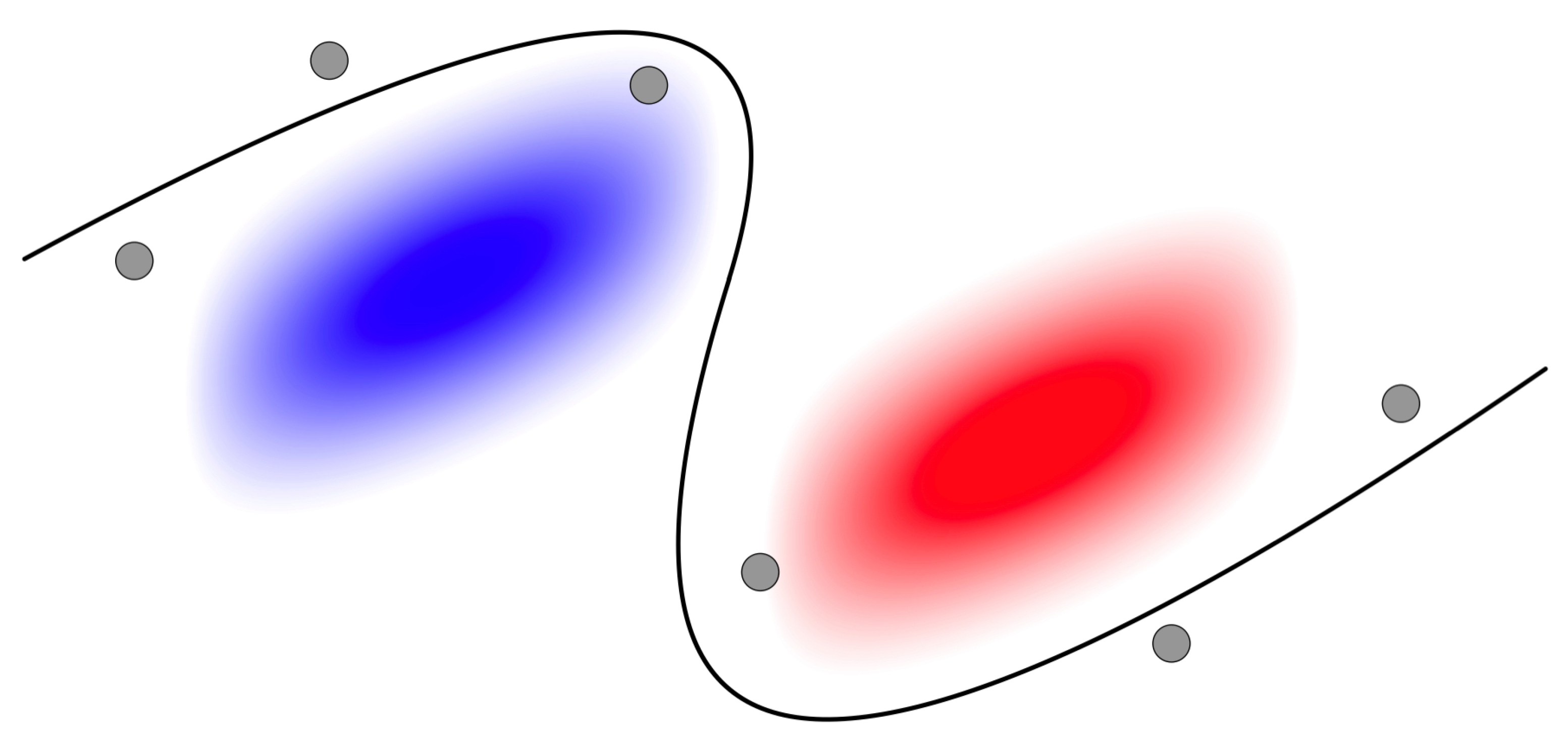
3.3. Relationship to Large-Scale Velocity Isocontour
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
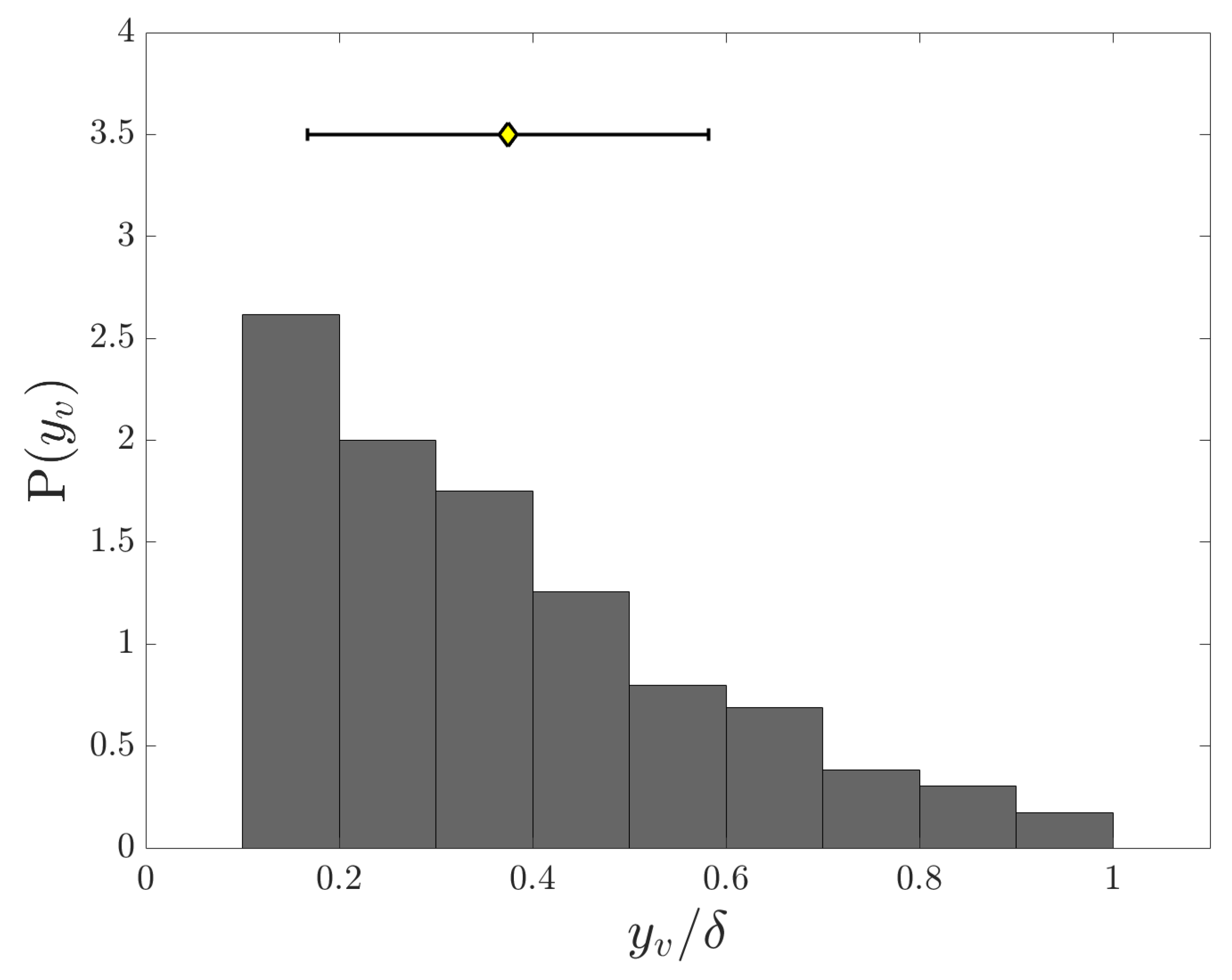
Appendix A. Probability Density Function of the Vortex Heights y v
References
- Robinson, S.K. Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 1991, 23, 601–639. [Google Scholar] [CrossRef]
- Adrian, R.J. Hairpin vortex organization in wall turbulence. Phys. Fluids 2007, 19, 041301. [Google Scholar] [CrossRef] [Green Version]
- Theodorsen, T. Mechanism of turbulence. In Proceedings of the Mid-Western Conference on Fluid Mechanics, Columbus, OH, USA, 17–19 March 1952. [Google Scholar]
- Head, M.R.; Bandyopadhyay, P.R. New aspects of turbulent boundary-layer structure. J. Fluid Mech. 1981, 107, 297–338. [Google Scholar] [CrossRef]
- Falco, R.E. Coherent motions in the outer region of turbulent boundary layers. Phys. Fluids 1977, 20, S124–S132. [Google Scholar] [CrossRef]
- Perry, A.E.; Chong, M.S. On the mechanism of wall turbulence. J. Fluid Mech. 1982, 119, 173–217. [Google Scholar] [CrossRef] [Green Version]
- Adrian, R.J.; Meinhart, C.D.; Tomkins, C.D. Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 2000, 422, 1–54. [Google Scholar] [CrossRef] [Green Version]
- Lozano-Durán, A.; Flores, O.; Jiménez, J. The three-dimensional structure of momentum transfer in turbulent channels. J. Fluid Mech. 2012, 694, 100–130. [Google Scholar] [CrossRef]
- De Silva, C.M.; Hutchins, N.; Marusic, I. Uniform momentum zones in turbulent boundary layers. J. Fluid Mech. 2016, 786, 309–331. [Google Scholar] [CrossRef] [Green Version]
- Eich, F.; de Silva, C.M.; Marusic, I.; Kähler, C.J. Towards an improved spatial representation of a boundary layer from the attached eddy model. Phys. Rev. Fluids 2020, 5, 034601. [Google Scholar] [CrossRef]
- Deshpande, R.; de Silva, C.M.; Lee, M.; Monty, J.P.; Marusic, I. Data-driven enhancement of coherent structure-based models for predicting instantaneous wall turbulence. arXiv 2021, arXiv:2107.01750. [Google Scholar]
- Kim, J.; Moin, P.; Moser, R.D. Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 1987, 177, 133–166. [Google Scholar] [CrossRef] [Green Version]
- Jiménez, J.; Hoyas, S.; Simens, M.P.; Mizuno, Y. Turbulent boundary layers and channels at moderate Reynolds numbers. J. Fluid Mech. 2010, 657, 335–360. [Google Scholar] [CrossRef] [Green Version]
- Eitel-Amor, G.; Örlü, R.; Schlatter, P.; Flores, O. Hairpin vortices in turbulent boundary layers. Phys. Fluids 2015, 27, 025108. [Google Scholar] [CrossRef]
- Motoori, Y.; Goto, S. Hairpin vortices in the largest scale of turbulent boundary layers. Int. J. Heat Fluid Flow 2020, 86, 108658. [Google Scholar] [CrossRef]
- Rao, K.N.; Narasimha, R.; Narayanan, M.A.B. The ‘bursting’ phenomenon in a turbulent boundary layer. J. Fluid Mech. 1971, 48, 339–352. [Google Scholar] [CrossRef] [Green Version]
- Heisel, M.; Dasari, T.; Liu, Y.; Hong, J.; Coletti, F.; Guala, M. The spatial structure of the logarithmic region in very-high-Reynolds-number rough wall turbulent boundary layers. J. Fluid Mech. 2018, 857, 704–747. [Google Scholar] [CrossRef]
- Heisel, M.; de Silva, C.M.; Hutchins, N.; Marusic, I.; Guala, M. Prograde vortices, internal shear layers and the Taylor microscale in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 2021, 920, A52. [Google Scholar] [CrossRef]
- Bautista, J.C.C.; Ebadi, A.; White, C.M.; Chini, G.P.; Klewicki, J.C. A uniform momentum zone–vortical fissure model of the turbulent boundary layer. J. Fluid Mech. 2019, 858, 609–633. [Google Scholar] [CrossRef]
- De Silva, C.M.; Philip, J.; Hutchins, N.; Marusic, I. Interfaces of uniform momentum zones in turbulent boundary layers. J. Fluid Mech. 2017, 820, 451–478. [Google Scholar] [CrossRef] [Green Version]
- Monty, J.P.; Hutchins, N.; Ng, H.C.H.; Marusic, I.; Chong, M.S. A comparison of turbulent pipe, channel and boundary layer flows. J. Fluid Mech. 2009, 632, 431–442. [Google Scholar] [CrossRef]
- Kim, K.C.; Adrian, R.J. Very large-scale motion in the outer layer. Phys. Fluids 1999, 11, 417–422. [Google Scholar] [CrossRef]
- Kline, S.J.; Reynolds, W.C.; Schraub, F.A.; Runstadler, P.W. The structure of turbulent boundary layers. J. Fluid Mech. 1967, 30, 741–773. [Google Scholar] [CrossRef] [Green Version]
- Blackwelder, R.F.; Eckelmann, H. On the wall structure of the turbulent boundary layer. J. Fluid Mech. 1967, 76, 89–112. [Google Scholar] [CrossRef]
- Jiménez, J.; Pinelli, A. The autonomous cycle of near-wall turbulence. J. Fluid Mech. 1999, 389, 335–359. [Google Scholar] [CrossRef] [Green Version]
- Mathis, R.; Hutchins, N.; Marusic, I. Large-scale amplitude modulation of the small-scale structures in turbulent boundary layers. J. Fluid Mech. 2009, 628, 311–337. [Google Scholar] [CrossRef] [Green Version]
- Bernardini, M.; Pirozzoli, S. Inner/outer layer interactions in turbulent boundary layers: A refined measure for the large-scale amplitude modulation mechanism. Phys. Fluids 2011, 23, 061701. [Google Scholar] [CrossRef]
- Baars, W.; Hutchins, N.; Marusic, I. Reynolds number trend of hierarchies and scale interactions in turbulent boundary layers. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2017, 375, 20160077. [Google Scholar] [CrossRef] [PubMed]
- Chung, D.; McKeon, B.J. Large-eddy simulation of large-scale structures in long channel flow. J. Fluid Mech. 2010, 661, 341–364. [Google Scholar] [CrossRef] [Green Version]
- Saxton-Fox, T.; McKeon, B.J. Coherent structures, uniform momentum zones and the streamwise energy spectrum in wall-bounded turbulent flows. J. Fluid Mech. 2017, 826, R6. [Google Scholar] [CrossRef]
- Kolář, V. Vortex identification: New requirements and limitations. Int. J. Heat Fluid Flow 2007, 28, 638–652. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shrivastava, S.; Saxton-Fox, T. Correlation between Large-Scale Streamwise Velocity Features and the Height of Coherent Vortices in a Turbulent Boundary Layer. Fluids 2021, 6, 286. https://doi.org/10.3390/fluids6080286
Shrivastava S, Saxton-Fox T. Correlation between Large-Scale Streamwise Velocity Features and the Height of Coherent Vortices in a Turbulent Boundary Layer. Fluids. 2021; 6(8):286. https://doi.org/10.3390/fluids6080286
Chicago/Turabian StyleShrivastava, Shaurya, and Theresa Saxton-Fox. 2021. "Correlation between Large-Scale Streamwise Velocity Features and the Height of Coherent Vortices in a Turbulent Boundary Layer" Fluids 6, no. 8: 286. https://doi.org/10.3390/fluids6080286
APA StyleShrivastava, S., & Saxton-Fox, T. (2021). Correlation between Large-Scale Streamwise Velocity Features and the Height of Coherent Vortices in a Turbulent Boundary Layer. Fluids, 6(8), 286. https://doi.org/10.3390/fluids6080286






