Intercomparison of Three Open-Source Numerical Flumes for the Surface Dynamics of Steep Focused Wave Groups
Abstract
1. Introduction
2. Methods and Testing Conditions
2.1. Experimental Conditions
2.2. Phase Decomposition
2.3. Correction Methodology
- The target amplitude spectrum is defined and the desired locations for the amplitude and phase corrections, namely AM and PF, respectively, are determined. Moreover, the focal time is selected, usually as half of the repeat time of the periodic signal.
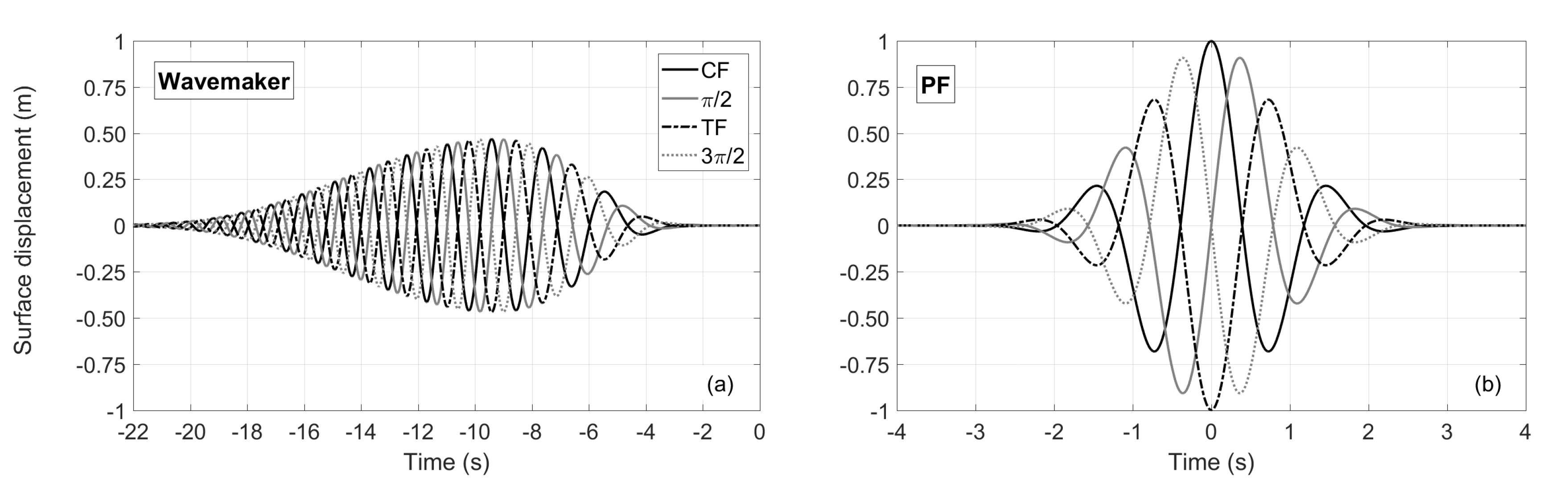
- Wave groups of different phase shifts are generated at the wave paddle. For a four-wave decomposition, four wave groups with phase shifts of 0, , and are used to generate CF, positive slope, TF and negative slope focused waves at the PF location, respectively. For the first run, the linear dispersion relation can be used to backwards propagate the signal from PF to the wavemaker, as a best guess. An example is given in Figure 2, where the contraction of the wave group towards focusing is also evident.
- The linear harmonics are extracted using a suitable linear combination of the four wave groups measured at PF, according to the four-wave decomposition (see Equation (4)) in the frequency domain after performing a Fast Fourier Transform (FFT) of the measured signals.
- The phases and amplitudes of the wave components of the linear harmonic are corrected using Equations (5).where are the input, measured and target amplitudes of the components of the linear spectrum respectively and are input, measured and target phases of the components of the linear spectrum, respectively.
- The corrected signal for the wavemaker can then be calculated: (a) the phases of wave components of the corrected linear spectrum are found by propagating backwards the signal from PF to the wavemaker using the linear dispersion relation, while (b) the corrected amplitudes of the components are not altered according to linear theory, being the same at AM and the wavemaker.
- The process is repeated iteratively from step 2 to 5 until the target values for and match the target values within the desired accuracy.
3. The Numerical Models
3.1. RANS: OpenFOAM
3.1.1. Description of the Solver
3.1.2. Design of the NWT
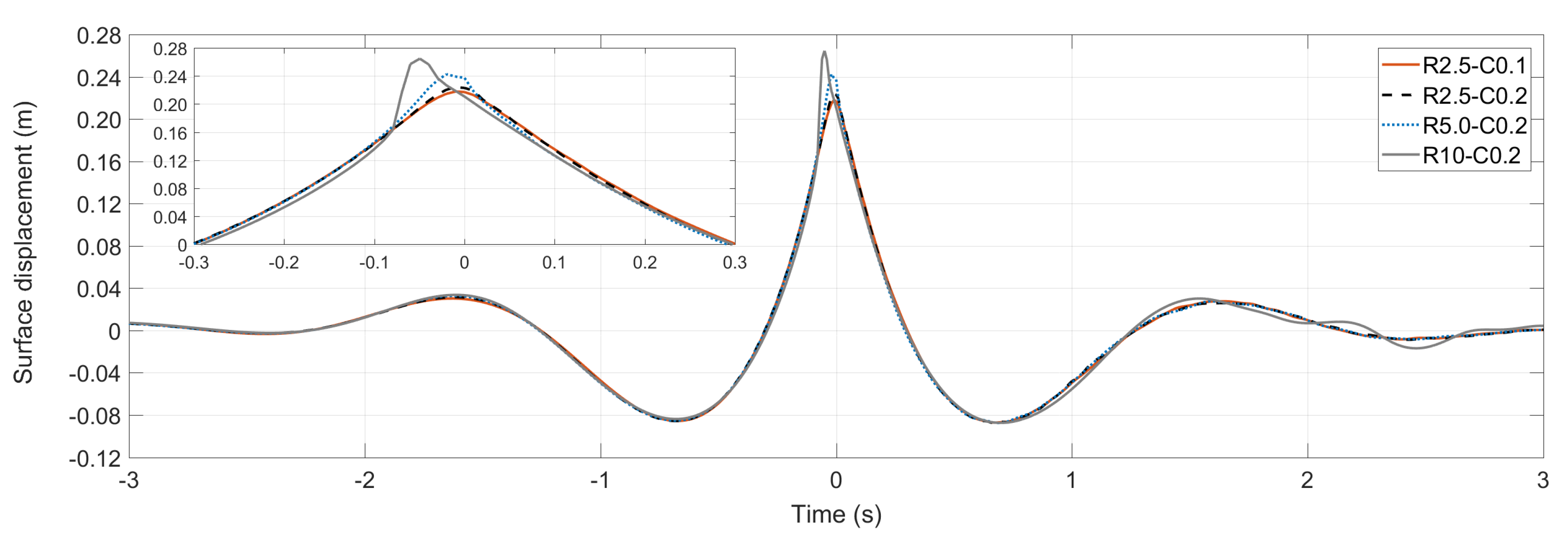
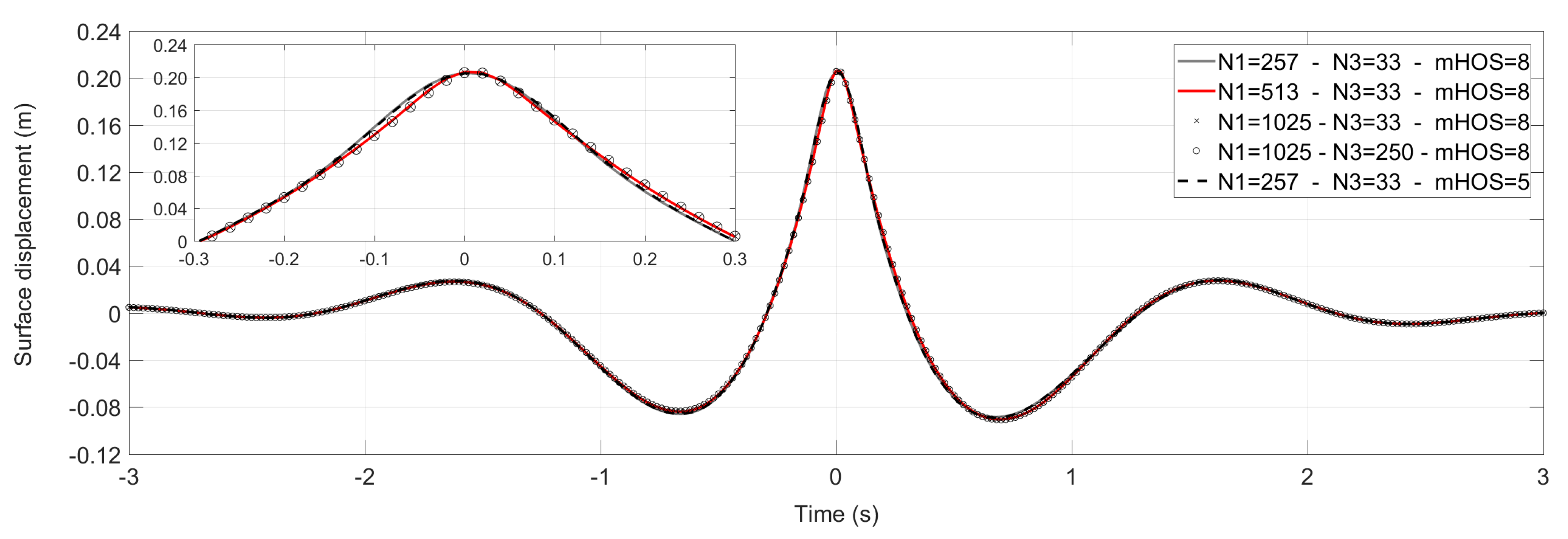
3.1.3. Convergence Tests
3.2. NLSWE: SWASH
3.2.1. Description of the Solver
3.2.2. Design of the NWT
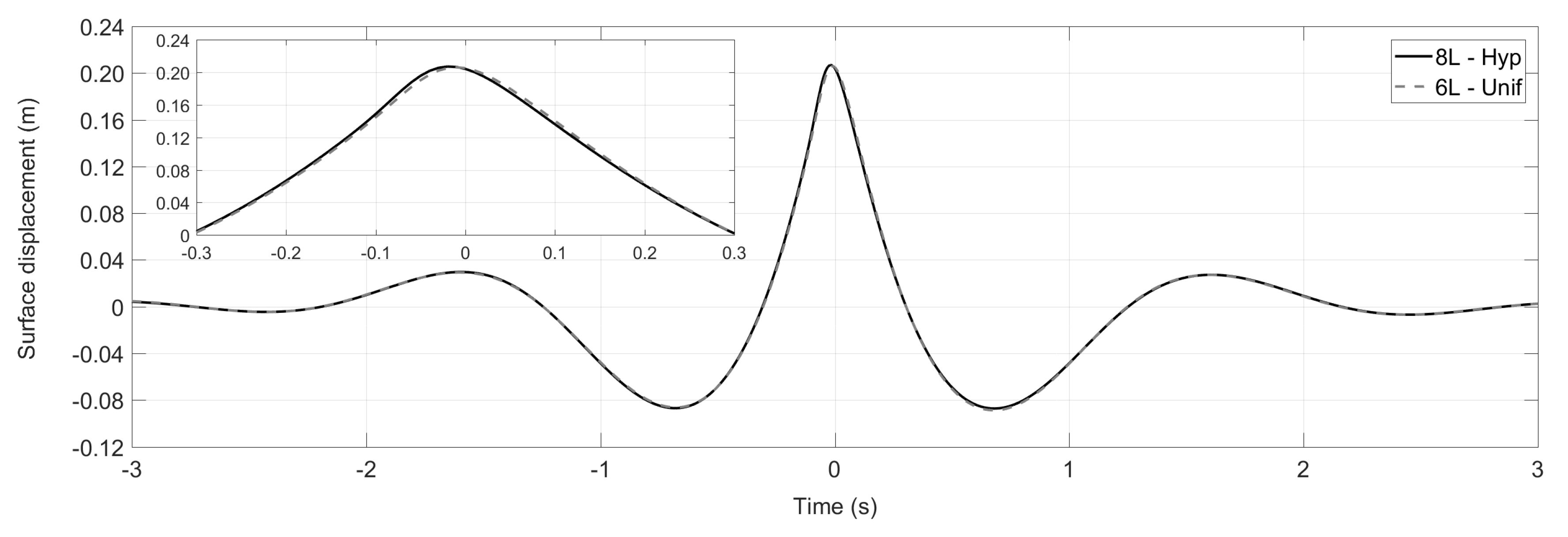
3.2.3. Convergence Tests
3.3. PFT: HOS-NWT
3.3.1. Description of the Solver
3.3.2. Design of the NWT
3.3.3. Convergence Tests
3.4. Summary of NWTs
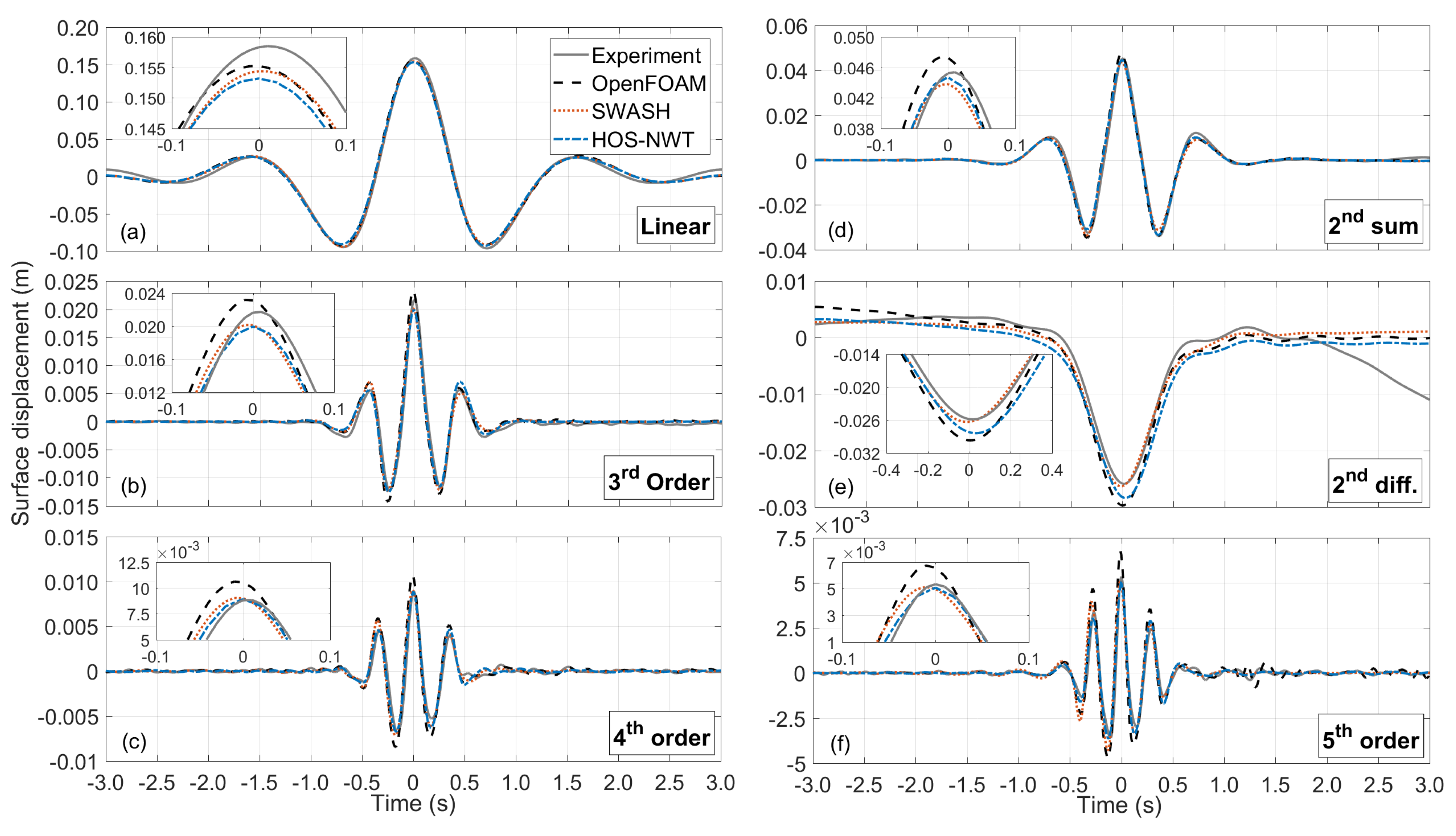
4. Results and Comparisons
4.1. Numerical Dispersion
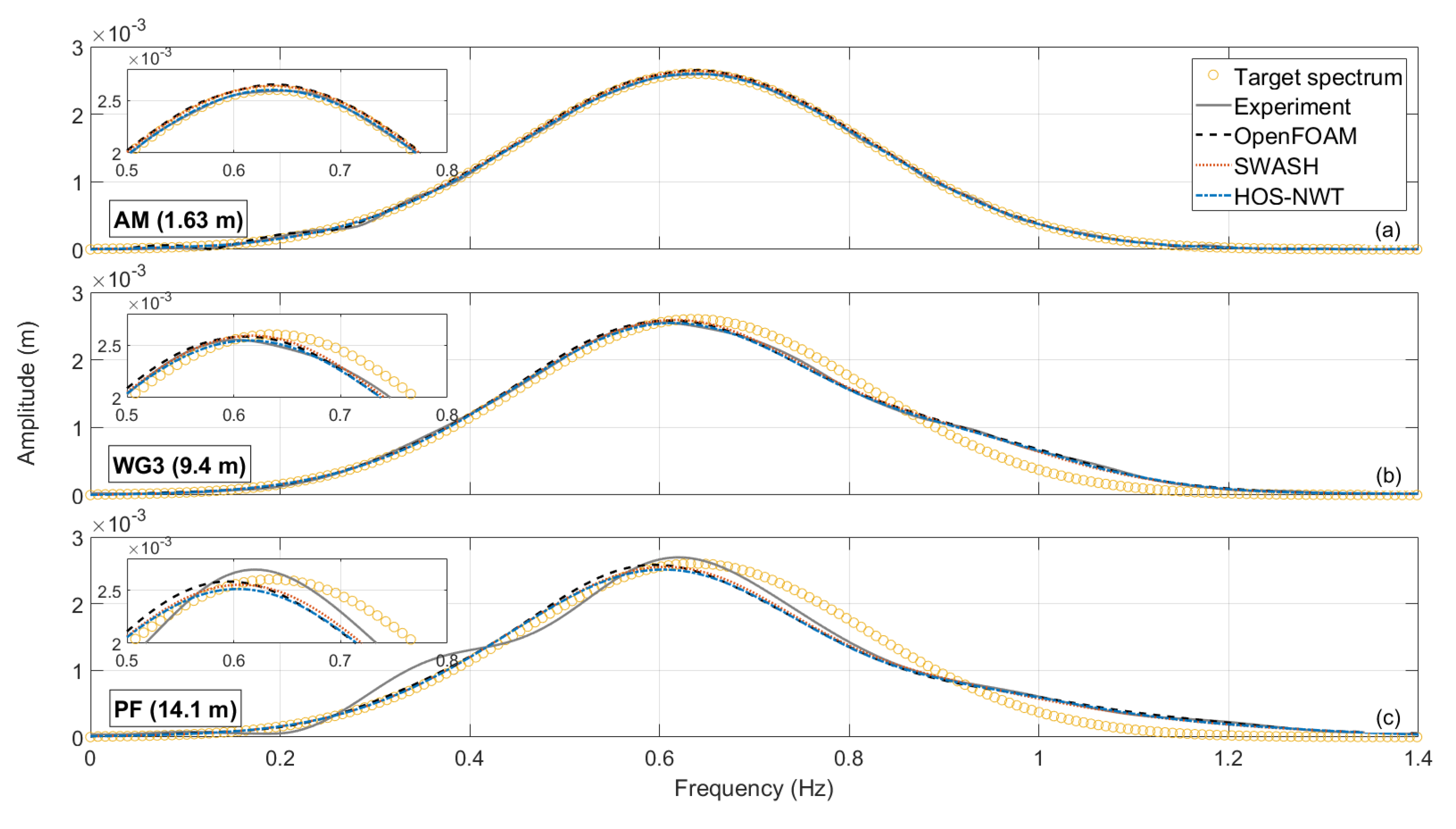
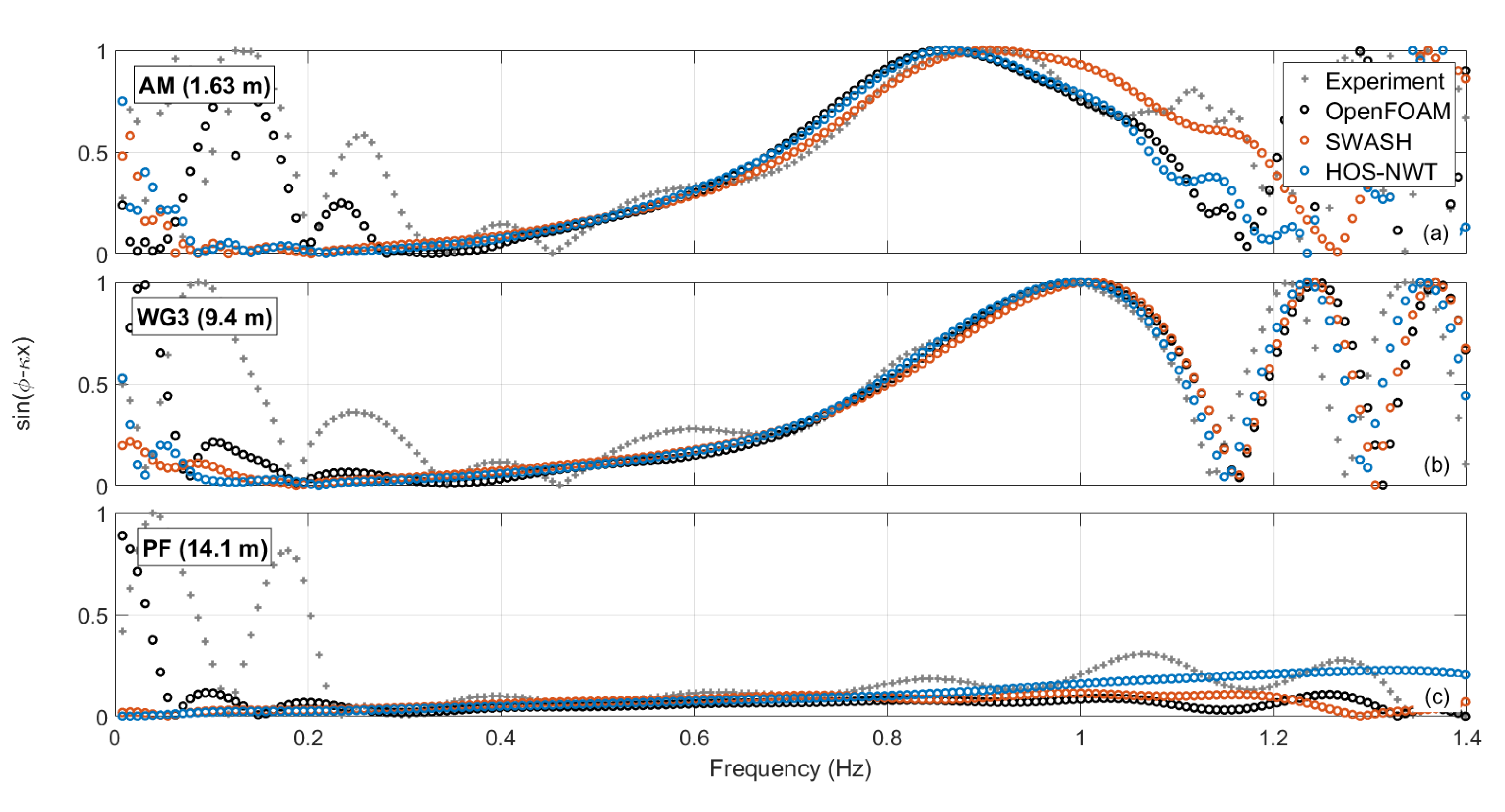
4.2. Evolution of the Linear Harmonics
4.3. Evolution of the 2nd Sum Harmonics
4.4. Evolution of the 2nd Difference Harmonics
4.5. Evolution of the 3rd Order Harmonics
4.6. Wave Group Evolution
4.7. Comparison at the Focal Point
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| AM | Amplitude matching location |
| CF | Crest-focused wave group |
| CFD | Computational Fluid Dynamics |
| FFT | Fast Fourier Transform |
| FVM | Finite Volume Method |
| HOS | High Order Spectral method |
| MWL | Mean water level |
| NSE | Navier-Stokes Equations |
| NSWE | Nonlinear Shallow Water Equations |
| NWT | Numerical Wave Tank |
| PF | Phase focal location |
| PFT | Potential Flow Theory |
| PM | Pierson-Moskowitz Spectrum |
| RANS | Reynolds Averaged Navier-Stokes equations |
| SWL | Still water level |
| TF | Trough-focused wave group |
| VoF | Volume of Fluid method |
| WG | Wave gauges |
Appendix A. Comparison with 2nd Order Theory

| Sum Crest | Sum Trough | Diff Trough | |
|---|---|---|---|
| 2nd order theory calculated (mm) | +40.3 | −24.2 | −22.9 |
| Experiment | +12.7% | −37.3% | −13.2% |
| OpenFOAM | +17.8% | −41.5% | −30.0% |
| SWASH | +8.8% | −33.7% | −15.3% |
| HOS-NWT | +11.0% | −33.8% | −24.3% |
References
- Tsai, C.H.; Su, M.Y.; Huang, S.J. Observations and conditions for occurrence of dangerous coastal waves. Ocean Eng. 2004, 31, 745–760. [Google Scholar] [CrossRef]
- Haver, S. Evidences of the Existence of Freak Waves. In Proceedings of the Rogues Waves 2000 Workshop, Brest, France, 29–30 November 2000; pp. 129–140. [Google Scholar]
- Christou, M.; Ewans, K. Field Measurements of Rogue Water Waves. J. Phys. Oceanogr. 2014, 44, 2317–2335. [Google Scholar] [CrossRef]
- Tromans, P.S.; Anatruk, A.H.R.; Hagemeijer, P. New model for the kinematics of large ocean waves application as a design wave. In Proceedings of the First International Offshore and Polar Engineering Conference, Edinburgh, UK, 11–16 August 1991; pp. 64–71. [Google Scholar]
- Vyzikas, T.; Stagonas, D.; Buldakov, E.; Greaves, D. The evolution of free and bound waves during dispersive focusing in a numerical and physical flume. Coast. Eng. 2018, 132, 95–109. [Google Scholar] [CrossRef]
- Baldock, T.E.; Swan, C.; Taylor, P.H. A laboratory study of nonlinear surface waves on water. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1996, 354, 649–676. [Google Scholar] [CrossRef]
- Johannessen, T.B.; Swan, C. On the nonlinear dynamics of wave groups produced by the focusing of surface-water waves. Proc. R. Soc. A Math. Phys. Eng. Sci. 2003, 459, 1021–1052. [Google Scholar] [CrossRef]
- Gibson, R.; Swan, C. The evolution of large ocean waves: The role of local and rapid spectral changes. Proc. R. Soc. A Math. Phys. Eng. Sci. 2007, 463, 21–48. [Google Scholar] [CrossRef]
- Katsardi, V.; Swan, C. The evolution of large non-breaking waves in intermediate and shallow water. I. Numerical calculations of uni-directional seas. Proc. R. Soc. A Math. Phys. Eng. Sci. 2011, 467, 778–805. [Google Scholar] [CrossRef]
- Fadaeiazar, E.; Leontini, J.; Onorato, M.; Waseda, T.; Alberello, A.; Toffoli, A. Fourier amplitude distribution and intermittency in mechanically generated surface gravity waves. Phys. Rev. E 2020. [Google Scholar] [CrossRef]
- Ning, D.Z.; Zang, J.; Liu, S.X.; Eatock Taylor, R.; Teng, B.; Taylor, P.H. Free-surface evolution and wave kinematics for nonlinear uni-directional focused wave groups. Ocean Eng. 2009, 36, 1226–1243. [Google Scholar] [CrossRef]
- Stagonas, D.; Buldakov, E.; Simons, R. Focusing unidirectional wave groups on finite water depth with and without currents. In Proceedings of the 34th International Conference on Coastal Engineering, Seoul, Korea, 15–20 June 2014. [Google Scholar] [CrossRef]
- Bitner-Gregersen, E.M.; Gramstad, O. Rogue Waves: Impact on Ships and Offshore Structures; DNV GL Strategic Research & Innovation Position Paper 05-2015, Technical Report; DNV GL: Oslo, Norway, 21 March 2016. [Google Scholar]
- Liu, P.L.F.; Losada, I.J. Wave propagation modeling in coastal engineering. J. Hydraul. Res. 2002, 40, 229–240. [Google Scholar] [CrossRef]
- Paulsen, B.T.; Bredmose, H.; Bingham, H.B. An efficient domain decomposition strategy for wave loads on surface piercing circular cylinders. Coast. Eng. 2014, 86, 57–76. [Google Scholar] [CrossRef]
- Alberello, A.; Pakodzi, C.; Nelli, F.; Bitner-Gregersen, E.M.; Toffoli, A. Three dimensional velocity field underneath a breaking rogue wave. In Proceedings of the ASME 2017 36th International Conference on Ocean, Offshore and Arctic Engineering, Trondheim, Norway, 25–30 June 2017. [Google Scholar]
- Zijlema, M.; Stelling, G.S.; Smit, P.B. SWASH: An operational public domain code for simulating wave fields and rapidly varied flows in coastal waters. Coast. Eng. 2011, 58, 992–1012. [Google Scholar] [CrossRef]
- Ducrozet, G.; Bonnefoy, F.; Le Touzé, D.; Ferrant, P. A modified High-Order Spectral method for wavemaker modeling in a numerical wave tank. Eur. J. Mech. B/Fluids 2012, 34, 19–34. [Google Scholar] [CrossRef]
- Stagonas, D.; Higuera, P.; Buldakov, E. Simulating Breaking Focused Waves in CFD: Methodology for Controlled Generation of First and Second Order. J. Waterw. Port Coast. Ocean. Eng. 2018, 144, 06017005. [Google Scholar] [CrossRef]
- Ransley, E.J.; Brown, S.A.; Hann, M.; Greaves, D.M.; Windt, C.; Ringwood, J.; Davidson, J.; Schmitt, P.; Yan, S.; Wang, J.X.; et al. Focused wave interactions with floating structures: A blind comparative study. Proc. Inst. Civ. Eng. Eng. Comput. Mech. 2020, 1–34. [Google Scholar] [CrossRef]
- Shemer, L.; Jiao, H.; Kit, E.; Agnon, Y. Evolution of a nonlinear wave field along a tank: Experiments and numerical simulations based on the spatial Zakharov equation. J. Fluid Mech. 2001, 427, 107–129. [Google Scholar] [CrossRef]
- Chen, L.F.; Zang, J.; Hillis, A.J.; Morgan, G.C.J.; Plummer, A.R. Numerical investigation of wave-structure interaction using OpenFOAM. Ocean Eng. 2014, 88, 91–109. [Google Scholar] [CrossRef]
- Johannessen, T.B.; Swan, C. A laboratory study of the focusing of transient and directionally spread surface water waves. Proc. R. Soc. A Math. Phys. Eng. Sci. 2001, 457, 971–1006. [Google Scholar] [CrossRef]
- Ning, D.Z.; Teng, B.; Zang, J.; Liu, S.X. An Efficient Model for Transient Surface Waves in Both Finite and Infinite Water Depths. China Ocean Eng. 2009, 23, 459–472. [Google Scholar]
- Johannessen, T.B. Calculations of kinematics underneath measured time histories of steep water waves. Appl. Ocean Res. 2010, 32, 391–403. [Google Scholar] [CrossRef]
- Fitzgerald, C.; Grice, J.; Taylor, P.H.; Eatock Taylor, R.; Zang, J. Phase manipulation and the harmonic components of ringing forces on a surface piercing column. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 2014, 470, 20130847. [Google Scholar] [CrossRef]
- Siddorn, P.D. Efficient Numerical Modelling of Wave-Structure Interaction. Ph.D. Thesis, University of Oxford, Oxford, UK, 2012. [Google Scholar]
- Zhao, W.; Wolgamot, H.A.; Taylor, P.H.; Eatock Taylor, R. Gap resonance and higher harmonics driven by focused transient wave groups. J. Fluid Mech. 2017, 812, 905–939. [Google Scholar] [CrossRef]
- Mai, T.; Greaves, D.; Raby, A.; Taylor, P.H. Physical modelling of wave scattering around fixed FPSO-shaped bodies. Phys. Procedia 2016, 61, 115–129. [Google Scholar] [CrossRef]
- Hann, M.; Greaves, D.; Raby, A.C. A new set of focused wave linear combinations to extract non-linear wave harmonics. In Proceedings of the 32nd International Workshop on Water Waves and Floating Bodies (IWWWFB29), Dalian, China, 23–26 April 2014. [Google Scholar]
- Vyzikas, T.; Stagonas, D.; Buldakov, E.; Greaves, D. On the simulation of focused waves with OpenFOAM & waves2Foam. In Proceedings of the Coastlab14: 5th International Conference on the Application of Physical Modelling to Port and Coastal Protection, Varna, Bulgaria, 29 September–2 October 2014; pp. 2, 237–282. [Google Scholar]
- Vyzikas, T.; Stagonas, D.; Buldakov, E.; Greaves, D. Efficient numerical modelling of focused wave groups for freak wave generation. In Proceedings of the ISOPE-2015: 25th International Offshore and Polar Engineering Conference, Kona, HI, USA, 21–26 June 2015. [Google Scholar]
- Buldakov, E.; Stagonas, D.; Simons, R. Lagrangian numerical wave-current flume. In Proceedings of the International Workshop for Water Waves and Floating Bodies (IWWWFB30), Bristol, UK, 12–15 April 2015. [Google Scholar]
- Buldakov, E.; Stagonas, D.; Simons, R. Extreme wave groups in a wave flume: Controlled generation and breaking onset. Coast. Eng. 2017, 128, 75–83. [Google Scholar] [CrossRef]
- Wang, H.; Draper, S.; Zhao, W.; Wolgamot, H.; Cheng, L. Development of a CFD Model to Simulate Three-Dimensional Gap Resonance Applicable to FLNG Side-by-Side Offloading. In Proceedings of the 36th International Conference on Ocean, Offshore and Arctic Engineering (OMAE2017), Trondheim, Norway, 25–30 June 2017. [Google Scholar]
- Jasak, H. Error Analysis and Estimation for the Finite Volume Method with Applications to Fluid Flows. Ph.D. Thesis, Imperical College of Science, Technology and Medicine, London, UK, 1996. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620–631. [Google Scholar] [CrossRef]
- Jasak, H.; Jemcov, A.; Tukovic, Z. OpenFOAM: A C ++ Library for Complex Physics Simulations. In Proceedings of the International Workshop on Coupled Methods in Numerical Dynamics, Dubrovnik, Croatia, 19–21 September 2007. [Google Scholar]
- Berberović, E.; van Hinsberg, N.P.; Jakirlić, S.; Roisman, I.V.; Tropea, C. Drop impact onto a liquid layer of finite thickness: Dynamics of the cavity evolution. Phys. Rev. E 2009, 79, 036306. [Google Scholar] [CrossRef]
- Higuera, P.; Lara, J.L.; Losada, I.J. Realistic wave generation and active wave absorption for Navier—Stokes models Application to OpenFOAM®. Coast. Eng. 2013, 71, 102–118. [Google Scholar] [CrossRef]
- Jacobsen, N.G.; Fuhrman, D.R.; Fredsøe, J. A wave generation toolbox for the open-source CFD library: OpenFoam®. Int. J. Numer. Methods Fluids 2012, 70, 1073–1088. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malaskekera, W. An Introduction to Computational Fluid Dynamics, The Finite Volume Method, 2nd ed.; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2007; p. 517. [Google Scholar] [CrossRef]
- Ferziger, J.; Peric, M. Computational Methods for Fluid Dynamics, 3rd ed.; Springer: Berlin, Germany, 2002. [Google Scholar]
- Morgan, G.C.J.; Zang, J. Using the rasInterFoam CFD model for non-linear wave interaction with a cylinder. In Proceedings of the Twentieth International Offshore and Polar Engineering Conference, Beijing, China, 20–25 June 2010; pp. 418–423. [Google Scholar]
- OpenCFD. OpenFOAM: The Open Source CFD Toolbox User Guide Version 2.1.1. Available online: https://openfoam.org/release/2-1-1/ (accessed on 1 November 2020).
- Courant, R.; Friedrichs, K.; Lewy, H. On the Partial Difference Equations of Mathematical Physics. IBM J. Res. Dev. 1967, 11, 215–234. [Google Scholar] [CrossRef]
- Stelling, G.S.; Zijlema, M. An accurate and efficient finite-difference algorithm for non-hydrostatic free-surface flow with application to wave propagation. Int. J. Numer. Methods Fluids 2003, 23, 1–23. [Google Scholar] [CrossRef]
- Stelling, G.S.; Duinmeijer, S.P. A staggered conservative scheme for every Froude number in rapidly varied shallow water flows. Int. J. Numer. Methods Fluids 2003, 43, 1329–1354. [Google Scholar] [CrossRef]
- Zijlema, M.; Stelling, G.S. Further experiences with computing non-hydrostatic free-surface flows involving water waves. Int. J. Numer. Methods Fluids 2005, 48, 169–197. [Google Scholar] [CrossRef]
- Zijlema, M.; Stelling, G.S. Efficient computation of surf zone waves using the nonlinear shallow water equations with non-hydrostatic pressure. Coast. Eng. 2008, 55, 780–790. [Google Scholar] [CrossRef]
- Rijnsdorp, D.P.; Smit, P.B.; Zijlema, M. Non-hydrostatic modelling of infragravity waves under laboratory conditions. Coast. Eng. 2014, 85, 30–42. [Google Scholar] [CrossRef]
- Ducrozet, G.; Bonnefoy, F.; Le Touzé, D.; Ferrant, P. HOS-ocean: Open-source solver for nonlinear waves in open ocean based on High-Order Spectral method. Comput. Phys. Commun. 2016, 203, 245–254. [Google Scholar] [CrossRef]
- Bonnefoy, F.; Touzé, D.L.; Ferrant, P. A fully-spectral 3D time-domain model for second-order simulation of wavetank experiments. Part A: Formulation, implementation and numerical properties. Appl. Ocean Res. 2006, 28, 121–132. [Google Scholar] [CrossRef]
- Ducrozet, G.; Bonnefoy, F.; Le Touzé, D.; Ferrant, P. Implementation and validation of nonlinear wavemaker models in a HOS numerical wave tank. Int. J. Offshore Polar Eng. 2006, 16, 161–167. [Google Scholar]
- Dommermuth, D.G.; Yue, D.K.P. A high-order spectral method for the study of nonlinear gravity waves. J. Fluid Mech. 1987, 184, 267. [Google Scholar] [CrossRef]
- West, J.; Brueckner, K.A. A New Numerical Method for Surface Hydrodynamics. J. Fluid Mech. 1987, 92, 11803–11824. [Google Scholar] [CrossRef]
- Zakharov, V.E. Stability of periodic waves of finite amplitude on the surface of a deep fluid. J. Appl. Mech. Tech. Phys. 1968, 9, 190–194. [Google Scholar] [CrossRef]
- Ducrozet, G.; Bonnefoy, F.; Le Touzé, D.; Ferrant, P. 3-D HOS simulations of extreme waves in open seas. Nat. Hazards Earth Syst. Sci. 2007, 7, 109–122. [Google Scholar] [CrossRef]
- Agnon, Y.; Bingham, H.B. A non-periodic spectral method with application to nonlinear water waves. Eur. J. Mech. B/Fluids 1999, 18, 527–534. [Google Scholar] [CrossRef]
- Vyzikas, T. Numerical Modelling of Extreme Waves: The Role of Nonlinear Wave-Wave Interactions. Ph.D. Thesis, University of Plymouth, Plymouth, UK, 2018. [Google Scholar]
- Adcock, T.A.A.; Taylor, P.H.; Yan, S.; Ma, Q.W.; Janssen, P.A.E.M. Did the Draupner wave occur in a crossing sea? Proc. R. Soc. A Math. Phys. Eng. Sci. 2011, 467, 3004–3021. [Google Scholar] [CrossRef]
- Higuera, P.; Losada, I.J.; Lara, J.L. Three-dimensional numerical wave generation with moving boundaries. Coast. Eng. 2015, 101, 35–47. [Google Scholar] [CrossRef]
- Chaplin, J.R.; Rainey, R.C.T.; Yemm, R.W. Ringing of a vertical cylinder in waves. J. Fluid Mech. 1997, 350, 119–147. [Google Scholar] [CrossRef]
- Orszaghova, J.; Taylor, P.H.; Borthwick, A.G.L.; Raby, A.C. Importance of second-order wave generation for focused wave group run-up and overtopping. Coast. Eng. 2014, 94, 63–79. [Google Scholar] [CrossRef]
- Whittaker, C.; Fitzgerald, C.; Raby, A.; Taylor, P.; Orszaghova, J.; Borthwick, A. Optimisation of focused wave group runup on a plane beach. Coast. Eng. 2017, 121, 44–55. [Google Scholar] [CrossRef]
- Bihs, H.; Wang, W.; Pakozdi, C.; Kamath, A. REEF3D::FNPF_A Flexible Fully Nonlinear Potential Flow Solver. J. Offshore Mech. Arct. Eng. 2020, 142. [Google Scholar] [CrossRef]
- Wang, W.; Kamath, A.; Pakozdi, C.; Bihs, H. Investigation of Focusing Wave Properties in a Numerical Wave Tank with a Fully Nonlinear Potential Flow Model. J. Mar. Sci. Eng. 2019, 7, 375. [Google Scholar] [CrossRef]
- Alberello, A.; Chabchoub, A.; Gramstad, O.; Babanin, A.V.; Toffoli, A. Non-Gaussian properties of second-order wave orbital velocity. Coast. Eng. 2016, 110, 42–49. [Google Scholar] [CrossRef]
- Alberello, A.; Chabchoub, A.; Monty, J.P.; Nelli, F.; Lee, J.H.; Elsnab, J.; Toffoli, A. An experimental comparison of velocities underneath focussed breaking waves. Ocean Eng. 2018, 155, 201–210. [Google Scholar] [CrossRef]
- Alberello, A.; Iafrati, A. The velocity field underneath a breaking rogue wave: Laboratory experiments versus numerical simulations. Fluids 2019, 4, 68. [Google Scholar] [CrossRef]
- Nelli, F.; Bennetts, L.G.; Skene, D.M.; Toffoli, A. Water wave transmission and energy dissipation by a floating plate in the presence of overwash. J. Fluid Mech. 2020, 889, A19. [Google Scholar] [CrossRef]
- Dalzell, J.F. A note on finite depth second-order wave—Wave interactions. Appl. Ocean Res. 1999, 21, 105–111. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. Resonant interactions between two trains of gravity waves. J. Fluid Mech. 1962, 12, 321–332. [Google Scholar] [CrossRef]
- Hu, Z.Z.; Greaves, D.; Raby, A.C. Numerical wave tank study of extrme waves and wave structure interaction using OpenFOAM. In Proceedings of the Coastlab14: 5th International Conference on the Application of Physical Modelling to Port and Coastal Protection, Varna, Bulgaria, 29 September–2 October 2014; pp. 2, 243–252. [Google Scholar]
- Vyzikas, T.; Prevosto, M.; Maisondieu, C.; Tassin, A.; Greaves, D. Reconstruction of an extreme wave profile with analytical methods. In Proceedings of the 33rd International Workshop for Water Waves and Floating Bodies (IWWWFB), Guidel-Plages, France, 4–7 April 2018; pp. 1–4. [Google Scholar]
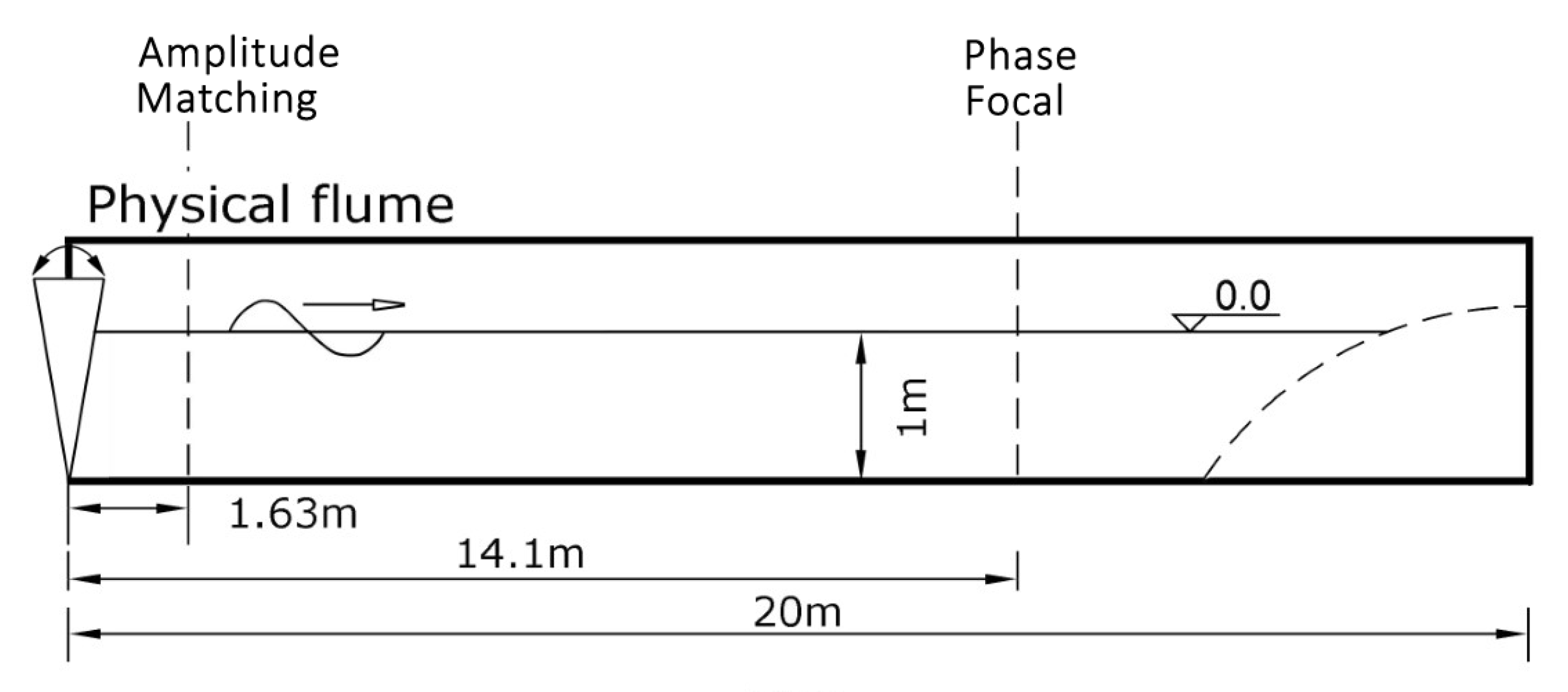
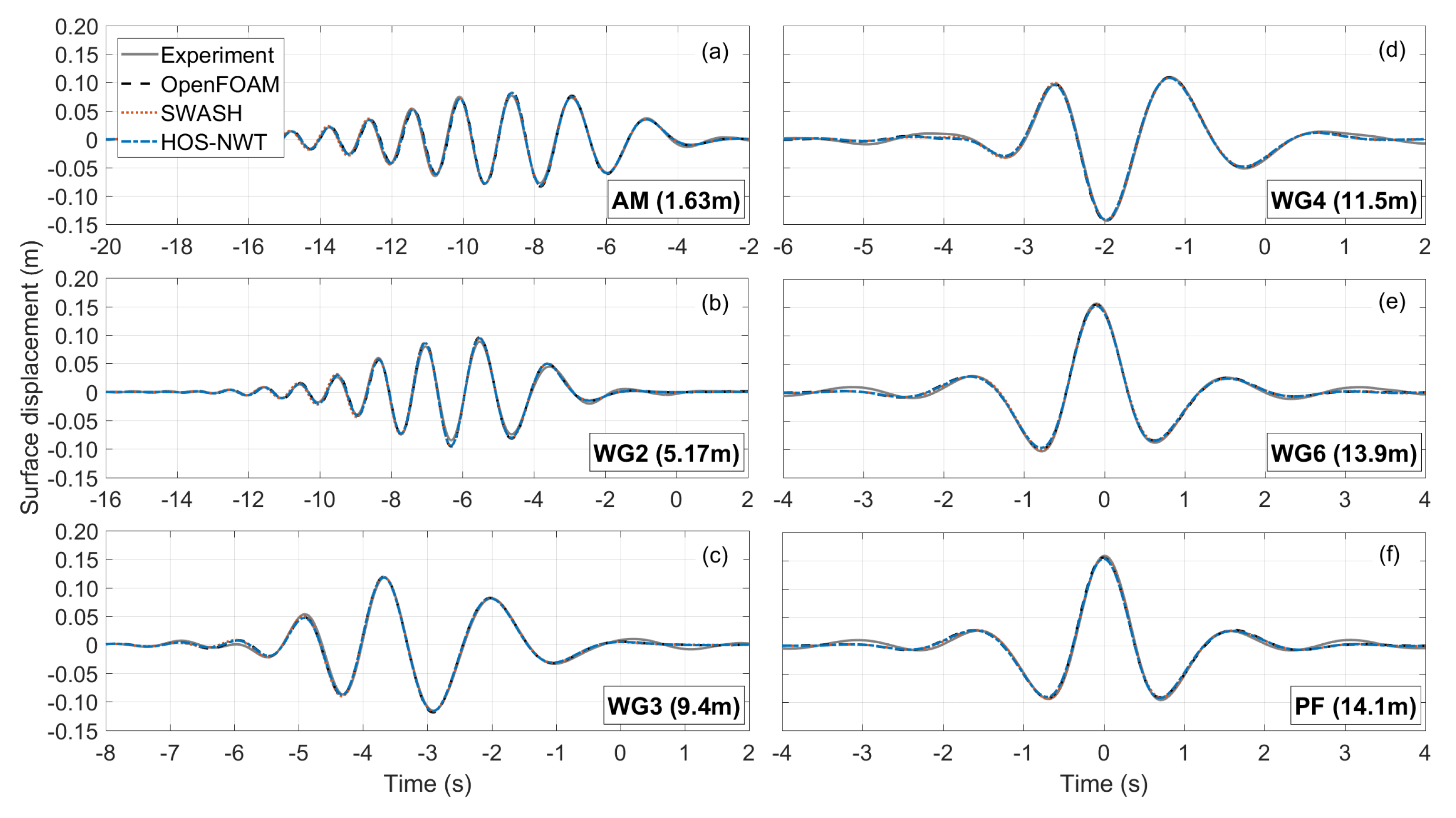
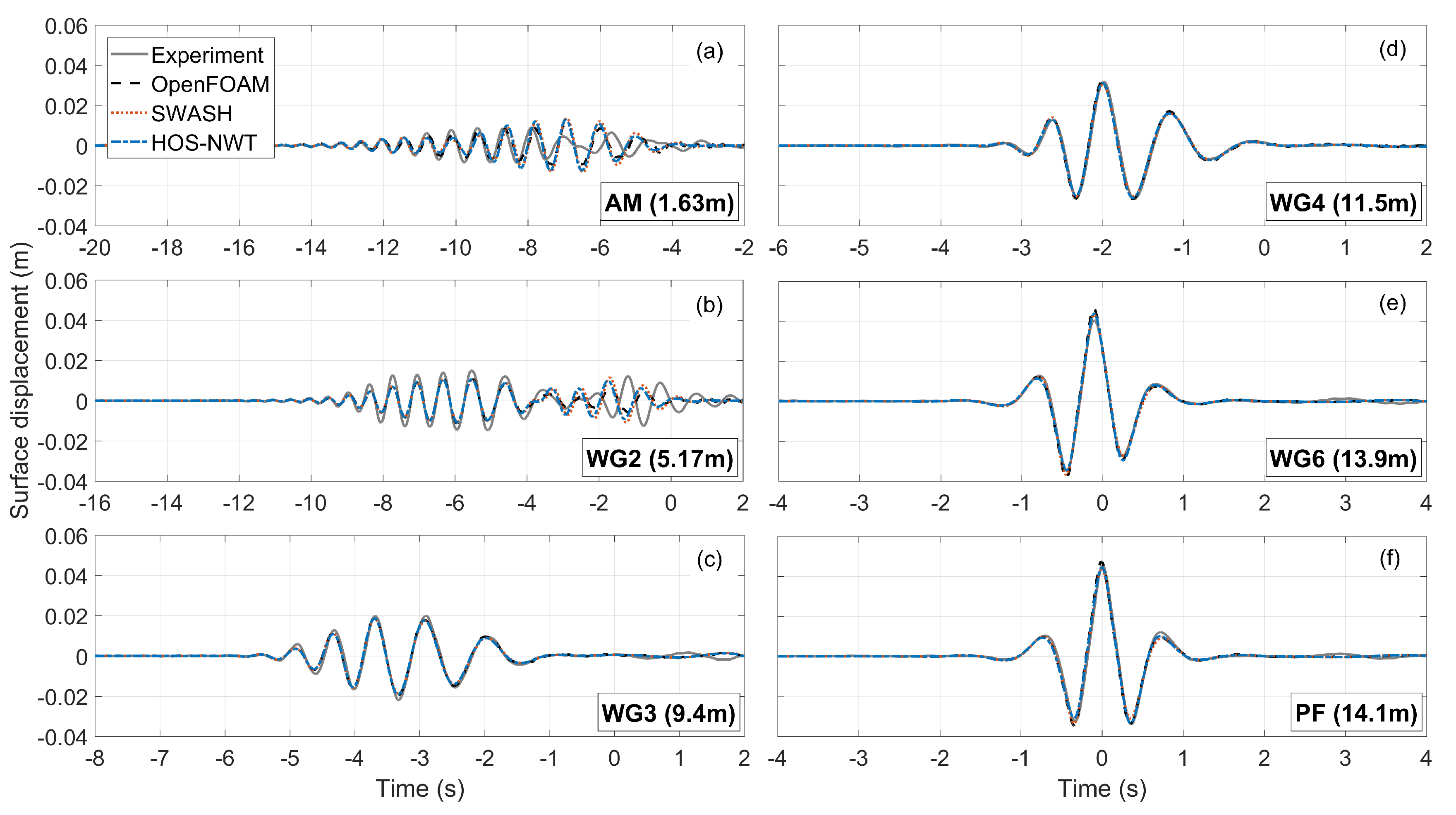
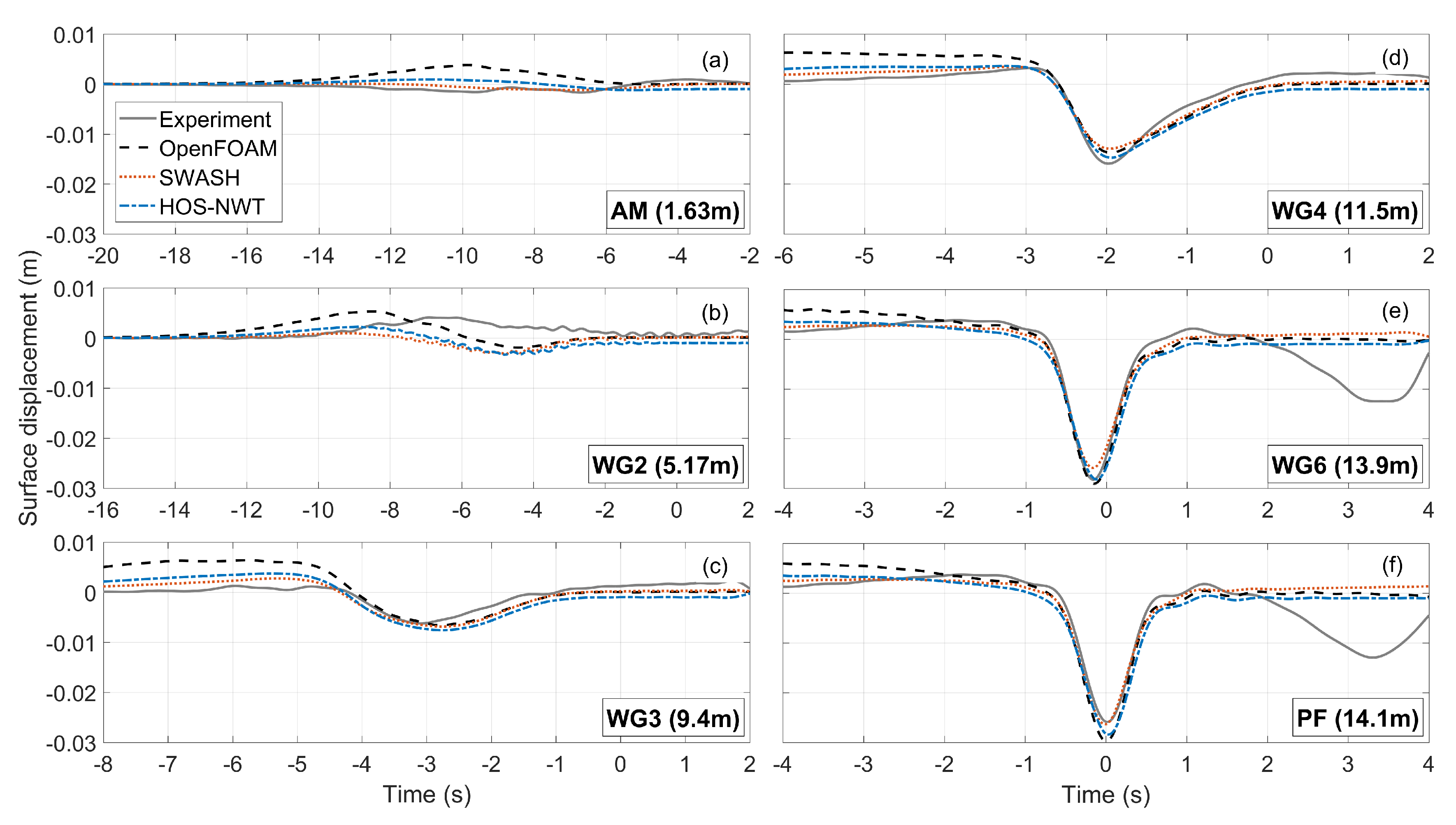
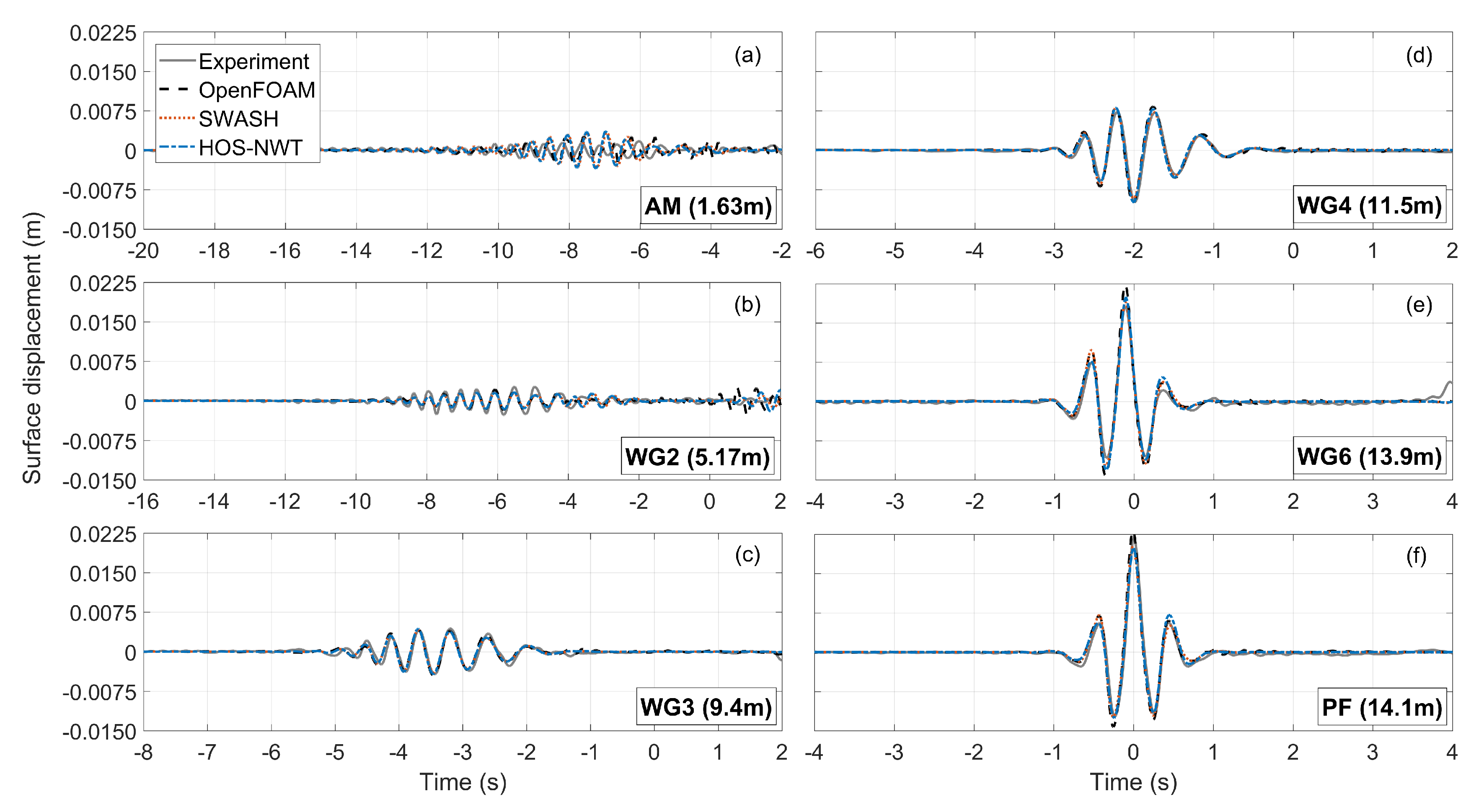
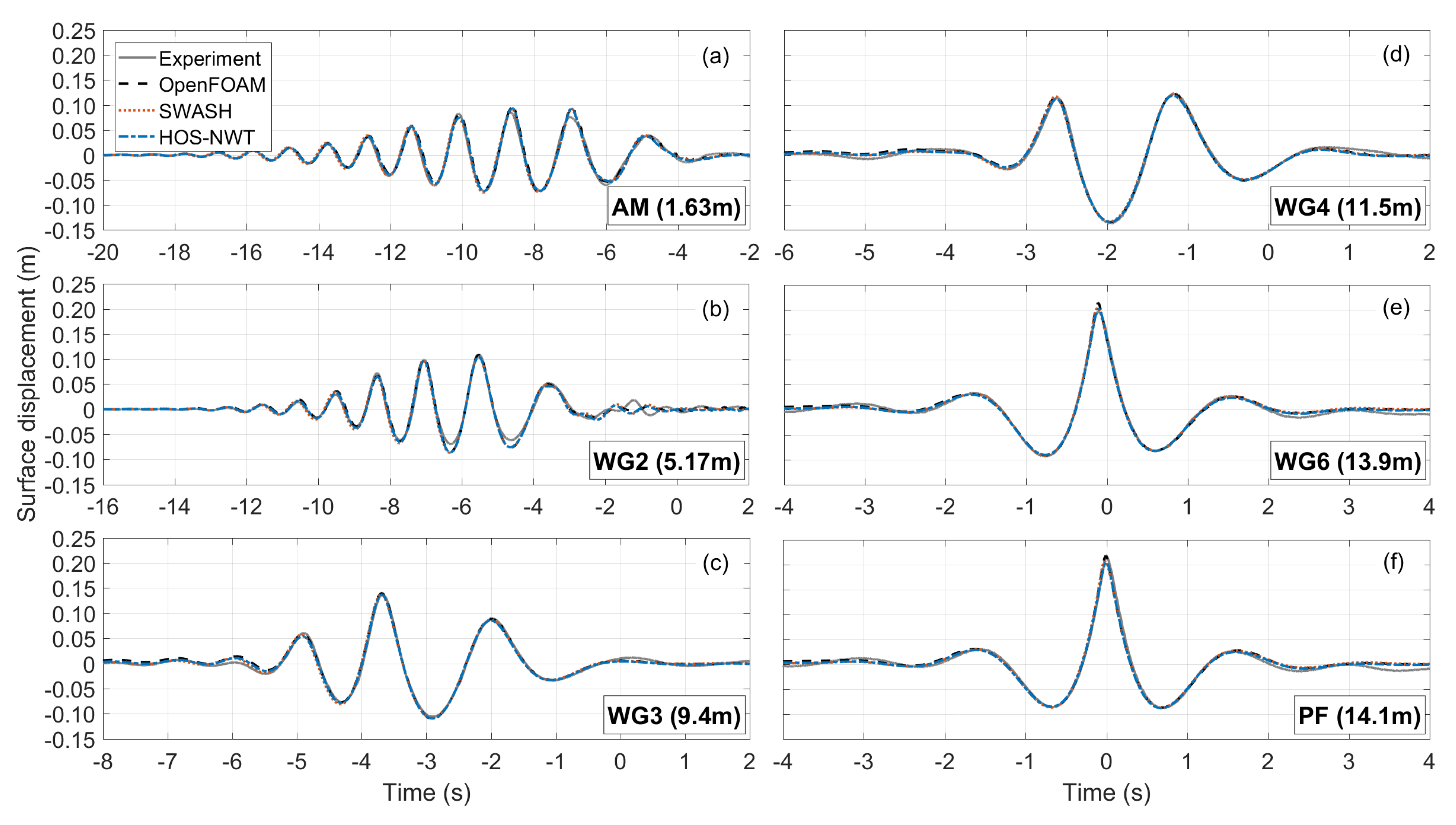

| WG1 (AM) | WG2 | WG3 | WG4 | WG5 | WG6 | WG7 (PF) |
|---|---|---|---|---|---|---|
| 1.63 | 5.17 | 9.40 | 11.50 | 13.80 | 13.90 | 14.10 |
| Gaussian Spectrum | |
|---|---|
| Peak frequency (fp) | 0.64 Hz |
| Standard Deviation (σ) | 0.13 |
| kpd | 1.75 |
| Linear crest amplitude ATh (m) | 0.154 |
| Boundary | |||
|---|---|---|---|
| Inlet | IH_Waves_InletAlpha | buoyantPressure | IH_Waves_InletVelocity |
| Outlet | zeroGradient | buoyantPressure | IH_3D_2DAbsorbtion_InletVelocity |
| Top | inletOutlet | totalPressure | presureInletOutletVelocity |
| Bottom | zeroGradient | buoyantPressure | fixedValue |
| Lateral walls | empty | empty | empty |
| NWT Parameters | OpenFOAM | SWASH | HOS-NWT |
|---|---|---|---|
| Equations | RANS | NLSWE | PFT |
| Mesh | quasi 3D static | 2D moving | 1D spectral |
| Wave generation | vel. distribution | vel. distribution | piston |
| Wavemaker motion | stationary | stationary | moving |
| Wave absorption | active | passive | passive |
| Length of NWT | 20 m | 30 m | 50 m |
| No. cells/nodes | 2.48 × 10 | 6 × 10 | 0.5 × 10 |
| Comp. cost (core hours) | 800 | 0.4 | 0.05 |
| Harmonics | Experiment | OpenFOAM | SWASH | HOS-NWT | |||
|---|---|---|---|---|---|---|---|
| Total (measured) | 217.5 | 0.2 | 0.1% | −10.5 | −4.8% | −12.5 | −5.7% |
| Linear | 158.5 | −3.3 | −2.1% | −4.1 | −2.6% | −5.3 | −3.3% |
| 2nd sum | 45.4 | 2.1 | 4.5% | −1.6 | −3.4% | −0.7 | −1.5% |
| 2nd difference | −25.9 | −3.8 | 14.8% | −0.5 | 1.8% | −2.5 | 9.7% |
| 3rd order | 21.7 | 1.5 | 6.7% | −1.6 | −7.3% | −1.8 | −8.1% |
| 4th order | 8.8 | 1.8 | 20.2% | 0.2 | 2.1% | 0.1 | 1.6% |
| 5th order | 5.3 | 1.4 | 26.1% | −0.2 | −3.8% | −0.3 | −4.8% |
| Sum of harmonics | 213.9 | −0.4 | 0.2% | −7.7 | −3.6% | −10.3 | −4.8% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vyzikas, T.; Stagonas, D.; Maisondieu, C.; Greaves, D. Intercomparison of Three Open-Source Numerical Flumes for the Surface Dynamics of Steep Focused Wave Groups. Fluids 2021, 6, 9. https://doi.org/10.3390/fluids6010009
Vyzikas T, Stagonas D, Maisondieu C, Greaves D. Intercomparison of Three Open-Source Numerical Flumes for the Surface Dynamics of Steep Focused Wave Groups. Fluids. 2021; 6(1):9. https://doi.org/10.3390/fluids6010009
Chicago/Turabian StyleVyzikas, Thomas, Dimitris Stagonas, Christophe Maisondieu, and Deborah Greaves. 2021. "Intercomparison of Three Open-Source Numerical Flumes for the Surface Dynamics of Steep Focused Wave Groups" Fluids 6, no. 1: 9. https://doi.org/10.3390/fluids6010009
APA StyleVyzikas, T., Stagonas, D., Maisondieu, C., & Greaves, D. (2021). Intercomparison of Three Open-Source Numerical Flumes for the Surface Dynamics of Steep Focused Wave Groups. Fluids, 6(1), 9. https://doi.org/10.3390/fluids6010009







