Three-Dimensional Simulation of New Car Profile

Abstract

Aerodynamics has identified remarkable development in the improvement of fuel efficiency, reducing wind noise and increasing engine cooling. Moving body profile controls fuel the consumption rate. This paper discusses a novel car profile consisting of two airfoils Roncz (car profile) and National Advisory Committee for Aeronautics NACA 10 (car sides). They are used to create a streamlined body. Three-Dimensional numerical simulations of the full scale model (half domain) are performed to examine the effect of car profile on the drag coefficient and thus fuel consumption. Simulations are considered over a range of air flow velocities, from 20 to 45 km/h in a step of 5 km/h. The ahmed body is used to validate the results. Results are shown graphically for coefficients of drag and lift and pressure and velocity contours. They show that the design of the car profile is effective.

1. Introduction

Aerodynamics is the most important factor when it comes to resistive forces [1]. Reducing the aerodynamic drag will not only open the door for higher top speed, but will also reduce the overall fuel consumption of the vehicle and increase comfort. The fuel consumption rate can be controlled by profiles of high-speed trains, real cars and racecars. Streamlined profiles can reduce fuel consumption dramatically.

Starting with Airplanes, they have a major role in aerodynamics research. Recently, Prasad and Rose [2] completed an experimental and computational study of ice accretion effects on aerodynamic performance. Ice accumulation changes the shapes of local airfoil sections and consequently affects the aerodynamic performance characteristics of the considered National Advisory Committee for Aeronautics (NACA 23012) profile.

In competition with airplanes, the recent development of high-speed trains led to a growing interest in aerodynamics. Tan et al. [3] conducted transient numerical simulations of maglev trains of different lengths. Luo et al. [4] established a slide rail high-speed train model by combining the aerodynamic experiments with a standard k-ε numerical simulation. Their simulations were able to predict the behavior of compression waves and to validate the model at a low cost. Liang et al. [5] reported aerodynamic loads on the overhead bridge bottom surface as a result of the train passing.

Studying air flow around ground vehicles is of great importance in the automobile industry. Implementing good aerodynamic design under technical constraints requires a broad understanding of the flow phenomena, especially how the aerodynamics is influenced by changes in body shape. Consequently, vehicle optimization occurs as a part of the design process, typically in an effort to enhance desirable aerodynamic characteristics. One obvious way to improve fuel economy for vehicles is to reduce aerodynamic drag by optimizing body shape. Ahmad et al. [6] proposed a mesh optimization strategy for accurately estimating the drag of a ground vehicle. They examined the effect of different mesh parameters. Their study was extended to take into account the effect of model size. Scaling the optimized mesh size with the length of car model was successfully used to predict the drag of the other car sizes with reasonable accuracy. Aljure et al. [7] carried out numerical simulations on the flow over a realistic generic fastback car. Pure Large Eddy Simulations (LES) and Wall-Modeled Large Eddy Simulations (WMLES) were used and compared to numerical and experimental results to assess the validity of these approaches when solving the flow field around complex automotive geometries. Their investigation showed how WMLES helped reduce computing cost and response versus pure LES, while providing high-quality unsteady data, although computational cost remained high. Fu et al. [8] Introduced turbulence modeling effects on Reynolds-averaged Navier–Stokes (RANS) Computational Fluid Dynamics (CFD) simulations of a full-scale “NASCAR Gen 6 Cup” car using one of the latest low Reynolds k-ε model, i.e., the one developed by Abe-Nagano-Kondoh (AKN), realizable k-ε and SST k-ω. Their simulation results suggested that the turbulence modeling effects were mainly marked in the recirculation and separated regions. Clearly, more exact simulations of the wake flow and of the separation process were essential for the accuracy of drag predictions. In general, AKN model appeared to be superior to the other two models. Its results better aligned with wind tunnel data in terms of drag, total downforce and front-to-rear vehicle balance. Moreover, Thangadurai et al. [9] examined the effect of added surfaces such as NACA 2412 wings and wedge type spoiler at the rear end of a sports car in detail using three-dimensional realizable k-ε turbulence model numerical simulations validated with lab scale experiments. Czyż et al. [10] performed numerical calculations using Ansys to identify the magnitude of the aerodynamic drag force generated on individual elements of a high energy efficiency vehicle body. Mariani et al. [11] investigated using CFD in the racecar from the University of Perugia.

At the Shell Eco-marathon competition [12], competitors demonstrated the variation in wake flow field of vehicles with different added surfaces using pressure and velocity contours, velocity vectors at the rear end and the turbulent kinetic energy distribution plots. Their simulations results were validated by experiments. Arpino et al. [13] designed their car with detailed 3D CFD modeling and then confirmed it against experiments. A model of the car was examined in an open wind tunnel. They evaluated the wake flow structures and estimated the drag coefficient. Cieslinski et al. [14] focused on optimizing their car body profile. They presented a numerical aerodynamic study of a number of vehicle shapes with fairing around its wheels inspired by the winning models. Ambarita et al. [15] numerically designed a prototype to participate in the energy-efficient competition. In their results, drag and lift coefficients were determined and velocity and pressure distributions were provided. Abo-Serie et al. [16] numerically proposed a low drag concept car with a non-rotating wheel using a modified “tear drop” shape.

New methods have appeared to minimize drag; active and passive flow controls are a promising way of drag reduction. Brunn et al. [17] tested two different configurations that had separate control approaches experimentally and numerically. Their results showed that targeted excitation of the dominant structures in the wake region leads to their effective attenuation. Fourrie’ et al. [18] experimentally studied passive flow control on a generic car model. Their control consisted of a deflector placed on the upper edge of the model rear window. The aerodynamic drag was measured using an external balance and calculated using a wake survey method. Drag reductions up to 9% were obtained depending on the deflector angle.

More than that, Dawi and Akkermans studied direct noise of two square cylinders [19], for automotive applications [20] and of a generic vehicle [21]. They [19] presented a comparison between two different approaches to calculate the far-field noise of two square cylinders in tandem arrangement. Then they [20] used a compressible flow solver for low Mach-number flows utilized with an IDDES approach for turbulence modeling. Furthermore, they [21] demonstrated the applicability of a finite volume method for the direct noise computation of road vehicles.

To meet the high records expectations of the competition, it’s decided to improve the car profile. The aim of this study is to reach the lowest coefficient of drag by using the chosen profile of the two airfoils (Roncz and NACA 10). The reason to choose these two airfoils is to minimize the drag and hence fuel consumption and to reach a higher C_L/C_D ratio. Based on that, numerical simulations are used to show the distribution of pressure and velocity and coefficients of lift and drag of the tested shape.

2. Physical Model

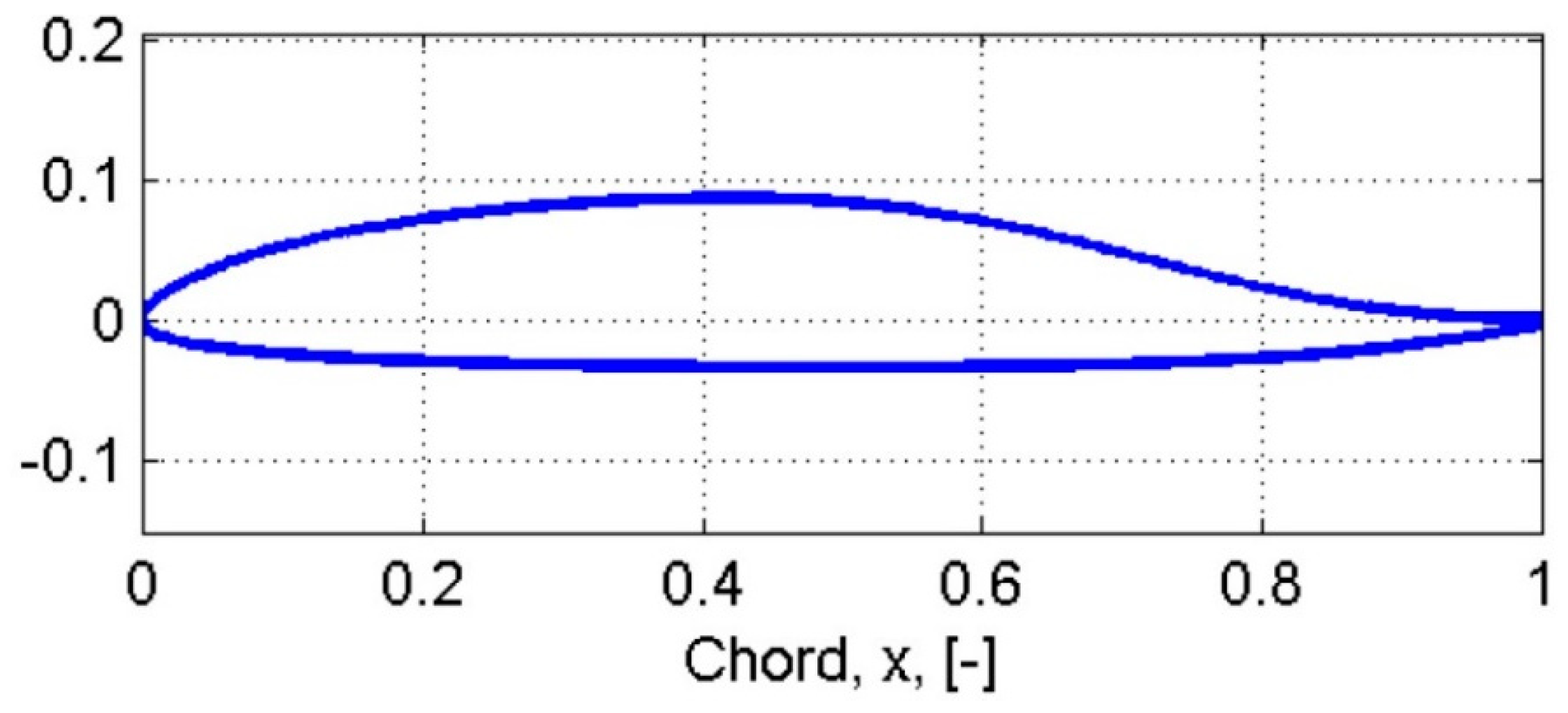
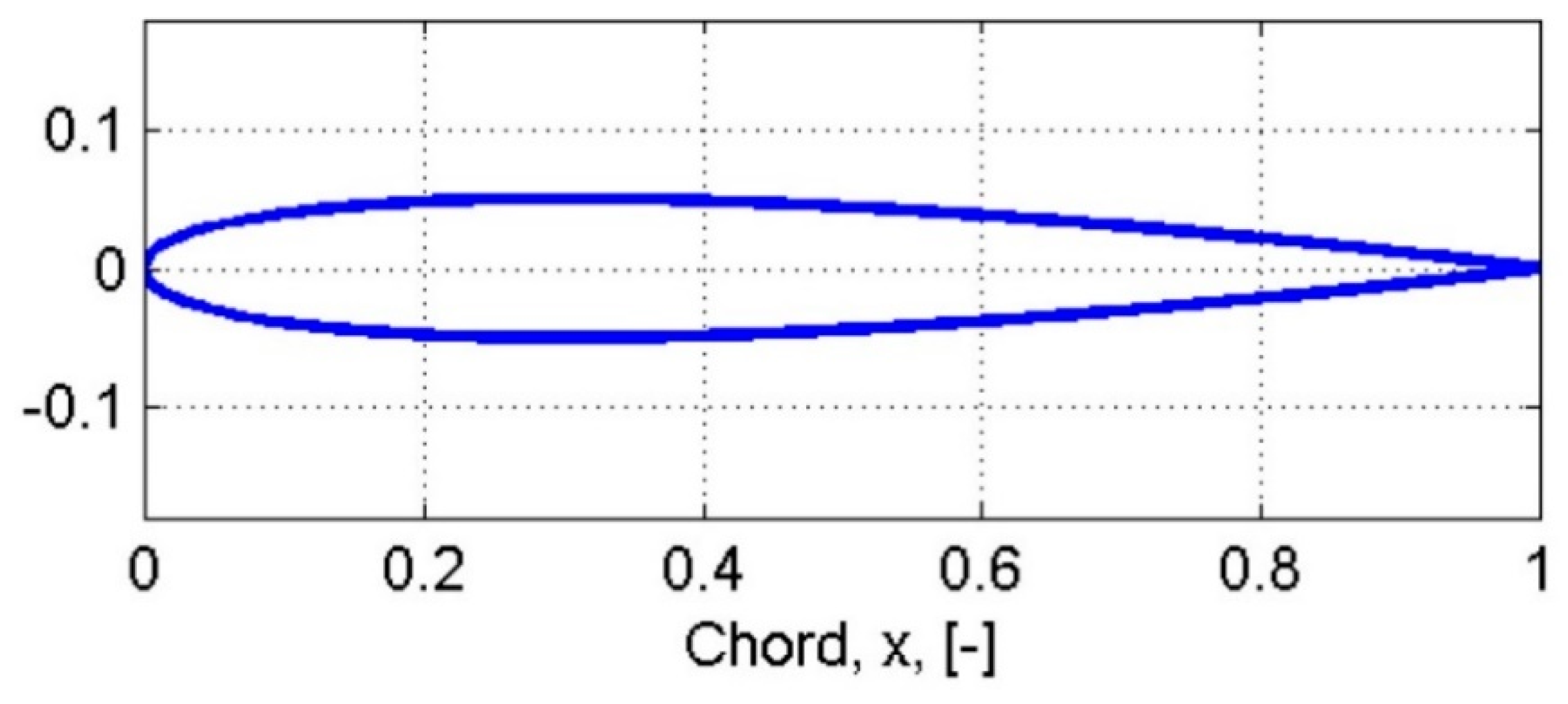
The car is designed using two airfoils (Roncz and NACA 10 [22]), shown in Figure 1 and Figure 2.

Table 1. Roncz geometrical properties [22].

Maximum Thickness	12.0605	% @0.43C
Maximum Camber	2.7931	% @0.42C
Trailing Edge Gap	0.40336	%C
Upper nose radius	1.0122	%C
Lower nose radius	21.7485	%C
Boat-Tail Angle	10.1132	deg
Release Angle	−5.276	deg
Nose Incidence	8.4245	deg
Camber Deflection	3.1485	deg

and

Table 2. NACA10 geometrical properties [22].

Maximum Thickness	9.8434	% @0.3C
Maximum Camber	0	% @1C
Trailing Edge Gap	0	%C
Upper nose radius	1.2071	%C
Lower nose radius	1.2071	%C
Boat-Tail Angle	14.3239	deg
Release Angle	0	deg
Nose Incidence	0	deg
Camber Deflection	0	deg

show the geometrical properties of Roncz and NACA10, respectively. Roncz is used to design the top section, Figure 3. Only the front part of this airfoil is used to give the driver a comfortable and enough space to be able to drive the car. Moreover, NACA 10 Airfoil is used to design the side sections, Figure 4. This combination of the two airfoils avoids any discontinuity; hopefully, it reduces the coefficient of drag.

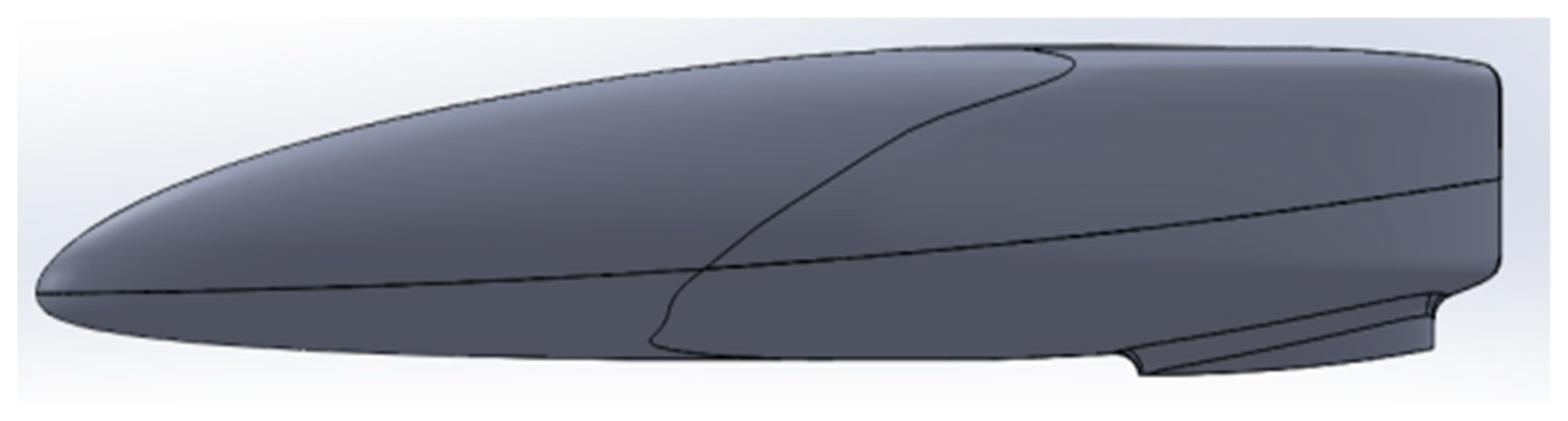
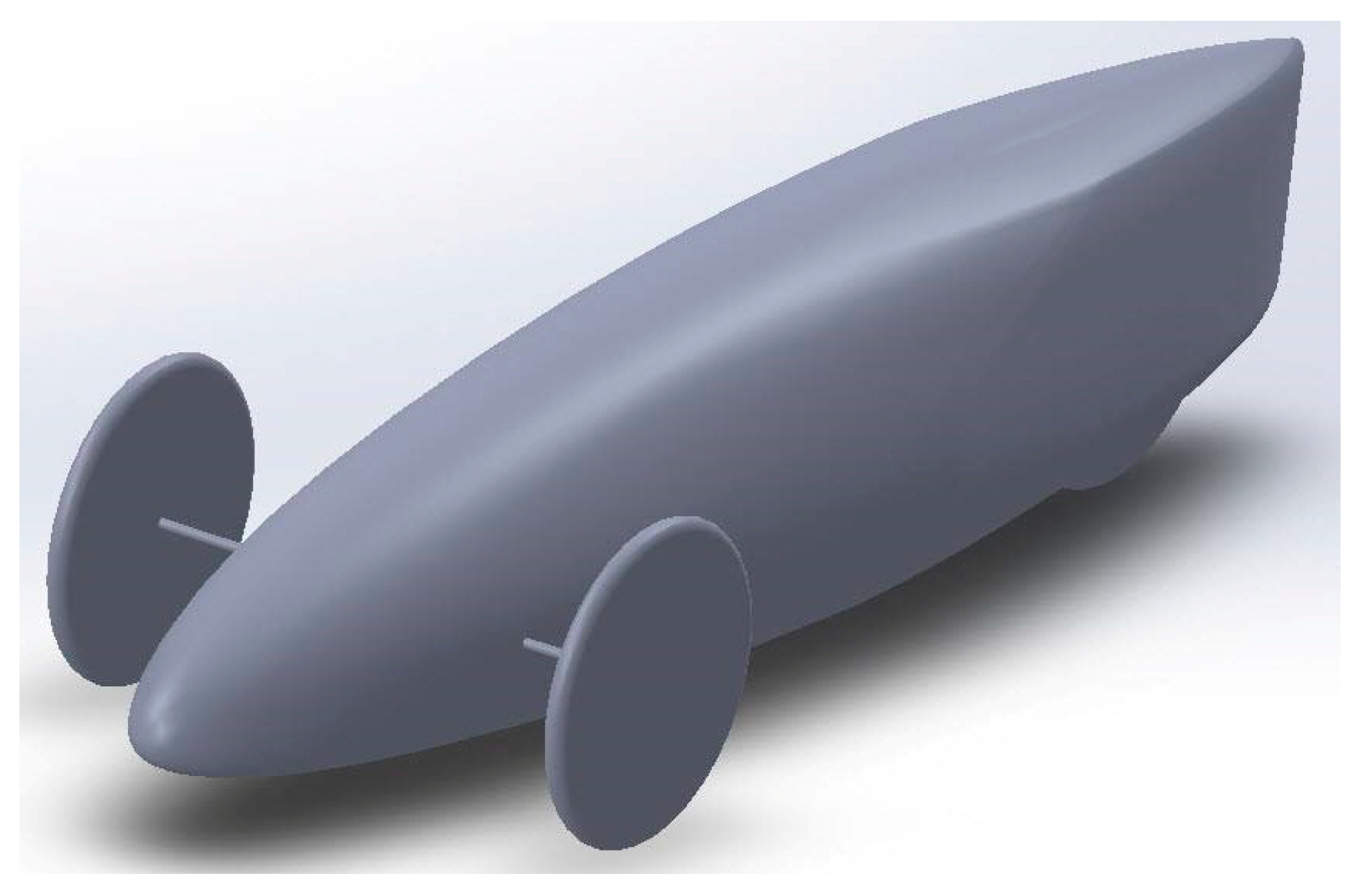
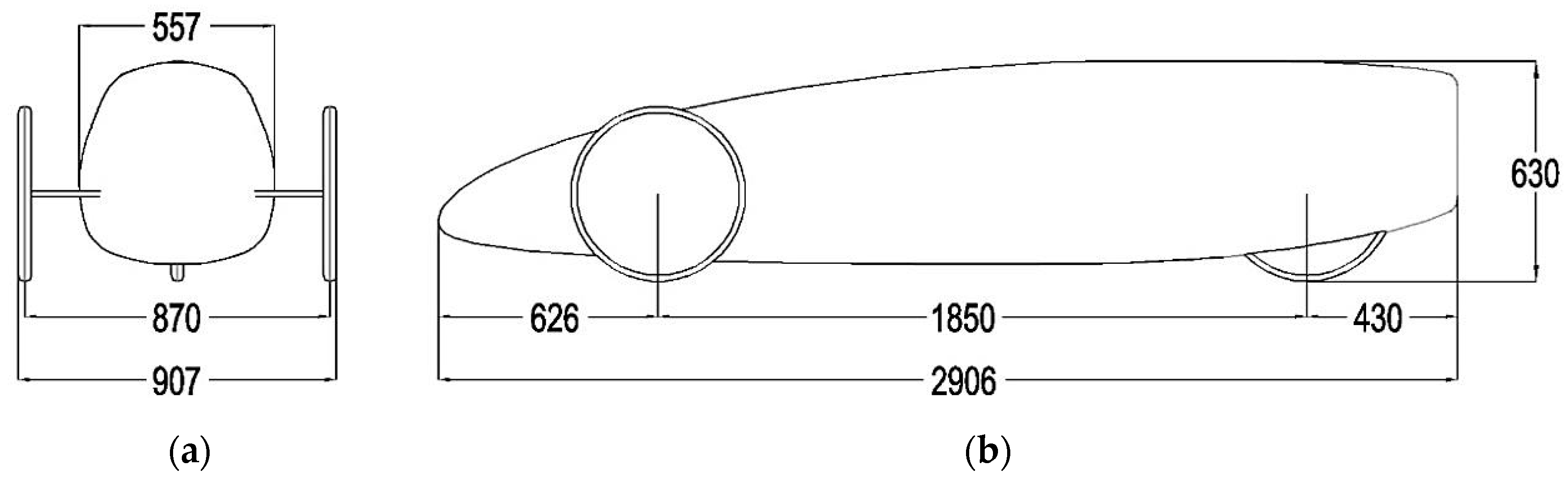
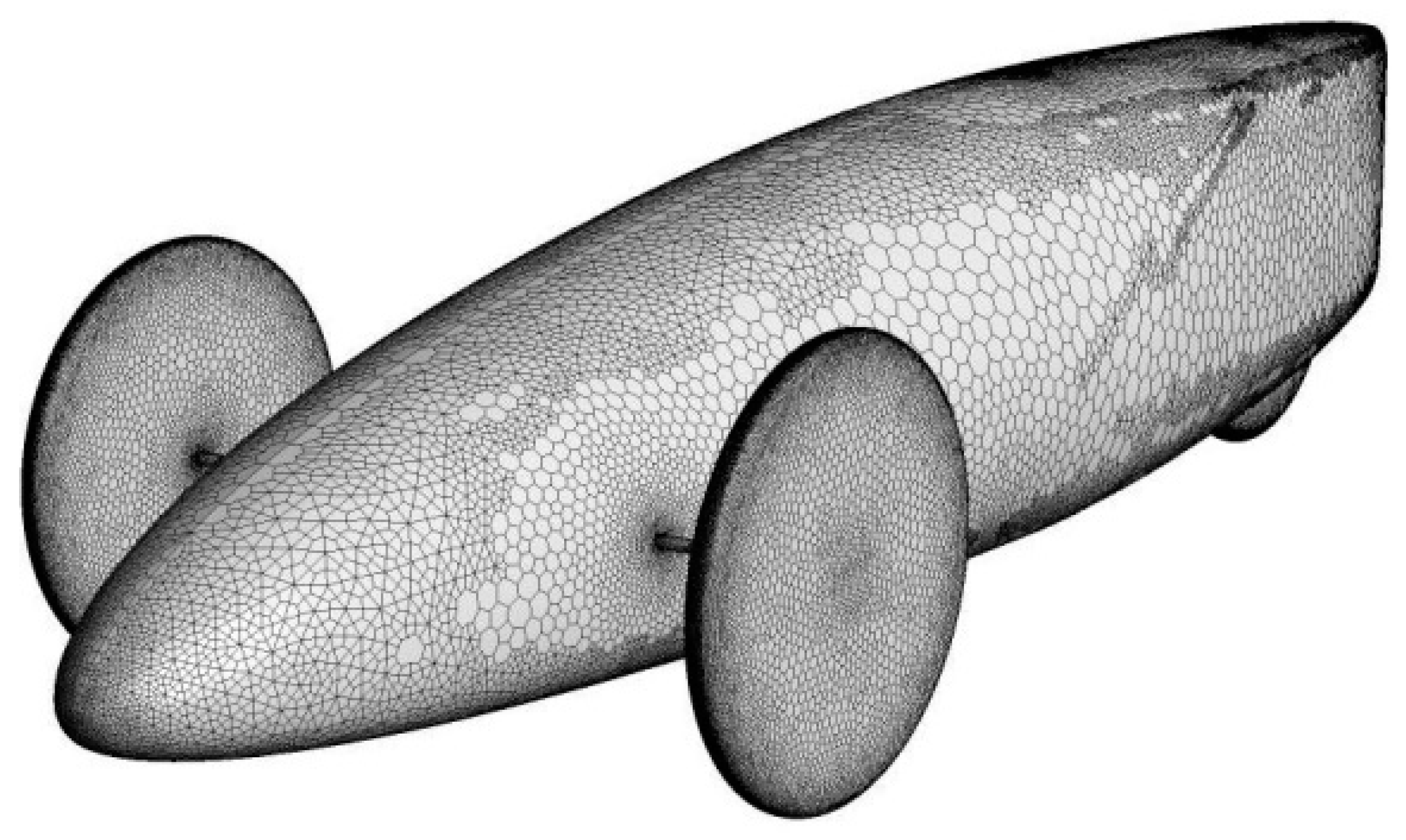
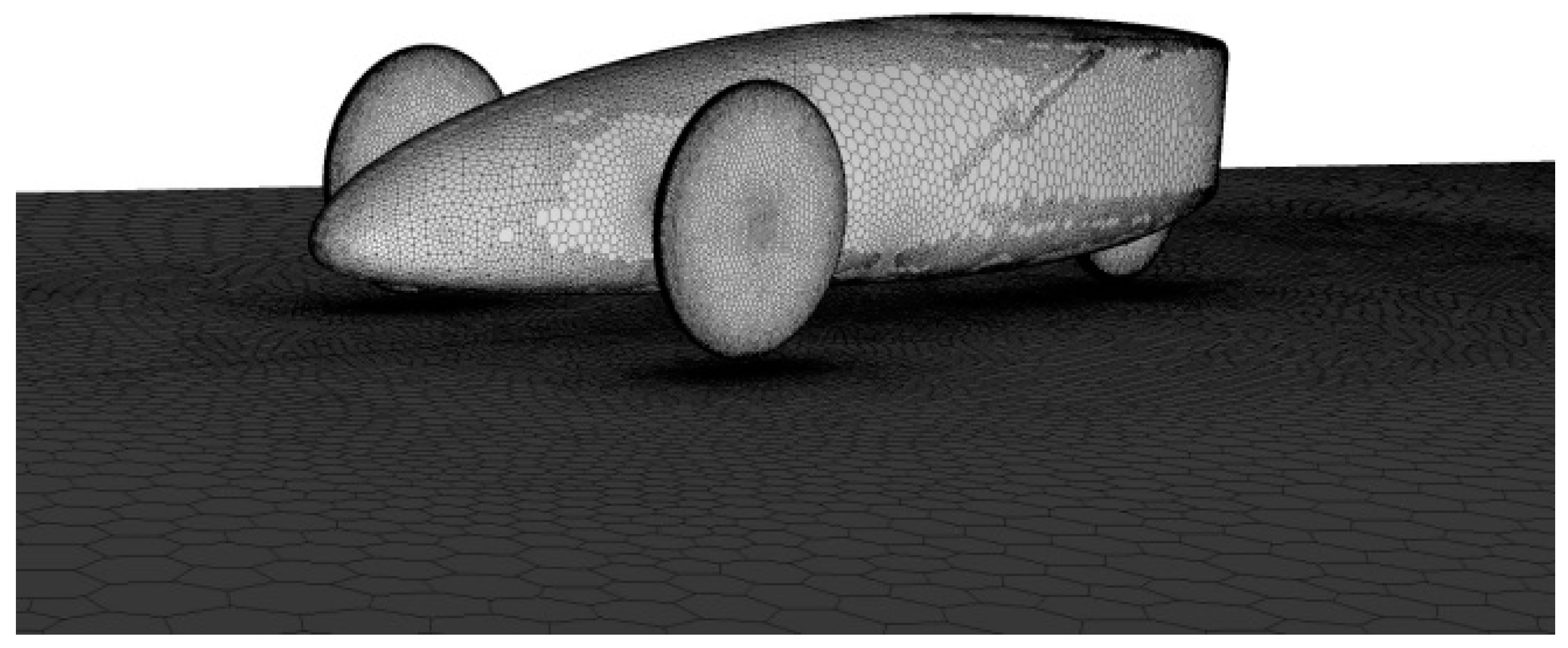
The car design, shown in Figure 5, is a Computer Aided Design (CAD) drawing model. Its front tires are covered with fairing. This made the car body more streamlined. The car has only three tires (two at front and one at rear) to decrease friction losses.

Figure 6 shows front and side views of the designed car. It has a maximum width, length and height of 907, 2906 and 630 mm, respectively. This car design has a frontal area = 0.2855 m².

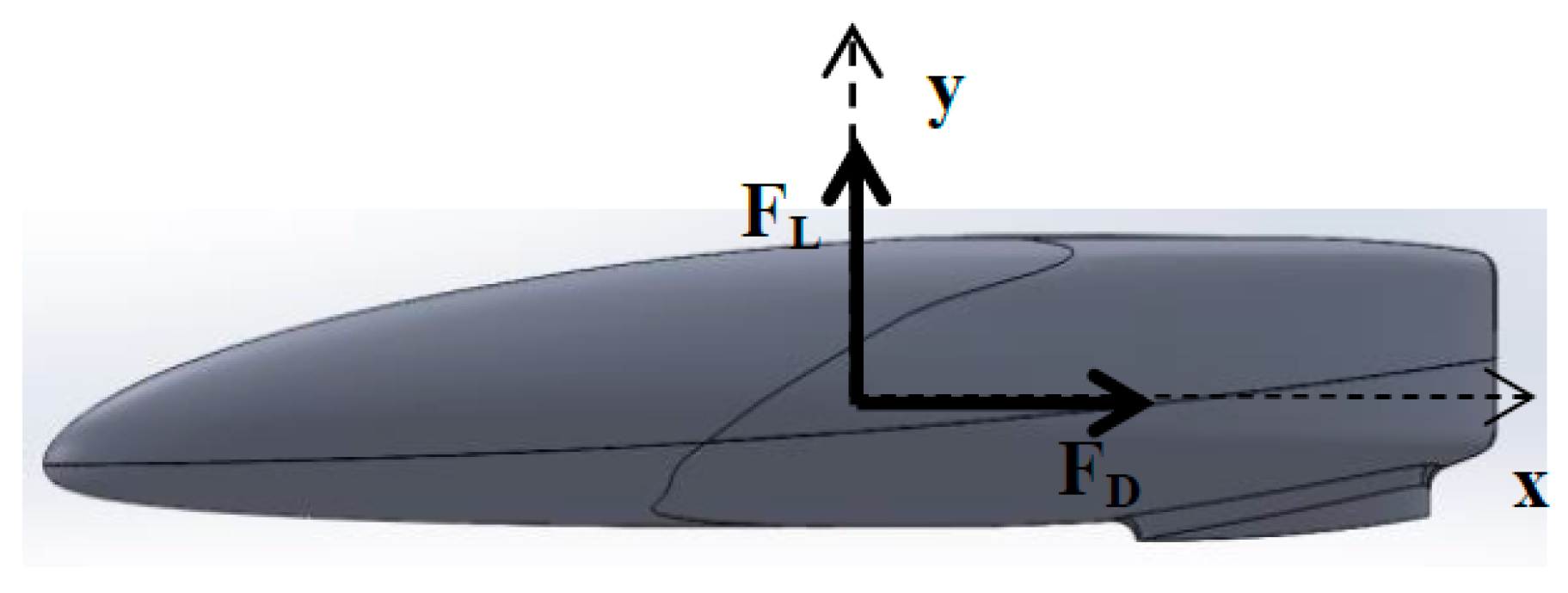
Drag force is one of the aerodynamic forces. It is the resistance force applied along the velocity vector, Figure 7. Lift force performs perpendicular to the velocity vector, see Figure 7.

The coefficients of drag (C_D), lift (C_L) and pressure (C_P) are given by the following equations:

$${\mathrm{C}}_{\mathrm{D}}={\mathrm{F}}_{\mathrm{D}}/\frac{1}{2}\mathsf{\rho }{\mathrm{V}}^2{\mathrm{A}}_{\mathrm{D}}$$

(1)

$${\mathrm{C}}_{\mathrm{L}}={\mathrm{F}}_{\mathrm{L}}/\frac{1}{2}\mathsf{\rho }{\mathrm{V}}^2{\mathrm{A}}_{\mathrm{L}}$$

(2)

$${\mathrm{C}}_{\mathrm{P}}=\mathsf{\Delta }\mathrm{P}/\frac{1}{2}\mathsf{\rho }{\mathrm{V}}^2$$

(3)

Aerodynamic drag created on the car body affects fuel consumption [23,24,25]. Modifications of car’s geometry can improve the flow around the car and reduce the aerodynamic drag. A relation between change in fuel consumption and change in drag coefficient is shown as follows [26]:

$$\frac{\mathsf{\Delta }\mathrm{FC}}{\mathrm{FC}}=\mathsf{\eta }\times \left(\frac{{\mathsf{\Delta }\mathrm{C}}_{\mathrm{D}}}{{\mathrm{C}}_{\mathrm{D}}}+\frac{{\mathsf{\Delta }\mathrm{A}}_{\mathrm{f}}}{{\mathrm{A}}_{\mathrm{f}}}+3\frac{\mathsf{\Delta }\mathrm{U}}{\mathrm{U}}\right)$$

(4)

3. Numerical Model

Test conditions are set as similar to real working conditions. Simulations of air flow (an ideal gas) around the car model are conducted using Ansys. The car wheel is assumed to be fixed in this study.

While all flows are compressible, flows are usually treated as incompressible when the Mach number (the ratio of the speed of the flow to the speed of sound) is smaller than 0.3 (since the density change due to velocity is about 5% in that case) [27]. For compressible flows, if the Mach number is less than 0.3, the flows are usually treated as incompressible since the density change due to the velocity is about 5%. However, for standard air at a temperature of 15C, the speed of sound is 340 m/s. For a Mach number of 0.3, the flow velocity will be 102 m/s. The studied velocity ranges from 20 to 45 km/h (5.6 to 12.5 m/s). However, this is assuming that the incompressible flow is valid.

3.1. Domain Dimensions

The numerical domain is included in the Wind Tunnel space, excluding the car, as shown in Figure 8. Due to the symmetry of the car, it was assumed that the flow on both sides was identical and only half of the model was to be used for the simulation. That assumption had a great impact on the mesh size, simulation time and computational domain shape. The upstream, downstream, height and width dimensions are 1CL, 16CL, 3.5CH and 3CW, respectively, as CL, CH and CW are the car length, height and width. The domain overall dimensions have not been tested; they were taken from references [14,15,16]. However, the dimensions effect is minimized if a distance three times the car height is allowed above and on the two sides of the car [16].

3.2. Boundary Conditions

Boundary conditions are shown in Figure 8 and

Table 3. Boundaries names and conditions.

a	Inlet	Velocity inlet (varied from 20 to 45 km/h)
b	Outlet	Pressure outlet (0 Pa)
c	Top	Pressure outlet (0 Pa)
d	Mid-vertical plane	Symmetry
e	Bottom/road	Moved wall in x-direction = velocity inlet
f	Car surface	Wall
g	Right side	Pressure outlet (0 Pa)

. The left boundary is defined as the inlet. The air flow has a range of velocities from 20 to 45 km/h in a step of 5 km/h. An outlet pressure boundary condition is assumed at the right side and at the top boundaries. The mid-vertical plane is assumed as a symmetry boundary condition. Other boundaries, including the car, are considered as walls.

3.3. Domain Meshing

Polyhedral mesh [6] is used to provide better resolution than other mesh types. It can capture the flow in the wake region with fewer cells, however less computational time and memory is needed. Having a fine mesh around the vehicle could capture flow features and use less memory and time than having a fine mesh all over the domain. To ensure a mesh size that does not affect the final results, a mesh independency study has been carried out. Figure 9 shows the relation between the coefficient of drag and number of elements at a velocity of 35 km/h. The number of elements 3 × 10⁶, 6.5 × 10⁶ and 8 × 10⁶ are used. Numbers of elements of 6.5 × 10⁶ and 8 × 10⁶ almost have the same coefficient of drag.

To be more accurate, velocity and pressure profiles are considered at the back of the car at X/CL = 1.03, see Figure 10a, for the same numbers of elements 3 × 10⁶, 6.5 × 10⁶ and 8 × 10⁶. Figure 10b,c shows the relation between velocity and pressure profiles and the number of elements at a velocity of 35 km/h. Moreover, the number of elements of 6.5 × 10⁶ and 8 × 10⁶ have almost the same velocity and pressure profiles. Based on that, the number of elements 6.5 × 10⁶ is used as iterations have residuals less than 10⁻⁴.

Meshing is an important part of the CFD set up in order to capture the flow details, as shown in Figure 11 and Figure 12. Two regions within the computation domain are defined (hybrid mesh), as shown in

Table 4. Properties of the domain’s mesh.

Used meshing software	ANSYS WB meshing
Domain description	Unstructured mesh with body of influence
Meshing method	Hybrid of polyhedron mesh and inflating prisms around the airfoil
Maximum skewness	0.67
Size functions	Proximity curvature
Total mesh count	6.5 × 105
Inflation method	First layer thickness calculated from desired y+ value of 1
Free-stream velocity	35 km/h

and Figure 13 and Figure 14. Quad elements are layered at the car walls to reach the required y+ value. The far-field mesh was set to be unstructured polyhedral elements. A polyhedral mesh is applied to the airflow domain as it can easily fit with different geometries, as shown in Figure 15.

Table 4 describes the properties of domain’s mesh. The used meshing method is a hybrid of polyhedron mesh and inflating prisms around the airfoils. A good mesh is achieved with a maximum skewness of 0.67 and a maximum aspect ratio of 286.

First layer thickness of 0.01 mm can be calculated from y+ of 1 using Equation (5) [28].

$${\mathrm{y}}^{+}=\frac{{\mathrm{u}}_{*}\mathrm{y}}{\mathsf{\nu }}.$$

(5)

3.4. Shear-Stress Transport (SST k-ω) Model

Computational Fluid Dynamics techniques are used to numerically solve the governing equations of a flow field to estimate flow variables. It is essential to define two equation sets: Navier-Stokes momentum and mass conservation equations, hereafter, introducing the problem of turbulence modeling. Once these models are defined, they are solved by ANSYS FLUENT [29]. All the simulations are configured in steady state and with an incompressible model. As demonstrated by the specialized literature [30,31,32] and among the various models tested, SST k-ω is supreme for capturing complex turbulent phenomena in terms of displacement from experimental evidence.

The k-ε model [30,31] is one of the most widely used turbulence models. It is a two-equation model; it includes two transport equations that represent the turbulent properties of the flow. The two equations take into account both the convective effect and the diffusion effect associated with the turbulent energy. The first transported variable is the turbulent kinetic energy k, which governs the energy in turbulence, while the second variable is the specific dissipation ε, which governs the scale of the turbulence.

SST k-ω turbulence model is a two-equation eddy-viscosity model. Shear Stress Transport (SST) formulation merges the best of the two models. The use of a k-ω formulation in the internal parts of the boundary layer creates a usable model at the wall through the viscous sub-layer. Hereafter, it is used as a Low-Re turbulence model without adding any additional damping functions. Moreover, SST formulation changes a k-ε behavior in the free-stream and avoids the sensitivity of k-ω model to the inlet free-stream turbulence properties. This approach effectively blends the far-field K-ε model with the near-wall K-ω model. Finally, SST k-ω model has good behavior in adverse pressure gradients and in separating flow conditions. The transport equations [33] for SST k-ω model are:

Turbulence kinetic energy equation:

$$\frac{\partial k}{\partial t}+\overline{u_j}\frac{\partial k}{\partial x_j}=P_k-{\beta }^{*}k\omega +\frac{\partial }{\partial x_j}\left[\left(\upsilon +{\sigma }_k{\upsilon }_T\right)\frac{\partial k}{\partial x_j}\right]$$

(6)

Specific dissipation rate equation:

$$\frac{\partial \omega }{\partial t}+\overline{u_j}\frac{\partial \omega }{\partial x_j}=\alpha S^2-\beta {\omega }^2+\frac{\partial }{\partial x_j}\left[\left(\upsilon +{\sigma }_w{\upsilon }_T\right)\frac{\partial \omega }{\partial x_j}\right]+2\left(1-F_1\right){\sigma }_{w2}\frac{1}{\omega }\frac{\partial k}{\partial x_i}\frac{\partial \omega }{\partial x_i}$$

(7)

Kinematic eddy viscosity equation:

$${\upsilon }_T=\frac{{\alpha }_{1}k}{\max \left({\alpha }_1\omega ,S{F}_2\right)}$$

(8)

The chosen fluid model for computation comprises air at 15C and a pressure of one atmosphere, isothermal heat transfer, and turbulent flow model.

4. Numerical Validation

For this turbulent steady flow, simulation run about 1000 iterations to reach steady state conditions and the residuals reach a value less than 10⁻⁴, as shown in Figure 16.

In the present work, Ahmed body (of the same size) is used as a reference to validate the present numerical simulation. The Ahmed body has a very simple shape that allows capturing characteristic features that are relevant to bodies in the automobile industry. The present model is used to compute drag coefficients of the Ahmed Body and drag coefficient of the present model.

Table 5. Comparison between coefficient of drag for the Ahmed body from previous (Experimental and Simulation) and present studies.

Comp.	Exp. Work [34]	Num. Work [34]	Num. Work [15]	Present Work
CD	0.2300	0.2335	0.239034	0.2381
Discrepancy	-	1.52%	3.93%	3.52%

shows the coefficient of drag for the Ahmed body from previous and present studies. The results of the present numerical method agree well with previous work. To check the results, discrepancy can be calculated from the following equation:

$$\mathrm{discrepancy}=\frac{{\mathrm{C}}_{{\mathrm{d}}_{\mathrm{present}}}-{\mathrm{C}}_{{\mathrm{d}}_{\mathrm{Exp}.}}}{{\mathrm{C}}_{{\mathrm{d}}_{\mathrm{Exp}.}}}$$

(9)

The discrepancy to the experimental work is only 3.52%. Thus, the present method will perform an efficient numerical analysis.

5. Results

Numerical simulation results provide parameters; coefficients of drag, lift and pressure and distributions of velocity and pressure, to declare whether the car profile is efficient or not.

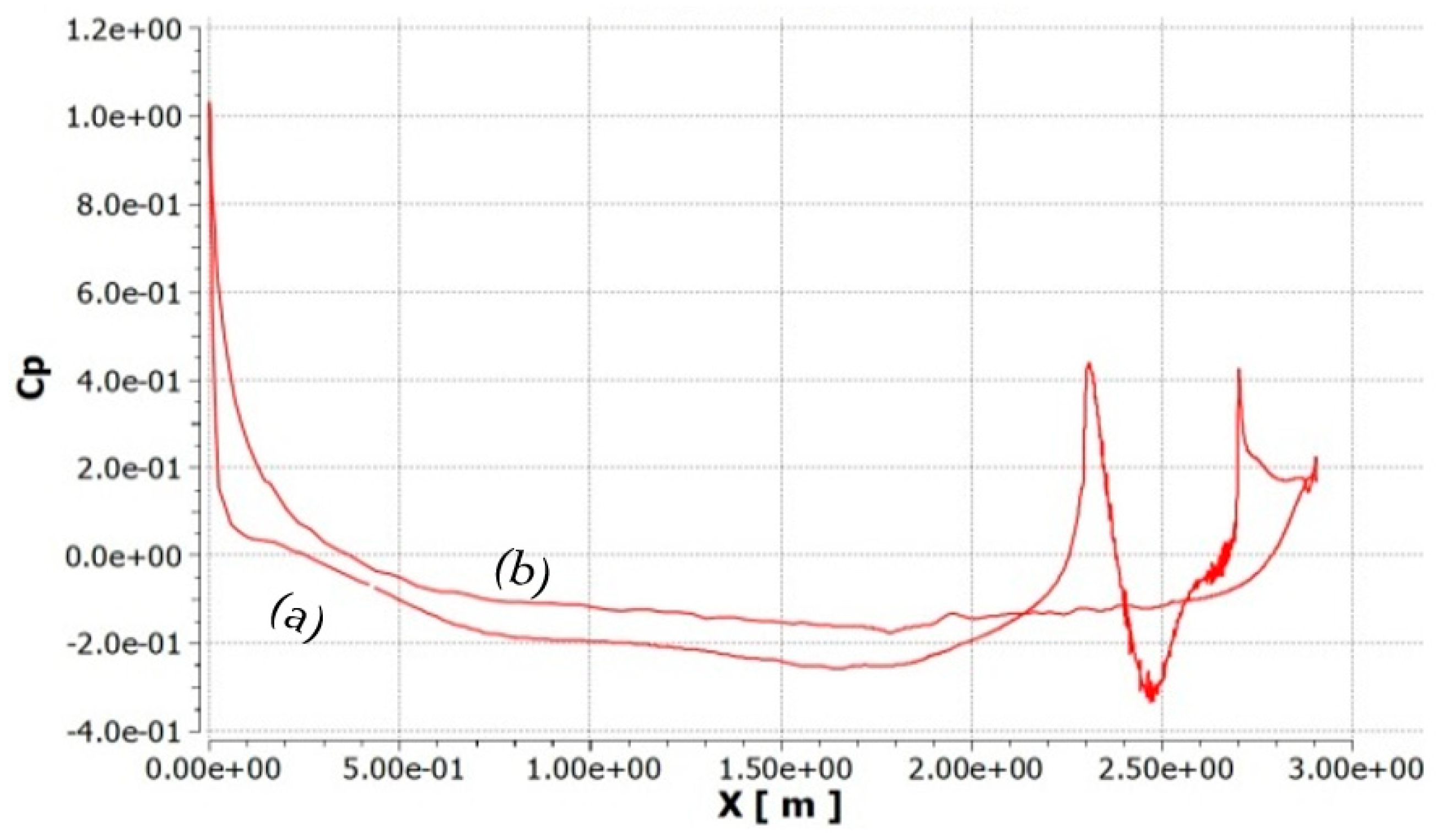
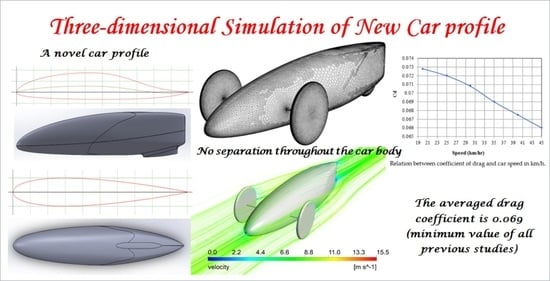
Drag coefficient is calculated based on integration of pressure and shear stress on the car surface. Relation between coefficient of drag and car speed in km/h is shown in Figure 17. Coefficient of drag decreases slightly from 0.073 to 0.066 as the car speed increases over the range of velocity. The decrease in coefficient of drag is mostly due to low frontal length and the flow is always associated with the car body.

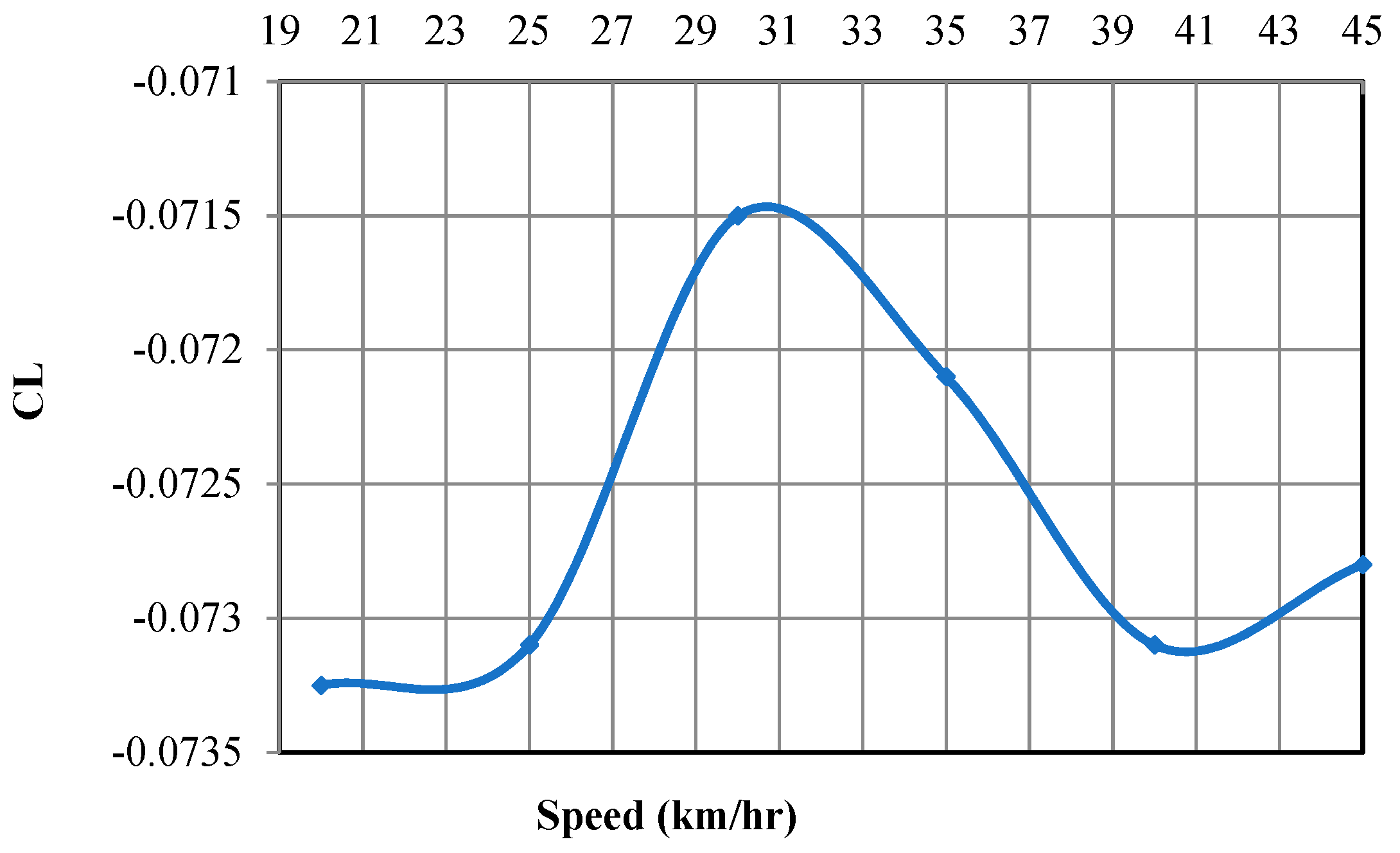
Relation between coefficient of lift and car speed in km/h is shown in Figure 18. The coefficient of lift increases as the car speed increases from 20 to 30 km/h and then decreases from 30 to 40 km/h. C_L is a measure of the difference in pressure created above and below a car’s body as it moves through the surrounding viscous air. The slight change in the coefficient of lift value from −0.0733 to −0.0715 is due to small viscous effects of air.

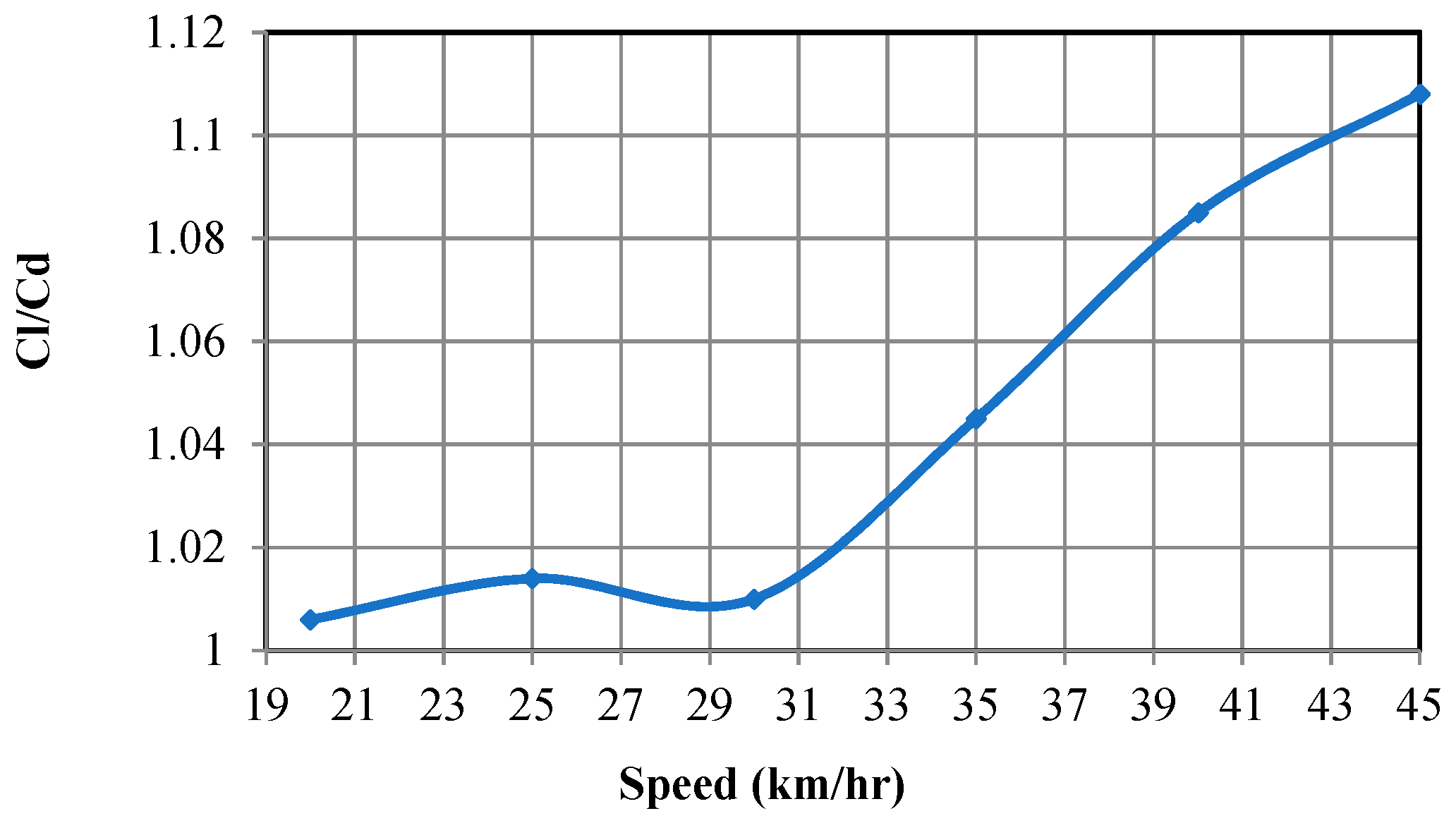
Lift to drag ratio, in aerodynamics, is the amount of lift produced by a vehicle divided by the drag created by movement through the air. As shown in Figure 19, Cl/Cd is almost unified until the car reaches a speed of 30 km/h, after which the ratio increases linearly. This may be due to an almost constant coefficient of lift and increases the coefficient of drag.

Figure 20 shows the pressure coefficient distribution at the middle vertical (x-y) plane of the car. The upper curve represents the bottom surface of the car and the lower curve represents the top one. Sudden changes in pressure are detected on the rear of the car. It can be observed that the positive pressure in front of the car is larger than that at the rear of the car due to some losses.

Air flow velocity and Pressure distributions on the car surface allow for the identification of the largest flow disturbances and the location of the highest pressure. Results of air velocity and pressure contours at a speed of 35 km/h (equals to 9.72 m/s) are shown in Figure 21, Figure 22, Figure 23, Figure 24 and Figure 25.

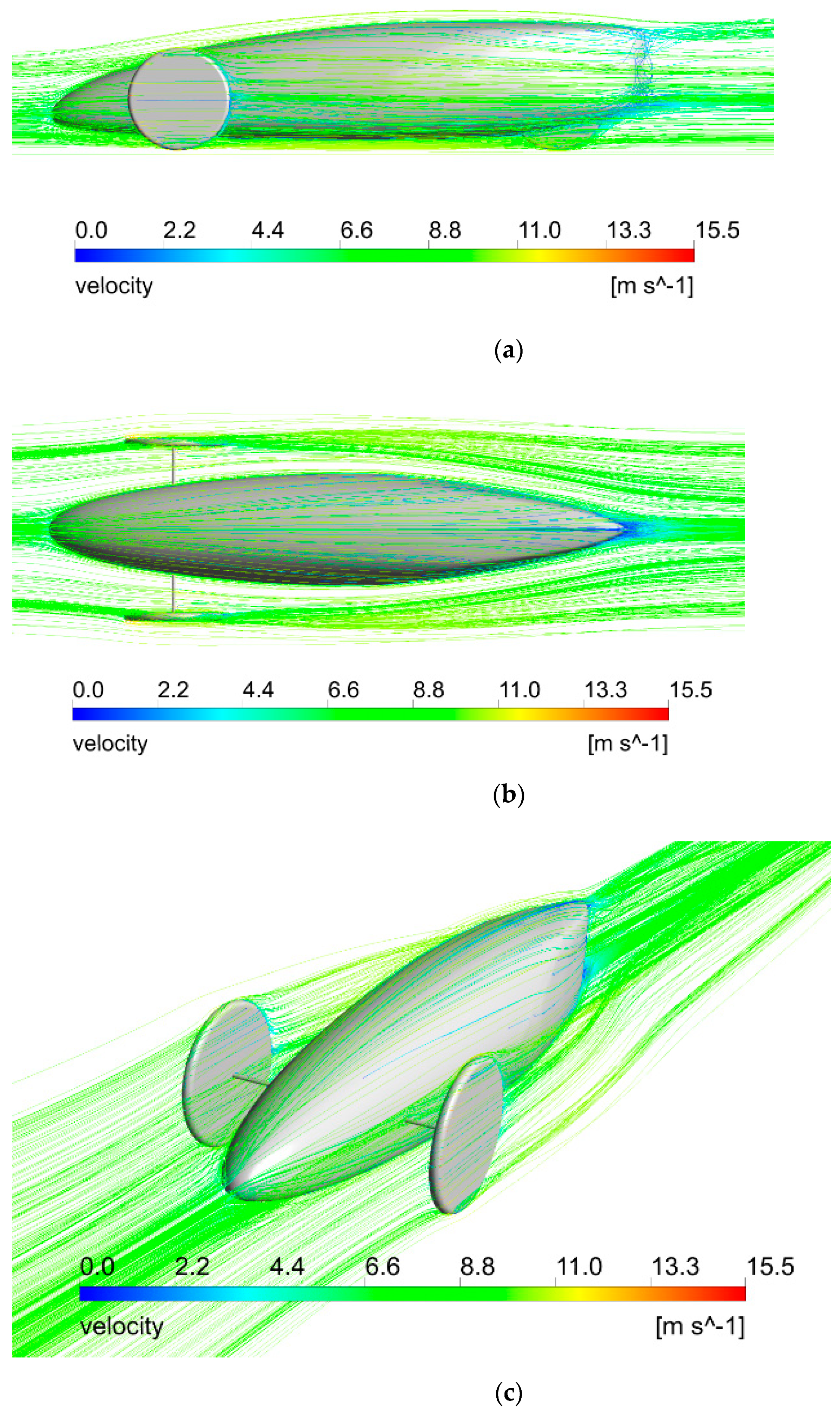
Figure 21, Figure 22 and Figure 23 display air flow velocity distribution around the car. A quite clean pattern appears and smooth velocity distribution lines are successfully achieved. It shows a good aerodynamic performance. The color of the streamlines shows a typical expected increase in speed as it passes on the top of the car surface. The airflow pattern on the side of the car also shows no evidence of flow separation on a large scale. At the rear of the car, the airflow is smoothly attached to the car body and joins the main airflow stream, eliminating the flow separation zone. The flow shows no separation throughout the car body.

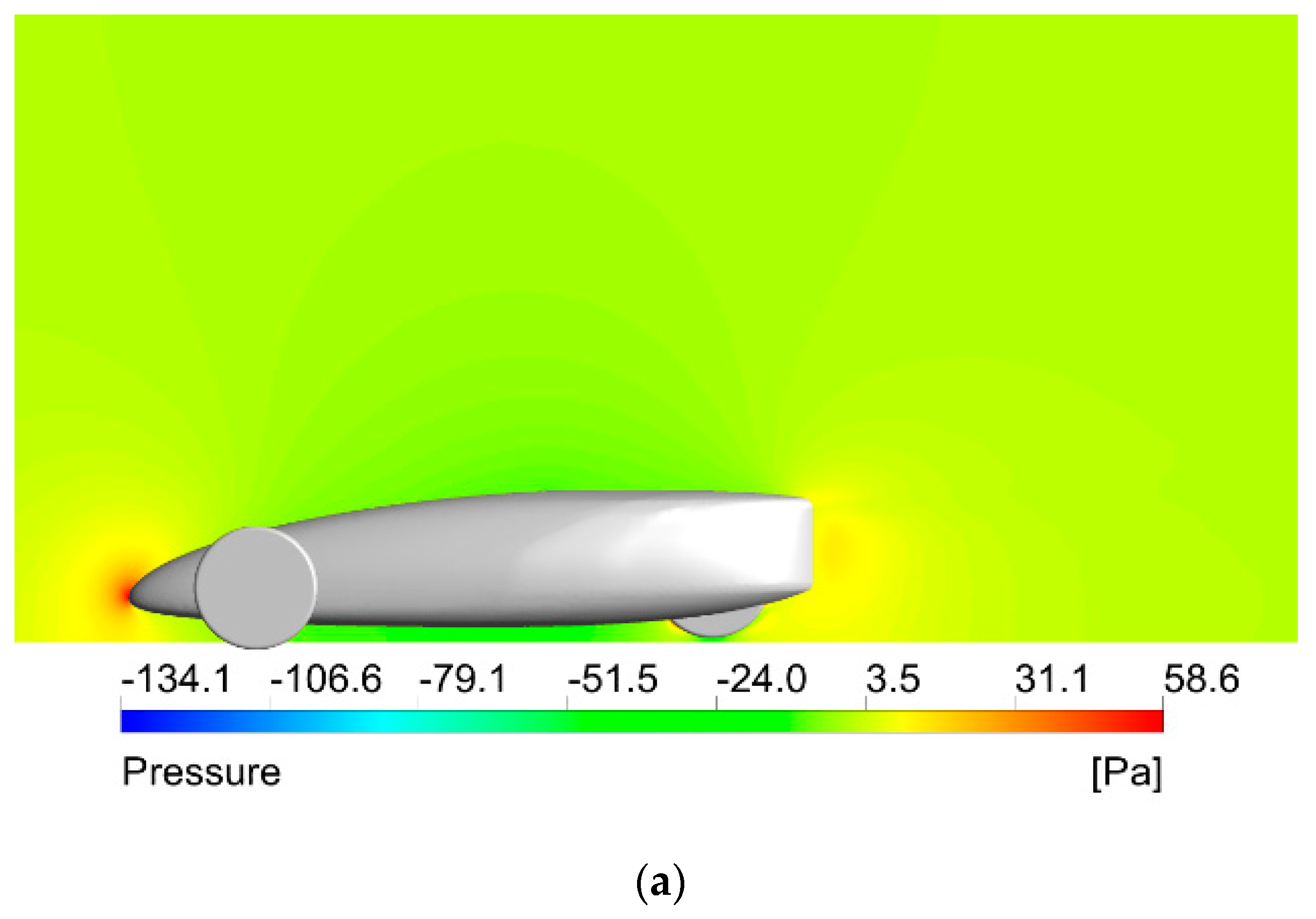
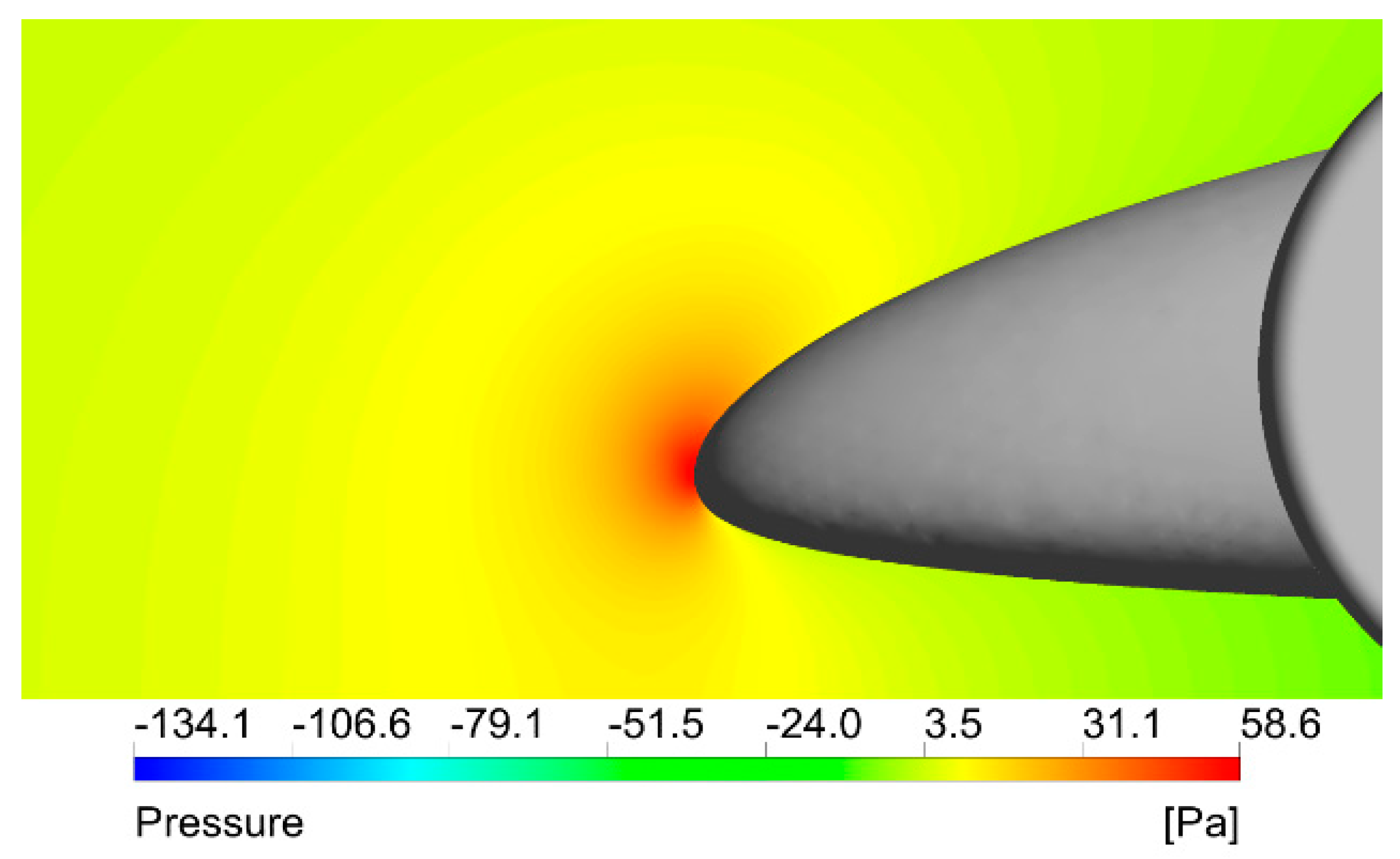
Figure 24 shows the static pressure distribution on the car surface. The maximum value of pressure appears at the front of the car because the fluid velocity on this area is close to zero. It reaches a value of 58.6 Pa, as shown in Figure 25.

6. Comparison with Previous Work

Cieslinski et al. [12] compared three different car body prototypes with fairing with their “Eco–Arrow” Figure 26.

Figure 27 and

Table 6. Comparison between coefficient of drag, area of drag, coefficient of lift and drag force for present car design and those from Reference [12] at a velocity of 25 km/h.

Model	EcoArrow [12]	Prototype 1 [12]	Prototype 2 [12]	Prototype 3 [12]	Present
CD	0.096	0.093	0.105	0.197	0.072
AD [m2]	0.0396	0.0244	0.0372	0.0492	0.0412
CL	0.083	−0.091	−0.091	0.008	−0.073
FD [N]	1.12	0.69	1.05	1.39	0.61

show the relation between the present design and those from Reference [12]. Along the speed range, 20 to 45 km, the present car design shows the lowest coefficient of drag.

Moreover,

Table 7. Comparison between coefficient of drag for Shell Eco-Marathon cars from previous (Experimental and Numerical) and present studies at a velocity of 25 km/h.

References	Method	CD
Cieslinski et al. [14]	Num.	0.092
“HorasMesin USU” [15]	Num.	0.24320
“Ford-Fiesta” [15]	Num.	0.29598
Abo-Serie et al. [16]	Num.	0.127
Santin et al. [35]	Num.	0.075
Present	Num.	0.072

shows a comparison between the coefficient of drag for Shell Eco-Marathon cars from previous (Experimental and Simulation) and present studies. The average drag coefficients of “Horas Mesin USU” and commercial city car “Ford-Fiesta” were 0.24320 and 0.29598, respectively [13]. Abo-Serie et al. [14] numerically proposed a low drag concept car with a tear drop shape and a non-rotating wheel with a coefficient of drag of 0.127.

Finally, the obtained coefficient of drag has a value lower than those of the best competition designs, which reaches a value as low as 0.075 [28]. The present car profile seems to be aerodynamically superior to others.

7. Conclusions

Using numerical analysis helps to investigate the aerodynamic properties of the present car model (shell eco-marathon car with Roncz and NACA 10 airfoils body profile) with non-rotating fairing wheels outside the car body. The numerical method is the first to be validated with previous experimental and numerical works on the Ahmed Body. The current numerical method shows an acceptable discrepancy. Using the validated method, a numerical study is performed and conclusions are as follows:

• The averaged drag coefficient is 0.069 (minimum value of all previous studies).
• The velocity vector shows a streamlined body.
• Pressure distribution shows the maximum pressure on the car front at a value of 58.6 Pa.

This fact indicates that the car design with Roncz and NACA 10 airfoils body profile is effective.