Some Observations on Shape Factors Influencing Aerodynamic Lift on Passenger Cars
Abstract
:1. Introduction
2. Experimental Details
3. Shape Effects
3.1. Basic Blocks
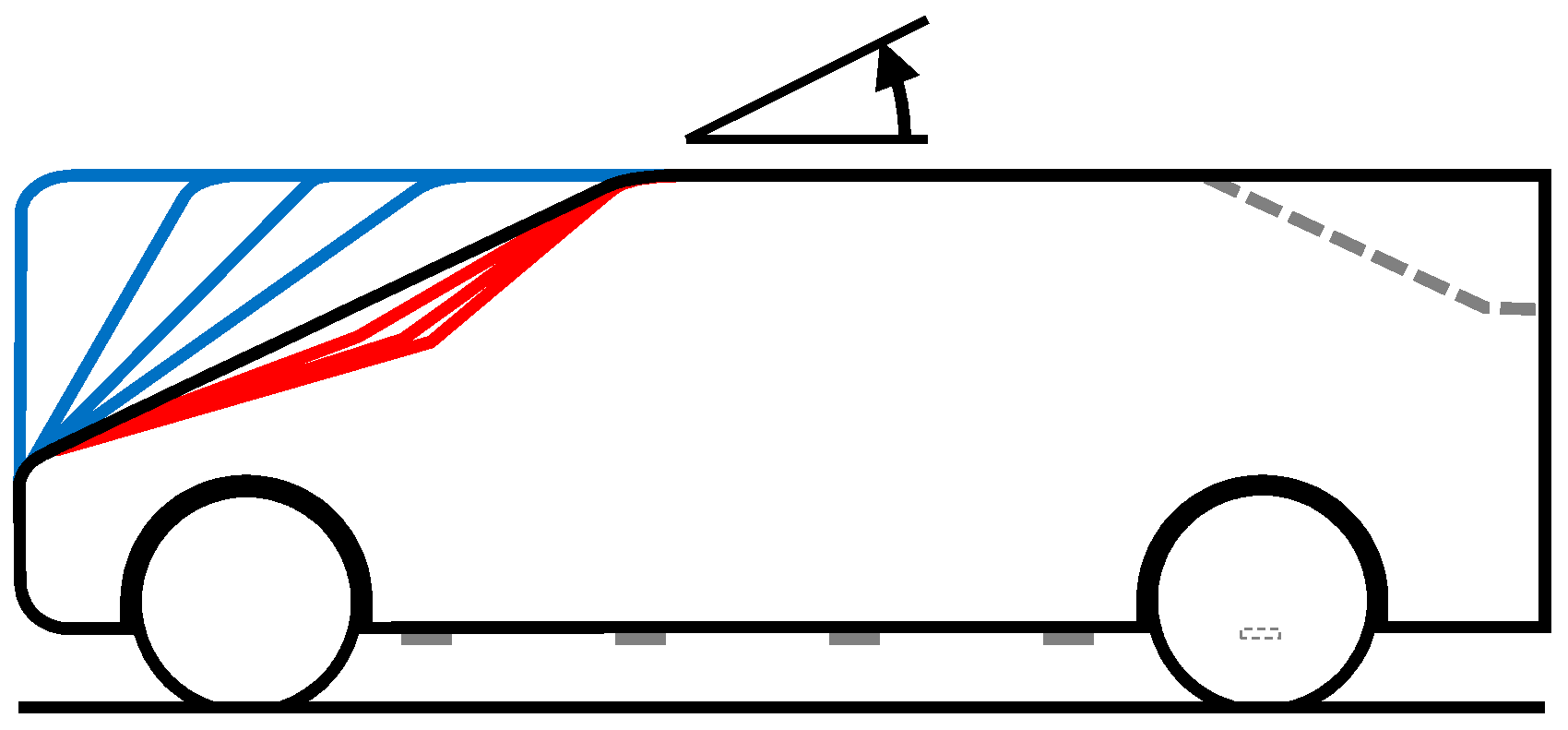
3.2. Front-End Shape
3.2.1. Leading Edge Radius
3.2.2. Front-End
3.3. Rear-End Shape
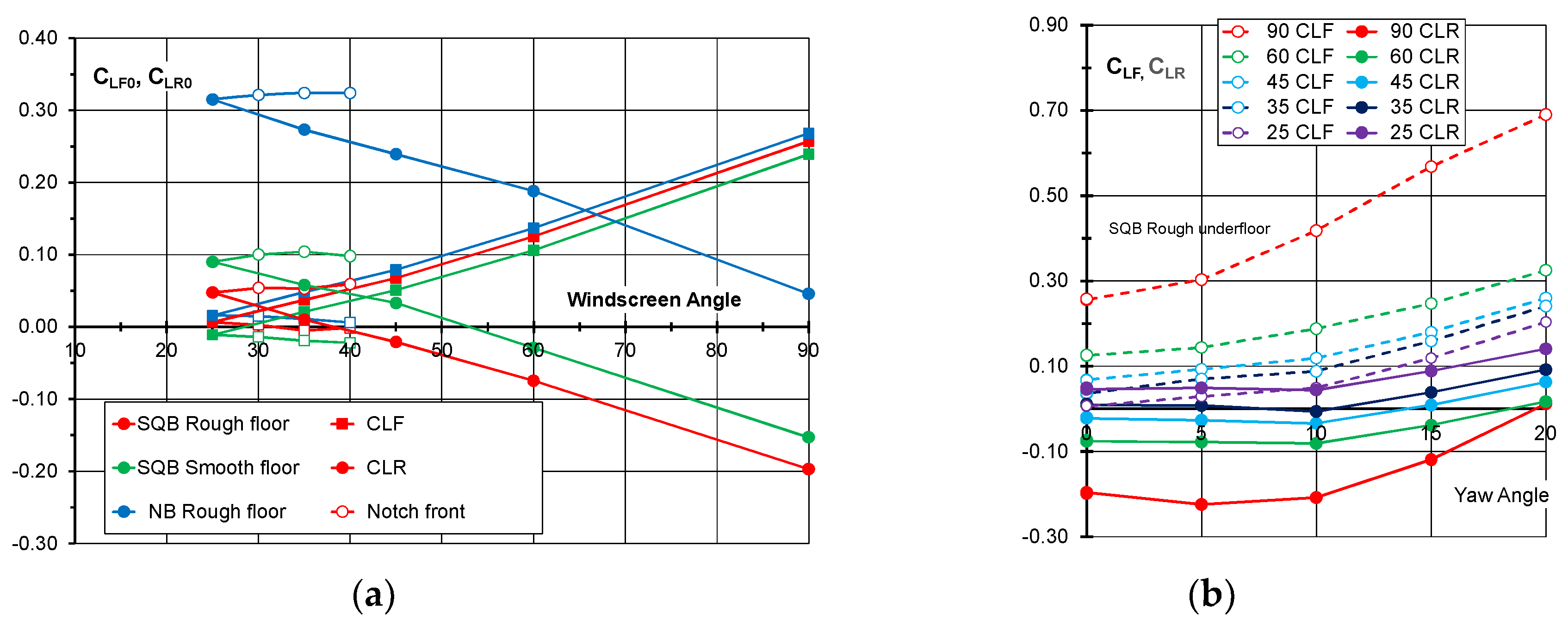
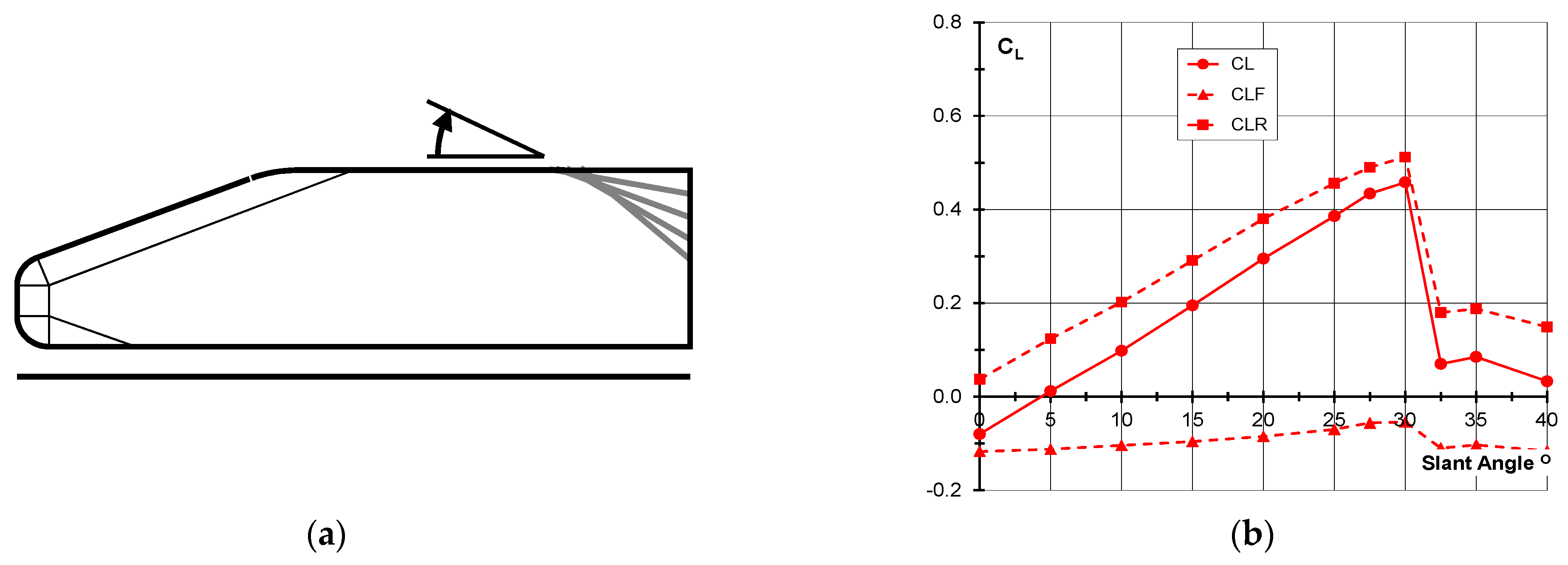
3.3.1. Roof Slope/Backlight Angle
3.3.2. Slant Aspect Ratio
3.3.3. Edge Rounding
3.3.4. Notchback
3.4. Roof Curvature
4. Discussion
4.1. Shape and Drag
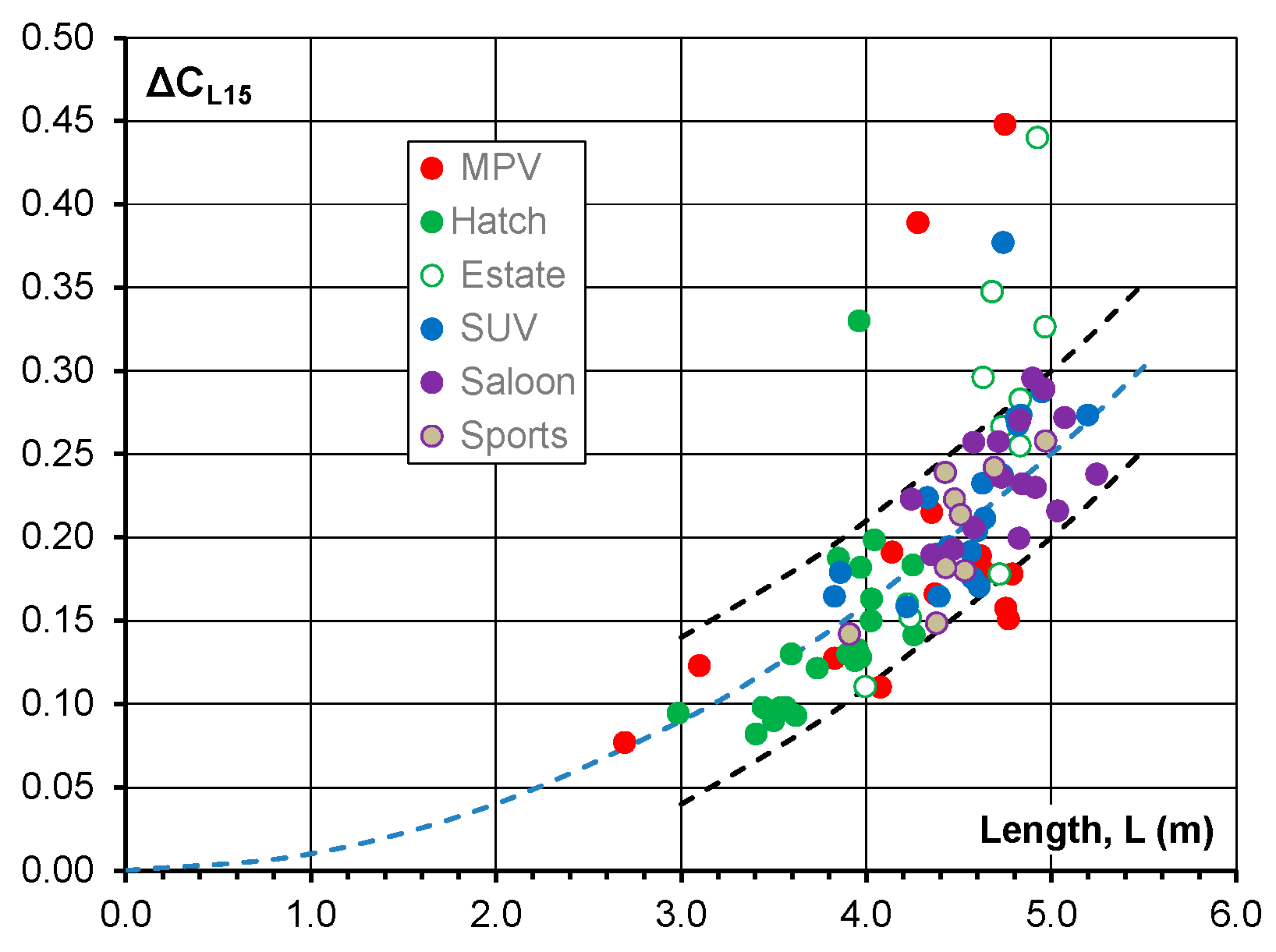
4.2. Other Lift Factors and Considerations
4.3. Influence of Camber-Line
4.4. Lift at Yaw
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Howell, J.P.; Le Good, G.M. The Influence of Aerodynamic Lift on High Speed Stability. SAE Tech. Pap. 1999, 1, 0651. [Google Scholar]
- Howell, J.P.; Le Good, G.M. Effect of Backlight Aspect Ratio on Vortex and Base Drag for a Simple Car-Like Shape. SAE Tech. Pap. 2008, 1, 0737. [Google Scholar]
- Howell, J.P.; Baden Fuller, J. A Relationship between Lift and Lateral Aerodynamic Characteristics for Passenger Cars. SAE Tech. Pap. 2010, 1, 1025. [Google Scholar]
- Carr, G.W. The Aerodynamics of Basic Shapes for Road Vehicles. Part 2: Saloon Car Bodies; MIRA Report No. 1968/9; MIRA: Nuneaton, UK, 1968. [Google Scholar]
- Gilhaus, A.M.; Renn, V.E. Drag and Driving Stability Related Aerodynamic Forces and their Interdependence—Results of Measurements on 3/8-Scale Basic Car Shapes. SAE Tech. Pap. 1986, 95, 1047–1062. [Google Scholar]
- Hucho, W.-H. (Ed.) Aerodynamics of Road Vehicles, 4th ed.; SAE R-177; SAE International: Warrendale, PA, USA, 1998; ISBN 0-7680-0029-7. [Google Scholar]
- Schütz, T. (Ed.) Aerodynamics of Road Vehicles, 5th ed.; SAE R-430; SAE International: Warrendale, PA, USA, 2016; ISBN 978-0-7680-7977-7. [Google Scholar]
- Morelli, A. Theoretical Method for Determining the Lift Distribution on a Vehicle; FISITA Congress: Tokyo, Japan, 1964. [Google Scholar]
- Carr, G.W.; Atkin, P.D.; Somerville, J. An Empirically Based Prediction Method for Car Aerodynamic Lift and Side Force. In Proceedings of the RAeS Vehicle Aerodynamics Conference, Loughborough University, Loughborough, UK, 18–19 July 1994. [Google Scholar]
- Carr, G.W. Wind Tunnel Blockage Corrections for Road Vehicles; MIRA Report No. 1971/4; MIRA: Nuneaton, UK, 1971. [Google Scholar]
- Howell, J.P. Force and Wake Characteristics of Simple Bluff Bodies in Ground Proximity. In Proceedings of the ASME Conference—Aerodynamics of Transportation, Niagara Falls, NY, USA, 18–20 June 1979. [Google Scholar]
- Newnham, P.S. The Influence of Real World Turbulence on the Aerodynamic Optimisation of Bluff Body Road Vehicles. Ph.D. Thesis, Loughborough University, Loughborough, UK, 2007. [Google Scholar]
- Pavia, G. Characterisation of the Unsteady Wake of a Squareback Road Vehicle. Ph.D. Thesis, Loughborough University, Loughborough, UK, 2019. [Google Scholar]
- Howell, J.; Passmore, M.; Windsor, S. A Drag Coefficient for Test Cycle Application. SAE Tech. Pap. 2018, 1, 0742. [Google Scholar] [CrossRef]
- Howell, J.P. Shape Features which Influence Crosswind Sensitivity. In Proceedings of the Ride and Handling Conference, Birmingham, UK, 15–17 November 1993; Paper C466/036/93. IMechE: London, UK, 1993. [Google Scholar]
- Howell, J. Shape and Drag. In Euromotor: Using Aerodynamics to Improve the Properties of Cars; FKFS: Stuttgart, Germany, 1998. [Google Scholar]
- Howell, J.P. The Influence of Ground Simulation on the Aerodynamics of Simple Car Shapes with an Underfloor Diffuser. In Proceedings of the RAeS Vehicle Aerodynamics Conference, Loughborough University, Loughborough, UK, 18–19 July 1994. [Google Scholar]
- Howell, J.P.; Hickman, D. The Influence of Ground Simulation on the Aerodynamics of a Simple Car Model. SAE Tech. Pap. 1997, 1, 970134. [Google Scholar]
- Howell, J.; Panigrahi, S. Aerodynamic Side Forces on Passenger Cars at Yaw. SAE Tech. Pap. 2016, 1, 1620. [Google Scholar]

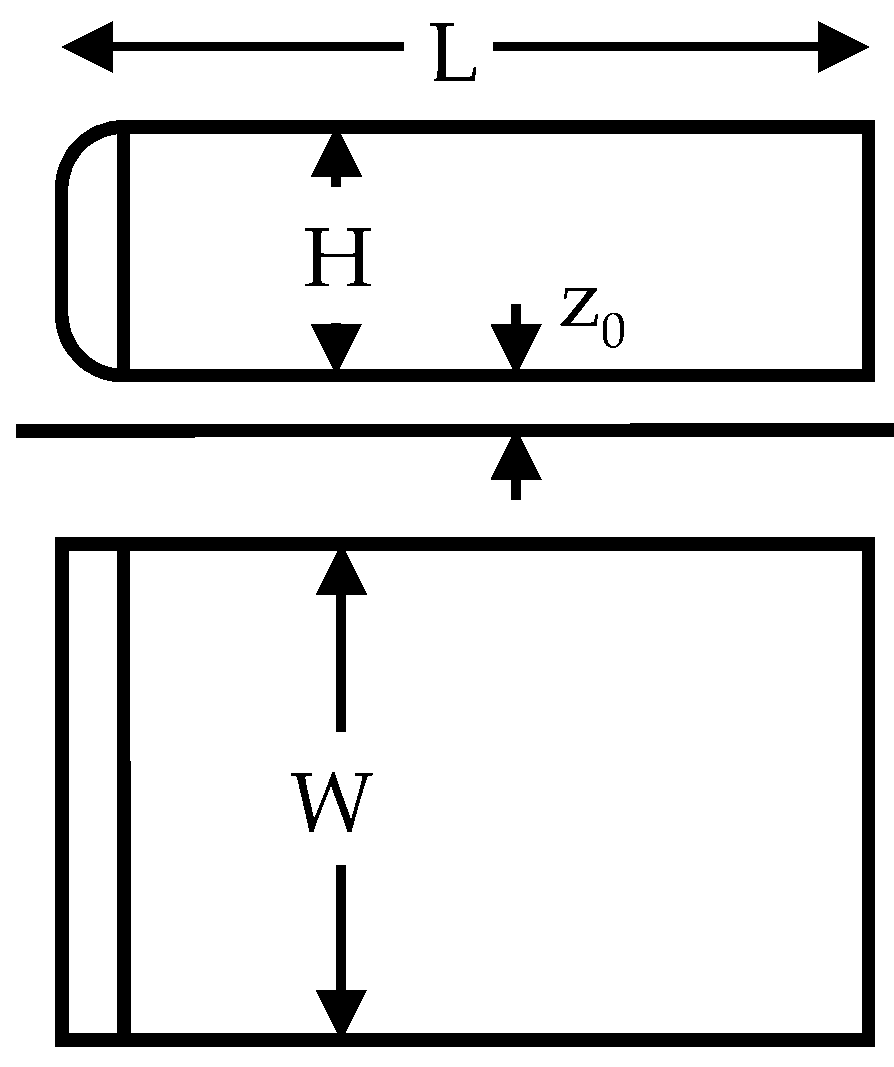




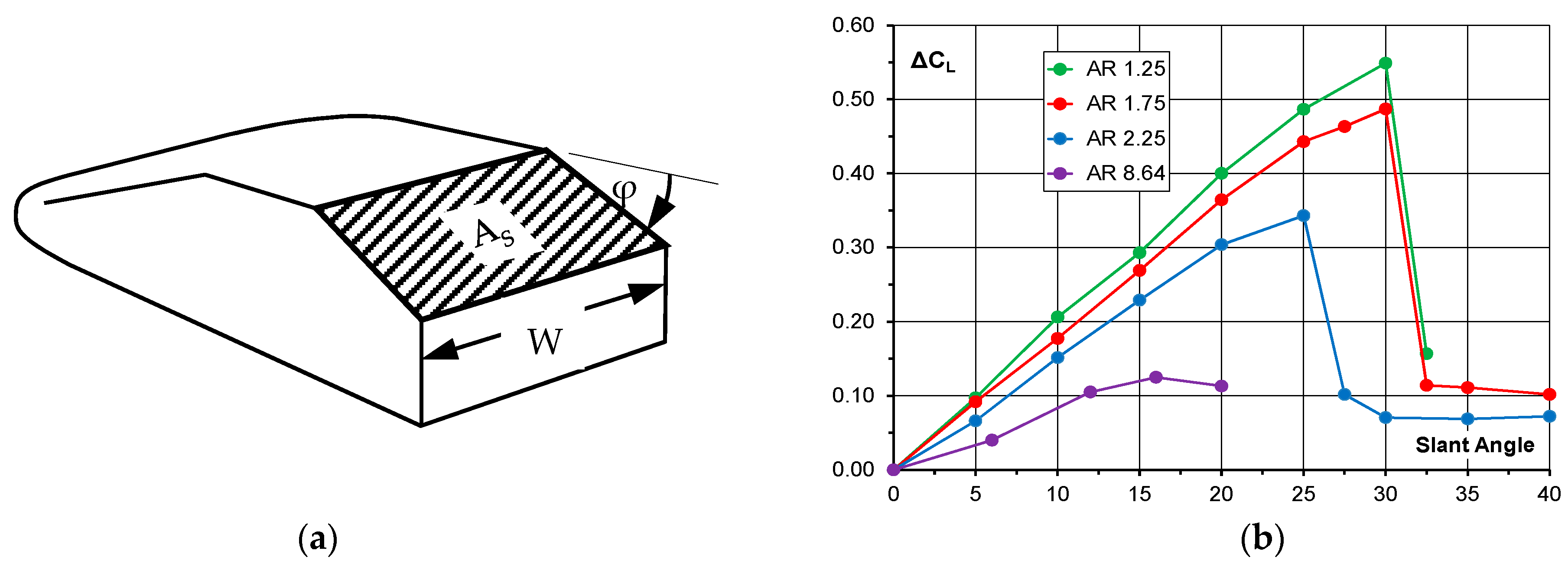
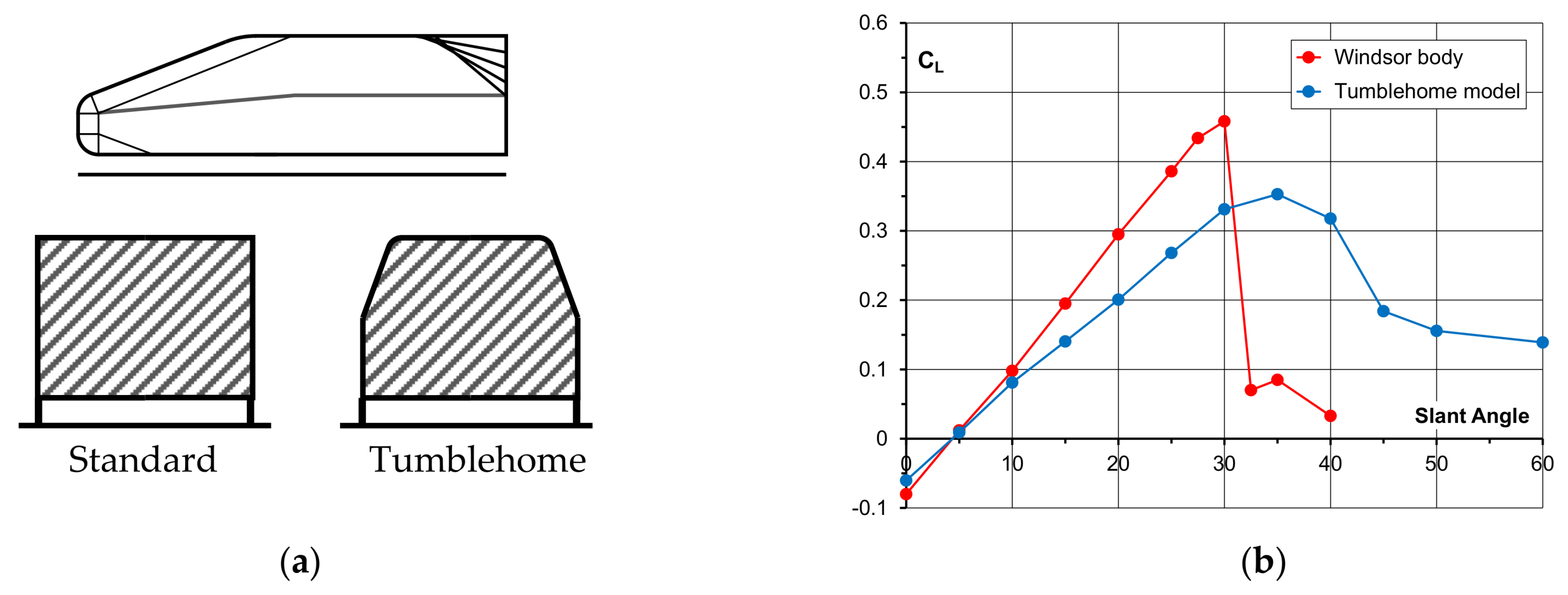

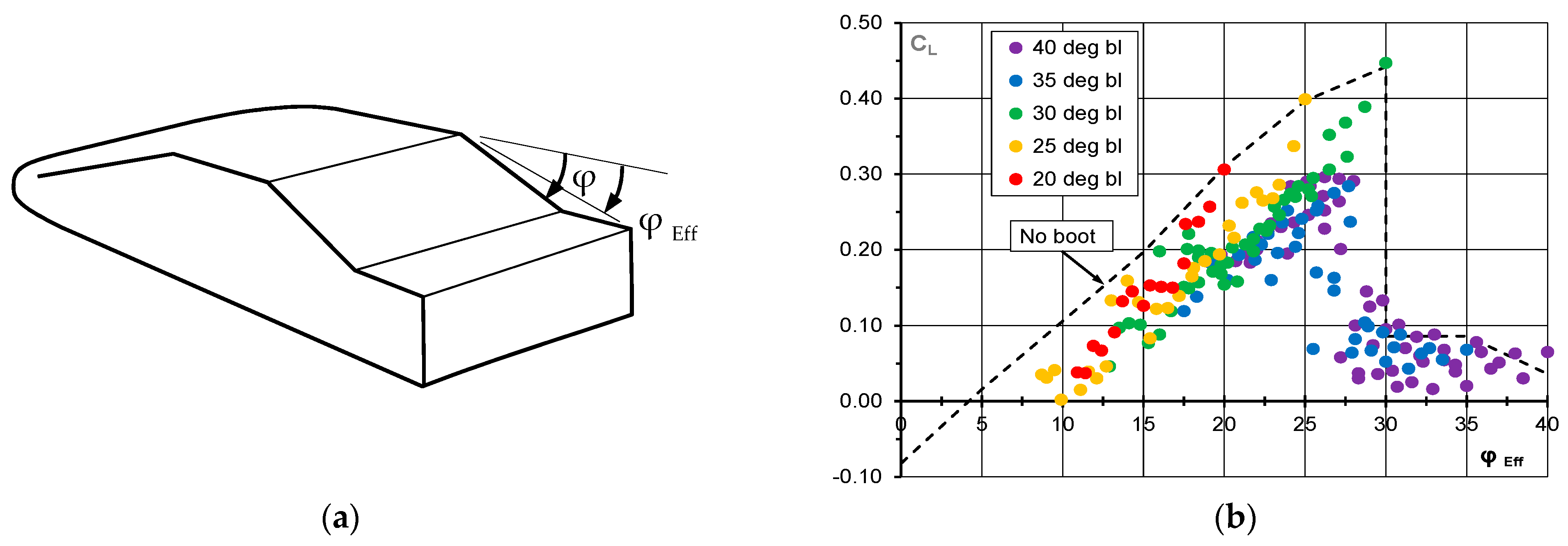

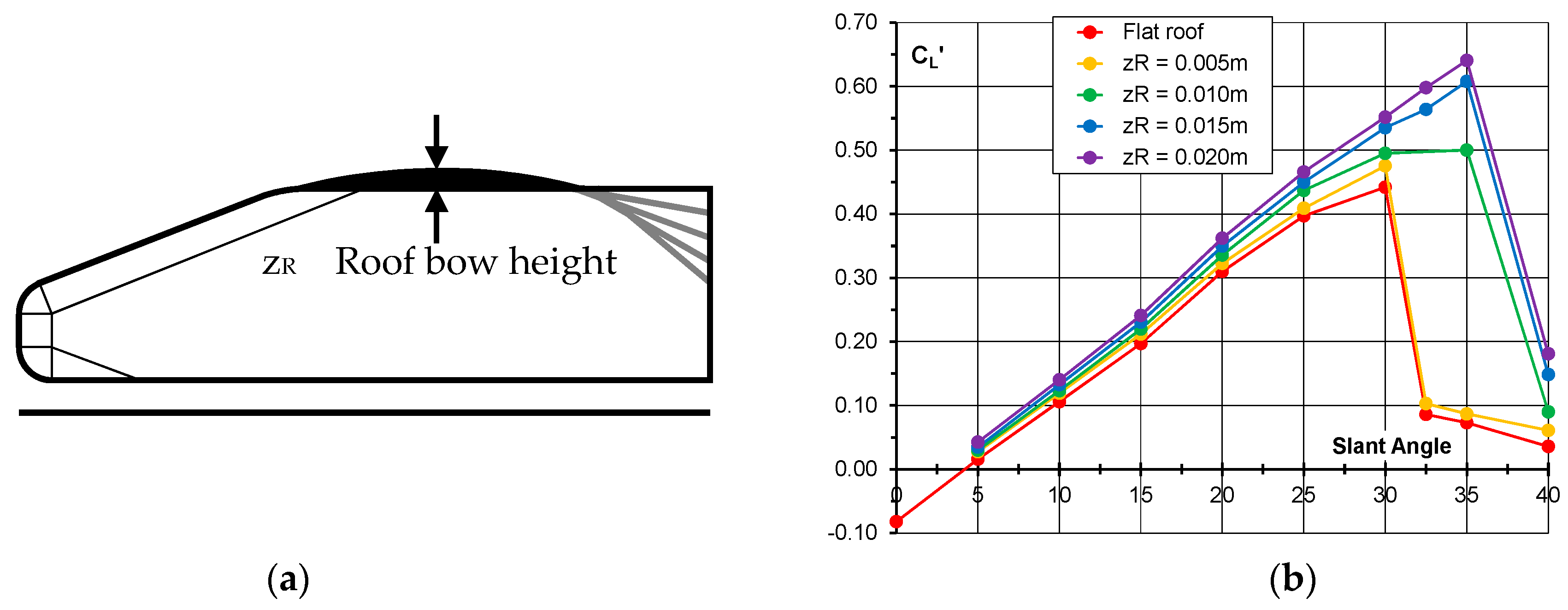
| Block | L (m) | W (m) | H (m) |
|---|---|---|---|
| 1 N | 0.325 | 0.1 | 0.1 |
| 2 N | 0.325 | 0.2 | 0.1 |
| 3 N | 0.325 | 0.3 | 0.1 |
| 4 N | 0.325 | 0.2 | 0.05 |
| 5 N | 0.325 | 0.2 | 0.025 |
| 6 N | 0.225 | 0.2 | 0.1 |
| 7 N | 0.425 | 0.2 | 0.1 |
| Model Type | Windscreen Angle° | Bonnet Angle° |
|---|---|---|
| 1-box | 90 | - |
| 60 | - | |
| 45 | - | |
| 35 | - | |
| 25 | - | |
| Notched front | 30 | 19 |
| 35 | 17 | |
| 40 | 15 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Howell, J.; Windsor, S.; Passmore, M. Some Observations on Shape Factors Influencing Aerodynamic Lift on Passenger Cars. Fluids 2021, 6, 44. https://doi.org/10.3390/fluids6010044
Howell J, Windsor S, Passmore M. Some Observations on Shape Factors Influencing Aerodynamic Lift on Passenger Cars. Fluids. 2021; 6(1):44. https://doi.org/10.3390/fluids6010044
Chicago/Turabian StyleHowell, Jeff, Steve Windsor, and Martin Passmore. 2021. "Some Observations on Shape Factors Influencing Aerodynamic Lift on Passenger Cars" Fluids 6, no. 1: 44. https://doi.org/10.3390/fluids6010044
APA StyleHowell, J., Windsor, S., & Passmore, M. (2021). Some Observations on Shape Factors Influencing Aerodynamic Lift on Passenger Cars. Fluids, 6(1), 44. https://doi.org/10.3390/fluids6010044





