Heat Transfer Enhancement inside Rectangular Channel by Means of Vortex Generated by Perforated Concave Rectangular Winglets
Abstract
:1. Introduction
2. Materials and Methods
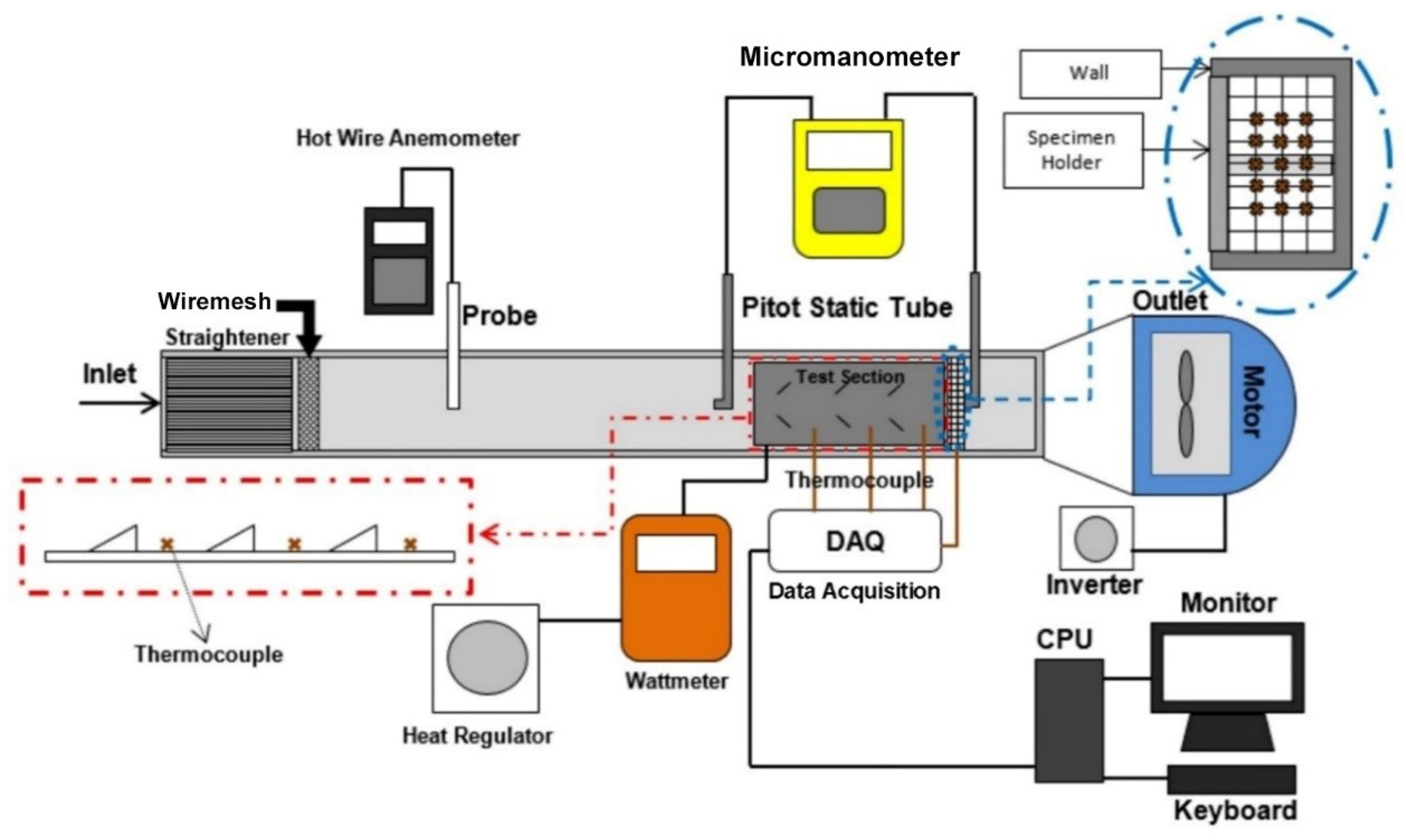
2.1. Experimental Set-Up
2.2. Physical Model
2.3. Governing Equation
2.3.1. Boundary Conditions
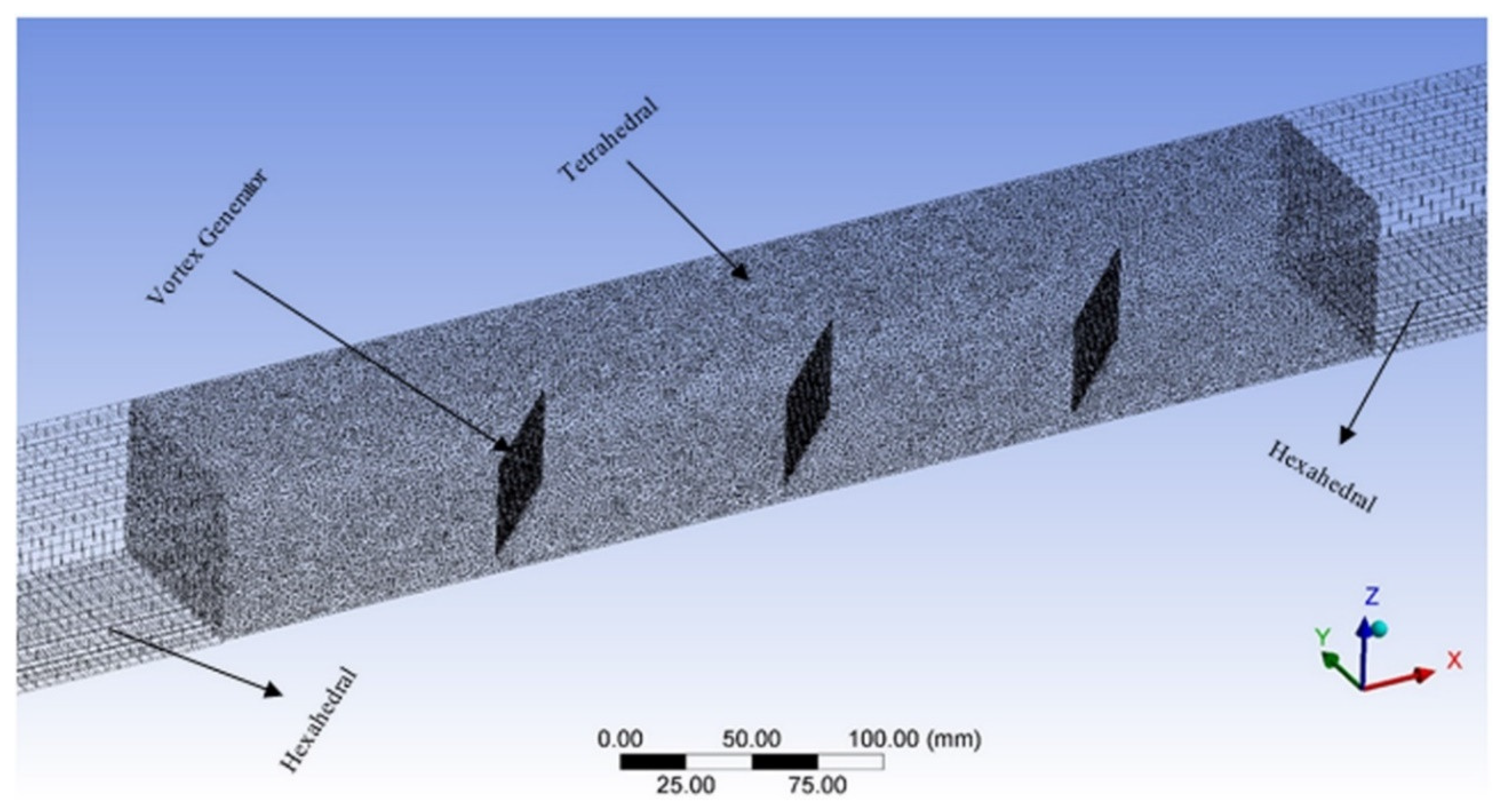
2.3.2. Numerical Method
2.4. Model Validation
3. Results and Discussion
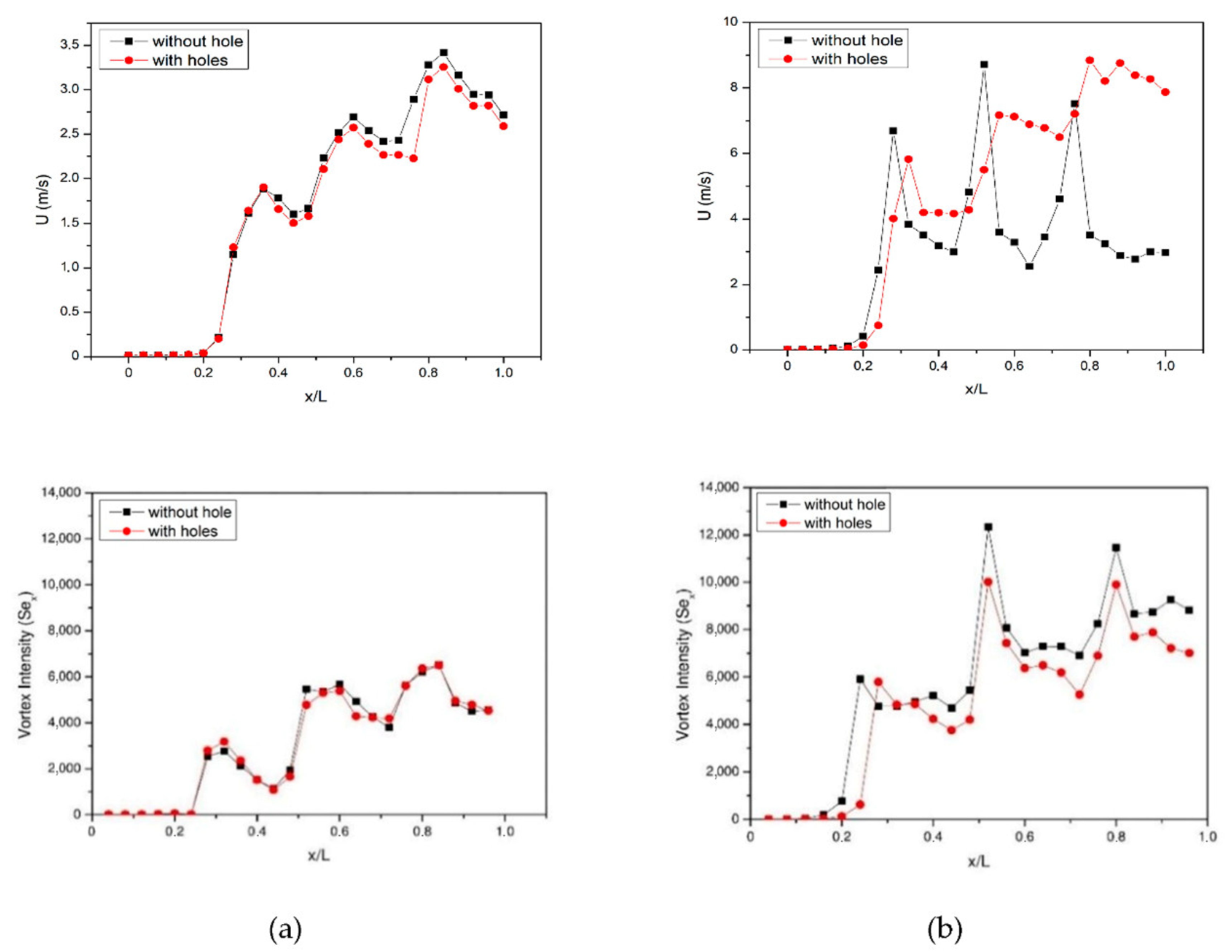
3.1. Velocity Vector and Streamline
3.2. Longitudinal Vortex Intensity
3.3. Temperature Distribution
3.4. Pressure Distribution
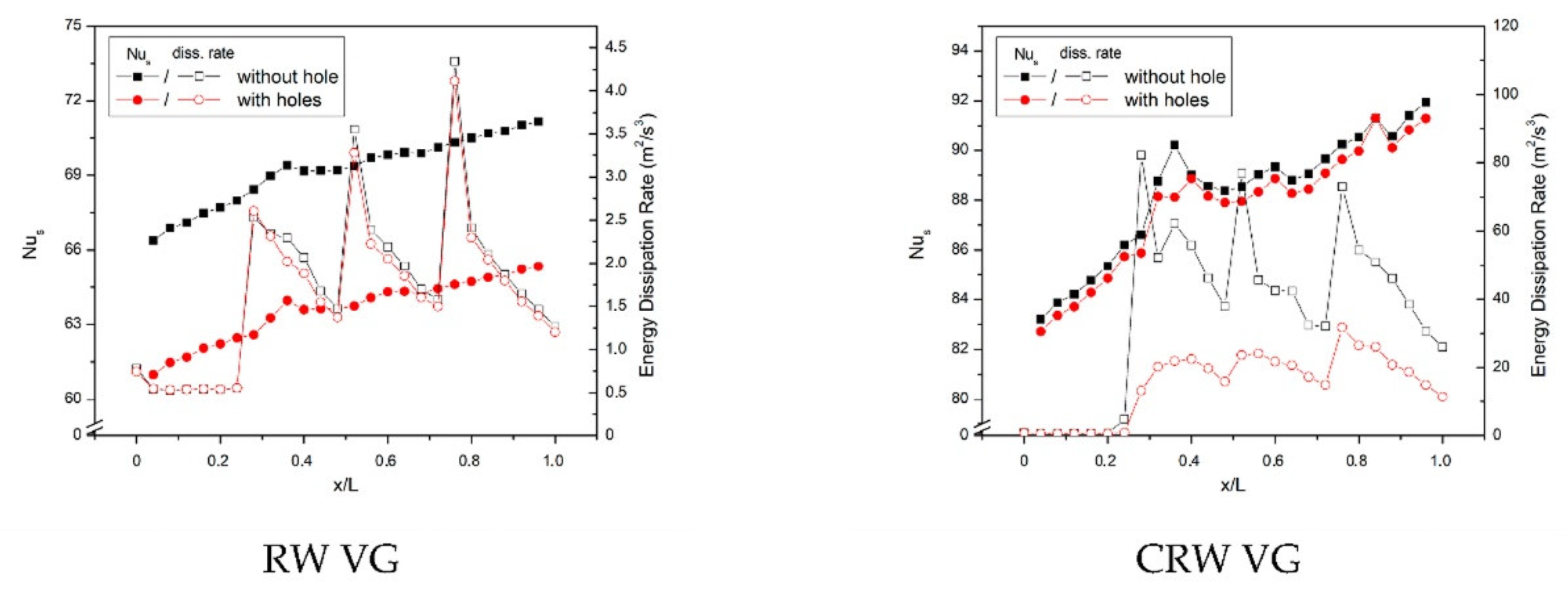
3.5. VG Impacts on Average Local Nusselt Number
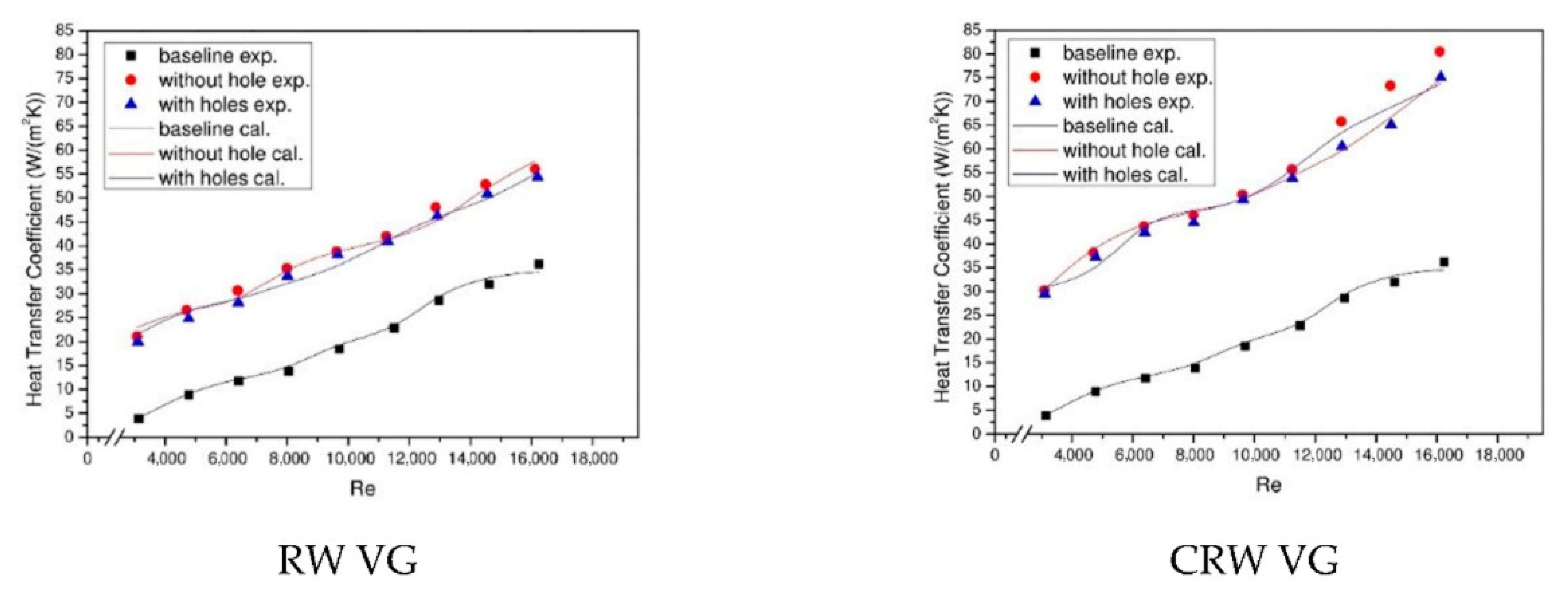
3.6. VG Effects on Heat Transfer Rate
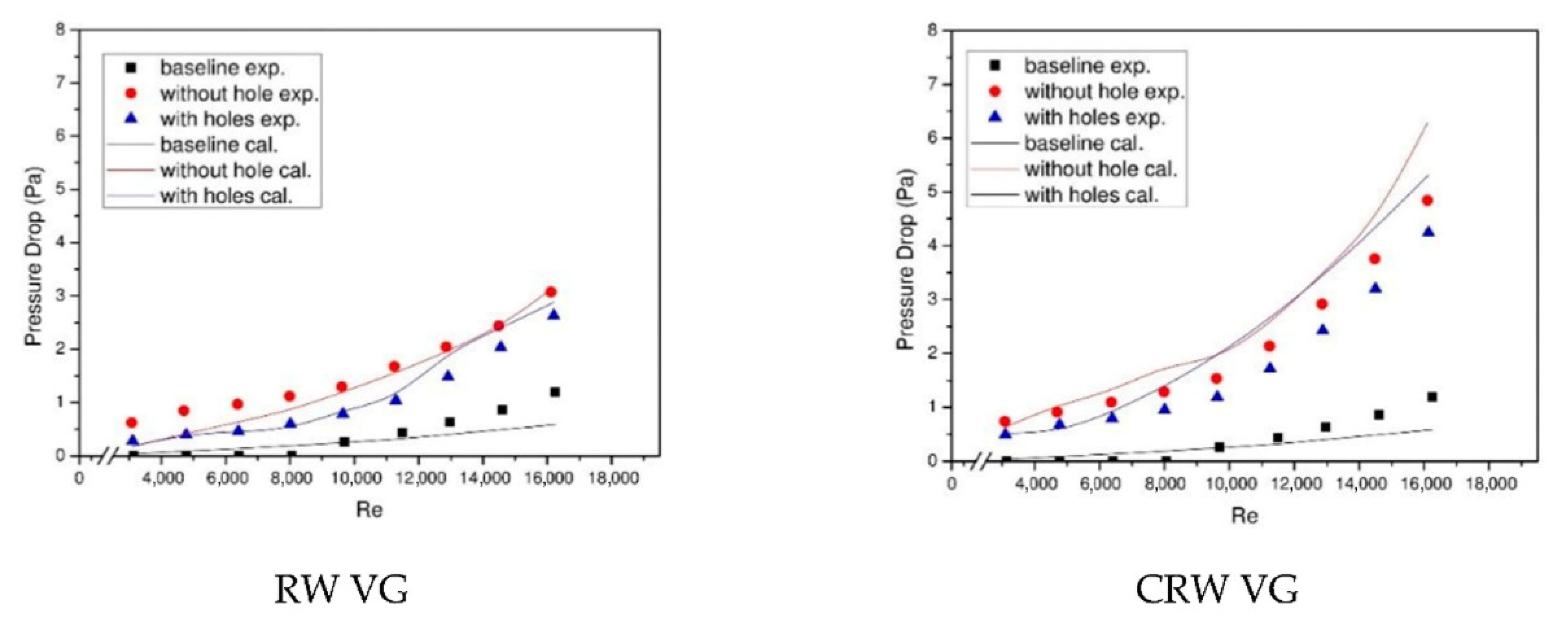
3.7. VG Effects on Flow Pressure Drop
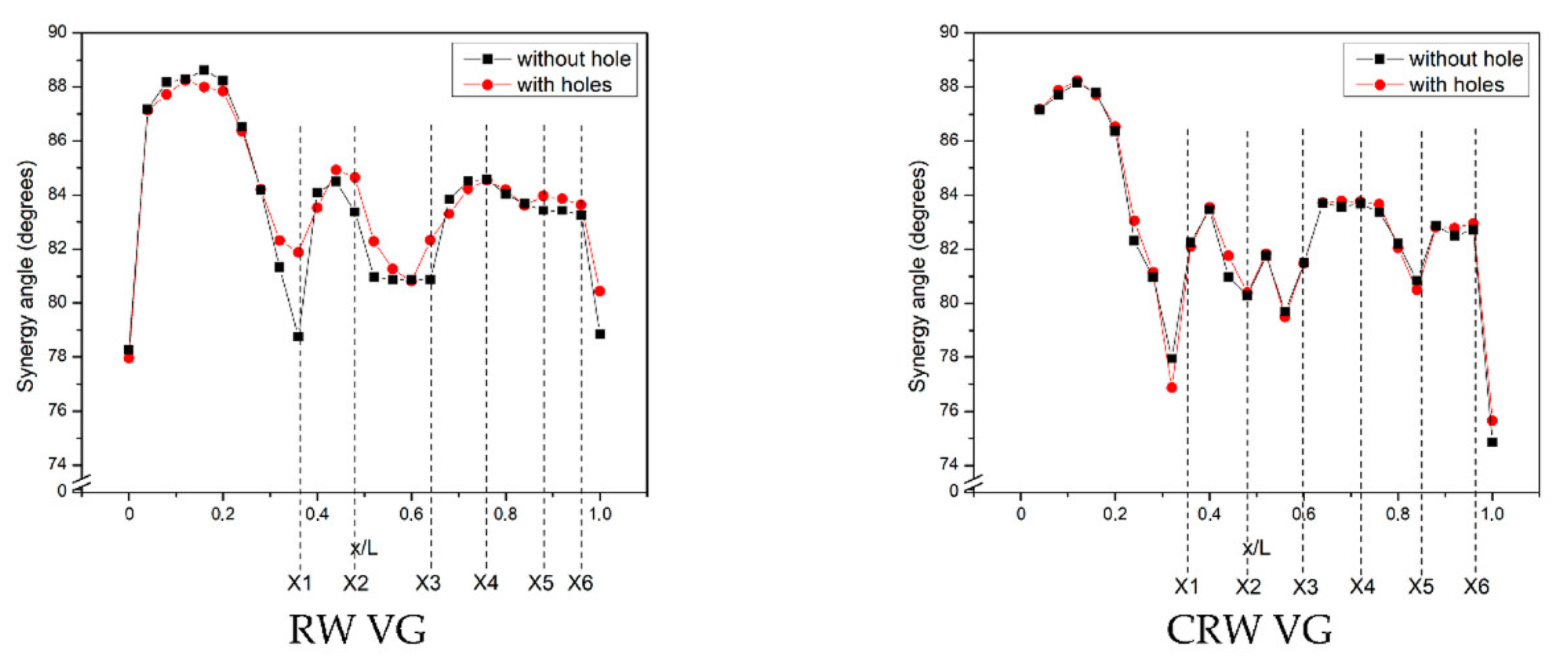
3.8. Field Synergy Principe Analysis
4. Conclusions
- The decrease in the convection heat transfer coefficient in the case of perforated CRW VG was 1.02% of the CRW VG without holes at a flow velocity of 2.0 m/s. Whereas in a similar case for RW VG, the convection heat transfer coefficient decreased by 4.06% from that of RW VG without holes at the same flow velocity.
- Pressure drop on perforated VG decreased by 15.38% in cases of CRW VG with holes and 7.69% in cases of RW VG with holes at the highest flow velocity.
- The average synergy angles in the RW VG case are 0.41° and 0.25° in the CRW VG for the highest flow velocity.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xu, Y.; Islam, M.D.; Kharoua, N. Experimental study of thermal performance and flow behaviour with winglet vortex gen-erators in a circular tube. Appl. Therm. Eng. 2018, 135, 257–268. [Google Scholar] [CrossRef]
- Wu, H.; Ting, D.S.-K.; Ray, S. Flow over a flat surface behind delta winglets of varying aspect ratios. Exp. Therm. Fluid Sci. 2018, 94, 99–108. [Google Scholar] [CrossRef]
- Qian, Z.; Wang, Q.; Cheng, J. Analysis of heat and resistance performance of plate fin-and-tube heat exchanger with rectangle-winglet vortex generator. Int. J. Heat Mass Transf. 2018, 124, 1198–1211. [Google Scholar] [CrossRef]
- Kashyap, U.; Das, K.; Debnath, B.K. Effect of surface modification of a rectangular vortex generator on heat transfer rate from a surface to fluid: An extended study. Int. J. Therm. Sci. 2018, 134, 269–281. [Google Scholar] [CrossRef]
- Awais, M.; Bhuiyan, A.A. Heat transfer enhancement using different types of vortex generators (VGs): A review on experimental and numerical activities. Therm. Sci. Eng. Prog. 2018, 5, 524–545. [Google Scholar] [CrossRef]
- Da Silva, F.A.; Dezan, D.J.; Pantaleão, A.V.; Salviano, L.O. Longitudinal vortex generator applied to heat transfer enhancement of a flat plate solar water heater. Appl. Therm. Eng. 2019, 158, 113790. [Google Scholar] [CrossRef]
- Modi, A.J.; Rathod, M.K. Comparative study of heat transfer enhancement and pressure drop for fin-and-circular tube compact heat exchangers with sinusoidal wavy and elliptical curved rectangular winglet vortex generator. Int. J. Heat Mass Transf. 2019, 141, 310–326. [Google Scholar] [CrossRef]
- Han, H.; Wang, S.; Sun, L.; Li, Y.; Wang, S. Numerical study of thermal and flow characteristics for a fin-and-tube heat exchanger with arc winglet type vortex generators. Int. J. Refrig. 2019, 98, 61–69. [Google Scholar] [CrossRef]
- Lu, G.; Zhai, X. Analysis on heat transfer and pressure drop of a microchannel heat sink with dimples and vortex generators. Int. J. Therm. Sci. 2019, 145, 105986. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, X.; Ye, X.; Chen, Y.; Cheng, Y.; Lan, Z. Heat transfer enhancement of annular finned tube exchanger using vortex generators: The effect of oriented functional circumferential arrangement. Therm. Sci. Eng. Prog. 2019, 10, 27–35. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, K.; Li, W.; Chen, Q.; Zheng, N. Investigations of the turbulent thermal-hydraulic performance in circular heat exchanger tubes with multiple rectangular winglet vortex generators. Appl. Therm. Eng. 2020, 168, 114838. [Google Scholar] [CrossRef]
- Zhang, L.; Yan, X.; Zhang, Y.; Feng, Y.; Li, Y.; Meng, H.; Zhang, J.; Wu, J. Heat transfer enhancement by streamlined winglet pair vortex generators for helical channel with rectangular cross section. Chem. Eng. Process. Process. Intensif. 2020, 147, 107788. [Google Scholar] [CrossRef]
- Gupta, A.; Roy, A.; Gupta, S.; Gupta, M. Numerical investigation towards implementation of punched winglet as vortex generator for performance improvement of a fin-and-tube heat exchanger. Int. J. Heat Mass Transf. 2020, 149, 119171. [Google Scholar] [CrossRef]
- Li, M.; Qu, J.; Zhang, J.; Wei, J.; Tao, W. Air side heat transfer enhancement using radiantly arranged winglets in fin-and-tube heat exchanger. Int. J. Therm. Sci. 2020, 156, 106470. [Google Scholar] [CrossRef]
- Promvonge, P.; Skullong, S. Thermo-hydraulic performance in heat exchanger tube with V-shaped winglet vortex generator. Appl. Therm. Eng. 2020, 164, 114424. [Google Scholar] [CrossRef]
- Syaiful; Siwi, A.R.; Utomo, T.S.; Yurianto; Wulandari, R. Numerical Analysis of Heat and Fluid Flow Characteristics of Airflow inside Rectangular Channel with Presence of Perforated Concave Delta Winglet Vortex Genera-tors. Int. J. Heat Technol. 2019, 37, 1059–1070. [Google Scholar] [CrossRef] [Green Version]
- Syaiful; Kusuma, N.; Muchammad; Wulandari, R.; Sinaga, N.; Siswantara, A.; Bae, M.-Y. Numerical investigation of heat transfer and pressure loss of flow through a heated plate mounted by perforated concave rectangular winglet vortex generators in a channel. In Proceedings of the AIP Conference Proceedings 2227, Padang, Indonesia, 22–24 July 2019. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics the Finite Volume Method, 1st ed.; Longman Scientific & Technical: London, UK, 1995; pp. 11–80. [Google Scholar]
- Tang, L.; Chu, W.; Ahmed, N.; Zeng, M. A new configuration of winglet longitudinal vortex generator to enhance heat transfer in a rectangular channel. Appl. Therm. Eng. 2016, 104, 74–84. [Google Scholar] [CrossRef]
- Song, K.; Liu, S.; Wang, L. Interaction of counter rotating longitudinal vortices and the effect on fluid flow and heat transfer. Int. J. Heat Mass Transf. 2016, 93, 349–360. [Google Scholar] [CrossRef]
- Esmaeilzadeh, A.; Amanifard, N.; Deylami, H.M. Comparison of simple and curved trapezoidal longitudinal vortex gener-ators for optimum flow characteristics and heat transfer augmentation in a heat exchanger. Appl. Therm. Eng. 2017, 125, 1414–1425. [Google Scholar] [CrossRef]
- Syarifudin, I.; Soetanto, M.F.; Bae, M.W. Numerical simulation of heat transfer augmentation in fin-and-tube heat exchanger with various number of rows of concave rectangular winglet vortex generator. In Proceedings of the 2nd International Joint Conference on Advanced Engineering and Technology (IJCAET 2017) and International Symposium on Advanced Mechanical and Power Engineering (ISAMPE 2017), Bali, Indonesia, 24–26 August 2017. [Google Scholar]
- Lu, G.; Zhou, G. Numerical simulation on performances of plane and curved winglet type vortex generator pairs with punched holes. Int. J. Heat Mass Transf. 2016, 102, 679–690. [Google Scholar] [CrossRef]
- Song, K.; Tagawa, T.; Chen, Z.; Zhang, Q. Heat transfer characteristics of concave and convex curved vortex generators in the channel of plate heat exchanger under laminar flow. Int. J. Therm. Sci. 2019, 137, 215–228. [Google Scholar] [CrossRef]
- Salviano, L.O.; Dezan, D.J.; Yanagihara, J.I. Thermal-hydraulic performance optimization of inline and staggered fin-tube compact heat exchangers applying longitudinal vortex generators. Appl. Therm. Eng. 2016, 95, 311–329. [Google Scholar] [CrossRef]
- Awais, M.; Bhuiyan, A.A. Enhancement of thermal and hydraulic performance of compact finned-tube heat exchanger using vortex generators (VGs): A parametric study. Int. J. Therm. Sci. 2019, 140, 154–166. [Google Scholar] [CrossRef]
- Hiravennavar, S.; Tulapurkara, E.; Biswas, G. A note on the flow and heat transfer enhancement in a channel with built-in winglet pair. Int. J. Heat Fluid Flow 2007, 28, 299–305. [Google Scholar] [CrossRef]
- Guo, Z.; Li, D.; Wang, B. A novel concept for convective heat transfer enhancement. Int. J. Heat Mass Transf. 1998, 41, 2221–2225. [Google Scholar] [CrossRef]

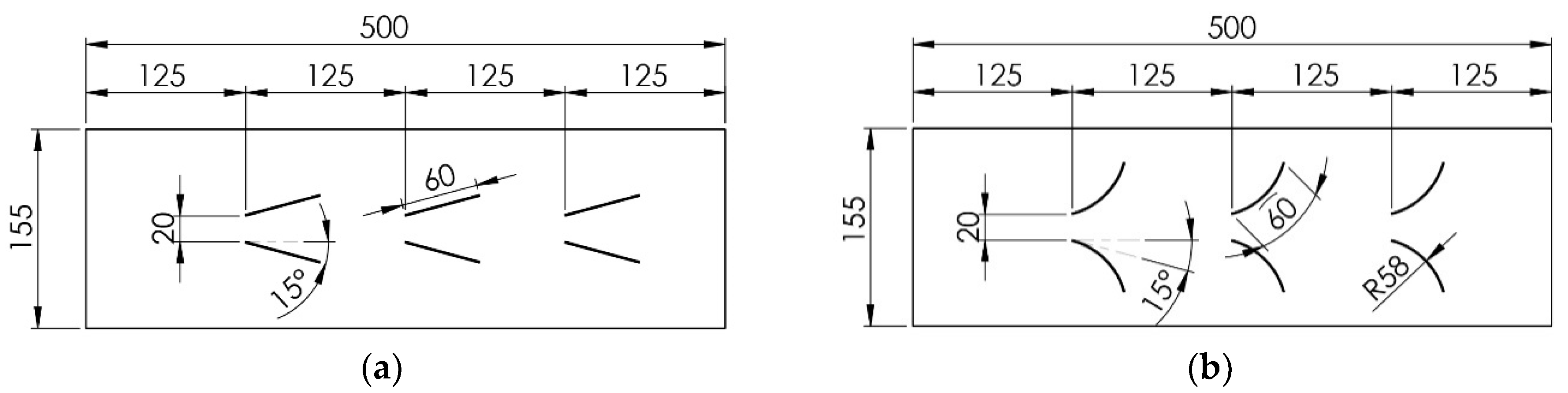





| VGs | α (o) | a (mm) | cv (mm) | dv (mm) | ev (mm) | ch (mm) | dh (mm) | eh (mm) | t (mm) | R (mm) |
|---|---|---|---|---|---|---|---|---|---|---|
| CRW without holes | 15 | 59 | - | - | - | - | - | - | 40 | 58 |
| CRW with holes | 15 | 59 | 29.56 | 44.56 | 14.56 | 20 | 30.15 | 9.85 | 40 | 58 |
| RW without holes | 15 | 60 | - | - | - | - | - | - | 40 | - |
| RW with holes | 15 | 60 | 30 | 45 | 15 | 20 | 30 | 10 | 40 | - |
| Element Number | hsimulation (W/m2·K) | hexperiment (W/m2·K) | Error (%) |
|---|---|---|---|
| 1,262,840 | 18.27726 | 18.18571 | 0.503 |
| 1,478,060 | 18.34781 | 18.18571 | 0.891 |
| 1,661,610 | 18.24699 | 18.18571 | 0.337 |
| 1,868,587 | 18.29429 | 18.18571 | 0.597 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Syaiful; Hendraswari, M.P.; S.U., M.S.K.T.; Soetanto, M.F. Heat Transfer Enhancement inside Rectangular Channel by Means of Vortex Generated by Perforated Concave Rectangular Winglets. Fluids 2021, 6, 43. https://doi.org/10.3390/fluids6010043
Syaiful, Hendraswari MP, S.U. MSKT, Soetanto MF. Heat Transfer Enhancement inside Rectangular Channel by Means of Vortex Generated by Perforated Concave Rectangular Winglets. Fluids. 2021; 6(1):43. https://doi.org/10.3390/fluids6010043
Chicago/Turabian StyleSyaiful, Monica Pranita Hendraswari, M.S.K. Tony S.U., and Maria F. Soetanto. 2021. "Heat Transfer Enhancement inside Rectangular Channel by Means of Vortex Generated by Perforated Concave Rectangular Winglets" Fluids 6, no. 1: 43. https://doi.org/10.3390/fluids6010043
APA StyleSyaiful, Hendraswari, M. P., S.U., M. S. K. T., & Soetanto, M. F. (2021). Heat Transfer Enhancement inside Rectangular Channel by Means of Vortex Generated by Perforated Concave Rectangular Winglets. Fluids, 6(1), 43. https://doi.org/10.3390/fluids6010043




