Time-Dependent Motion of a Floating Circular Elastic Plate
Abstract
1. Introduction
2. Equations of Motion
3. Eigenfunction Matching
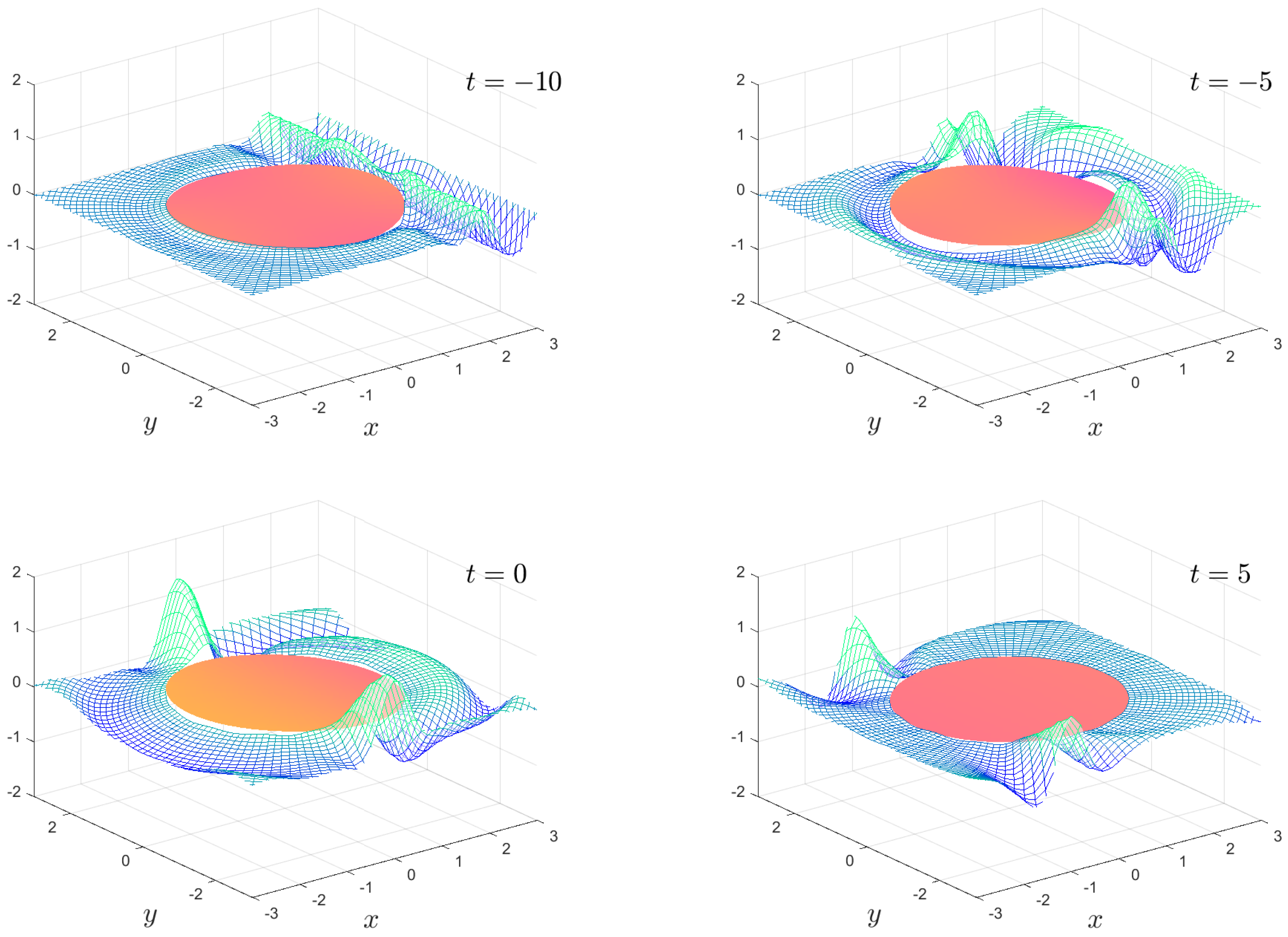
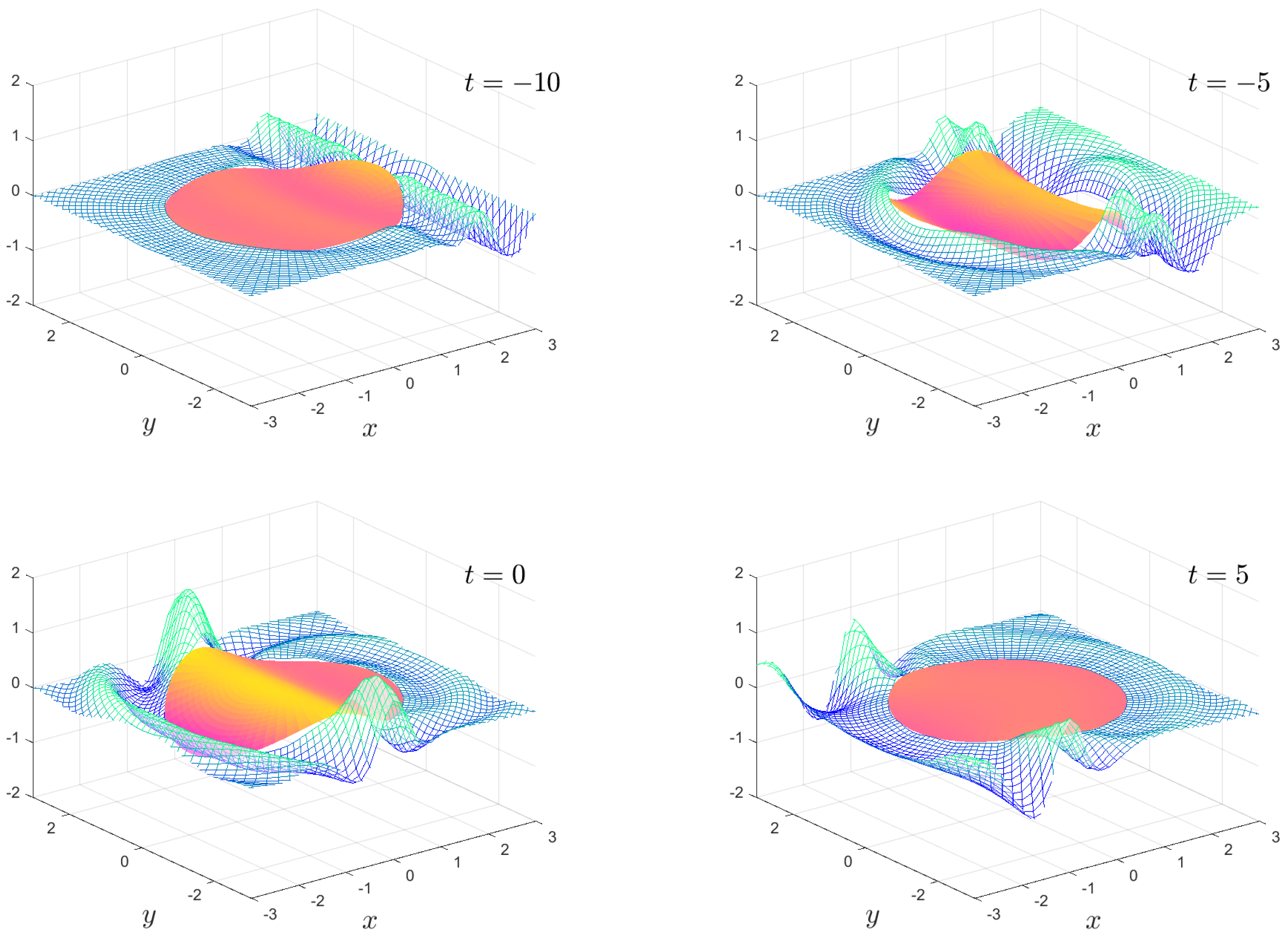
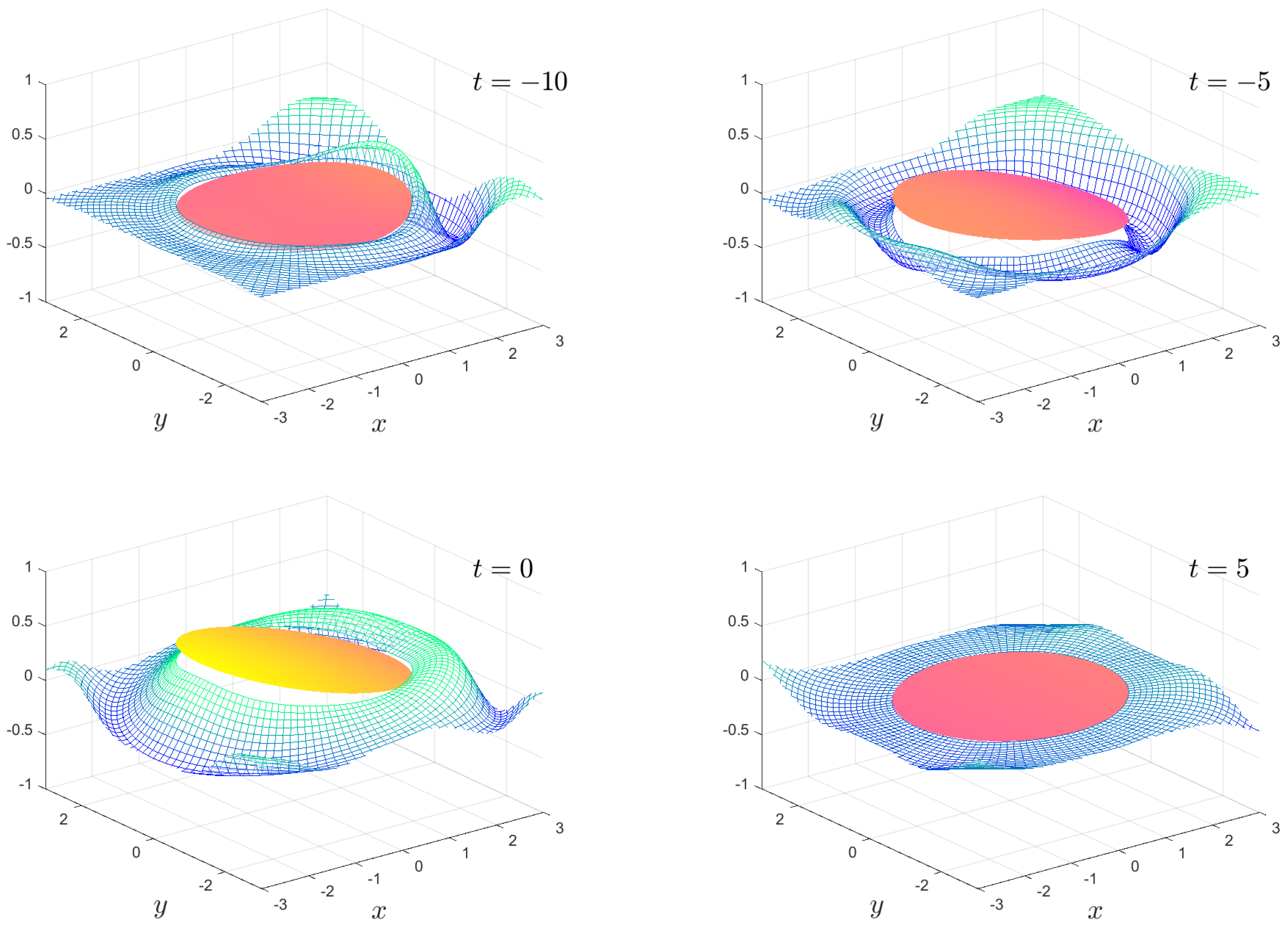
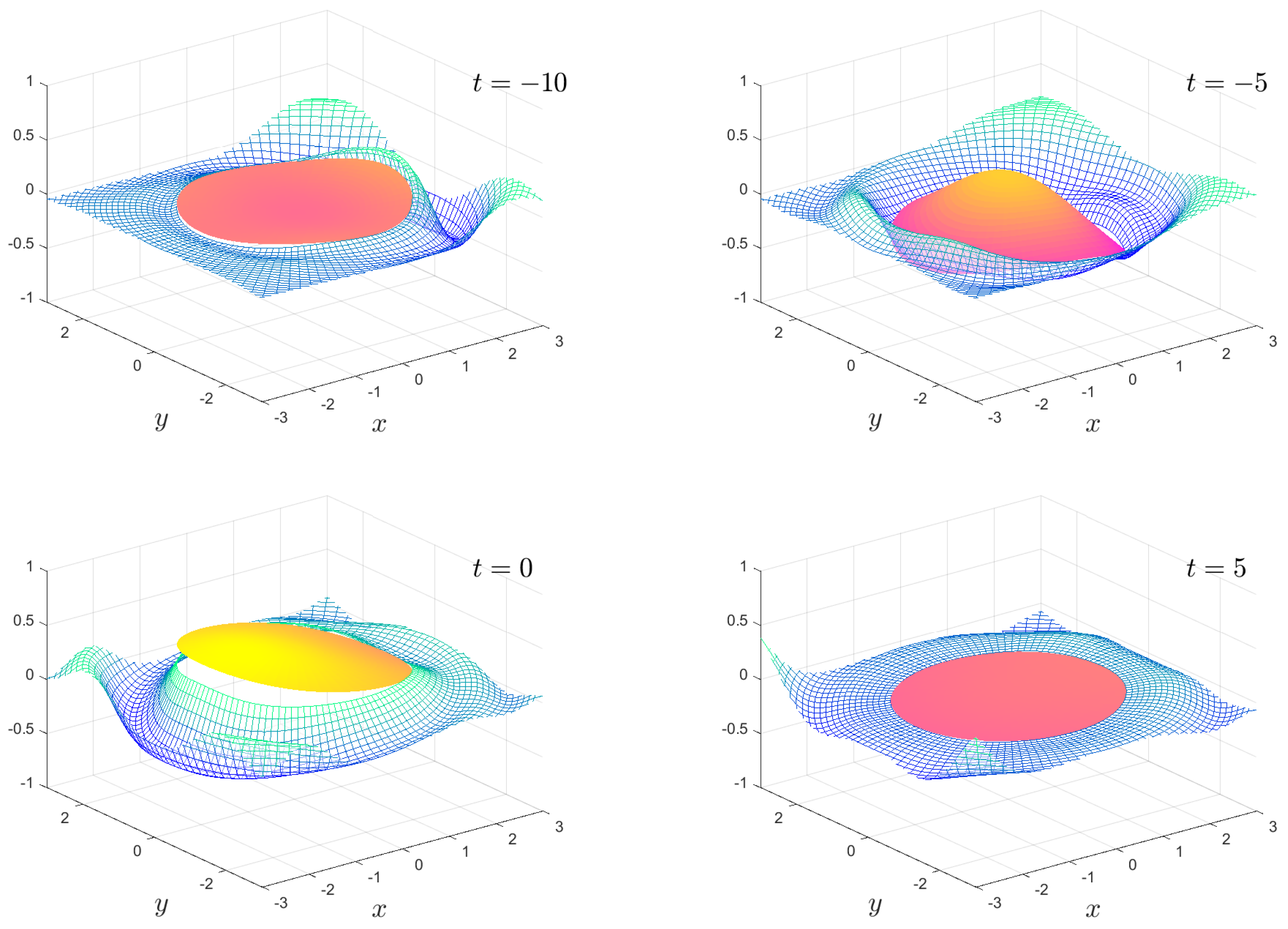
4. Time-Dependent Forcing and Numerical Results
4.1. Plane Incident Wave Forcing
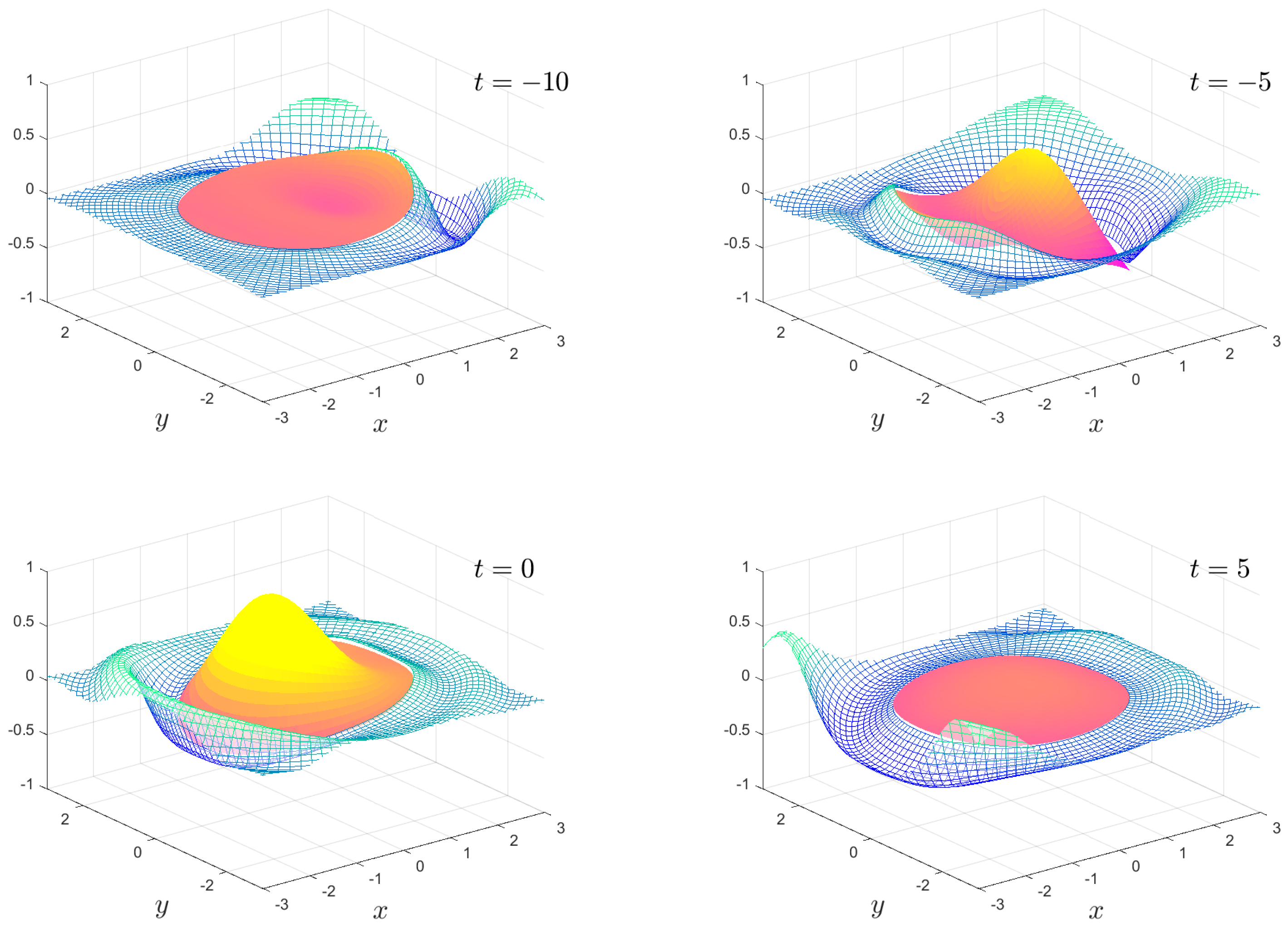
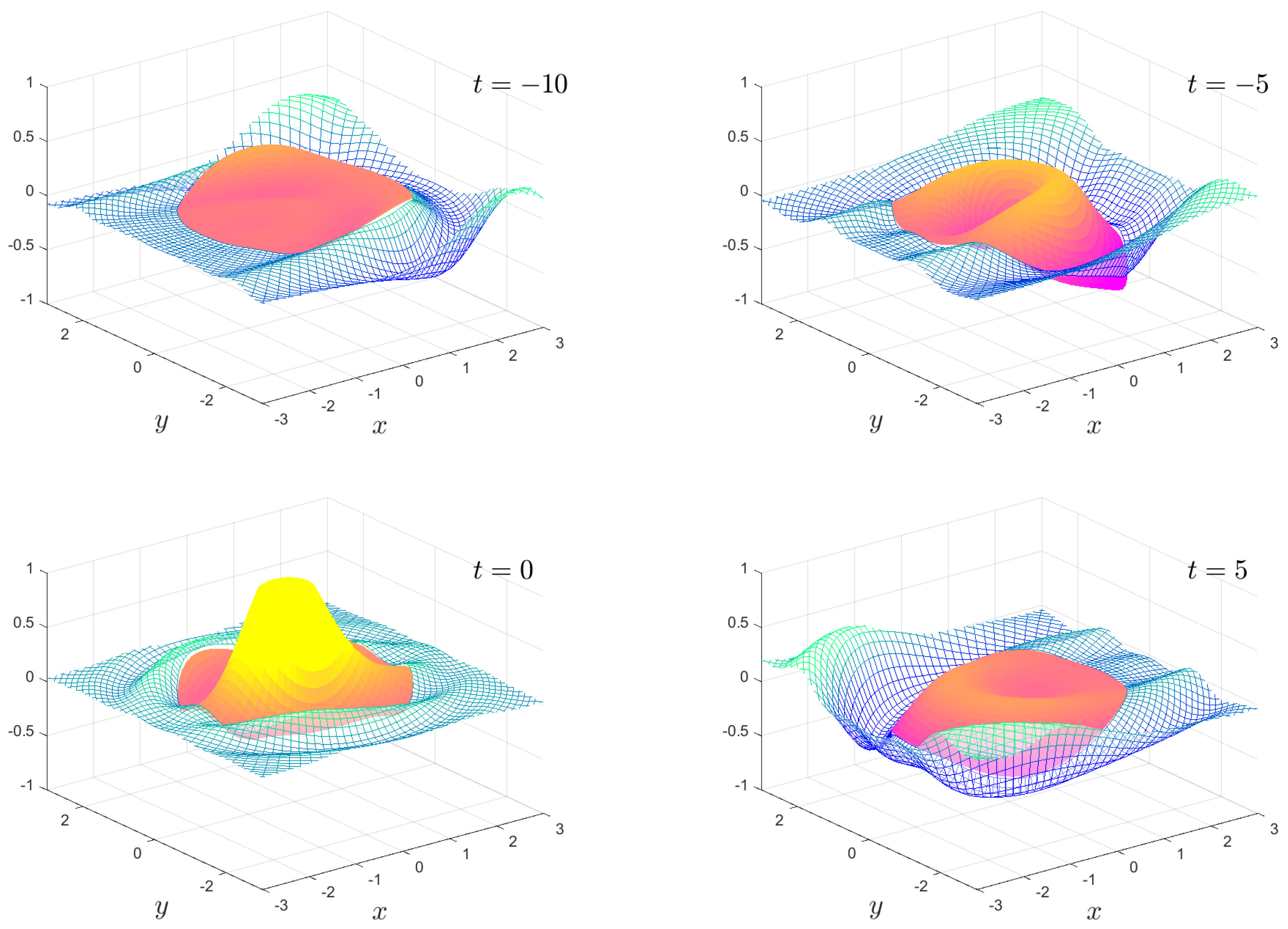
4.2. Focused Wave Group
5. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Conflicts of Interest
References
- Bishop, R.E.D.; Price, W.G.; Wu, Y. A General Linear Hydroelasticity Theory of Floating Structures Moving in a Seaway. Philos. Trans. R. Soc. 1986, 316, 375–426. [Google Scholar]
- Kashiwagi, M. Research on Hydroelastic Response of VLFS: Recent Progressand Future Work. Int. J. Offshore Polar Eng. 2000, 10, 81–90. [Google Scholar]
- Squire, V.A. Synergies Between VLFS Hydroelasticity and Sea Ice Research. Int. J. Offshore Polar Eng. 2008, 18, 1–13. [Google Scholar]
- Watanabe, E.; Utsunomiya, T.; Wang, C.M. Hydroelastic analysis of pontoon-type VLFS: A literature survey. Eng. Struct. 2004, 26, 245–256. [Google Scholar] [CrossRef]
- Newman, J.N. Wave effects on deformable bodies. Appl. Ocean Res. 1994, 16, 45–101. [Google Scholar] [CrossRef]
- Meylan, M.H.; Squire, V.A. The Response of Ice Floes to Ocean Waves. J. Geophy. Res. 1994, 99, 891–900. [Google Scholar] [CrossRef]
- Fox, C.; Squire, V.A. On the Oblique Reflexion and Transmission of Ocean Waves at Shore Fast Sea Ice. Philos. Trans. R. Soc. Lond. A 1994, 347, 185–218. [Google Scholar]
- Zilman, G.; Miloh, T. Hydroelastic Buoyant Circular Plate in Shallow Water: A Closed Form Solution. Appl. Ocean Res. 2000, 22, 191–198. [Google Scholar] [CrossRef]
- Peter, M.A.; Meylan, M.H.; Chung, H. Wave scattering by a circular elastic plate in water of finite depth: A closed form solution. Int. J. Offshore Polar Eng. 2004, 14, 81–85. [Google Scholar]
- Bennetts, L.G.; Biggs, N.R.T.; Porter, D. A multi-mode approximation to wave scattering by ice sheets of varying thickness. J. Fluid Mech. 2007, 579, 413–443. [Google Scholar] [CrossRef]
- Balmforth, N.; Craster, R. Ocean waves and ice sheets. J. Fluid Mech. 1999, 395, 89–124. [Google Scholar]
- Chung, H.; Fox, C. Calculation of wave-ice interaction using the Wiener-Hopf technique. N. Z. J. Math. 2002, 31, 1–18. [Google Scholar]
- Davys, J.W.; Hosking, R.J.; Sneyd, A.D. Waves due to a Steadily Moving Source on a Floating Ice Plate. J. Fluid Mech. 1985, 158, 269–287. [Google Scholar] [CrossRef]
- Hosking, R.J.; Sneyd, A.D.; Waugh, D.W. Viscoelastic Response of a Floating Ice Plate to a Steadily Moving Load. J. Fluid Mech. 1988, 196, 409–430. [Google Scholar] [CrossRef]
- Milinazzo, F.; Shinbrot, M.; Evans, N.W. A Mathematical Analysis of the Steady Response of Floating Ice to the Uniform Motion of a Rectangular Load. J. Fluid Mech. 1995, 287, 173–197. [Google Scholar]
- Squire, V.A.; Hosking, R.J.; Kerr, A.D.; Langhorne, P.J. Moving Loads on Ice Plates; Kluwer: Alphen aan den Rijn, The Netherlands, 1996. [Google Scholar]
- Nugroho, W.; Wang, K.; Hosking, R.; Milinazzo, F. Time-dependent response of a floating flexible plate to an impulsively started steadily moving load. J. Fluid Mech. 1999, 381, 337–355. [Google Scholar]
- Wang, K.; Hosking, R.; Milinazzo, F. Time-dependent response of a floating viscoelastic plate to an impulsively started moving load. J. Fluid Mech. 2004, 521, 295–317. [Google Scholar] [CrossRef]
- Bonnefoy, F.; Meylan, M.; Ferrant, P. Nonlinear higher-order spectral solution for a two-dimensional moving load on ice. J. Fluid Mech. 2009, 621, 215–242. [Google Scholar]
- Meylan, M.H. The forced vibration of a thin plate floating on an infinite liquid. J. Sound Vib. 1997, 205, 581–591. [Google Scholar]
- Meylan, M.H. Spectral Solution of Time Dependent Shallow Water Hydroelasticity. J. Fluid Mech. 2002, 454, 387–402. [Google Scholar]
- Sturova, I.V. Unsteady behavior of an elastic beam floating on shallow water under external loading. J. Appl. Mech. Tech. Phys. 2002, 43, 415–423. [Google Scholar] [CrossRef]
- Sturova, I.V. The action of an unsteady external load on a circular elastic plate floating on shallow water. J. Appl. Maths Mechs. 2003, 67, 407–416. [Google Scholar] [CrossRef]
- Meylan, M.H. The time-dependent vibration of forced floating elastic plates by eigenfunction matching in two and three dimensions. Wave Motion 2019, 88, 21–33. [Google Scholar] [CrossRef]
- Tkacheva, L. Plane problem of vibrations of an elastic floating plate under periodic external loading. J. Appl. Mech. Tech. Phys. 2004, 45, 420–427. [Google Scholar] [CrossRef]
- Tkacheva, L. Action of a periodic load on an elastic floating plate. Fluid Dyn. 2005, 40, 282–296. [Google Scholar] [CrossRef]
- Hazard, C.; Meylan, M.H. Spectral theory for a two-dimensional elastic thin plate floating on water of finite depth. SIAM J. Appl. Math. 2007, 68, 629–647. [Google Scholar] [CrossRef]
- Meylan, M.H.; Sturova, I.V. Time-Dependent Motion of a Two-Dimensional Floating Elastic Plate. J. Fluid. Struct. 2009, 25, 445–460. [Google Scholar]
- Kashiwagi, M. A Time-Domain Mode-Expansion Method for Calculating Transient Elastic Responses of a Pontoon-Type VLFS. J. Mar. Sci. Technol. 2000, 5, 89–100. [Google Scholar] [CrossRef]
- Kashiwagi, M. Transient responses of a VLFS during landing and take-off of an airplane. J. Mar. Sci. Technol. 2004, 9, 14–23. [Google Scholar] [CrossRef]
- Qui, L. Numerical simulation of transient hydroelastic response of a floating beam induced by landing loads. Appl. Ocean Res. 2007, 29, 91–98. [Google Scholar]
- Matiushina, A.A.; Pogorelova, A.V.; Kozin, V.M. Effect of Impact Load on the Ice Cover During the Landing of an Airplane. Int. J. Offshore Polar Eng. 2016, 26, 6–12. [Google Scholar] [CrossRef]
- Endo, H.; Yago, K. Time history response of a large floating structure subjected to dynamic load. J. Soc. Nav. Archit. Jpn. 1999, 1999, 369–376. [Google Scholar] [CrossRef]
- Montiel, F.; Bennetts, L.; Squire, V. The transient response of floating elastic plates to wavemaker forcing in two dimensions. J. Fluids Struct. 2012, 28, 416–433. [Google Scholar] [CrossRef]
- Skene, D.; Bennetts, L.; Wright, M.; Meylan, M.; Maki, K. Water wave overwash of a step. J. Fluid Mech 2018, 839, 293–312. [Google Scholar] [CrossRef]
- Huang, L.; Ren, K.; Li, M.; Tuković, Ž.; Cardiff, P.; Thomas, G. Fluid-structure interaction of a large ice sheet in waves. Ocean Eng. 2019, 182, 102–111. [Google Scholar] [CrossRef]
- Nelli, F.; Bennetts, L.G.; Skene, D.M.; Toffoli, A. Water wave transmission and energy dissipation by a floating plate in the presence of overwash. J. Fluid Mech. 2020, 889, A19. [Google Scholar] [CrossRef]
- Tran-Duc, T.; Meylan, M.H.; Thamwattana, N.; Lamichhane, B.P. Wave Interaction and Overwash with a Flexible Plate by Smoothed Particle Hydrodynamics. Water 2020, 12, 3354. [Google Scholar] [CrossRef]
- Meylan, M.; Bennetts, L.; Cavaliere, C.; Alberello, A.; Toffoli, A. Experimental and theoretical models of wave-induced flexure of a sea ice floe. Phys. Fluids 2015, 27, 041704. [Google Scholar] [CrossRef]
- Kohout, A.L.; Meylan, M.H. Wave Scattering by Multiple Floating Elastic Plates with Spring or Hinged Boundary Conditions. Mar. Struct. 2009, 22, 712–729. [Google Scholar] [CrossRef]
- Kohout, A.L.; Meylan, M.H. An elastic plate model for wave attenuation and ice floe breaking in the marginal ice zone. J. Geophys. Res. 2008, 113. [Google Scholar] [CrossRef]
- Kohout, A.L.; Meylan, M.H.; Sakai, S.; Hanai, K.; Leman, P.; Brossard, D. Linear Water Wave Propagation Through Multiple Floating Elastic Plates of Variable Properties. J. Fluid. Struct. 2007, 23, 649–663. [Google Scholar] [CrossRef]
- Mahmood-ul-Hassan; Meylan, M.H.; Peter, M.A. Water-Wave Scattering by Submerged Elastic Plates. Quart. J. Mech. Appl. Math. 2009, 62, 321–344. [Google Scholar] [CrossRef]
- Behera, H.; Sahoo, T. Hydroelastic analysis of gravity wave interaction with submerged horizontal flexible porous plate. J. Fluids Struct. 2015, 54, 643–660. [Google Scholar] [CrossRef]
- Montiel, F.; Bennetts, L.G.; Squire, V.A.; Bonnefoy, F.; Ferrant, P. Hydroelastic response of floating elastic discs to regular waves. Part 1. Wave basin experiments. J. Fluid Mech. 2013, 723, 604–628. [Google Scholar] [CrossRef]
- Montiel, F.; Bennetts, L.G.; Squire, V.A.; Bonnefoy, F.; Ferrant, P. Hydroelastic response of floating elastic discs to regular waves. Part 1. Modal analysis. J. Fluid Mech. 2013, 723, 629–652. [Google Scholar] [CrossRef]
- Meylan, M.H. The Wave Response of Ice Floes of Arbitrary Geometry. J. Geophys. Res. 2002, 107. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meylan, M.H. Time-Dependent Motion of a Floating Circular Elastic Plate. Fluids 2021, 6, 29. https://doi.org/10.3390/fluids6010029
Meylan MH. Time-Dependent Motion of a Floating Circular Elastic Plate. Fluids. 2021; 6(1):29. https://doi.org/10.3390/fluids6010029
Chicago/Turabian StyleMeylan, Michael H. 2021. "Time-Dependent Motion of a Floating Circular Elastic Plate" Fluids 6, no. 1: 29. https://doi.org/10.3390/fluids6010029
APA StyleMeylan, M. H. (2021). Time-Dependent Motion of a Floating Circular Elastic Plate. Fluids, 6(1), 29. https://doi.org/10.3390/fluids6010029




