Toward Incorporation of Membrane Properties Non-Uniformity in Spiral Wound Module Performance Simulators—Effect of Non-Uniform Permeability on Fouling Layer Evolution

Abstract

A performance simulator of spiral wound membrane (SWM) modules used for desalination is a valuable tool for process design and optimization. The existing state-of-the-art mesoscale simulation tools account for the spatial non-uniformities created by the operation itself (flow, pressure, and concentration distributions) but they assume uniform membrane properties. However, experimental studies reveal that membrane properties are by no means uniform. Therefore, the need arises to account for this non-uniformity in simulation tools thus enabling a systematic assessment of its impact, among other benefits; a first step toward this goal is presented herein. In particular, the issue of an organic fouling layer growing on a membrane with non-uniform permeability is analyzed. Several mathematical treatments of the problem are discussed and indicative results are presented. The concept of fouling layer thickness probability density function is suggested as a means to introduce sub-grid level calculations in existing simulation tools. The analysis leads to the selection of an appropriate methodology to incorporate this effect in the dynamic simulation of fouling layer evolution at the membrane-sheet scale.

1. Introduction

The spiral wound membrane (SWM) modules have been established in reverse osmosis desalination as well as in water treatment and other applications due to their elegant design and their capability of achieving efficient performance with modest energy consumption. In SWM modules, reverse osmosis (RO), nanofiltration (NF) and ultrafiltration (UF) type of membranes are employed for various applications. However, the performance of SWM modules is commonly degraded due mainly to several types of material accumulation on the membranes [1]; i.e., colloidal fouling [2,3], scaling caused by sparingly soluble salts [4], organic matter fouling [5,6] and biofouling [7]. The focus in the present work will be on the common case of fouling by organic macromolecules [6,8] that are ever-present in feed-waters to membrane plants.

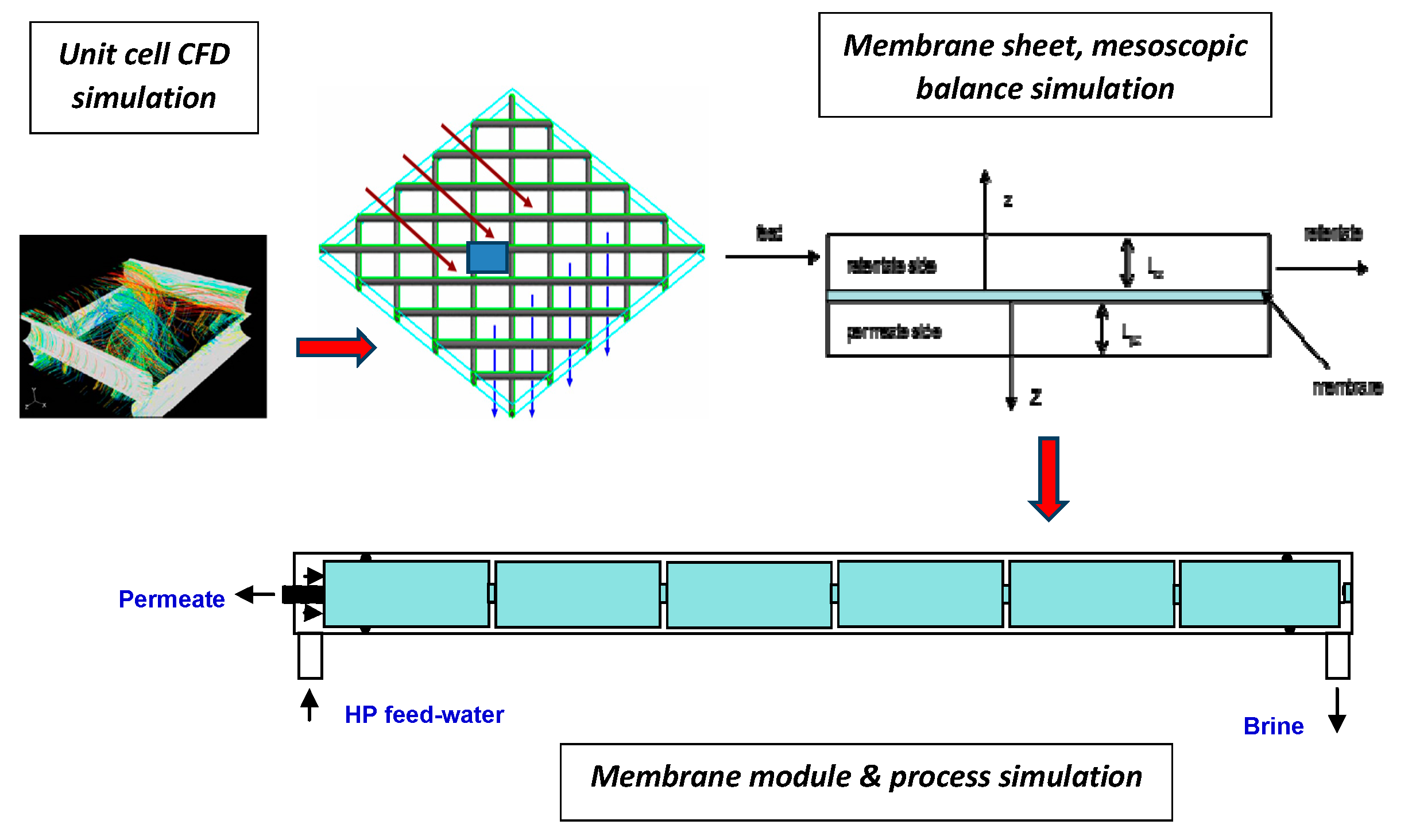
Computational simulation tools of the membrane operation are considered necessary for several types of applications including design and optimization of SWM modules and of entire water treatment plants. In pursuing the development of such model-based tools, it has been recognized that simulating the SWM-module operation necessitates a multi-scale approach; thus, models at several scales are available in the literature, from the small scale (“unit cell”) of feed-spacers in membrane channels [9] to the large scale of an entire membrane sheet/envelope [10]. In efforts to systematically tackle this problem, the present authors have proposed a multiscale approach based on scale decomposition [11]. This approach, indicated in Figure 1, combines the results of spacer-scale Computational Fluid Dynamics simulations [12] with detailed mesoscale balances for the entire membrane sheet [13] and flowsheet level treatment of the membrane module. Regarding organic fouling, the above studies suggest that the fouling layer develops non-uniformly at the membrane-sheet scale due to the spatial variation of trans-membrane pressure and permeate flux, which are caused by the respective spatial variation of retentate-side pressure, permeate-side pressure and osmotic pressure [14]. Moreover, it has been shown that (for a constant permeate recovery operation) as the fouling layer thickness increases there is a tendency for increased uniformity throughout a membrane sheet [14]. This kind of spatial smoothing effect on the evolving fouling layer is due to the increased resistance to permeate flow presented by the growing layer.

In parallel, regarding membrane-material properties, it has been found that the RO membrane permeability is not uniform throughout a membrane sheet, but it can significantly vary with a characteristic length that could be even on the order of a few centimeters [15]. Very recently it has been also shown that disc-shaped ultrafiltration membrane samples (of active area 12.7 cm²) can exhibit a relatively large variation of their permeability [16]. The non-uniform permeability behavior and the related effect on the fouling layer development have not been considered so far in modeling and simulation of membrane operation. However, the impact of membrane permeability on the uniformity of fouling layer thickness can be very significant, since the respective non-uniformity seems to be larger than that due to trans-membrane pressure variation (already considered [13,17]). Therefore, the scope of this work is to analyze the effect of the spatial variation of membrane permeability on the morphology of the evolving organic fouling layer. Recently, the concern about issues dealt with in this paper, and in particular about membrane (non-uniform) permeability effects on fouling, is growing because of the novel high permeability membranes (e.g., aquaporin-and carbon-nanotube-based membranes) which are under development [18]. In addition to improved understanding of the aforementioned effect, a key target of this study is the development of an appropriate methodology to take into account the membrane permeability variation in existing membrane-performance computer-aided simulation tools [13,14,17], whose improvement has been systematically pursued by the authors in recent years.

2. Problem Formulation for Fouling Layer Thickness Evolution

To simplify matters (with no impact on the problem to be analyzed), a membrane module is considered with (i) negligible pressure drop in the permeate side, (ii) negligible osmotic pressure (low solute concentration) and (iii) negligible pressure drop in the retentate side (compared to the trans-membrane pressure). The resulting model can be also considered to hold “locally” on a size scale that is too small compared to the membrane-sheet scale (being of order 10⁴ cm²) but large enough to contain considerable permeability variation. The relation between wall velocity or wall flux and trans-membrane pressure is typically given in terms of membrane flow resistance. The effect of the fouling layer or “cake”, as usually called, is to increase the flow resistance; thus [19]:

$${\mathrm{u}}_{\mathrm{w}}=\frac{1}{\mathsf{\mu }({\mathrm{R}}_{\mathrm{m}}+{\mathrm{R}}_{\mathrm{c}})}\Delta \mathrm{p}$$

(1)

where u_w is the wall flux, R_m and R_c are the clean membrane resistance and the time-dependent fouling layer/cake resistance, respectively, μ is the fluid viscosity and Δp is the trans-membrane pressure which for the present conditions is the same as the feed liquid pressure p(t). The fouling layer resistance is proportional to the deposit mass per unit surface area m; i.e., R_c = αm, where α is the specific cake resistance. Typically, α is considered to be affected by permeate flux, pressure and possibly deposit thickness [6,8]. Moreover, recent experimental studies of organic fouling layer formation in channels with spacers have shown that the results can be described by a rather simple relation between specific fouling/cake resistance α and wall flux [20]; i.e., α = γ(u_w)^β. This type of relation (obtained in the authors’ laboratory) greatly simplifies the mathematical analysis of fouling layer non-uniformity and will be adopted here. The analysis will be made in terms of membrane permeability (inverse of resistance) instead of membrane resistance since it facilitates handling of averages; it is noted that arithmetic instead of harmonic average must be considered.

Furthermore, it is considered that the membrane permeability is non-uniform on the membrane sheet and it is described by k(x,y) where x and y designate planar coordinates in the direction along the mean flow and normal to this flow, respectively. Summarizing the above discussion, it can be readily shown that the local wall flux is given as

$${\mathrm{u}}_{\mathrm{w}}=\frac{\mathrm{k}\mathrm{p}}{\mathsf{\mu }(1+\mathrm{k}\mathsf{\gamma }{\mathrm{u}}_{\mathrm{w}}^{\mathsf{\beta }}\mathrm{m})}$$

(2)

The mass surface density m on the membrane is related to the fouling layer thickness h as h = m/ρ_f where ρ_f is the layer density. A simple evolution equation for h is obtained by considering that all the organic material contained in the filtered water is deposited (as the macromolecules completely follow the fluid streamlines), thus contributing to fouling layer formation:

$${\mathsf{\rho }}_{\mathrm{f}}\frac{\mathrm{d}\mathrm{h}}{\mathrm{d}\mathrm{t}}={\mathrm{u}}_{\mathrm{w}}\mathrm{c}$$

(3)

where c is the mass concentration of depositing organic compounds in the liquid. In general, under conditions of constant-flux operation, the feed pressure tends to increase with time due to increasing fouling resistance. Its initial value (at t = 0) is designated as p_o. The average value of the permeability is defined as ${\mathrm{k}}_{\mathrm{m}}=\frac{1}{\mathrm{A}}{\displaystyle \underset{\mathrm{A}}{\overset{}{\int }}\mathrm{k}\mathrm{d}\mathrm{A}}$, where A is the membrane area; furthermore, the reference wall flux is defined as ${\mathrm{u}}_{\mathrm{w}\mathrm{o}}={\mathrm{k}}_{\mathrm{m}}{\mathrm{p}}_{\mathrm{o}}/\mathsf{\mu }$. Introducing the following dimensionless variables: $\mathrm{P}=\mathrm{p}/{\mathrm{p}}_{\mathrm{o}}$,${\mathrm{U}}_{\mathrm{w}}={\mathrm{u}}_{\mathrm{w}}/{\mathrm{u}}_{\mathrm{w}\mathrm{o}}$, $\mathrm{K}=\mathrm{k}/{\mathrm{k}}_{\mathrm{m}}$, $\mathrm{H}=\mathsf{\gamma }{\mathrm{k}}_{\mathrm{m}}{\mathrm{u}}_{\mathrm{w}\mathrm{o}}^{\mathsf{\beta }}{\mathsf{\rho }}_{\mathrm{f}}\mathrm{h}$, $\mathrm{T}=\mathsf{\gamma }{\mathrm{k}}_{\mathrm{m}}{\mathrm{u}}_{\mathrm{w}\mathrm{o}}^{\mathsf{\beta }+1}\mathrm{c}\mathrm{t}$, the governing equation of fouling layer growth takes the form:

$$\frac{\mathrm{d}\mathrm{H}}{\mathrm{d}\mathrm{T}}={\mathrm{U}}_{\mathrm{w}}$$

(4)

$${\mathrm{U}}_{\mathrm{w}}=\frac{\mathrm{P}\mathrm{K}}{1+\mathrm{K}{\mathrm{U}}_{\mathrm{w}}^{\mathsf{\beta }}\mathrm{H}}$$

(5)

The above system must be solved for each location of the membrane (x,y) with initial condition H = 0. The solution of the system will yield the spatio-temporal evolution of the layer thickness H(x,y,T). It is noted that Equation (5) is implicit with respect to U_w, thus it must be solved numerically at each time step and spatial location. In case of constant pressure operation of the membrane, one can simply set P = 1 in Equation (5). In case of constant flux operation, the additional condition

$$\frac{1}{\mathrm{A}}{\displaystyle \underset{\mathrm{A}}{\overset{}{\int }}\frac{\mathrm{d}\mathrm{H}}{\mathrm{d}\mathrm{T}}\mathrm{d}\mathrm{A}}=1$$

(6)

is used to determine the feed pressure evolution P(T) due to fouling. This equation is just a statement that all the foulant mass entering the system contributes to the increase of fouling layer. A major simplification arises for the particular case of β = 1 (which is in the range of β values found experimentally [20]). In this case, Equation (5) can be solved analytically, leading to the following explicit equation for the layer thickness evolution:

$$\frac{\mathrm{d}\mathrm{H}}{\mathrm{d}\mathrm{T}}=\frac{-1+{(1+4{\mathrm{K}}^2\mathrm{H}\mathrm{P})}^{1/2}}{2\mathrm{K}\mathrm{H}}$$

(7)

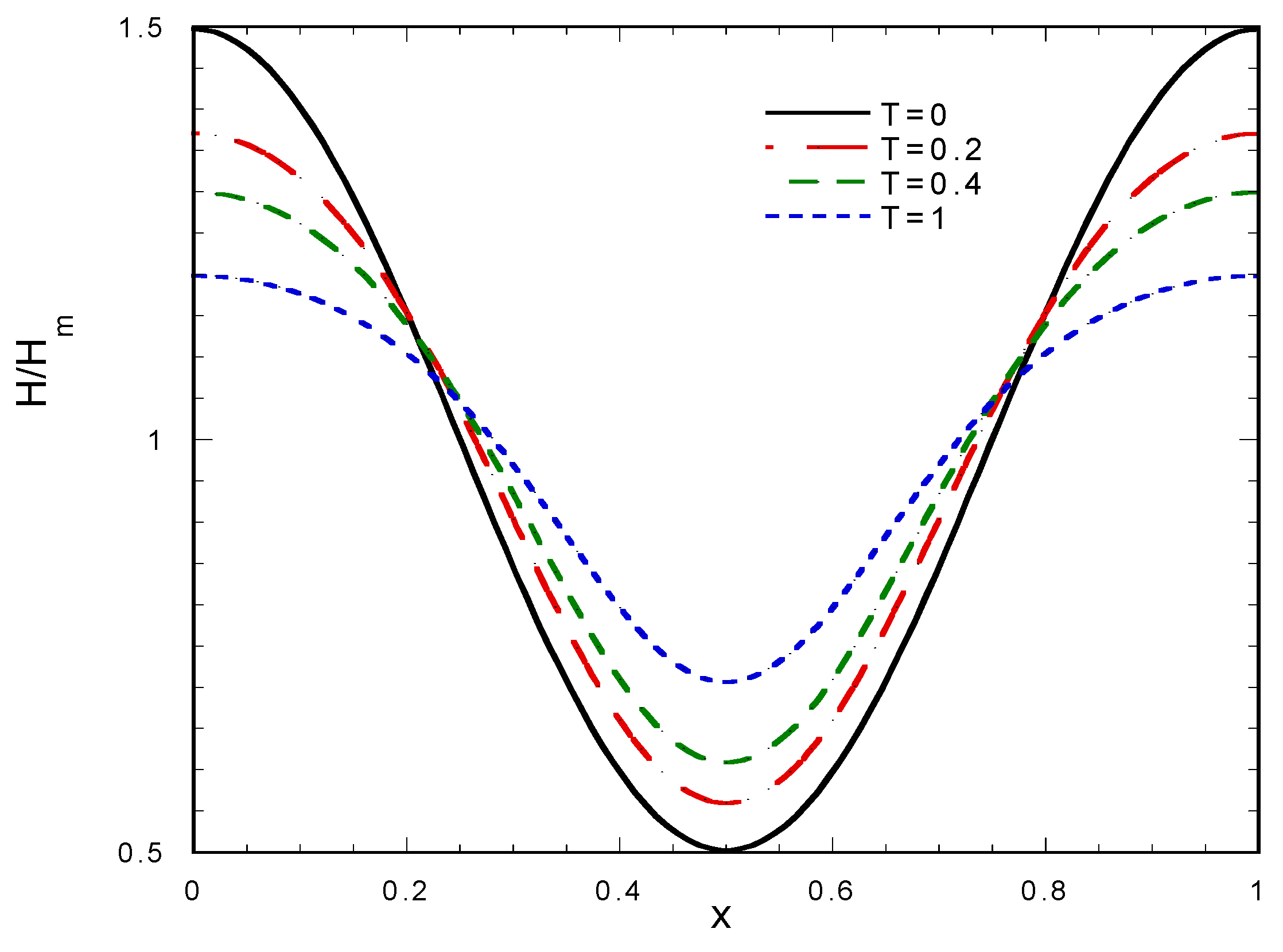
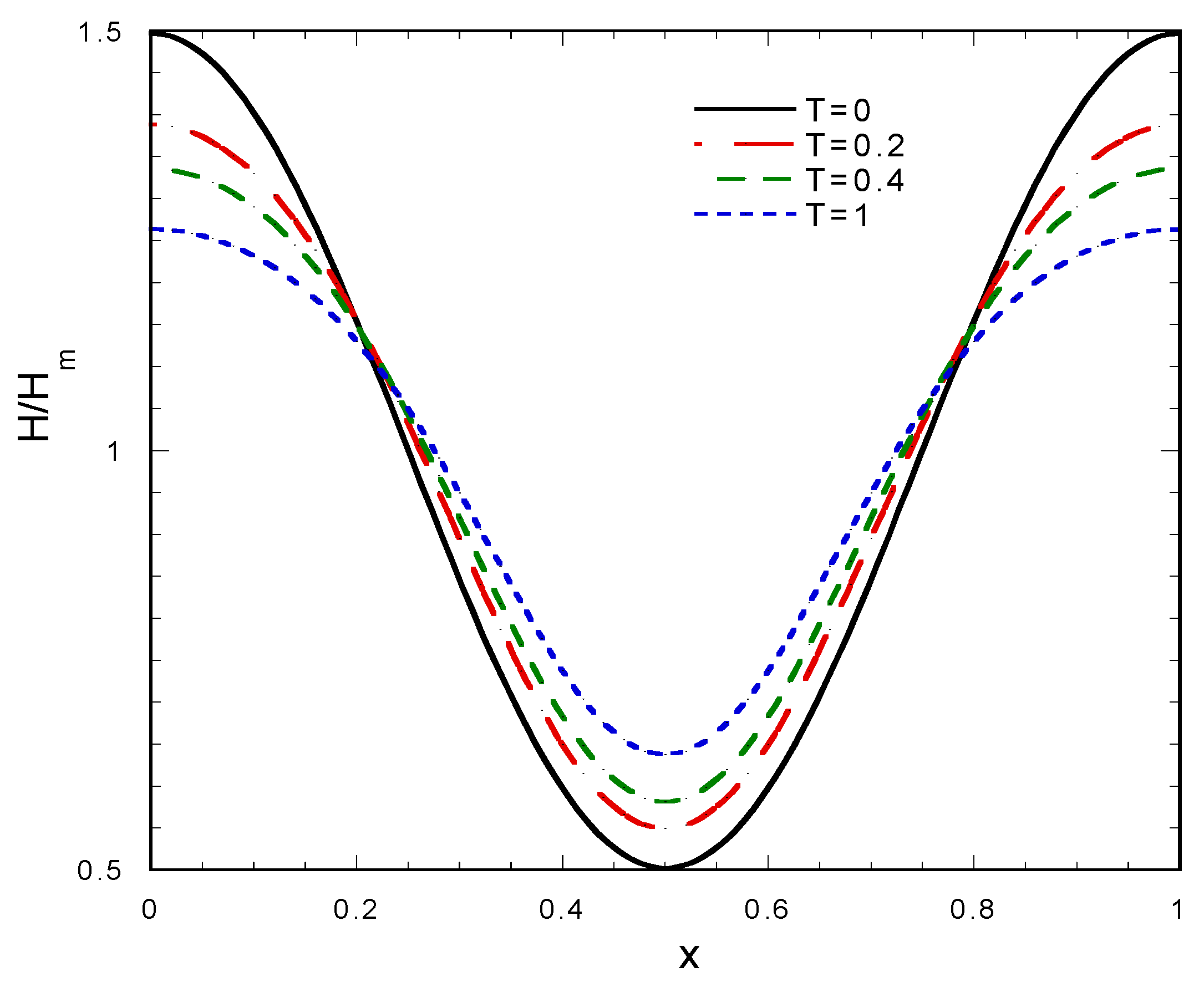
A one-dimensional permeability profile of the form K(x) = 1 + 0.5cos(2πx) is assumed and the corresponding fouling layer evolution problem is solved for constant pressure (P = 1) and constant flux (P from condition (6)). The use of harmonic functions, as the one employed here, to represent non-uniform property or geometry variation is typical in science and engineering literature [21]. This treatment is focused on the evolution of non-uniformity of H; therefore, the evolution of the ratio H/H_m (where H_m is the average film thickness) is shown in Figure 2 and Figure 3 for operation under constant pressure and constant flux, respectively.

In both cases the initial profile of H is similar to that of K. As the layer grows, the relative non-uniformity decreases due to the smoothing effect of H in the denominator of Equation (5). The higher the H the smaller the wall flux, leading to a self-regulation of the growing fouling layer non-uniformity. On the other hand, the quantity U_w in the denominator of Equation (5) also affects this smoothing process. The normalized profile depends mainly on the total amount of material accumulated (equivalent to H_m) which increases faster with T for constant flux, compared to constant pressure, because in the latter case the flux tends to be reduced with time. Dealing with characteristic length of K variability will be discussed in subsequent Section 5.

3. Problem Formulation for the Evolution of Thickness Probability Density Function

The above procedure of computing the fouling layer shape evolution requires many discretization points in order to cope with the shape of the function K(x,y). For example, a membrane sheet with dimensions 1 m × 1 m and characteristic area of K variation 1 cm × 1 cm needs 10,000 discretization points to be modeled. For the present simplified example, such a computational effort is acceptable. However, in case of a realistic membrane simulation, fully coupled non-linear balances must be solved for each discretization point; thus, the problem becomes computationally cumbersome [22]. To proceed, an important observation is that the fouling layer evolution model is non-topological; i.e., the thickness evolution at one point is independent of the shape of the fouling layer in its neighborhood. This means that the fouling layer, and its evolution, can be described by its probability density function (PDF) instead of its detailed shape. In the same spirit, very recently, the use of the PDF of mass transfer coefficient (computed by CFD codes) is proposed for the computation of permeate concentration without incorporating wall flow in CFD simulations [23]. Dealing with the evolution of PDF is computationally much easier than with the evolution of the detailed layer shape. Therefore, to proceed, the permeability distribution K(x,y) is described through its probability density function g(K). The physical meaning of this function is that the fraction of the membrane surface having permeability between K and K + dK is g(K)dK. By definition, and considering that K is a normalized variable, one obtains:

$${\displaystyle \underset{0}{\overset{\infty }{\int }}\mathrm{g}(\mathrm{K})\mathrm{d}\mathrm{K}}=1,{\displaystyle \underset{0}{\overset{\infty }{\int }}\mathrm{K}\mathrm{g}(\mathrm{K})\mathrm{d}\mathrm{K}}=1$$

(8)

The lower bound of integral K = 0 denotes a completely impermeable region of the membrane. The upper bound K = ∞ denotes a kind of rupture in the membrane and for any practical purpose g(K) goes to zero as K goes to infinity; thus, the upper bound in the integrals is retained only for generality (instead of truncating at a finite value). Furthermore, the function H(K,t) is introduced describing the local thickness evolution at a location with permeability K. The equation governing dynamics of this function is

$$\frac{\partial \mathrm{H}(\mathrm{K},\mathrm{T})}{\partial \mathrm{T}}={\mathrm{U}}_{\mathrm{w}}$$

(9)

In the following, the focus will be on the case β = 1 (i.e., for specific resistance α = γu_w) that allows more explicitly derived model equations; then, Equation (9) takes the form:

$$\frac{\partial \mathrm{H}(\mathrm{K},\mathrm{T})}{\partial \mathrm{T}}=\frac{-1+{(1+4{\mathrm{K}}^2\mathrm{H}\mathrm{P})}^{1/2}}{2\mathrm{K}\mathrm{H}}$$

(10)

The average thickness H_m is computed by integrating over the permeability PDF:

$${\mathrm{H}}_{\mathrm{m}}(\mathrm{T})={\displaystyle \underset{0}{\overset{\infty }{\int }}\mathrm{H}(\mathrm{K},\mathrm{T})\mathrm{g}(\mathrm{K})\mathrm{d}\mathrm{K}}$$

(11)

The quantity of interest here is the PDF of fouling layer thickness f(H). The fraction of membrane surface covered by layer of thickness between H and H + dH is f(H)dH. The function f(H) can be constructed at each time step using the functions H(K) and g(K) as:

$$\mathrm{f}(\mathrm{H})=\mathrm{g}(\mathrm{K}){\left(\frac{\mathrm{d}\mathrm{H}}{\mathrm{d}\mathrm{K}}\right)}^{-1}$$

(12)

The discretization points, in this case, are determined by the need to sample the function g(K) and to construct H(K). Their number can be a few decades instead of thousands needed to follow the detailed shape evolution. The initial condition for the model is H(K) = 0; additionally, for the constant pressure case P = 1, whereas for constant flux the pressure evolution must be determined from the following condition, which must hold always (i.e., for every T value).

$${\displaystyle \underset{0}{\overset{\infty }{\int }}\frac{-1+{(1+4{\mathrm{K}}^2\mathrm{H}\mathrm{P})}^{1/2}}{2\mathrm{K}\mathrm{H}}\mathrm{g}(\mathrm{K})\mathrm{d}\mathrm{K}=1}$$

(13)

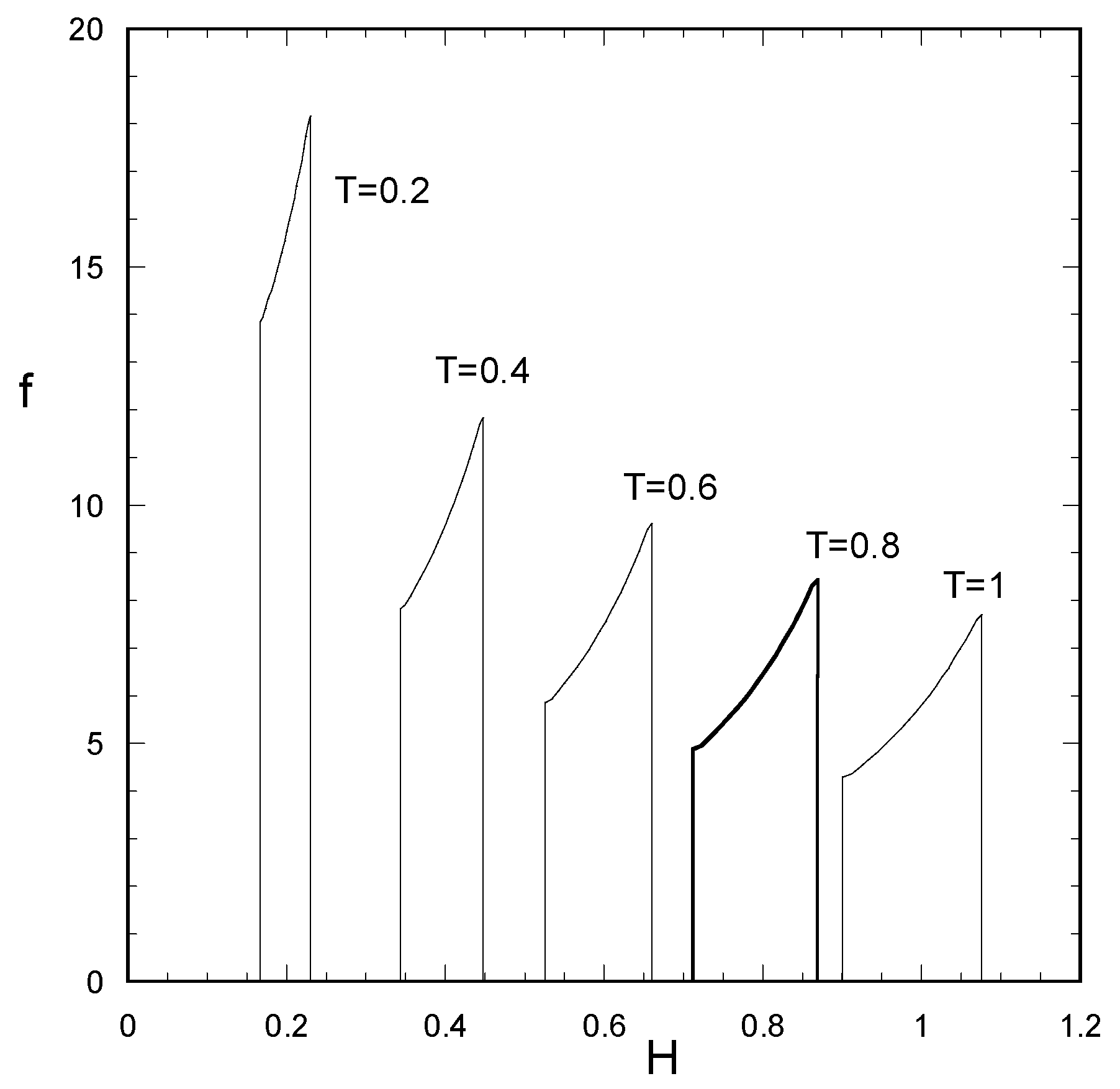
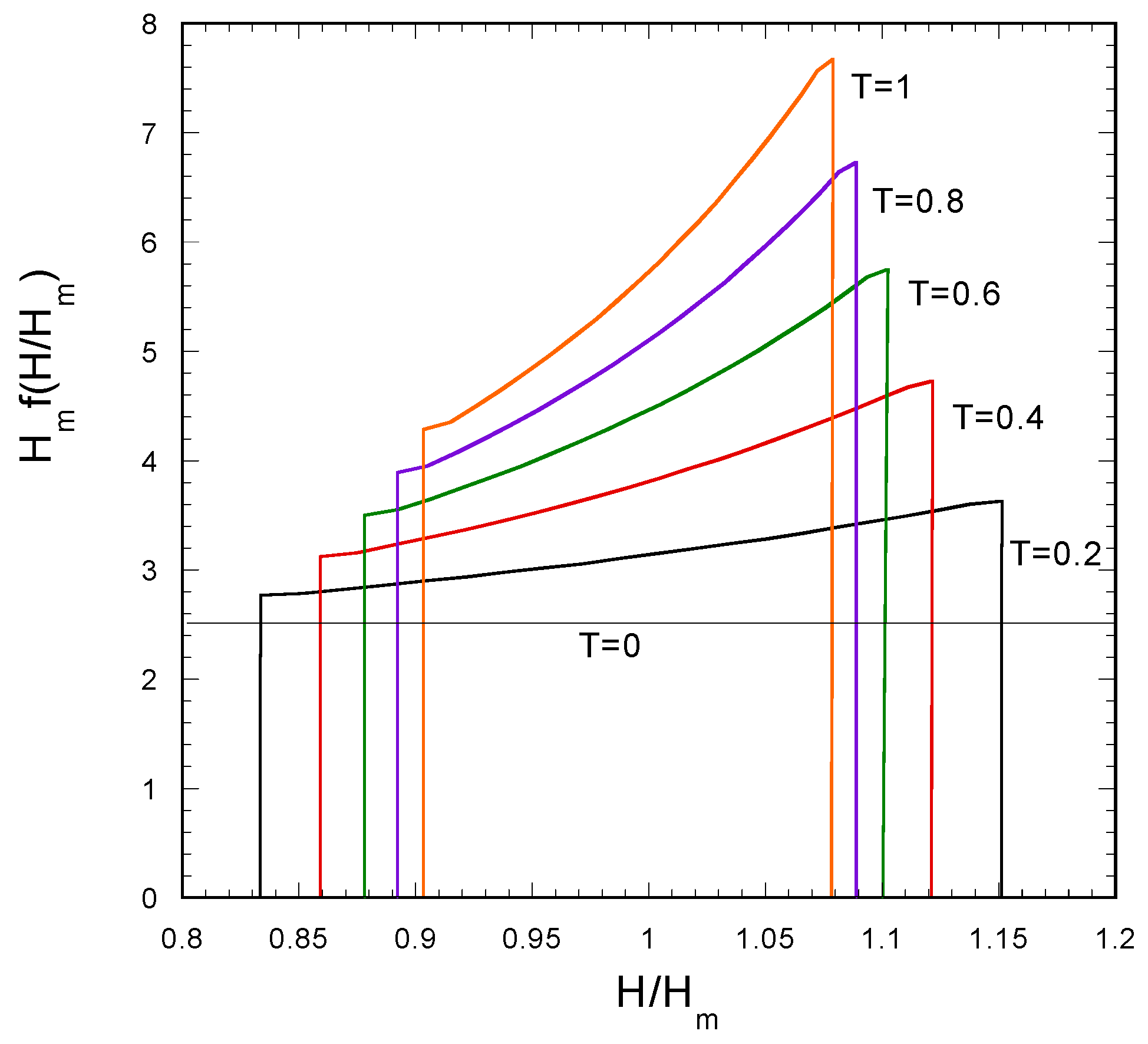
To demonstrate this approach, a membrane is considered with uniformly distributed permeability between 80% and 120% of its average value. The evolution of the PDF of H for the case of constant flux is shown in Figure 4. The way of reading this figure is the following: for a particular value of H in horizontal axis, the corresponding probability of existence appears in vertical axis. As time proceeds, the existing values of H increase and the PDF appears to broaden; however, this picture is misleading. In order to assess the evolution of the morphology, the normalized thickness H/H_m and the corresponding PDF should be considered (i.e., the same approach followed in presenting layer shape in Figure 1 and Figure 2). The evolution of the PDF of the variable H/H_m, under the same conditions as those of Figure 4, is shown in Figure 5. Initially, the PDF of H/H_m is the same with the PDF of K. As time proceeds the PDF starts narrowing. This is due to the fact that the growth rate is not the same for all H; actually it decreases with increasing H, so there is an accumulation of material (i.e., increase of layer thicknesses) in a smaller region of H/H_m followed by reduction of the spread of the corresponding PDF. The shape of the PDF also varies in time. The initial uniform distribution evolves to one with higher probability at its upper edge. This shape is also due to the reduction of growth rate as H increases, leading to probability accumulation in the region of high H/H_m values.

A Special Result: Small H First Order Perturbation Solution

To get additional analytical insight into the problem of thickness-PDF evolution, a first order perturbation expansion (small H limit) will be dealt with. The perturbation will be with respect to the basic solution with U_w = PK and H = PKT, resulting from Equations (4) and (5) in the limit H→0. Let us consider that

$${\mathrm{U}}_{\mathrm{w}}=\mathrm{P}\mathrm{K}+{{\mathrm{U}}^{\prime }}_{\mathrm{w}}^{}$$

(14)

where the prime denotes the first-order correction term. Replacing in Equation (5) and taking the Taylor expansion of the denominator leads to

$$\mathrm{P}\mathrm{K}+{{\mathrm{U}}^{\prime }}_{\mathrm{w}}^{}=\mathrm{P}\mathrm{K}(1-\mathrm{K}\mathrm{H}{(\mathrm{P}\mathrm{K}+{{\mathrm{U}}^{\prime }}_{\mathrm{w}}^{})}^{\mathsf{\beta }})$$

(15)

Taking the Taylor expansion of the power term, and retaining only the dominant term for the primed flux, leads to

$${{\mathrm{U}}^{\prime }}_{\mathrm{w}}^{}=-\mathrm{P}{\mathrm{K}}^2\mathrm{H}{(\mathrm{P}\mathrm{K})}^{\mathsf{\beta }}$$

(16)

Keeping only the zeroth and first order expansion terms of U_w leads to the following evolution equation for H:

$$\frac{\mathrm{d}\mathrm{H}}{\mathrm{d}\mathrm{T}}=\mathrm{P}\mathrm{K}-{\mathrm{P}}^{1+\mathsf{\beta }}{\mathrm{K}}^{2+\mathsf{\beta }}\mathrm{H}$$

(17)

In the particular case of constant pressure (i.e., P = 1) the above differential equation can be solved analytically leading to

$$\mathrm{H}={\mathrm{K}}^{-1-\mathsf{\beta }}(1-\exp (-{\mathrm{K}}^{2+\mathsf{\beta }}\mathrm{T}))$$

(18)

Keeping in mind that the analysis refers to first order correction in H (so, based on the zero order solution, KT is also small) and given that K is of order unity, the above exponential can be expanded to give

$$\mathrm{H}=\mathrm{K}\mathrm{T}(1-\frac{1}{2}{\mathrm{K}}^{2+\mathsf{\beta }}\mathrm{T})$$

(19)

Substitution of this result in Equation (12) leads, after some algebra, to the following first order analytical solution for the evolving PDF of H

$$\mathrm{f}(\mathrm{H})=\mathrm{g}(\frac{\mathrm{H}}{\mathrm{T}}(1+\frac{1}{2}\frac{{\mathrm{H}}^{2+\mathsf{\beta }}}{{\mathrm{T}}^{1+\mathsf{\beta }}}))(\frac{1}{\mathrm{T}}+\frac{(3+\mathsf{\beta })}{2}\frac{{\mathrm{H}}^{2+\mathsf{\beta }}}{{\mathrm{T}}^{2+\mathsf{\beta }}})$$

(20)

Furthermore, F_i and G_i define the i-th moments of the functions f and g, respectively; i.e.,

$${\mathrm{F}}_{\mathrm{i}}={\displaystyle \underset{0}{\overset{\infty }{\int }}{\mathrm{H}}^{\mathrm{i}}\mathrm{f}(\mathrm{H})\mathrm{d}\mathrm{H}},{\mathrm{G}}_{\mathrm{i}}={\displaystyle \underset{0}{\overset{\infty }{\int }}{\mathrm{K}}^{\mathrm{i}}\mathrm{g}(\mathrm{K})\mathrm{d}\mathrm{K}}$$

(21)

Substitution of the relation (20) into the equation for the moments F_i leads to:

F_o = 1

(22)

$${\mathrm{F}}_1=\mathrm{T}-\frac{1}{2}{\mathrm{G}}_{3+\mathsf{\beta }}{\mathrm{T}}^2$$

(23)

$${\mathrm{F}}_2={\mathrm{G}}_2{\mathrm{T}}^2-{\mathrm{G}}_{4+\mathsf{\beta }}{\mathrm{T}}^3+\frac{1}{4}{\mathrm{G}}_{6+2\mathsf{\beta }}{\mathrm{T}}^4$$

(24)

The standard deviation σ_f of the layer thickness is given as

$${\mathsf{\sigma }}_{\mathrm{f}}={(\frac{{\mathrm{F}}_2}{{\mathrm{F}}_{\mathrm{o}}}-{(\frac{{\mathrm{F}}_1}{{\mathrm{F}}_{\mathrm{o}}})}^2)}^{1/2}$$

(25)

Substituting in Equation (25) the relations for F_i, expanding with respect to T and retaining only the first two terms, leads to

$${\mathsf{\sigma }}_{\mathrm{f}}={\mathsf{\sigma }}_{\mathrm{g}}\mathrm{T}(1-\frac{1}{2}\frac{{\mathrm{G}}_{4+\mathsf{\beta }}-{\mathrm{G}}_{3+\mathsf{\beta }}}{{\mathrm{G}}_2-1}\mathrm{T})$$

(26)

where σ_g = (G₂-1)^1/2 is the standard deviation of the permeability. Consequently, the standard deviation of the layer thickness increases in proportion to the standard deviation of the permeability, whereas the retarding effect of the denominator of Equation (5) is expressed through the coefficient ${\mathrm{C}}_{\mathrm{f}}=\frac{1}{2}\frac{{\mathrm{G}}_{4+\mathsf{\beta }}-{\mathrm{G}}_{3+\mathsf{\beta }}}{{\mathrm{G}}_2-1}$. To demonstrate the effect of β and of the spread of the permeability distribution on retarding the non-uniformity of layer thickness distribution, a uniform shape of g between K = 1 − ε and K = 1 + ε will be considered. The value of ε is between 0 and 1 and denotes the spread of the permeability distribution. Replacing in equation for C_f leads to

$${\mathrm{C}}_{\mathrm{f}}=\frac{1}{2}[\frac{1}{5+\mathsf{\beta }}({(1+\mathsf{\epsilon })}^{5+\mathsf{\beta }}-{(1-\mathsf{\epsilon })}^{5+\mathsf{\beta }})-\frac{1}{4+\mathsf{\beta }}({(1+\mathsf{\epsilon })}^{4+\mathsf{\beta }}-{(1-\mathsf{\epsilon })}^{4+\mathsf{\beta }})]{[\frac{1}{3}({(1+\mathsf{\epsilon })}^3-{(1-\mathsf{\epsilon })}^3)-2\mathsf{\epsilon }]}^{-1}$$

(27)

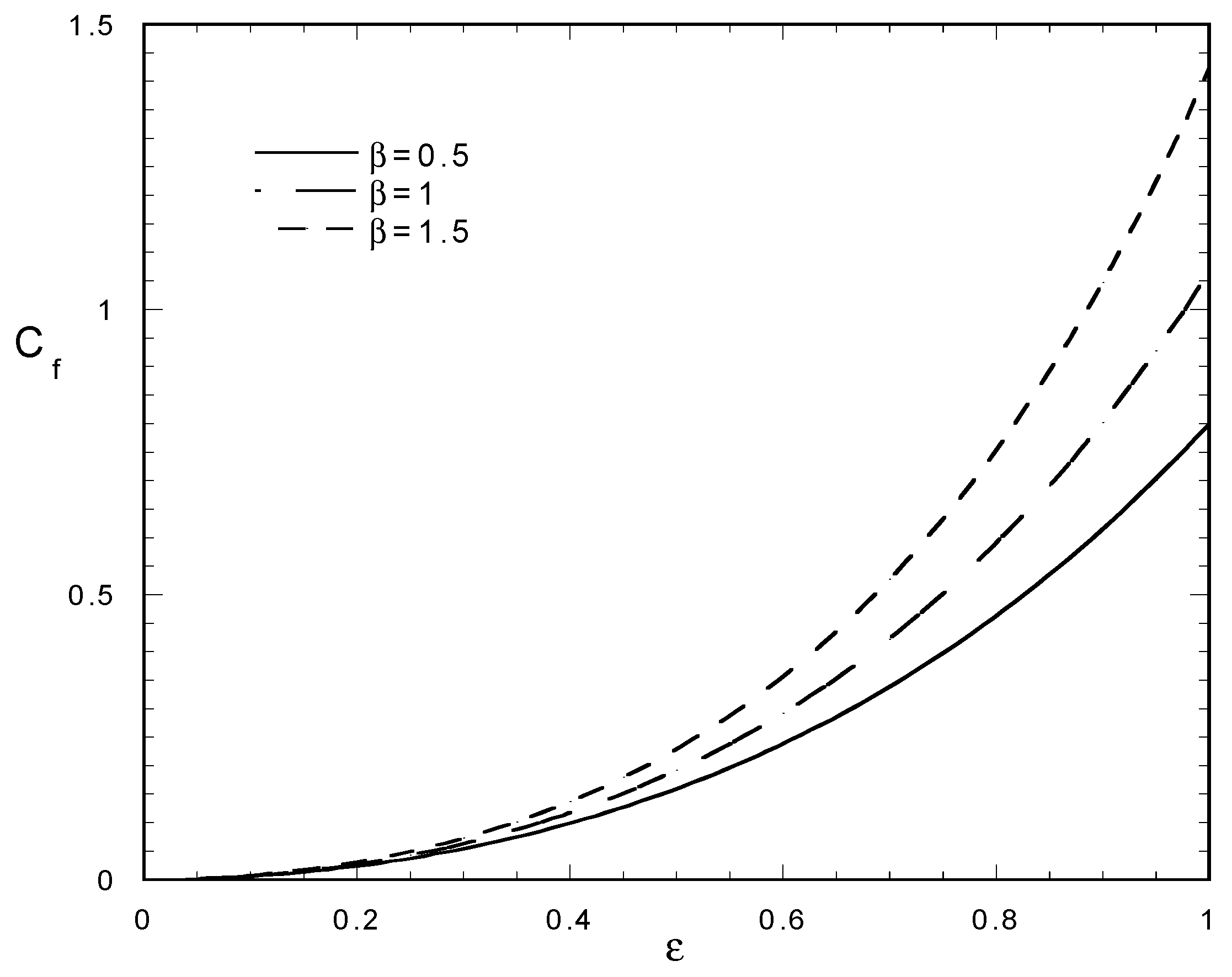
In Figure 6, the coefficient C_f is shown as a function of ε, for three values of β. As expected, the retardation coefficient tends to increase with increasing spread of the permeability distribution (leading to spread of wall fluxes). It is interesting that C_f also increases with the exponent β. This can be explained by observing that the larger the β the larger the dampening of the permeability nonuniformity by wall flux. It is noted that Equation (26) resulted from a first order perturbation expansion, thus it holds for T much smaller than one.

4. Effect of Concentration Polarization on Thickness Uniformity

The approach presented herein can be generalized to include additional phenomena such as the concentration polarization [24] and the cake enhanced concentration polarization [25,26]. Let us consider that the bulk concentration of the solute is non-negligible (in terms of osmotic pressure) and in the examined region of the module it is equal to c_b. The wall concentration in this region (assuming complete solute rejection) will be ${\mathrm{c}}_{\mathrm{w}}={\mathrm{c}}_{\mathrm{b}}{\mathrm{e}}^{{\mathrm{u}}_{\mathrm{w}}/{\mathrm{k}}_{\mathrm{m}\mathrm{t}}}$, where k_mt is the mass transfer coefficient. In the presence of spacers, the exponent in the above relation is much smaller than one [12], so a linearization is possible; i.e., c_w = c_b(1 + u_w/k_mt). The mass transfer coefficient has contributions from two resistances in series; the first is the convective diffusion in the liquid and the second the diffusion through the fouling layer (i.e., the so-called “cake” enhanced polarization). In particular, ${\mathrm{k}}_{\mathrm{m}\mathrm{t}}={(\frac{1}{{\mathrm{k}}_{\mathrm{m}\mathrm{t}\mathrm{o}}}+\frac{\mathrm{h}}{{\mathrm{D}}_{\mathrm{e}}})}^{-1}$ where k_mto is the liquid side mass transfer coefficient and D_e is the effective solute diffusivity in the fouling layer. Summarizing, the relation for wall flux takes the form:

$${\mathrm{u}}_{\mathrm{w}}=\frac{\mathrm{K}(\mathrm{p}-\mathsf{\Gamma }{\mathrm{c}}_{\mathrm{b}}(1+{\mathrm{u}}_{\mathrm{w}}(1/{\mathrm{k}}_{\mathrm{m}\mathrm{t}\mathrm{o}}+\mathrm{h}/{\mathrm{D}}_{\mathrm{e}}))}{\mathsf{\mu }(1+\mathrm{K}\mathsf{\gamma }{\mathrm{u}}_{\mathrm{w}}^{\mathsf{\beta }}\mathrm{h}{\mathsf{\rho }}_{\mathrm{f}})}$$

(28)

A non-dimensionalization different than the previous one will be introduced here. The constant pressure case is examined, so p is constant and equal to p_o. The reference flux is u_wo = K(p_o − Γc_b)/μ. The other dimensionless variables are as before. The dimensionless equation for the flux is:

$${\mathrm{U}}_{\mathrm{w}}=\frac{\mathrm{K}(1+\mathrm{E}{\mathrm{U}}_{\mathrm{w}}(\mathrm{Z}+\mathrm{H}/\Delta ))}{1+\mathrm{K}{\mathrm{U}}_{\mathrm{w}}^{\mathsf{\beta }}\mathrm{H}}$$

(29)

where E = Γc_b/(p_o − Γc_b), Z = u_wo/k_mto, $\Delta ={\mathrm{D}}_{\mathrm{e}}/(\mathsf{\gamma }{\mathrm{k}}_{\mathrm{m}}{\mathrm{u}}_{\mathrm{w}\mathrm{o}}^{\mathsf{\beta }+1}{\mathsf{\rho }}_{\mathrm{f}})$. A typical value of Z for a spiral wound module equal to 0.2 will be assumed here in order to demonstrate the effect of dimensionless osmotic coefficient E, and of dimensionless diffusivity in the fouling layer Δ, on fouling layer uniformity evolution. The particular case β = 1 will be considered that allows a closed form solution for the wall flux:

$${\mathrm{U}}_{\mathrm{w}}=(-(1+\mathrm{K}\mathrm{E}(\mathrm{Z}+\mathrm{H}/\Delta ))+{[{(1+\mathrm{K}\mathrm{E}(\mathrm{Z}+\mathrm{H}/\Delta ))}^2-4{\mathrm{K}}^2\mathrm{H}]}^{1/2})/(2\mathrm{K}\mathrm{H})$$

(30)

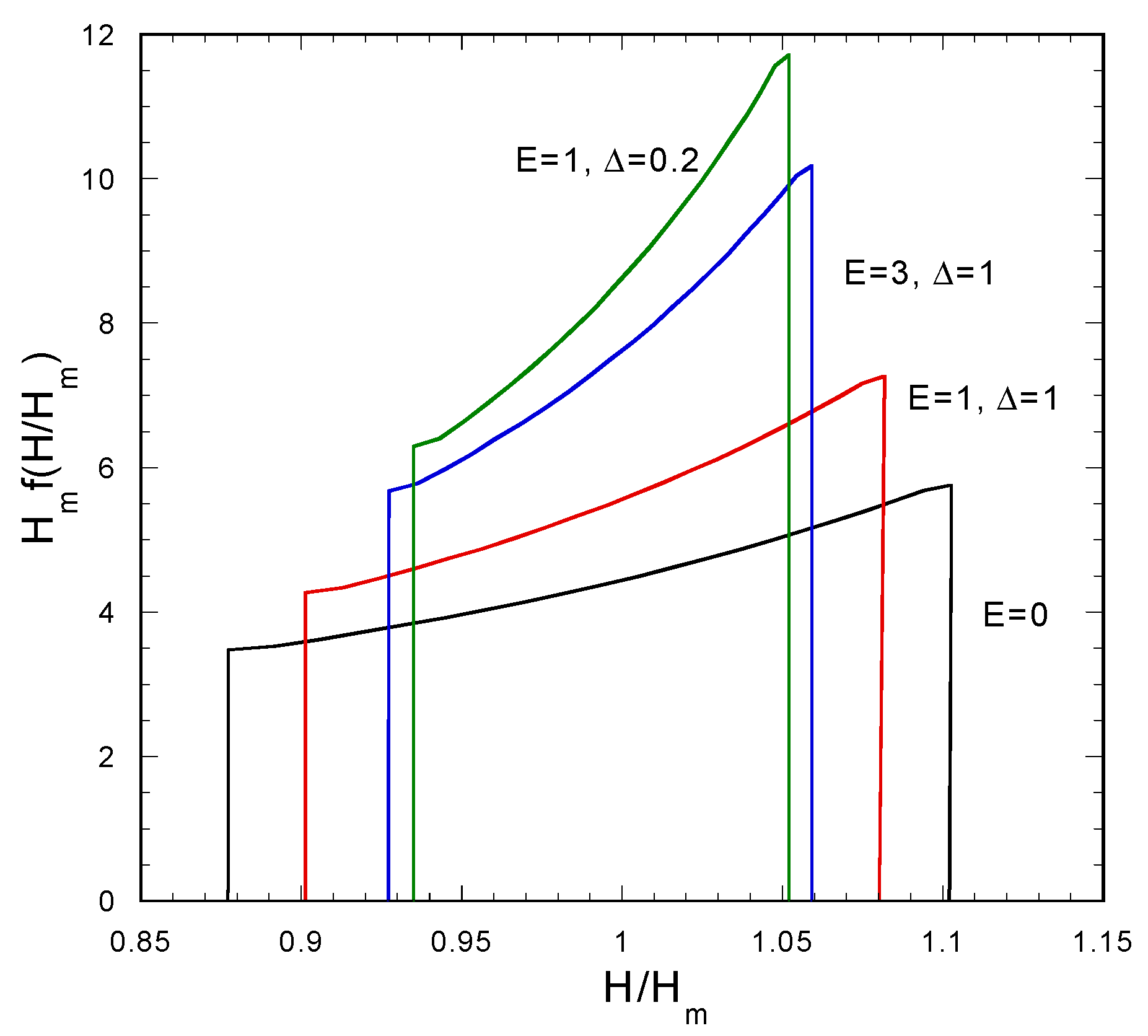
The distribution of permeability is the same as in the previous case (i.e., uniform between 80% and 120% of its average value). The probability density function of the variable H/Hm at the moment T = 1 for several combinations of the parameters E and Δ are presented in Figure 7. The case E = 0 corresponds to the absence of concentration polarization. The smoothing effect of concentration polarization on evolution of non-uniformity of layer thickness is evident. The explanation is that the thicker the film, the greater the “cake” enhanced concentration polarization and the smaller the wall flux leading to reduction of non-uniformity. As expected, the tendency of concentration polarization to promote uniformity of the film thickness tends to increase with increasing osmotic coefficient (E) and with decreasing diffusivity in the fouling layer (Δ). Thus, the scope of the present section is to indicate/demonstrate that the proposed simulation approach (in a generalized sense and not with particular details considered here) using PDF can be employed for any fouling layer growth model and not only for the simplest one considered in the preceding sections.

5. Discussion

A key issue here is how the spatial variation of the membrane permeability can be dealt with in the current multiscale framework of modeling membrane operation [13]. The smallest scale of the analysis is that of unit cell; i.e., a periodic unit of the spacer pattern [12]. This is the finest scale for which variation can be considered. If the spatial distribution of permeability is exactly known, a direct discretization is possible. However, this information is typically not available, and it can be replaced by the probability density function of the permeability. The length scale of membrane sheet cannot be treated with the techniques presented here, since there are topological contributions to the film evolution (due to variation of transmembrane pressure) in addition to the non-topological ones (due to non-uniform permeability). The permeability variation can be treated in a statistical way; i.e., values assigned randomly, from the prevailing permeability PDF, to domains of the membrane. This technique has been used to simulate the non-uniform spatial distribution of the hollow fibers in dealing with fluid dynamics in devices like hemodialyzers [27,28]. The question is, which will be the characteristic size of regions considered to be of uniform permeability? One might consider using the small unit cell scale (1 cm × 1 cm) but then the computational load to simulate the whole sheet would be unnecessarily high. The recommendation here is the use of a coarse grid to capture the topological variation of fouling layer thickness (and also the large-scale permeability variation) combined with a subgrid non-topological handling in terms of a PDF for each mesh region. This approach bears similarities to the large eddy simulation technique for turbulence flow analysis [29]. The subgrid analysis can be implemented with the techniques presented here; i.e., at each time step and at each discretization point the probability density function distribution of thickness has to be determined by considering several permeability values and their PDF. If, instead of its complete PDF, only the average values and standard deviation of thickness are of interest (i.e., similarly to the well-known methods of moments in population balance literature [30]), the number of considered permeability values can be minimized by their appropriate choice. For example, in the case of Gaussian permeability PDF, the normalized zeros of corresponding Hermite polynomials is the optimum choice [31]. The proposed approach allows the handling of high-resolution non-uniformities in membrane permeability and fouling layer thickness without a considerable increase of the computational load of the existing simulation approaches. This approach can be also extended to the modeling of other membrane processes (e.g., membrane distillation [32]) that exhibit the same non-uniformity problems. It is important to clarify that the present work refers to intrinsic non-uniformities of the membrane itself. Their computational treatment is important not only for the fouling process but also for the clean membrane operation. The organic fouling is deterministic since it is convection dominated. Not only it does not produce non-uniformities, but it also smears out the intrinsic membrane permeability non-uniformity as it is shown above. On the opposite, biofouling is a highly stochastic process that creates non-uniformities even for an otherwise uniform membrane. This type of fouling associated non-uniformities have been treated in detail in [33] regarding their effect of membrane permeate flux. Three models for the computation of this flux are assessed in [33] and it was found that the preferred one that combines accuracy and simplicity is the so-called 1-d model which is equivalent to the model used here (i.e., Equations (2) and (3)) for the flow through the fouled membrane.

6. Conclusions

This study deals with the problem of evolution of organic fouling layer spatial non-uniformity during operation of spiral wound desalination-membrane modules. A new approach to model fouling layer thickness evolution is proposed. Two types of non-uniformities are considered; i.e., topological (induced by trans-membrane pressure variation) and non-topological (induced by membrane permeability variation). The latter can be simulated through the use of probability density functions. Several results are presented indicating the tendency for self-regulation of the fouling layer (toward uniform spatial distribution) during its growth. A methodology is suggested for the dynamic simulation of fouling layer evolution at the membrane sheet scale, which is based on a coarse grid to account for topological thickness non-uniformity combined with a subgrid treatment of non-topological non-uniformities. The results of this work are considered a necessary building block in the systematic efforts aiming to develop reliable simulators of membrane-desalination processes; incorporation of these results in an existing general modeling framework is the next step.