Characterizing Quantum Effects in Optically Induced Nanowire Self-Oscillations: Coherent Properties
Abstract
1. Introduction
2. Methods
3. Results and Discussion
3.1. Solution for the Eigenvalue
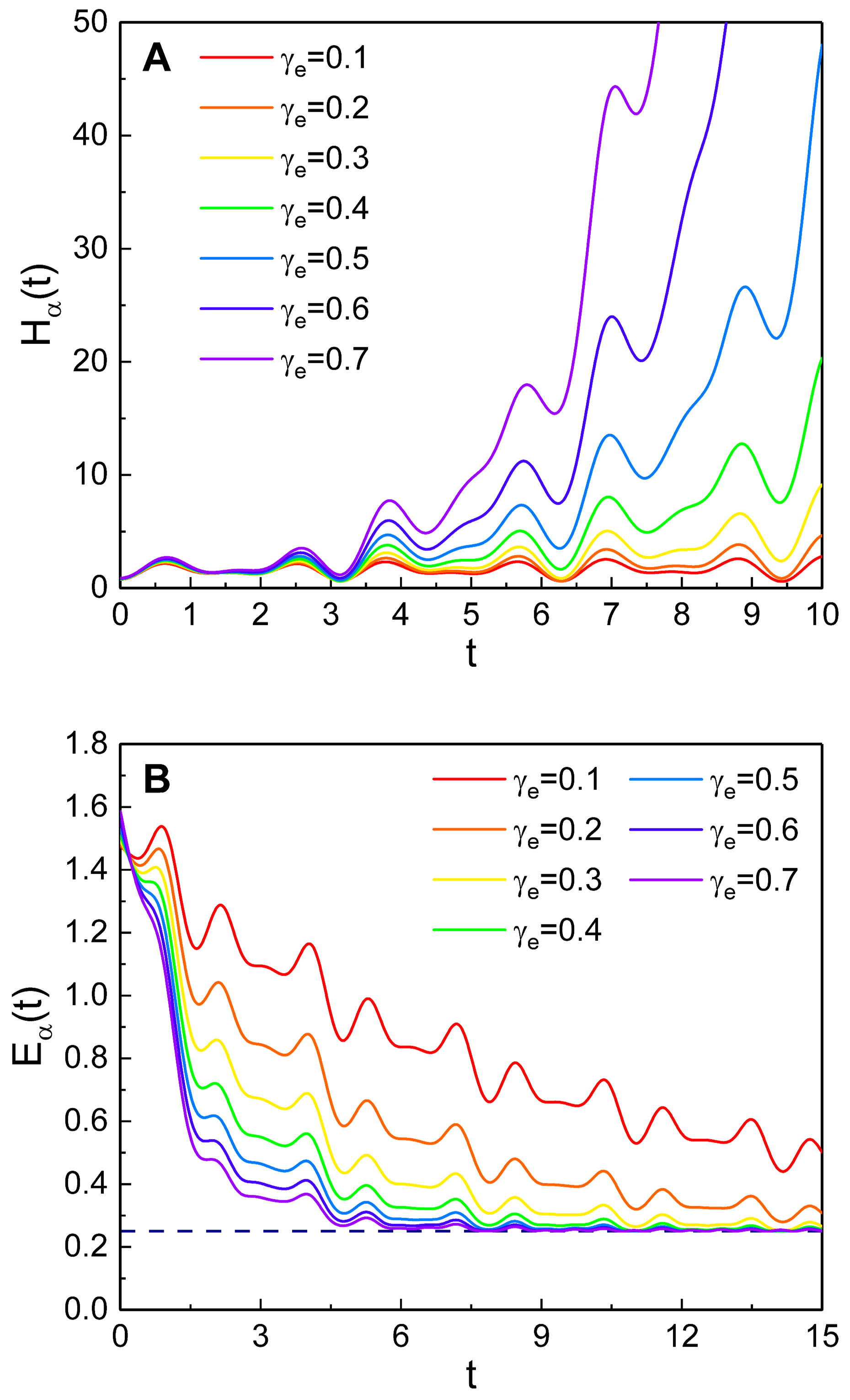
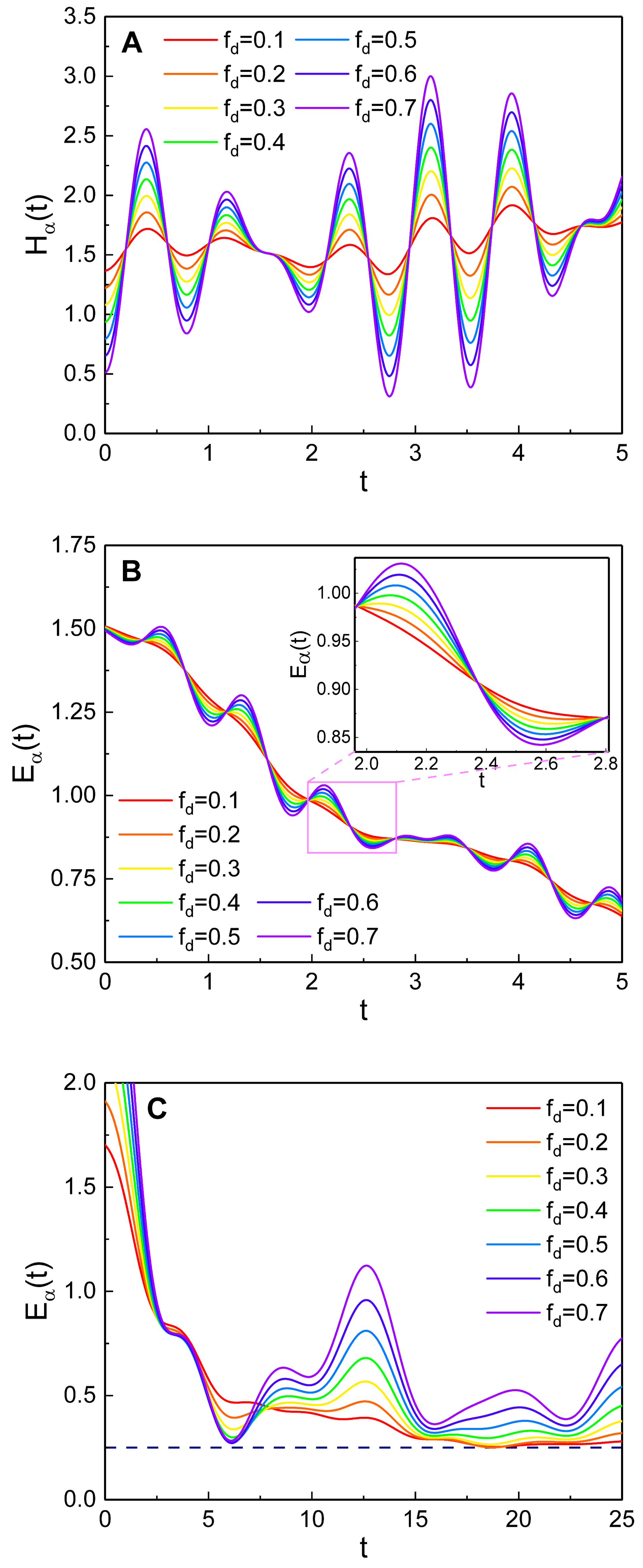
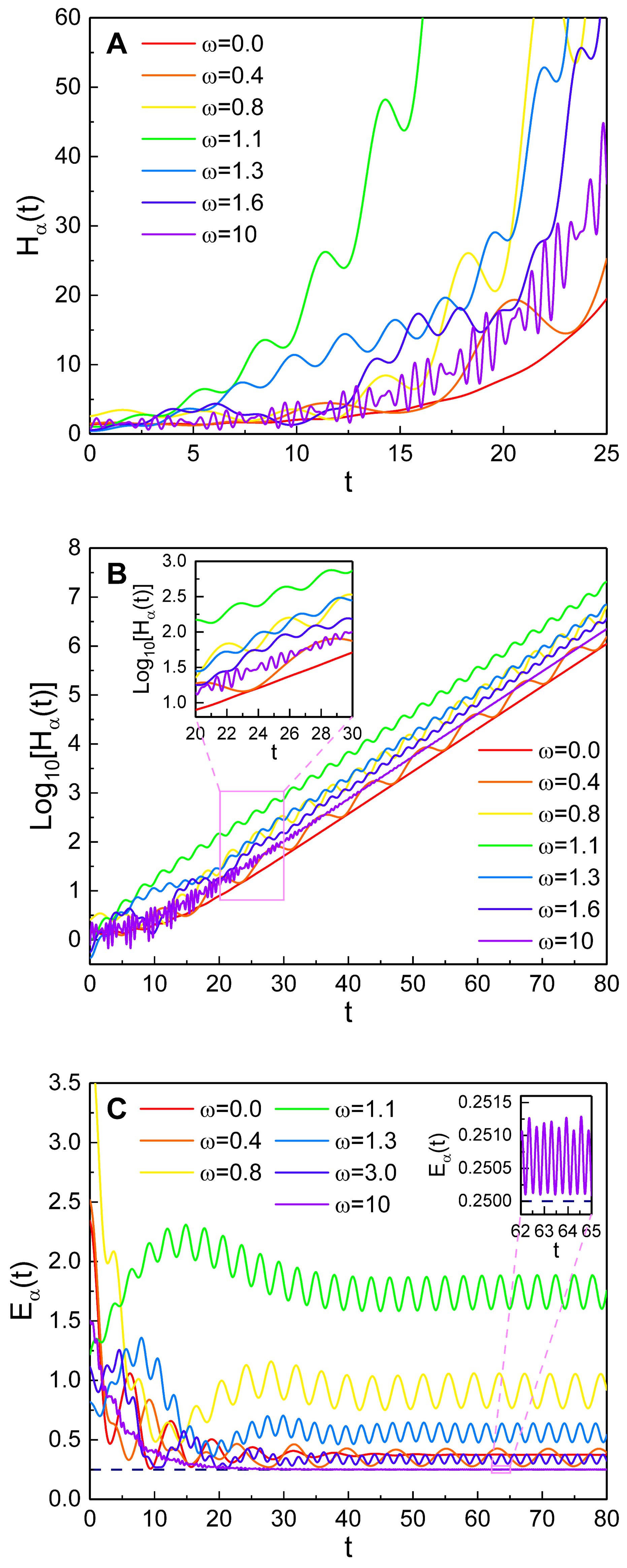
3.2. Fluctuations
3.3. Evolution of the Quantum Energy
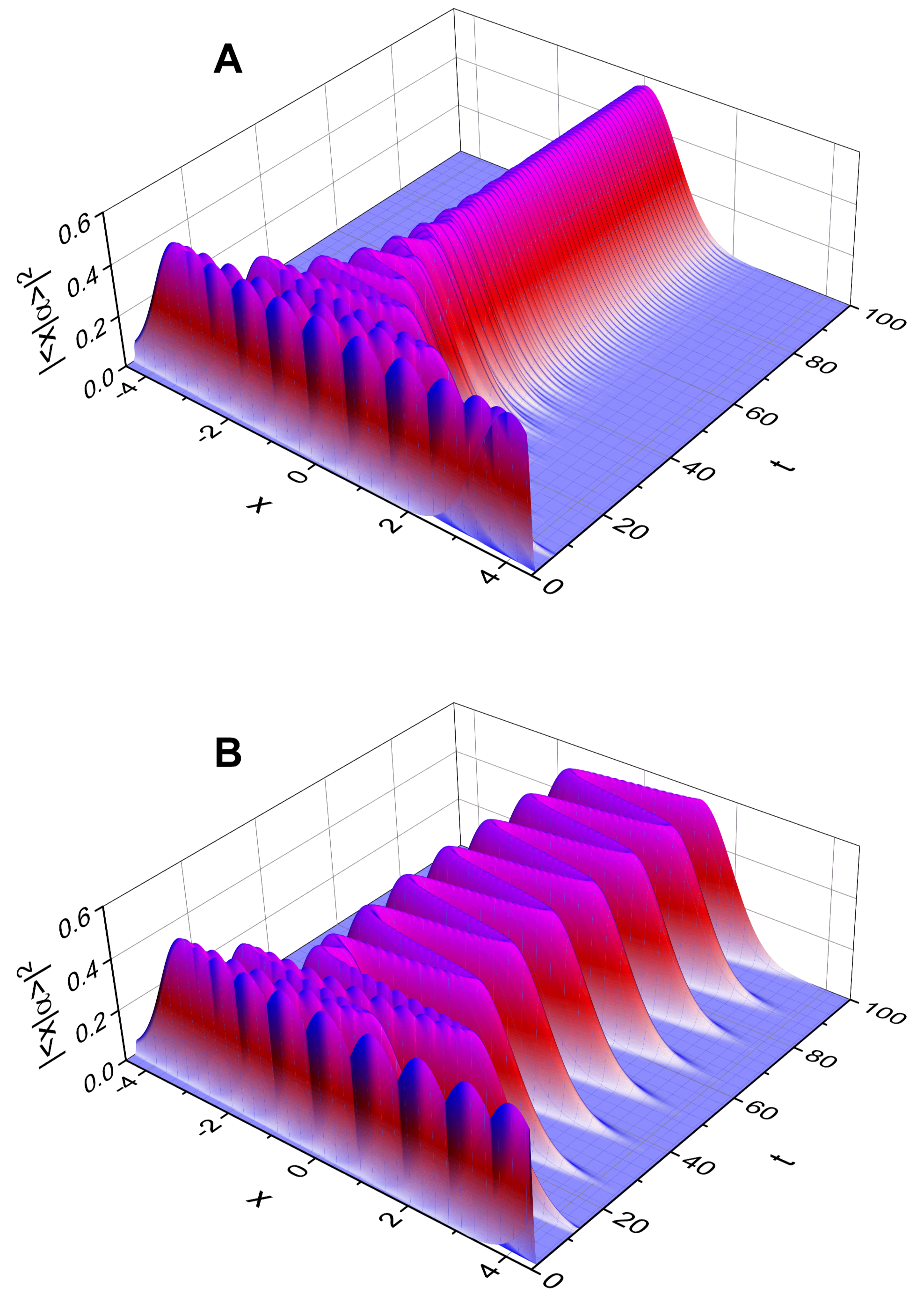
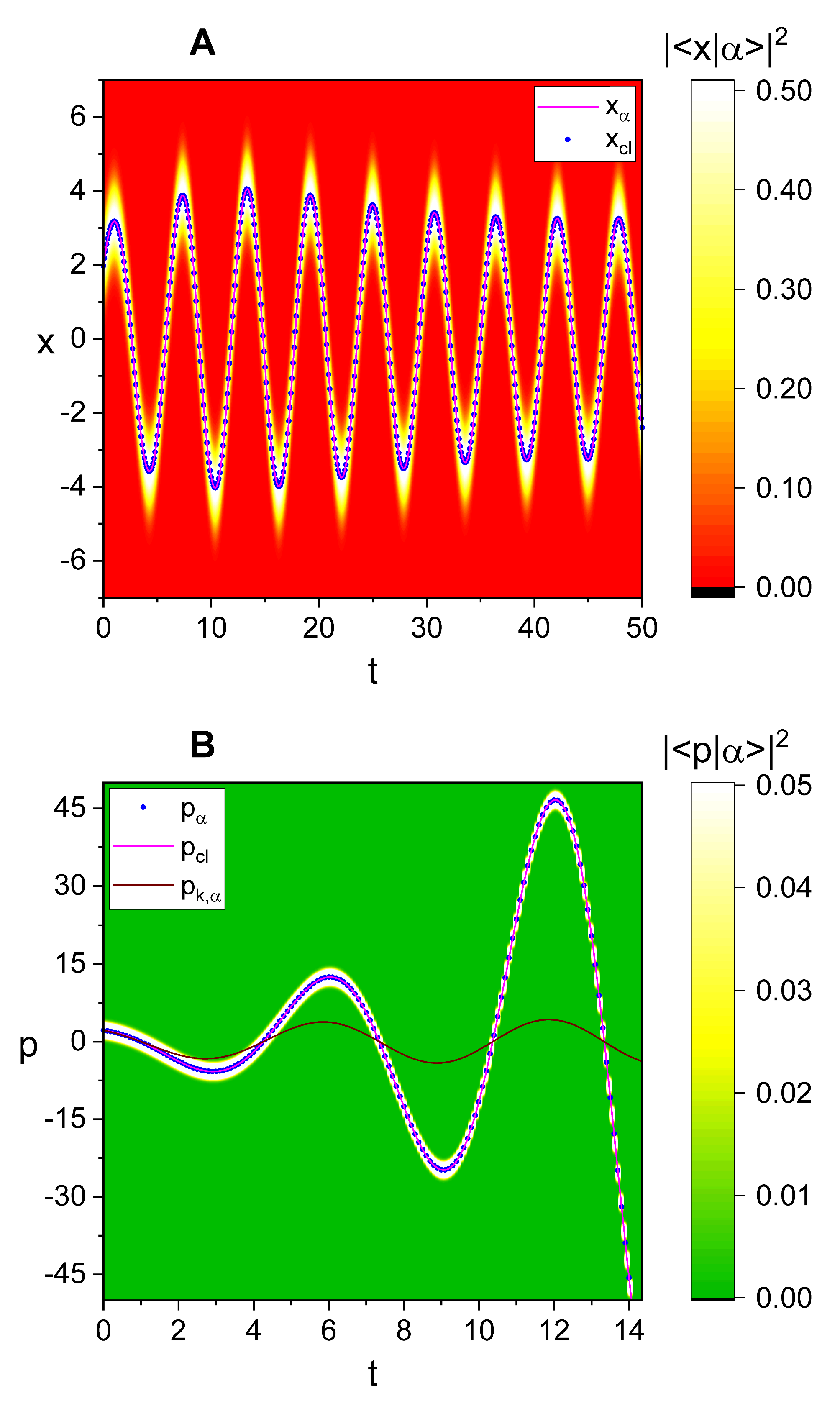
3.4. Analysis of the Eigenstate
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Classical Energy
References
- Roxworthy, B.J.; Aksyuk, V.A. Electrically tunable plasmomechanical oscillators for localized modulation, transduction, and amplification. Optica 2018, 5, 71–79. [Google Scholar] [CrossRef]
- Feng, X.L.; White, C.J.; Hajimiri, A.; Roukes, M.L. A self-sustaining ultrahigh-frequency nanoelectromechanical oscillator. Nat. Nanotechnol. 2008, 3, 342–346. [Google Scholar] [CrossRef] [PubMed]
- Arkan, E.F.; Sacchetto, D.; Yildiz, I.; Leblebici, Y.; Alaca, B.E. Monolithic integration of Si nanowires with metallic electrodes: NEMS resonator and switch applications. J. Micromech. Microeng. 2011, 21, 125018. [Google Scholar] [CrossRef]
- Gavartin, E.; Verlot, P.; Kippenberg, T.J. A hybrid on-chip optomechanical transducer for ultrasensitive force measurements. Nat. Nanotechnol. 2012, 7, 509–514. [Google Scholar] [CrossRef]
- Chaste, J.; Eichler, A.; Moser, J.; Ceballos, G.; Rurali, R.; Bachtold, A. A nanomechanical mass sensor with yoctogram resolution. Nat. Nanotechnol. 2012, 7, 301–304. [Google Scholar] [CrossRef] [PubMed]
- Steele, G.A.; Huttel, A.K.; Witkamp, B.; Poot, M.; Meerwaldt, H.B.; Kouwenhoven, L.P.; van der Zant, H.S.J. Strong coupling between single-electron tunneling and nanomechanical motion. Science 2009, 325, 1103–1107. [Google Scholar] [CrossRef]
- Lassagne, B.; Tarakanov, Y.; Kinaret, J.; Garcia-Sanchez, D.; Bachtold, A. Coupling mechanics to charge transport in carbon nanotube mechanical resonators. Science 2009, 325, 1107–1110. [Google Scholar] [CrossRef]
- Jensen, K.; Weldon, J.; Garcia, H.; Zettl, A. Nanotube radio. Nano Lett. 2007, 7, 3508–3511. [Google Scholar] [CrossRef]
- Gouttenoire, V.; Barois, T.; Perisanu, S.; Leclercq, J.-L.; Purcell, S.T. Vincent, P.; Ayari, A. Digital and FM demodulation of a doubly clamped single-walled carbon-nanotube oscillator: Towards a nanotube cell phone. Small 2010, 6, 1060–1065. [Google Scholar] [CrossRef]
- He, R.; Feng, X.L.; Roukes, M.L.; Yang, P. Self-transducing silicon nanowire electromechanical systems at room temperature. Nano Lett. 2008, 8, 1756–1761. [Google Scholar] [CrossRef]
- Choi, J.R.; Ju, S. Analyzing the geometric phase for self-oscillations in field emission nanowire mechanical resonators. Nonlinear Dyn. 2019, 97, 599–608. [Google Scholar] [CrossRef]
- Ayari, A.; Vincent, P.; Perisanu, S.; Choueib, M.; Gouttenoire, V.; Bechelany, M.; Cornu, D.; Purcell, S.T. Self-oscillations in field emission nanowire mechanical resonators: A nanometric dc-ac conversion. Nano Lett. 2007, 7, 2252–2257. [Google Scholar] [CrossRef] [PubMed]
- Alba, R.D.; Abhilash, T.S.; Rand, R.H.; Craighead, H.G.; Parpia, J.M. Low-power photothermal self-oscillation of bimetallic nanowires. Nano Lett. 2017, 17, 3995–4002. [Google Scholar] [CrossRef]
- Chen, C.; Zanette, D.H.; Guest, J.R.; Czaplewski, D.A.; López, D. Self-sustained micromechanical oscillator with linear feedback. Phys. Rev. Lett. 2016, 117, 017203. [Google Scholar] [CrossRef] [PubMed]
- Colinet, E.; Duraffourg, L.; Labarthe, S.; Hentz, S.; Robert, P.; Andreucci, P. Self-oscillation conditions of a resonant nanoelectromechanical mass sensor. J. Appl. Phys. 2009, 105, 124908. [Google Scholar] [CrossRef]
- Lazarus, A.; Barois, T.; Perisanu, S.; Poncharal, P.; Manneville, P.; de Langre, E.; Purcell, S.T.; Vincent, P.; Ayari, A. Simple modeling of self-oscillations in nanoelectromechanical systems. Appl. Phys. Lett. 2010, 96, 193114. [Google Scholar] [CrossRef]
- Barois, T.; Perisanu, S.; Vincent, P.; Purcell, S.T.; Ayari, A. Frequency modulated self-oscillation and phase inertia in a synchronized nanowire mechanical resonator. New J. Phys. 2014, 16, 083009. [Google Scholar] [CrossRef]
- Vincent, P.; Perisanu, S.; Ayari, A.; Choueib, M.; Gouttenoire, V.; Bechelany, M.; Brioude, A.; Cornu, D.; Purcell, S.T. Driving self-sustained vibrations of nanowires with a constant electron beam. Phys. Rev. B 2007, 76, 085435. [Google Scholar] [CrossRef]
- Barois, T.; Perisanu, S.; Vincent, P.; Purcell, S.T.; Ayari, A. Role of fluctuations and nonlinearities on field emission nanomechanical self-oscillators. Phys. Rev. B 2013, 88, 195428. [Google Scholar] [CrossRef]
- Barois, T.; Ayari, A.; Vincent, P.; Perisanu, S.; Poncharal, P.; Purcell, S.T. Ultra low power consumption for self-oscillating nanoelectromechanical systems constructed by contacting two nanowires. Nano Lett. 2013, 13, 1451–1456. [Google Scholar] [CrossRef][Green Version]
- Yu, H.; Zhang, W.W.; Lei, S.Y.; Lu, L.B.; Sun, C.; Huang, Q.A. Study on vibration behavior of doubly clamped silicon nanowires by molecular dynamics. J. Nanomater. 2012, 2012, 342329. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and incoherent states of the radiation field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Buot, F.A. Mesoscopic physics and nanoelectronics: Nanoscience and nanotechnology. Phys. Rep. 1993, 234, 73–174. [Google Scholar] [CrossRef]
- Berezin, F.A. General concept of quantization. Commun. Math. Phys. 1975, 40, 153–174. [Google Scholar] [CrossRef]
- Greenberger, D.M. A critique of the major approaches to damping in quantum theory. J. Math. Phys. 1979, 20, 762–770. [Google Scholar] [CrossRef]
- Yeon, K.-H.; Kim, S.-S.; Moon, Y.-M.; Hong, S.-K.; Um, C.-I.; George, T.F. The quantum under-, critical- and over-damped driven harmonic oscillators. J. Phys. A Math. Gen. 2001, 34, 7719–7732. [Google Scholar] [CrossRef]
- Dybiec, B.; Gudowska-Nowak, E. Underdamped stochastic harmonic oscillator driven by Le´vy noise. Phys. Rev. E 2017, 96, 042118. [Google Scholar] [CrossRef]
- Wang, H.; Dhayalan, Y.; Buks, E. Devil’s staircase in an optomechanical cavity. Phys. Rev. E 2016, 93, 023007. [Google Scholar] [CrossRef]
- Blocher, D.B.; Zehnder, A.T.; Rand, R.H.J. Entrainment of micromechanical limit cycle oscillators in the presence of frequency instability. Microelectromech. Syst. 2013, 22, 835–845. [Google Scholar] [CrossRef][Green Version]
- Choi, J.R. Analysis of quantum energy for Caldirola-Kanai Hamiltonian systems in coherent states. Results Phys. 2013, 3, 115–121. [Google Scholar] [CrossRef]
- Louisell, W.H. Quantum Statistical Properties of Radiation; John Wiley & Sons: New York, NY, USA, 1973; p. 109. [Google Scholar]
- Miller, J.M.L.; Ansari, A.; Heinz, D.B.; Chen, Y.; Flader, I.B.; Shin, D.D.; Villanueva, L.G.; Kenny, T.W. Effective quality factor tuning mechanisms in micromechanical resonators. Appl. Phys. Rev. 2018, 5, 041307. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Choi, J.R. Characterizing Quantum Effects in Optically Induced Nanowire Self-Oscillations: Coherent Properties. Photonics 2021, 8, 237. https://doi.org/10.3390/photonics8070237
Choi JR. Characterizing Quantum Effects in Optically Induced Nanowire Self-Oscillations: Coherent Properties. Photonics. 2021; 8(7):237. https://doi.org/10.3390/photonics8070237
Chicago/Turabian StyleChoi, Jeong Ryeol. 2021. "Characterizing Quantum Effects in Optically Induced Nanowire Self-Oscillations: Coherent Properties" Photonics 8, no. 7: 237. https://doi.org/10.3390/photonics8070237
APA StyleChoi, J. R. (2021). Characterizing Quantum Effects in Optically Induced Nanowire Self-Oscillations: Coherent Properties. Photonics, 8(7), 237. https://doi.org/10.3390/photonics8070237



