Generation and Manipulation of Airy Breathing Solitons in an Inhomogeneous Medium with Periodic Potential
Abstract
1. Introduction
2. Theoretical Model
3. Analysis of Numerical Calculation Results
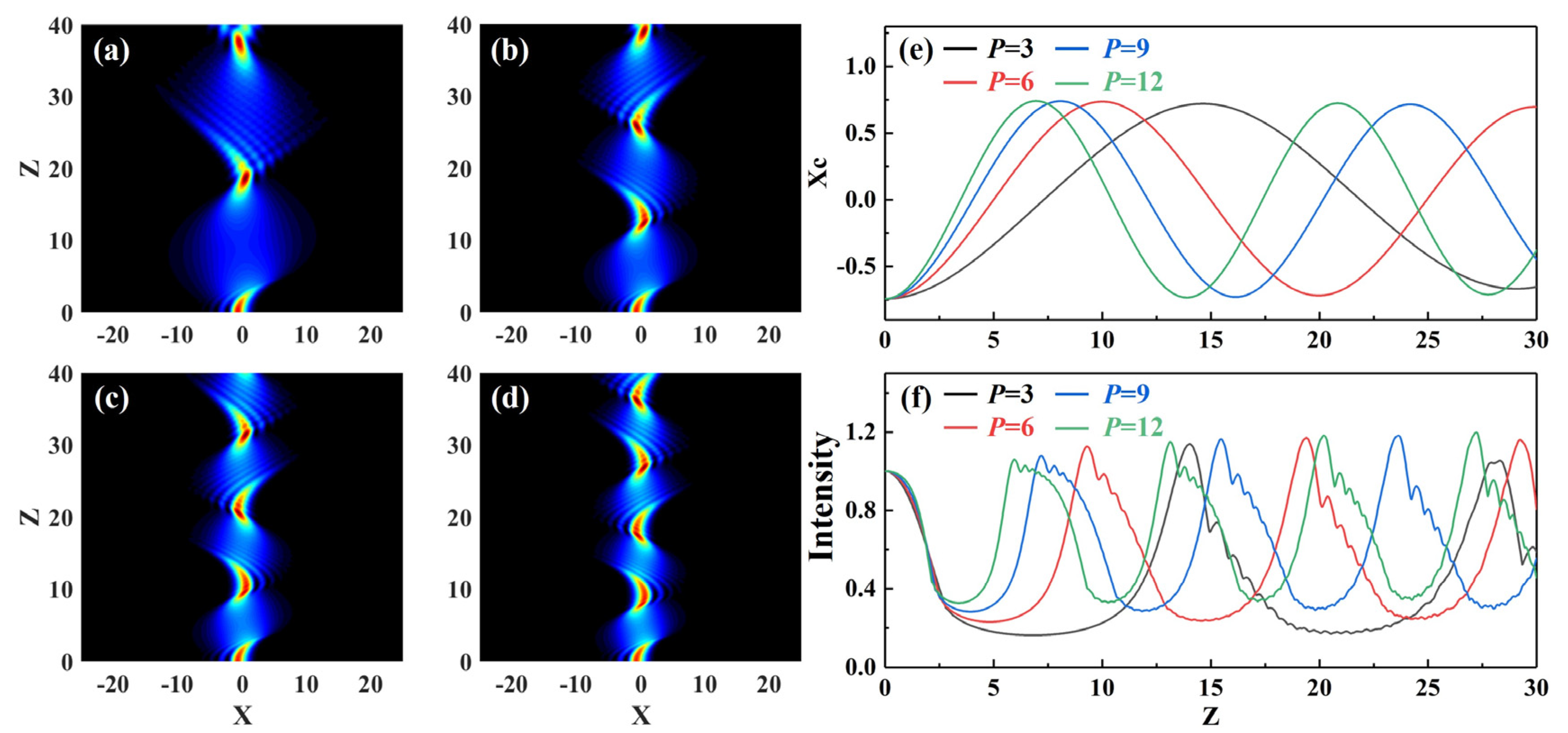
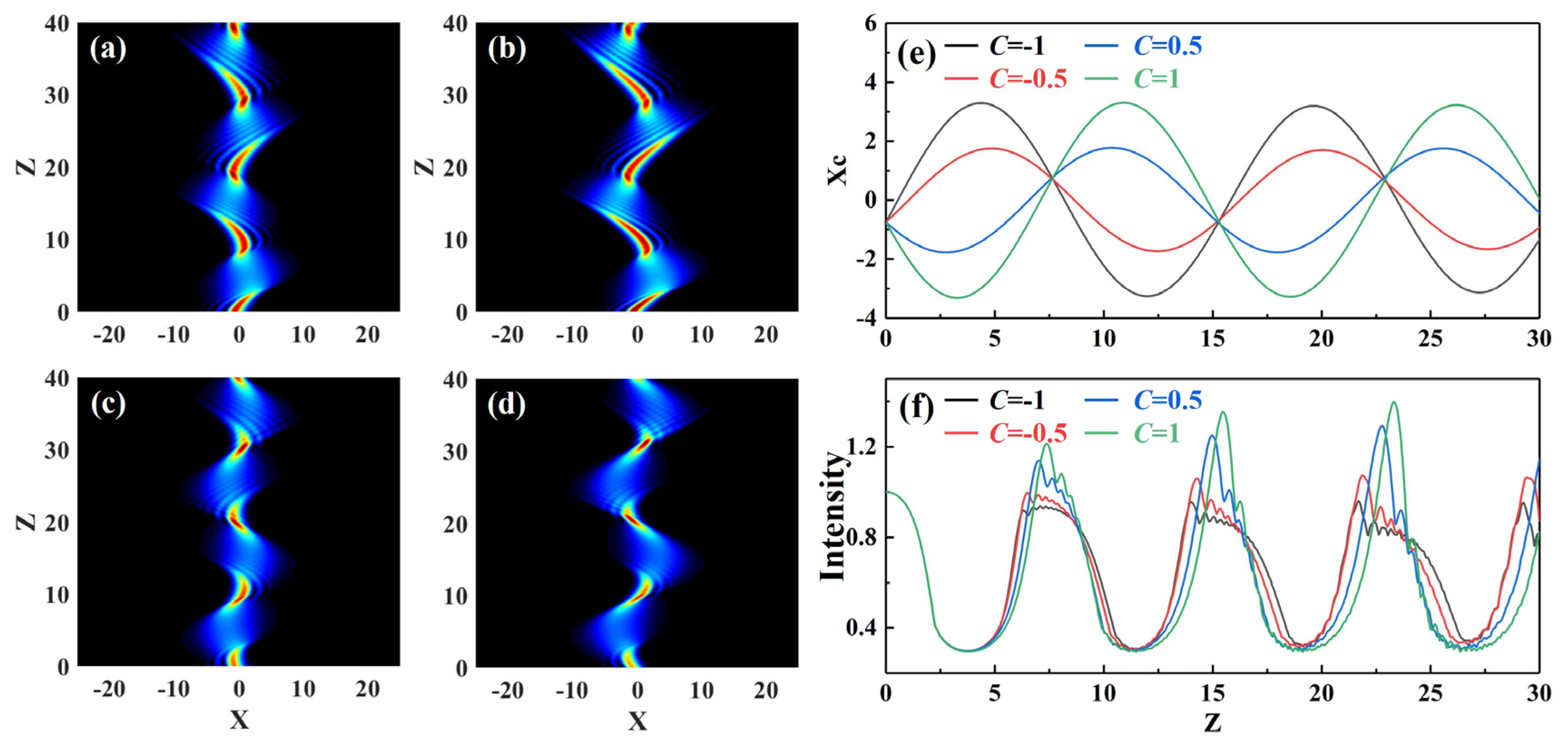
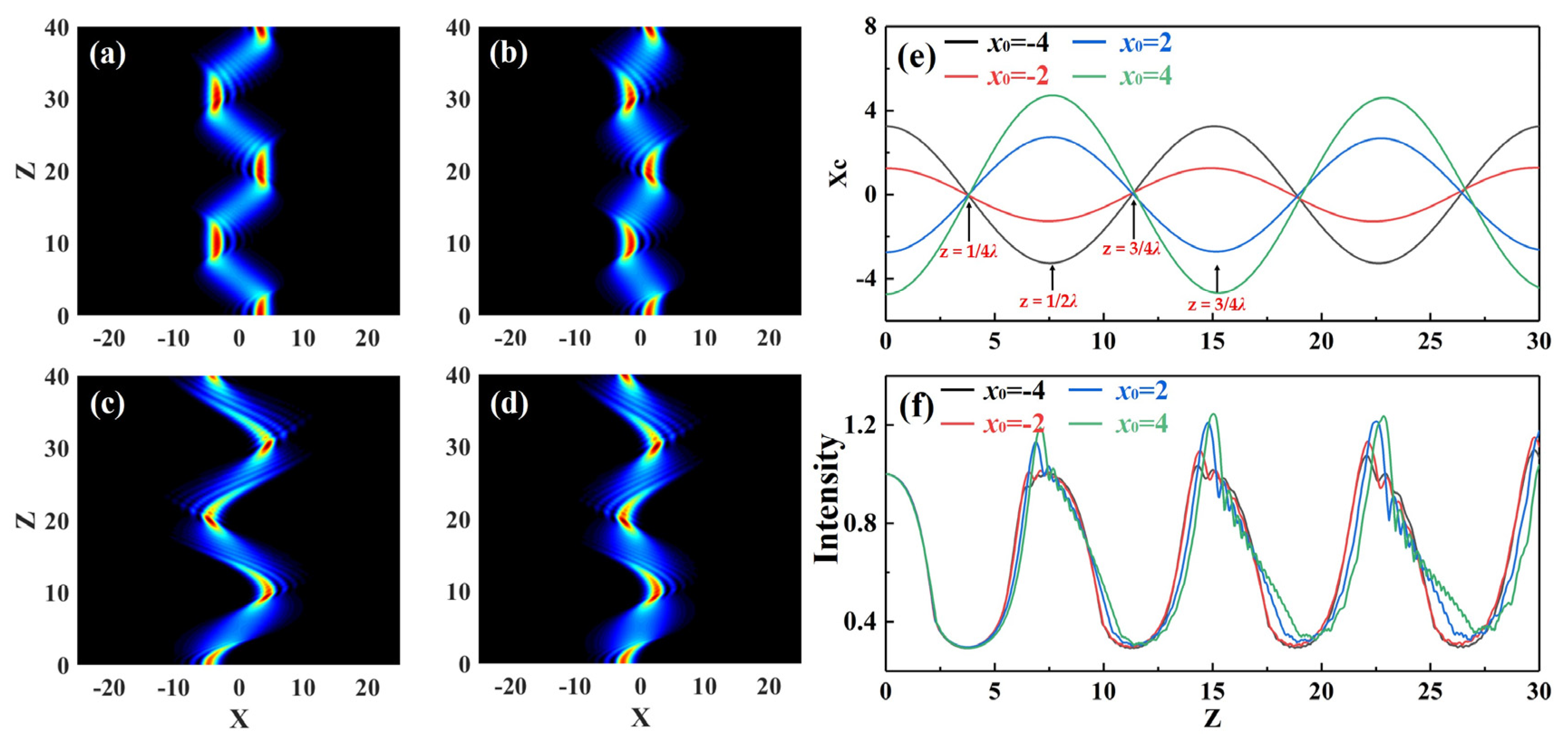
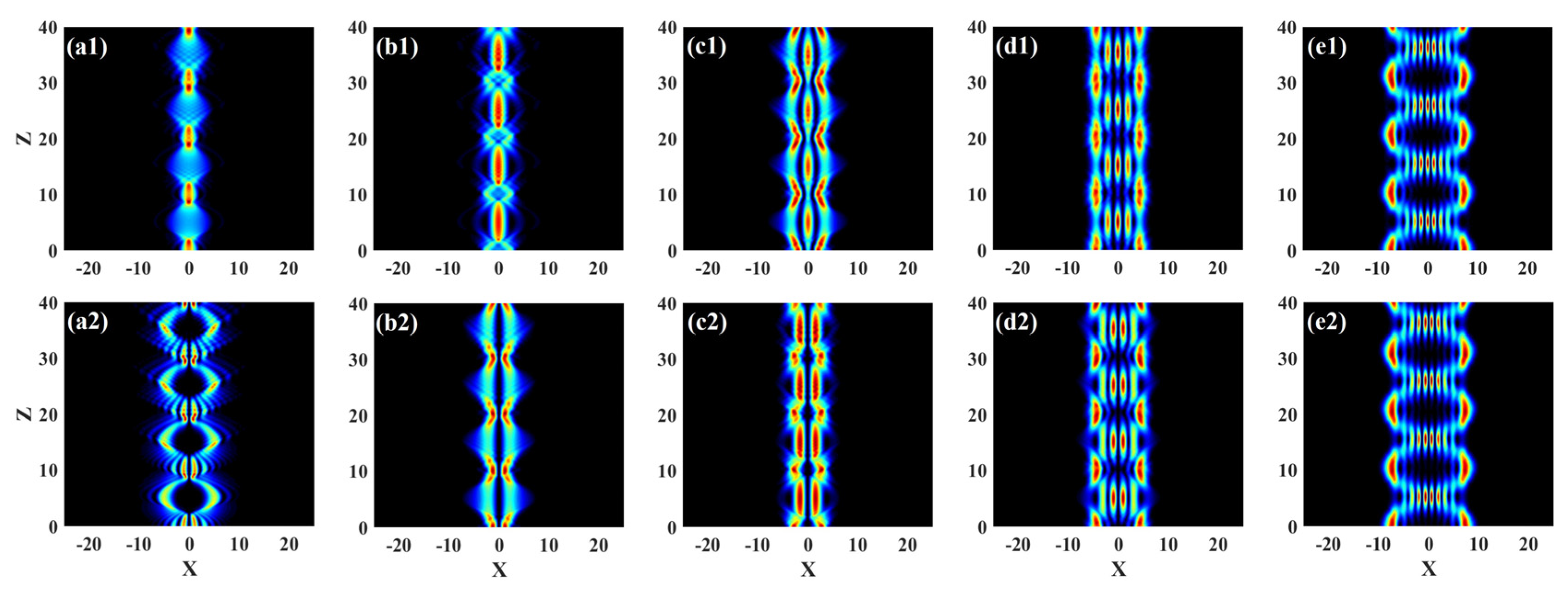
3.1. Propagation Characteristics of a Single Airy Beam in an Inhomogeneous Medium with Periodic Potential
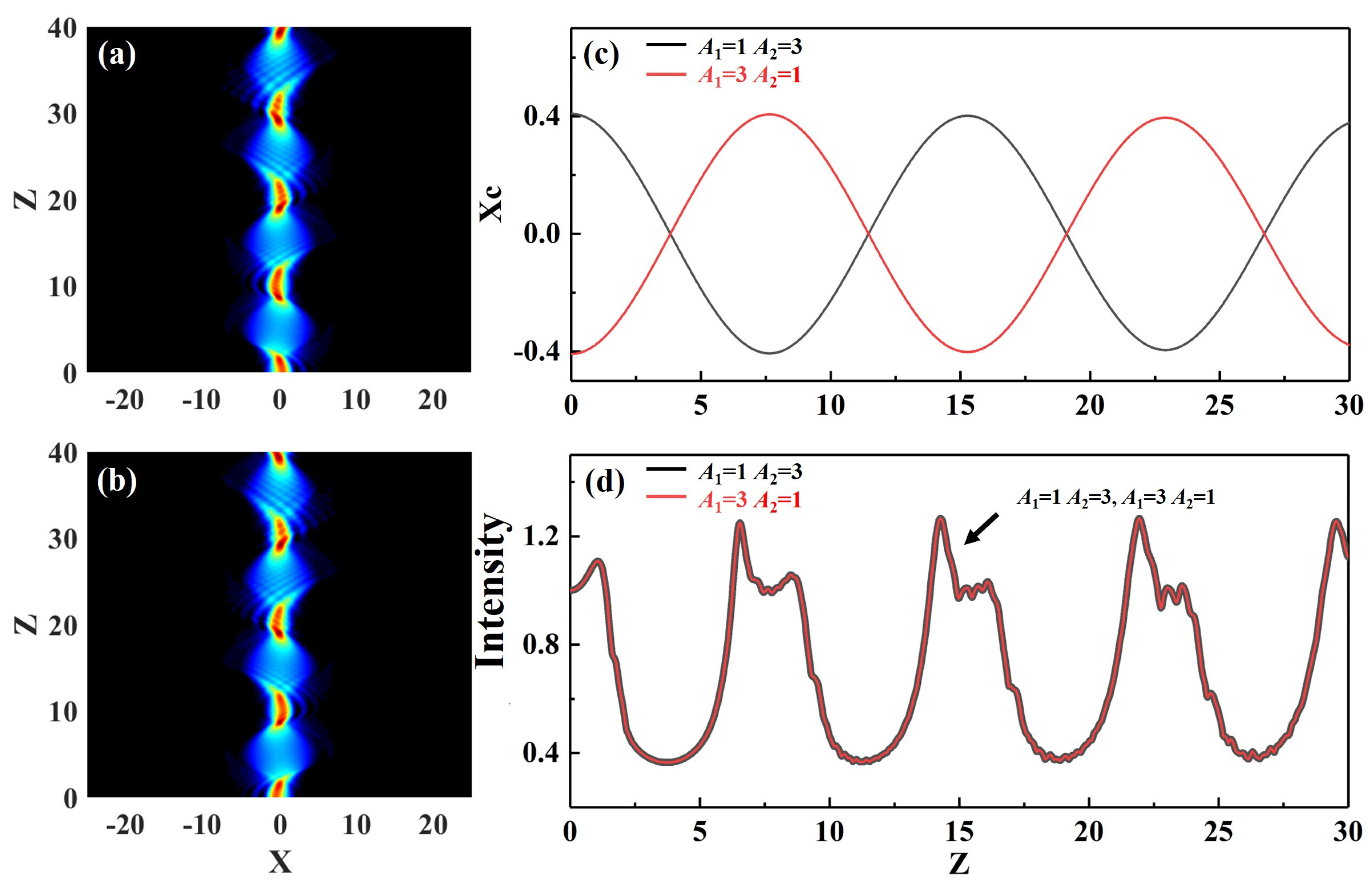
3.2. Propagation Characteristics of Two Airy Beams with Opposite Spatial Positions in an Inhomogeneous Medium with Periodic Potential
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Berry, M.V.; Balazs, N.L. Nonspreading wave packets. Am. J. Phys. 1979, 47, 264–267. [Google Scholar] [CrossRef]
- Broky, J.; Siviloglou, G.A.; Dogariu, A.; Christodoulides, D.N. Self-healing properties of optical airy beams. Opt. Express 2008, 16, 12880–12891. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Zhang, P.; Lou, C.; Huang, S.; Xu, J.; Chen, Z. Optimal control of the ballistic motion of airy beams. Opt. Lett. 2010, 35, 2260–2262. [Google Scholar] [CrossRef]
- Gu, Y.; Gbur, G. Scintillation of airy beam arrays in atmospheric turbulence. Opt. Lett. 2010, 35, 3456–3458. [Google Scholar] [CrossRef]
- Chu, X. Evolution of an airy beam in turbulence. Opt. Lett. 2011, 36, 2701–2703. [Google Scholar] [CrossRef] [PubMed]
- Peng, X.; Peng, Y.; Li, D.; Zhang, L.; Zhuang, J.; Zhao, F.; Chen, X.; Yang, X.; Deng, D. Propagation properties of spatiotemporal chirped Airy Gaussian vortex wave packets in a quadratic index medium. Opt. Express 2017, 25, 13527–13538. [Google Scholar] [CrossRef]
- Chen, C.; Peng, X.; Chen, B.; Peng, Y.; Zhou, M.; Yang, X.; Deng, D. Propagation of an Airy–Gaussian vortex beam in linear and nonlinear media. J. Opt. 2016, 18, 055505. [Google Scholar] [CrossRef]
- Deng, F.; Deng, D.M. Nonparaxial propagation of an Airy-Gaussian beam in uniaxial crystal orthogonal to the optical axis. Opt. Commun. 2016, 380, 280–286. [Google Scholar] [CrossRef]
- Zhu, W.; Guan, J.; Deng, F.; Deng, D.; Huang, J. The propagation properties of the first-order and the second-order Airy vortex beams through strongly nonlocal nonlinear medium. Opt. Commun. 2016, 380, 434–441. [Google Scholar] [CrossRef]
- Zhang, J.; Zhou, K.; Liang, J.; Lai, Z.; Yang, X.; Deng, D. Nonparaxial propagation of the chirped Airy vortex beams in uniaxial crystal orthogonal to the optical axis. Opt. Express 2018, 26, 1290–1304. [Google Scholar] [CrossRef]
- Peng, Y.; Peng, X.; Chen, B.; Zhou, M.; Chen, C.; Deng, D. Interaction of Airy–Gaussian beams in Kerr media. Opt. Commun. 2016, 359, 116–122. [Google Scholar] [CrossRef]
- Li, J.X.; Fan, X.L.; Zang, W.P.; Tian, J.G. Vacuum electron acceleration driven by two crossed airy beams. Opt. Lett. 2011, 36, 648–650. [Google Scholar] [CrossRef] [PubMed]
- Chong, A.; Renninger, W.H.; Christodoulides, D.N.; Wise, F.W. Airy-bessel wave packets as versatile linear light bullets. Nat. Photonics 2010, 4, 103–106. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.; Papazoglou, D.G.; Couairon, A.; Tzortzakis, S. Sharply autofocused ring-airy beams transforming into non-linear intense light bullets. Nat. Commun. 2013, 4, 2622. [Google Scholar] [CrossRef]
- Zhang, P.; Prakash, J.; Zhang, Z.; Mills, M.S.; Efremidis, N.K.; Christodoulides, D.N.; Chen, Z. Trapping and guiding microparticles with morphing autofocusing airy beams. Opt. Lett. 2011, 36, 2883–2885. [Google Scholar] [CrossRef]
- Baumgartl, J.; Mazilu, M.; Dholakia, K. Optically mediated partical clearing using airy wavepackets. Nat. Photonics 2008, 2, 675–678. [Google Scholar] [CrossRef]
- Zheng, Z.; Zhang, B.F.; Chen, H.; Ding, J.; Wang, H.T. Optical trapping with focused airy beams. Appl. Opt. 2011, 50, 43–49. [Google Scholar] [CrossRef]
- Rose, P.; Diebel, F.; Boguslawski, M.; Denz, C. Airy beam induced optical routing. Appl. Phys. Lett. 2013, 102, 101101. [Google Scholar] [CrossRef]
- Liu, C.F.; Zhu, A.J. Matter-wave exact periodic solutions in optical lattices with periodic potential. AIP Conf. Proc. 2013, 1558, 1947–1950. [Google Scholar]
- Trombettoni, A.; Smerzi, A. Discrete solitons and breathers with dilute Bose-Einstein condensates. Phys. Rev. Lett. 2001, 86, 2353–2356. [Google Scholar] [CrossRef]
- Chabchoub, A.; Hoffmann, N.P.; Akhmediev, N. Rogue wave observation in a water wave tank. Phys. Rev. Lett. 2011, 106, 204502. [Google Scholar] [CrossRef] [PubMed]
- Dudley, J.M.; Dias, F.; Erkintalo, M.; Genty, G. Instabilities breathers and rogue waves in optics. Nat. Photonics 2014, 8, 755–764. [Google Scholar] [CrossRef]
- Qin, C.; Yuan, L.; Wang, B.; Fan, S.; Lu, P. Effective electric-field for a photon in a synthetic frequency lattice created in a waveguide modulator. Phys. Rev. A 2018, 97, 063838. [Google Scholar] [CrossRef]
- Li, W.; Qin, C.; Han, T.; Chen, H.; Wang, B.; Lu, P. Bloch oscillations in photonic spectral lattices through phase-mismatched four-wave mixing. Opt. Lett. 2019, 44, 5430–5433. [Google Scholar] [CrossRef]
- Chen, H.; Yang, N.; Qin, C.; Li, W.; Wang, B.; Han, T.; Zhang, C.; Liu, W.; Wang, K.; Long, H.; et al. Real-time observation of frequency Bloch oscillations with fiber loop modulation. Light Sci. Appl. 2021, 10, 48. [Google Scholar] [CrossRef]
- Block, A.; Etrich, C.; Limboeck, T.; Bleckmann, F.; Soergel, E.; Rockstuhl, C.; Linden, S. Bloch oscillations in plasmonic waveguide arrays. Nat. Commun. 2014, 5, 3843. [Google Scholar] [CrossRef]
- Kratzer, P.; Neugebauer, J. The basics of electronic structure theory for periodic systems. Front. Chem. 2019, 7, 106. [Google Scholar] [CrossRef]
- Dong, S.H.; Dong, S.; Dong, Q.; Sun, G.H.; Femmam, S. Exact Solutions of the Razavy Cosine Type Potential. Adv. High Energy Phys. 2018, 2018, 5824271. [Google Scholar] [CrossRef]
- Huang, X.W.; Deng, Z.X.; Fu, X.Q. Dynamics of finite energy Airy beams modeled by the fractional Schrödinger equation with a linear potential. J. Opt. Soc. Am. B 2017, 35, 976–982. [Google Scholar] [CrossRef]
- Zhang, L.; Li, H.; Liu, Z.; Zhang, J.; Cai, W.; Gao, Y.; Fan, D. Controlling cosine-Gaussian beams in linear media with quadratic external potential. Opt. Express 2021, 29, 5128–5140. [Google Scholar] [CrossRef]
- Wen, B.; Deng, Y.; Wei, J.; Chen, D.; Leng, X. Evolution of Cos–Gaussian Beams in the Periodic Potential Optical Lattice. Crystals 2022, 12, 1097. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, C.; Wen, B.; Deng, Y.; Fan, Y.; Wei, J.; Chen, D. Generation and Manipulation of Airy Breathing Solitons in an Inhomogeneous Medium with Periodic Potential. Photonics 2023, 10, 486. https://doi.org/10.3390/photonics10050486
Gao C, Wen B, Deng Y, Fan Y, Wei J, Chen D. Generation and Manipulation of Airy Breathing Solitons in an Inhomogeneous Medium with Periodic Potential. Photonics. 2023; 10(5):486. https://doi.org/10.3390/photonics10050486
Chicago/Turabian StyleGao, Chunhui, Bing Wen, Yangbao Deng, Yingqi Fan, Jiamou Wei, and Depeng Chen. 2023. "Generation and Manipulation of Airy Breathing Solitons in an Inhomogeneous Medium with Periodic Potential" Photonics 10, no. 5: 486. https://doi.org/10.3390/photonics10050486
APA StyleGao, C., Wen, B., Deng, Y., Fan, Y., Wei, J., & Chen, D. (2023). Generation and Manipulation of Airy Breathing Solitons in an Inhomogeneous Medium with Periodic Potential. Photonics, 10(5), 486. https://doi.org/10.3390/photonics10050486




