Temperature-Insensitive Ferrofluid-Clad Microfiber Bragg Grating for Magnetic Field Sensing
Abstract
1. Introduction
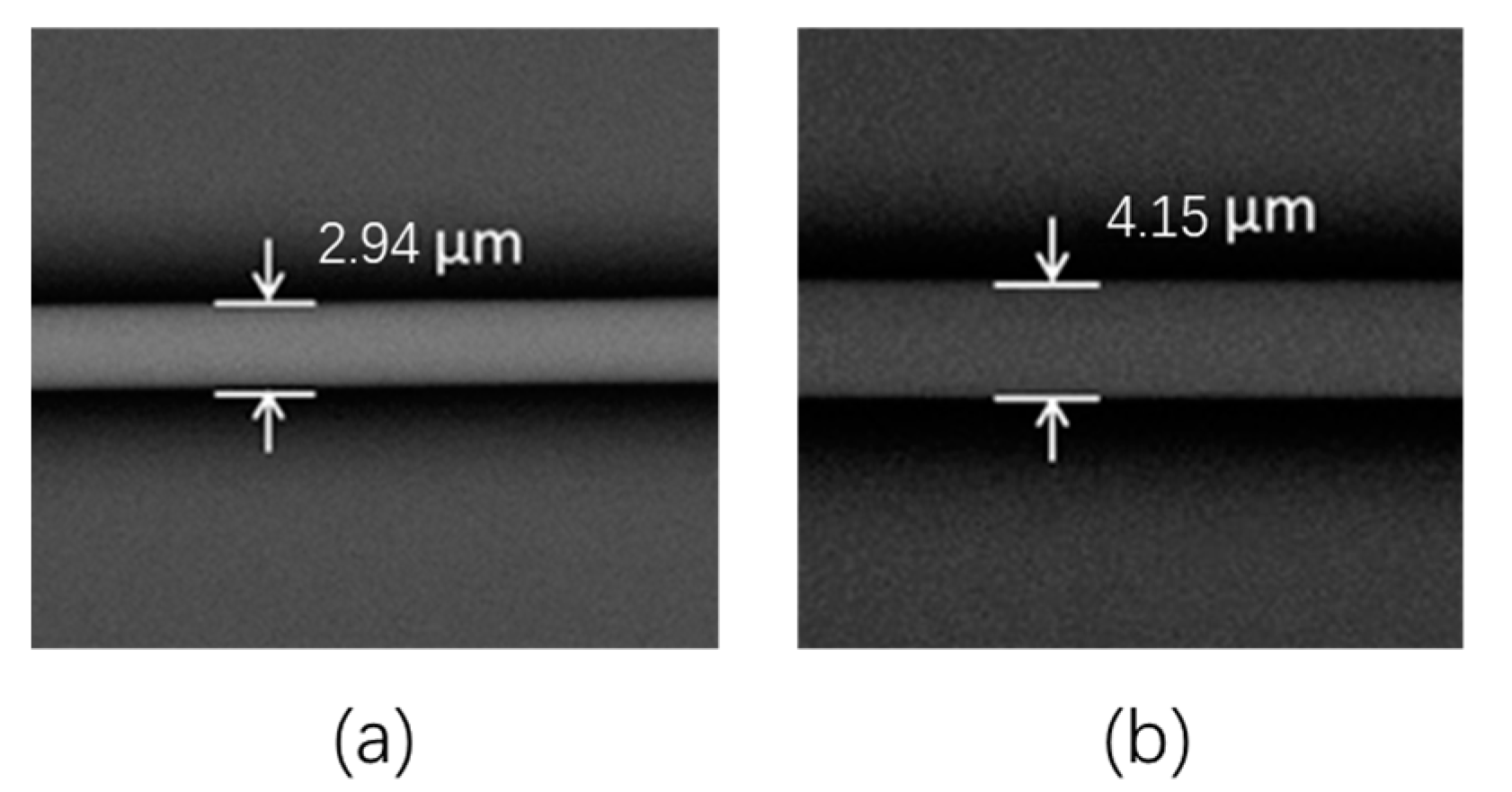
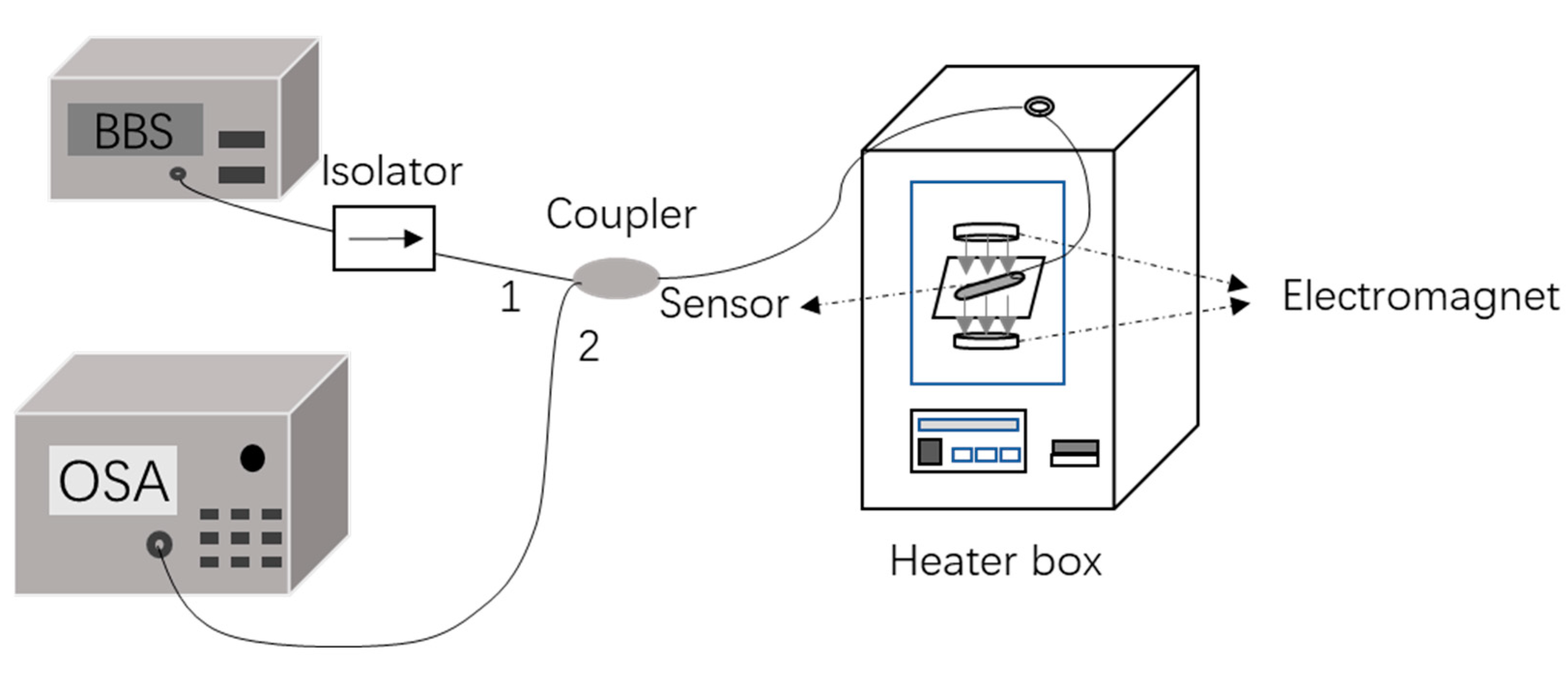
2. Device Fabrication and Working Principle
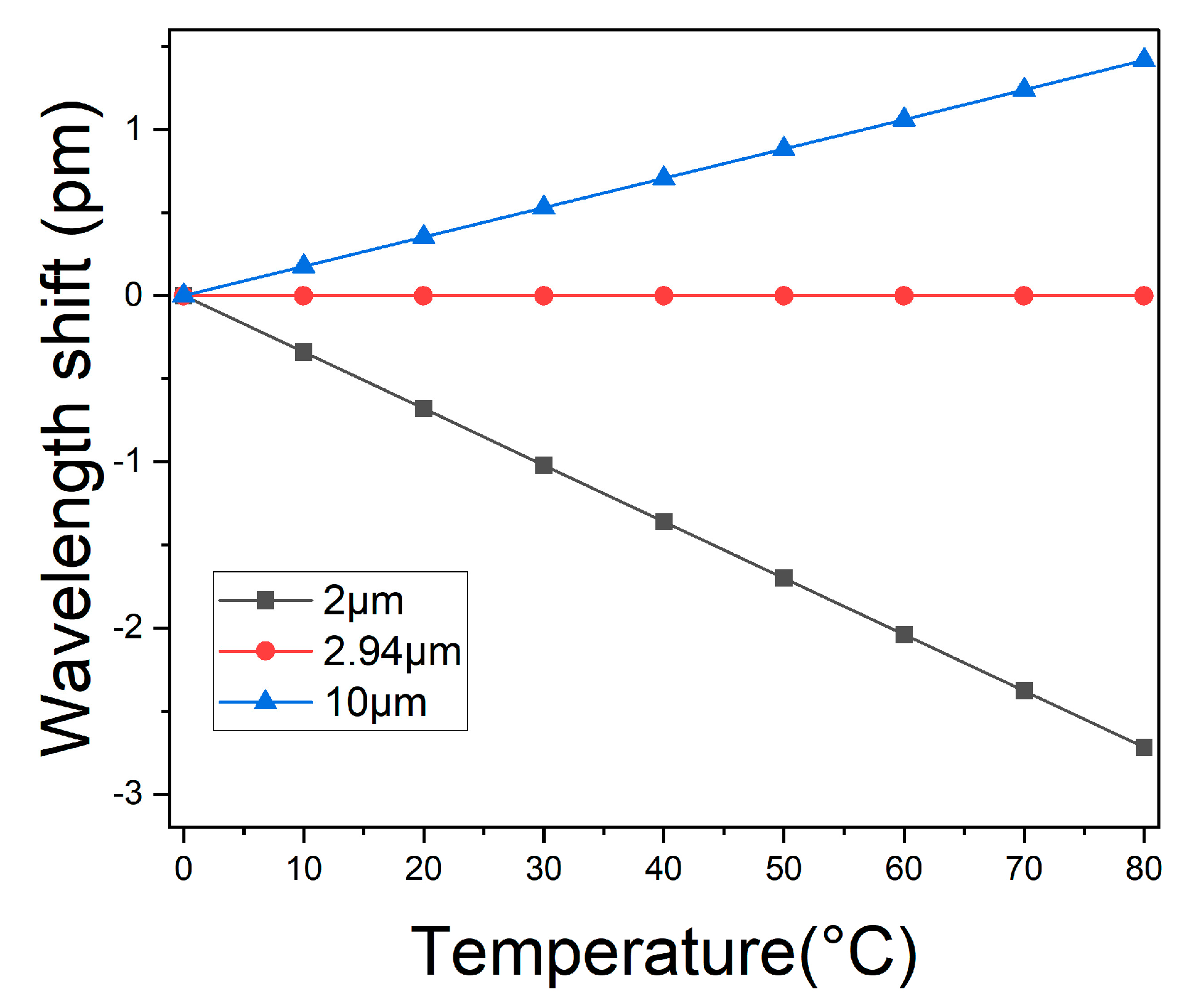
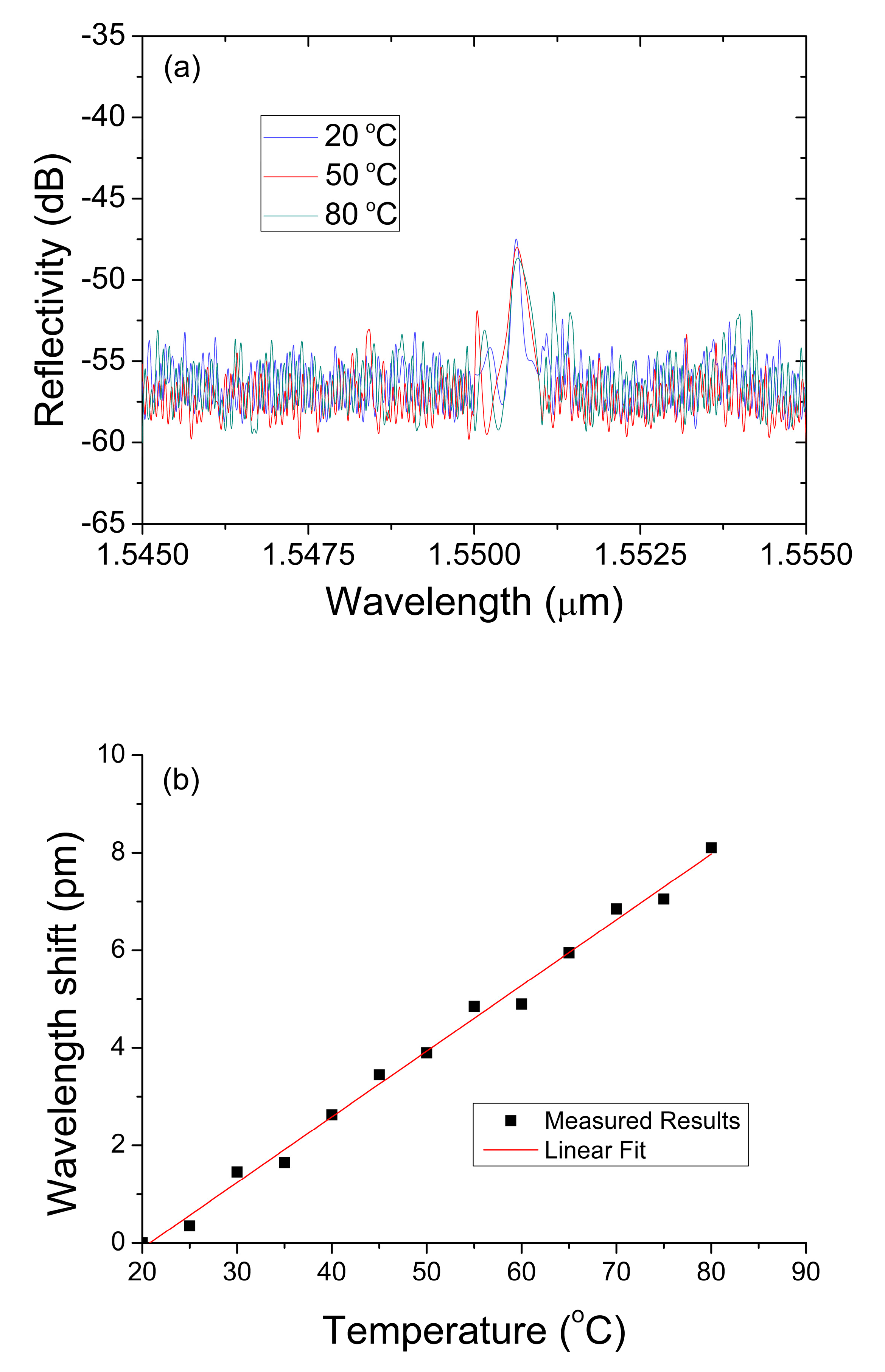
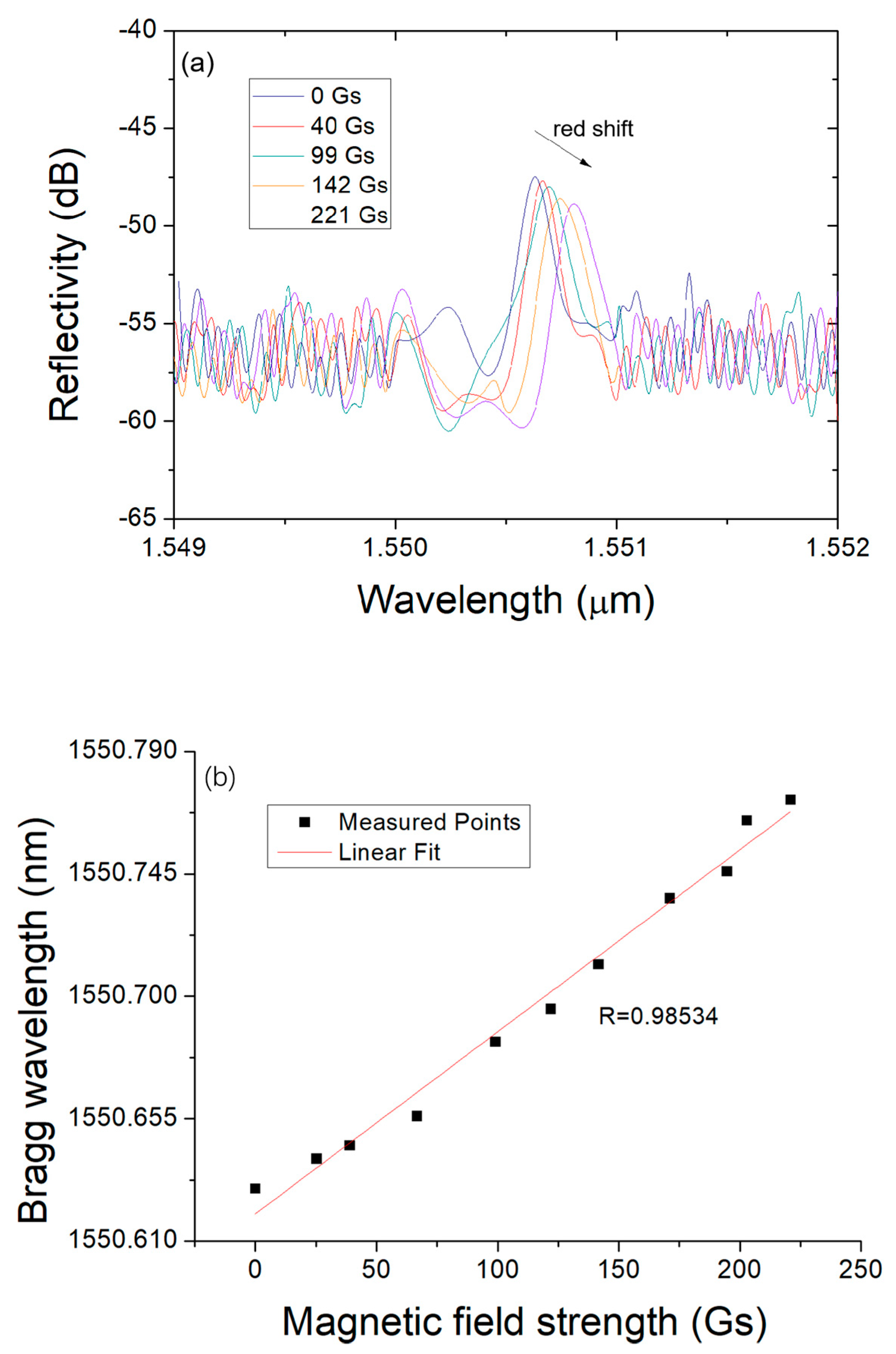
3. Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, B.; Lu, C.; Mao, B.-M.; Tam, H.-Y.; He, S. Magnetic field sensor of enhanced sensitivity and temperature self-calibration based on silica fiber Fabry-Perot resonator with silicone cavity. Opt. Express 2017, 25, 8108–8114. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Han, Q.; Liu, T.; Lan, X.; Xiao, H. Optical fiber magnetic field sensor based on single-mode-multimode-single-mode structure and magnetic fluid. Opt. Lett. 2013, 38, 3999–4001. [Google Scholar] [CrossRef] [PubMed]
- Tian, Q.; Feng, Z.; Rong, Q.; Wan, Y.; Qiao, X.; Hu, M.; Yang, H.; Wang, R.; Shao, Z.; Yang, T. A temperature-independent fibre-optic magnetic-field sensor using thincore fibre tailored fibre Bragg grating. Opt. Commun. 2017, 393, 169–172. [Google Scholar] [CrossRef]
- Horng, H.E.; Chen, C.S.; Fang, K.L.; Yang, S.Y.; Chieh, J.J.; Hong, C.-Y.; Yang, H.C. Tunable optical switch using magnetic fluids. Appl. Phys. Lett. 2004, 85, 5592–5594. [Google Scholar] [CrossRef]
- Zu, P.; Chan, C.C.; Koh, G.W.; Lew, W.S.; Jin, Y.; Liew, H.F.; Wong, W.C.; Dong, X. Enhancement of the sensitivity of magneto-optical fiber sensor by magnifying the birefringence of magnetic fluid film with Loyt-Sagnac interferometer. Sens. Actuators B Chem. 2013, 191, 19–23. [Google Scholar] [CrossRef]
- Yang, S.Y.; Horng, H.; Hong, C.; Yang, H.C. Structures, optical properties and properties and potentially electro-optical applications of magnetic fluid films. Tamkang J. Sci. Eng. 2002, 5, 85–93. [Google Scholar]
- Wei, F.; Liu, D.; Mallik, A.K.; Farrell, G.; Wu, Q.; Peng, G.-D.; Semenova, Y. Temperature-compensated magnetic field sensing with a dual-ring structure consisting of microfiber coupler-Sagnac loop and fiber Bragg grating-assisted resonant cavity. Appl. Opt. 2019, 58, 2334–2339. [Google Scholar] [CrossRef]
- Lv, F.; Han, C.; Ding, H.; Wu, Z.; Li, X. Magnetic Field Sensor Based on Microfiber Sagnac Loop Interferometer and Ferrofluid. IEEE Photon. J. 2015, 27, 2327–2330. [Google Scholar] [CrossRef]
- Li, Z.; Liao, C.; Song, J.; Wang, Y.; Zhu, F.; Dong, X. Ultrasensitive magnetic field sensor based on an in-fiber Mach–Zehnder interferometer with a magnetic fluid component. Photon. Res. 2016, 4, 197–201. [Google Scholar] [CrossRef]
- Zhu, R.-Z.; Wan, S.-J.; Xiong, Y.-F.; Feng, H.-G.; Chen, Y.; Lu, Y.; Xu, F. Magnetic Field Sensing Based on Multimode Fiber Specklegrams. IEEE J. Light. Technol. 2021, 39, 3614–3619. [Google Scholar] [CrossRef]
- Zu, P.; Chan, C.; Lew, W.; Hu, L.; Jin, Y.; Liew, H.; Chen, L.; Wong, W.; Dong, X. Temperature-insensitive magnetic field sensor based on nanoparticle magnetic fluid and photonic crystal fiber. IEEE Photon. J. 2012, 4, 491–498. [Google Scholar]
- Li, X.; Ding, H. Temperature Insensitive Magnetic Field Sensor Based on Ferrofluid Clad Microfiber Resonator. IEEE Photon. Technol. Lett. 2014, 26, 2426–2429. [Google Scholar] [CrossRef]
- Ying, Y.; Zhang, R.; Si, G.; Wang, X.; Qi, Y. D-shaped titled fiber Bragg grating using magnetic fluid for magnetic field sensor. Opt. Commun. 2017, 405, 228–232. [Google Scholar] [CrossRef]
- Zhang, N.M.Y.; Dong, X.; Shum, P.; Hu, D.; Su, H.; Lew, W.; Wei, L. Magnetic field sensor based on magnetic-fluid-coated long-period fiber grating. J. Opt. 2015, 17, 065402. [Google Scholar] [CrossRef]
- Sahu, S.; Ali, J.; Yupapin, P.P.; Singh, G.; Grattan, K.T.V. High-Q and temperature stable photonic biosensor based on grating waveguides. Opt. Quantum Electron. 2018, 50, 307. [Google Scholar] [CrossRef]
- Sahu, S.; Ali, J.; Yupapin, P.P.; Singh, G. Optical biosensor based on a cladding modulated grating waveguide. Optik 2018, 166, 103–109. [Google Scholar] [CrossRef]
- Prabhathan, P.; Murukeshan, V.M.; Jing, Z.; Ramana, P.V. Compact SOI nanowire refractive index sensor using phase shifted Bragg grating. Opt. Express 2009, 17, 15330–15341. [Google Scholar] [CrossRef]
- Sahu, S.; Ali, J.; Singh, G. Refractive index biosensor using sidewall gratings in dual-slot waveguide. Opt. Commun. 2017, 402, 408–412. [Google Scholar] [CrossRef]
- Gunawardena, D.S.; Cheng, X.; Cui, J.; Edbert, G.; Lu, L.; Ho, Y.T.; Tam, H.-Y. Regenerated polymer optical fiber Bragg gratings with thermal treatment for high temperature measurements. Photon. Res. 2022, 10, 1011–1021. [Google Scholar] [CrossRef]
- D’Amato, R.; Polimadei, A.; Terranova, G.; Caponero, M.A. Humidity sensing by chitosan-coated fiber Bragg gratings (FBG). Sensors 2021, 21, 3348. [Google Scholar] [CrossRef]
- Abro, Z.A.; Hong, C.; Zhang, Y.; Siddiqui, M.; Abbasi, A.; Abro, Z.; Tariq, S.Q.B. Development of FBG pressure sensors using FDM technique for monitoring sleeping postures. Sens. Actuators A Phys. 2021, 331, 112921. [Google Scholar] [CrossRef]
- Kuse, N.; Ozawa, A.; Kobayashi, Y. Static FBG strain sensor with high resolution and large dynamic range by dual-comb spectroscopy. Opt. Express 2013, 21, 11141–11149. [Google Scholar] [CrossRef] [PubMed]
- Zheng, J.; Dong, X.; Zu, P.; Ji, J.; Su, H. Intensity-modulated magnetic field sensor based on magnetic fluid and optical fiber gratings. Appl. Phys. Lett. 2013, 103, 183511. [Google Scholar] [CrossRef]
- Dai, J.; Yang, M.; Li, X.; Liu, H.; Tong, X. Magnetic field sensor based on magnetic fluid clad etched fiber Bragg grating. Opt. Fiber Technol. 2011, 17, 210–213. [Google Scholar] [CrossRef]
- Zheng, J.; Dong, X.; Zu, P.; Shao, L.Y.; Chan, C.; Cui, Y.; Shum, P.P. Magnetic field sensor using tilted fiber grating interacting with magnetic fluid. Opt. Express 2013, 21, 17863–17868. [Google Scholar] [CrossRef] [PubMed]
- Yang, D.; Xu, L.D.Z.; Jiang, Y.; Xu, J.; Wang, M.; Bai, Y.; Wang, H. Magnetic field sensing based on tilted fiber Bragg grating coated with nanoparticle magnetic fluid. Appl. Phys. Lett. 2014, 104, 061903. [Google Scholar] [CrossRef]
- Bao, L.; Dong, X.; Zhang, S.; Shen, C.; Shum, P.P. Magnetic Field Sensor Based on Magnetic Fluid-Infiltrated Phase-Shifted Fiber Bragg Grating. IEEE Sens. J. 2018, 18, 4008–4012. [Google Scholar] [CrossRef]
- Ruan, Z.; Pei, L.; Ning, T.; Wang, J.; Wang, J.; Li, J.; Xie, Y.; Zhao, Q.; Zheng, J. Simple structure of tapered FBG filled with magnetic fluid to realize magnetic field sensor. Opt. Fiber Technol. 2021, 67, 102698. [Google Scholar] [CrossRef]
- Bao, W.; Qiao, X.; Rong, Q.; Chen, F. Fiber-Optic Vector Magnetometer Based on Magnetic Fluid and Fiber Bragg Grating Written on a Multi-Clad Fiber. IEEE Sens. J. 2018, 18, 7486–7491. [Google Scholar] [CrossRef]
- Nie, L.; Wu, Y.; Gao, X.; Luo, H.; Li, X.; Wang, X.; Liu, G. Optimized optical tunable microfiber-Bragg grating. Optik 2022, 270, 170086. [Google Scholar] [CrossRef]
- Gao, S.; Jin, L.; Ran, Y.; Sun, L.-P.; Li, J.; Guan, B.-O. Temperature compensated microfiber Bragg gratings. Opt. Express 2012, 20, 18281–18286. [Google Scholar] [CrossRef] [PubMed]
- Luo, H.; Li, X.; Zou, W.; Jiang, W.; Chen, J. Modal Interferometer Based on a C-Shaped Ultrathin Fiber Taper for High-Sensitivity Refractive Index Measurement. Appl. Phys. Express 2012, 5, 012502. [Google Scholar] [CrossRef]
- Kersey, A.D.; Davis, M.A.; Patrick, H.J.; LeBlanc, M.; Koo, K.P.; Askins, C.G.; Friebele, E.J. Fiber grating sensors. IEEE J. Light. Technol. 1997, 15, 1442–1463. [Google Scholar] [CrossRef]



| Ref. | Sensor Structure | Measurement Range | Sensitivity | Temperature Range | Temperature Sensitivity |
| [11] | PCF | 300–500 Gs | 2.367 pm/Gs | 30–100 °C | 3.2 pm/°C |
| [12] | MKR | 0–220 Gs | 0.56 pm/Gs | 20–80 °C | 0.17 pm/°C |
| [25,26] | Tilted FBG | 0–190 Gs | 0.451 pm/Gs | 20–60 °C | 8.4 pm/°C |
| Our work | MF-BG | 0–221 Gs | 0.667 pm/Gs | 20–80 °C | 0.13 pm/°C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, H.; Liu, Y.; Nie, L.; Wang, Z.; Gao, X.; Wang, Y.; Zhao, J.; Liu, G.; Xu, S. Temperature-Insensitive Ferrofluid-Clad Microfiber Bragg Grating for Magnetic Field Sensing. Photonics 2023, 10, 249. https://doi.org/10.3390/photonics10030249
Luo H, Liu Y, Nie L, Wang Z, Gao X, Wang Y, Zhao J, Liu G, Xu S. Temperature-Insensitive Ferrofluid-Clad Microfiber Bragg Grating for Magnetic Field Sensing. Photonics. 2023; 10(3):249. https://doi.org/10.3390/photonics10030249
Chicago/Turabian StyleLuo, Haimei, Yangyang Liu, Liyuan Nie, Zeng Wang, Xiaoyong Gao, Yifan Wang, Jiajia Zhao, Guiqiang Liu, and Shaoyi Xu. 2023. "Temperature-Insensitive Ferrofluid-Clad Microfiber Bragg Grating for Magnetic Field Sensing" Photonics 10, no. 3: 249. https://doi.org/10.3390/photonics10030249
APA StyleLuo, H., Liu, Y., Nie, L., Wang, Z., Gao, X., Wang, Y., Zhao, J., Liu, G., & Xu, S. (2023). Temperature-Insensitive Ferrofluid-Clad Microfiber Bragg Grating for Magnetic Field Sensing. Photonics, 10(3), 249. https://doi.org/10.3390/photonics10030249





