Diffraction Characteristics of a Digital Micromirror Device for Computer Holography Based on an Accurate Three-Dimensional Phase Model
Abstract
1. Introduction
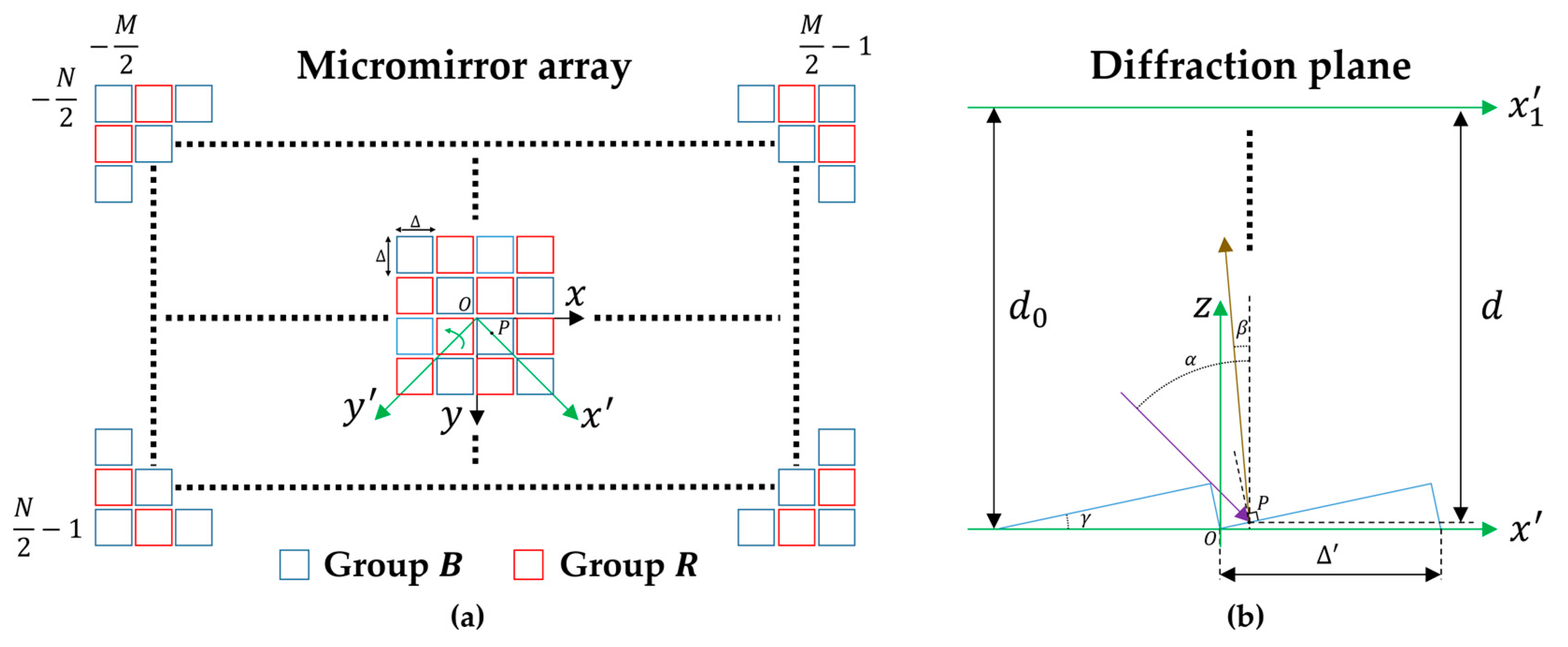
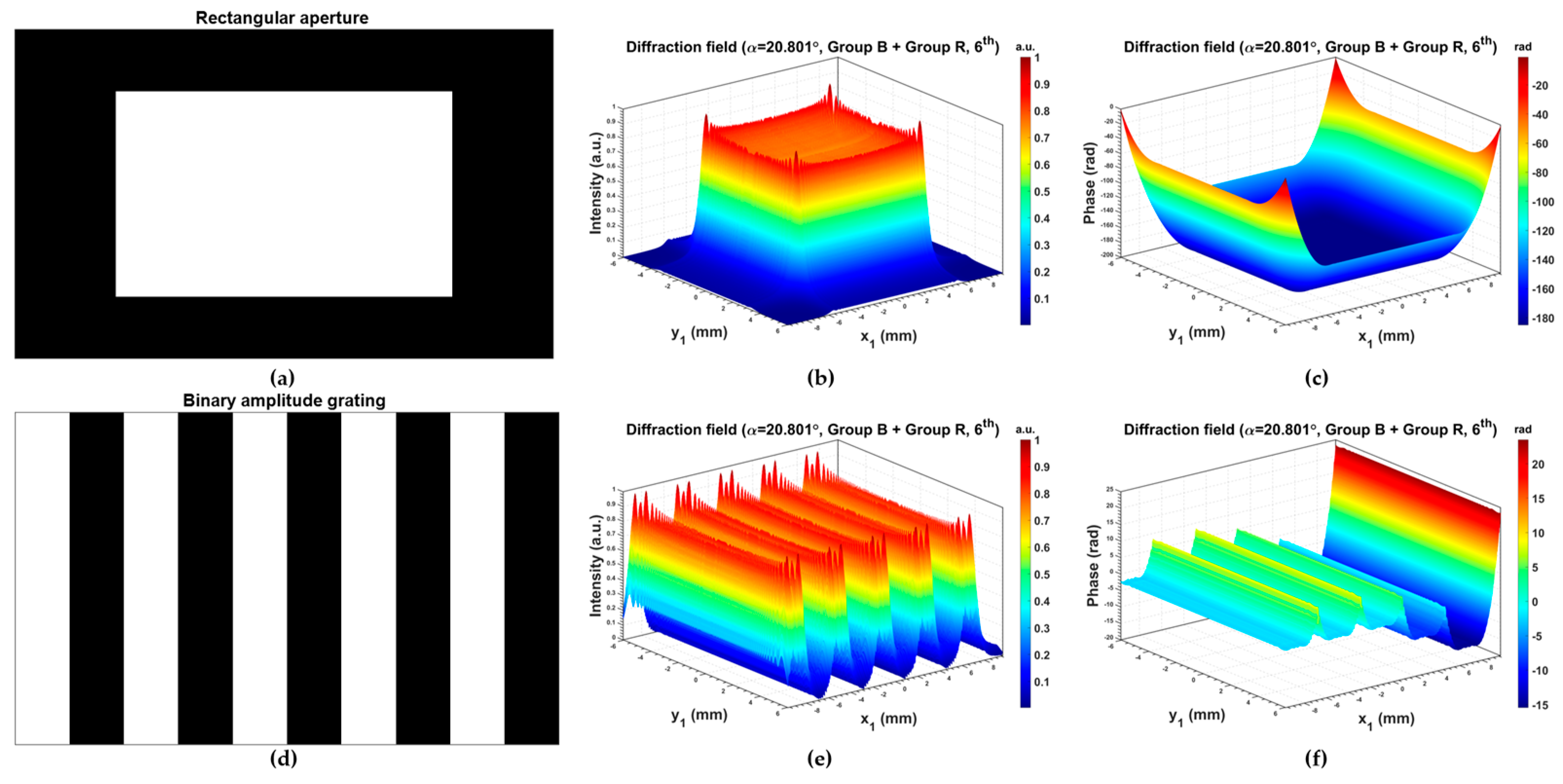
2. Diffraction Characteristics Analysis
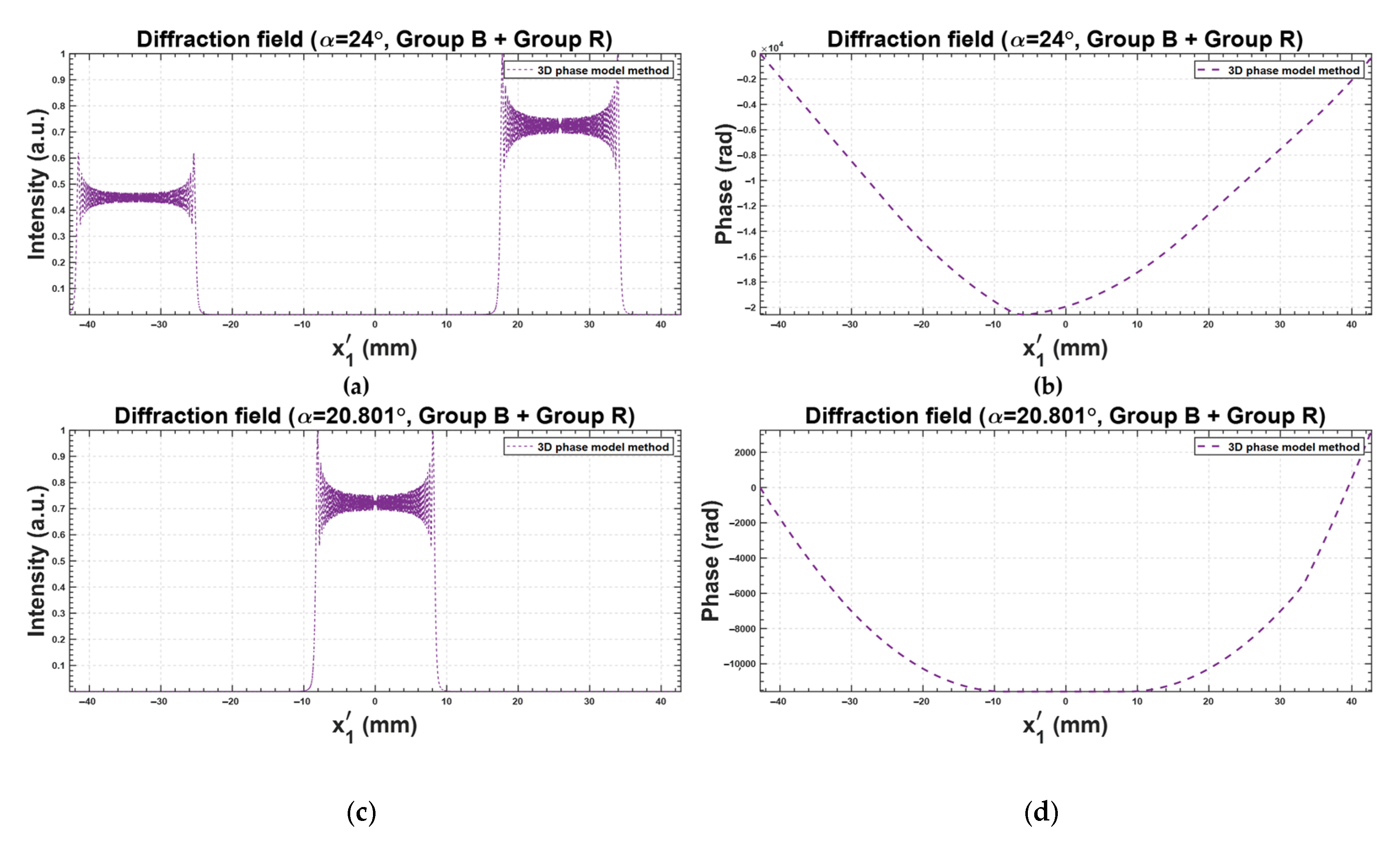
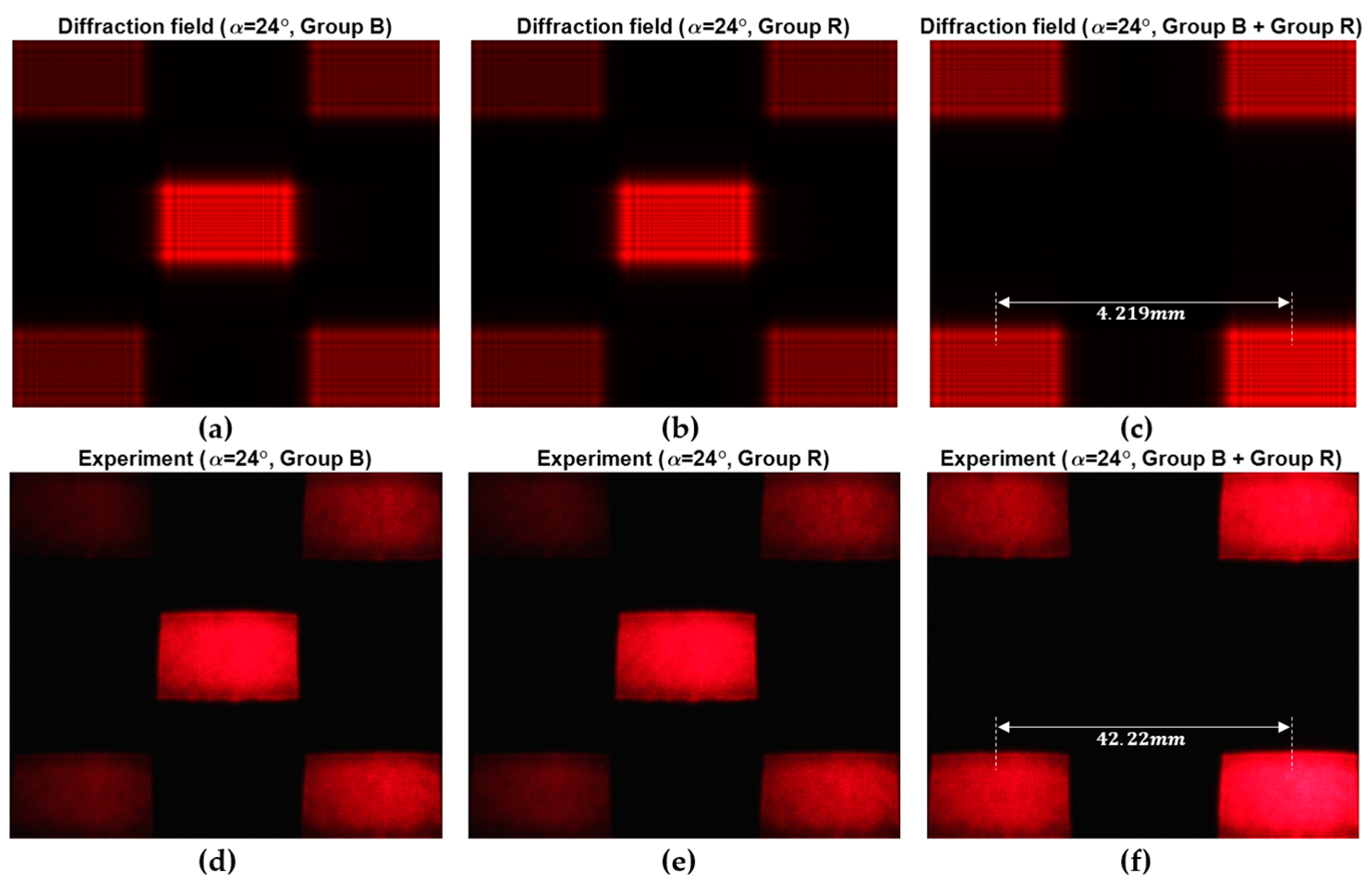
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Benton, S.A.; Bove Jr, V.M. Holographic Imaging; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Zhang, H.; Zhao, Y.; Cao, L.; Jin, G. Three-dimensional display technologies in wave and ray optics: A review. Chin. Opt. Lett. 2014, 12, 060002. [Google Scholar] [CrossRef]
- Blinder, D.; Birnbaum, T.; Ito, T.; Shimobaba, T. The state-of-the-art in computer generated holography for 3D display. Light Adv. Manuf. 2022, 3, 572–600. [Google Scholar] [CrossRef]
- Sun, B.; Salter, P.S.; Roider, C.; Jesacher, A.; Strauss, J.; Heberle, J.; Schmidt, M.; Booth, M.J. Four-dimensional light shaping: Manipulating ultrafast spatiotemporal foci in space and time. Light Sci. Appl. 2018, 7, 17117. [Google Scholar] [CrossRef]
- Rubinsztein-Dunlop, H.; Forbes, A.; Berry, M.V.; Dennis, M.R.; Andrews, D.L.; Mansuripur, M.; Denz, C.; Alpmann, C.; Banzer, P.; Bauer, T.; et al. Roadmap on structured light. J. Opt. 2017, 19, 013001. [Google Scholar] [CrossRef]
- Fang, Z.-X.; Zhao, H.-Z.; Chen, Y.; Lu, R.-D.; He, L.-Q.; Wang, P. Accelerating polygon beam with peculiar features. Sci. Rep. 2018, 8, 8593. [Google Scholar] [CrossRef] [PubMed]
- Chlipala, M.; Kozacki, T. Color LED DMD holographic display with high resolution across large depth. Opt. Lett. 2019, 44, 4255–4258. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Zhang, H.; Cao, L.; Jin, G. Generalized single-sideband three-dimensional computer-generated holography. Opt. Express 2019, 27, 2612–2620. [Google Scholar] [CrossRef]
- Lee, B.; Yoo, D.; Jeong, J.; Lee, S.; Lee, D.; Lee, B. Wide-angle speckleless DMD holographic display using structured illumination with temporal multiplexing. Opt. Lett. 2020, 45, 2148–2151. [Google Scholar] [CrossRef]
- Gao, H.; Wang, Y.; Fan, X.; Jiao, B.; Li, T.; Shang, C.; Zeng, C.; Deng, L.; Xiong, W.; Xia, J.; et al. Dynamic 3D meta-holography in visible range with large frame number and high frame rate. Sci. Adv. 2020, 6, eaba8595. [Google Scholar] [CrossRef]
- Li, J.; Smithwick, Q.; Chu, D. Holobricks: Modular coarse integral holographic displays. Light: Sci. Appl. 2022, 11, 57. [Google Scholar] [CrossRef]
- Bartlett, T.A.; McDonald, W.C.; Hall, J.N. Adapting Texas Instruments DLP technology to demonstrate a phase spatial light modulator. In Proceedings of the Emerging Digital Micromirror Device Based Systems and Applications XI, San Francisco, CA, USA, 4 March 2019. [Google Scholar]
- Bartlett, T.A.; McDonald, W.C.; Hall, J.N.; Oden, P.I.; Doane, D.; Ketchum, R.S.; Byrum, T. Recent advances in the development of the Texas Instruments phase-only microelectromechanical systems (MEMS) spatial light modulator. In Proceedings of the Emerging Digital Micromirror Device Based Systems and Applications XIII, Online, 9 March 2021. [Google Scholar]
- Ketchum, R.S.; Blanche, P.-A. Diffraction Efficiency Characteristics for MEMS-Based Phase-Only Spatial Light Modulator with Nonlinear Phase Distribution. Photonics 2021, 8, 62. [Google Scholar] [CrossRef]
- Douglass, M.R.; Hall, J.N.; Oden, P.I.; Byrum, T.M. Reliability assessment of the Texas Instruments phase light modulator. In Proceedings of the Emerging Digital Micromirror Device Based Systems and Applications XIV, San Francisco, CA, USA, 1 March 2022. [Google Scholar]
- Choi, S.; Gopakumar, M.; Peng, Y.; Kim, J.; O’Toole, M.; Wetzstein, G. Time-multiplexed Neural Holography: A Flexible Framework for Holographic Near-eye Displays with Fast Heavily-quantized Spatial Light Modulators. In Proceedings of the ACM SIGGRAPH 2022 Conference Proceedings, Vancouver, BC, Canada, 7–11 August 2022. [Google Scholar]
- Hornbeck, L. Deformable-Mirror Spatial Light Modulators; SPIE: Philadelphia, PA, USA, 1990; Volume 1150. [Google Scholar]
- Brennesholtz, M.S.; Stupp, E.H. Projection Displays; Wiley Publishing: Indianapolis, IN, USA, 2008. [Google Scholar]
- Son, J.-Y.; Lee, B.-R.; Chernyshov, O.O.; Moon, K.-A.; Lee, H. Holographic display based on a spatial DMD array. Opt. Lett. 2013, 38, 3173–3176. [Google Scholar] [CrossRef] [PubMed]
- Park, M.-C.; Lee, B.-R.; Son, J.-Y.; Chernyshov, O. Properties of DMDs for holographic displays Properties of DMDs for holographic displays. J. Mod. Opt. 2015, 62, 1600–1607. [Google Scholar] [CrossRef]
- Son, J.-Y.; Son, W.-H.; Kim, J.-H.; Choo, H. Image reconstruction in an electro-holographic display. J. Opt. 2017, 19, 055604. [Google Scholar] [CrossRef]
- Cheremkhin, P.A.; Kurbatova, E.A. Comparative appraisal of global and local thresholding methods for binarisation of off-axis digital holograms. Opt. Lasers Eng. 2019, 115, 119–130. [Google Scholar] [CrossRef]
- Kreis, T.; Aswendt, P.; Hoefling, R. Hologram reconstruction using a digital micromirror device. OPTICE 2001, 40, 926–933. [Google Scholar]
- Wang, D.; Wei, S. Characterization of a Digital Micromirror Device for Computer Generated Video Holography. In Proceedings of the Fourth International Conference on Image and Graphics (ICIG 2007), Chengdu, China, 22–24 August 2007; pp. 855–862. [Google Scholar]
- Scholes, S.; Kara, R.; Pinnell, J.; Rodríguez-Fajardo, V.; Forbes, A. Structured light with digital micromirror devices: A guide to best practice. OPTICE 2019, 59, 041202. [Google Scholar] [CrossRef]
- Chen, X.; Yan, B.-b.; Song, F.-j.; Wang, Y.-q.; Xiao, F.; Alameh, K. Diffraction of digital micromirror device gratings and its effect on properties of tunable fiber lasers. Appl. Opt. 2012, 51, 7214–7220. [Google Scholar] [CrossRef]
- Xiong, Z.; Liu, H.; Tan, X.; Lu, Z.; Li, C.; Song, L.; Wang, Z. Diffraction analysis of digital micromirror device in maskless photolithography system. J. Micro/Nanolithogr. MEMS MOEMS 2014, 13, 043016. [Google Scholar] [CrossRef]
- Ren, Y.-X.; Lu, R.-D.; Gong, L. Tailoring light with a digital micromirror device. Ann. Der Phys. 2015, 527, 447–470. [Google Scholar] [CrossRef]
- Han, Q.; Zhang, J.; Wang, J.; Sun, Q. Diffraction analysis for DMD-based scene projectors in the long-wave infrared. Appl. Opt. 2016, 55, 8016–8021. [Google Scholar] [CrossRef] [PubMed]
- Dong, X.; Shi, Y.; Xiao, X.; Zhang, Q.; Chen, F.; Sun, X.; Yuan, W.; Yu, Y. Non-paraxial diffraction analysis for developing DMD-based optical systems. Opt. Lett. 2022, 47, 4758–4761. [Google Scholar] [CrossRef] [PubMed]
- Casini, R.; Nelson, P.G. On the intensity distribution function of blazed reflective diffraction gratings. J. Opt. Soc. Am. A 2014, 31, 2179–2184. [Google Scholar] [CrossRef] [PubMed]
- Molodtsov, D.Y.; Rodin, V. Object recognition in non-coherent optical correlator based on DMD-modulator. In Proceedings of the Asia-Pacific Conference on Fundamental Problems of Opto-and Microelectronics, Khabarovsk, Russia, 14 December 2016. [Google Scholar]
- Stsepuro, N.; Kovalev, M.; Zlokazov, E.; Kudryashov, S. Wavelength-Independent Correlation Detection of Aberrations Based on a Single Spatial Light Modulator. Photonics 2022, 9, 909. [Google Scholar] [CrossRef]
- Molodtsov, D.Y.; Cheremkhin, P.A.; Krasnov, V.V.; Rodin, V.G. Impact of DMD-SLMs errors on reconstructed Fourier holograms quality. J. Phys. Conf. Ser. 2016, 737, 012074. [Google Scholar] [CrossRef]
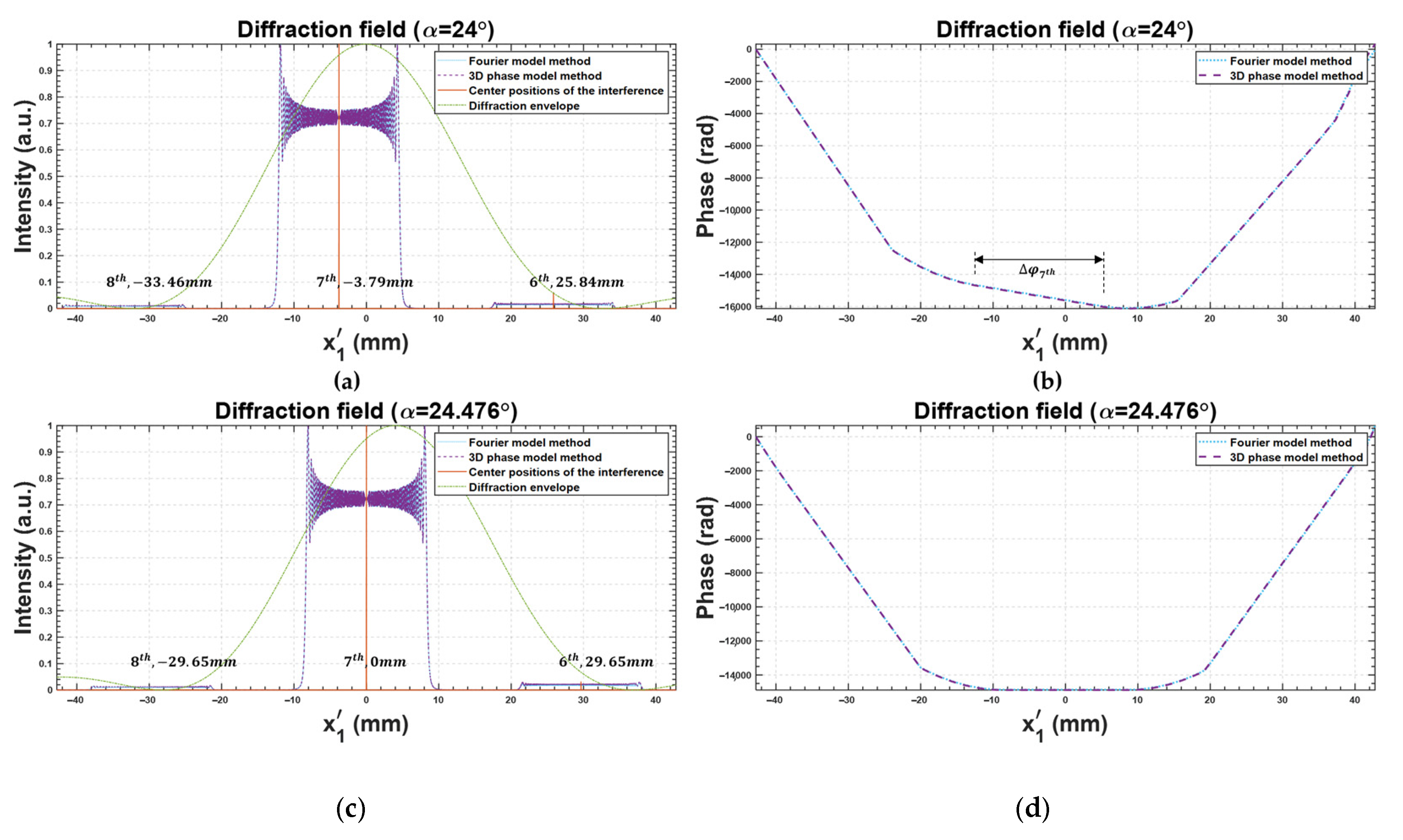


| Order (Center Positions) | 3D Phase Model /Fourier Model (°) | 3D Phase Model/ Fourier Model (mm) | Theory (°) | Theory (mm) |
|---|---|---|---|---|
| 6th | 2.96 | 25.84 | 2.96 | 25.84 |
| 7th | −0.43 | −3.79 | −0.43 | −3.79 |
| 8th | −3.83 | −33.46 | −3.83 | −33.46 |
| 3D Phase Model (Rad) | Fourier Model (Rad) | Theory (Rad) | |
|---|---|---|---|
| 6th | 8765.00 | 8765.00 | 8766.38 |
| 7th | −1286.52 | −1286.52 | −1286.72 |
| 8th | −11,337.98 | −11,337.97 | −11,339.82 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Zhang, H. Diffraction Characteristics of a Digital Micromirror Device for Computer Holography Based on an Accurate Three-Dimensional Phase Model. Photonics 2023, 10, 130. https://doi.org/10.3390/photonics10020130
Wang X, Zhang H. Diffraction Characteristics of a Digital Micromirror Device for Computer Holography Based on an Accurate Three-Dimensional Phase Model. Photonics. 2023; 10(2):130. https://doi.org/10.3390/photonics10020130
Chicago/Turabian StyleWang, Xiaoyu, and Hao Zhang. 2023. "Diffraction Characteristics of a Digital Micromirror Device for Computer Holography Based on an Accurate Three-Dimensional Phase Model" Photonics 10, no. 2: 130. https://doi.org/10.3390/photonics10020130
APA StyleWang, X., & Zhang, H. (2023). Diffraction Characteristics of a Digital Micromirror Device for Computer Holography Based on an Accurate Three-Dimensional Phase Model. Photonics, 10(2), 130. https://doi.org/10.3390/photonics10020130






