1. Introduction
In the last few decades, the structured light beam [
1,
2] has gained significant importance which can be understood from the ever-growing list of reported beam properties and applications. The unique characteristics exhibited by structured beams enable them to be used in applications such as optical communication [
3], optical computation [
4], optical trapping [
5], optical metrology [
6], material characterization [
7], cryptography [
8], short-pulse interferometry [
9], etc. The Laguerre–Gaussian (LG) beam is one member of the structured beam family that is extensively studied. It has a donut-shaped symmetric intensity profile and a helical-shaped phase profile, whose respective size and shape depend on a quantized parameter known as the mode number. Due to the helical phase profile and the dark spot at the center, they are also called vortex beams. LG beams can be mathematically defined as an exact paraxial solution to the Helmholtz wave equation in the circular-cylindrical coordinate system. Different methods for its practical generation [
10,
11] and detection [
12] have been reported in the past. Asymmetric vortex beams’ with fractional topological charge were experimentally generated using a multilevel spiral phase Fresnel zone plate [
13] and Fresnel zone lens with spiral phase [
14]. For the first time, Kotlyar et al. have introduced ‘asymmetricity’ in the fundamental Helmholtz beams for integer topological charges through a family of asymmetric Bessel [
15] and asymmetric Bessel-Gauss beams [
16]. Later, several other asymmetric structured beams [
17,
18,
19,
20,
21,
22] including asymmetric LG beam [
23] were reported. An asymmetric LG beam can be generated by adding a complex shift along the cartesian plane of the symmetric LG beam. The complex shift determines the amount of asymmetricity in the LG beam. These complex modulations are practically realized by employing devices such as digital micromirror devices (DMD) [
24], spatial light modulators (SLM) [
23], astigmatic mode converters [
25] etc. Please note, an ‘asymmetric LG beam’ and an ‘asymmetric Gaussian optical vortex beam’ are different due to the different orbital angular momentum (OAM) characteristics exhibited by them [
18]. An asymmetric LG beam can have both integral and fractional OAM which offers certain advantages over symmetric LG beams. The transverse intensity profile of an asymmetric LG beam assumes a crescent shape which reduces the heat exposure in a particle trapping setup when compared to a symmetric LG beam [
26]. The asymmetric intensity profile can also act as an additional degree of freedom which offers more data to be encoded within a given beam mode. However, in order to harness this potential it is first required to generate a densely packed large ‘array’ of these beams. This is usually achieved through angular or spatial multiplexing techniques.
Several reports exist on ‘structured beam array’ generation where different tools and methods were investigated. Lin et al. have discussed the generation of the Bessel beam array using metamaterials [
27]. Lu et al. have shown the generation of the Gaussian vortex array [
28] using the computer-generated holography(CGH) technique. The same technique was used by Suarez et al. for the generation of an Airy-vortex array [
29]. Jackin et al. have shown high-density generation of the vortex beam array using azocarbazole polymer, CGH, and digital hologram printing technique [
30]. The generation of a large spatial mode array has been reported by Rosales et al. [
31]. From the above, it can be understood that CGH has evolved as the most popular technique for generating structured beam arrays. This is because a CGH can be easily tailored for any arbitrary set of beams, to be included in an array. There exist several CGH encoding and multiplexing techniques for realizing this tailoring process. Wang et al. have reported the generation of a
Ince-Gaussian beam array [
32] and then later an arbitrary circular Airy-Gaussian vortex beam array [
33], using the ‘spatial multiplexing’ technique. Jackin et al. have employed an ‘angular multiplexing’ technique to generate a
vortex array from a compact device as small as 1 mm
[
30]. Now considering CGH encoding techniques, phase-only encoding [
34], and complex amplitude encoding using super-pixel hologram [
35], and phase hologram [
36] are the most popular ones used for structured beam generation.
To be best of our knowledge all previous reports on structured beam array are limited to symmetric beams only and the usage of asymmetric beams are yet to be reported. Here we report for the first time the practical generation of a large array of asymmetric LG beams. This was achieved using spatially multiplexed and complex amplitude-encoded phase holograms. The beam array was practically realized using an optical system that employs an SLM to display the computed holograms. We show that each beam in the array can have different asymmetricity (that is distinguishable) for the same mode number and hence can encode more information compared to a symmetric LG beam array.
2. Theory and Methods
In this section, we start our discussion with the basic theory of asymmetric Laguerre–Gaussian beams and then present our method for the practical generation of a large array of these beams. The paraxial solution of the Helmholtz wave equation in circular cylindrical coordinates results in an LG beam which can be written in Cartesian coordinates as (Equation (
1))
where,
,
p and
l are radial and azimuthal index respectively,
is the spot size of the beam which is related to beam waist (
), and Rayleigh distance (
) as,
,
R(z) is the radius of curvature of the wavefront in the z-plane which is given as
and
is the Gouy phase. A complex shift (
) to the symmetric beam in the cartesian plane adds asymmetricity and the beam is thereafter known as an ‘asymmetric LG beam’ [
23] which is defined by Equation (
2)
where
and (
) are complex shifts that decide the amount of asymmetricity in the LG beam. If the value
, and
are finite and real then an off-axis LG beam can be obtained. The asymmetric LG beams also obey the same diffraction properties as that of symmetric LG beams and hence experience rotation and expansion in their transverse intensity profile during propagation. This rotation can be visualized from the asymmetric transverse intensity profile of an asymmetric LG beam which is usually not visible in the case of symmetric LG beams [
23]. In order to compute the CGH of an asymmetric LG beam, the electric field at the distance z = 0 is required, which is given by Equation (
3).
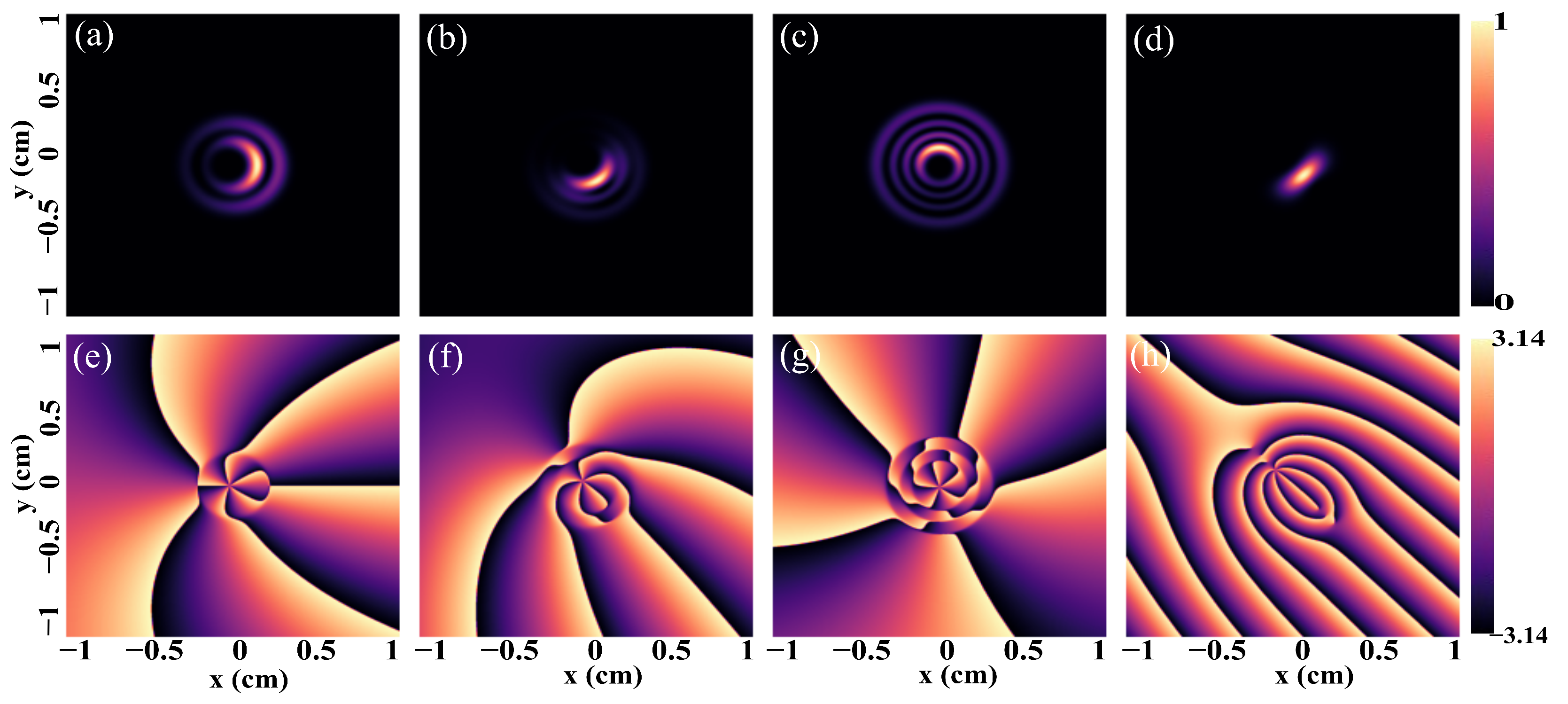
The simulated intensity and phase profile of the asymmetric LG beam computed using Equation (
3), is shown in the first and second rows of
Figure 1 respectively. The asymmetric LG beams were set with a beam waist of 1.2 mm, a wavelength of 532 nm, and a distance of propagation z = 0 m, for varying asymmetricity and mode numbers as follows, (a,e)
,
,
p = 1 and
l = 5 (b,f)
,
,
p = 2 and
l = 5 (c,g)
,
,
p = 3 and
l = 5 (d,h)
,
,
p = 3 and
l = 5. It can be clearly seen that the intensity distribution across the transverse plane of the beam assumes a crescent-shaped asymmetric profile. When the asymmetricity parameters (
) take large values the crescent shape turns into a sheet-like structure (
Figure 1d), and is often called a sheet beam.
The direction of the opening of the crescent-shaped transverse intensity pattern depends on the sign and value of asymmetric parameters
and
(Equation (
3)) which is graphically represented in
Figure 2. As described in
Figure 2, the crescent-like shape should have a left side opening when
= 0 and
> 0 which is shown in
Figure 1a. When the magnitude of both asymmetric parameters is positive (
> 0 and
> 0) then the crescent has a top-left side opening which can be seen from
Figure 1b. Similarly, the
and
parameters that represent any other directional orientation of the crescent-shaped intensity profile, can be understood from
Figure 2. Thus using the values (
) any desired asymmetricity and its directional orientation can be obtained.
Now we will move our attention to beam array generation for which we employ the multiplexed hologram technique. Though the random/angularly multiplexed holograms (due to their larger size) offer higher numerical aperture (for a given hologram size) compared to spatially multiplexed ones, here we resort to the spatial multiplexing technique for the following reason. In the random/angular multiplexing technique, the complex amplitudes of all the individual beams in the array are spatially overlapped (added), each with a different random/carrier function. However, this overlap reduces the fringe contrast significantly since a ‘Gaussian envelope’ term (such as an amplitude mask) is dominant in an LG beam. Discarding the Gaussian envelope works to some extent in the case of a symmetric beam but significantly affects the characteristics of an asymmetric LG beam (especially the directional orientation). On the other hand, in a spatial multiplexing technique, the holograms that correspond to each beam in the array are spatially separated (tiled), thereby alleviating the issue. In order to realize this, first, a meshgrid of size
consisting of
pixels where
and
are the physical dimension (size) and
and
are the total numbers of pixels in the x and y-directions respectively. In order to generate a beam array of size
, the meshgrid is divided into
tiles such that each tile possesses an area of
and the total number of pixels associated with each tile is
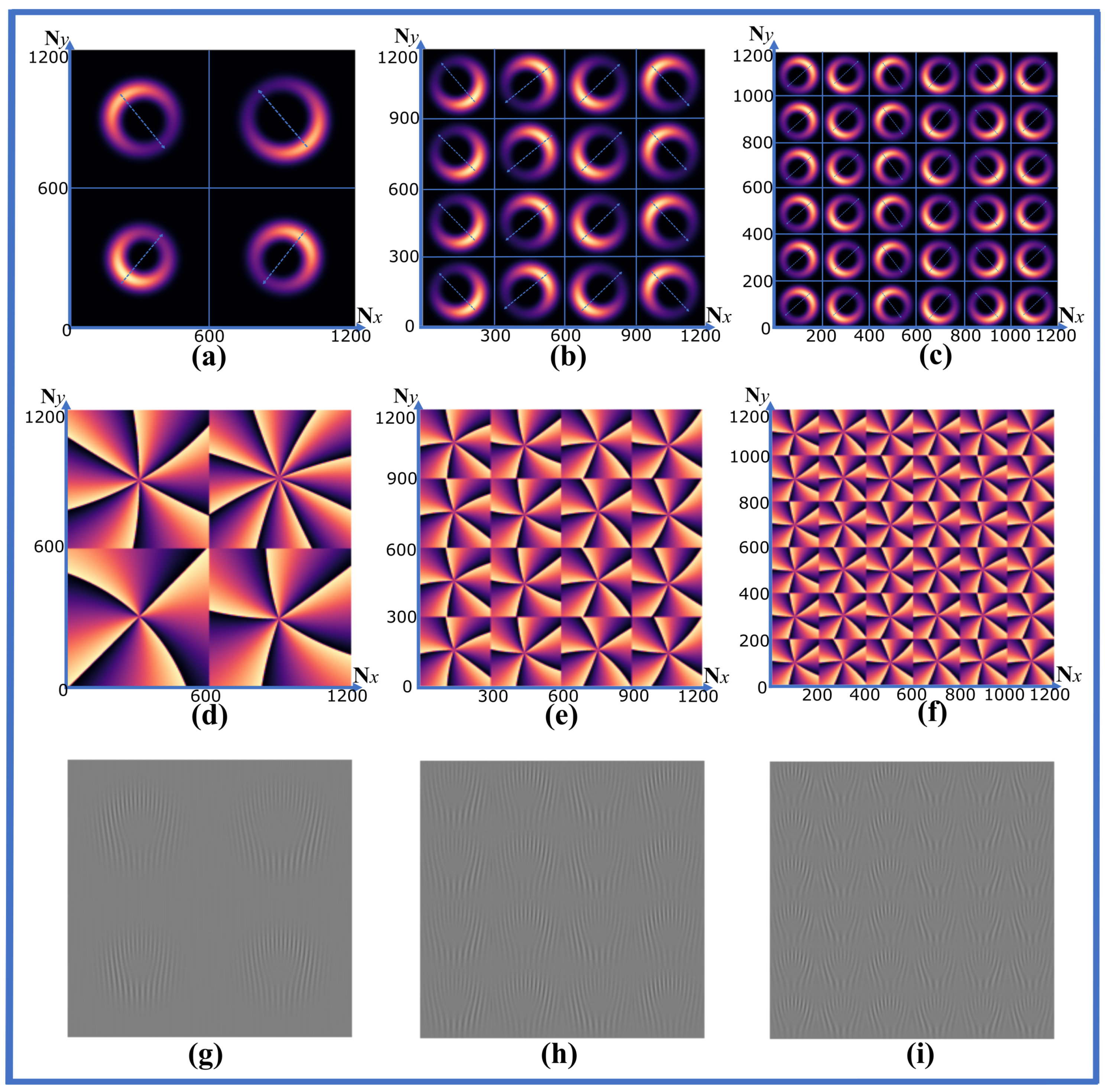
. Here each tile corresponds to one asymmetric LG beam in the array. The simulated intensity and phase profile of an array consisting of different asymmetric LG beams are shown in the first and second row of
Figure 3 respectively.
Figure 3a,d shows a
array in which the total area of the meshgrid is
and the area of each tile (i.e, individual asymmetric beam) is
. The total number of pixels associated with each individual beam in the array is
. Similarly,
Figure 3b,e shows a
array, and
Figure 3c,f shows a
array in which the area of the individual asymmetric beams are
and
respectively. The total number of pixels associated with the individual beam, in this case, is
for a
array and
for a
array. It is important to note that, while the array size (the number of beams in the array) is increased, the beam waist of each individual asymmetric beam decreases accordingly. In
Figure 3a,d the beam waist of the single beam is 1.3 mm which reduces to 1 mm in the case of
array as shown in
Figure 3b,e and 0.65 mm in case of
array as shown in
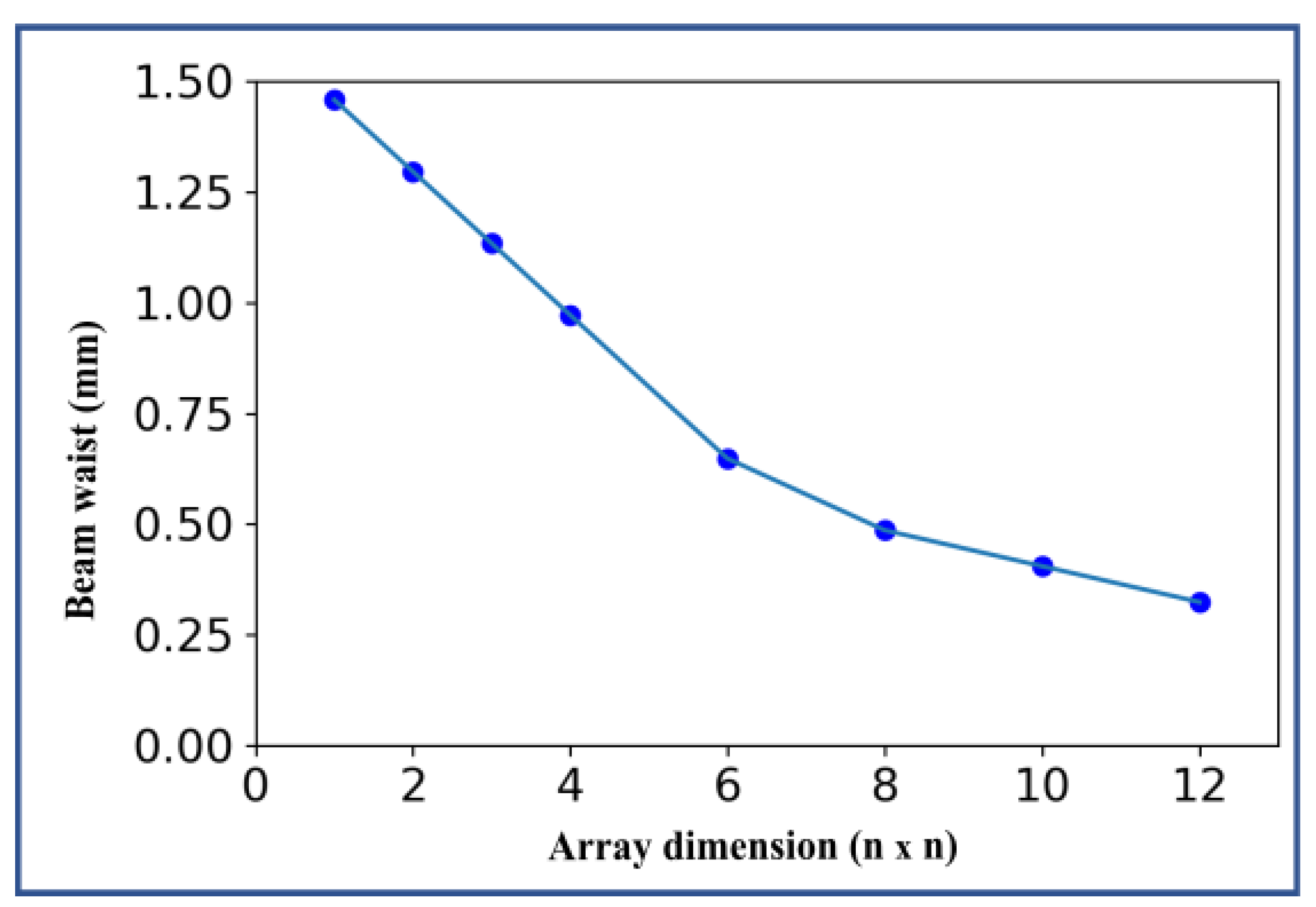
Figure 3c,f. The variation of the beam waist (
) of the individual beam of the array with the size of the array (n × n) is shown in
Figure 4.
Now we will discuss the actual CGH computation process. For simplicity, we concatenate all the tiles in the meshgrid and represent the resulting concatenated complex amplitude as
(Equation (
4))
where
A(x,y) and
corresponds to the amplitude and phase of the asymmetric array (consisting of multiple beams, spatially separated) which can be calculated using Equation (
3). The CGH, which is also the transmittance function of the SLM (
) is computed using the hologram encoding technique reported by Arrizon et al. [
36] and is described in Equation (
5),
where,
is the inverse of the first order Bessel function,
represents a linear phase grating in which
are reciprocal of the linear grating period,
is the normalized amplitude,
is phase profile of the array and
. The computed CGH pattern of
(
Figure 3g),
(
Figure 3h) and
(
Figure 3h) asymmetric LG beam array for
= 15 and
= 0 is shown in the third row of
Figure 3. These holograms also correspond to the amplitude and phase patterns shown in the above two rows of
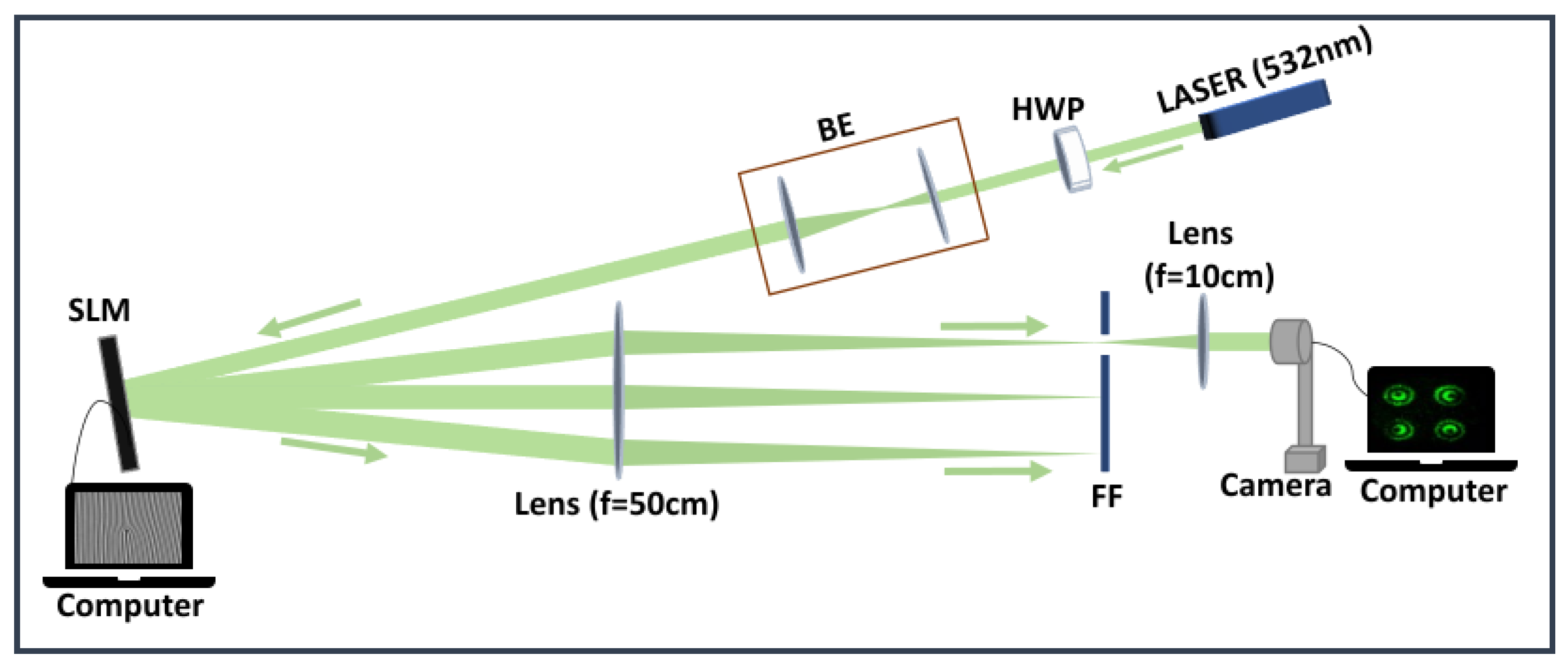
Figure 3, respectively. The experimental setup used for the practical generation of an asymmetric LG beam array is shown in
Figure 5. The SLM used in the experiment was the LC-R 1080 reflective LCOS SLM from HOLOEYE Photonics AG (HOLOEYE Photonics AG, Berlin, Germany), which has a resolution of 1920 × 1200, a pixel pitch of 8.1
m, and a frame rate of 60 Hz. A half-wave plate (HWP) was used to adjust the polarization of the input beam being fed into the SLM. A 4f optical relay setup was used to separate the +1-order beam from the other diffracted and non-diffracted orders. The two lenses that were used in the 4f setup were of focal lengths of 50 cm and 10 cm, respectively. Finally, the intensity profile of the reconstructed beam array was captured using a CMOS camera (HAYEAR, model- HY6100 with pixel Size 2.0 × 2.0
m and Resolution of 3840 × 2160) kept at a distance of 120 cm (4f) from the SLM. It should be noted that the beam intensities must be captured as close as possible to the 4f plane. Away from the 4f plane, the beams start to overlap each other and also become distorted with distance.
3. Results
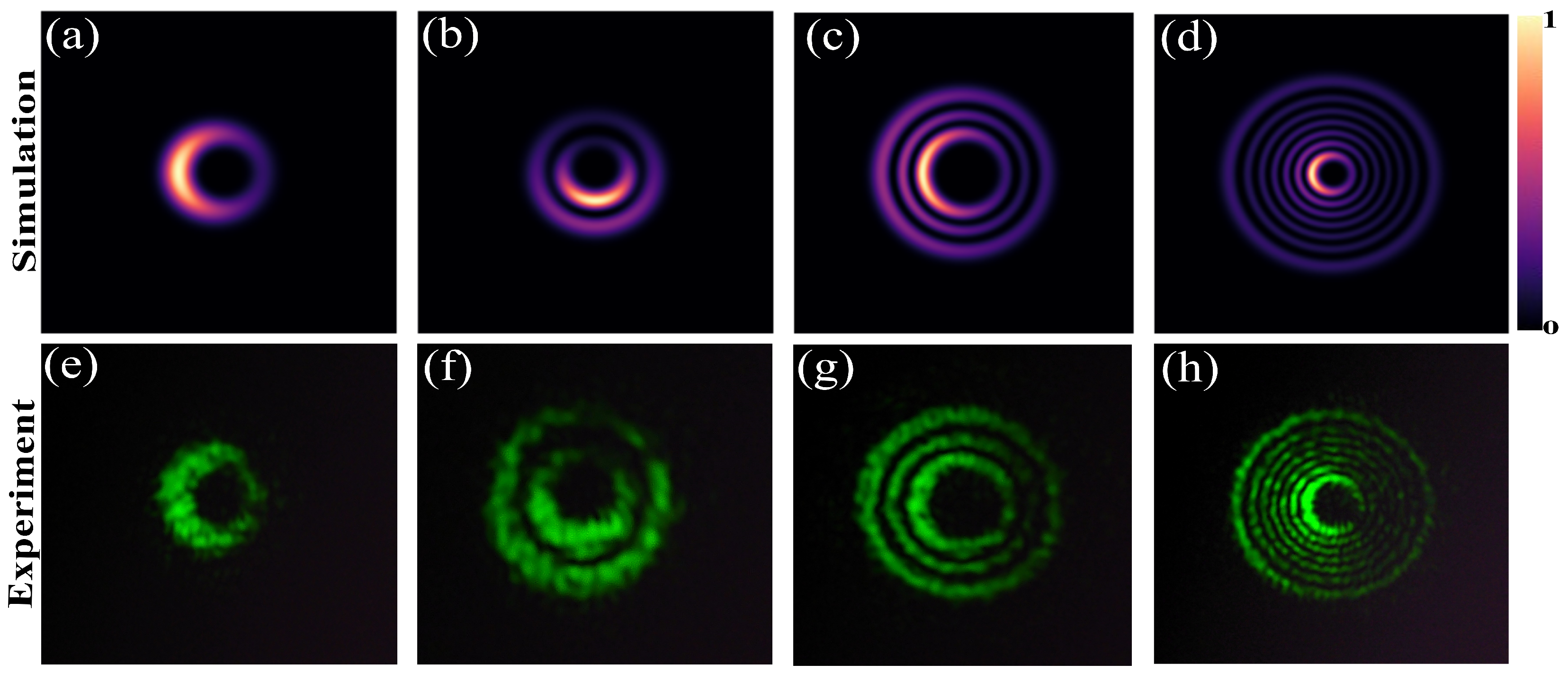
The captured intensity profile at the output plane for individual asymmetric LG beams (not array) for different set parameters is shown in
Figure 6. The first and second rows show simulated and experimental results, respectively, for fixed beam waist (
) and wavelength (
532 nm). The asymmetricity and mode indices are varied as follows, (a,e)
,
,
p= 0,
l= 5, (b,f)
,
,
p= 1,
l= 5, (c,g)
,
,
p= 2,
l= 10 and (d,h)
,
,
p= 6,
l= 5. The area of the meshgrid in this case is
. We can see that the experimentally-captured intensity profile agrees well with that of the simulated ones.
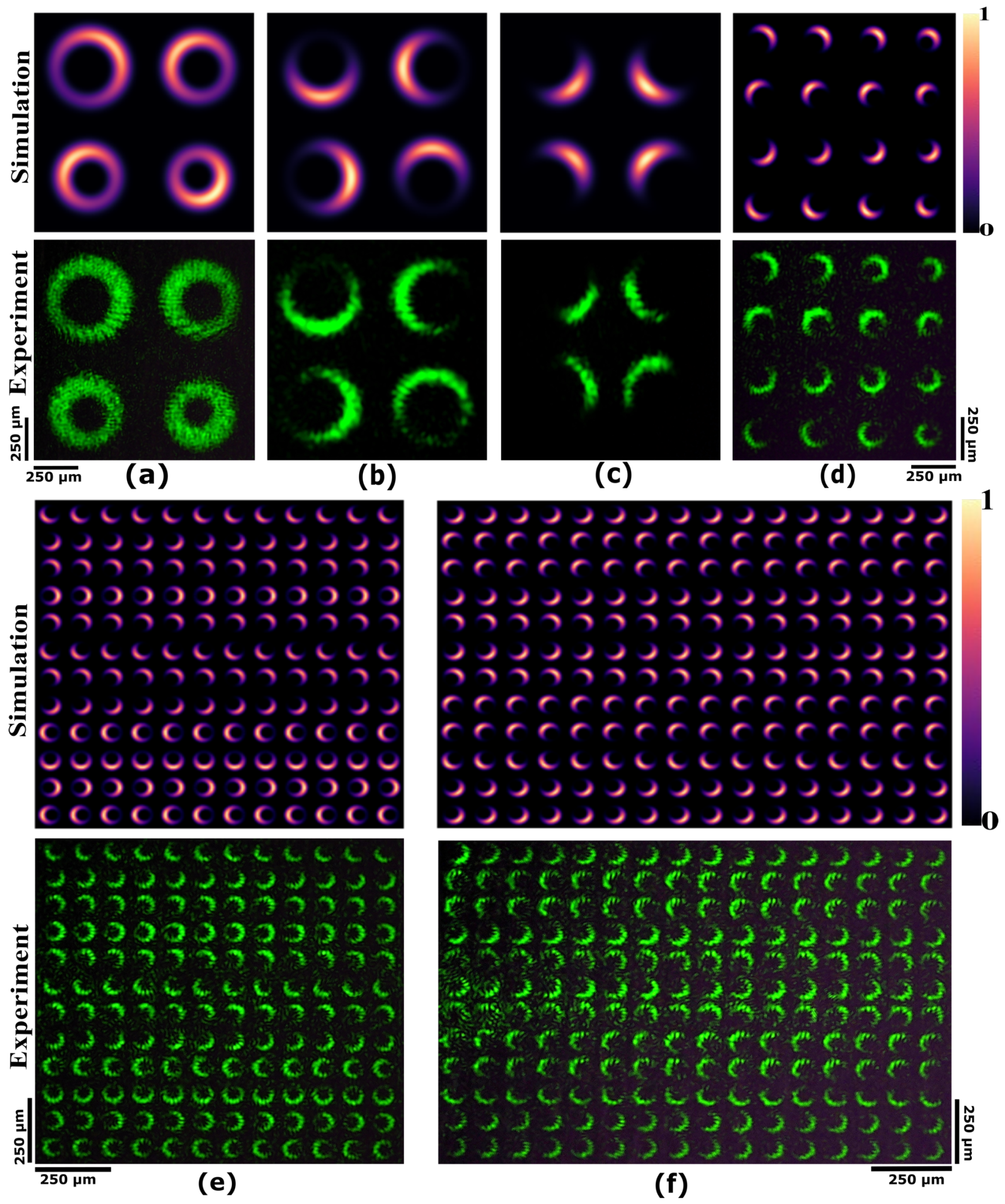
Now, we will discuss the characteristics of a practically generated asymmetric LG beam array. Here, the directional orientation of the crescent-shaped intensity profile varies with the sign of asymmetricity values (
and
) as defined in
Figure 2.
Figure 7 shows the simulated and corresponding experimentally-observed intensity profiles for six different asymmetric LG beam arrays. Each array has a collection of individual beams with different asymmetricities and beam waists, as shown in
Figure 7. In all the arrays, the radial index was kept fixed at
p = 0, while the azimuthal index and asymmetric parameters
and
were allowed to vary.
Figure 7a shows a
array where each beam in the array exhibits a different orientation, asymmetricity, and azimuthal mode. It can be observed that the individual beam size (intensity profile) increases with mode indices
l = 5, 6, 7 and 8. On the other hand, all the individual beams in the array shown in
Figure 7b,c have a fixed azimuthal index (
l = 5) but have different directional orientation w.r.t asymmetricity.
Figure 7d shows a
array, where the directional orientation of each beam varies from top to bottom and the beam modes vary from left to right with azimuthal index
l = 6, 5, 4 and 3, respectively. No two beams in this array have the same directional orientation and mode index. From
Figure 7b–d, we find that different information can be encoded in terms of directional orientation for a given mode index. In
Figure 7e,f, we try to create patterned intensity profiles that can encode information in the ‘spatial distribution of modes’, similar to a QR code but at a higher density.
Figure 7e shows a
array with the same azimuthal index
l = 5, but a distribution of asymmetric orientations. The orientations are chosen such that the array spans a unique pattern in the transverse plane. Here, multiple beams possess the same directional orientation and mode index but are spatially distributed in a unique fashion in accordance with some information (to be encoded). The maximum array size that has been generated with radial and azimuthal indices
p = 0 and
l = 5, respectively, is
, which is shown in
Figure 7f. Here, each beam has a beam waist of 0.2 mm and is enclosed in a tile of size
with
effective number of pixels (in each tile). The
array shown in
Figure 7e agrees well with the simulated intensity profile but the
array (
Figure 7f), shows some crosstalk. Any further increase in array size or mode number will exceed the space-bandwidth limit of the SLM resulting in increased crosstalk. From the above results, we can conclude that an asymmetric beam array can be used to encode information in the mode number and directional orientation of each individual beam itself (
Figure 7a–d), or be used to create a unique intensity pattern that encodes data in the unique spatial distribution of mode number and directional orientation (
Figure 7e,f).
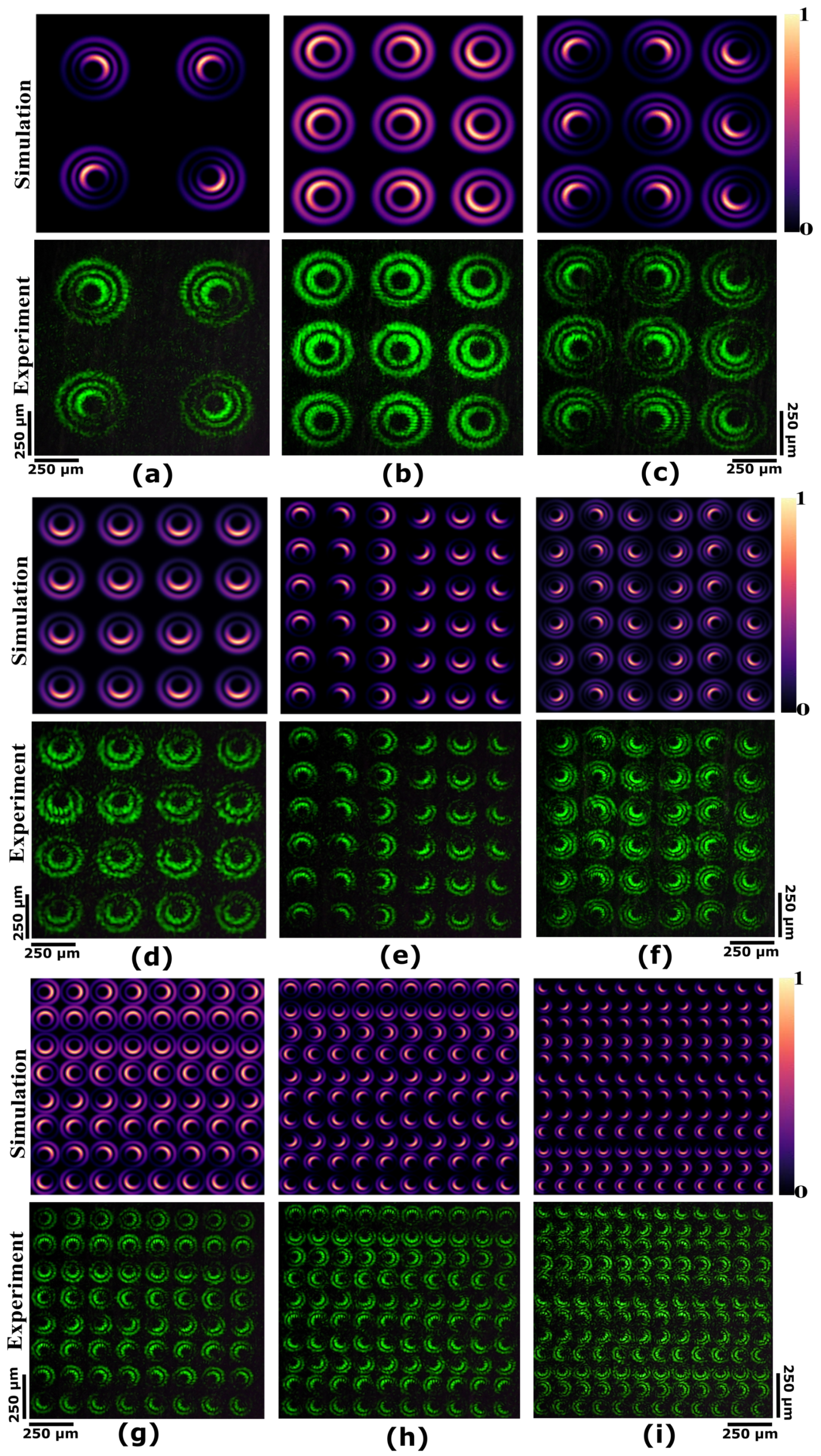
The encoded information can be further increased by setting a non-zero radial index to individual beams. The simulated and experimentally obtained intensity profile of asymmetric LG beam array with non-zero radial index (p) is shown in
Figure 8.
Figure 8a,c,f show asymmetric LG array of sizes of
,
, and
respectively for beam indices
p = 2 and
l = 5.
Figure 8b,d,e,g–i shows an asymmetric LG array for varying array sizes up to
for beam indices
p = 1 and
l = 5. Here again, the direction of the opening in the crescent is determined according to the sign of asymmetric parameters
and
described in
Figure 2. The experimentally obtained intensity profile of the array is well-matched with the simulated intensity profile, confirming the successful generation of an asymmetric LG beam array with non-zero radial(p) and azimuthal(l) indices. Here again, we can see that the information can be encoded either in mode number and directional orientation of individual beams or be used to create unique intensity patterns that encode data in the spatial distribution. We also would like to note that the increase in the azimuthal or radial index will result in the reduction of the total number of beams that can be accommodated in an array for a given hologram size. This is because the larger mode indices consume more spatial bandwidth of the hologram and also the beam diameter (transverse intensity distribution) increases with mode indices, occupying a larger area. The experiments reported here used a green laser (532 nm), but any other wavelength can be used as well, provided the desired wavelength is used in the hologram computation and the SLM is calibrated for that particular wavelength. If a DMD is used, then wavelength calibration will not be necessary [
37].